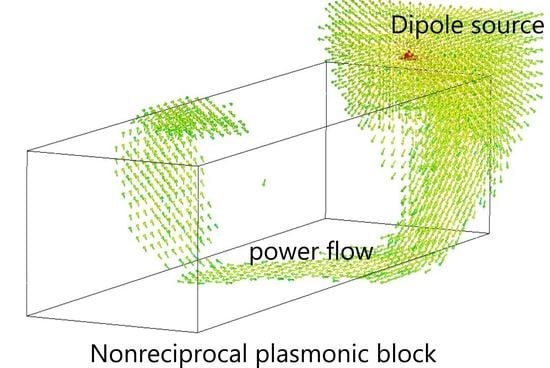
Nonreciprocal and Topological Plasmonics
Abstract
1. Introduction
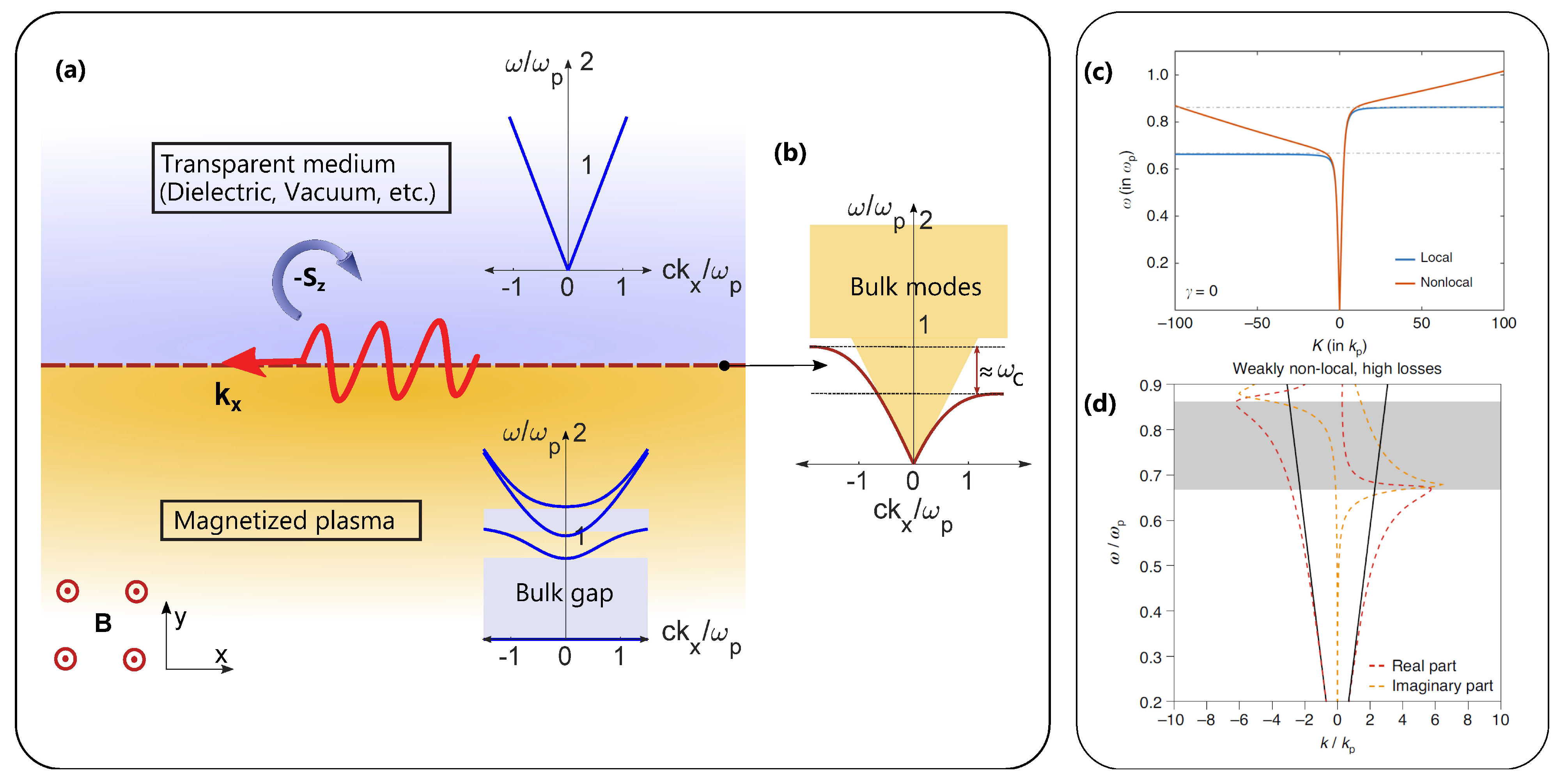
2. Non-Reciprocal Modes of Magnetized Plasmonic Media
2.1. Bulk Properties–Constraints on Permittivity Tensor, Dispersion Diagram, Topological Properties
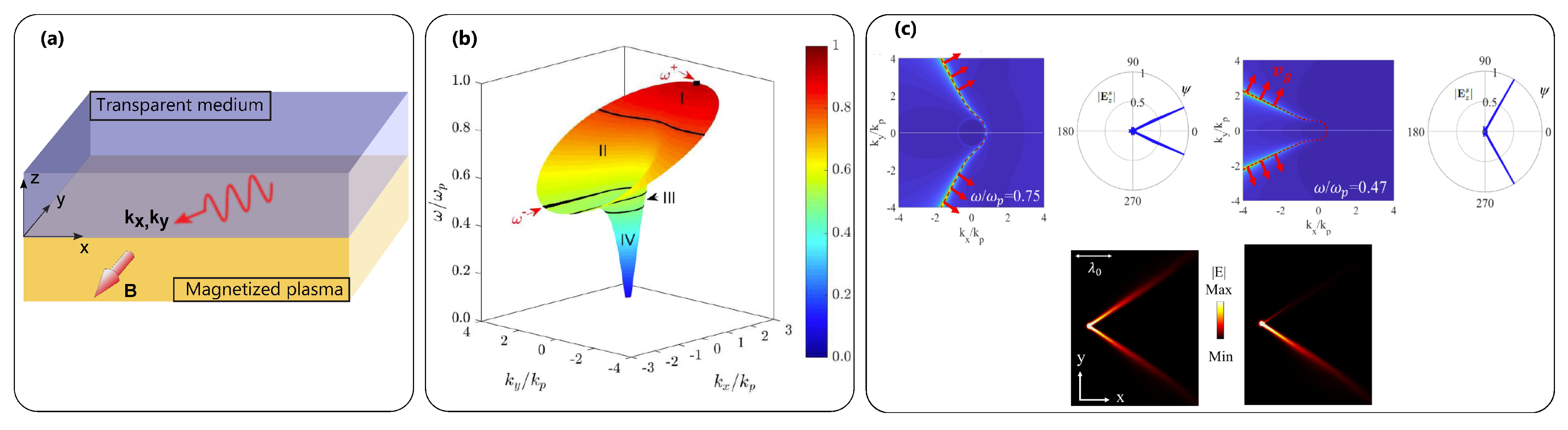
2.2. Surface States—Transparent, Opaque and Other Interfaces
2.3. Edge States on Two-Dimensional Biased Plasmas
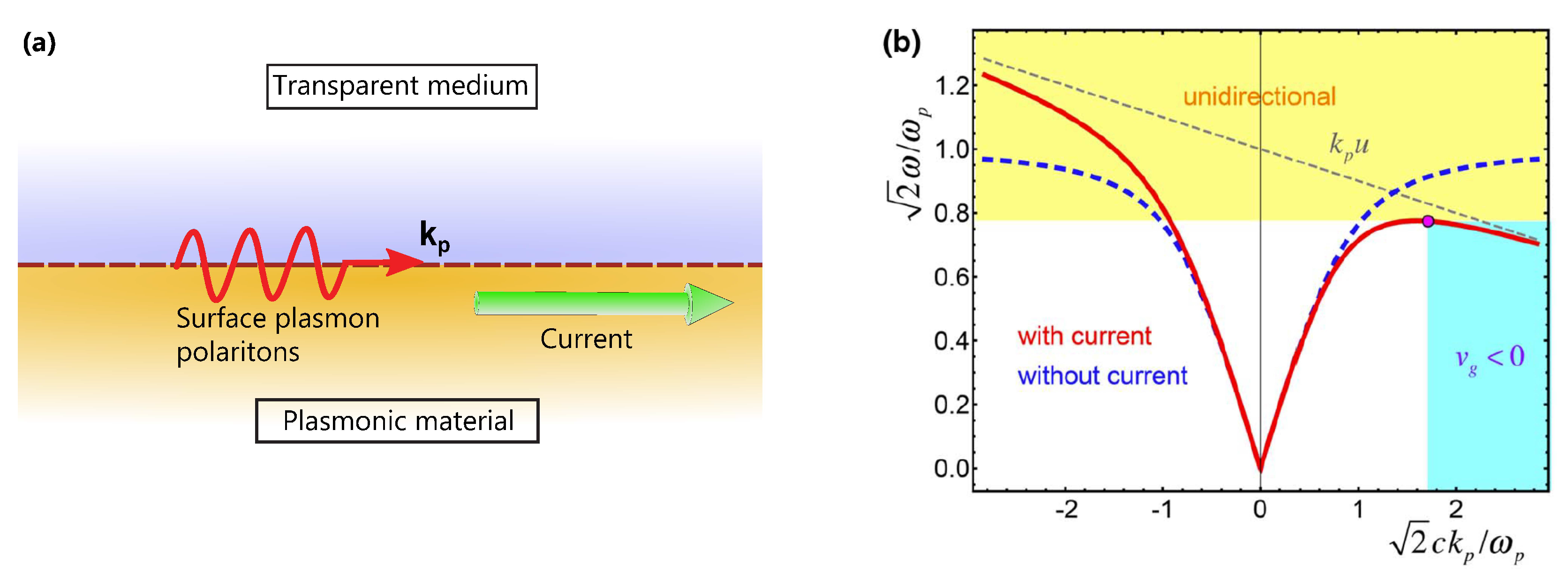
3. Current-Induced Unidirectional Surface Waves on Plasmonic Media
4. Topologically Protected Surface Waves on Reciprocal Plasmonic Media
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Trivelpiece, A.W.; Gould, R.W. Space Charge Waves in Cylindrical Plasma Columns. J. Appl. Phys. 1959, 30, 1784–1793. [Google Scholar] [CrossRef]
- Adachi, S.; Mushiake, Y. Surface waves along a perfectly conducting plane covered with semi-infinite magneto-plasma. J. Res. Natl. Bur. Stand. Sect. Radio Sci. 1965, 69, 171. [Google Scholar] [CrossRef]
- Seshadri, S.R.; Pickard, W.F. Surface Waves on an Anisotropic Plasma Sheath. IEEE Trans. Microw. Theory Tech. 1964, 12, 529–541. [Google Scholar] [CrossRef]
- Aers, G.C.; Boardman, A.D. The theory of semiconductor magnetoplasmon-polariton surface modes: Voigt geometry. J. Phys. C Solid State Phys. 1978, 11, 945–959. [Google Scholar] [CrossRef]
- Brion, J.J.; Wallis, R.F.; Hartstein, A.; Burstein, E. Theory of Surface Magnetoplasmons in Semiconductors. Phys. Rev. Lett. 1972, 28, 1455–1458. [Google Scholar] [CrossRef]
- Camley, R.E. Nonreciprocal surface waves. Surf. Sci. Rep. 1987, 7, 103–187. [Google Scholar] [CrossRef]
- Chin, J.Y.; Steinle, T.; Wehlus, T.; Dregely, D.; Weiss, T.; Belotelov, V.I.; Stritzker, B.; Giessen, H. Nonreciprocal plasmonics enables giant enhancement of thin-film Faraday rotation. Nat. Commun. 2013, 4, 1599. [Google Scholar] [CrossRef]
- Davoyan, A.R.; Engheta, N. Nonreciprocal Rotating Power Flow within Plasmonic Nanostructures. Phys. Rev. Lett. 2013, 111, 047401. [Google Scholar] [CrossRef] [PubMed]
- Hu, B.; Wang, Q.J.; Zhang, Y. Broadly tunable one-way terahertz plasmonic waveguide based on nonreciprocal surface magneto plasmons. Opt. Lett. 2012, 37, 1895–1897. [Google Scholar] [CrossRef] [PubMed]
- You, Y.; Xiao, S.; Wu, C.; Zhang, H.; Deng, X.; Shen, L. Unidirectional-propagating surface magnetoplasmon based on remanence and its application for subwavelength isolators. Opt. Mater. Express 2019, 9, 2415–2425. [Google Scholar] [CrossRef]
- Chettiar, U.K.; Davoyan, A.R.; Engheta, N. Hotspots from nonreciprocal surface waves. Opt. Lett. 2014, 39, 1760–1763. [Google Scholar] [CrossRef]
- Gangaraj, S.A.H.; Jin, B.; Argyropoulos, C.; Monticone, F. Broadband Field Enhancement and Giant Nonlinear Effects in Terminated Unidirectional Plasmonic Waveguides. Phys. Rev. Appl. 2020, 14, 054061. [Google Scholar] [CrossRef]
- Abdelrahman, M.I.; Monticone, F. Broadband and giant nonreciprocity at the subwavelength scale in magnetoplasmonic materials. Phys. Rev. B 2020, 102, 155420. [Google Scholar] [CrossRef]
- Mann, S.A.; Mekawy, A.; Alù, A. Broadband Field Localization, Density of States, and Nonlinearity Enhancement in Nonreciprocal and Topological Hotspots. Phys. Rev. Appl. 2021, 15, 034064. [Google Scholar] [CrossRef]
- Caloz, C.; Alù, A.; Tretyakov, S.; Sounas, D.; Achouri, K.; Deck-Léger, Z.-L. Electromagnetic Nonreciprocity. Phys. Rev. Appl. 2018, 10, 047001. [Google Scholar] [CrossRef]
- Remer, L.; Mohler, E.; Grill, W.; Lüthi, B. Nonreciprocity in the optical reflection of magnetoplasmas. Phys. Rev. B 1984, 30, 3277–3282. [Google Scholar] [CrossRef]
- Potton, R.J. Reciprocity in optics. Rep. Prog. Phys. 2004, 67, 717–754. [Google Scholar] [CrossRef]
- Parker, J.B.; Marston, J.B.; Tobias, S.M.; Zhu, Z. Topological Gaseous Plasmon Polariton in Realistic Plasma. Phys. Rev. Lett. 2020, 124, 195001. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Bulk-edge correspondence for topological photonic continua. Phys. Rev. B 2016, 94, 205105. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Proof of the Bulk-Edge Correspondence through a Link between Topological Photonics and Fluctuation-Electrodynamics. Phys. Rev. X 2019, 9, 011037. [Google Scholar] [CrossRef]
- Jin, D.; Lu, L.; Wang, Z.; Fang, C.; Joannopoulos, J.D.; Soljačić, M.; Fu, L.; Fang, N.X. Topological magnetoplasmon. Nat. Commun. 2016, 7, 13486. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Smirnova, D.; Nori, F. Quantum spin Hall effect of light. Science 2015, 348, 1448–1451. [Google Scholar] [CrossRef] [PubMed]
- Bliokh, K.Y.; Nori, F. Transverse spin of a surface polariton. Phys. Rev. A 2012, 85, 061801. [Google Scholar] [CrossRef]
- Van Mechelen, T.; Jacob, Z. Universal spin-momentum locking of evanescent waves. Optica 2016, 3, 118–126. [Google Scholar] [CrossRef]
- Ishimaru, A. Dispersion and Anisotropic Media. In Electromagnetic Wave Propagation, Radiation, and Scattering; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2017; pp. 233–283. [Google Scholar]
- McIsaac, P.R. A General Reciprocity Theorem. IEEE Trans. Microw. Theory Tech. 1979, 27, 340–342. [Google Scholar] [CrossRef]
- Lindell, I.V.; Dahl, F.M. Conditions for the parameter dyadics of lossy bianisotropic media. Microw. Opt. Technol. Lett. 2001, 29, 175–178. [Google Scholar] [CrossRef]
- Lindell, I.V.; Sihvola, A.H.; Puska, P.; Ruotanen, L.H. Conditions for the parameter dyadics of lossless bianisotropic media. Microw. Opt. Technol. Lett. 1995, 8, 268–272. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Chapter IX—The electromagnetic wave equations. In Electrodynamics of Continuous Media, 2nd ed.; Pergamon: Amsterdam, The Netherlands, 1984; Volume 8, pp. 257–289. [Google Scholar]
- Zhao, B.; Guo, C.; Garcia, C.A.C.; Narang, P.; Fan, S. Axion-Field-Enabled Nonreciprocal Thermal Radiation in Weyl Semimetals. Nano Lett. 2020, 20, 1923–1927. [Google Scholar] [CrossRef]
- Sommerfeld, A. Lectures on Theoretical Physics; Academic Press: Cambridge, MA, USA, 1950; Available online: http://books.google.com/books?id=VgFRAAAAMAAJ (accessed on 9 April 2021).
- Arikawa, T.; Wang, X.; Belyanin, A.A.; Kono, J. Giant tunable Faraday effect in a semiconductor magneto-plasma for broadband terahertz polarization optics. Opt. Express 2012, 20, 19484–19492. [Google Scholar] [CrossRef]
- Wang, X.; Belyanin, A.A.; Crooker, S.A.; Mittleman, D.M.; Kono, J. Interference-induced terahertz transparency in a semiconductor magneto-plasma. Nat. Phys. 2010, 6, 126–130. [Google Scholar] [CrossRef]
- Gao, W.; Yang, B.; Lawrence, M.; Fang, F.; Béri, B.; Zhang, S. Photonic Weyl degeneracies in magnetized plasma. Nat. Commun. 2016, 7, 12435. [Google Scholar] [CrossRef]
- Shastri, K.; Monticone, F. Dissipation-induced topological transitions in continuous Weyl materials. Phys. Rev. Res. 2020, 2, 033065. [Google Scholar] [CrossRef]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological insulators. Rev. Mod. Phys. 2010, 82, 3045–3067. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Topological classification of Chern-type insulators by means of the photonic Green function. Phys. Rev. B 2018, 97, 115146. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer US: New York, NY, USA, 2007. [Google Scholar]
- Yu, H.; Peng, Y.; Yang, Y.; Li, Z.-Y. Plasmon-enhanced light–matter interactions and applications. NPJ Comput. Mater. 2019, 5, 45. [Google Scholar] [CrossRef]
- West, P.R.; Ishii, S.; Naik, G.V.; Emani, N.K.; Shalaev, V.M.; Boltasseva, A. Searching for better plasmonic materials. Laser Photonics Rev. 2010, 4, 795–808. [Google Scholar] [CrossRef]
- Naik, G.V.; Shalaev, V.M.; Boltasseva, A. Alternative Plasmonic Materials: Beyond Gold and Silver. Adv. Mater. 2013, 25, 3264–3294. [Google Scholar] [CrossRef] [PubMed]
- Chochol, J.; Postava, K.; Čada, M.; Pištora, J. Experimental demonstration of magnetoplasmon polariton at InSb(InAs)/dielectric interface for terahertz sensor application. Sci. Rep. 2017, 7, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Boltasseva, A.; Atwater, H.A. Low-Loss Plasmonic Metamaterials. Science 2011, 331, 290–291. [Google Scholar] [CrossRef]
- Simovski, C.R.; Belov, P.A.; Atrashchenko, A.V.; Kivshar, Y.S. Wire Metamaterials: Physics and Applications. Adv. Mater. 2012, 24, 4229–4248. [Google Scholar] [CrossRef]
- Silveirinha, M.G. Chern invariants for continuous media. Phys. Rev. B 2015, 92, 125153. [Google Scholar] [CrossRef]
- Buddhiraju, S.; Shi, Y.; Song, A.; Wojcik, C.; Minkov, M.; Williamson, I.A.D.; Dutt, A.; Fan, S. Absence of unidirectionally propagating surface plasmon-polaritons at nonreciprocal metal-dielectric interfaces. Nat. Commun. 2020, 11, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Souslov, A.; Dasbiswas, K.; Fruchart, M.; Vaikuntanathan, S.; Vitelli, V. Topological Waves in Fluids with Odd Viscosity. Phys. Rev. Lett. 2019, 122, 128001. [Google Scholar] [CrossRef]
- Gangaraj, S.A.H.; Monticone, F. Physical Violations of the Bulk-Edge Correspondence in Topological Electromagnetics. Phys. Rev. Lett. 2020, 124, 153901. [Google Scholar] [CrossRef] [PubMed]
- Kaliteevski, M.; Iorsh, I.; Brand, S.; Abram, R.A.; Chamberlain, J.M.; Kavokin, A.V.; Shelykh, I.A. Tamm plasmon-polaritons: Possible electromagnetic states at the interface of a metal and a dielectric Bragg mirror. Phys. Rev. B 2007, 76, 165415. [Google Scholar] [CrossRef]
- Goto, T.; Dorofeenko, A.V.; Merzlikin, A.M.; Baryshev, A.V.; Vinogradov, A.P.; Inoue, M.; Lisyansky, A.A.; Granovsky, A.B. Optical Tamm States in One-Dimensional Magnetophotonic Structures. Phys. Rev. Lett. 2008, 101, 113902. [Google Scholar] [CrossRef] [PubMed]
- Bikbaev, R.G.; Vetrov, S.Y.; Timofeev, I.V. Hybrid Tamm and surface plasmon polaritons in resonant photonic structure. J. Quant. Spectrosc. Radiat. Transf. 2020, 253, 107156. [Google Scholar] [CrossRef]
- Gangaraj, S.A.H.; Monticone, F. Do truly unidirectional surface plasmon-polaritons exist? Optica 2019, 6, 1158–1165. [Google Scholar] [CrossRef]
- Mortensen, N.A.; Raza, S.; Wubs, M.; Søndergaard, T.; Bozhevolnyi, S.I. A generalized non-local optical response theory for plasmonic nanostructures. Nat. Commun. 2014, 5, 1–7. [Google Scholar] [CrossRef]
- Raza, S.; Bozhevolnyi, S.I.; Wubs, M.; Mortensen, N.A. Nonlocal optical response in metallic nanostructures. J. Phys. Condens. Matter 2015, 27, 183204. [Google Scholar] [CrossRef]
- Fernández-Domínguez, A.I.; Wiener, A.; García-Vidal, F.J.; Maier, S.A.; Pendry, J.B. Transformation-Optics Description of Nonlocal Effects in Plasmonic Nanostructures. Phys. Rev. Lett. 2012, 108, 106802. [Google Scholar] [CrossRef]
- Maack, J.R.; Mortensen, N.A.; Wubs, M. Size-dependent nonlocal effects in plasmonic semiconductor particles. EPL Europhys. Lett. 2017, 119, 17003. [Google Scholar] [CrossRef]
- Monticone, F. A truly one-way lane for surface plasmon polaritons. Nat. Photonics 2020, 14, 461–465. [Google Scholar] [CrossRef]
- Pakniyat, S.; Holmes, A.M.; Hanson, G.W.; Gangaraj, S.A.H.; Antezza, M.; Silveirinha, M.G.; Jam, S.; Monticone, F. Non-Reciprocal, robust surface plasmon polaritons on gyrotropic interfaces. IEEE Trans. Antennas Propag. 2020, 68, 3718–3729. [Google Scholar] [CrossRef]
- Gangaraj, S.A.H.; Hanson, G.W.; Silveirinha, M.G.; Shastri, K.; Antezza, M.; Monticone, F. Unidirectional and diffractionless surface plasmon polaritons on three-dimensional nonreciprocal plasmonic platforms. Phys. Rev. B 2019, 99, 245414. [Google Scholar] [CrossRef]
- Wang, D.; Yang, B.; Gao, W.; Jia, H.; Yang, Q.; Chen, X.; Wei, M.; Liu, C.; Navarro-Cía, M.; Han, J.; et al. Photonic Weyl points due to broken time-reversal symmetry in magnetized semiconductor. Nat. Phys. 2019, 15, 1150–1155. [Google Scholar] [CrossRef]
- Armitage, N.P.; Mele, E.J.; Vishwanath, A. Weyl and Dirac semimetals in three-dimensional solids. Rev. Mod. Phys. 2018, 90, 015001. [Google Scholar] [CrossRef]
- Shastri, K.; Monticone, F. Robust surface-wave propagation and leaky-wave radiation based on three-dimensional topological plasmonic materials. In Proceedings of the Active Photonic Platforms XI, San Diego, CA, USA, 11–15 August 2019; Volume 11081, p. 1108107. [Google Scholar]
- Ding, F.; Bozhevolnyi, S.I. A Review of Unidirectional Surface Plasmon Polariton Metacouplers. IEEE J. Sel. Top. Quantum Electron. 2019, 25, 1–11. [Google Scholar] [CrossRef]
- Yu, Z.; Veronis, G.; Wang, Z.; Fan, S. One-Way Electromagnetic Waveguide Formed at the Interface between a Plasmonic Metal under a Static Magnetic Field and a Photonic Crystal. Phys. Rev. Lett. 2008, 100, 023902. [Google Scholar] [CrossRef]
- Gangaraj, S.A.H.; Silveirinha, M.G.; Hanson, G.W. Berry Phase, Berry Connection, and Chern Number for a Continuum Bianisotropic Material From a Classical Electromagnetics Perspective. IEEE J. Multiscale Multiphysics Comput. Tech. 2017, 2, 3–17. [Google Scholar] [CrossRef]
- Gangaraj, S.A.H.; Nemilentsau, A.; Hanson, G.W. The effects of three-dimensional defects on one-way surface plasmon propagation for photonic topological insulators comprised of continuum media. Sci. Rep. 2016, 6, 30055. [Google Scholar] [CrossRef]
- Hayran, Z.; Gangaraj, S.A.H.; Monticone, F. Topologically protected broadband rerouting of propagating waves around complex objects. Nanophotonics 2019, 8, 1371–1378. [Google Scholar] [CrossRef]
- Pakniyat, S.; Liang, Y.; Xiang, Y.; Cen, C.; Chen, J.; Hanson, G.W. Indium antimonide—Constraints on practicality as a magneto-optical platform for topological surface plasmon polaritons. J. Appl. Phys. 2020, 128, 183101. [Google Scholar] [CrossRef]
- Xia, L.; Gao, W.; Yang, B.; Guo, Q.; Liu, H.; Han, J.; Zhang, W.; Zhang, S. Stretchable Photonic ‘Fermi Arcs’ in Twisted Magnetized Plasma. Laser Photonics Rev. 2018, 12, 1700226. [Google Scholar] [CrossRef]
- Tauber, C.; Delplace, P.; Venaille, A. Anomalous bulk-edge correspondence in continuous media. Phys. Rev. Res. 2020, 2. [Google Scholar] [CrossRef]
- Van Mechelen, T.; Jacob, Z. Quantum gyroelectric effect: Photon spin-1 quantization in continuum topological bosonic phases. Phys. Rev. A 2018, 98, 023842. [Google Scholar] [CrossRef]
- Van Mechelen, T.; Jacob, Z. Unidirectional Maxwellian spin waves. Nanophotonics 2019, 8, 1399–1416. [Google Scholar] [CrossRef]
- Stern, F. Polarizability of a Two-Dimensional Electron Gas. Phys. Rev. Lett. 1967, 18, 546–548. [Google Scholar] [CrossRef]
- Jablan, M.; Buljan, H.; Soljačić, M. Plasmonics in graphene at infrared frequencies. Phys. Rev. B 2009, 80, 245435. [Google Scholar] [CrossRef]
- Chiu, K.W.; Quinn, J.J. Plasma oscillations of a two-dimensional electron gas in a strong magnetic field. Phys. Rev. B 1974, 9, 4724–4732. [Google Scholar] [CrossRef]
- Mast, D.B.; Dahm, A.J.; Fetter, A.L. Observation of Bulk and Edge Magnetoplasmons in a Two-Dimensional Electron Fluid. Phys. Rev. Lett. 1985, 54, 1706–1709. [Google Scholar] [CrossRef] [PubMed]
- Glattli, D.C.; Andrei, E.Y.; Deville, G.; Poitrenaud, J.; Williams, F.I.B. Dynamical Hall Effect in a Two-Dimensional Classical Plasma. Phys. Rev. Lett. 1985, 54, 1710–1713. [Google Scholar] [CrossRef] [PubMed]
- Jin, D.; Xia, Y.; Christensen, T.; Freeman, M.; Wang, S.; Fong, K.Y.; Gardner, G.C.; Fallahi, S.; Hu, Q.; Wang, Y.; et al. Topological kink plasmons on magnetic-domain boundaries. Nat. Commun. 2019, 10, 1–9. [Google Scholar] [CrossRef]
- Sounas, D.L.; Alù, A. Non-reciprocal photonics based on time modulation. Nat. Photonics 2017, 11, 774–783. [Google Scholar] [CrossRef]
- Morgado, T.A.; Silveirinha, M.G. Drift-Induced Unidirectional Graphene Plasmons. ACS Photonics 2018, 5, 4253–4258. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Rodríguez-Fortuño, F.J.; Bekshaev, A.Y.; Kivshar, Y.S.; Nori, F. Electric-current-induced unidirectional propagation of surface plasmon-polaritons. Opt. Lett. 2018, 43, 963–966. [Google Scholar] [CrossRef]
- Correas-Serrano, D.; Gomez-Diaz, J.S. Nonreciprocal and collimated surface plasmons in drift-biased graphene metasurfaces. Phys. Rev. B 2019, 100, 081410. [Google Scholar] [CrossRef]
- Xiao, M.; Lin, Q.; Fan, S. Hyperbolic Weyl Point in Reciprocal Chiral Metamaterials. Phys. Rev. Lett. 2016, 117, 057401. [Google Scholar] [CrossRef]
- Gao, W.; Lawrence, M.; Yang, B.; Liu, F.; Fang, F.; Béri, B.; Li, J.; Zhang, S. Topological Photonic Phase in Chiral Hyperbolic Metamaterials. Phys. Rev. Lett. 2015, 114, 037402. [Google Scholar] [CrossRef] [PubMed]
- Yang, B.; Guo, Q.; Tremain, B.; Barr, L.E.; Gao, W.; Liu, H.; Béri, B.; Xiang, Y.; Fan, D.; Hibbins, A.P.; et al. Direct observation of topological surface-state arcs in photonic metamaterials. Nat. Commun. 2017, 8, 1–7. [Google Scholar] [CrossRef]
- Khanikaev, A.B.; Mousavi, S.H.; Tse, W.-K.; Kargarian, M.; MacDonald, A.H.; Shvets, G. Photonic topological insulators. Nat. Mater. 2012, 12, 233–239. [Google Scholar] [CrossRef] [PubMed]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shastri, K.; Abdelrahman, M.I.; Monticone, F. Nonreciprocal and Topological Plasmonics. Photonics 2021, 8, 133. https://doi.org/10.3390/photonics8040133
Shastri K, Abdelrahman MI, Monticone F. Nonreciprocal and Topological Plasmonics. Photonics. 2021; 8(4):133. https://doi.org/10.3390/photonics8040133
Chicago/Turabian StyleShastri, Kunal, Mohamed Ismail Abdelrahman, and Francesco Monticone. 2021. "Nonreciprocal and Topological Plasmonics" Photonics 8, no. 4: 133. https://doi.org/10.3390/photonics8040133
APA StyleShastri, K., Abdelrahman, M. I., & Monticone, F. (2021). Nonreciprocal and Topological Plasmonics. Photonics, 8(4), 133. https://doi.org/10.3390/photonics8040133