Numerical Simulation of Thermo-Optic Effects in an Nd: Glass Slab with Low Thermally Induced Wavefront Distortion
Abstract
1. Introduction
2. Theoretical Simulation
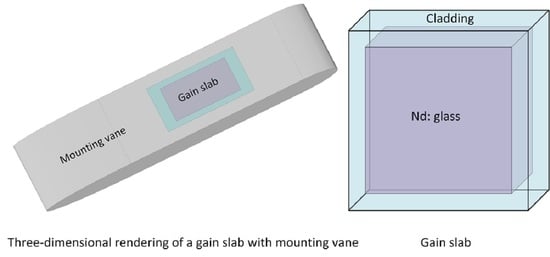
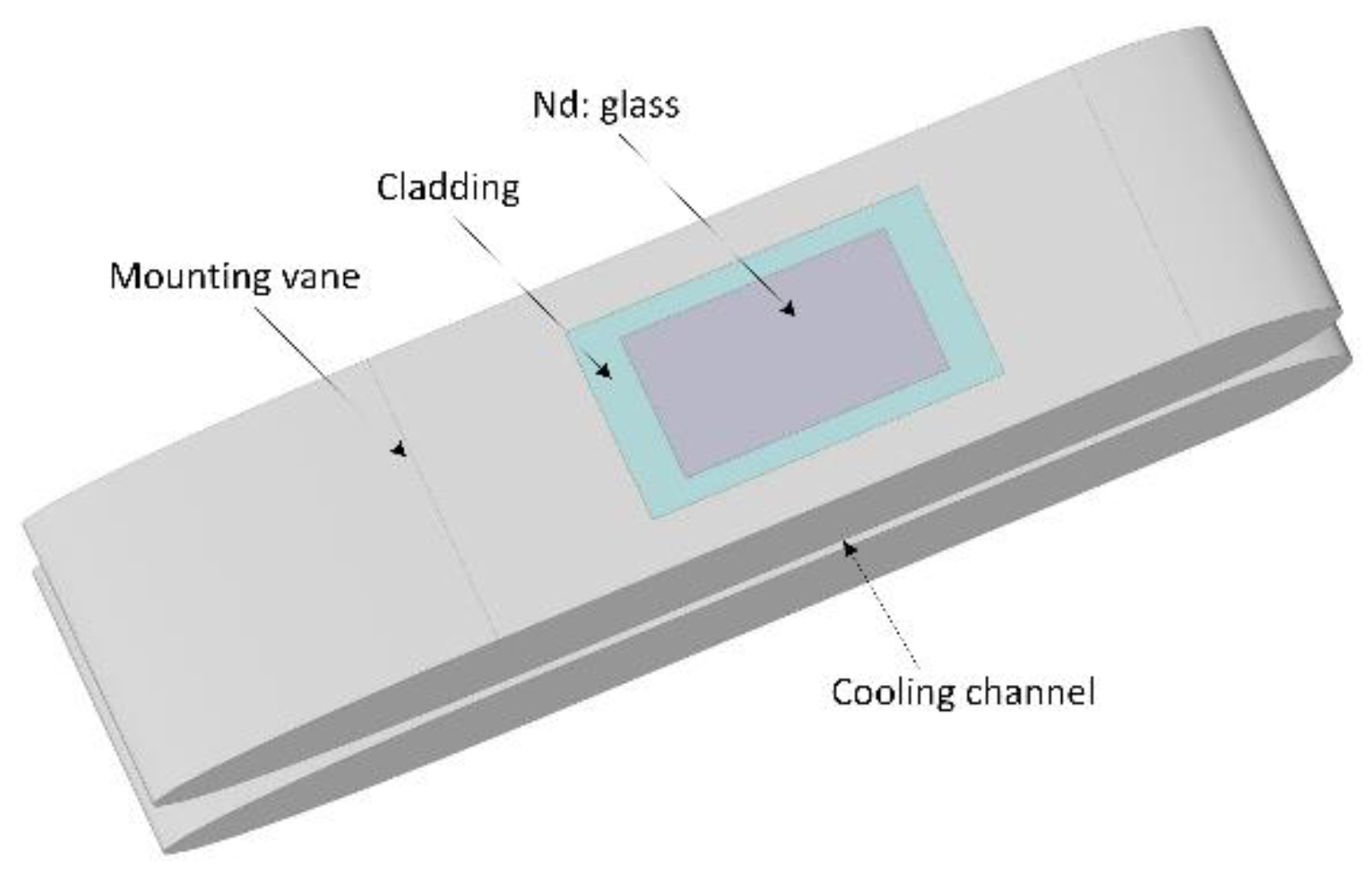
3. Geometry of the Laser Amplifier
4. Principle of Reduction of the Thermo-Optic Effects
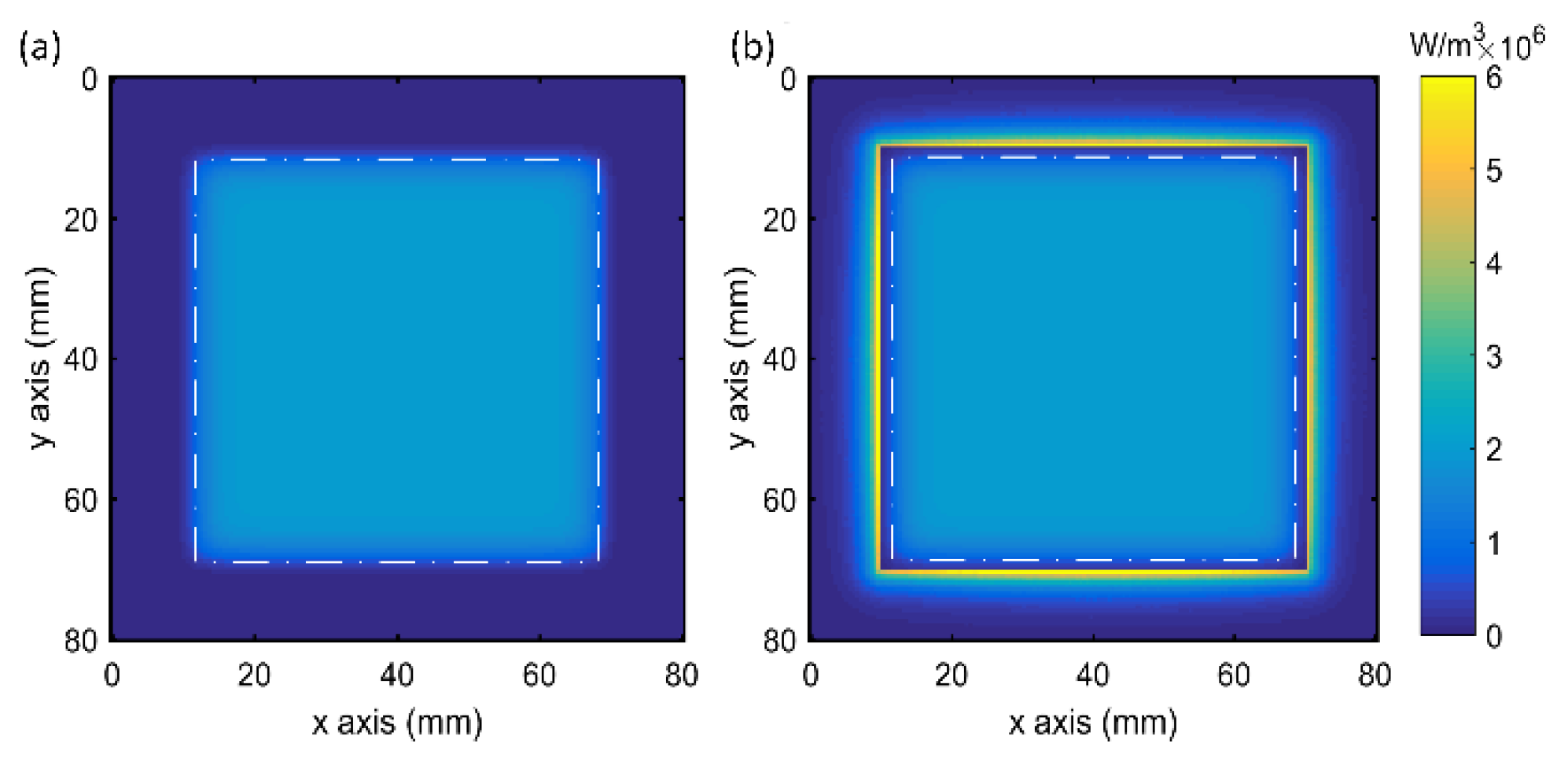
4.1. Heat Density Distribution
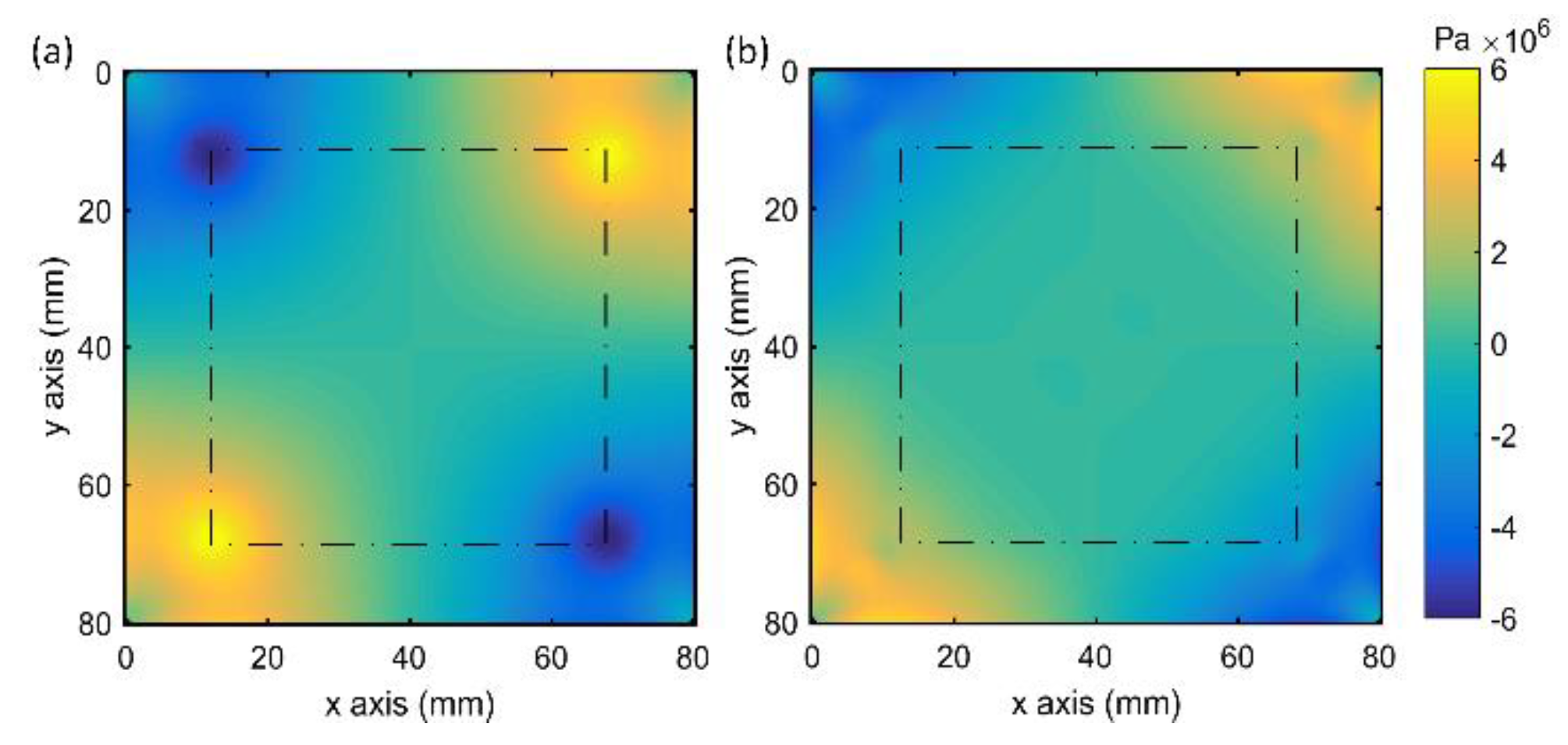
4.2. Temperature and Stress
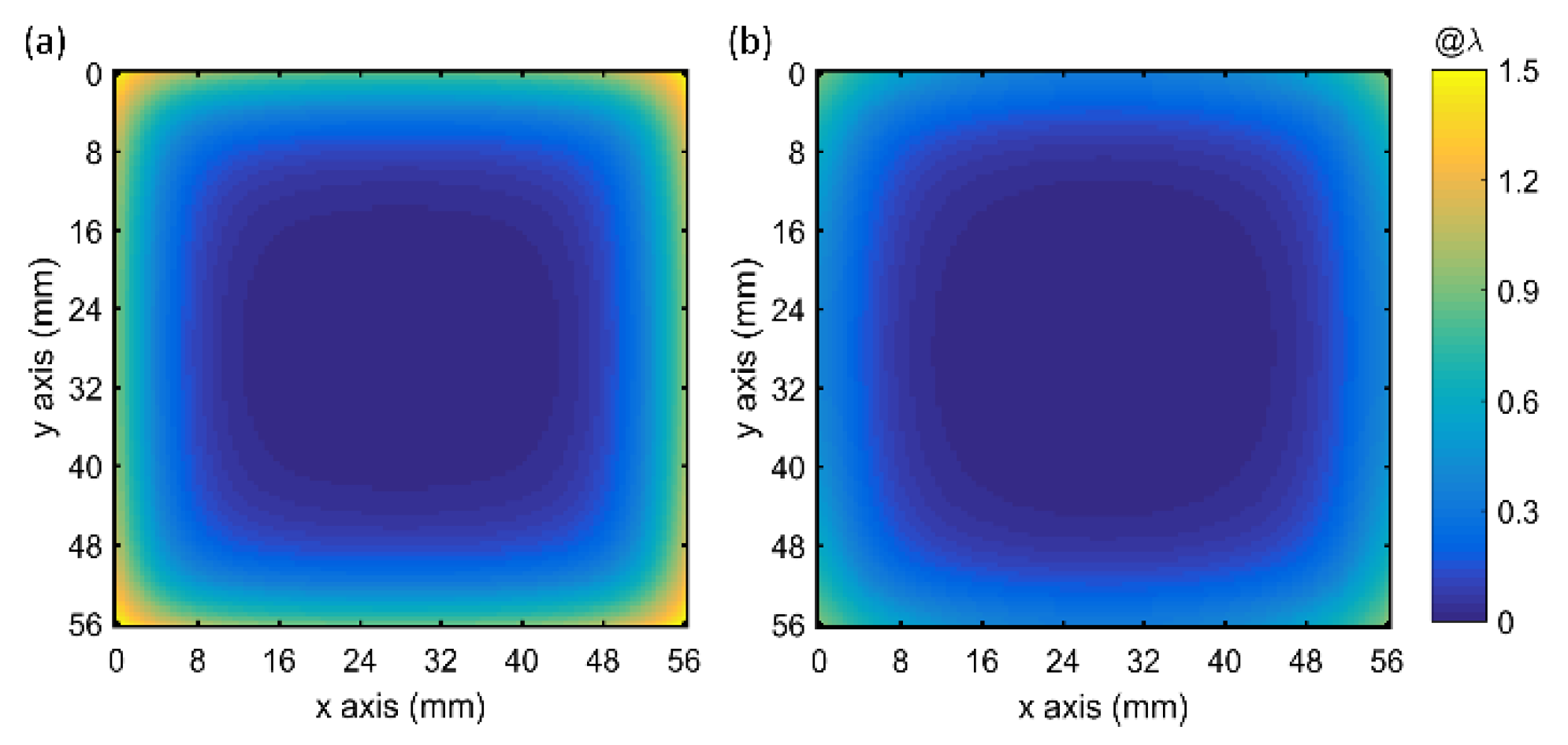
4.3. Wavefront Distribution
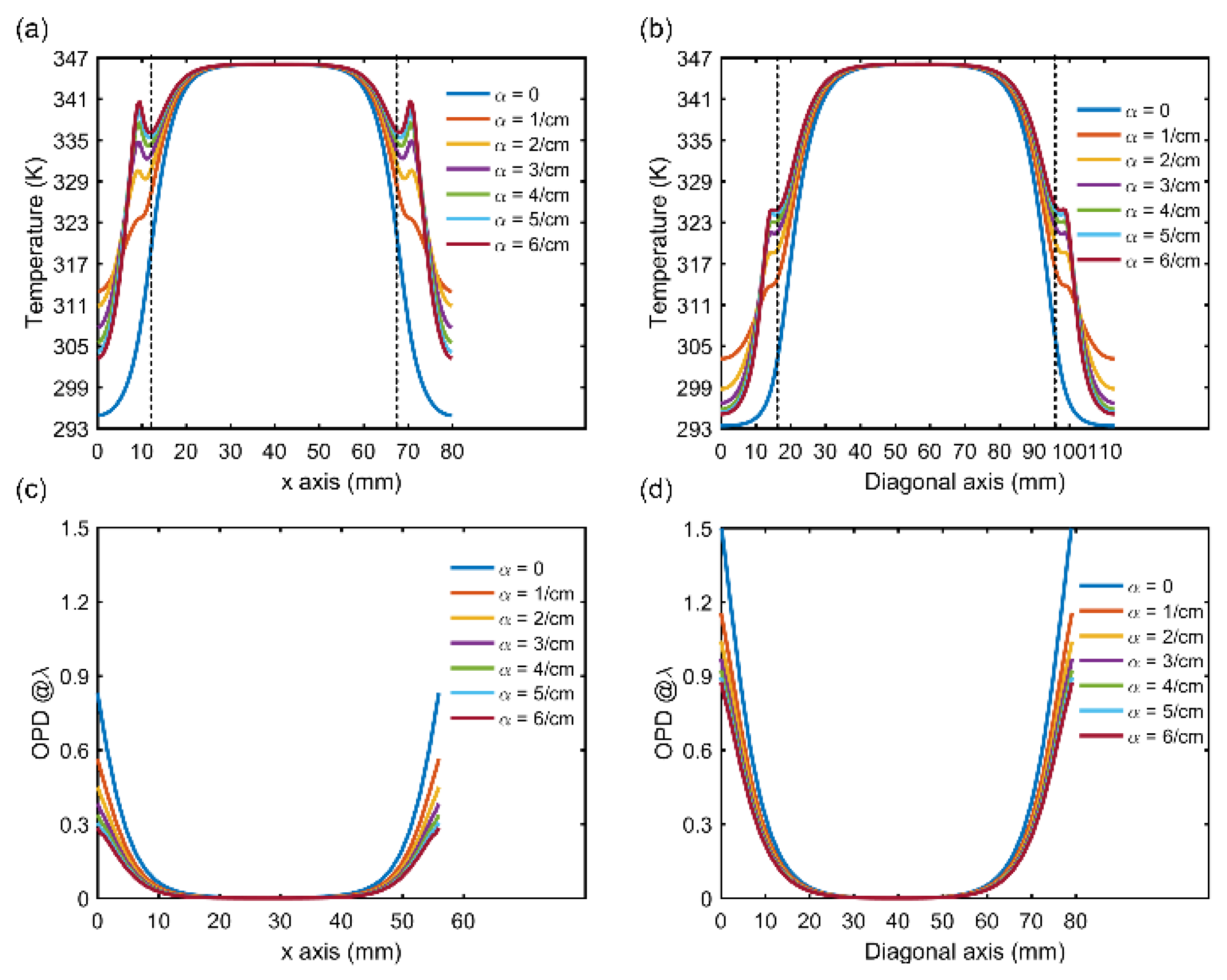
5. Optimal Design of the Gain Slab Architecture
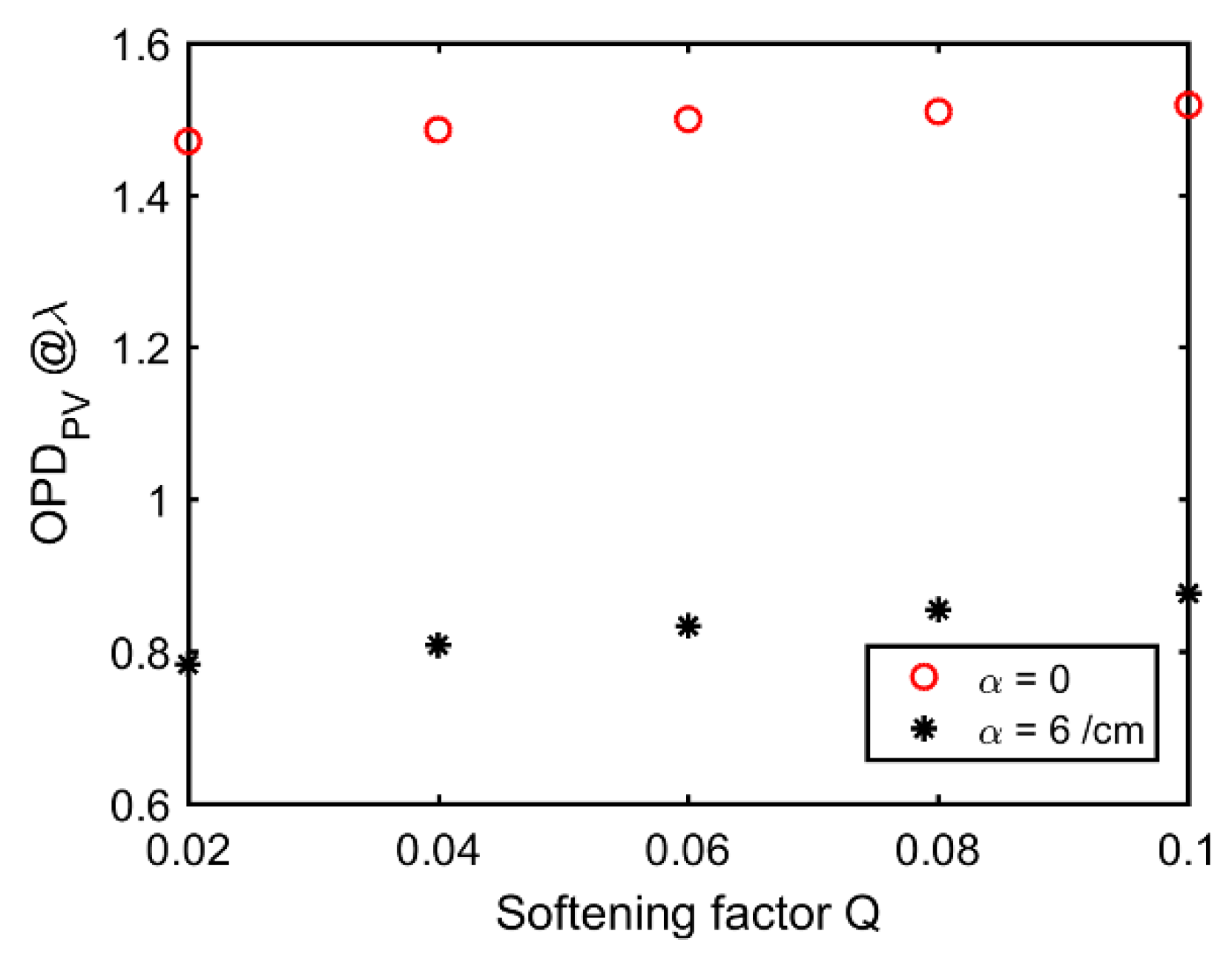
5.1. of the Absorbing Cladding Layer
5.2. Shape of the Pump Intensity
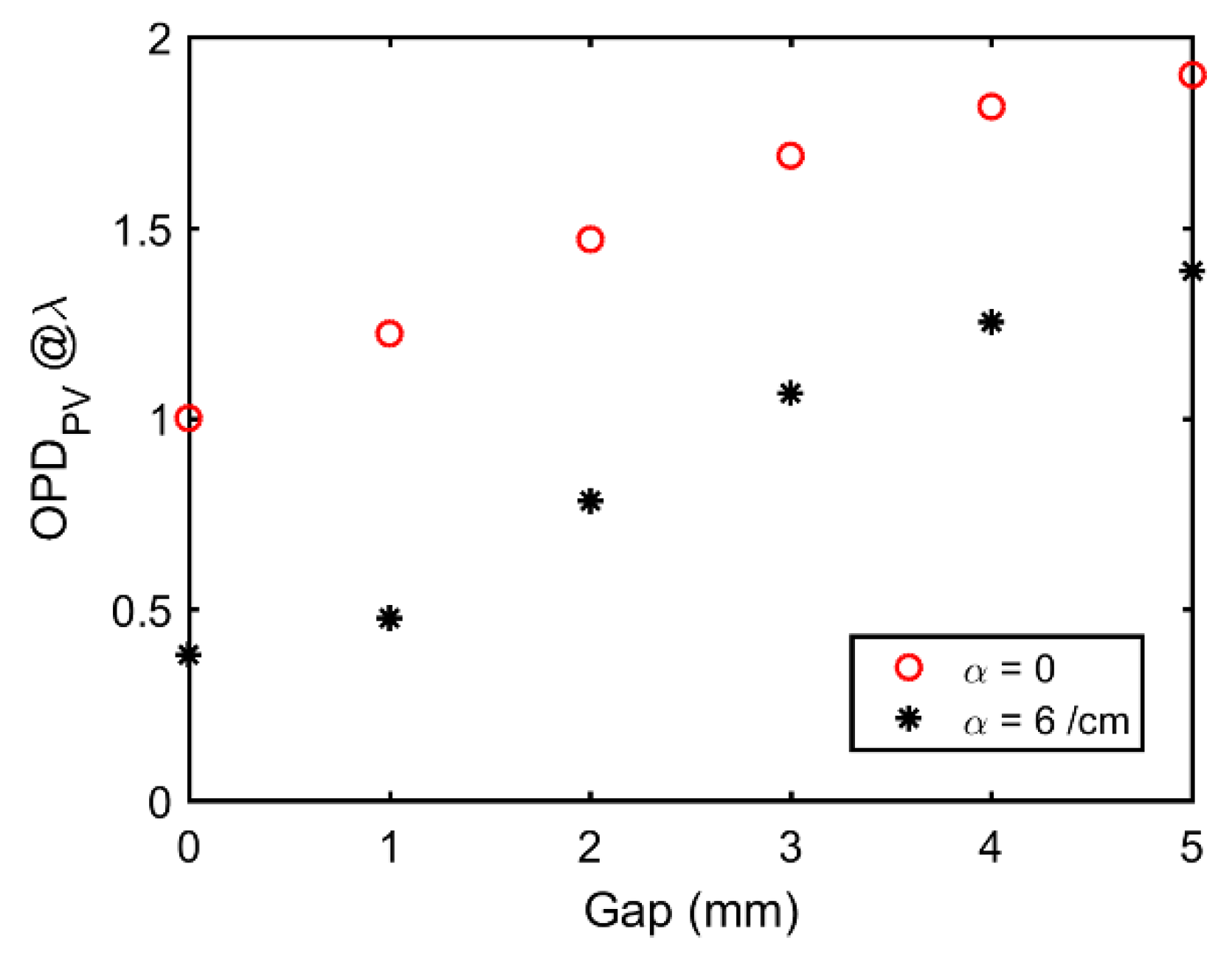
5.3. Gap between the Pump Area and Absorbing Cladding Layer
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Moses, E.I. The National Ignition Campaign: Status and progress. Nucl. Fusion 2013, 53. [Google Scholar] [CrossRef]
- Paul Drake, R. A journey through high-energy-density physics. Nucl. Fusion 2019, 59. [Google Scholar] [CrossRef]
- George, K.M.; Morrison, J.T.; Feister, S.; Ngirmang, G.K.; Smith, J.R.; Klim, A.J.; Snyder, J.; Austin, D.; Erbsen, W. High-repetition-rate(kHz)targets and optics from liquid microjets for high-intensity laser–plasma interactions. High Power Laser Sci. Eng. 2019, 7, e50. [Google Scholar] [CrossRef]
- Danson, C.N.; Haefner, C.; Bromage, J.; Butcher, T.; Chanteloup, J.-C.F.; Chowdhury, E.A.; Galvanauskas, A.; Gizzi, L.A.; Hein, J.; Hillier, D.I.; et al. Petawatt and exawatt class lasers worldwide. High Power Laser Sci. Eng. 2019, 7, e54. [Google Scholar] [CrossRef]
- Brenner, C.M.; Mirfayzi, S.R.; Rusby, D.R.; Armstrong, C.; Alejo, A.; Wilson, L.A.; Clarke, R.; Ahmed, H.; Butler, N.M.H.; Haddock, D.; et al. Laser-driven x-ray and neutron source development for industrial applications of plasma accelerators. Plasma Phys. Controll. Fusion 2016, 58. [Google Scholar] [CrossRef]
- Kawashima, T.; Kurita, T.; Matsumoto, O.; Ikegawa, T.; Sekine, T.; Miyamoto, M.; Iyama, K.; Kan, H.; Tsuchiya, Y.; Yasuhara, R. 20-J Diode-Pumped Zig-Zag Slab Laser with 2-GW Peak Power and 200-W Average Power. OSA Trends Opt. Photonics 2005, 98, 578–582. [Google Scholar]
- Gonçalvès-Novo, T.; Albach, D.; Vincent, B.; Arzakantsyan, M.; Chanteloup, J.-C. 14 J/2 Hz Yb 3+: YAG diode pumped solid state laser chain. Opt. Expr. 2013, 21, 855–866. [Google Scholar] [CrossRef]
- Banerjee, S.; Ertel, K.; Mason, P.D.; Phillips, P.J.; De Vido, M.; Smith, J.M.; Butcher, T.J.; Hernandez-Gomez, C.; Greenhalgh, R.J.; Collier, J.L. DiPOLE: A 10 J, 10 Hz cryogenic gas cooled multi-slab nanosecond Yb:YAG laser. Opt. Expr. 2015, 23, 19542–19551. [Google Scholar] [CrossRef]
- Hein, J.; Mason, P.D.; Banerjee, S.; Ertel, K.; Phillips, P.J.; Butcher, T.J.; Smith, J.M.; De Vido, M.; Tomlinson, S.; Chekhlov, O.; et al. DiPOLE100: A 100 J, 10 Hz DPSSL using cryogenic gas cooled Yb:YAG multi slab amplifier technology. In Proceedings of the High-Power, High-Energy, and High-Intensity Laser Technology II, Prague, Czech Republic, 14–15 April 2015; p. 951302. [Google Scholar]
- Banerjee, S.; Mason, P.; Phillips, J.; Smith, J.; Butcher, T.; Spear, J.; De Vido, M.; Quinn, G.; Clarke, D.; Ertel, K.; et al. Pushing the boundaries of diode-pumped solid-state lasers for high-energy applications. High Power Laser Sci. Eng. 2020, 8, e20. [Google Scholar] [CrossRef]
- Bayramian, A.; Aceves, S.; Anklam, T.; Baker, K.; Bliss, E.; Boley, C.; Bullington, A.; Caird, J.; Chen, D.; Deri, R.; et al. Compact, Efficient Laser Systems Required for Laser Inertial Fusion Energy. Fusion Technol. 2011, 60, 28–48. [Google Scholar] [CrossRef]
- Moses, E.I.; de la Rubia, T.D.; Storm, E.; Latkowski, J.F.; Farmer, J.C.; Abbott, R.P.; Peterson, P.F.; Shaw, H.F.; Lehman, R.F., II. A sustainable nuclear fuel cycle based on laser inertial fusion energy. Fusion Sci. Technol. 2009, 56, 547–565. [Google Scholar] [CrossRef]
- Bayramian, A.; Armstrong, P.; Ault, E.; Beach, R.; Bibeau, C.; Caird, J.; Campbell, R.; Chai, B.; Dawson, J.; Ebbers, C.; et al. The Mercury Project: A High Average Power, Gas-Cooled Laser for Inertial Fusion Energy Development. Fusion Sci. Technol. 2007, 52, 383–387. [Google Scholar] [CrossRef]
- Bayramian, A.; Armstrong, J.; Beer, G. High-average-power femto-petawatt laser pumped by the Mercury laser facility. J. Opt. Soc. Am. B 2008, 25, B57–B61. [Google Scholar] [CrossRef]
- Lucianetti, A.; Sawicka, M.; Slezak, O.; Divoky, M.; Pilar, J.; Jambunathan, V.; Bonora, S.; Antipenkov, R.; Mocek, T. Design of a kJ-class HiLASE laser as a driver for inertial fusion energy. High Power Laser Sci. Eng. 2014, 2, e13. [Google Scholar] [CrossRef]
- Mason, P.; Divoký, M.; Ertel, K.; Pilař, J.; Butcher, T.; Hanuš, M.; Banerjee, S.; Phillips, J.; Smith, J.; De Vido, M.; et al. Kilowatt average power 100 J-level diode pumped solid state laser. Optica 2017, 4, 1270–1276. [Google Scholar] [CrossRef]
- Yagi, H.; Bisson, J.F.; Ueda, K.; Yanagitani, T. Y3Al5O12 ceramic absorbers for the suppression of parasitic oscillation in high-power Nd:YAG lasers. J. Lumin. 2006, 121, 88–94. [Google Scholar] [CrossRef]
- Korn, G.; Silva, L.O.; Haefner, C.L.; Bayramian, A.; Betts, S.; Bopp, R.; Buck, S.; Cupal, J.; Drouin, M.; Erlandson, A.; et al. High average power, diode pumped petawatt laser systems: A new generation of lasers enabling precision science and commercial applications. In Proceedings of the Research Using Extreme Light: Entering New Frontiers with Petawatt-Class Lasers III, Prague, Czech Republic, 15–18 April 2013; p. 1024102. [Google Scholar]
- Sistrunk, E.F.; Spinka, T.; Bayramian, A.; Armstrong, P.; Haefner, C. All Diode-Pumped, High-repetition-rate Advanced Petawatt Laser System (HAPLS). In Proceedings of the Cleo: Science and Innovations, San Jose, CA, USA, 4–19 May 2017; p. STh1L-2. [Google Scholar]
- Huang, T.; Huang, W.; Wang, J.; Lu, X.; Li, X. Thermal-induced wavefront aberration in sapphire-cooled Nd:glass slab. Appl. Phys. B 2016, 122, 1–9. [Google Scholar] [CrossRef]
- Kuz’min, A.A.; Luchinin, A.G.; Poteomkin, A.K.; Soloviev, A.A.; Khazanov, E.A.; Shaikin, A.A. Thermally induced distortions in neodymium glass rod amplifiers. Quantum Electron. 2009, 39, 895–900. [Google Scholar] [CrossRef]
- Koechner, W. Thermal Lensing in a Nd:YAG Laser Rod. Appl. Opt. 1970, 9, 2548–2553. [Google Scholar] [CrossRef]
- Slezak, O.; Lucianetti, A.; Divoky, M.; Sawicka, M.; Mocek, T. Optimization of Wavefront Distortions and Thermal-Stress Induced Birefringence in a Cryogenically-Cooled Multislab Laser Amplifier. IEEE J. Quantum Electron. 2013, 49, 960–966. [Google Scholar] [CrossRef]
- Slezak, O.; Lucianetti, A.; Mocek, T. Efficient ASE Management in Disk Laser Amplifiers With Variable Absorbing Clads. IEEE J. Quantum Electron. 2014, 50, 1–9. [Google Scholar] [CrossRef]
- Sawicka-Chyla, M.; Divoky, M.; Slezak, O.; De Vido, M.; Lucianetti, A.; Mocek, T. Numerical Analysis of Thermal Effects in a Concept of a Cryogenically Cooled Yb: YAG Multislab 10 J/100-Hz Laser Amplifier. IEEE J. Quantum Electron. 2019, 55, 1–8. [Google Scholar] [CrossRef]
- Mason, P.D.; Fitton, M.; Lintern, A.; Banerjee, S.; Ertel, K.; Davenne, T.; Hill, J.; Blake, S.P.; Phillips, P.J.; Butcher, T.J.; et al. Scalable design for a high energy cryogenic gas cooled diode pumped laser amplifier. Appl. Opt. 2015, 54. [Google Scholar] [CrossRef]
- De Vido, M.; Mason, P.D.; Fitton, M.; Eardley, R.W.; Quinn, G.; Clarke, D.; Ertel, K.; Butcher, T.J.; Jonathan Phillips, P.; Banerjee, S.; et al. Modelling and measurement of thermal stress-induced depolarisation in high energy, high repetition rate diode-pumped Yb:YAG lasers. Opt. Expr. 2021, 29. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.; Sui, Z.; Chen, L.; Li, Z.; Liu, Q.; Gong, M.; Fu, X. 12 J, 10 Hz diode-pumped Nd:YAG distributed active mirror amplifier chain with ASE suppression. Opt. Expr. 2017, 25, 21981–21992. [Google Scholar] [CrossRef] [PubMed]
- Yasuhara, R.; Kawashima, T.; Sekine, T.; Kurita, T.; Ikegawa, T.; Matsumoto, O.; Miyamoto, M.; Kan, H.; Yoshida, H.; Kawanaka, J.; et al. 213 W average power of 2.4 GW pulsed thermally controlled Nd glass zigzag slab laser with a stimulated Brillouin scattering mirror. Opt. Lett. 2008, 33, 1711–1713. [Google Scholar] [CrossRef]
- Campbell, J.H.; Suratwala, T.I. Nd-doped phosphate glasses for high-energy/high-peak-power lasers. J. Non.·Cryst. Solids 2000, 263, 318–341. [Google Scholar] [CrossRef]
- Zhu, J.; Zhu, J.; Li, X.; Zhu, B.; Ma, W.; Lu, X.; Fan, W.; Liu, Z.; Zhou, S.; Xu, G.; et al. Status and development of high-power laser facilities at the NLHPLP. High Power Laser Sci. Eng. 2018, 6, e55. [Google Scholar] [CrossRef]
- Hu, L.; Chen, S.; Tang, J.; Wang, B.; Meng, T.; Chen, W.; Wen, L.; Hu, J.; Li, S.; Xu, Y.; et al. Large aperture N31 neodymium phosphate laser glass for use in a high power laser facility. High Power Laser Sci. Eng. 2014, 2, e1. [Google Scholar] [CrossRef]
- Gaul, E.W.; Martinez, M.; Blakeney, J.; Jochmann, A.; Ringuette, M.; Hammond, D.; Borger, T.; Escamilla, R.; Douglas, S.; Henderson, W. Demonstration of a 1.1 petawatt laser based on a hybrid optical parametric chirped pulse amplification/mixed Nd:glass amplifier. Appl. Opt. 2015, 49, 1676–1681. [Google Scholar] [CrossRef]
- Jacinto, C.; Oliveira, S.L.; Catunda, T.; Andrade, A.A.; Myers, J.D.; Myers, M.J. Upconversion effect on fluorescence quantum efficiency and heat generation in Nd3+-doped materials. Opt. Expr. 2005, 13, 2040–2046. [Google Scholar] [CrossRef]
- Powell, R.C.; Payne, S.A.; Chase, L.L.; Wilke, G.D. Index-of-refraction change in optically pumped solid-state laser materials. Opt. Lett. 1989, 14, 1204–1206. [Google Scholar] [CrossRef]
- Antipov, O.L.; Anashkina, E.A.; Fedorova, K.A. Electronic and thermal lensing in diode end-pumped Yb:YAG laser rods and discs. Quantum Electron. 2009, 39, 1131–1136. [Google Scholar] [CrossRef]
- Eggleston, J.M.; Kane, T.J.; Kuhn, K.; Unternahrer, J.; Byer, R.L. The Slab Geometry Laser-Part I: Theory. IEEE J. Quantum Electron. 1984, 20, 289–301. [Google Scholar] [CrossRef]
- Cousins, A.K. Temperature and Thermal Stress Scaling In Finite-Length End-Pumped Laser Rods. IEEE J. Quantum Electron. 1992, 28, 1057–1069. [Google Scholar] [CrossRef]
- Hu, J.; Men, T.; Chen, Y.; Wen, L.; He, H.; Hu, L. Investigation on the temperature rise and thermal stress of edge-cladding. In Proceedings of the Selected Papers of the Chinese Society for Optical Engineering Conferences, Chengdu, China; 2016; p. 102550. [Google Scholar]
- Banerjee, S.; Mason, P.D.; Ertel, K.; Jonathan Phillips, P.; De Vido, M.; Chekhlov, O.; Divoky, M.; Pilar, J.; Smith, J.; Butcher, T.; et al. 100 J-level nanosecond pulsed diode pumped solid state laser. Opt. Lett. 2016, 41, 2089–2092. [Google Scholar] [CrossRef] [PubMed]
- Ye, Z.; Liu, C.; Tu, B.; Wang, K.; Gao, Q.; Tang, C.; Cai, Z. Kilowatt-level direct-’refractive index matching liquid’-cooled Nd:YLF thin disk laser resonator. Opt. Expr. 2016, 24, 1758–1772. [Google Scholar] [CrossRef]




| Properties | Nd: Glass | Cladding Layer |
|---|---|---|
| Stimulation emission cross-section/(10−20cm2) | 3.8 | - |
| Fluorescence lifetime/µs | 351 | - |
| Density/(g/cm3) | 2.87 | 2.86 |
| Refractive index at the lasing wavelength | 1.533 | 1.5306 |
| Thermo-optical coefficient (dn/dT)/(10−7K−1) | −43 | - |
| Coefficient of the thermal expansion/(10−7K−1) | 116 | 112.7 |
| Thermal conductivity/(W·m−1·K−1) | 0.56 | 0.56 |
| Specific heat/(J·g−1·K−1) | 0.75 | 0.75 |
| Young’s modulus/(GPa) | 56.4 | 52.7 |
| Poisson’s ratio | 0.26 | 0.27 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Wang, J.; Guo, J.; Lu, X.; Wang, Y.; Xiao, Q.; Fan, W.; Li, X. Numerical Simulation of Thermo-Optic Effects in an Nd: Glass Slab with Low Thermally Induced Wavefront Distortion. Photonics 2021, 8, 91. https://doi.org/10.3390/photonics8040091
Wang X, Wang J, Guo J, Lu X, Wang Y, Xiao Q, Fan W, Li X. Numerical Simulation of Thermo-Optic Effects in an Nd: Glass Slab with Low Thermally Induced Wavefront Distortion. Photonics. 2021; 8(4):91. https://doi.org/10.3390/photonics8040091
Chicago/Turabian StyleWang, Xiaoqin, Jiangfeng Wang, Jiangtao Guo, Xinghua Lu, Yamin Wang, Qi Xiao, Wei Fan, and Xuechun Li. 2021. "Numerical Simulation of Thermo-Optic Effects in an Nd: Glass Slab with Low Thermally Induced Wavefront Distortion" Photonics 8, no. 4: 91. https://doi.org/10.3390/photonics8040091
APA StyleWang, X., Wang, J., Guo, J., Lu, X., Wang, Y., Xiao, Q., Fan, W., & Li, X. (2021). Numerical Simulation of Thermo-Optic Effects in an Nd: Glass Slab with Low Thermally Induced Wavefront Distortion. Photonics, 8(4), 91. https://doi.org/10.3390/photonics8040091