Multiple Filamentation Effects on THz Radiation Pattern from Laser Plasma in Air
Abstract
1. Introduction
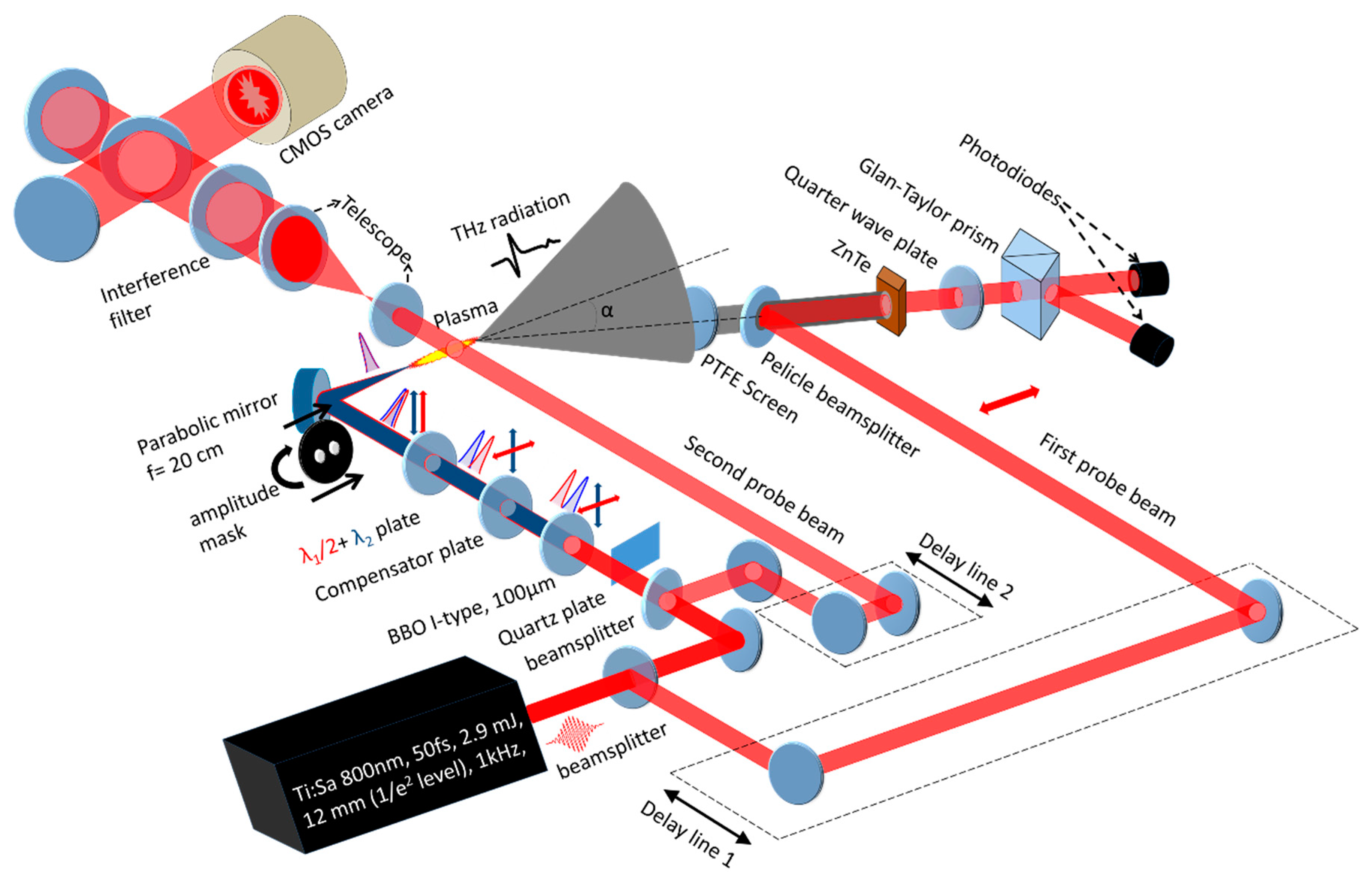
2. Experimental Setup
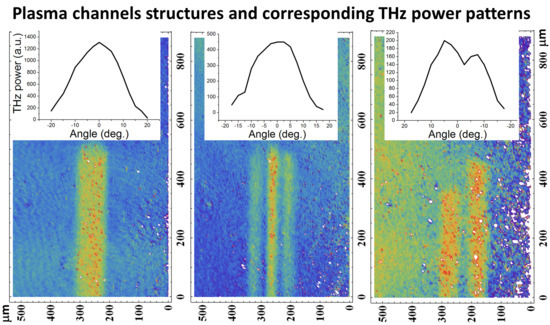
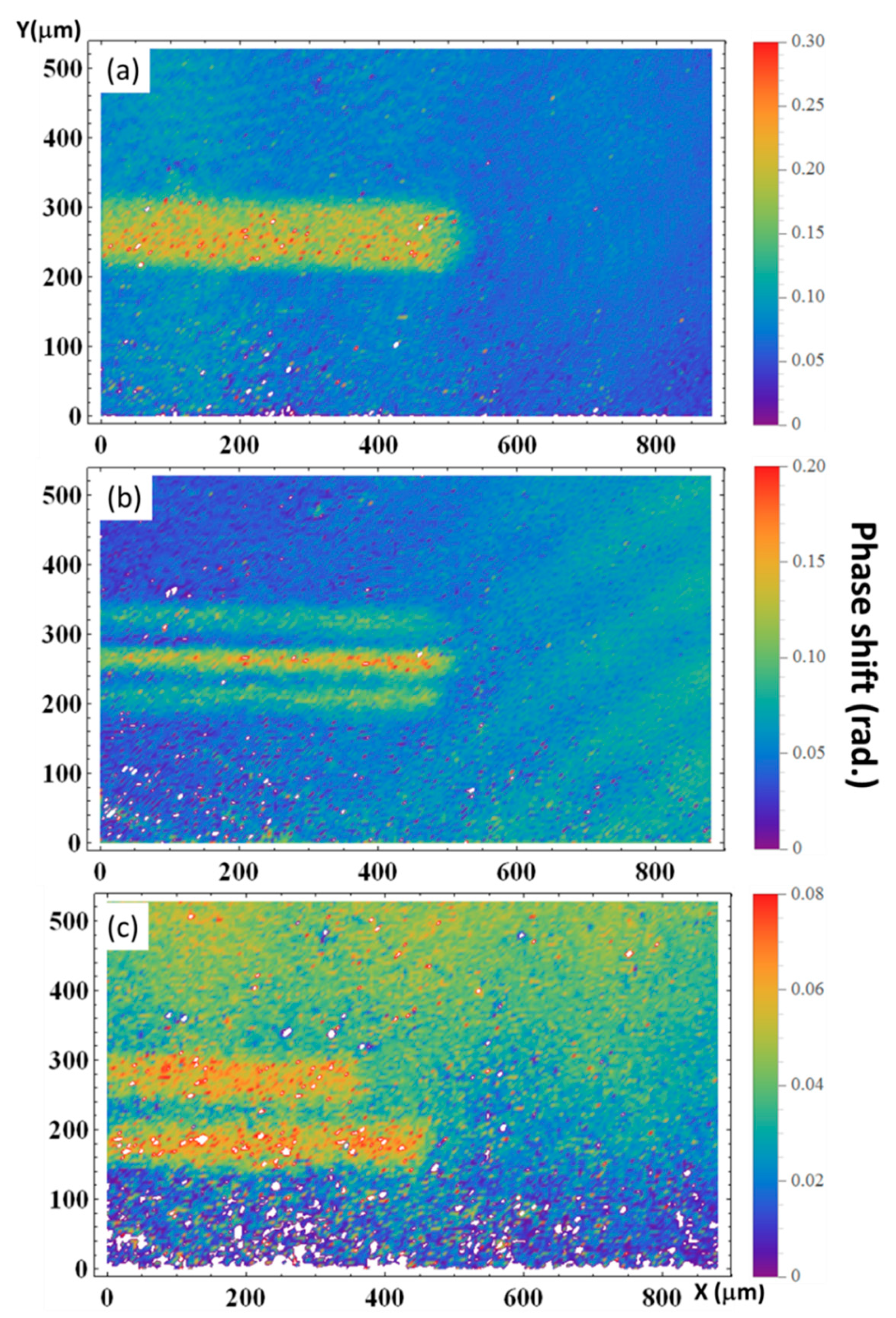
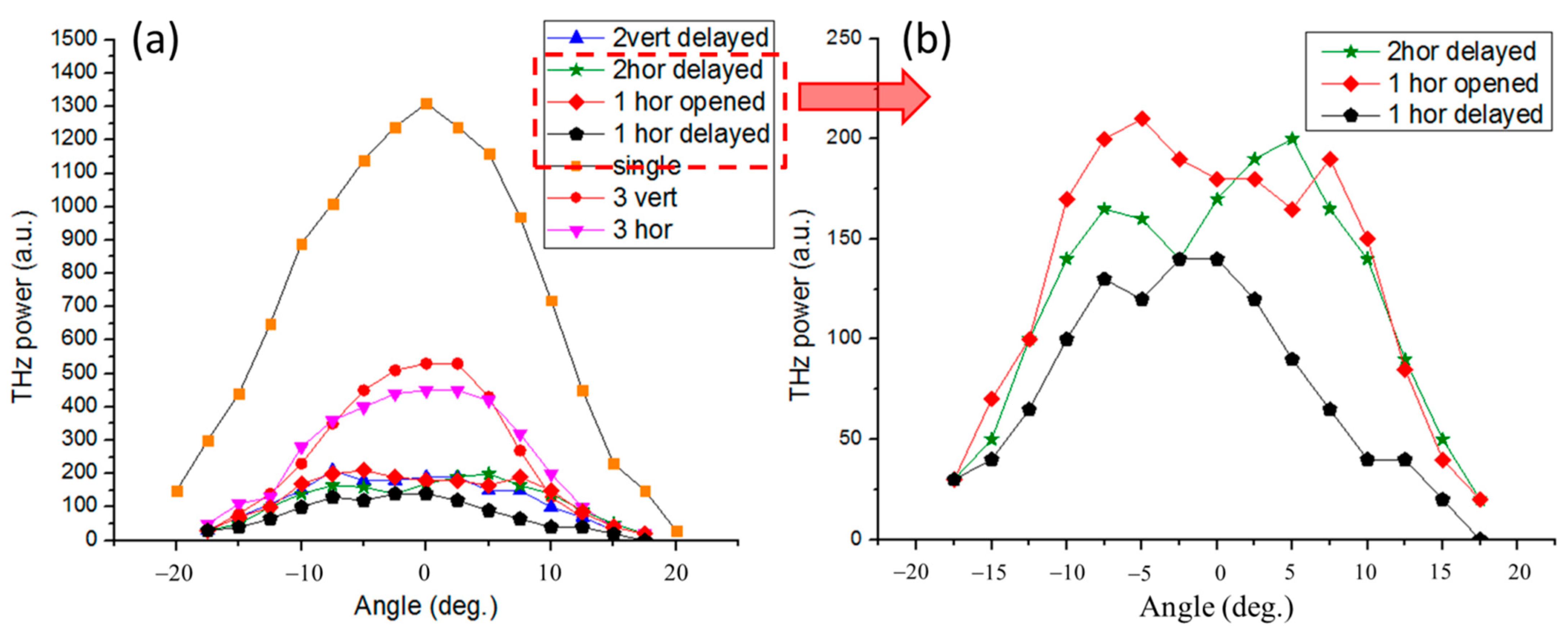
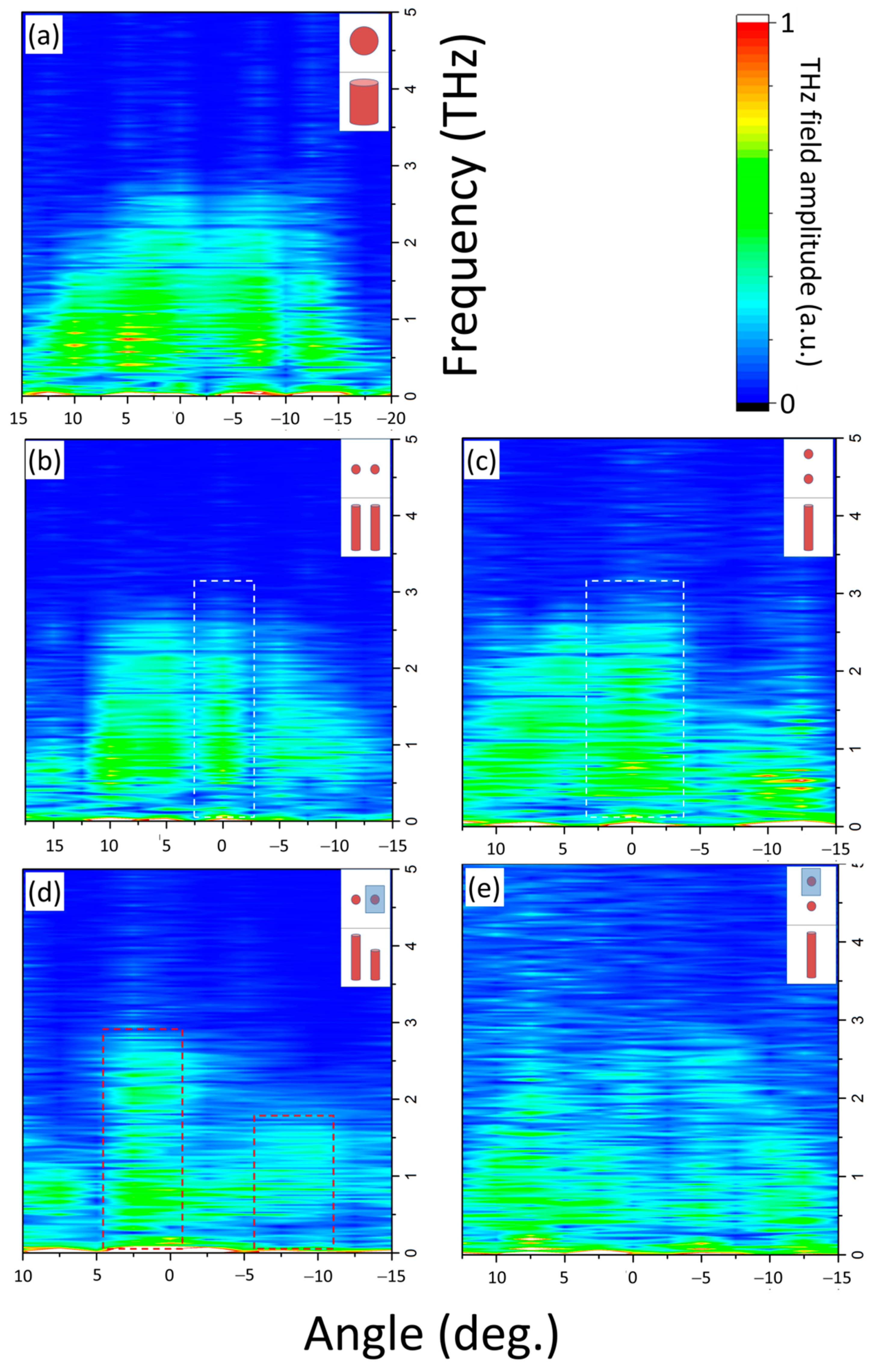
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, X.-C.; Xu, J. Introduction to THz Wave Photonics; Springer: New York, NY, USA, 2010; pp. 10–200. [Google Scholar]
- Matsubara, E.; Nagai, M.; Ashida, M. Ultrabroadband coherent electric field from far infrared to 200 THz using air plasma induced by 10 fs pulses. Appl. Phys. Lett. 2012, 101, 011105. [Google Scholar] [CrossRef]
- Zhang, X.C.; Shkurinov, A.; Zhang, Y. Extreme terahertz science. Nat. Photonics 2017, 11, 16–18. [Google Scholar] [CrossRef]
- Hamster, H.; Sullivan, A.; Gordon, S.; White, W.; Falcone, R.W. Subpicosecond, Electromagnetic Pulses from Intence Laser-Plasma Interaction. Phys. Rev. Lett. 1993, 71, 2725–2728. [Google Scholar] [CrossRef] [PubMed]
- Cook, D.J.; Hochstrasser, R.M. Intense terahertz pulses by four-wave rectification in air. Opt. Lett. 2000, 25, 1210–1212. [Google Scholar] [CrossRef]
- Chizhov, P.A.; Ushakov, A.; Bukin, V.V.; Garnov, S.V. Terahertz radiation from extended two-colour air filaments. Laser Phys. Lett. 2019, 16, 075301. [Google Scholar] [CrossRef]
- Chizhov, P.A.; Volkov, R.V.; Bukin, V.V.; Ushakov, A.A.; Garnov, S.V.; Savel’ev-Trofimov, A.B. Generation of terahertz ra-diation by focusing femtosecond bichromatic laser pulses in a gas or plasma. Quantum Electron. 2013, 43, 347–349. [Google Scholar] [CrossRef]
- Kim, K.Y.; Glownia, J.H.; Taylor, A.J.; Rodriguez, G. Terahertz emission from ultrafast ionizing air in symmetry-broken laser fields. Opt. Express 2007, 15, 4577–4584. [Google Scholar] [CrossRef]
- Löffler, T.; Roskos, H.G. Gas-pressure dependence of terahertz-pulse generation in a laser-generated nitrogen plasma. J. Appl. Phys. 2002, 91, 2611–2614. [Google Scholar] [CrossRef]
- Volkov, R.V.; Chizhov, P.; Ushakov, A.; Bukin, V.V.; Garnov, S.V.; Savel’ev, A. Optimal polarization of a two-colored pump for terahertz generation with a phase-unstable scheme. Laser Phys. 2015, 25, 065403. [Google Scholar] [CrossRef]
- Ponomareva, E.A.; Tcypkin, A.N.; Smirnov, S.V.; Putilin, S.E.; Yiwen, E.; Kozlov, S.A.; Zhang, X.-C. Double-pump technique—One step closer towards efficient liquid-based THz sources. Opt. Express 2019, 27, 32855–32862. [Google Scholar] [CrossRef]
- Xie, X.; Dai, J.; Zhang, X.-C. Coherent Control of THz Wave Generation in Ambient Air. Phys. Rev. Lett. 2006, 96, 075005. [Google Scholar] [CrossRef] [PubMed]
- Houard, A.; Liu, Y.; Prade, B.; Mysyrowicz, A. Polarization analysis of terahertz radiation generated by four-wave mixing in air. Opt. Lett. 2008, 33, 1195–1197. [Google Scholar] [CrossRef] [PubMed]
- Manceau, J.-M.; Massaouti, M.; Tzortzakis, S. Coherent control of THz pulses polarization from femtosecond laser filaments in gases. Opt. Express 2010, 18, 18894–18899. [Google Scholar] [CrossRef] [PubMed]
- Kosareva, O.G.; Panov, N.A.; Volkov, R.V.; Andreeva, V.; Borodin, A.V.; Esaulkov, M.N.; Chen, Y.; Marceau, C.; Makarov, V.A.; Shkurinov, A.; et al. Analysis of Dual Frequency Interaction in the Filament with the Purpose of Efficiency Control of THz Pulse Generation. J. Infrared Millim. Terahertz Waves 2011, 32, 1157–1167. [Google Scholar] [CrossRef]
- Dai, J.; Karpowicz, N.; Zhang, X.-C. Coherent Polarization Control of Terahertz Waves Generated from Two-Color Laser-Induced Gas Plasma. Phys. Rev. Lett. 2009, 103, 023001. [Google Scholar] [CrossRef] [PubMed]
- Esaulkov, M.N.; Kosareva, O.G.; Makarov, V.; Panov, N.; Shkurinov, A. Simultaneous generation of nonlinear optical harmonics and terahertz radiation in air: Polarization discrimination of various nonlinear contributions. Front. Optoelectron. 2015, 8, 73–80. [Google Scholar] [CrossRef]
- Zhong, H.; Karpowicz, N.; Zhang, X.-C. Terahertz emission profile from laser-induced air plasma. Appl. Phys. Lett. 2006, 88, 261103. [Google Scholar] [CrossRef]
- D’Amico, C.; Houard, A.; Franco, M.; Prade, B.; Mysyrowicz, A.; Couairon, A.; Tikhonchuk, V. Conical Forward THz Emission from Femtosecond-Laser-Beam Filamentation in Air. Phys. Rev. Lett. 2007, 98, 235002. [Google Scholar] [CrossRef]
- Borodin, A.V.; Esaulkov, M.N.; Kuritsyn, I.I.; Kotelnikov, I.A.; Shkurinov, A. On the role of photoionization in generation of terahertz radiation in the plasma of optical breakdown. J. Opt. Soc. Am. B 2012, 29, 1911–1919. [Google Scholar] [CrossRef]
- You, Y.S.; Oh, T.I.; Kim, K.Y. Off-Axis Phase-Matched Terahertz Emission from Two-Color Laser-Induced Plasma Filaments. Phys. Rev. Lett. 2012, 109, 183902. [Google Scholar] [CrossRef]
- Oh, T.I.; You, Y.S.; Jhajj, N.; Rosenthal, E.W.; Milchberg, H.M.; Kim, K.Y. Intense terahertz generation in two-color laser fil-amentation: Energy scaling with terawatt laser systems. New J. Phys. 2013, 15, 075002. [Google Scholar] [CrossRef]
- Blank, V.; Thomson, M.D.; Roskos, H.G. Spatio-spectral characteristics of ultra-broadband THz emission from two-colour photoexcited gas plasmas and their impact for nonlinear spectroscopy. New J. Phys. 2013, 15, 075023. [Google Scholar] [CrossRef]
- Gorodetsky, A.; Koulouklidis, A.D.; Massaouti, M.; Tzortzakis, S. Physics of the conical broadband terahertz emission from two-color laser-induced plasma filaments. Phys. Rev. A 2014, 89, 033838. [Google Scholar] [CrossRef]
- Buccheri, F.; Zhang, X. Terahertz emission from laser-induced microplasma in ambient air. Optica 2015, 2, 366. [Google Scholar] [CrossRef]
- Andreeva, V.A.; Kosareva, O.G.; Panov, N.A.; Shipilo, D.E.; Solyankin, P.M.; Esaulkov, M.N.; Martínez, P.G.D.A.; Shkurinov, A.P.; Makarov, V.A.; Bergé, L.; et al. Ultrabroad Terahertz Spectrum Generation from an Air-Based Filament Plasma. Phys. Rev. Lett. 2016, 116, 063902. [Google Scholar] [CrossRef]
- Shkurinov, A.; Sinko, A.S.; Solyankin, P.M.; Borodin, A.V.; Esaulkov, M.N.; Annenkov, V.V.; Kotelnikov, I.A.; Timofeev, I.V.; Zhang, X. Impact of the dipole contribution on the terahertz emission of air-based plasma induced by tightly focused femtosecond laser pulses. Phys. Rev. E 2017, 95, 043209. [Google Scholar] [CrossRef]
- Stremoukhov, S.Y.; Andreev, A.V. Spatial variations of the intensity of THz radiation emitted by extended media in two-color laser fields. Laser Phys. Lett. 2014, 12, 15402. [Google Scholar] [CrossRef]
- Ichii, T.; Hazama, Y.; Naka, N.; Tanaka, K. Study of detailed balance between excitons and free carriers in diamond using broadband terahertz time-domain spectroscopy. Appl. Phys. Lett. 2020, 116, 231102. [Google Scholar] [CrossRef]
- Han, P.; Wang, X.; Zhang, Y. Time-Resolved Terahertz Spectroscopy Studies on 2D Van der Waals Materials. Adv. Opt. Mater. 2020, 8, 1900533. [Google Scholar] [CrossRef]
- Chai, X.; Ropagnol, X.; Ovchinnikov, A.; Chefonov, O.; Ushakov, A.A.; García-Rosas, C.M.; Isgandarov, E.; Agranat, M.; Ozaki, T.; Savel’ev, A. Observation of crossover from intraband to interband nonlinear terahertz optics. Opt. Lett. 2018, 43, 5463–5466. [Google Scholar] [CrossRef]
- Point, G.; Brelet, Y.; Houard, A.; Jukna, V.; Milián, C.; Carbonnel, J.; Liu, Y.; Couairon, A.; Mysyrowicz, A. Superfilamentation in Air. Phys. Rev. Lett. 2014, 112, 223902. [Google Scholar] [CrossRef] [PubMed]
- Ushakov, A.A.; Chizhov, P.A.; Andreeva, V.A.; Panov, N.A.; Shipilo, D.E.; Matoba, M.; Nemoto, N.; Kanda, N.; Konishi, K.; Bukin, V.V.; et al. Ring and unimodal angular-frequency distribution of THz emission from two-color femtosecond plasma spark. Opt. Express 2018, 26, 18202. [Google Scholar] [CrossRef] [PubMed]
- Milián, C.; Jukna, V.; Couairon, A.; Houard, A.; Forestier, B.; Carbonnel, J.; Liu, Y.; Prade, B.; Mysyrowicz, A. Laser beam self-symmetrization in air in the multifilamentation regime. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 94013. [Google Scholar] [CrossRef][Green Version]
- Pushkarev, D.; Mitina, E.; Shipilo, D.; Panov, N.; Uryupina, D.; Ushakov, A.; Volkov, R.; Karabutov, A.; Babushkin, I.; Demircan, A.; et al. Transverse structure and energy deposition by a subTW femtosecond laser in air: From single filament to superfilament. New J. Phys. 2019, 21, 033027. [Google Scholar] [CrossRef]
- Pushkarev, D.; Shipilo, D.; Lar’kin, A.; Mitina, E.; Panov, N.; Uryupina, D.; Ushakov, A.; Volkov, R.; Karpeev, S.; Khonina, S.; et al. Effect of phase front modulation on the merging of multiple regularized femtosecond filaments. Laser Phys. Lett. 2018, 15, 045402. [Google Scholar] [CrossRef]
- Chu, C.; Shipilo, D.E.; Lu, D.; Zhang, Z.; Chuchupal, S.V.; Panov, N.A.; Kosareva, O.G.; Liu, W. Femtosecond filament emer-gence vetween π-shifted beamlets in air. Opt. Express 2020, 28, 1002–1013. [Google Scholar] [CrossRef]
- Liu, W.; Chin, S.L. Direct measurement of the critical power of femtosecond Ti:sapphire laser pulse in air. Opt. Express 2005, 13, 5750–5755. [Google Scholar] [CrossRef]
- Panov, N.; Andreeva, V.; Kosareva, O.; Shkurinov, A.; Makarov, V.; Bergé, L.; Chin, S.L. Directionality of terahertz radiation emitted from an array of femtosecond filaments in gases. Laser Phys. Lett. 2014, 11, 125401. [Google Scholar] [CrossRef]
- Jahangiri, F.; Hashida, M.; Tokita, S.; Nagashima, T.; Hangyo, M.; Sakabe, S. Enhancing the energy of terahertz radiation from plasma produced by intense femtosecond laser pulses. Appl. Phys. Lett. 2013, 102, 191106. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ushakov, A.; Chizhov, P.; Bukin, V.; Shipilo, D.; Panov, N.; Kosareva, O.; Garnov, S. Multiple Filamentation Effects on THz Radiation Pattern from Laser Plasma in Air. Photonics 2021, 8, 4. https://doi.org/10.3390/photonics8010004
Ushakov A, Chizhov P, Bukin V, Shipilo D, Panov N, Kosareva O, Garnov S. Multiple Filamentation Effects on THz Radiation Pattern from Laser Plasma in Air. Photonics. 2021; 8(1):4. https://doi.org/10.3390/photonics8010004
Chicago/Turabian StyleUshakov, Aleksandr, Pavel Chizhov, Vladimir Bukin, Daniil Shipilo, Nikolay Panov, Olga Kosareva, and Sergey Garnov. 2021. "Multiple Filamentation Effects on THz Radiation Pattern from Laser Plasma in Air" Photonics 8, no. 1: 4. https://doi.org/10.3390/photonics8010004
APA StyleUshakov, A., Chizhov, P., Bukin, V., Shipilo, D., Panov, N., Kosareva, O., & Garnov, S. (2021). Multiple Filamentation Effects on THz Radiation Pattern from Laser Plasma in Air. Photonics, 8(1), 4. https://doi.org/10.3390/photonics8010004