Numerical Investigation on a Hyperlens with a Large Radius Inner-Surface for Super-Resolution Imaging
Abstract
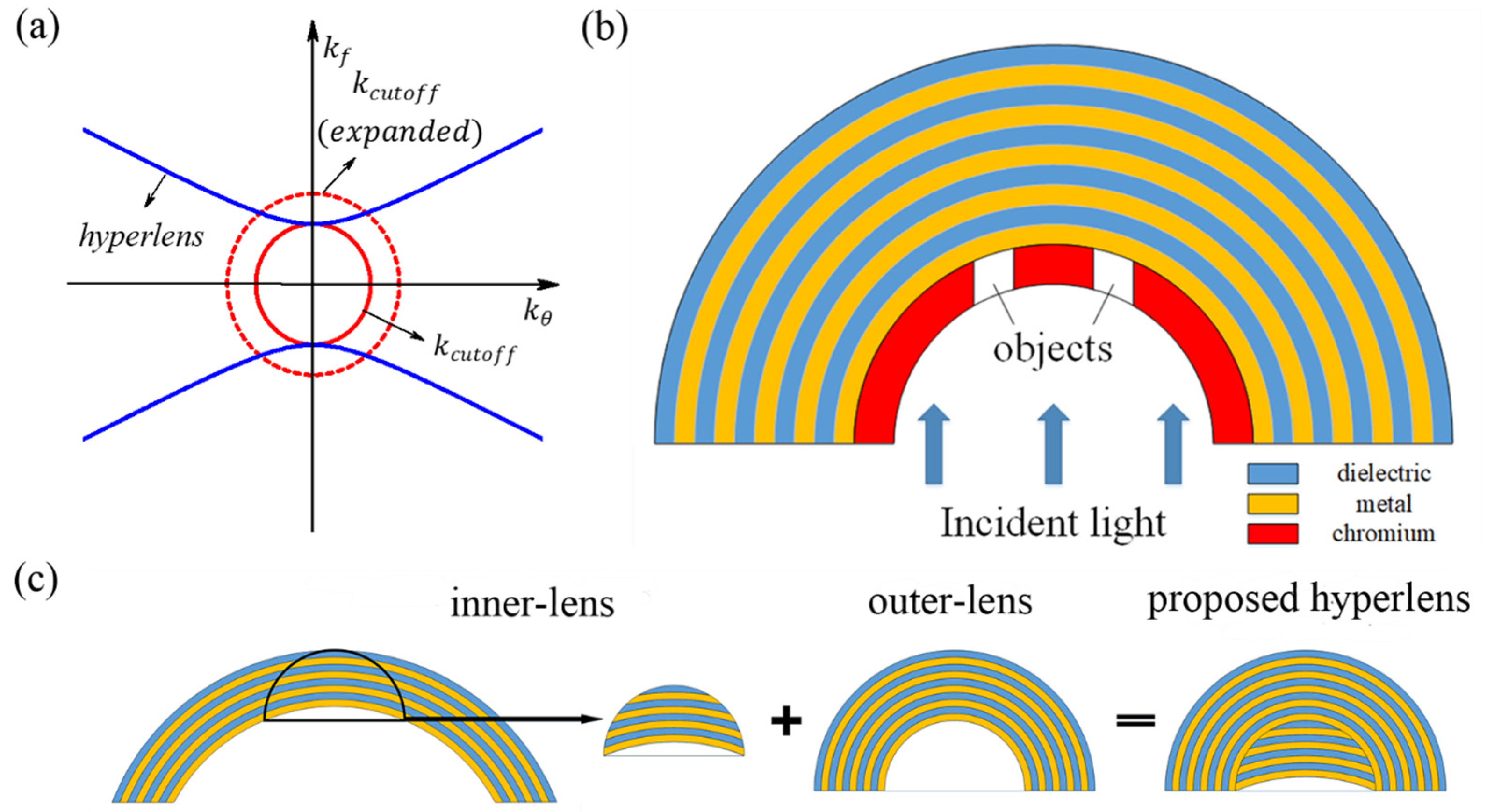
1. Introduction
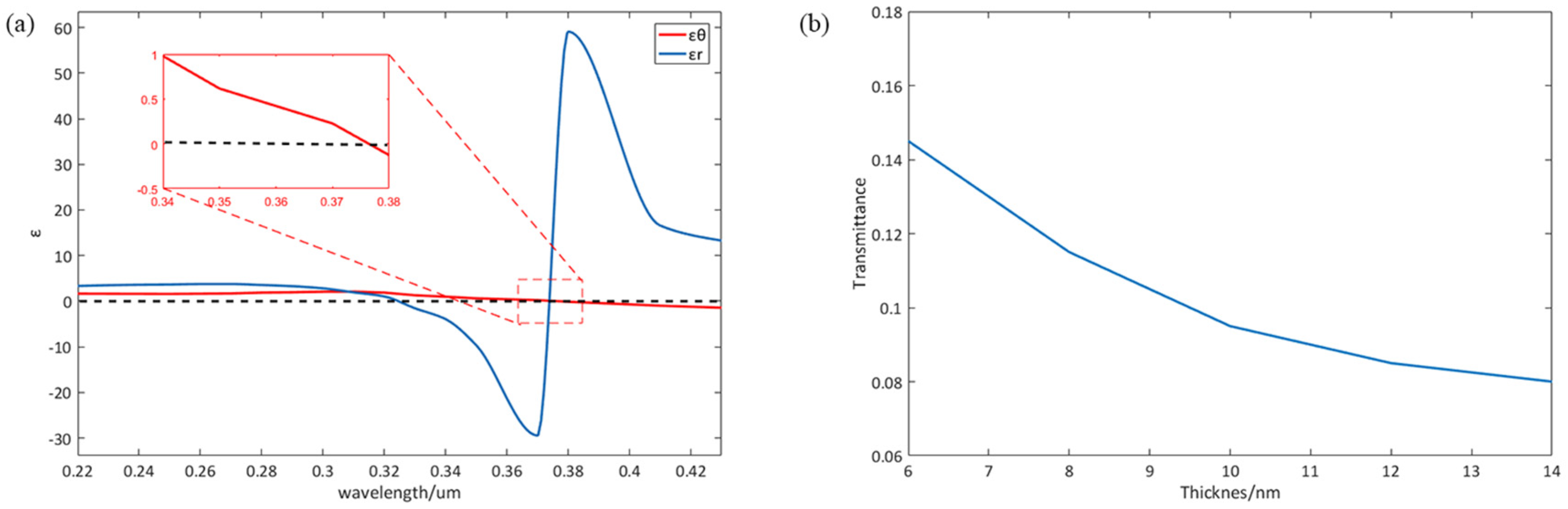
2. Materials and Methods
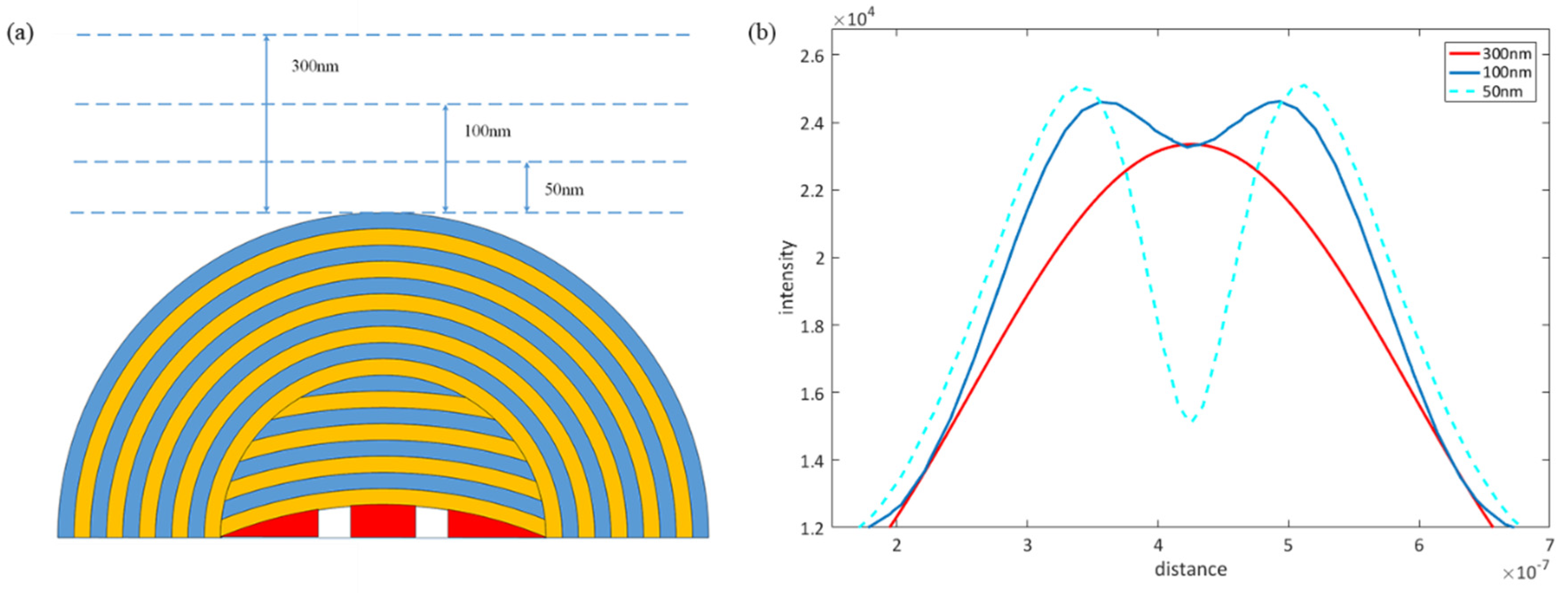
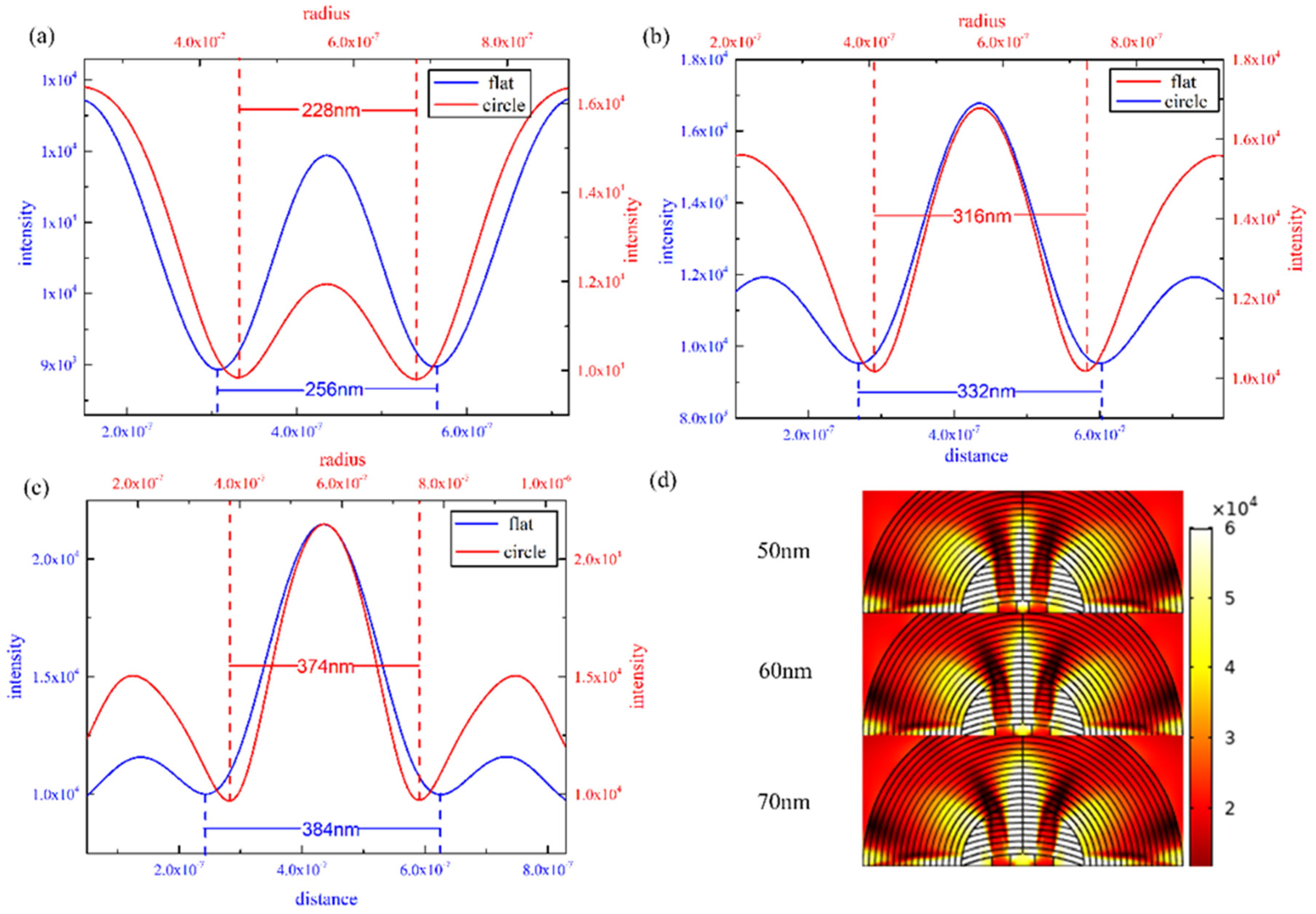
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Kohei, O.; Terumasa, H.; Yuichi, K.; Tomomi, N. STED microscopy—Super-resolution bio-imaging utilizing a stimulated emission depletion. Microscopy 2015, 64, 227–236. [Google Scholar]
- Chen, H.K.; Wei, S.B.; Wu, X.J.; Yang, Y.; Zhang, Y.Q.; Du, L.P.; Liu, J.; Zhu, S.W.; Yuan, X.C. Improved interference configuration for structured illumination microscopy. Opt. Commun. 2017, 384, 59–64. [Google Scholar] [CrossRef]
- Zhang, W.; Chen, Y. Visibility of subsurface nanostructures in scattering-type scanning near-field optical microscopy imaging. Opt. Express 2020, 28, 6696–6707. [Google Scholar] [CrossRef]
- Pendry, J.B. Negative refraction makes a perfect lens. Phys. Rev. Lett. 2000, 85, 3966–3969. [Google Scholar] [CrossRef] [PubMed]
- Mang, M.M.; Lloyd, D.T.; Anderson, P.N.; Treacher, D.; Wyatt, A.S.; Hooker, S.M.; Walmsley, I.A.; O’Keeffe, K. Spatially resolved common-path high-order harmonic interferometry. Opt. Lett. 2018, 43, 5275–5278. [Google Scholar] [CrossRef]
- Zhao, C.; Zhou, Y.S.; Gao, Q.; Zhang, Y. The imaging properties of the curved superlens. Opt. Commun. 2018, 407, 41–45. [Google Scholar] [CrossRef]
- Wang, J.; Sheng, Y.L. Thin metal superlens imaging in nanolithography. Int. J. Opt. 2019, 2019, 6513836. [Google Scholar] [CrossRef]
- Du, W.J.; Kong, W.J.; Liu, H.C.; Liu, K.P.; Wang, C.T.; Luo, X.G. Design of a structured bulk plasmon illumination source for enhancing plasmonic cavity superlens imaging. Plasmonics 2017, 13, 1387–1392. [Google Scholar] [CrossRef]
- Aronovich, D.; Bartal, G. Nonlinear hyperlens. Opt. Lett. 2013, 38, 413–415. [Google Scholar] [CrossRef]
- Sun, J.B.; Shalaev, M.I.; Litchinitser, N.M. Experimental demonstration of a non-resonant hyperlens in the visible spectral range. Nat. Commun. 2015, 6, 7201. [Google Scholar] [CrossRef]
- So, S.; Rho, J. Geometrically flat hyperlens designed by transformation optics. J. Phys. D Appl. Phys. 2019, 52, 194003. [Google Scholar] [CrossRef]
- Wang, J.X.; Xu, Y.; Chen, H.S.; Zhang, B.L. Ultraviolet dielectric hyperlens with layered graphene and boron nitride. J. Mater. Chem. 2012, 22, 15863–15868. [Google Scholar] [CrossRef]
- Lee, D.; Kim, Y.D.; Kim, M.; So, S.; Choi, H.J.; Mun, J.; Nguyen, D.M.; Badloe, T.; Ok, J.G.; Kim, K.; et al. Realization of wafer-scale hyperlens device for sub-diffractional biomolecular imaging. ACS Photonics 2017, 5, 2549–2554. [Google Scholar] [CrossRef]
- Si, G.Y.; Zhao, Y.H.; Lv, J.T.; Wang, F.W.; Liu, H.L.; Teng, J.H.; Liu, Y.J. Direct and accurate patterning of plasmonic nanostructures with ultrasmall gaps. Nanoscale 2013, 5, 4309–4313. [Google Scholar] [CrossRef]
- Jiang, X.X.; Gu, Q.C.; Wang, F.W.; Lv, J.T.; Ma, Z.H.; Si, G.Y. Fabrication of coaxial plasmonic crystals by focused ion beam milling and electron-beam lithography. Mater. Lett. 2013, 100, 192–194. [Google Scholar] [CrossRef]
- Si, G.Y.; Jiang, X.X.; Lv, J.T.; Gu, Q.C.; Wang, F.W. Fabrication and characterization of well-aligned plasmonic nanopillars with ultrasmall separations. Nanoscale Res. Lett. 2014, 9, 299. [Google Scholar] [CrossRef] [PubMed]
- Su, D.; Zhang, X.Y.; Ma, Y.L.; Shan, F.; Wu, J.Y.; Fu, X.C.; Zhang, L.J.; Yuan, K.Q.; Zhang, T. Real-time electro-optical tunable hyperlens under subwavelength scale. IEEE Photonics J. 2017, 10, 1–9. [Google Scholar] [CrossRef]
- Chen, Y.A.; Chang, I.L.; Chen, L.W. Spiral hyperlens with enhancements of image resolution and magnification. J. Mod. Opt. 2015, 63, 1029–1034. [Google Scholar] [CrossRef]
- Si, G.Y.; Zhao, Y.H.; Lv, J.T.; Lu, M.Q.; Wang, F.W.; Liu, H.L.; Xiang, N.; Huang, T.J.; Danner, A.J.; Teng, J.H.; et al. Reflective plasmonic color filters based on lithographically patterned silver nanorod arrays. Nanoscale 2013, 5, 6243–6248. [Google Scholar] [CrossRef]
- Novitsky, A.; Repan, T.; Zhukovsky, S.V.; Lavrinenko, A.V. Subwavelength hyperlens resolution with perfect contrast function. Ann. Phys. 2018, 530, 1700300. [Google Scholar] [CrossRef]
- Otomori, M.; Yamada, T.; Izui, K.; Nishiwaki, S.; Andkjaer, J. Topology optimization of hyperbolic metamaterials for an optical hyperlens. Struct. Multidiscip. Optim. 2016, 55, 913–923. [Google Scholar] [CrossRef]
- Si, G.Y.; Zhao, Y.H.; Leong, E.S.P.; Lv, J.T.; Liu, Y.J. Incident-angle dependent color tuning from a single plasmonic chip. Nanotechnology 2014, 25, 455203. [Google Scholar] [CrossRef]
- Wang, W.Z.; Yadav, N.P.; Shen, Z.; Cao, Y.; Liu, J.; Liu, X.F. Two-stage magnifying hyperlens structure based on metamaterials for super-resolution imaging. Optik 2018, 174, 199–206. [Google Scholar] [CrossRef]
- Si, G.Y.; Zhao, Y.H.; Liu, H.; Teo, S.; Zhang, M.S.; Huang, T.J.; Danner, A.J.; Teng, J.H. Annular aperture array based color filter. Appl. Phys. Lett. 2011, 99, 033105. [Google Scholar] [CrossRef]
- Liu, Z.W.; Lee, H.; Xiong, Y.; Sun, C.; Zhang, X. Far-Field optical hyperlens magnifying sub-diffraction-limited objects. Science 2007, 315, 1686. [Google Scholar] [CrossRef]
- Yan, K.; Liu, L.; Yao, N.; Liu, K.P.; Du, W.J.; Zhang, W.; Yan, W.; Wang, C.T.; Luo, X.G. Far-field super-resolution imaging of nano-transparent objects by hyperlens with plasmonic resonant cavity. Plasmonics 2015, 11, 475–481. [Google Scholar] [CrossRef]
- Cheng, L.; Cao, P.F.; Meng, Q.Q.; Zhang, X.P. Semi-cylindrical hyperlens made of Al/MgO for 20nm lithography node. Adv. Mater. Res. 2011, 179–180, 1047–1052. [Google Scholar] [CrossRef]
- Zhang, W.B.; Chen, H.S.; Moser, H.O. Subwavelength imaging in a cylindrical hyperlens based on S-string resonators. Appl. Phys. Lett. 2011, 98, 073501. [Google Scholar]
- Liang, G.F.; Zhao, Z.Y.; Yao, N.; Wang, C.T.; Jiang, B.; Zhao, Q.; Luo, X.G. Plane demagnifying nanolithography by hybrid hyperlens–superlens structure. J. Nanophotonics 2014, 8, 083080. [Google Scholar] [CrossRef]
- Lee, H.; Liu, Z.; Xiong, Y.; Sun, C.; Zhang, X. Development of optical hyperlens for imaging below the diffraction limit. Opt. Express 2007, 15, 15886–15891. [Google Scholar] [CrossRef]
- Tao, C.-W.; Yen, T.-J.; Huang, T.-Y. Achieving sub-wavelength imaging through a flat hyperlens in a modified anodic aluminum oxide template. Sci. Rep. 2020, 10, 5296. [Google Scholar] [CrossRef] [PubMed]
- Deel, A.J.; Alighanbari, A. Planar cascaded triangular hyperlens structures. Appl. Opt. 2020, 59, 2050–2056. [Google Scholar] [CrossRef]
- Zhang, H.; Jiao, Z.; Mcleod, E. Tunable terahertz hyperbolic metamaterial slabs and super-resolving hyperlenses. Appl. Opt. 2020, 59, G64–G70. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Lv, J.; Gu, Q.; Ying, Y.; Jiang, X.; Si, G. Numerical Investigation on a Hyperlens with a Large Radius Inner-Surface for Super-Resolution Imaging. Photonics 2020, 7, 107. https://doi.org/10.3390/photonics7040107
Yang J, Lv J, Gu Q, Ying Y, Jiang X, Si G. Numerical Investigation on a Hyperlens with a Large Radius Inner-Surface for Super-Resolution Imaging. Photonics. 2020; 7(4):107. https://doi.org/10.3390/photonics7040107
Chicago/Turabian StyleYang, Jiming, Jiangtao Lv, Qiongchan Gu, Yu Ying, Xiaoxiao Jiang, and Guangyuan Si. 2020. "Numerical Investigation on a Hyperlens with a Large Radius Inner-Surface for Super-Resolution Imaging" Photonics 7, no. 4: 107. https://doi.org/10.3390/photonics7040107
APA StyleYang, J., Lv, J., Gu, Q., Ying, Y., Jiang, X., & Si, G. (2020). Numerical Investigation on a Hyperlens with a Large Radius Inner-Surface for Super-Resolution Imaging. Photonics, 7(4), 107. https://doi.org/10.3390/photonics7040107




