Coupling of Integrated Waveguide and Optomechanic Cavity for Microwave Phonon Excitation in Si Nanobeams
Abstract
:1. Introduction
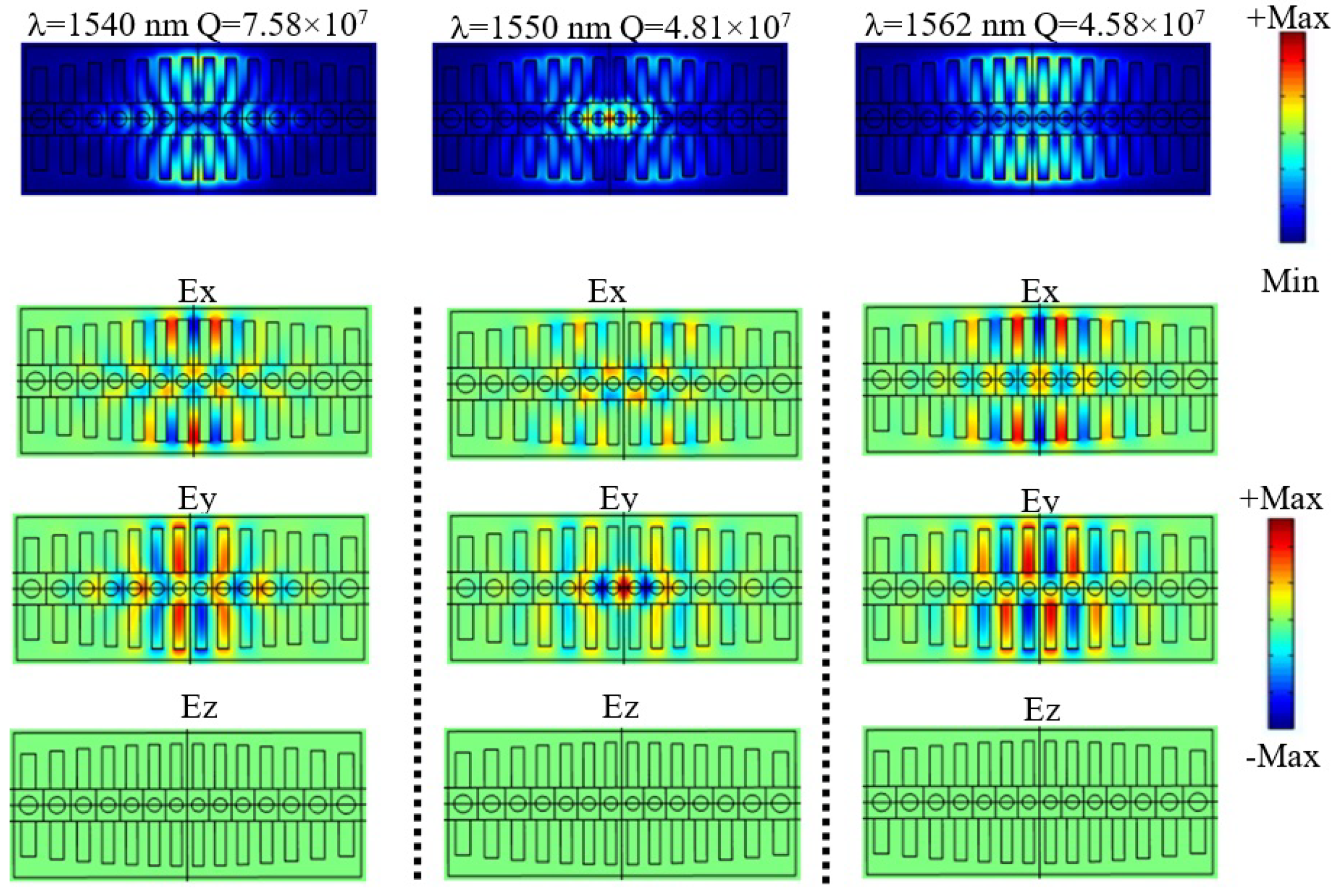
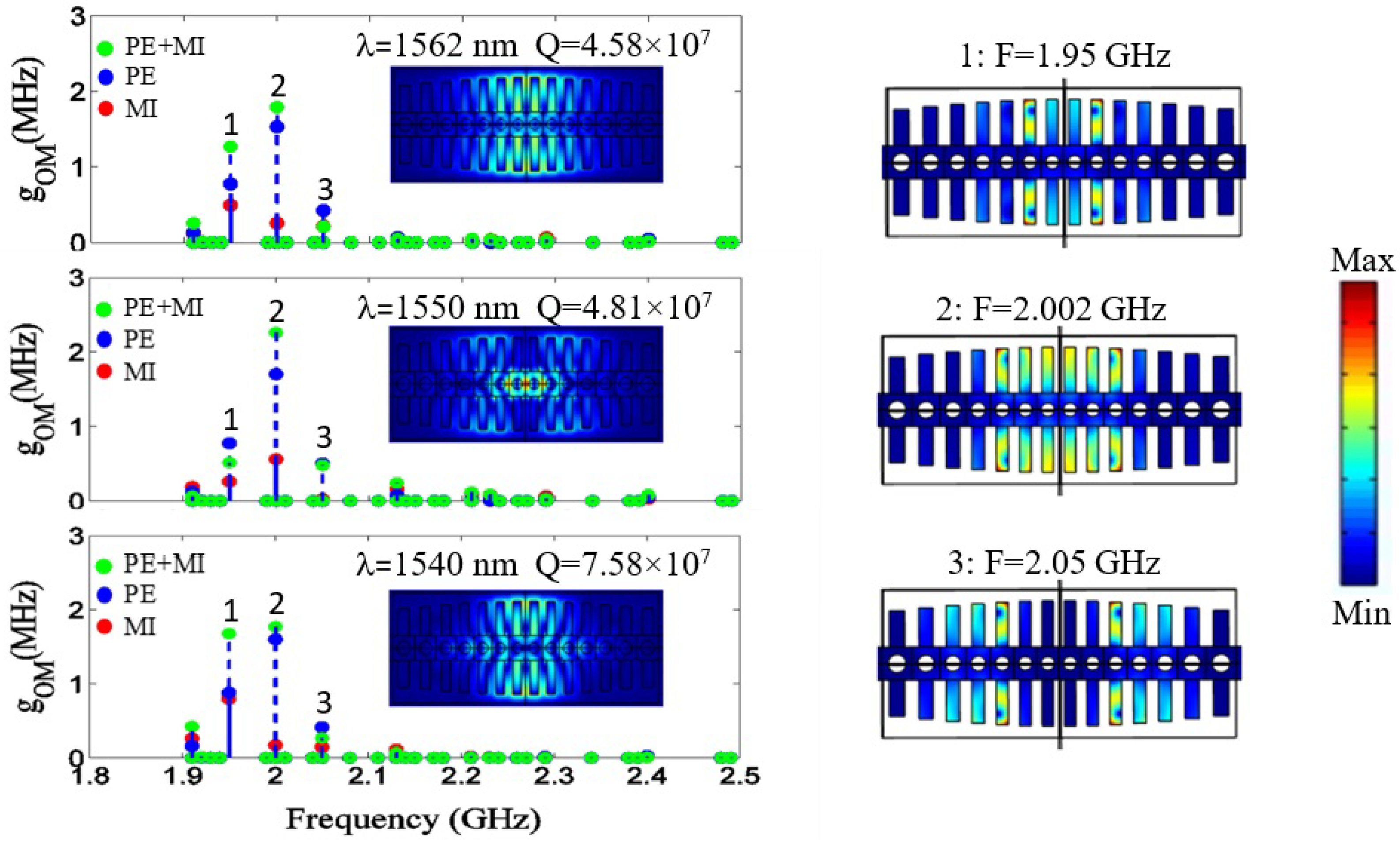
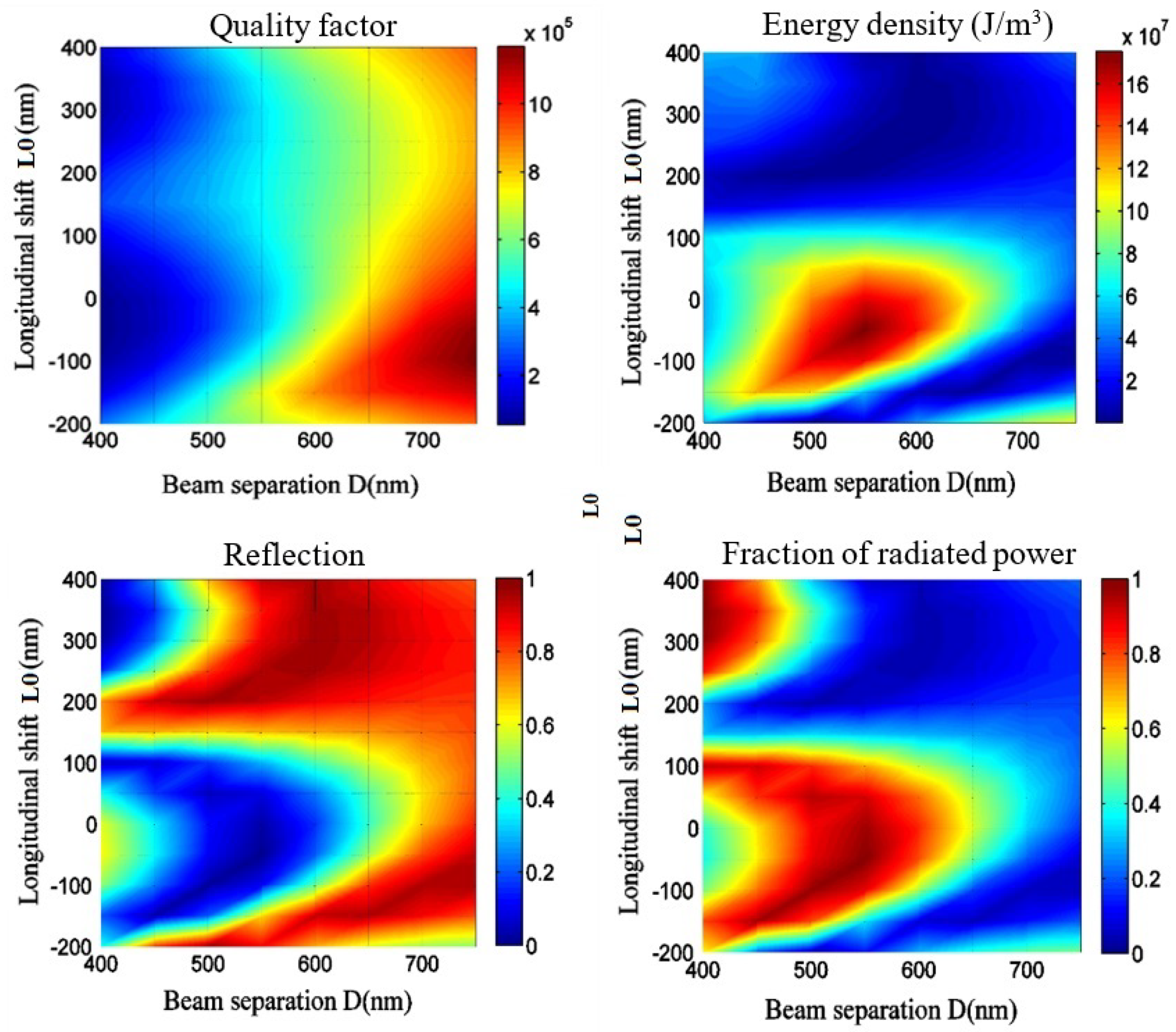
2. Results
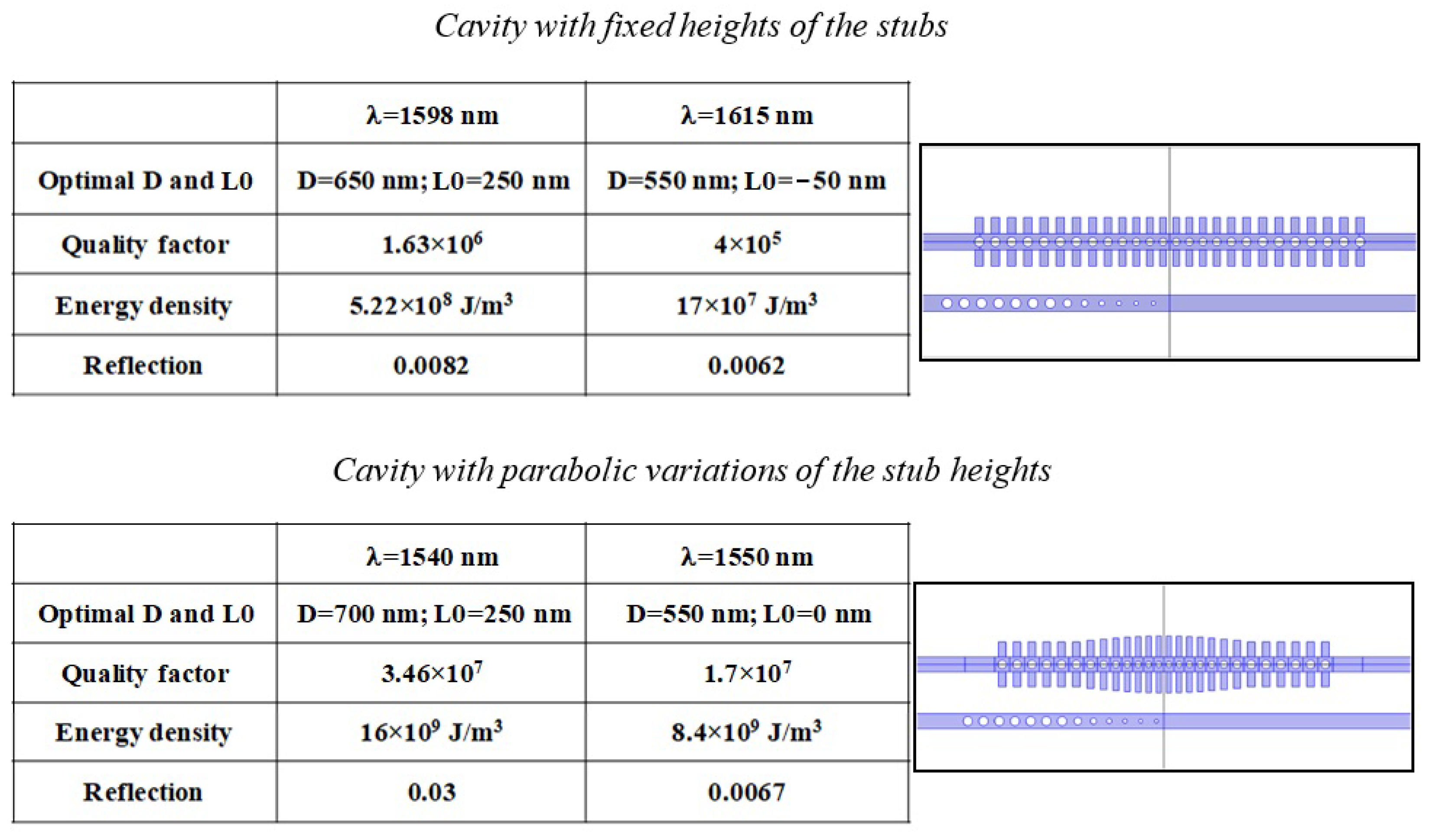
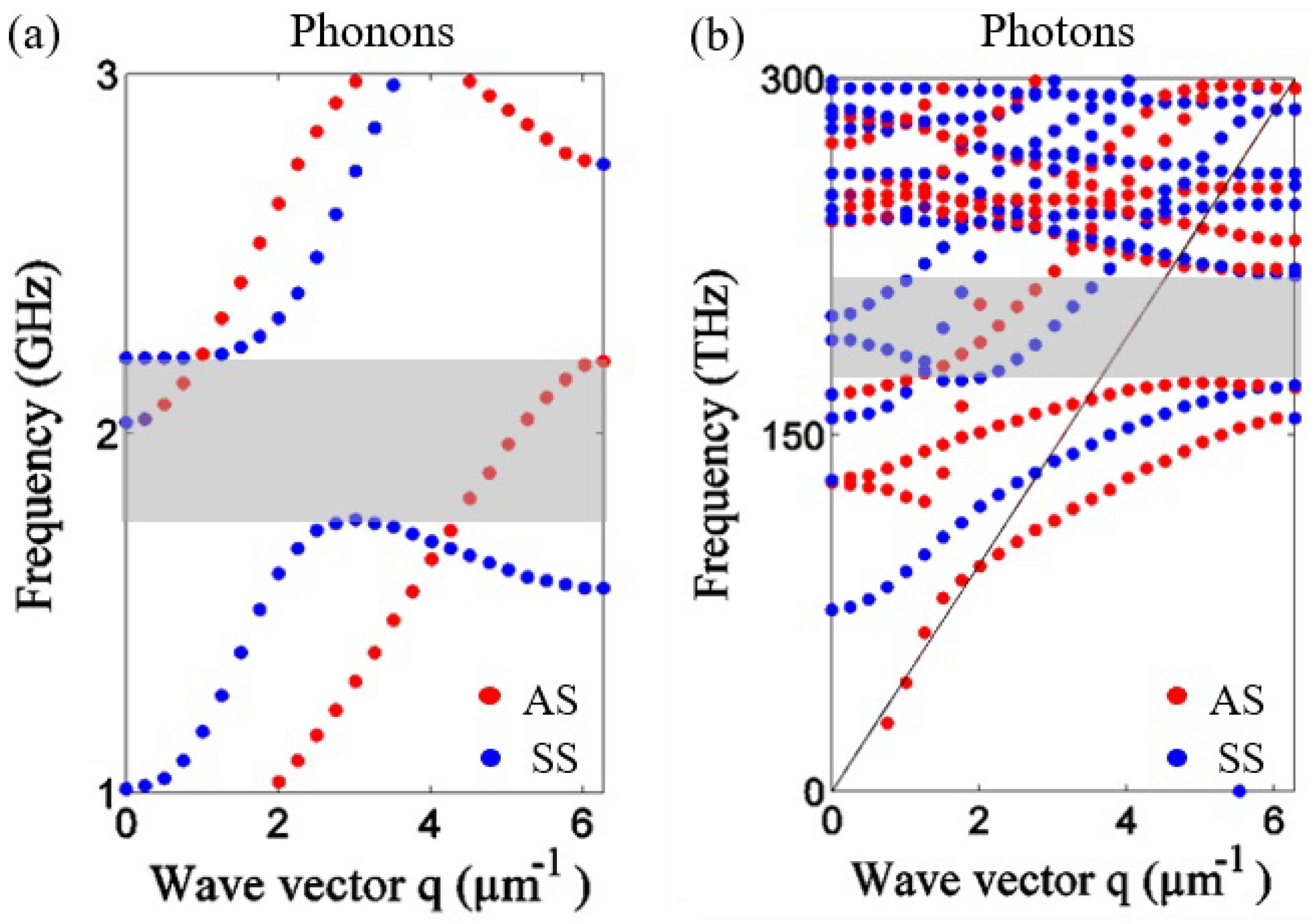
2.1. Case of Study 1: Cavity with Fixed Heights of the Stubs
2.2. Case of Study 2: Phoxonic Cavity with Parabolic Variations of the Stub Heights
3. Discussion
4. Materials and Methods
- -
- spatial mesh: changing any geometrical parameter means changing the mesh, then the numerical impact of changing the mesh must be minimized;
- -
- radiating boundaries: even a small reflection from the radiating boundary could strongly impact the final results due the high Q of the cavity, so PML thickness and distance can become critical parameters. In order to overcome this issue, several tests have been made varying PML thickness and position, until consistent and repeatable results were obtained;
- -
- e.m. excitation waveports: port size could be too small to correctly capture the slab guided mode, but ports cannot be made too large otherwise (i) they overlap when reducing D and (ii) they indesirably intercept radiated field. In general a good compromize for the port size is needed, and, anyway, other approaches are possible: the computational domain can be extended to allow proper calibration of the electromagnetic field excitation coming from the waveports.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| OM | Opto-mechanical |
| AO | Acousto-Optic |
| PE | Photo-elastic |
| e.m. | electromagnetic |
| MI | Moving Interface |
| SS | Symmetric–Symmetric |
References
- Aspelmeyer, M.; Kippenberg, T.; Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 2014, 86, 1391–1452. [Google Scholar] [CrossRef]
- Kippenberg, T.; Vahala, K. Cavity optomechanics: Back-action at the mesoscale. Science 2008, 321, 1172–1176. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Favero, I.; Karrai, K. Optomechanics of deformable optical cavities. Nat. Photonics 2009, 3, 201–205. [Google Scholar] [CrossRef]
- Eichenfield, M.; Chan, J.; Camacho, R.; Vahala, K.; Painter, O. Optomechanical crystals. Nature 2009, 462, 78–82. [Google Scholar] [CrossRef] [PubMed]
- Gavartin, E.; Braive, R.; Sagnes, I.; Arcizet, O.; Beveratos, A.; Kippenberg, T.; Robert-Philip, I. Optomechanical coupling in a two-dimensional photonic crystal defect cavity. Phys. Rev. Lett. 2011, 106, 203902. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fuhrmann, D.; Thon, S.; Kim, H.; Bouwmeester, D.; Petroff, P.; Wixforth, A.; Krenner, H. Dynamic modulation of photonic crystal nanocavities using gigahertz acoustic phonons. Nat. Photonics 2011, 5, 605–609. [Google Scholar] [CrossRef]
- Chan, J.; Safavi-Naeini, A.; Hill, J.; Meenehan, S.; Painter, O. Optimized optomechanical crystal cavity with acoustic radiation shield. Appl. Phys. Lett. 2012, 101, 081115. [Google Scholar] [CrossRef] [Green Version]
- Rolland, Q.; Oudich, M.; El-Jallal, S.; Dupont, S.; Pennec, Y.; Gazalet, J.; Kastelik, J.; Lévêque, G.; Djafari-Rouhani, B. Acousto-optic couplings in two-dimensional phoxonic crystal cavities. Appl. Phys. Lett. 2012, 101, 061109. [Google Scholar] [CrossRef]
- Djafari-Rouhani, B.; El-Jallal, S.; Pennec, Y. Phoxonic crystals and cavity optomechanics [Cristaux phoxoniques et optomécaniques dans les cavités]. C. R. Phys. 2016, 17, 555–564. [Google Scholar] [CrossRef] [Green Version]
- Oudich, M.; El-Jallal, S.; Pennec, Y.; Djafari-Rouhani, B.; Gomis-Bresco, J.; Navarro-Urrios, D.; Sotomayor Torres, C.M.; Martínez, A.; Makhoute, A. Optomechanic interaction in a corrugated phoxonic nanobeam cavity. Phys. Rev. B 2014, 89, 245122. [Google Scholar] [CrossRef] [Green Version]
- Gomis-Bresco, J.; Navarro-Urrios, D.; Oudich, M.; El-Jallal, S.; Griol, A.; Puerto, D.; Chavez, E.; Pennec, Y.; Djafari-Rouhani, B.; Alzina, F.; et al. A one-dimensional optomechanical crystal with a complete phononic band gap. Nat. Commun. 2014, 5, 4452. [Google Scholar] [CrossRef] [PubMed]
- Maire, J.; Arregui, G.; Capuj, N.; Colombano, M.; Griol, A.; Martinez, A.; Sotomayor-Torres, C.; Navarro-Urrios, D. Optical modulation of coherent phonon emission in optomechanical cavities. APL Photonics 2018, 3, 126102. [Google Scholar] [CrossRef] [Green Version]
- Mohammade, S.; Eftekhar, A.; Khelif, A.; Adibi, A. Simultaneous two-dimensional phononic and photonic band gaps in opto-mechanical crystal slabs. Opt. Express 2010, 18, 9164–9172. [Google Scholar] [CrossRef] [PubMed]
- Pennec, Y.; Djafari Rouhani, B.; El Boudouti, E.; Li, C.; El Hassouani, Y.; Vasseur, J.; Papanikolaou, N.; Benchabane, S.; Laude, V.; Martinez, A. Simultaneous existence of phononic and photonic band gaps in periodic crystal slabs. Opt. Express 2010, 18, 14301–14310. [Google Scholar] [CrossRef]
- Maldovan, M. Sound and heat revolutions in phononics. Nature 2013, 503, 209–217. [Google Scholar] [CrossRef]
- Arcizet, O.; Cohadon, P.F.; Briant, T.; Pinard, M.; Heidmann, A. Radiation-pressure cooling and optomechanical instability of a micromirror. Nature 2006, 444, 71–74. [Google Scholar] [CrossRef] [Green Version]
- Gigan, S.; Böhm, H.; Paternostro, M.; Blaser, F.; Langer, G.; Hertzberg, J.; Schwab, K.; Bäuerle, D.; Aspelmeyer, M.; Zeilinger, A. Self-cooling of a micromirror by radiation pressure. Nature 2006, 444, 67–70. [Google Scholar] [CrossRef] [Green Version]
- Aspelmeyer, M.; Meystre, P.; Schwab, K. Quantum optomechanics. Phys. Today 2012, 65, 29–35. [Google Scholar] [CrossRef]
- Balram, K.; Davanço, M.; Song, J.; Srinivasan, K. Coherent coupling between radiofrequency, optical and acoustic waves in piezo-optomechanical circuits. Nat. Photonics 2016, 10, 346–352. [Google Scholar] [CrossRef] [Green Version]
- Vainsencher, A.; Satzinger, K.; Peairs, G.; Cleland, A. Bi-directional conversion between microwave and optical frequencies in a piezoelectric optomechanical device. Appl. Phys. Lett. 2016, 109, 033107. [Google Scholar] [CrossRef] [Green Version]
- Balram, K.; Davanço, M.; Ilic, B.; Kyhm, J.H.; Song, J.; Srinivasan, K. Acousto-Optic Modulation and Optoacoustic Gating in Piezo-Optomechanical Circuits. Phys. Rev. Appl. 2017, 7, 024008. [Google Scholar] [CrossRef] [PubMed]
- Munk, D.; Katzman, M.; Hen, M.; Priel, M.; Feldberg, M.; Sharabani, T.; Levy, S.; Bergman, A.; Zadok, A. Surface acoustic wave photonic devices in silicon on insulator. Nat. Commun. 2019, 10, 4214. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Korovin, A.; Pennec, Y.; Stocchi, M.; Mencarelli, D.; Pierantoni, L.; Makkonen, T.; Ahopelto, J.; Djafari Rouhani, B. Conversion between surface acoustic waves and guided modes of a quasi-periodic structured nanobeam. J. Phys. D Appl. Phys. 2019, 52, 32. [Google Scholar] [CrossRef]
- Forsch, M.; Stockill, R.; Wallucks, A.; Marinković, I.; Gärtner, C.; Norte, R.; van Otten, F.; Fiore, A.; Srinivasan, K.; Gröblacher, S. Microwave-to-optics conversion using a mechanical oscillator in its quantum ground state. Nat. Phys. 2020, 16, 69–74. [Google Scholar] [CrossRef]
- Yu, W.; Jiang, W.; Lin, Q.; Lu, T. Cavity optomechanical spring sensing of single molecules. Nat. Commun. 2016, 7, 12311. [Google Scholar] [CrossRef]
- Delić, U.; Reisenbauer, M.; Dare, K.; Grass, D.; Vuletić, V.; Kiesel, N.; Aspelmeyer, M. Cooling of a levitated nanoparticle to the motional quantum ground state. Science 2020, 367, 892–895. [Google Scholar] [CrossRef] [Green Version]
- Djorwe, P.; Pennec, Y.; Djafari-Rouhani, B. Exceptional Point Enhances Sensitivity of Optomechanical Mass Sensors. Phys. Rev. Appl. 2019, 12, 024002. [Google Scholar] [CrossRef] [Green Version]
- Fang, K.; Matheny, M.; Luan, X.; Painter, O. Optical transduction and routing of microwave phonons in cavity-optomechanical circuits. Nat. Photonics 2016, 10, 489–496. [Google Scholar] [CrossRef]
- Colombano, M.F.; Arregui, G.; Capuj, N.E.; Pitanti, A.; Maire, J.; Griol, A.; Garrido, B.; Martinez, A.; Sotomayor-Torres, C.M.; Navarro-Urrios, D. Synchronization of Optomechanical Nanobeams by Mechanical Interaction. Phys. Rev. Lett. 2019, 123, 017402. [Google Scholar] [CrossRef] [Green Version]
- Gil-Santos, E.; Labousse, M.; Baker, C.; Goetschy, A.; Hease, W.; Gomez, C.; Lemaître, A.; Leo, G.; Ciuti, C.; Favero, I. Light-Mediated Cascaded Locking of Multiple Nano-Optomechanical Oscillators. Phys. Rev. Lett. 2017, 118, 063605. [Google Scholar] [CrossRef] [Green Version]
- Stockill, R.; Forsch, M.; Beaudoin, G.; Pantzas, K.; Sagnes, I.; Braive, R.; Gröblacher, S. Gallium Phosphide as a Piezoelectric Platform for Quantum Optomechanics. Phys. Rev. Lett. 2019, 123, 163602. [Google Scholar] [CrossRef] [Green Version]
- Stocchi, M.; Mencarelli, D.; Pennec, Y.; Djafari-Rouhani, B.; Pierantoni, L. Rigorous simulation of nonlinear optomechanical coupling in micro-and nano-structured resonant cavities. Int. J. Optomechatron. 2018, 12, 11–19. [Google Scholar] [CrossRef] [Green Version]
- Mencarelli, D.; Stocchi, M.; Pierantoni, L. Electromagnetic amplification of microwave phonons in nonlinear resonant microcavities. IEEE Trans. Microw. Theory Tech. 2018, 66, 3603–3610. [Google Scholar] [CrossRef]
- Ghadimi, A.H.; Fedorov, S.A.; Engelsen, N.J.; Bereyhi, M.J.; Schilling, R.; Wilson, D.J.; Kippenberg, T.J. Elastic strain engineering for ultralow mechanical dissipation. Science 2018, 360, 764–768. [Google Scholar] [CrossRef] [PubMed] [Green Version]
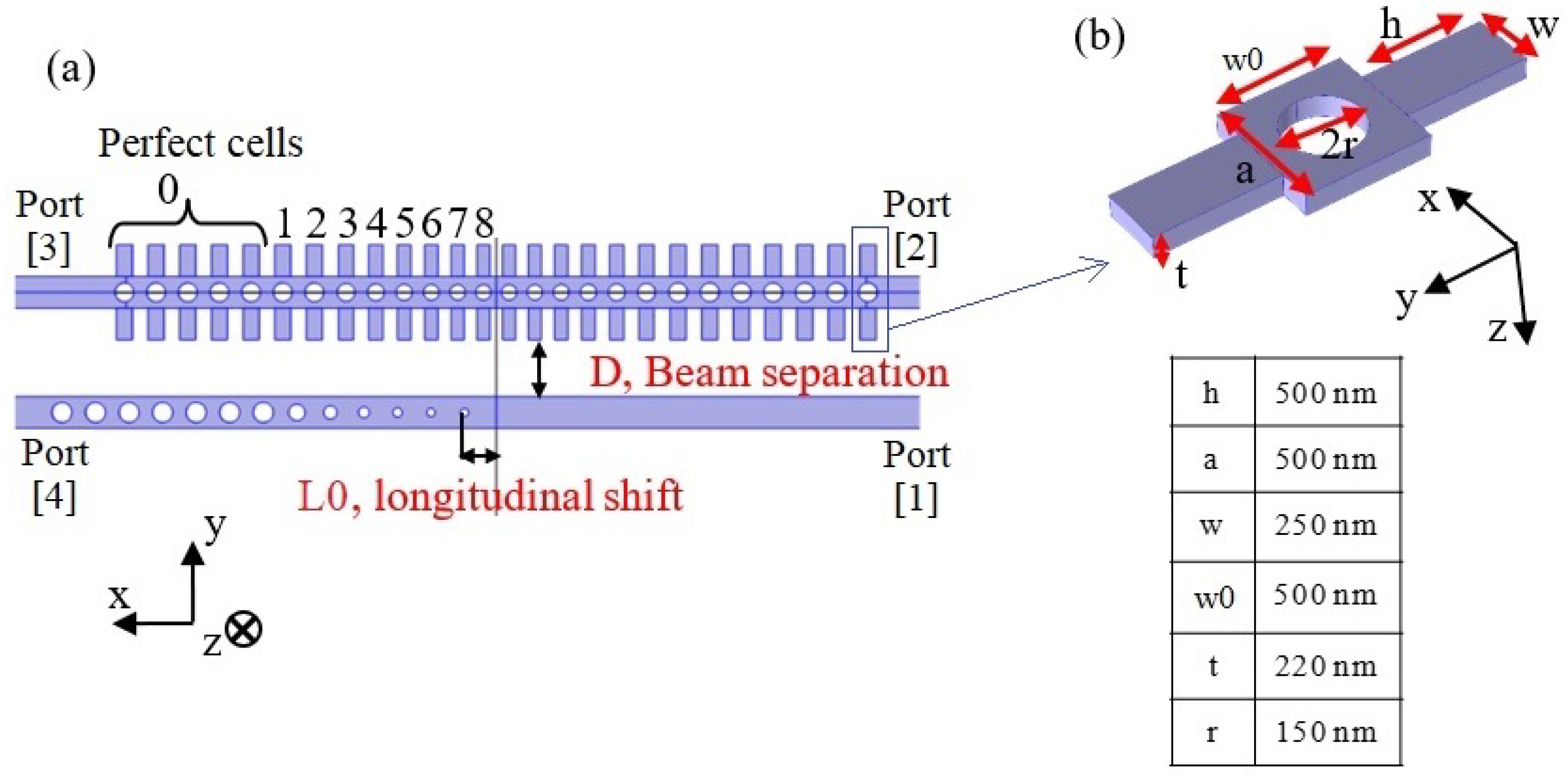
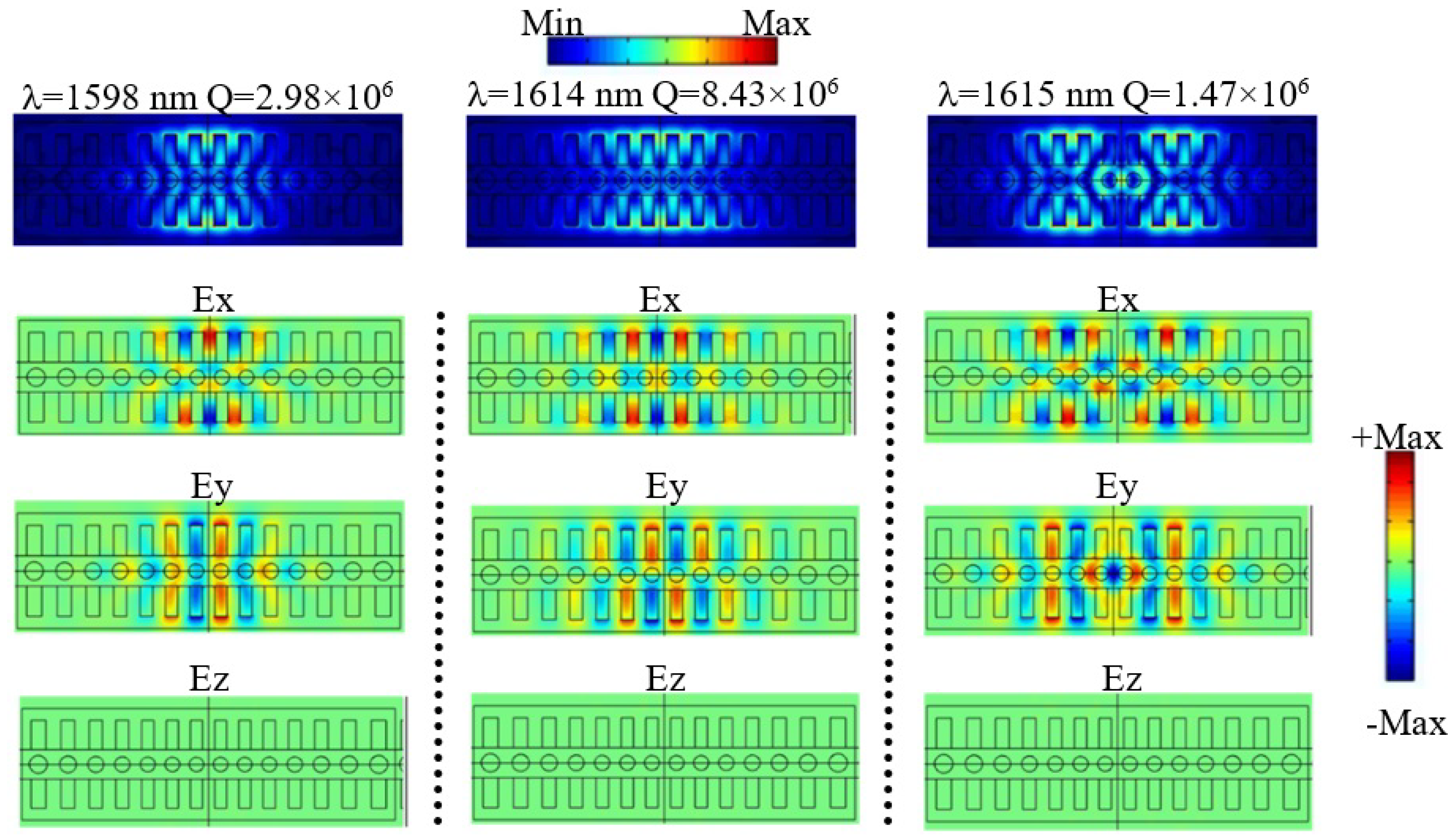

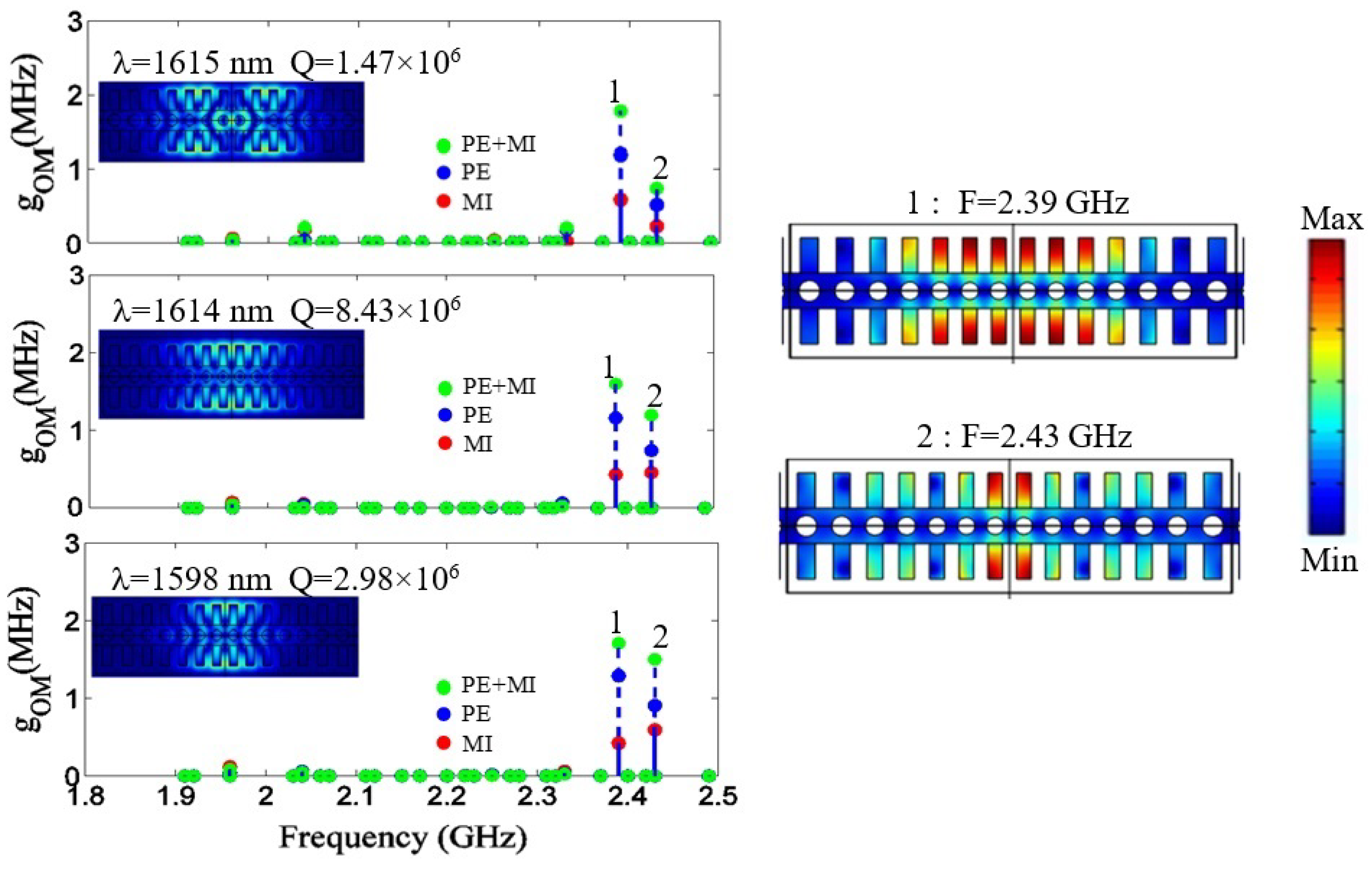
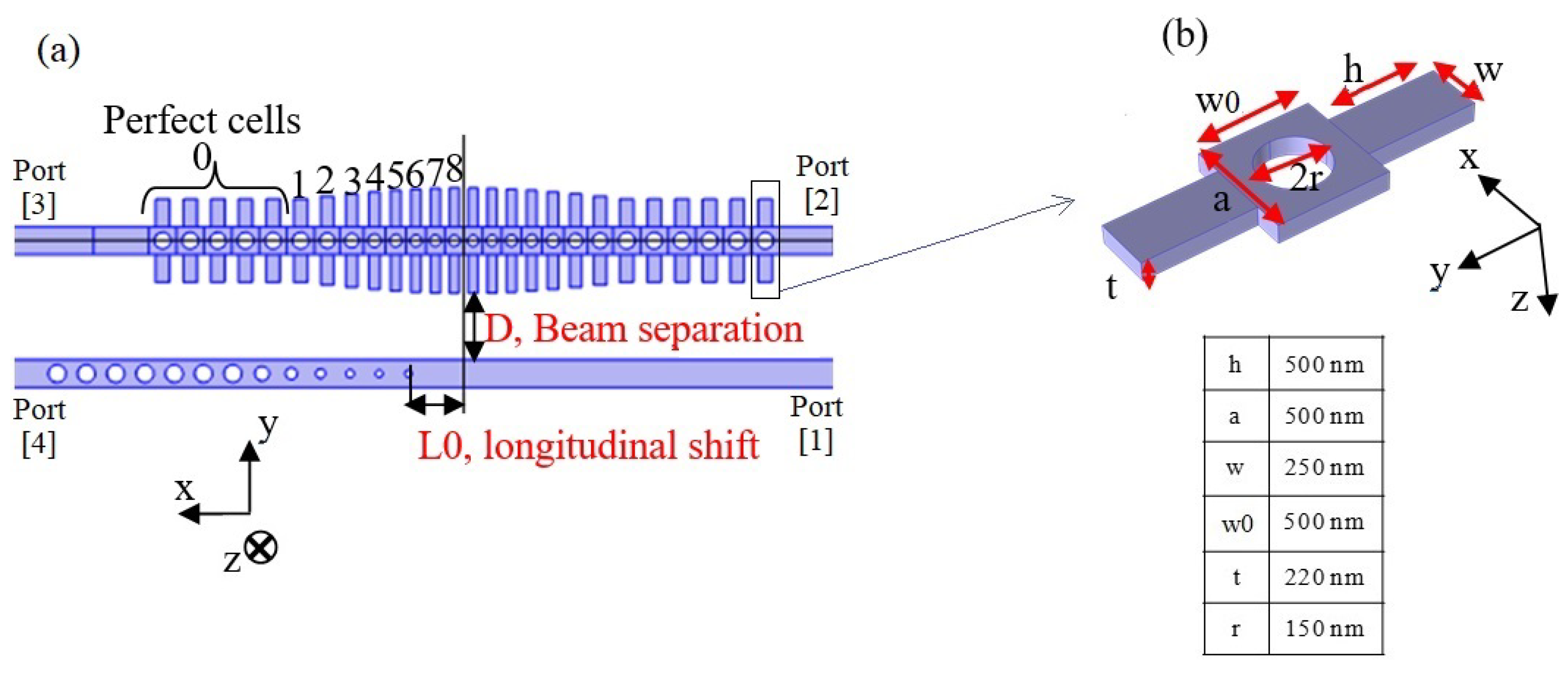


| N Cell | (nm) | (nm) | (nm) |
|---|---|---|---|
| 0 | 500 | 250 | 150 |
| 1 | 500 | 250 | 150 |
| 2 | 500 | 250 | 150 |
| 3 | 470.97 | 235.48 | 141.29 |
| 4 | 447.22 | 223.61 | 134.165 |
| 5 | 428.75 | 214.375 | 128.63 |
| 6 | 415.54 | 207.77 | 124.67 |
| 7 | 407.64 | 203.82 | 122.3 |
| 8 | 405 | 202.5 | 121.5 |
| N Cell | (nm) | (nm) | (nm) | (nm) |
|---|---|---|---|---|
| 0 | 500 | 500 | 250 | 150 |
| 1 | 500.08 | 500.17 | 250.085 | 150.51 |
| 2 | 550.06 | 460.13 | 230.065 | 138.04 |
| 3 | 592.9 | 425.81 | 212.905 | 127.74 |
| 4 | 628.6 | 397.21 | 198.605 | 119.16 |
| 5 | 657.16 | 374.33 | 187.165 | 112.29 |
| 6 | 678.58 | 357.27 | 178.64 | 107.18 |
| 7 | 692.9 | 347.735 | 172.9 | 103.72 |
| 8 | 700 | 340 | 170 | 102 |
| C11 (GPa) | C12 (GPa) | C44 (GPa) | (kg/m) | P11 | P12 | P44 | n |
|---|---|---|---|---|---|---|---|
| 165.7 | 63.9 | 79.9 | 2330 | −0.1 | 0.01 | −0.051 | 3.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gueddida, A.; Djafari Rouhani, B.; Pennec, Y.; Di Donato, A.; Pierantoni, L.; Korovin, A.; Mencarelli, D. Coupling of Integrated Waveguide and Optomechanic Cavity for Microwave Phonon Excitation in Si Nanobeams. Photonics 2020, 7, 67. https://doi.org/10.3390/photonics7030067
Gueddida A, Djafari Rouhani B, Pennec Y, Di Donato A, Pierantoni L, Korovin A, Mencarelli D. Coupling of Integrated Waveguide and Optomechanic Cavity for Microwave Phonon Excitation in Si Nanobeams. Photonics. 2020; 7(3):67. https://doi.org/10.3390/photonics7030067
Chicago/Turabian StyleGueddida, Abdellatif, Bahram Djafari Rouhani, Yan Pennec, Andrea Di Donato, Luca Pierantoni, Alexander Korovin, and Davide Mencarelli. 2020. "Coupling of Integrated Waveguide and Optomechanic Cavity for Microwave Phonon Excitation in Si Nanobeams" Photonics 7, no. 3: 67. https://doi.org/10.3390/photonics7030067
APA StyleGueddida, A., Djafari Rouhani, B., Pennec, Y., Di Donato, A., Pierantoni, L., Korovin, A., & Mencarelli, D. (2020). Coupling of Integrated Waveguide and Optomechanic Cavity for Microwave Phonon Excitation in Si Nanobeams. Photonics, 7(3), 67. https://doi.org/10.3390/photonics7030067







