Transient EUV Reflectivity Measurements of Carbon upon Ultrafast Laser Heating
Abstract
:1. Introduction
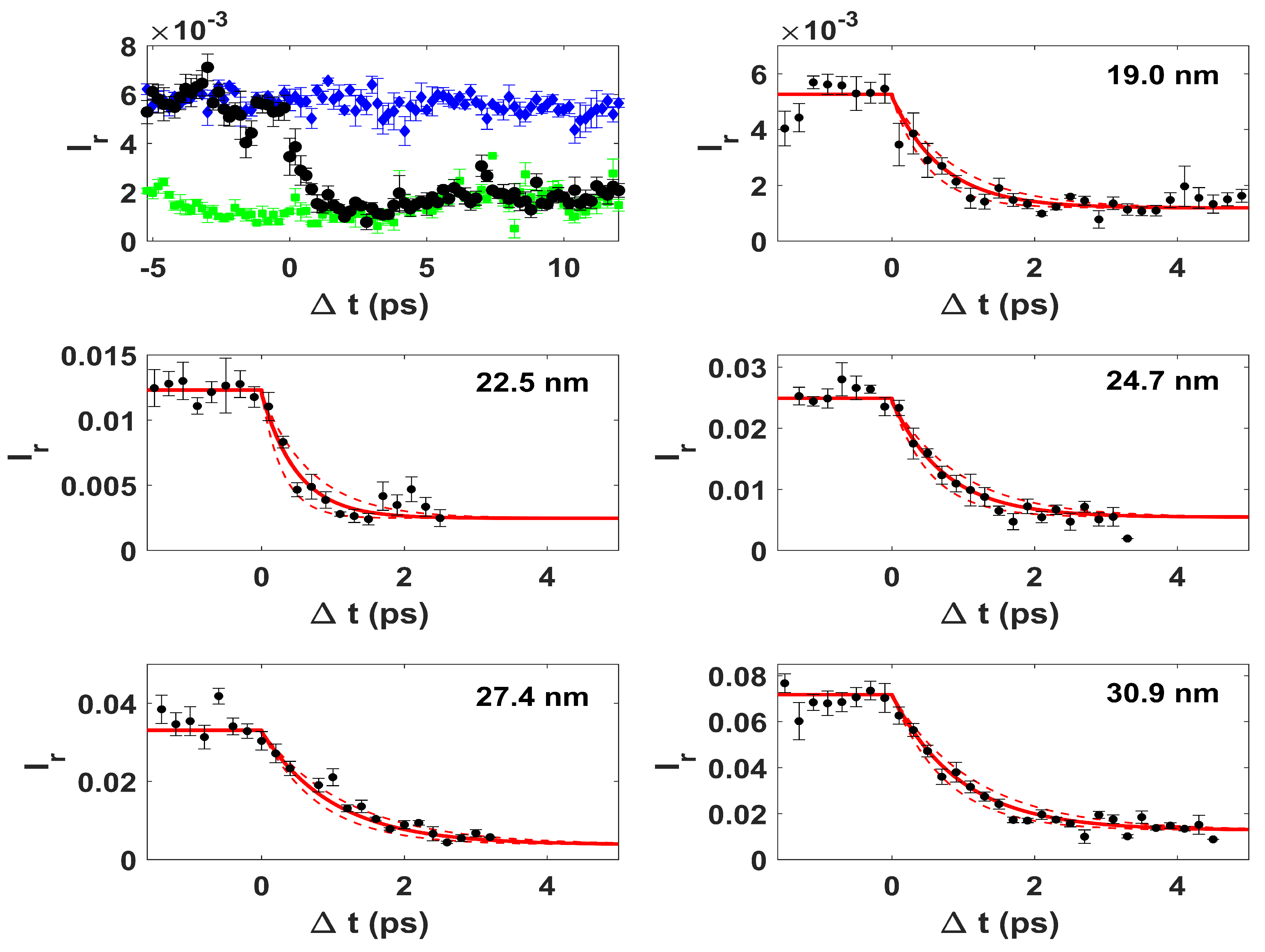
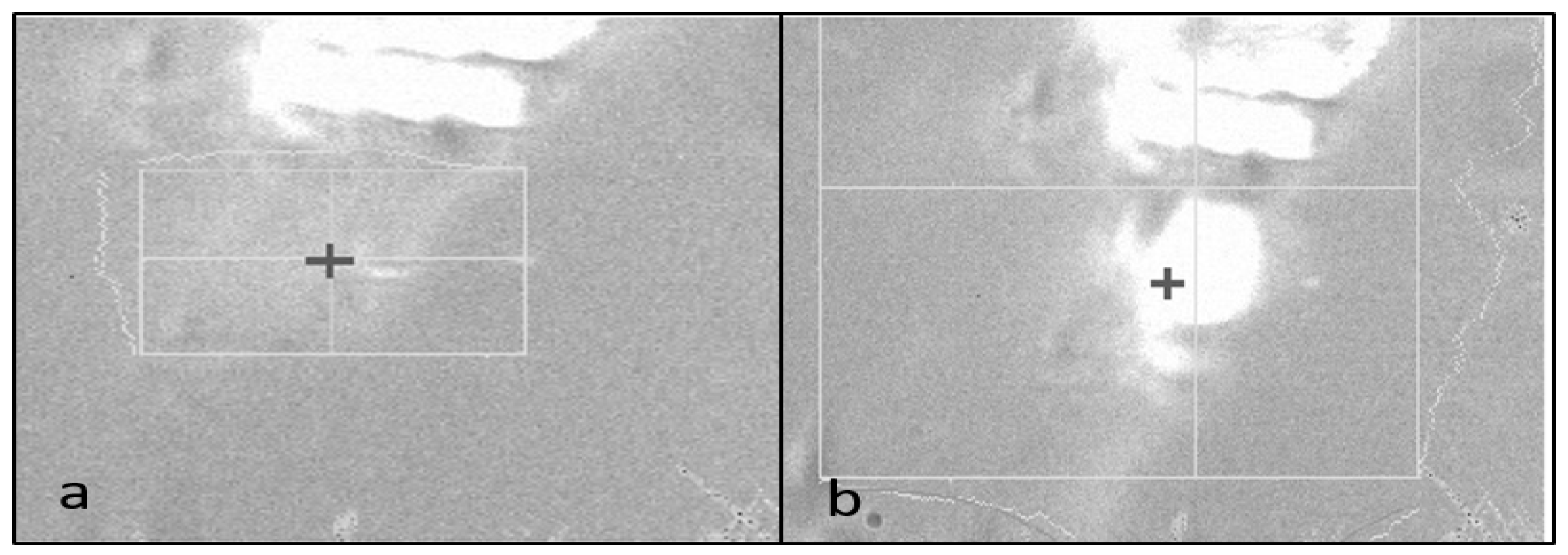
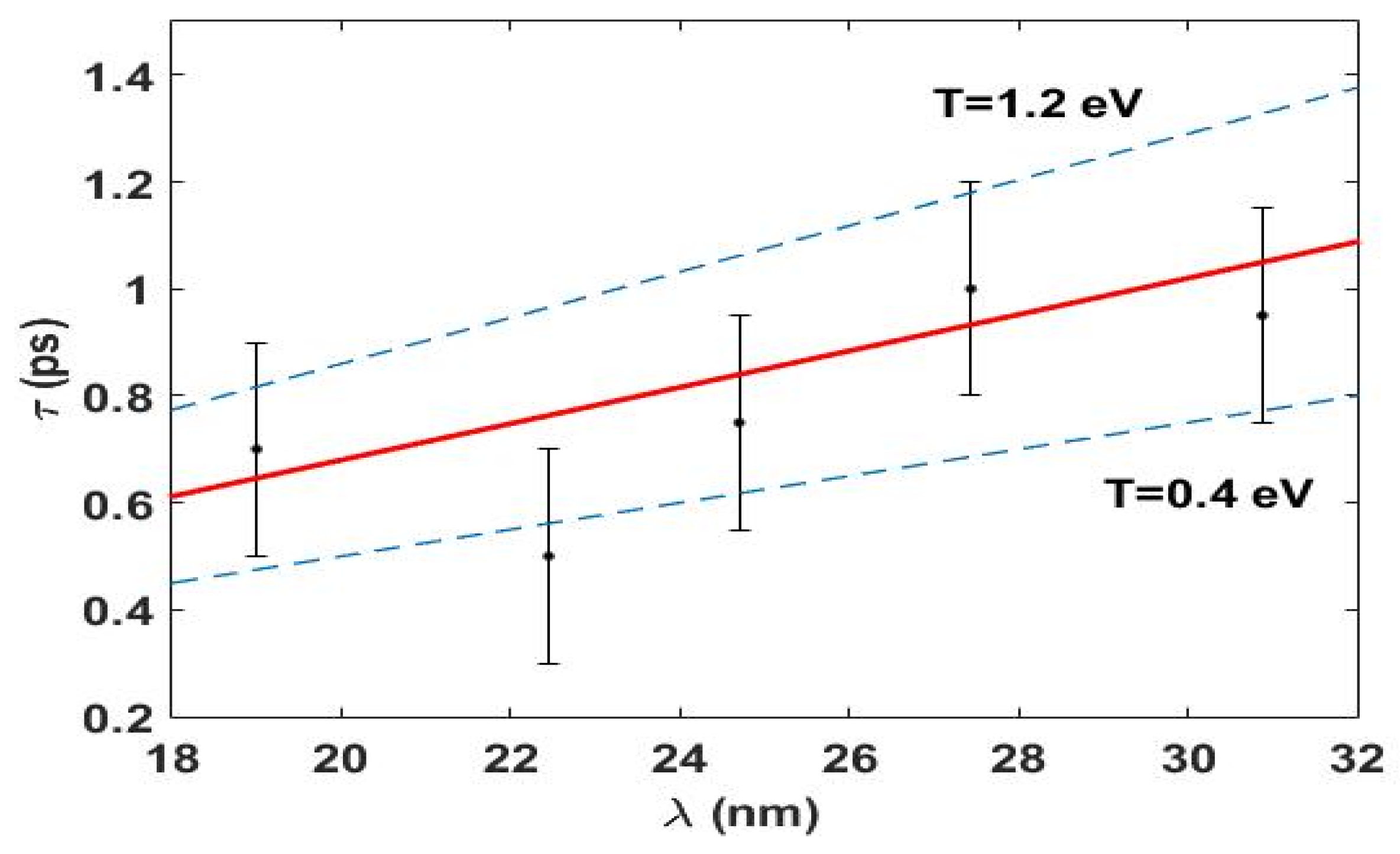
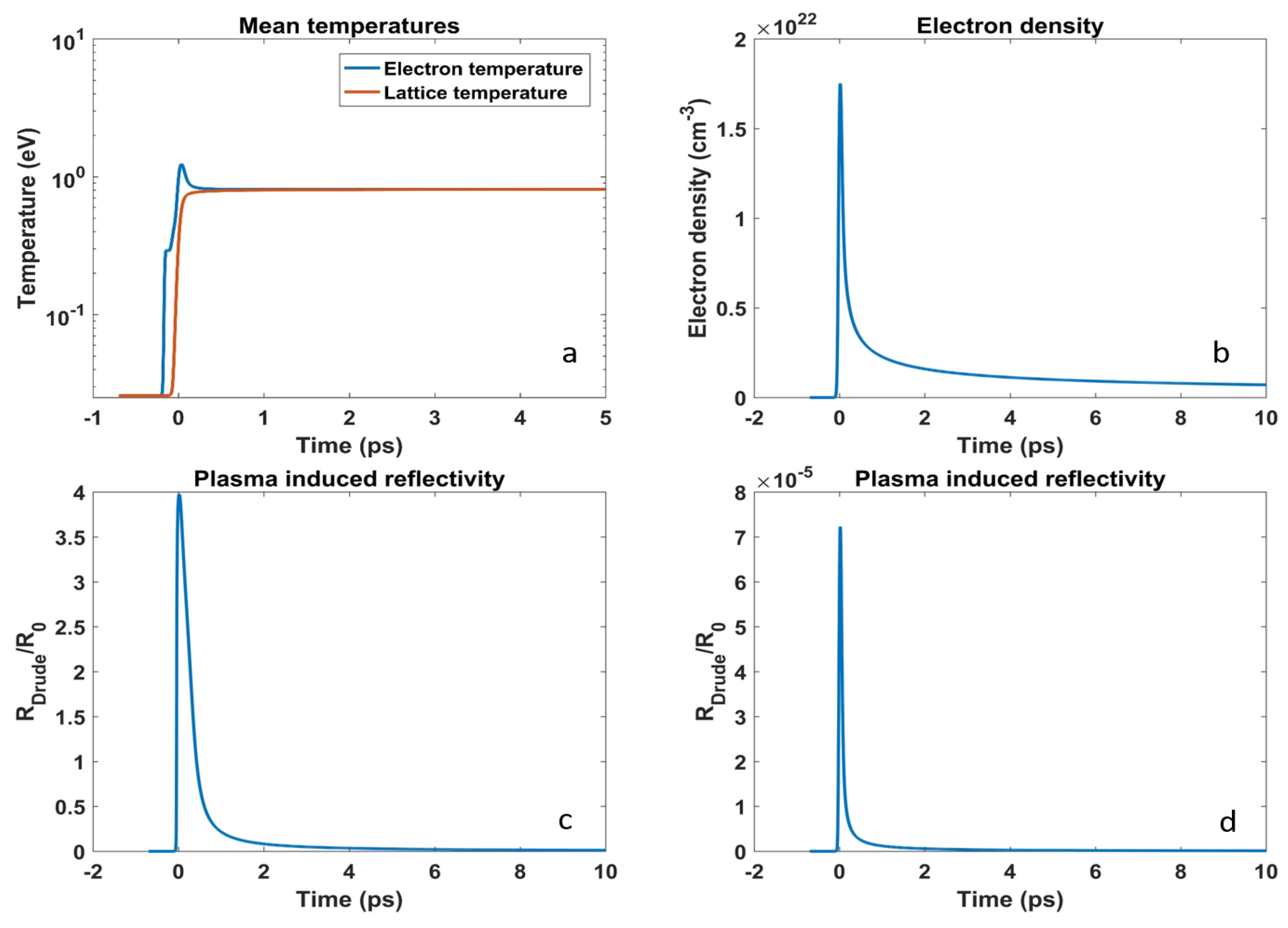
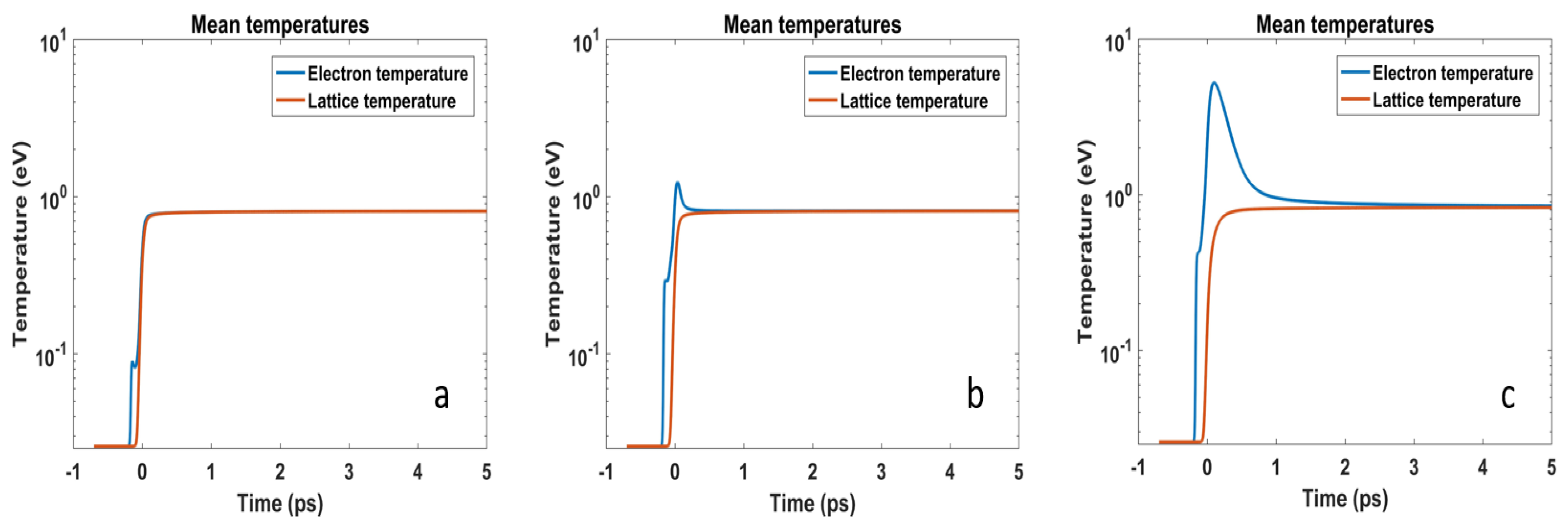
2. Results
3. Discussion
4. Materials and Methods
- (i)
- three EUV reflectivity values well before the arrival of the pump (un-pumped reflectivity);
- (ii)
- one value for the pumped reflected intensity (main pump–probe sequence);
- (iii)
- one value 100 ms after the arrival of the pump (post-expansion reflectivity).
5. Conclusions
Author Contributions
Conflicts of Interest
Abbreviations
| FEL | free electron laser |
| EUV | Extreme Ultra Violet |
| a-C | amorphous carbon |
| FWHM | full-width-half-maximum |
| EP | electron phonon coupling |
References
- Mariager, S.O.; Pressacco, F.; Ingold, G.; Caviezel, A.; Möhr-Vorobeva, E.; Beaud, P.; Johnson, S.L.; Milne, C.J.; Mancini, E.; Moyerman, S.; et al. Structural and magnetic dynamics of a laser induced phase transition in FeRh. Phys. Rev. Lett. 2012, 108, 1–5. [Google Scholar] [CrossRef] [PubMed]
- Wang, N.; Rokhlin, S.I.; Farson, D.F. Ultrafast laser melting of Au nanoparticles: Atomistic simulations. J. Nanoparticle Res. 2011, 13, 4491–4509. [Google Scholar] [CrossRef]
- Koenig, M.; Benuzzi-Mounaix, A.; Ravasio, A.; Vinci, T.; Ozaki, N.; Lepape, S.; Batani, D.; Huser, G.; Hall, T.; Hicks, D.; et al. Progress in the study of warm dense matter. Plasma Phys. Control. Fusion 2005, 47, B441–B449. [Google Scholar] [CrossRef]
- Vinko, S.M.; Ciricosta, O.; Cho, B.I.; Engelhorn, K.; Chung, H.K.; Brown, C.R.D.; Burian, T.; Chalupský, J.; Falcone, R.W.; Graves, C.; et al. Creation and diagnosis of a solid-density plasma with an X-ray free-electron laser. Nature 2012, 482, 59–62. [Google Scholar] [PubMed]
- Savvatimskiy, A.I. Measurements of the melting point of graphite and the properties of liquid carbon (A review for 1963–2003). Carbon 2005, 43, 1115–1142. [Google Scholar] [CrossRef]
- Togaya, M. Pressure Dependences of the Melting Temperature of Graphite and the Electrical Resistivity of Liquid Carbon. Phys. Rev. Lett. 1997, 79, 4–7. [Google Scholar] [CrossRef]
- Galli, G.; Martin, R.M.; Car, R.; Parrinello, M. Ab initio calculation of properties of carbon in the amorphous and liquid states. Phys. Rev. B 1990, 42, 7470–7482. [Google Scholar] [CrossRef]
- Errington, J.; Debenedetti, P.G. Relationship between structural order and the anomalies of liquid water. Nature 2001, 409, 318–321. [Google Scholar] [CrossRef] [PubMed]
- Mishima, O.; Stanley, H.E. The relationship between liquid, supercooled and glassy water. Nature 1998, 396, 329–335. [Google Scholar]
- Bundy, F.P. Melting of Graphite at Very High Pressure. J. Chem. Phys. 1963, 38, 618. [Google Scholar] [CrossRef]
- Reitze, D.H.; Ahn, H.; Downer, M.C. Optical properties of liquid carbon measured by femtosecond spectroscopy. Phys. Rev. B 1992, 45, 2677–2693. [Google Scholar] [CrossRef]
- Rämer, A.; Osmani, O.; Rethfeld, B. Laser damage in silicon: Energy absorption, relaxation, and transport. J. Appl. Phys. 2014, 116, 1–12. [Google Scholar] [CrossRef]
- Shin, T.; Teitelbaum, S.W.; Wolfson, J.; Kandyla, M.; Nelson, K.A. Extended two-temperature model for ultrafast thermal response of band gap materials upon impulsive optical excitation. J. Chem. Phys. 2015, 143, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Masciovecchio, C.; Battistoni, A.; Giangrisostomi, E.; Bencivenga, F.; Principi, E.; Mincigrucci, R.; Cucini, R.; Gessini, A.; D’Amico, F.; Borghes, R.; et al. EIS: The scattering beamline at FERMI. J. Synchrotron Radiat. 2015, 22, 553–564. [Google Scholar] [CrossRef] [PubMed]
- Ratner, D.; Fry, A.; Stupakov, G.; White, W. Laser phase errors in seeded free electron lasers. Phys. Rev. ST Accel. Beams 2012, 15, 030702. [Google Scholar] [CrossRef]
- Danailov, M.B.; Bencivenga, F.; Capotondi, F.; Casolari, F.; Cinquegrana, P.; Demidovich, A.; Giangrisostomi, E.; Kiskinova, M.P.; Kurdi, G.; Manfredda, M.; et al. Towards jitter-free pump–probe measurements at seeded free electron laser facilities. Opt. Express 2014, 22, 12869–12879. [Google Scholar] [CrossRef] [PubMed]
- Chalupský, J.; Hájková, V.; Altapova, V.; Burian, T.; Gleeson, A.J.; Juha, L.; Jurek, M.; Sinn, H.; Störmer, M.; Sobierajski, R.; et al. Damage of amorphous carbon induced by soft X-ray femtosecond pulses above and below the critical angle. Appl. Phys. Lett. 2009, 95, 3–6. [Google Scholar] [CrossRef]
- Allaria, E.; Appio, R.; Badano, L.; Barletta, W.; Bassanese, S.; Biedron, S.; Borga, A.; Busetto, E.; Castronovo, D.; Cinquegrana, P.; et al. Highly coherent and stable pulses from the FERMI seeded free-electron laser in the extreme ultraviolet. Nat. Photon. 2012, 6, 699–704. [Google Scholar] [CrossRef]
- Allaria, E.; Castronovo, D.; Cinquegrana, P.; Craievich, P.; Dal Forno, M.; Danailov, M.B.; D’Auria, G.; Demidovich, A.; De Ninno, G.; Di Mitri, S.; et al. Two-stage seeded soft-X-ray free-electron laser. Nat. Photon. 2013, 7, 913–918. [Google Scholar] [CrossRef]
- The Center for X-Ray Optics. Available online: http://www.cxro.lbl.gov/ (accessed on 16 March 2017).
- Tobey, R.I.; Siemens, M.E.; Cohen, O.; Murnane, M.M.; Kapteyn, H.C.; Nelson, K.A. Ultrafast extreme ultraviolet holography: Dynamic monitoring of surface deformation. Opt. Lett. 2007, 32, 286. [Google Scholar] [CrossRef] [PubMed]
- Fedosejevs, R.; Ottmann, R.; Sigel, R.; Kühnle, G.; Szatmari, S.; Schäfer, F.P. Absorption of femtosecond laser pulses in high-density plasma. Phys. Rev. Lett. 1990, 64, 1250–1253. [Google Scholar] [CrossRef] [PubMed]
- Milchberg, H.M.; Freeman, R.R. Light Absorption in Ultrashort Scale Length Plasmas. J. Opt. Soc. Am. B 1989, 6, 1351–1355. [Google Scholar] [CrossRef]
- Meitzner, J.; Moore, F.G.; Tillotson, B.M.; Kevan, S.D.; Richmond, G.L. Time-resolved measurement of free carrier absorption, diffusivity, and internal quantum efficiency in silicon. Appl. Phys. Lett. 2013, 103, 092101. [Google Scholar] [CrossRef]
- Zel’dovich, Y.B.; Raizer, Y.P. Physics of Shock Waves and High-Temperature Hydrodynamic Phenomena; Dover Publication: New York, NY, USA, 1966. [Google Scholar]
- More, R.M. Pressure ionization, resonances, and the continuity of bound and free states. Adv. At. Mol. Phys. 1985, 21, 305. [Google Scholar]
- Silva, S.R.P. Properties of Amorphous Carbon; Institution of Engineering and Technology: London, UK, 2003. [Google Scholar]
- Tandon, N.; Albrecht, J.D.; Ram-Mohan, L.R. Electron-phonon coupling and associated scattering rates in diamond. Diam. Relat. Mater. 2015, 56, 1–5. [Google Scholar] [CrossRef]
- Chen, J.; Tzou, D.; Beraun, J. A semiclassical two-temperature model for ultrafast laser heating. Int. J. Heat Mass Transf. 2006, 49, 307–316. [Google Scholar] [CrossRef]
- Baydogan, N.D. Evaluation of optical properties of the amorphous carbon film on fused silica. Mater. Sci. Eng. B 2004, 107, 70–77. [Google Scholar] [CrossRef]
- Butland, A.T.D.; Maddison, R.J. The Specific Heat of Graphite: An Evaluation of Measurements. J. Nucl. Mater. 1973, 49, 45–56. [Google Scholar]
- Shimano, R.; Nagai, M.; Horiuch, K.; Kuwata-Gonokami, M. Formation of a high Tc electron-hole liquid in diamond. Phys. Rev. Lett. 2002, 88, 574041–574044. [Google Scholar]
- Watanabe, T.; Teraji, T.; Ito, T.; Kamakura, Y.; Taniguchi, K. Monte Carlo simulations of electron transport properties of diamond in high electric fields using full band structure. J. Appl. Phys. 2004, 95, 4866–4874. [Google Scholar] [CrossRef]
- Verburg, P.C.; Römer, G.R.B.E.; Huis, A.J. Two-temperature model for pulsed-laser-induced subsurface modifications in Si. Appl. Phys. A Mater. Sci. Process. 2014, 114, 1135–1143. [Google Scholar] [CrossRef]

© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mincigrucci, R.; Principi, E.; Bencivenga, F.; Foglia, L.; Gessini, A.; Kurdi, G.; Simoncig, A.; Masciovecchio, C. Transient EUV Reflectivity Measurements of Carbon upon Ultrafast Laser Heating. Photonics 2017, 4, 23. https://doi.org/10.3390/photonics4020023
Mincigrucci R, Principi E, Bencivenga F, Foglia L, Gessini A, Kurdi G, Simoncig A, Masciovecchio C. Transient EUV Reflectivity Measurements of Carbon upon Ultrafast Laser Heating. Photonics. 2017; 4(2):23. https://doi.org/10.3390/photonics4020023
Chicago/Turabian StyleMincigrucci, Riccardo, Emiliano Principi, Filippo Bencivenga, Laura Foglia, Alessandro Gessini, Gabor Kurdi, Alberto Simoncig, and Claudio Masciovecchio. 2017. "Transient EUV Reflectivity Measurements of Carbon upon Ultrafast Laser Heating" Photonics 4, no. 2: 23. https://doi.org/10.3390/photonics4020023
APA StyleMincigrucci, R., Principi, E., Bencivenga, F., Foglia, L., Gessini, A., Kurdi, G., Simoncig, A., & Masciovecchio, C. (2017). Transient EUV Reflectivity Measurements of Carbon upon Ultrafast Laser Heating. Photonics, 4(2), 23. https://doi.org/10.3390/photonics4020023






