Theoretical Research on Large Field-of-View Polarization Imaging Based on Dynamic Vision Sensors
Abstract
1. Introduction
2. DVS-Based Polarized Imaging Methods
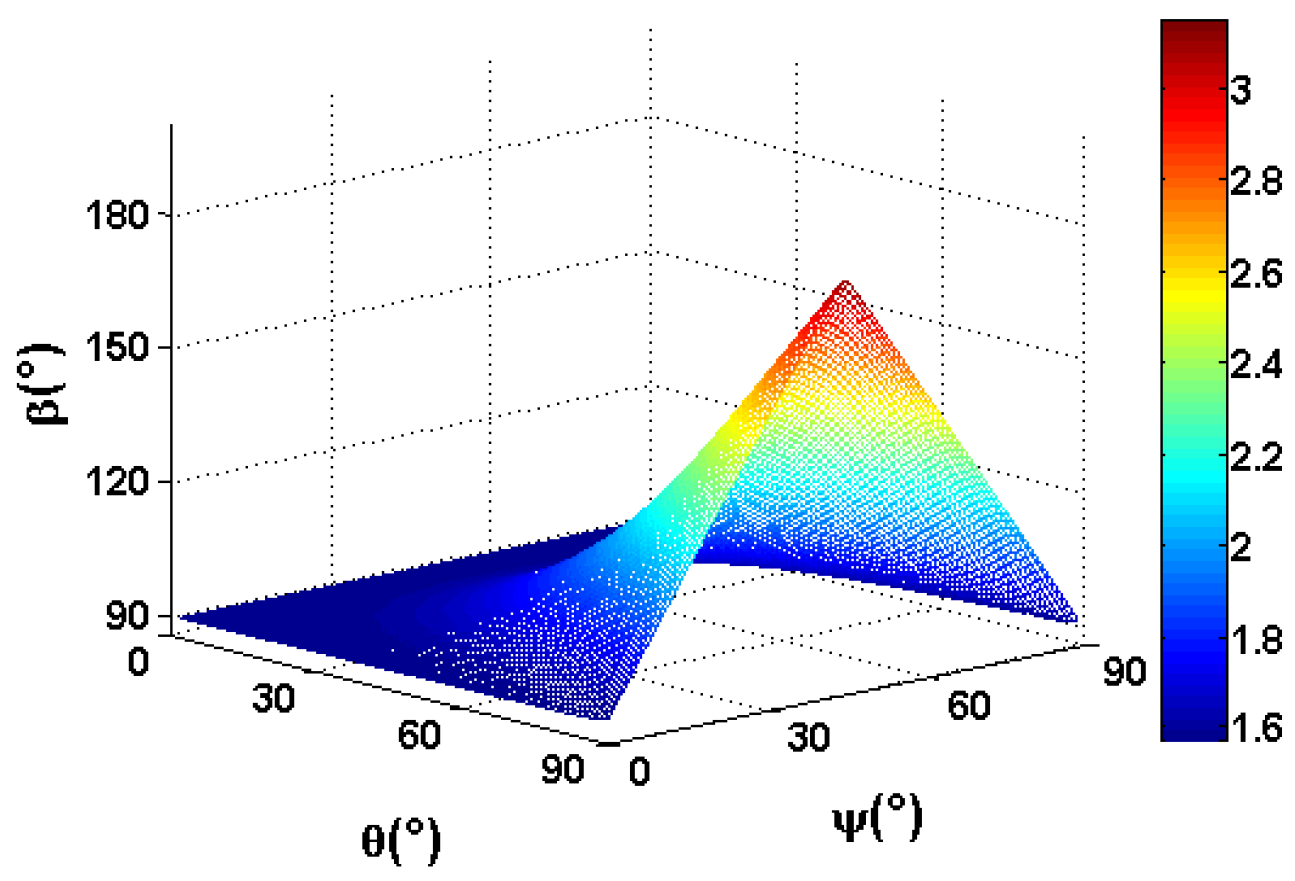
3. Polarization Imaging Theory of Large FOV
4. DVS-Based Large FOV Polarization Imaging Theory
4.1. Incidence of Natural Light
4.2. Linearly Polarized Light Incidence
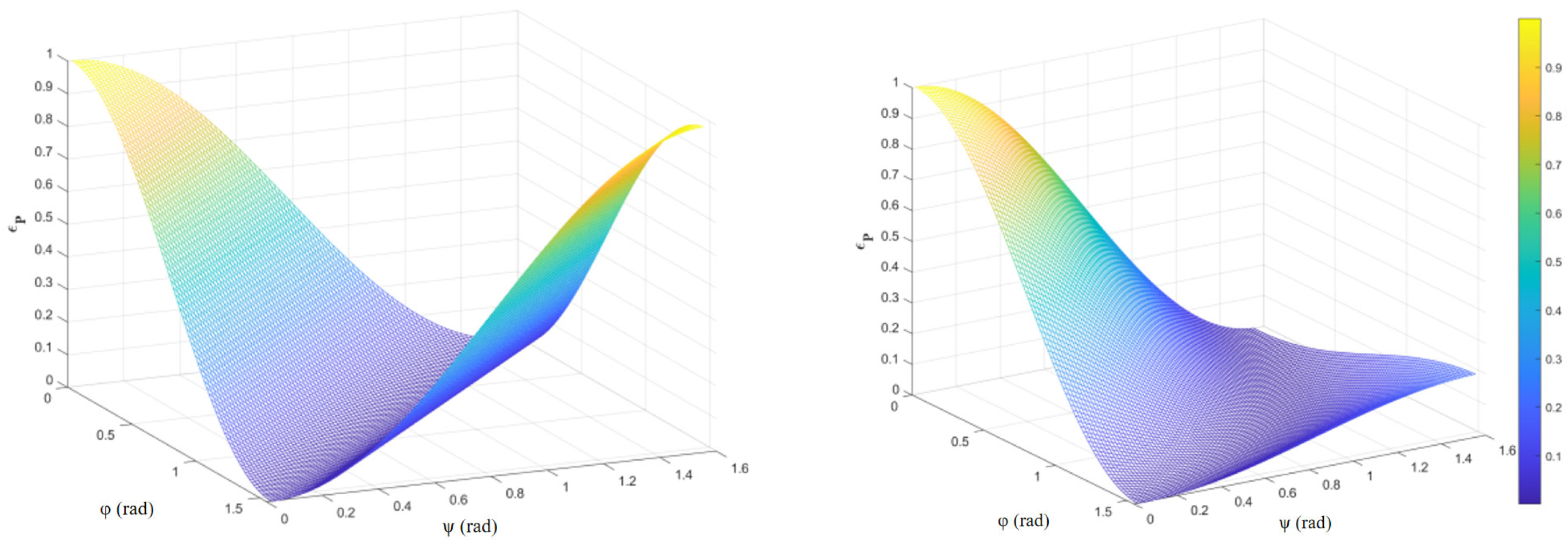
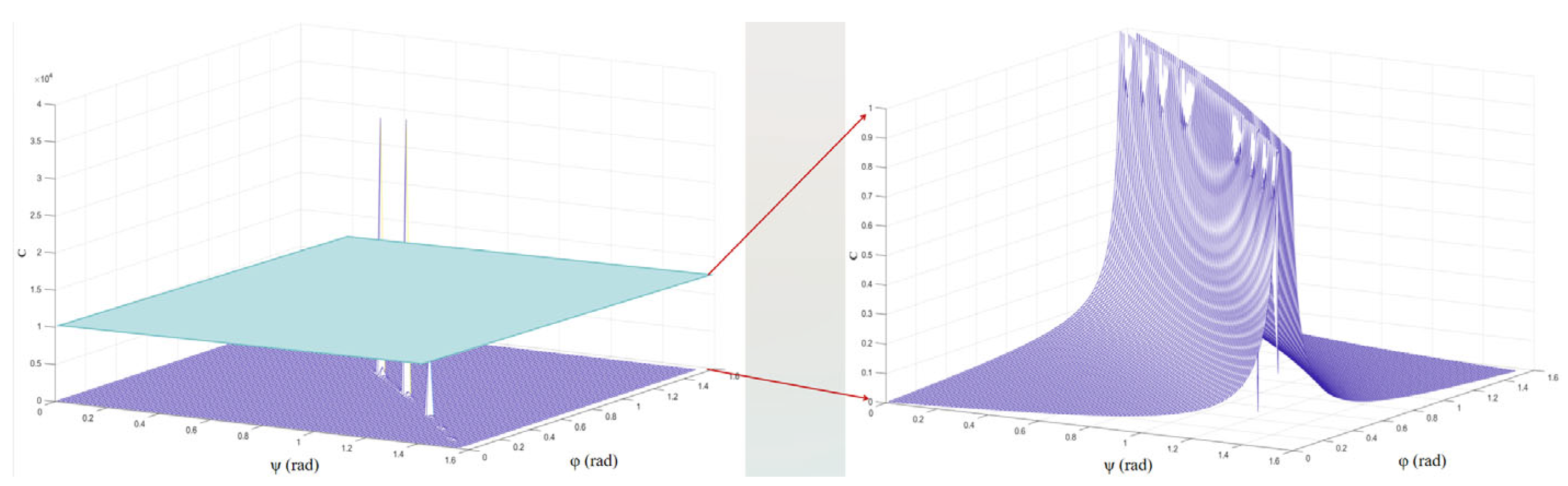
4.3. Partially Polarized Light Incidence
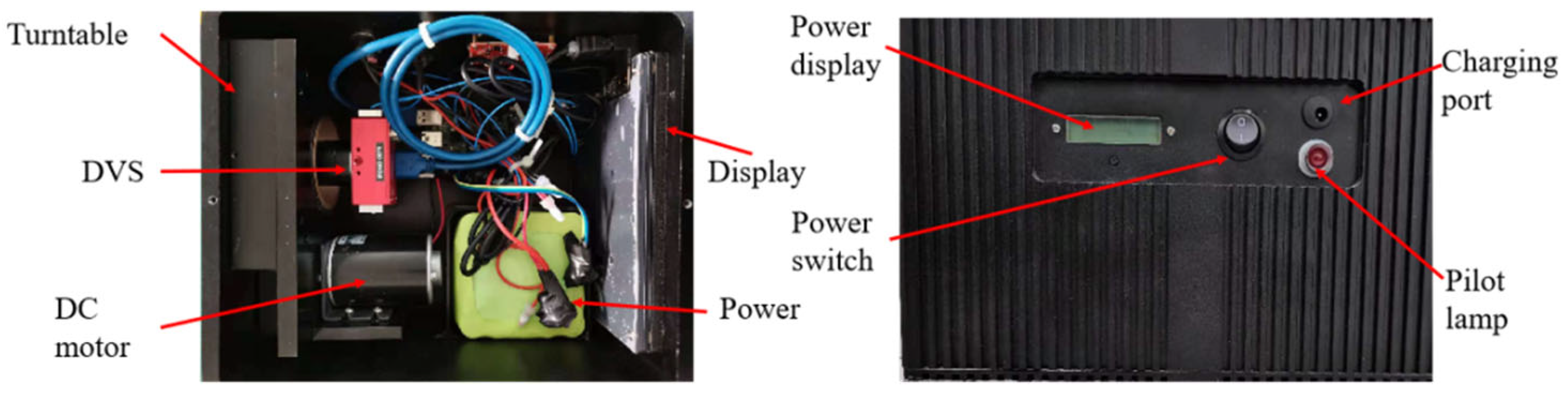
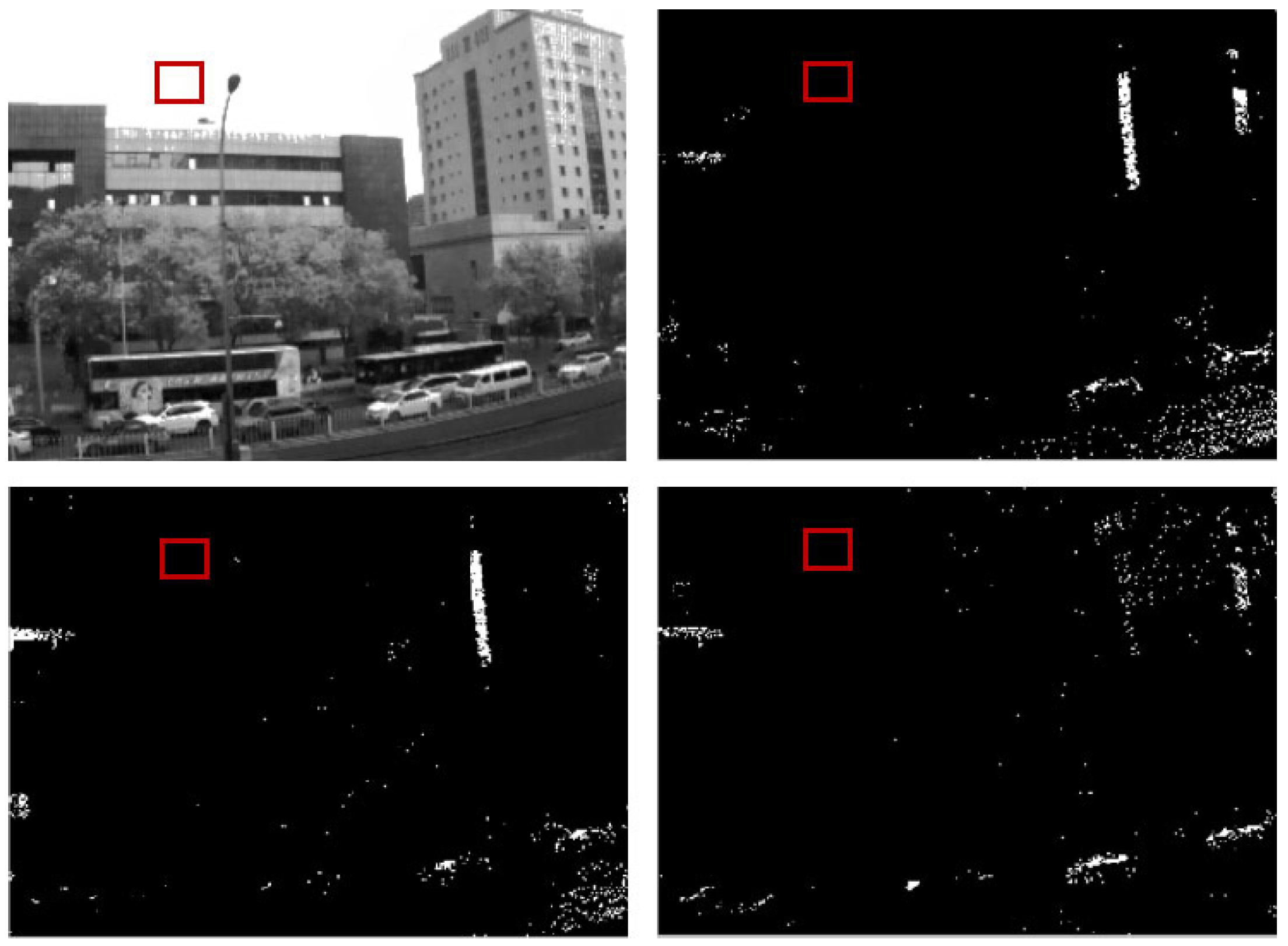
5. Experiments
5.1. Linearly Polarized Light Incidence
5.2. Incidence of Natural Light
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, G.; Cao, H.; Conradt, J.; Tang, H.; Rohrbein, F.; Knoll, A. Event-based neuromorphic vision for autonomous driving: A paradigm shift for bio-inspired visual sensing and perception. IEEE Signal Process. Mag. 2020, 37, 34–49. [Google Scholar] [CrossRef]
- Yang, Y.; Pan, C.; Li, Y.; Yangdong, X.; Wang, P.; Li, Z.-A.; Wang, S.; Yu, W.; Liu, G.; Cheng, B.; et al. In-sensor dynamic computing for intelligent machine vision. Nat. Electron. 2024, 7, 225–233. [Google Scholar] [CrossRef]
- Gallego, G.; Delbrück, T.; Orchard, G.; Bartolozzi, C.; Taba, B.; Censi, A.; Leutenegger, S.; Davison, A.J.; Conradt, J.; Daniilidis, K.; et al. Event-based vision: A survey. IEEE Trans. Pattern Anal. Mach. Intell. 2020, 44, 154–180. [Google Scholar] [CrossRef] [PubMed]
- Linares-Barranco, A.; Perez-Pena, F.; Moeys, D.P.; Gomez-Rodriguez, F.; Jimenez-Moreno, G.; Liu, S.-C.; Delbruck, T. Low Latency Event-Based Filtering and Feature Extraction for Dynamic Vision Sensors in Real-Time FPGA Applications. IEEE Access 2019, 7, 134926–134942. [Google Scholar] [CrossRef]
- Drazen, D.; Lichtsteiner, P.; Häfliger, P.; Delbrück, T.; Jensen, A. Toward real-time particle tracking using an event-based dynamic vision sensor. Exp. Fluids 2011, 51, 1465–1469. [Google Scholar] [CrossRef]
- Cai, Z.; Tan, H.; Zhao, J.; Wang, Y. Target detection and obstacle avoidance for UAV based on dynamic vision sensor. J. Appl. Artif. Intell. 2024, 1, 192–214. [Google Scholar] [CrossRef]
- Wang, C.; Wang, X.; Fang, Y.; Yan, C.; Zhang, X.; Zuo, Y. Event-Enhanced Passive Non-Line-of-Sight Imaging for Moving Objects With Physical Embedding. IEEE Sens. J. 2024, 24, 37970–37985. [Google Scholar] [CrossRef]
- Fischer, T.; Milford, M.J. Event-Based Visual Place Recognition with Ensembles of Temporal Windows. IEEE Robot. Autom. Lett. 2020, 5, 6924–6931. [Google Scholar] [CrossRef]
- Wang, Y.; Zhou, Q.; Xiong, H.; Shen, Y.; Cheng, L. A Novel Dynamic Vision Sensor Image Rain Removal Method Based on Event Density Clustering. In Proceedings of the 2024 International Conference on Networking, Sensing and Control (ICNSC), Hangzhou, China, 18–20 October 2024; pp. 1–7. [Google Scholar]
- Lenz, G.; Ieng, S.-H.; Benosman, R. Event-Based Face Detection and Tracking Using the Dynamics of Eye Blinks. Front. Neurosci. 2020, 14, 587. [Google Scholar] [CrossRef]
- Zundel, A.; Demonceaux, C.; Hueber, N.; Spittler, D.; Strub, G.; Changey, S. Bimodal vision system combining standard camera and dynamic vision sensor for detecting and tracking fast UAVs. In Proceedings of the Emerging Imaging and Sensing Technologies for Security and Defence IX, SPIE, Edinburgh, UK, 17–18 September 2024; Volume 13204, p. 1320402. [Google Scholar]
- Yang, J.; Jin, W.Q.; Qiu, S.; Xue, F.D. Correction model for visible light polarization imaging considering non-ideality of polarizers. Opt. Precis. Eng. 2020, 28, 334–339. [Google Scholar]
- Wu, Y.Z.; Liu, X.; Yao, L. Polarization characteristics of the paint plate based on single reflection. In Proceedings of the AOPC 2017: Optical Sensing and Imaging Technology and Applications, Beijing, China, 4–6 June 2017; Volume 10462, pp. 487–494. [Google Scholar]
- Chen, H.S.; Rao, C.R.N. Polarization of light on reflection by some natural surfaces. J. Phys. D Appl. Phys. 1968, 1, 1191. [Google Scholar] [CrossRef]
- Wolff, L. Polarization-based material classification from specular reflection. IEEE Trans. Pattern Anal. Mach. Intell. 1990, 12, 1059–1071. [Google Scholar] [CrossRef]
- Kliger, D.S.; Lewis, J.W.; Randall, C.E. Polarized Light in Optics and Spectroscopy; Elsevier: Amsterdam, The Netherlands, 1990. [Google Scholar]
- Andreou, A.; Kalayjian, Z. Polarization imaging: Principles and integrated polarimeters. IEEE Sens. J. 2002, 2, 566–576. [Google Scholar] [CrossRef]
- Bueno, J.M.; Berrio, E.; Ozolinsh, M.; Artal, P. Degree of polarization as an objective method of estimating scattering. J. Opt. Soc. Am. A 2004, 21, 1316–1321. [Google Scholar] [CrossRef]
- Lu, X.; Li, F.; Xiao, B.; Yang, X.; Xin, L.; Liu, Z. Rotary polarization detection imaging system based on dynamic vision sensor. Opt. Precis. Eng. 2021, 29, 2754–2762. [Google Scholar] [CrossRef]
- Lu, X.; Li, F.; Xiao, B.; Yang, X.; Xin, L.; Liu, Z. Polarization imaging detection method based on dynamic vision sensor. In Proceedings of the Seventh Symposium on Novel Photoelectronic Detection Technology and Applications, SPIE, Kunming, China, 5–7 November 2020; Volume 11763, pp. 242–251. [Google Scholar]
- Nowak, M.; Beninati, A.; Douard, N.; Puran, A.; Barnes, C.; Kerwick, A.; Giakos, G.K. Polarimetric dynamic vision sensor p(DVS) neural network architecture for motion classification. Electron. Lett. 2021, 57, 624–626. [Google Scholar] [CrossRef]
- Hawks, M.; Dexter, M. Event-based imaging polarimeter. Opt. Eng. 2022, 61, 053101. [Google Scholar] [CrossRef]
- Haessig, G.; Joubert, D.; Haque, J.; Milde, M.B.; Delbruck, T.; Gruev, V. Pdavis: Bio-inspired polarization event camera. In Proceedings of the IEEE/CVF Conference on Computer Vision and Pattern Recognition, Vancouver, BC, Canada, 18–22 June 2023; pp. 3963–3972. [Google Scholar]
- Yan, C.; Wang, X.; Zhang, X.; Wang, C. Event-based imaging polarimeter simulation with a single DoFP image. Opt. Lett. 2023, 48, 739–742. [Google Scholar] [CrossRef] [PubMed]
- Nowak, M.; Beninati, A.; Douard, N.; Giakos, G.C. Polarimetric dynamic vision sensor p(DVS) principles. IEEE Instrum. Meas. Mag. 2020, 23, 18–23. [Google Scholar] [CrossRef]
- Douard, N.; Surovich, M.; Bauman, G.; Giakos, Z.; Giakos, G. A novel cognitive neuromorphic polarimetric dynamic vision system (pDVS) with enhanced discrimination and temporal contrast. In Proceedings of the 2018 Conference on Precision Electromagnetic Measurements (CPEM 2018), Paris, France, 8–13 July 2018; pp. 1–2. [Google Scholar]
- Morel, O.; Fofi, D. Visual behavior based bio-inspired polarization techniques in computer vision and robotics. In Developing and Applying Biologically-Inspired Vision Systems: Interdisciplinary Concepts; IGI Global: Hershey, PA, USA, 2013; pp. 243–272. [Google Scholar]
- Campbell, J.B.; Wynne, R.H. Introduction to Remote Sensing; Guilford Press: New York, NY, USA, 2011. [Google Scholar]
- Elachi, C.; Zimmerman, P.D. Introduction to the physics and techniques of remote sensing. Phys. Today 1988, 41, 126. [Google Scholar] [CrossRef]
- Lu, X.; Jin, W.; Li, L.; Wang, X.; Qiu, S.; Liu, J. Theory and analysis of a large field polarization imaging system with obliquely incident light. Opt. Express 2018, 26, 2495–2508. [Google Scholar] [CrossRef] [PubMed]
- Takenaka, T.; Yokota, M.; Fukumitsu, O. Propagation of light beams beyond the paraxial approximation. J. Opt. Soc. Am. A 1985, 2, 826–829. [Google Scholar] [CrossRef]
- Setälä, T.; Shevchenko, A.; Kaivola, M.; Friberg, A.T. Degree of polarization for optical near fields. Phys. Rev. E 2002, 66, 016615. [Google Scholar] [CrossRef] [PubMed]
- Weikersdorfer, D.; Adrian, D.B.; Cremers, D.; Conradt, J. Event-based 3D SLAM with a depth-augmented dynamic vision sensor. In Proceedings of the 2014 IEEE International Conference on Robotics and Automation (ICRA), IEEE, Hong Kong, China, 31 May–7 June 2014; pp. 359–364. [Google Scholar]
- Yang, M.; Liu, S.-C.; Delbruck, T. A dynamic vision sensor with 1% temporal contrast sensitivity and in-pixel asynchronous delta modulator for event encoding. IEEE J. Solid-State Circuits 2015, 50, 2149–2160. [Google Scholar] [CrossRef]
- DeBoo, B.; Sasian, J.; Chipman, R. Degree of polarization surfaces and maps for analysis of depolarization. Opt. Express 2004, 12, 4941–4958. [Google Scholar] [CrossRef]
- Schaefer, B.; Collett, E.; Smyth, R.; Barrett, D.; Fraher, B. Measuring the Stokes polarization parameters. Am. J. Phys. 2007, 75, 163–168. [Google Scholar] [CrossRef]
- Demos, S.G.; Alfano, R.R. Optical polarization imaging. Appl. Opt. 1997, 36, 150–155. [Google Scholar] [CrossRef]
- Brukner, C.; Zeilinger, A. Malus’ law and quantum information. Acta Phys. Slovaca 1999, 49, 647–652. [Google Scholar]
- Chen, X.Y.; San, M. Description of effect of polarimeter with slanting ray. Opt. Technol. 2006, 32, 425–427. [Google Scholar]
- Sharma, K.K. Optics: Principles and Applications; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Ivchenko, V. Malus’ law revisited. Rev. Cuba. Física 2023, 40, 75–77. [Google Scholar]
- Godo, K.; Kinoshita, K.; Nakazawa, Y.; Ishida, K.; Fujiki, A.; Nikai, M.; Niimi, Y.; Teranishi, H.; Nishioka, T. LED-based standard source providing CIE standard illuminant A for replacing incandescent standard lamps. Measurement 2024, 239, 115479. [Google Scholar] [CrossRef]
- Fu, Q.; Liu, X.; Wang, L.; Zhan, J.; Zhang, S.; Zhang, T.; Li, Z.; Duan, J.; Li, Y.; Jiang, H. Analysis of target surface polarization characteristics and inversion of complex refractive index based on three-component model optimization. Opt. Laser Technol. 2023, 162, 109225. [Google Scholar] [CrossRef]
- Muglikar, M.; Bauersfeld, L.; Moeys, D.P.; Scaramuzza, D. Event-based shape from polarization. In Proceedings of the IEEE/CVF Conference on Computer Vision and Pattern Recognition, Vancouver, BC, Canada, 18–22 June 2023; pp. 1547–1556. [Google Scholar]




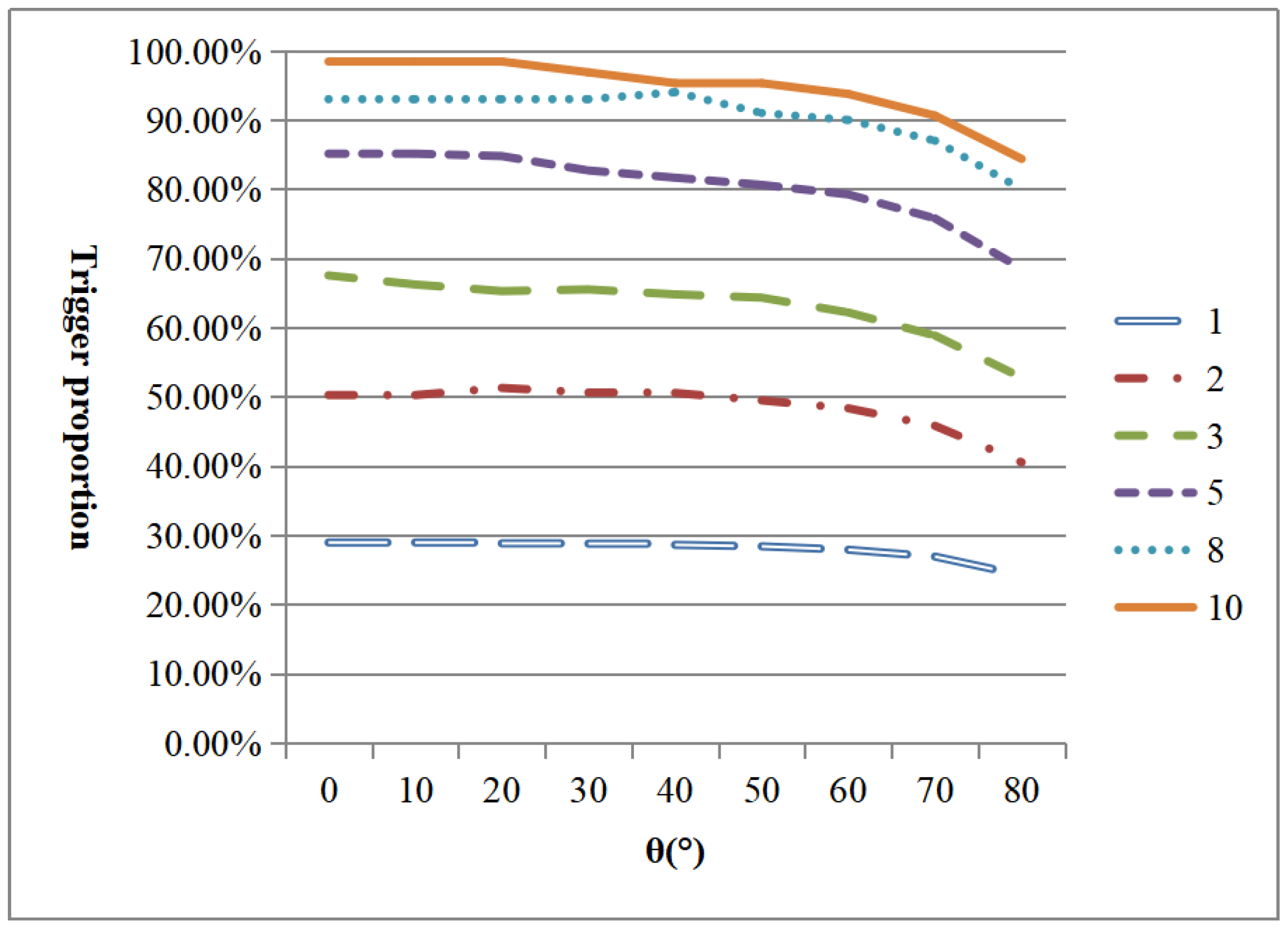



| Rotation Step (°) | θ (°) | Total Number of Detections | Number of Triggering Events | Trigger Proportion (%) | Rotation Step (°) | θ (°) | Total Number of Detections | Number of Triggering Events | Trigger Proportion (%) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 89 | 0 | 0.00% | 2 | 0 | 44 | 0 | 0.00% |
| 10 | 89 | 0 | 0.00% | 10 | 44 | 0 | 0.00% | ||
| 20 | 89 | 0 | 0.00% | 20 | 44 | 0 | 0.00% | ||
| 30 | 89 | 0 | 0.00% | 30 | 44 | 0 | 0.00% | ||
| 40 | 89 | 0 | 0.00% | 40 | 44 | 0 | 0.00% | ||
| 50 | 89 | 0 | 0.00% | 50 | 44 | 0 | 0.00% | ||
| 60 | 89 | 0 | 0.00% | 60 | 44 | 0 | 0.00% | ||
| 70 | 89 | 0 | 0.00% | 70 | 44 | 0 | 0.00% | ||
| 80 | 89 | 0 | 0.00% | 80 | 44 | 8 | 18.18% | ||
| 3 | 0 | 29 | 0 | 0.00% | 5 | 0 | 17 | 0 | 0.00% |
| 10 | 29 | 0 | 0.00% | 10 | 17 | 0 | 0.00% | ||
| 20 | 29 | 0 | 0.00% | 20 | 17 | 0 | 0.00% | ||
| 30 | 29 | 0 | 0.00% | 30 | 17 | 0 | 0.00% | ||
| 40 | 29 | 0 | 0.00% | 40 | 17 | 0 | 0.00% | ||
| 50 | 29 | 0 | 0.00% | 50 | 17 | 0 | 0.00% | ||
| 60 | 29 | 0 | 0.00% | 60 | 17 | 0 | 0.00% | ||
| 70 | 29 | 0 | 0.00% | 70 | 17 | 6 | 35.29% | ||
| 80 | 29 | 10 | 34.48% | 80 | 17 | 9 | 52.94% | ||
| 8 | 0 | 10 | 0 | 0.00% | 10 | 0 | 8 | 0 | 0.00% |
| 10 | 10 | 0 | 0.00% | 10 | 8 | 0 | 0.00% | ||
| 20 | 10 | 0 | 0.00% | 20 | 8 | 0 | 0.00% | ||
| 30 | 10 | 0 | 0.00% | 30 | 8 | 0 | 0.00% | ||
| 40 | 10 | 0 | 0.00% | 40 | 8 | 0 | 0.00% | ||
| 50 | 10 | 0 | 0.00% | 50 | 8 | 1 | 12.50% | ||
| 60 | 10 | 5 | 50.00% | 60 | 8 | 5 | 62.50% | ||
| 70 | 10 | 6 | 60.00% | 70 | 8 | 5 | 62.50% | ||
| 80 | 10 | 6 | 60.00% | 80 | 8 | 6 | 75.00% |
| Rotation Step (°) | θ (°) | Total Number of Detections | Number of Triggering Events | Trigger Proportion (%) | Rotation Step (°) | θ (°) | Total Number of Detections | Number of Triggering Events | Trigger Proportion (%) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 7921 | 2294 | 28.96% | 2 | 0 | 1936 | 973 | 50.26% |
| 10 | 7921 | 2294 | 28.96% | 10 | 1936 | 973 | 50.26% | ||
| 20 | 7921 | 2284 | 28.83% | 20 | 1936 | 993 | 51.29% | ||
| 30 | 7921 | 2281 | 28.80% | 30 | 1936 | 980 | 50.62% | ||
| 40 | 7921 | 2267 | 28.62% | 40 | 1936 | 979 | 50.57% | ||
| 50 | 7921 | 2250 | 28.41% | 50 | 1936 | 958 | 49.48% | ||
| 60 | 7921 | 2211 | 27.91% | 60 | 1936 | 936 | 48.35% | ||
| 70 | 7921 | 2133 | 26.93% | 70 | 1936 | 887 | 45.82% | ||
| 80 | 7921 | 1920 | 24.24% | 80 | 1936 | 785 | 40.55% | ||
| 3 | 0 | 841 | 568 | 67.54% | 5 | 0 | 289 | 246 | 85.12% |
| 10 | 841 | 557 | 66.23% | 10 | 289 | 246 | 85.12% | ||
| 20 | 841 | 549 | 65.28% | 20 | 289 | 245 | 84.78% | ||
| 30 | 841 | 551 | 65.52% | 30 | 289 | 239 | 82.70% | ||
| 40 | 841 | 545 | 64.80% | 40 | 289 | 236 | 81.66% | ||
| 50 | 841 | 541 | 64.33% | 50 | 289 | 233 | 80.62% | ||
| 60 | 841 | 523 | 62.19% | 60 | 289 | 229 | 79.24% | ||
| 70 | 841 | 495 | 58.86% | 70 | 289 | 219 | 75.78% | ||
| 80 | 841 | 444 | 52.79% | 80 | 289 | 198 | 68.51% | ||
| 8 | 0 | 100 | 93 | 93.00% | 10 | 0 | 64 | 63 | 98.44% |
| 10 | 100 | 93 | 93.00% | 10 | 64 | 63 | 98.44% | ||
| 20 | 100 | 93 | 93.00% | 20 | 64 | 63 | 98.44% | ||
| 30 | 100 | 93 | 93.00% | 30 | 64 | 62 | 96.88% | ||
| 40 | 100 | 94 | 94.00% | 40 | 64 | 61 | 95.31% | ||
| 50 | 100 | 91 | 91.00% | 50 | 64 | 61 | 95.31% | ||
| 60 | 100 | 90 | 90.00% | 60 | 64 | 60 | 93.75% | ||
| 70 | 100 | 87 | 87.00% | 70 | 64 | 58 | 90.63% | ||
| 80 | 100 | 80 | 80.00% | 80 | 64 | 54 | 84.38% |
| Rotation Step (°) | θ (°) | Total Number of Detections | Number of Triggering Events | Trigger Proportion (%) | Rotation Step (°) | θ (°) | Total Number of Detections | Number of Triggering Events | Trigger Proportion (%) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 7921 | 0 | 0.00% | 2 | 0 | 1936 | 0 | 0.00% |
| 10 | 7921 | 0 | 0.00% | 10 | 1936 | 0 | 0.00% | ||
| 20 | 7921 | 0 | 0.00% | 20 | 1936 | 0 | 0.00% | ||
| 30 | 7921 | 0 | 0.00% | 30 | 1936 | 0 | 0.00% | ||
| 40 | 7921 | 0 | 0.00% | 40 | 1936 | 0 | 0.00% | ||
| 50 | 7921 | 0 | 0.00% | 50 | 1936 | 0 | 0.00% | ||
| 60 | 7921 | 0 | 0.00% | 60 | 1936 | 0 | 0.00% | ||
| 70 | 7921 | 0 | 0.00% | 70 | 1936 | 108 | 5.58% | ||
| 80 | 7921 | 0 | 0.00% | 80 | 1936 | 366 | 18.90% | ||
| 3 | 0 | 841 | 110 | 13.08% | 5 | 0 | 289 | 199 | 68.86% |
| 10 | 841 | 119 | 14.15% | 10 | 289 | 199 | 68.86% | ||
| 20 | 841 | 148 | 17.60% | 20 | 289 | 192 | 66.44% | ||
| 30 | 841 | 196 | 23.31% | 30 | 289 | 189 | 65.40% | ||
| 40 | 841 | 231 | 27.47% | 40 | 289 | 198 | 68.51% | ||
| 50 | 841 | 267 | 31.75% | 50 | 289 | 203 | 70.24% | ||
| 60 | 841 | 302 | 35.91% | 60 | 289 | 205 | 70.93% | ||
| 70 | 841 | 338 | 40.19% | 70 | 289 | 202 | 69.90% | ||
| 80 | 841 | 353 | 41.97% | 80 | 289 | 193 | 66.78% | ||
| 8 | 0 | 100 | 84 | 84.00% | 10 | 0 | 64 | 56 | 87.50% |
| 10 | 100 | 84 | 84.00% | 10 | 64 | 61 | 95.31% | ||
| 20 | 100 | 84 | 84.00% | 20 | 64 | 61 | 95.31% | ||
| 30 | 100 | 84 | 84.00% | 30 | 64 | 57 | 89.06% | ||
| 40 | 100 | 89 | 89.00% | 40 | 64 | 58 | 90.63% | ||
| 50 | 100 | 89 | 89.00% | 50 | 64 | 59 | 92.19% | ||
| 60 | 100 | 88 | 88.00% | 60 | 64 | 60 | 93.75% | ||
| 70 | 100 | 86 | 86.00% | 70 | 64 | 58 | 90.63% | ||
| 80 | 100 | 82 | 82.00% | 80 | 64 | 56 | 87.50% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, X.; Xing, K.; Li, S.; Gu, Z.; Xin, L. Theoretical Research on Large Field-of-View Polarization Imaging Based on Dynamic Vision Sensors. Photonics 2025, 12, 426. https://doi.org/10.3390/photonics12050426
Lu X, Xing K, Li S, Gu Z, Xin L. Theoretical Research on Large Field-of-View Polarization Imaging Based on Dynamic Vision Sensors. Photonics. 2025; 12(5):426. https://doi.org/10.3390/photonics12050426
Chicago/Turabian StyleLu, Xiaotian, Kunpeng Xing, Siran Li, Ziyu Gu, and Lei Xin. 2025. "Theoretical Research on Large Field-of-View Polarization Imaging Based on Dynamic Vision Sensors" Photonics 12, no. 5: 426. https://doi.org/10.3390/photonics12050426
APA StyleLu, X., Xing, K., Li, S., Gu, Z., & Xin, L. (2025). Theoretical Research on Large Field-of-View Polarization Imaging Based on Dynamic Vision Sensors. Photonics, 12(5), 426. https://doi.org/10.3390/photonics12050426





