Phase-Conjugate Feedback Effects in Electrically Pumped Semiconductor Nano-Laser Arrays
Abstract
1. Introduction
2. Model
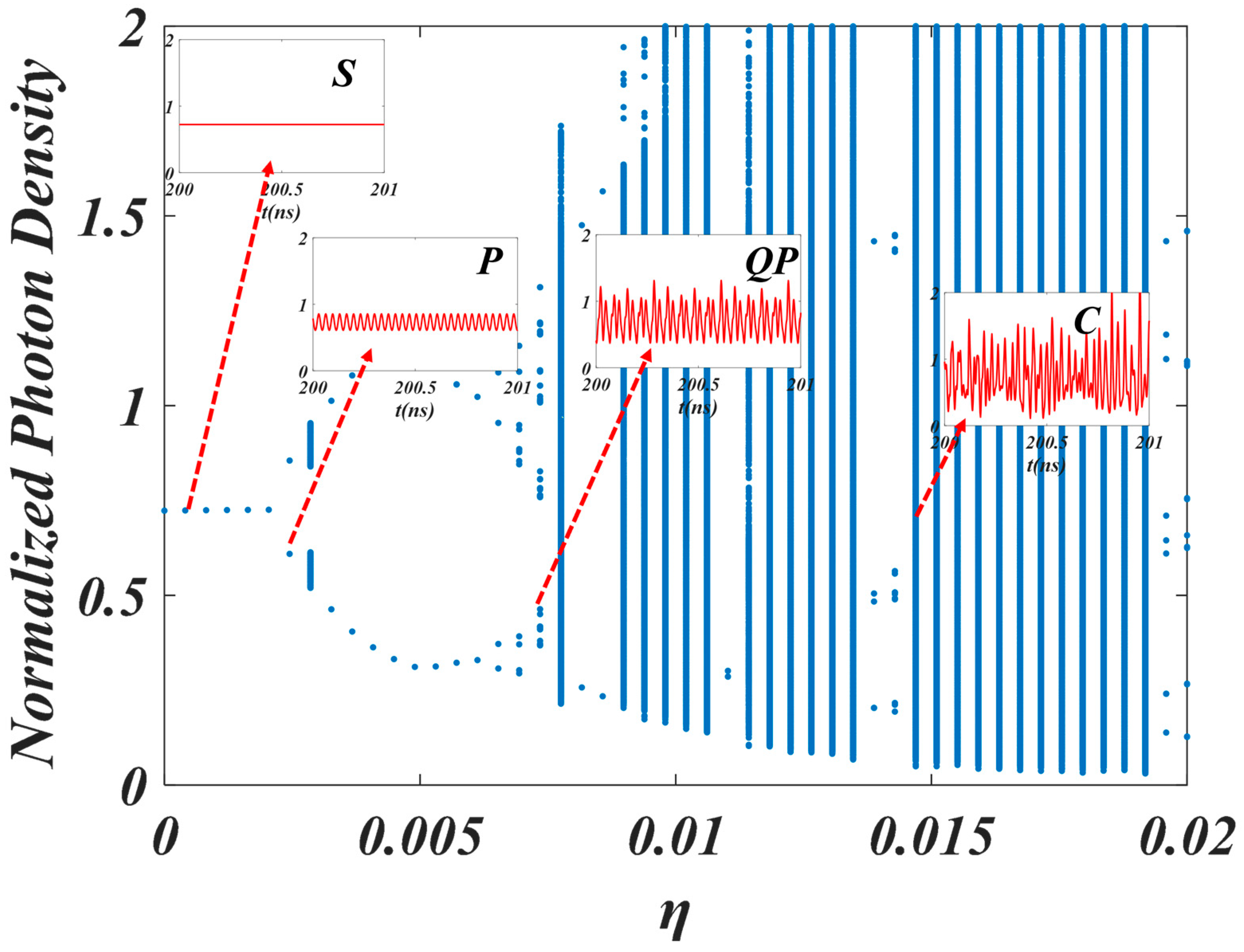
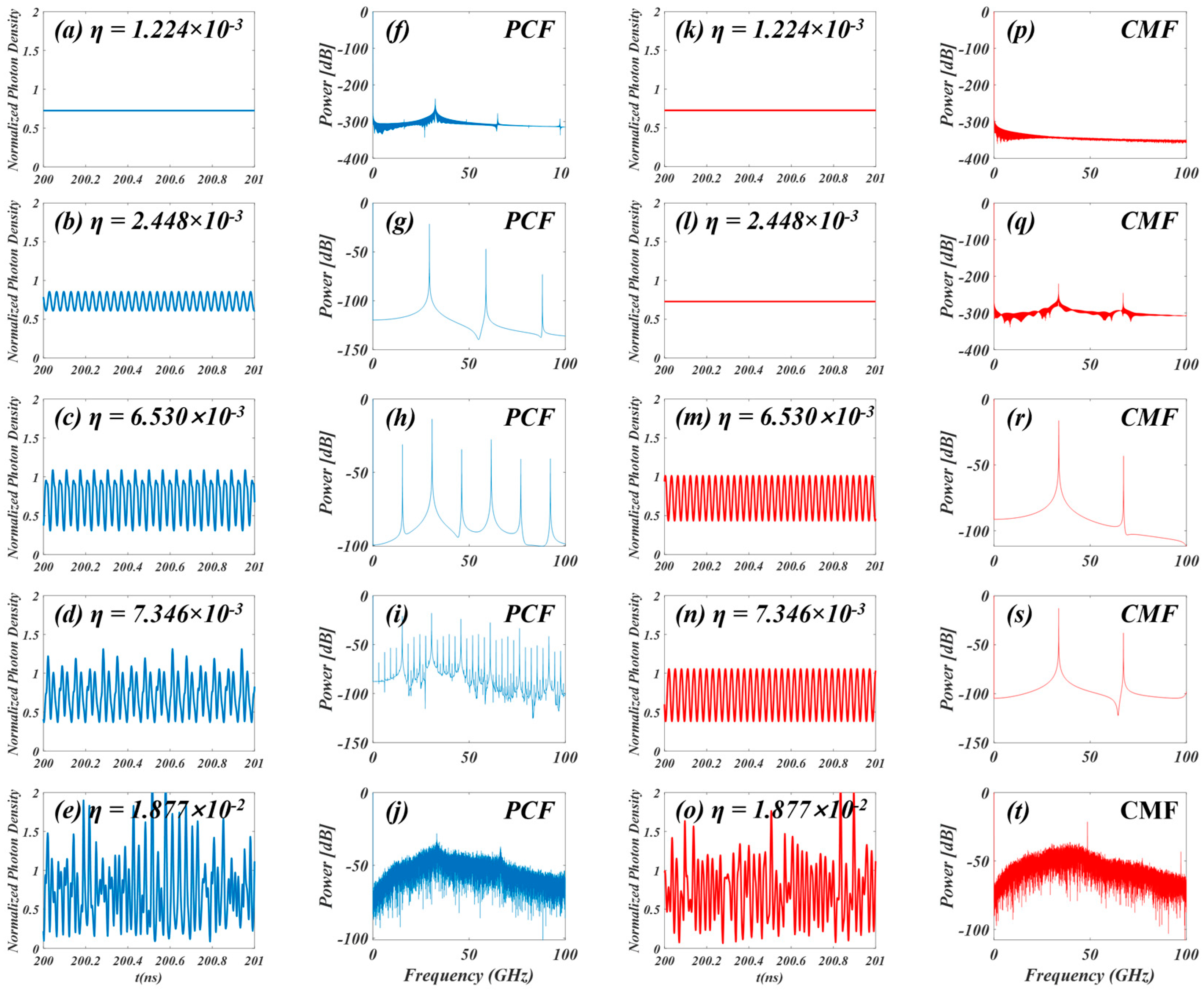
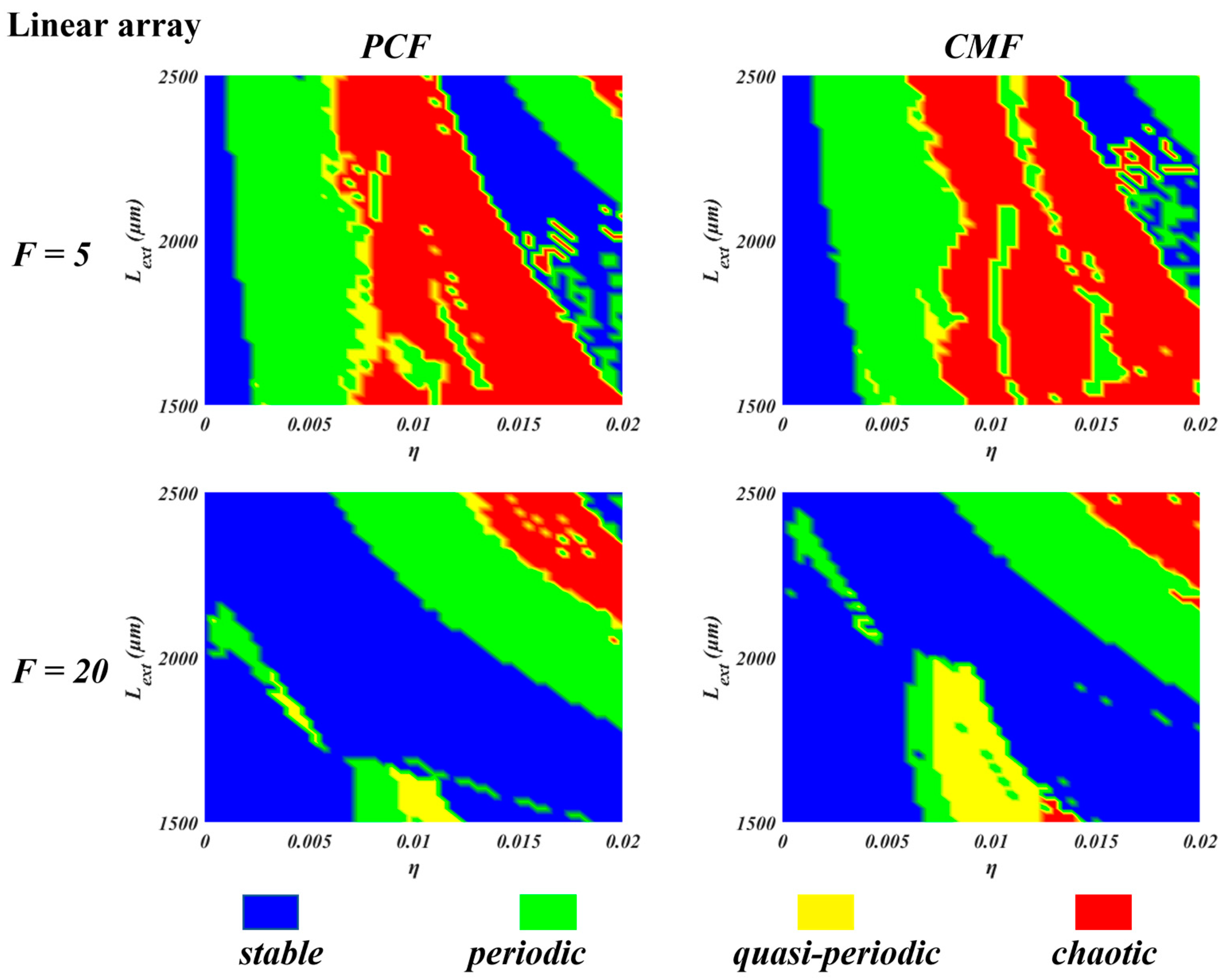
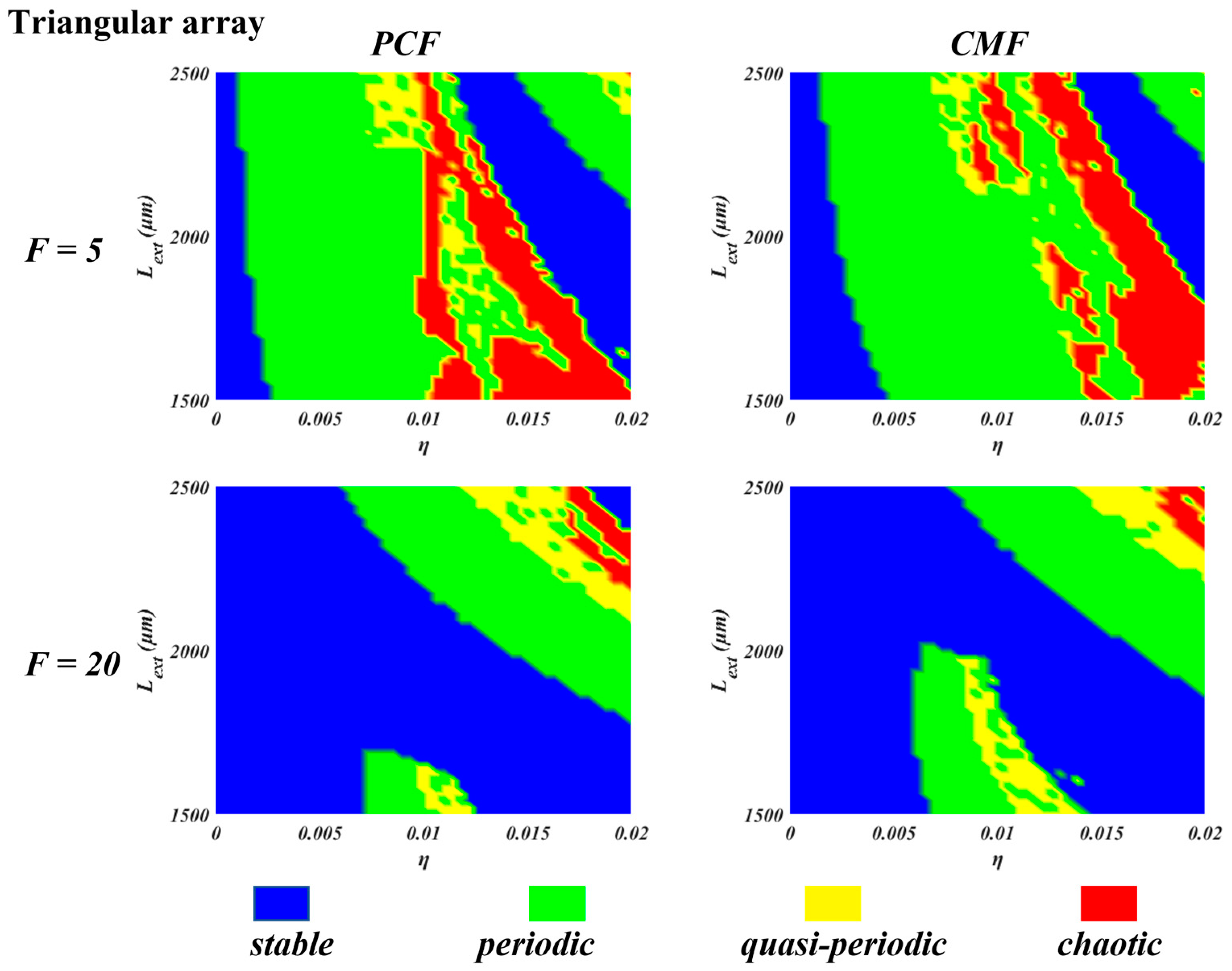
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Jiang, P.; Zhou, P.; Li, N.; Mu, P.; Li, X. Optically Injected Nano-lasers for Time-Delay Signature Suppression and Communications. Opt. Express 2020, 28, 26421. [Google Scholar] [CrossRef] [PubMed]
- Fan, Y.; Shore, K.A.; Shao, X. Dynamics of Electrically Pumped Semiconductor Nano-Laser Arrays. Photonics 2023, 10, 1249. [Google Scholar] [CrossRef]
- Purcell, E.M. Spontaneous emission probabilities at radio frequencies. Phys. Rev. 1946, 69, 681. [Google Scholar]
- Gu, Q.; Slutsky, B.; Vallini, F.; Smalley, J.S.; Nezhad, M.P.; Frateschi, N.C.; Fainman, Y. Purcell effect in sub-wavelength semiconductor lasers. Opt. Express 2013, 21, 15603–15617. [Google Scholar] [CrossRef] [PubMed]
- Romeira, B.; Fiore, A. Purcell Effect in the Stimulated and Spontaneous Emission Rates of Nanoscale Semiconductor Lasers. IEEE J. Quantum Electron. 2018, 54, 2000412. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Gray, G.R. Effect of phase-conjugate feedback on the noise characteristics of semiconductor lasers. Phys. Rev. A 1992, 46, 5890–5898. [Google Scholar] [CrossRef] [PubMed]
- Gu, Q.; Shane, J.; Vallini, F.; Wingad, B.; Smalley, J.S.; Frateschi, N.C.; Fainman, Y. Amorphous Al2O3 shield for thermal management in electrically pumped metallo-dielectric nanolasers. IEEE J. Quantum Electron. 2014, 50, 499–509. [Google Scholar] [CrossRef]

| Symbol | Physical Meaning | Value |
|---|---|---|
| κ | Feedback strength | 0~100.59 ns−1 |
| τ | External cavity roundtrip delay | 10.0~16.7 ps |
| F | Purcell factor | 5.20 |
| β | Spontaneous emission coupling factor | 0.05 |
| Γ | Confinement factor | 0.645 |
| τn | Carrier lifetime | 2.00 × 10−9 s |
| gn | Differential gain | 1.65 × 10−12 m3/s |
| N0 | Carrier density at transparency | 1.10 × 1024 m−3 |
| τp | Photon lifetime | 0.36 × 10−12 s |
| α | Linewidth enhancement factor | 5 |
| Δω | Frequency detuning | 0 GHz |
| I | Injection current | 2.2 mA |
| e | Elementary charge | 1.60 × 10−19 C |
| Va | Volume of the active region | 3.96 × 10−19 m3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, Y.; Zhang, J.; Shore, K.A. Phase-Conjugate Feedback Effects in Electrically Pumped Semiconductor Nano-Laser Arrays. Photonics 2025, 12, 176. https://doi.org/10.3390/photonics12030176
Fan Y, Zhang J, Shore KA. Phase-Conjugate Feedback Effects in Electrically Pumped Semiconductor Nano-Laser Arrays. Photonics. 2025; 12(3):176. https://doi.org/10.3390/photonics12030176
Chicago/Turabian StyleFan, Yuanlong, Jing Zhang, and K. Alan Shore. 2025. "Phase-Conjugate Feedback Effects in Electrically Pumped Semiconductor Nano-Laser Arrays" Photonics 12, no. 3: 176. https://doi.org/10.3390/photonics12030176
APA StyleFan, Y., Zhang, J., & Shore, K. A. (2025). Phase-Conjugate Feedback Effects in Electrically Pumped Semiconductor Nano-Laser Arrays. Photonics, 12(3), 176. https://doi.org/10.3390/photonics12030176





