Photonic Angular Momentum in Intense Light–Matter Interactions
Abstract
1. Introduction
2. Review of Plane-Wave Fields
2.1. The Helmholtz Equation
2.2. Spin Angular Momentum
2.3. Orbital Angular Momentum
2.3.1. Analogies with Classical Mechanics
2.3.2. Wave Interpretation of Electromagnetic Radiation
2.3.3. The Magnetic Quantum Number
2.3.4. Azimuthal Quantum Number L
2.3.5. Plane-Wave OAM
2.4. Complete Orbital and Spin Representation of Radiation Fields
2.5. Plane-Wave Selection Rules
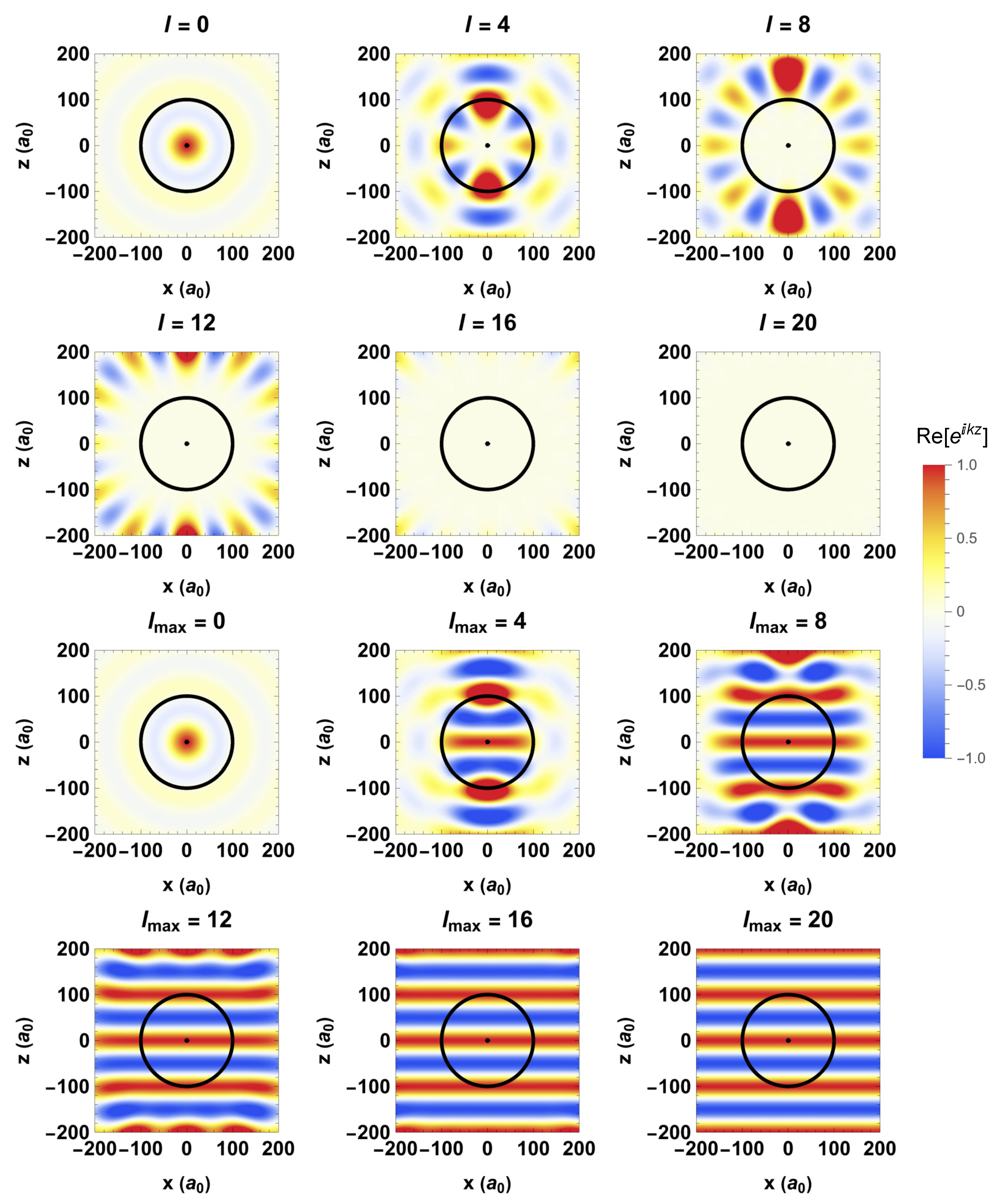
3. Vortex Fields
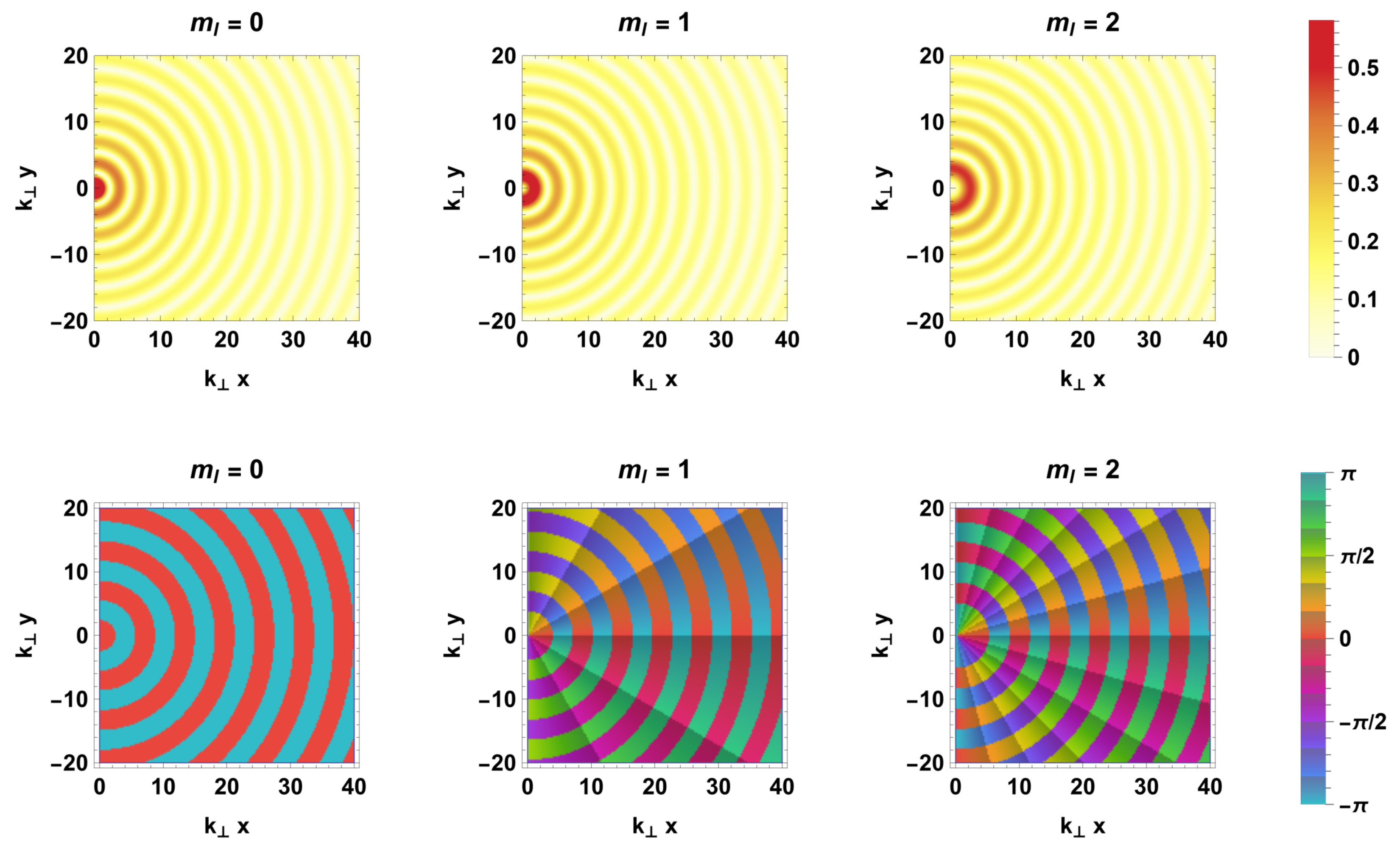
3.1. Bessel Beams
Transverse and Longitudinal Angular Momentum
3.2. The Paraxial Approximation
3.2.1. Bessel Beam Limits
3.2.2. Violation of the Transversality Condition
3.2.3. The General Case
3.2.4. Laguerre–Gaussian Beams
3.2.5. Scale Considerations and Refinements to the Paraxial Theory
3.3. The Angular Momentum of a Vortex Field
3.3.1. Classical Electrodynamics
3.3.2. Wave Operators
3.3.3. How Much OAM and SAM Do Individual Photons Have?
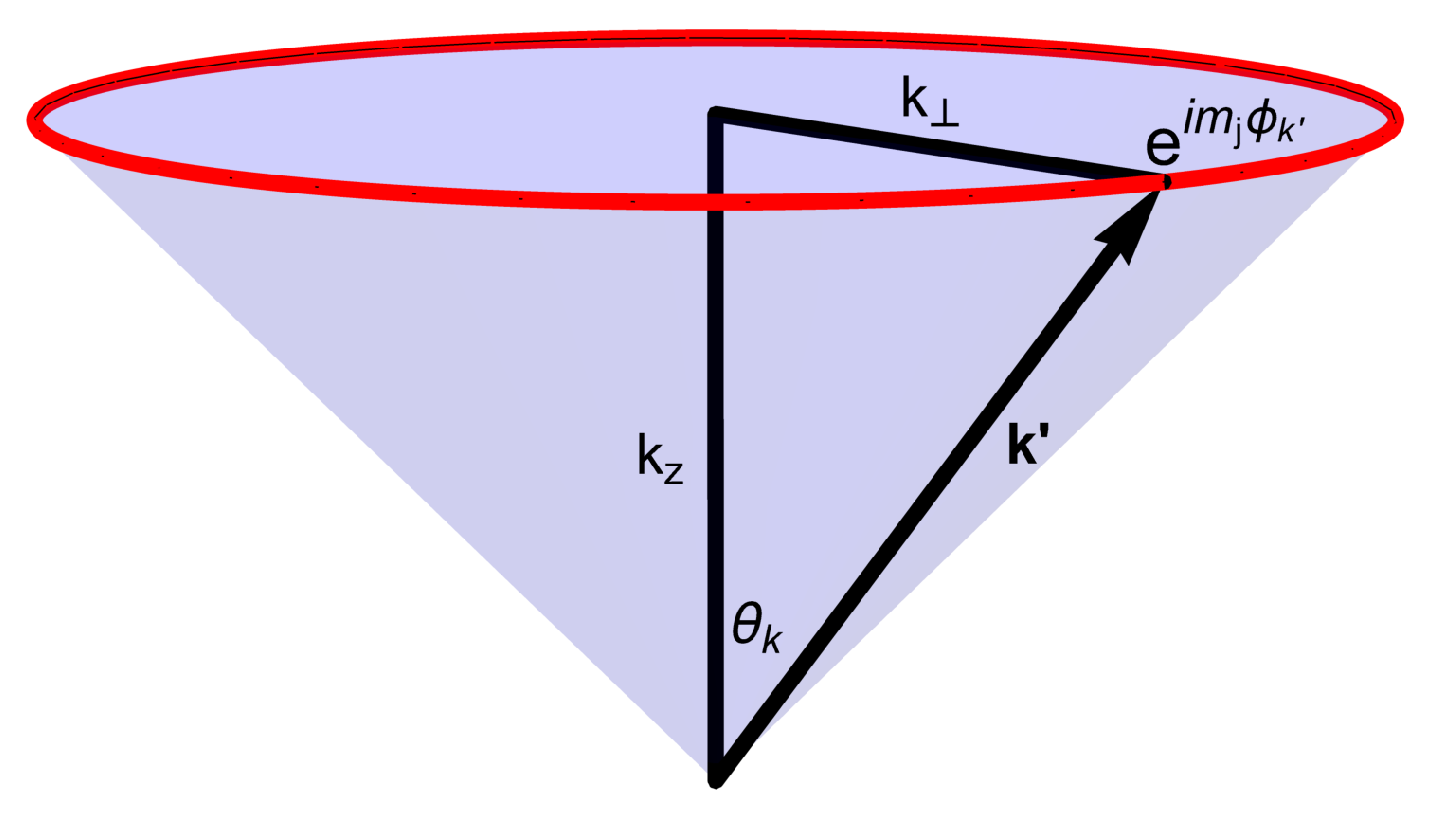
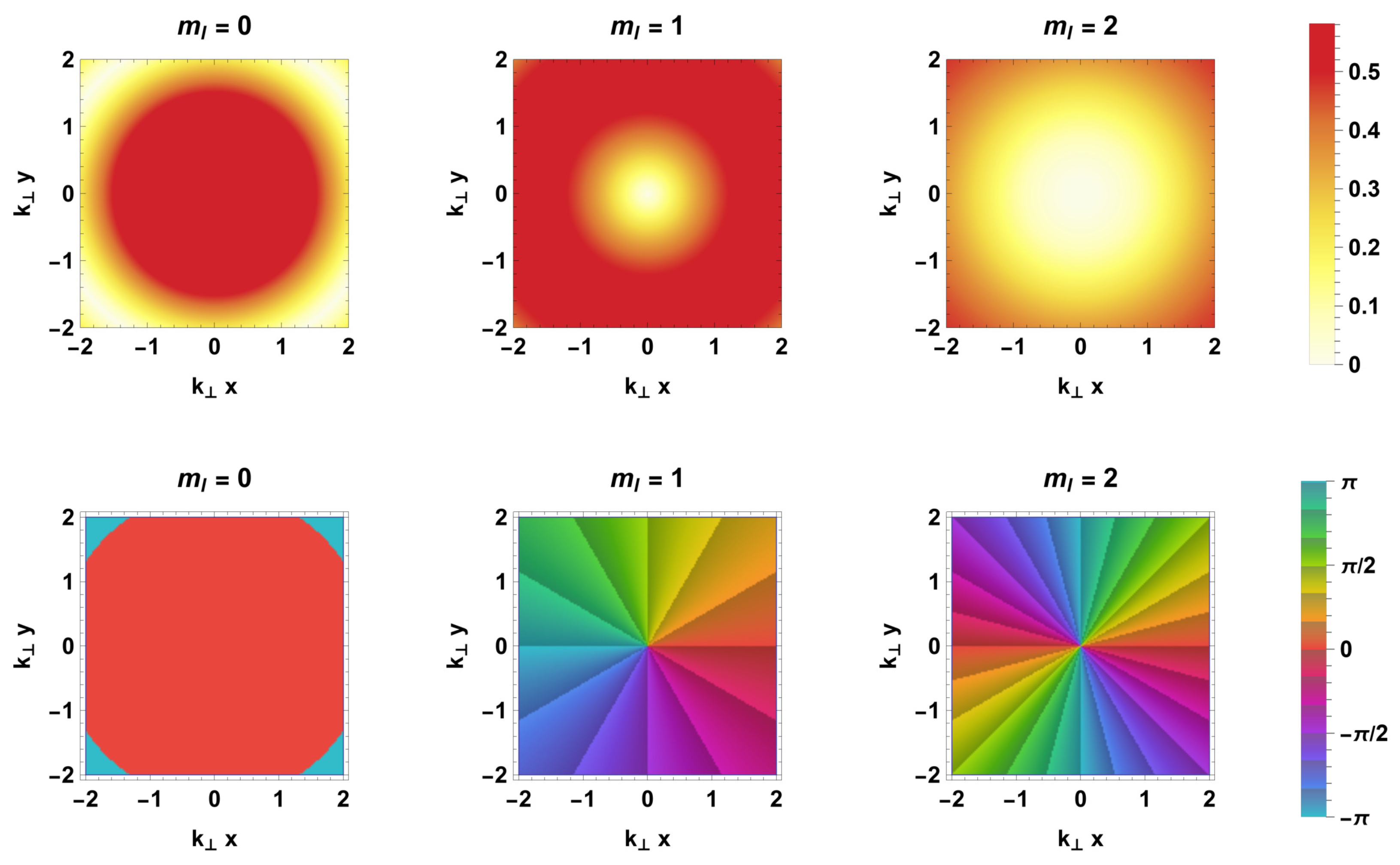
4. Single- and Few-Photon Ionization with Vortex Fields
4.1. Perturbative Single Photon Ionization
4.2. What If an Atom Is Placed Exactly at the Vortex Center?
4.3. The Long Wavelength Limit
4.4. What If Atoms Are Displaced Far from the Vortex Center?
5. Strong-Field Ionization by Vortex Fields
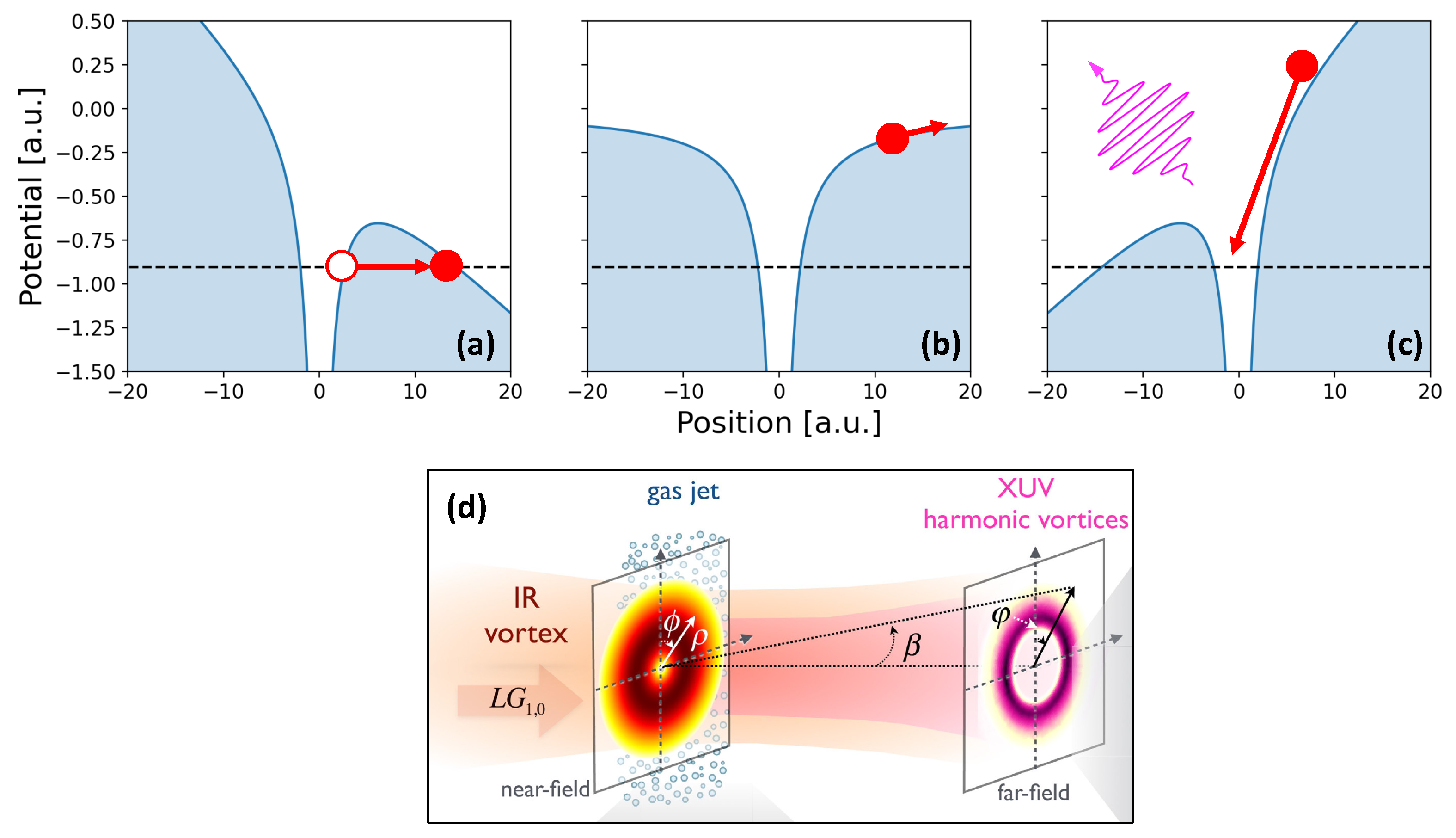

6. High-Harmonic Generation and OAM Conservation
7. Discussion
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| HHG | High-harmonic generation |
| LG | Laguerre–Gaussian |
| OAM | Orbital angular momentum |
| SAM | Spin angular momentum |
| TAM | Total angular momentum |
Appendix A. Plane-Wave OAM
Appendix B. Classical Electrodynamics
References
- Heckenberg, N.; McDuff, R.; Smith, C.; White, A. Generation of optical phase singularities by computer-generated holograms. Opt. Lett. 1992, 17, 221–223. [Google Scholar] [CrossRef] [PubMed]
- Tamm, C.; Weiss, C.O. Bistability and optical switching of spatial patterns in a laser. JOSA B 1990, 7, 1034–1038. [Google Scholar] [CrossRef]
- Bazhenov, V.Y.; Vasnetsov, M.; Soskin, M. Laser beams with screw dislocations in their wavefronts. Jetp Lett. 1990, 52, 429–431. [Google Scholar]
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef] [PubMed]
- Allen, L.; Padgett, M.J.; Babiker, M. IV The Orbital Angular Momentum of Light. In Progress in Optics; Wolf, E., Ed.; Elsevier: Amsterdam, The Netherlands, 1999; Volume 39, pp. 291–372. [Google Scholar] [CrossRef]
- Shen, Y.; Wang, X.; Xie, Z.; Min, C.; Fu, X.; Liu, Q.; Gong, M.; Yuan, X. Optical vortices 30 years on: OAM manipulation from topological charge to multiple singularities. Light. Sci. Appl. 2019, 8, 90. [Google Scholar] [CrossRef]
- Gibson, G.; Courtial, J.; Padgett, M.J.; Vasnetsov, M.; Pas’ko, V.; Barnett, S.M.; Franke-Arnold, S. Free-space information transfer using light beams carrying orbital angular momentum. Opt. Express 2004, 12, 5448–5456. [Google Scholar] [CrossRef]
- He, H.; Friese, M.E.J.; Heckenberg, N.R.; Rubinsztein-Dunlop, H. Direct Observation of Transfer of Angular Momentum to Absorptive Particles from a Laser Beam with a Phase Singularity. Phys. Rev. Lett. 1995, 75, 826–829. [Google Scholar] [CrossRef]
- Andersen, M.; Ryu, C.; Cladé, P.; Natarajan, V.; Vaziri, A.; Helmerson, K.; Phillips, W.D. Quantized rotation of atoms from photons with orbital angular momentum. Phys. Rev. Lett. 2006, 97, 170406. [Google Scholar] [CrossRef] [PubMed]
- Araoka, F.; Verbiest, T.; Clays, K.; Persoons, A. Interactions of twisted light with chiral molecules: An experimental investigation. Phys. Rev. A—Atomic Mol. Opt. Phys. 2005, 71, 055401. [Google Scholar] [CrossRef]
- Löffler, W.; Broer, D.; Woerdman, J. Circular dichroism of cholesteric polymers and the orbital angular momentum of light. Phys. Rev. A—Atomic. Mol. Opt. Phys. 2011, 83, 065801. [Google Scholar] [CrossRef]
- Mathevet, R.; de Lesegno, B.V.; Pruvost, L.; Rikken, G.L. Negative experimental evidence for magneto-orbital dichroism. Opt. Express 2013, 21, 3941–3945. [Google Scholar] [CrossRef] [PubMed]
- Kaneyasu, T.; Hikosaka, Y.; Fujimoto, M.; Konomi, T.; Katoh, M.; Iwayama, H.; Shigemasa, E. Limitations in photoionization of helium by an extreme ultraviolet optical vortex. Phys. Rev. A 2017, 95, 023413. [Google Scholar] [CrossRef]
- Picón, A.; Mompart, J.; Aldana, J.R.V.d.; Plaja, L.; Calvo, G.F.; Roso, L. Photoionization with orbital angular momentum beams. Opt. Express 2010, 18, 3660–3671. [Google Scholar] [CrossRef] [PubMed]
- Picón, A.; Benseny, A.; Mompart, J.; Aldana, J.R.V.d.; Plaja, L.; Calvo, G.F.; Roso, L. Transferring orbital and spin angular momenta of light to atoms. New J. Phys. 2010, 12, 083053. [Google Scholar] [CrossRef]
- Schmiegelow, C.T.; Schulz, J.; Kaufmann, H.; Ruster, T.; Poschinger, U.G.; Schmidt-Kaler, F. Transfer of optical orbital angular momentum to a bound electron. Nat. Commun. 2016, 7, 12998. [Google Scholar] [CrossRef] [PubMed]
- Afanasev, A.; Carlson, C.E.; Schmiegelow, C.T.; Schulz, J.; Schmidt-Kaler, F.; Solyanik, M. Experimental verification of position-dependent angular-momentum selection rules for absorption of twisted light by a bound electron. New J. Phys. 2018, 20, 023032. [Google Scholar] [CrossRef]
- De Ninno, G.; Wätzel, J.; Ribič, P.R.; Allaria, E.; Coreno, M.; Danailov, M.B.; David, C.; Demidovich, A.; Di Fraia, M.; Giannessi, L.; et al. Photoelectric effect with a twist. Nat. Photonics 2020, 14, 554–558. [Google Scholar] [CrossRef]
- Sen, A.; Sinha, A.; Sen, S.; Sharma, V.; Gopal, R. Above-threshold ionization of argon with ultrashort orbital-angular-momentum beams. Phys. Rev. A 2022, 106, 023103. [Google Scholar] [CrossRef]
- Hernández-García, C.; Picón, A.; San Román, J.; Plaja, L. Attosecond Extreme Ultraviolet Vortices from High-Order Harmonic Generation. Phys. Rev. Lett. 2013, 111, 083602. [Google Scholar] [CrossRef] [PubMed]
- Gariepy, G.; Leach, J.; Kim, K.T.; Hammond, T.; Frumker, E.; Boyd, R.W.; Corkum, P. Creating High-Harmonic Beams with Controlled Orbital Angular Momentum. Phys. Rev. Lett. 2014, 113, 153901. [Google Scholar] [CrossRef] [PubMed]
- Berestetskii, V.B.; Lifshitz, E.M.; Pitaevskii, L.P. Quantum Electrodynamics: Volume 4; Butterworth-Heinemann: Oxford, UK, 1982; Volume 4. [Google Scholar]
- Faisal, F.H. Theory of Multiphoton Processes; Springer: New York, NY, USA, 1987. [Google Scholar] [CrossRef]
- Eichmann, H.; Egbert, A.; Nolte, S.; Momma, C.; Wellegehausen, B.; Becker, W.; Long, S.; McIver, J. Polarization-dependent high-order two-color mixing. Phys. Rev. A 1995, 51, R3414. [Google Scholar] [CrossRef] [PubMed]
- Lambert, G.; Vodungbo, B.; Gautier, J.; Mahieu, B.; Malka, V.; Sebban, S.; Zeitoun, P.; Luning, J.; Perron, J.; Andreev, A.; et al. Towards enabling femtosecond helicity-dependent spectroscopy with high-harmonic sources. Nat. Commun. 2015, 6, 6167. [Google Scholar] [CrossRef] [PubMed]
- Gaumnitz, T.; Jain, A.; Pertot, Y.; Huppert, M.; Jordan, I.; Ardana-Lamas, F.; Wörner, H.J. Streaking of 43-attosecond soft-X-ray pulses generated by a passively CEP-stable mid-infrared driver. Opt. Express 2017, 25, 27506–27518. [Google Scholar] [CrossRef] [PubMed]
- Barreau, L.; Veyrinas, K.; Gruson, V.; Weber, S.J.; Auguste, T.; Hergott, J.F.; Lepetit, F.; Carré, B.; Houver, J.C.; Dowek, D.; et al. Evidence of depolarization and ellipticity of high harmonics driven by ultrashort bichromatic circularly polarized fields. Nat. Commun. 2018, 9, 4727. [Google Scholar] [CrossRef]
- Galli, M.; Wanie, V.; Lopes, D.P.; Månsson, E.P.; Trabattoni, A.; Colaizzi, L.; Saraswathula, K.; Cartella, A.; Frassetto, F.; Poletto, L.; et al. Generation of deep ultraviolet sub-2-fs pulses. Opt. Lett. 2019, 44, 1308–1311. [Google Scholar] [CrossRef] [PubMed]
- Walker, S.; Reiff, R.; Jaron-Becker, A.; Becker, A. Characterization of vacuum and deep ultraviolet pulses via two-photon autocorrelation signals. Opt. Lett. 2021, 46, 3083–3086. [Google Scholar] [CrossRef] [PubMed]
- Ghomashi, B.; Walker, S.; Becker, A. Enabling elliptically polarized high harmonic generation with short cross polarized laser pulses. Sci. Rep. 2023, 13, 12843. [Google Scholar] [CrossRef]
- Finger, K.; Walker, S.; Becker, A. Pulse characterization via two-photon auto- and cross-correlation. Opt. Express 2024, 32, 34732–34748. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics; John Wiley & Sons: Hoboken, NJ, USA, 2021. [Google Scholar]
- Landau, L.D. The Classical Theory of Fields; Elsevier: Amsterdam, The Netherlands, 2013; Volume 2. [Google Scholar]
- Zee, A. Quantum Field Theory in a Nutshell, 2nd ed.; Princeton University Press: Princeton, NJ, USA, 2010. [Google Scholar]
- Schwartz, M.D. Quantum Field Theory and the Standard Model; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Fierz, M.; Weisskopf, V.F. Theoretical Physics in the Twentieth Century: A Memorial Volume to Wolfgang Pauli; Interscience Publishers: Genève, Switzerland, 1960. [Google Scholar]
- Wei, E.; Lan, Z.; Chen, M.L.; Chen, Y.P.; Sun, S. Spin and Orbital Angular Momenta of Electromagnetic Waves: From Classical to Quantum Forms. IEEE J. Multiscale Multiphysics Comput. Tech. 2024, 9, 113–117. [Google Scholar] [CrossRef]
- Rose, M.E. Multipole Fields; Wiley: New York, NY, USA, 1955. [Google Scholar]
- Yang, L.P.; Khosravi, F.; Jacob, Z. Quantum field theory for spin operator of the photon. Phys. Rev. Res. 2022, 4, 023165. [Google Scholar] [CrossRef]
- Blatt, J.M.; Weisskopf, V.F. Theoretical Nuclear Physics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Quantum Mechanics: Non-Relativistic Theory; Elsevier: Amsterdam, The Netherlands, 2013; Volume 3. [Google Scholar]
- Merzbacher, E. Quantum Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 1998. [Google Scholar]
- Merzbacher, E. Single valuedness of wave functions. Am. J. Phys. 1962, 30, 237–247. [Google Scholar] [CrossRef]
- Herzberg, G.; Spinks, J.W.T. Atomic Spectra and Atomic Structure; Courier Corporation: North Chelmsford, MA, USA, 1944. [Google Scholar]
- Bethe, H.A.; Salpeter, E.E. Quantum Mechanics of One- and Two-Electron Atoms; Springer: Boston, MA, USA, 1977. [Google Scholar] [CrossRef]
- Shankar, R. Principles of Quantum Mechanics; Springer: New York, NY, USA, 1994. [Google Scholar] [CrossRef]
- Sakurai, J.J.; Napolitano, J. Modern Quantum Mechanics, 3rd ed.; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Baz, A.I.; Zeldovich, Y.B.; Perelomov, A.M. Scattering, Reactions and Decay in Nonrelativistic Quantum Mechanics; Israel Program for Scientific Translations: Springfield, VA, USA, 1969. [Google Scholar]
- Rose, M.E. Elementary Theory of Angular Momentum; Courier Corporation: North Chelmsford, MA, USA, 1995. [Google Scholar]
- National Institute of Standards and Technology. 10.53 Power Series. NIST Digital Library of Mathematical Functions. 2024. Available online: https://dlmf.nist.gov/10.53 (accessed on 4 July 2024).
- Wang, B.; Li, L.; Zhang, Y.; Zhai, C.; Zhu, X.; Lan, P.; Lu, P. Non-dipole effect in vortex high-order harmonic generation. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 215601. [Google Scholar] [CrossRef]
- Starace, A.F. Photoionization of atoms. In Springer Handbook of Atomic, Molecular, and Optical Physics; Springer: Berlin/Heidelberg, Germany, 2006; pp. 383–394. [Google Scholar] [CrossRef]
- Krässig, B.; Jung, M.; Gemmell, D.; Kanter, E.; LeBrun, T.; Southworth, S.; Young, L. Nondipolar asymmetries of photoelectron angular distributions. Phys. Rev. Lett. 1995, 75, 4736. [Google Scholar] [CrossRef] [PubMed]
- Hemmers, O.; Guillemin, R.; Kanter, E.; Krässig, B.; Lindle, D.W.; Southworth, S.; Wehlitz, R.; Baker, J.; Hudson, A.; Lotrakul, M.; et al. Dramatic Nondipole Effects in Low-Energy Photoionization: Experimental and Theoretical Study of Xe 5s. Phys. Rev. Lett. 2003, 91, 053002. [Google Scholar] [CrossRef]
- Kanter, E.; Krässig, B.; Southworth, S.; Guillemin, R.; Hemmers, O.; Lindle, D.W.; Wehlitz, R.; Amusia, M.Y.; Chernysheva, L.; Martin, N. E1 − E2 interference in the vuv photoionization of He. Phys. Rev. A 2003, 68, 012714. [Google Scholar] [CrossRef]
- Lindle, D.W.; Hemmers, O. Breakdown of the dipole approximation in soft-X-ray photoemission. J. Electron Spectrosc. Relat. Phenom. 1999, 100, 297–311. [Google Scholar] [CrossRef]
- Brumboiu, I.E.; Eriksson, O.; Norman, P. Atomic photoionization cross sections beyond the electric dipole approximation. J. Chem. Phys. 2019, 150. [Google Scholar] [CrossRef]
- Rezvan, D.; Klyssek, K.; Grundmann, S.; Pier, A.; Novikovskiy, N.; Strenger, N.; Tsitsonis, D.; Kircher, M.; Vela-Peréz, I.; Fehre, K.; et al. Observation of Nondipole-Induced Asymmetry in the Angular Emission Distribution of Photoelectrons from Fixed-in-Space CO Molecules. Phys. Rev. Lett. 2022, 129, 253201. [Google Scholar] [CrossRef]
- Hosaka, K.; Adachi, J.; Golovin, A.; Takahashi, M.; Teramoto, T.; Watanabe, N.; Yagishita, A.; Semenov, S.; Cherepkov, N. Non-dipole effects in the angular distribution of photoelectrons from the K-shell of N2 molecule. J. Phys. B At. Mol. Opt. Phys. 2005, 39, L25. [Google Scholar] [CrossRef]
- Ayuso, D.; Neufeld, O.; Ordonez, A.F.; Decleva, P.; Lerner, G.; Cohen, O.; Ivanov, M.; Smirnova, O. Synthetic chiral light for efficient control of chiral light–matter interaction. Nat. Photonics 2019, 13, 866–871. [Google Scholar] [CrossRef]
- Rosales-Guzmán, C.; Volke-Sepulveda, K.; Torres, J.P. Light with enhanced optical chirality. Opt. Lett. 2012, 37, 3486–3488. [Google Scholar] [CrossRef] [PubMed]
- Matula, O.; Hayrapetyan, A.G.; Serbo, V.G.; Surzhykov, A.; Fritzsche, S. Atomic ionization of hydrogen-like ions by twisted photons: Angular distribution of emitted electrons. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 205002. [Google Scholar] [CrossRef]
- Ivanov, I.P. Colliding particles carrying nonzero orbital angular momentum. Phys. Rev. D 2011, 83, 093001. [Google Scholar] [CrossRef]
- Ivanov, I.; Serbo, V. Scattering of twisted particles: Extension to wave packets and orbital helicity. Phys. Rev. A 2011, 84, 033804. [Google Scholar] [CrossRef]
- Durnin, J.; Miceli Jr, J.; Eberly, J.H. Diffraction-free beams. Phys. Rev. Lett. 1987, 58, 1499. [Google Scholar] [CrossRef] [PubMed]
- Durnin, J.; Miceli, J.; Eberly, J.H. Comparison of Bessel and Gaussian beams. Opt. Lett. 1988, 13, 79–80. [Google Scholar] [CrossRef] [PubMed]
- Vasara, A.; Turunen, J.; Friberg, A.T. Realization of general nondiffracting beams with computer-generated holograms. JOSA A 1989, 6, 1748–1754. [Google Scholar] [CrossRef]
- Aiello, A.; Banzer, P.; Neugebauer, M.; Leuchs, G. From transverse angular momentum to photonic wheels. Nat. Photonics 2015, 9, 789–795. [Google Scholar] [CrossRef]
- Saito, S. Quantum field theory for coherent photons: Isomorphism between Stokes parameters and spin expectation values. Front. Phys. 2024, 11. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Nori, F. Transverse and longitudinal angular momenta of light. Phys. Rep. 2015, 592, 1–38. [Google Scholar] [CrossRef]
- Bekshaev, A.Y.; Bliokh, K.Y.; Nori, F. Transverse spin and momentum in two-wave interference. Phys. Rev. X 2015, 5, 011039. [Google Scholar] [CrossRef]
- Lax, M.; Louisell, W.H.; McKnight, W.B. From Maxwell to paraxial wave optics. Phys. Rev. A 1975, 11, 1365–1370. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Pattanayak, D.N. Gaussian beam propagation beyond the paraxial approximation. JOSA 1979, 69, 575–578. [Google Scholar] [CrossRef]
- Weisstein, E.W. Cylindrical Coordinates. 2024. From MathWorld—A Wolfram Web Resource. Available online: https://mathworld.wolfram.com/CylindricalCoordinates.html (accessed on 4 July 2024).
- Olver, F.W.J.; Maximon, L.C. NIST Digital Library of Mathematical Functions, Chapter 10: Bessel Functions. 2024. Available online: https://dlmf.nist.gov/10 (accessed on 28 July 2024).
- Vaveliuk, P.; Ruiz, B.; Lencina, A. Limits of the paraxial approximation in laser beams. Opt. Lett. 2007, 32, 927–929. [Google Scholar] [CrossRef] [PubMed]
- de Boer, M.; Beetle, C. On the paraxial approximation in quantum optics II: Henochromatic modes of a Maxwell field. arXiv 2023, arXiv:2302.05478. [Google Scholar] [CrossRef]
- Arlt, J.; Hitomi, T.; Dholakia, K. Atom guiding along Laguerre-Gaussian and Bessel light beams. Appl. Phys. B 2000, 71, 549–554. [Google Scholar] [CrossRef]
- Beijersbergen, M.W.; Allen, L.; Van der Veen, H.; Woerdman, J. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 1993, 96, 123–132. [Google Scholar] [CrossRef]
- Wang, A.; Yu, L.; Li, J.; Liang, X. Evolution of orbital angular momentum spectrum of broadband Laguerre–Gaussian beam in OPCPA process. Sci. Rep. 2023, 13, 55. [Google Scholar] [CrossRef]
- Cerjan, A.; Cerjan, C. Orbital angular momentum of Laguerre–Gaussian beams beyond the paraxial approximation. JOSA A 2011, 28, 2253–2260. [Google Scholar] [CrossRef][Green Version]
- Bouwkamp, C.J. Diffraction Theory. Rep. Prog. Phys. 1954, 17, 35. [Google Scholar] [CrossRef]
- Moulton, P.F. Spectroscopic and laser characteristics of Ti:Al2O3. J. Opt. Soc. Am. B 1986, 3, 125–133. [Google Scholar] [CrossRef]
- Rapoport, W.R.; Khattak, C.P. Efficient, Tunable Ti:Sapphire Laser. In Proceedings of the Tunable Solid-State Lasers II, Zigzag, OR, USA, 4–6 June 1986; Budgor, A.B., Esterowitz, L., DeShazer, L.G., Eds.; Springer: Berlin/Heidelberg, Germany, 1986; pp. 212–217. [Google Scholar]
- Barty, C.; Gordon Iii, C.; Lemoff, B. Multiterawatt 30-fs Ti: Sapphire laser system. Opt. Lett. 1994, 19, 1442–1444. [Google Scholar] [CrossRef] [PubMed]
- Loh, Z.H.; Khalil, M.; Correa, R.E.; Santra, R.; Buth, C.; Leone, S.R. Quantum state-resolved probing of strong-field-ionized xenon atoms using femtosecond high-order harmonic transient absorption spectroscopy. Phys. Rev. Lett. 2007, 98, 143601. [Google Scholar] [CrossRef]
- Pathak, V.B.; Lee, S.K.; Pae, K.H.; Hojbota, C.I.; Kim, C.M.; Nam, C.H. Strong field physics pursued with petawatt lasers. AAPPS Bull. 2021, 31, 1–20. [Google Scholar] [CrossRef]
- Novotny, L.; Hecht, B. Principles of Nano-Optics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar] [CrossRef]
- Andrews, D.L.; Babiker, M. (Eds.) The Angular Momentum of Light; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar] [CrossRef]
- Götte, J.B. Integral and Fractional Orbital Angular Momentum of Light. Ph.D. Thesis, University of Strathclyde, Glasgow, Scotland, 2006. [Google Scholar]
- Pavlov, I.; Chaikovskaia, A.; Karlovets, D. Generation of vortex electrons by atomic photoionization. arXiv 2024, arXiv:2405.15030. [Google Scholar] [CrossRef]
- Kiselev, M.D.; Gryzlova, E.V.; Grum-Grzhimailo, A.N. Angular distribution of photoelectrons generated in atomic ionization by twisted radiation. Phys. Rev. A 2023, 108, 023117. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Ludwig, A.; Maurer, J.; Mayer, B.; Phillips, C.; Gallmann, L.; Keller, U. Breakdown of the Dipole Approximation in Strong-Field Ionization. Phys. Rev. Lett. 2014, 113, 243001. [Google Scholar] [CrossRef] [PubMed]
- Maurer, J.; Keller, U. Ionization in intense laser fields beyond the electric dipole approximation: Concepts, methods, achievements and future directions. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 094001. [Google Scholar] [CrossRef]
- Böning, B.; Paufler, W.; Fritzsche, S. Above-threshold ionization by few-cycle Bessel pulses carrying orbital angular momentum. Phys. Rev. A 2018, 98, 023407. [Google Scholar] [CrossRef]
- Ivanov, M.Y.; Spanner, M.; Smirnova, O. Anatomy of strong field ionization. J. Mod. Opt. 2005, 52, 165–184. [Google Scholar] [CrossRef]
- Amini, K.; Biegert, J.; Calegari, F.; Chacón, A.; Ciappina, M.F.; Dauphin, A.; Efimov, D.K.; Faria, C.F.d.M.; Giergiel, K.; Gniewek, P.; et al. Symphony on strong field approximation. Rep. Prog. Phys. 2019, 82, 116001. [Google Scholar] [CrossRef] [PubMed]
- Ortmann, L. Trajectory-Based Analyses of Ultrafast Strong Field Phenomena. Ph.D. Thesis, Max-Planck-Institüt für Physik komplexer Systeme, Dresden, Germany, 2019. [Google Scholar]
- Freeman, R.R.; Bucksbaum, P.H.; Milchberg, H.; Darack, S.; Schumacher, D.; Geusic, M.E. Above-threshold ionization with subpicosecond laser pulses. Phys. Rev. Lett. 1987, 59, 1092–1095. [Google Scholar] [CrossRef] [PubMed]
- Ammosov, M.V.; Delone, N.B.; Krainov, V.P. Tunnel ionization of complex atoms and atomic ions in electromagnetic field. High Intensity Laser Process 1986, 664, 138–141. [Google Scholar]
- Hernández-García, C.; Vieira, J.; Mendonça, J.T.; Rego, L.; San Román, J.; Plaja, L.; Ribic, P.R.; Gauthier, D.; Picón, A. Generation and Applications of Extreme-Ultraviolet Vortices. Photonics 2017, 4, 28. [Google Scholar] [CrossRef]
- Paufler, W. Strong-field ionization with twisted laser pulses. Phys. Rev. A 2018, 97. [Google Scholar] [CrossRef]
- Fang, Y.; Guo, Z.; Ge, P.; Ma, X.; Han, M.; Yu, X.; Deng, Y.; Gong, Q.; Liu, Y. Strong-field photoionization of intense laser fields by controlling optical singularities. Sci. China Phys. Mech. Astron. 2021, 64, 274211. [Google Scholar] [CrossRef]
- Fang, Y.; Guo, Z.; Ge, P.; Dou, Y.; Deng, Y.; Gong, Q.; Liu, Y. Probing the orbital angular momentum of intense vortex pulses with strong-field ionization. Light. Sci. Appl. 2022, 11, 34. [Google Scholar] [CrossRef] [PubMed]
- Pasquinilli, H.; Schimmoller, A.; Walker, S.; Landsman, A.S. Determining the Orbital Angular Momentum of a Vortex Beam Using Strong Field Ionization. Photonics 2023, 10, 1322. [Google Scholar] [CrossRef]
- Paufler, W.; Böning, B.; Fritzsche, S. High harmonic generation with Laguerre–Gaussian beams. J. Opt. 2019, 21, 094001. [Google Scholar] [CrossRef]
- Toda, Y.; Honda, S.; Morita, R. Dynamics of a paired optical vortex generated by second-harmonic generation. Opt. Express 2010, 18, 17796–17804. [Google Scholar] [CrossRef] [PubMed]
- Zürch, M.; Kern, C.; Hansinger, P.; Dreischuh, A.; Spielmann, C. Strong-field physics with singular light beams. Nat. Phys. 2012, 8, 743–746. [Google Scholar] [CrossRef]
- Gauthier, D.; Ribič, P.R.; Adhikary, G.; Camper, A.; Chappuis, C.; Cucini, R.; DiMauro, L.F.; Dovillaire, G.; Frassetto, F.; Géneaux, R.; et al. Tunable orbital angular momentum in high-harmonic generation. Nat. Commun. 2017, 8, 14971. [Google Scholar] [CrossRef] [PubMed]
- Pandey, A.K.; de las Heras, A.; Larrieu, T.; San Román, J.; Serrano, J.; Plaja, L.; Baynard, E.; Pittman, M.; Dovillaire, G.; Kazamias, S.; et al. Characterization of Extreme Ultraviolet Vortex Beams with a Very High Topological Charge. ACS Photonics 2022, 9, 944–951. [Google Scholar] [CrossRef]
- Lewenstein, M.; Balcou, P.; Ivanov, M.Y.; L’Huillier, A.; Corkum, P.B. Theory of high-harmonic generation by low-frequency laser fields. Phys. Rev. A 1994, 49, 2117–2132. [Google Scholar] [CrossRef] [PubMed]
- Le, A.T.; Wei, H.; Jin, C.; Lin, C.D. Strong-field approximation and its extension for high-order harmonic generation with mid-infrared lasers. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 053001. [Google Scholar] [CrossRef]
- Lewenstein, M.; Salières, P.; L’Huillier, A. Phase of the atomic polarization in high-order harmonic generation. Phys. Rev. A 1995, 52, 4747–4754. [Google Scholar] [CrossRef] [PubMed]
- Corkum, P.B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 1993, 71, 1994–1997. [Google Scholar] [CrossRef] [PubMed]
- Balcou, P.; Salières, P.; L’Huillier, A.; Lewenstein, M. Generalized phase-matching conditions for high harmonics: The role of field-gradient forces. Phys. Rev. A 1997, 55, 3204–3210. [Google Scholar] [CrossRef]
- Rego, L.; Dorney, K.M.; Brooks, N.J.; Nguyen, Q.L.; Liao, C.T.; San Román, J.; Couch, D.E.; Liu, A.; Pisanty, E.; Lewenstein, M.; et al. Generation of extreme-ultraviolet beams with time-varying orbital angular momentum. Science 2019, 364, eaaw9486. [Google Scholar] [CrossRef]
- Paufler, W.; Böning, B.; Fritzsche, S. Tailored orbital angular momentum in high-order harmonic generation with bicircular Laguerre-Gaussian beams. Phys. Rev. A 2018, 98, 011401. [Google Scholar] [CrossRef]
- Dorney, K.M.; Rego, L.; Brooks, N.J.; San Román, J.; Liao, C.T.; Ellis, J.L.; Zusin, D.; Gentry, C.; Nguyen, Q.L.; Shaw, J.M.; et al. Controlling the polarization and vortex charge of attosecond high-harmonic beams via simultaneous spin–orbit momentum conservation. Nat. Photonics 2019, 13, 123–130. [Google Scholar] [CrossRef]
- Rego, L.; Brooks, N.J.; Nguyen, Q.L.D.; Román, J.S.; Binnie, I.; Plaja, L.; Kapteyn, H.C.; Murnane, M.M.; Hernández-García, C. Necklace-structured high-harmonic generation for low-divergence, soft x-ray harmonic combs with tunable line spacing. Sci. Adv. 2022, 8, eabj7380. [Google Scholar] [CrossRef] [PubMed]
- Pazourek, R.; Nagele, S.; Burgdörfer, J. Attosecond chronoscopy of photoemission. Rev. Mod. Phys. 2015, 87, 765–802. [Google Scholar] [CrossRef]
- Wätzel, J.; Berakdar, J. Discerning on a sub-optical-wavelength the attosecond time delays in electron emission from magnetic sublevels by optical vortices. Phys. Rev. A 2016, 94, 033414. [Google Scholar] [CrossRef]
- Giri, S.; Ivanov, M.; Dixit, G. Signatures of the orbital angular momentum of an infrared light beam in the two-photon transition matrix element: A step toward attosecond chronoscopy of photoionization. Phys. Rev. A 2020, 101, 033412. [Google Scholar] [CrossRef]
- Ansari, I.N.; Jadoun, D.S.; Dixit, G. Angle-Resolved Attosecond Streaking of Twisted Attosecond Pulses. arXiv 2020, arXiv:2006.01582. [Google Scholar] [CrossRef]
- Wätzel, J.; Berakdar, J. Spatiotemporal delay in photoionization by polarization-structured laser fields. Phys. Rev. A 2021, 103, 063107. [Google Scholar] [CrossRef]
- Han, M.; Ji, J.B.; Leung, C.S.; Ueda, K.; Wörner, H.J. Separation of photoionization and measurement-induced delays. Sci. Adv. 2024, 10, eadj2629. [Google Scholar] [CrossRef]
- Liang, J.; Han, M.; Liao, Y.; Ji, J.b.; Leung, C.S.; Jiang, W.C.; Ueda, K.; Zhou, Y.; Lu, P.; Wörner, H.J. Attosecond-resolved non-dipole photoionization dynamics. Nat. Photonics 2024, 18, 311–317. [Google Scholar] [CrossRef]
- Rouxel, J.R.; Rösner, B.; Karpov, D.; Bacellar, C.; Mancini, G.F.; Zinna, F.; Kinschel, D.; Cannelli, O.; Oppermann, M.; Svetina, C.; et al. Hard X-ray helical dichroism of disordered molecular media. Nat. Photonics 2022, 16, 570–574. [Google Scholar] [CrossRef]
- Mayer, N.; Ayuso, D.; Decleva, P.; Khokhlova, M.; Pisanty, E.; Ivanov, M.; Smirnova, O. Chiral topological light for detecting robust enantio-sensitive observables. arXiv 2023, arXiv:2303.10932. [Google Scholar] [CrossRef]
- Wanie, V.; Bloch, E.; Månsson, E.P.; Colaizzi, L.; Ryabchuk, S.; Saraswathula, K.; Ordonez, A.F.; Ayuso, D.; Smirnova, O.; Trabattoni, A.; et al. Capturing electron-driven chiral dynamics in UV-excited molecules. Nature 2024, 630, 109–115. [Google Scholar] [CrossRef] [PubMed]
- Quinteiro Rosen, G.F.; Tamborenea, P.I.; Kuhn, T. Interplay between optical vortices and condensed matter. Rev. Mod. Phys. 2022, 94, 035003. [Google Scholar] [CrossRef]
- Hertel, R. Theory of the inverse Faraday effect in metals. J. Magn. Magn. Mater. 2006, 303, L1–L4. [Google Scholar] [CrossRef]
- Ali, S.; Davies, J.R.; Mendonca, J.T. Inverse Faraday Effect with Linearly Polarized Laser Pulses. Phys. Rev. Lett. 2010, 105, 035001. [Google Scholar] [CrossRef] [PubMed]
- Longman, A.; Fedosejevs, R. Kilo-Tesla axial magnetic field generation with high intensity spin and orbital angular momentum beams. Phys. Rev. Res. 2021, 3, 043180. [Google Scholar] [CrossRef]
- Fujita, H.; Sato, M. Ultrafast generation of skyrmionic defects with vortex beams: Printing laser profiles on magnets. Phys. Rev. B 2017, 95, 054421. [Google Scholar] [CrossRef]
- Gao, L.; Prokhorenko, S.; Nahas, Y.; Bellaiche, L. Dynamical Control of Topology in Polar Skyrmions via Twisted Light. Phys. Rev. Lett. 2024, 132, 026902. [Google Scholar] [CrossRef] [PubMed]
- Weisstein, E.W. Spherical Bessel Differential Equation. 2024. Available online: https://mathworld.wolfram.com/SphericalBesselDifferentialEquation.html (accessed on 21 July 2024).
- Griffiths, D.J. Introduction to Electrodynamics; Cambridge University Press: Cambridge, UK, 2023. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schimmoller, A.; Walker, S.; Landsman, A.S. Photonic Angular Momentum in Intense Light–Matter Interactions. Photonics 2024, 11, 871. https://doi.org/10.3390/photonics11090871
Schimmoller A, Walker S, Landsman AS. Photonic Angular Momentum in Intense Light–Matter Interactions. Photonics. 2024; 11(9):871. https://doi.org/10.3390/photonics11090871
Chicago/Turabian StyleSchimmoller, Alex, Spencer Walker, and Alexandra S. Landsman. 2024. "Photonic Angular Momentum in Intense Light–Matter Interactions" Photonics 11, no. 9: 871. https://doi.org/10.3390/photonics11090871
APA StyleSchimmoller, A., Walker, S., & Landsman, A. S. (2024). Photonic Angular Momentum in Intense Light–Matter Interactions. Photonics, 11(9), 871. https://doi.org/10.3390/photonics11090871




