Analysis and Selection of the Optimal Pump Laser Power Density for SERF Co-Magnetometer Used in Rotation Sensing
Abstract
1. Introduction
2. Theory
2.1. Principle of the SERF CM
2.2. AC Response Model and Equivalent Models for Each Frequency Band
2.3. Output Noise Model
3. Experimental Setup
4. Results and Discussion
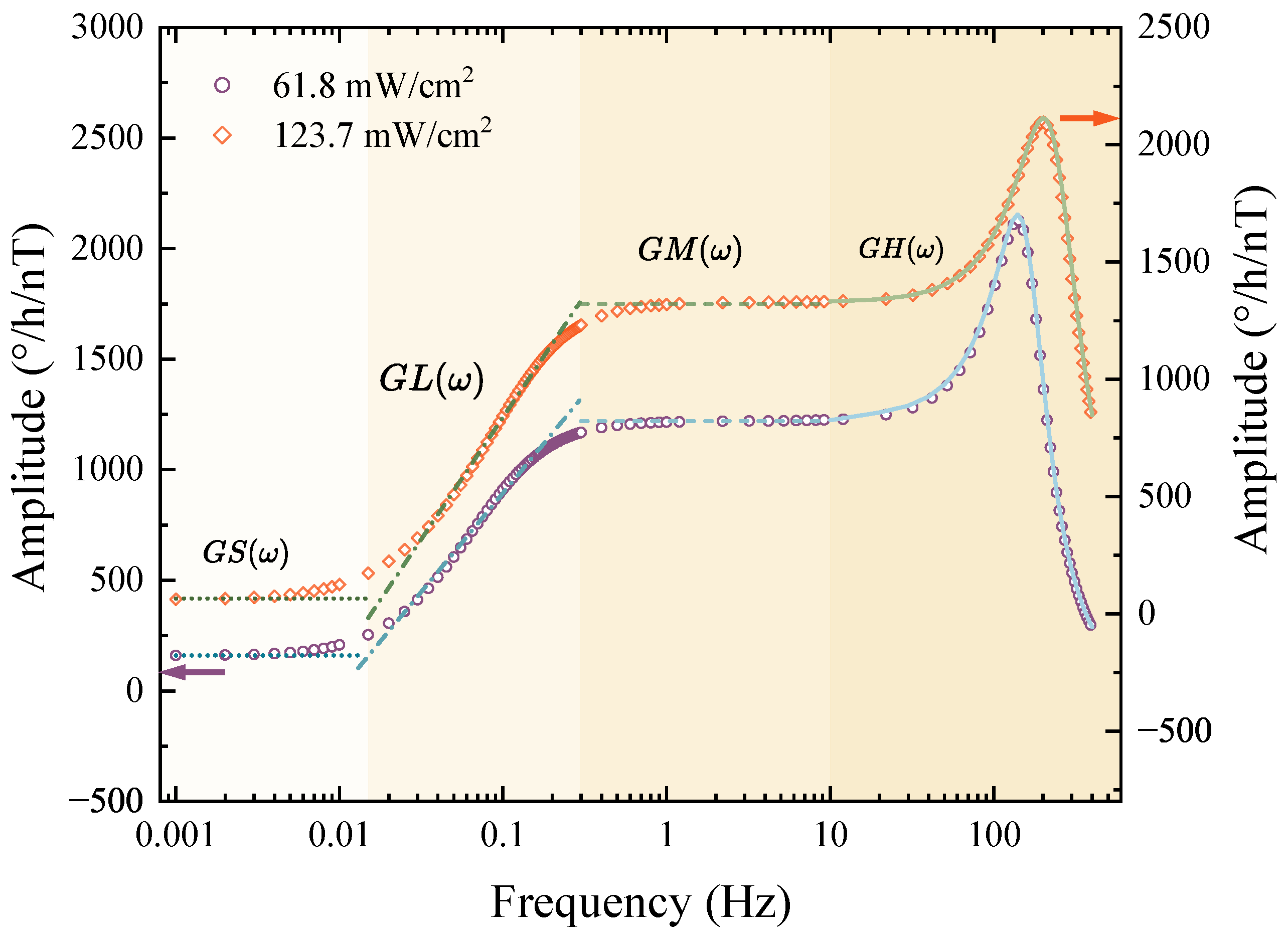
4.1. Parameter Measurements and Equivalent Model Validation
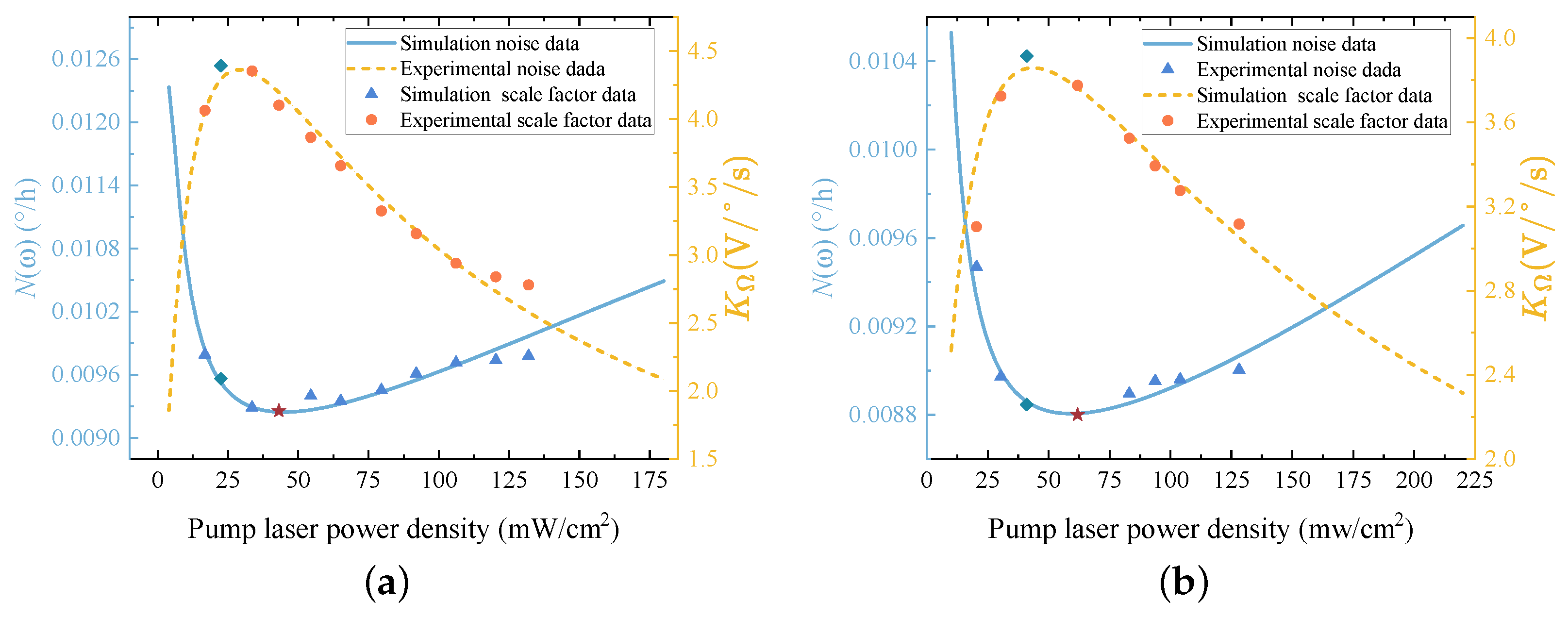
4.2. Minimum Output Noise and the Optimal Pump Laser Power Density
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Flambaum, V.V.; Romalis, M.V. Limits on Lorentz Invariance Violation from Coulomb Interactions in Nuclei and Atoms. Phys. Rev. Lett. 2017, 118, 142501.1–142501.4. [Google Scholar] [CrossRef] [PubMed]
- Terrano, W.A.; Romalis, M.V. Comagnetometer probes of dark matter and new physics. Quantum Sci. Technol. 2021, 7, 014001. [Google Scholar]
- Wang, Z.; Peng, X.; Zhang, R.; Luo, H.; Guo, H. Single-Species Atomic Comagnetometer Based on 87Rb Atoms. Phys. Rev. Lett. 2020, 124, 193002. [Google Scholar] [CrossRef]
- Wei, K.; Ji, W.; Fu, C.; Wickenbrock, A.; Flambaum, V.V.; Fang, J.; Budker, D. Constraints on exotic spin-velocity-dependent interactions. Nat. Commun. 2022, 13, 7387. [Google Scholar] [CrossRef] [PubMed]
- Shi, M. Investigation on magnetic field response of a 87Rb- 129Xe atomic spin comagnetometer. Opt. Express 2020, 28, 32033. [Google Scholar] [CrossRef]
- Pei, H.; Duan, L.; Ma, L.; Fan, S.; Cai, Z.; Wu, Z.; Fan, W.; Quan, W. Real-time quantum control of spin-coupling damping and application in atomic spin gyroscopes. Cell Rep. Phys. Sci. 2024, 5, 101832. [Google Scholar] [CrossRef]
- Kornack, T.W.; Ghosh, R.K.; Romalis, M.V. Nuclear Spin Gyroscope Based on an Atomic Comagnetometer. Phys. Rev. Lett. 2005, 95, 230801. [Google Scholar] [CrossRef]
- Fang, J.; Wan, S.; Yuan, H. Dynamics of an all-optical atomic spin gyroscope. Appl. Opt. 2013, 52, 7220–7227. [Google Scholar] [CrossRef]
- Ledbetter, M.P.; Savukov, I.M.; Acosta, V.M.; Budker, D.; Romalis, M.V. Spin-exchange relaxation free magnetometry with Cs vapor. Phys. Rev. 2007, 77, 1012–1015. [Google Scholar] [CrossRef]
- Fang, J.; Chen, Y.; Zou, S.; Liu, X.; Hu, Z.; Quan, W.; Yuan, H.; Ding, M. Low frequency magnetic field suppression in an atomic spin co-magnetometer with a large electron magnetic field. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 065006. [Google Scholar] [CrossRef]
- Chen, Y.; Quan, W.; Duan, L.; Lu, Y.; Jiang, L.; Fang, J. Spin-exchange collision mixing of the K and Rb ac Stark shifts. Phys. Rev. A 2016, 94, 052705. [Google Scholar] [CrossRef]
- Fan, W.; Quan, W.; Liu, F.; Pang, H.; Xing, L.; Liu, G. Performance of Low-Noise Ferrite Shield in a K-Rb-21Ne Co-Magnetometer. IEEE Sens. J. 2020, 20, 2543–2549. [Google Scholar] [CrossRef]
- Li, R.; Quan, W.; Fan, W.; Xing, L.; Fang, J. Influence of magnetic fields on the bias stability of atomic gyroscope operated in spin-exchange relaxation-free regime. Sens. Actuators A Phys. 2017, 266, 130–134. [Google Scholar] [CrossRef]
- Fu, Y.; Fan, W.; Ruan, J.; Liu, Y.; Lu, Z.; Quan, W. Effects of Probe Laser Intensity on Co-Magnetometer Operated in Spin-Exchange Relaxation-Free Regime. IEEE Trans. Instrum. Meas. 2022, 71, 9501607. [Google Scholar] [CrossRef]
- Jiang, L.; Quan, W.; Liang, Y.; Liu, J.; Duan, L.; Fang, J. Effects of pump laser power density on the hybrid optically pumped comagnetometer for rotation sensing. Opt. Express 2019, 27, 27420–27430. [Google Scholar] [CrossRef]
- Zhao, T.; Zhai, Y.; Liu, C.; Xie, H.; Cao, Q.; Fang, X. Spin polarization characteristics of hybrid optically pumped comagnetometers with different density ratios. Opt. Express 2022, 30, 28067–28078. [Google Scholar] [CrossRef]
- Liu, Y.; Fan, W.; Fu, Y.; Pang, H.; Pei, H.; Quan, W. Suppression of Low-Frequency Magnetic Drift Based on Magnetic Field Sensitivity in K-Rb-21Ne Atomic Spin Comagnetometer. IEEE Trans. Instrum. Meas. 2022, 71, 13361–13371. [Google Scholar] [CrossRef]
- Fu, Y.; Fan, W.; Ruan, J.; Liu, Y.; Wang, Z.; Zhou, X.; Quan, W. Analysis on the effect of electron spin polarization on a hybrid optically pumped K-Rb-21Ne co-magnetometer. Opt. Express 2022, 30, 42114–42128. [Google Scholar] [CrossRef]
- Fan, W.; Quan, W.; Liu, F.; Xing, L.; Liu, G. Suppression of the Bias Error Induced by Magnetic Noise in a Spin-Exchange Relaxation-Free Gyroscope. IEEE Sens. J. 2019, 19, 9712–9721. [Google Scholar] [CrossRef]
- Wu, Z.; Pang, H.; Wang, Z.; Fan, W.; Liu, F.; Liu, Y.; Quan, W. A New Design Method for a Triaxial Magnetic Field Gradient Compensation System Based on Ferromagnetic Boundary. IEEE Trans. Ind. Electron. 2024, 71, 13361–13371. [Google Scholar] [CrossRef]
- Kornack, T.W.; Romalis, M.V. Dynamics of two overlapping spin ensembles interacting by spin exchange—Art. no. 253002. Phys. Rev. Lett. 2002, 89, 253002. [Google Scholar] [CrossRef]
- Wei, K.; Zhao, T.; Fang, X.; Li, H.; Quan, W. Simultaneous Determination of the Spin Polarizations of Noble-Gas and Alkali-Metal Atoms Based on the Dynamics of the Spin Ensembles. Phys. Rev. Appl. 2020, 13, 044027. [Google Scholar] [CrossRef]
- Cates, G.D.; Schaefer, S.R.; Happer, W. Relaxation of spins due to field inhomogeneities in gaseous samples at low magnetic fields and low pressures. Phys. Rev. A 1988, 37, 2877. [Google Scholar] [CrossRef]
- Romalis, M.V.; Cates, G.D. Accurate 3He polarimetry using the Rb Zeeman frequency shift due to the Rb-3He spin-exchange collisions. Phys. Rev. A 1998, 58, 3004. [Google Scholar] [CrossRef]
- Romalis, M.V. Hybrid Optical Pumping of Optically Dense Alkali-Metal Vapor without Quenching Gas. Phys. Rev. Lett. 2010, 105, 243001. [Google Scholar] [CrossRef]
- Fan, W.; Quan, W.; Zhang, W.; Xing, L.; Liu, G. Analysis on the Magnetic Field Response for Nuclear Spin Co-Magnetometer Operated in Spin-Exchange Relaxation-Free Regime. IEEE Access 2019, 7, 28574–28580. [Google Scholar] [CrossRef]
- Fu, Y.; Sun, J.; Ruan, J.; Quan, W. A nanocrystalline shield for high precision co-magnetometer operated in spin-exchange relaxation-free regime. Sens. Actuators A Phys. 2022, 339, 113487. [Google Scholar] [CrossRef]
- Pei, H.; Yu, W.; Fan, W.; Du, P.; Quan, W. Bandwidth Expansion of Atomic Spin Gyroscope With Transient Response. IEEE Trans. Instrum. Meas. 2022, 71, 1502308. [Google Scholar] [CrossRef]
- Xu, Z.; Wei, K.; Zhao, T.; Cao, Q.; Liu, Y.; Hu, D.; Zhai, Y. Fast Dynamic Frequency Response-Based Multiparameter Measurement in Spin-Exchange Relaxation-Free Comagnetometers. IEEE Trans. Instrum. Meas. 2021, 70, 7007110. [Google Scholar] [CrossRef]
- Lu, Y.; Zhai, Y.; Fan, W.; Zhang, Y.; Xing, L.; Jiang, L.; Quan, W. Nuclear magnetic field measurement of the spin-exchange optically pumped noble gas in a self-compensated atomic comagnetometer. Opt. Express 2020, 28, 17683–17696. [Google Scholar] [CrossRef]
- Liu, J.; Jiang, L.; Liang, Y.; Liu, F.; Quan, W. A Fast Measurement for Relaxation Rates and Fermi-Contact Fields in Spin-Exchange Relaxation-Free Comagnetometers. IEEE Trans. Instrum. Meas. 2020, 69, 7805–7812. [Google Scholar] [CrossRef]
- Yuan, L.; Huang, J.; Fan, W.; Wang, Z.; Zhang, K.; Pei, H.; Cai, Z.; Gao, H.; Liu, S.; Quan, W. Measurement and analysis of polarization gradient relaxation in the atomic comagnetometer. Measurement 2023, 217, 113043. [Google Scholar] [CrossRef]



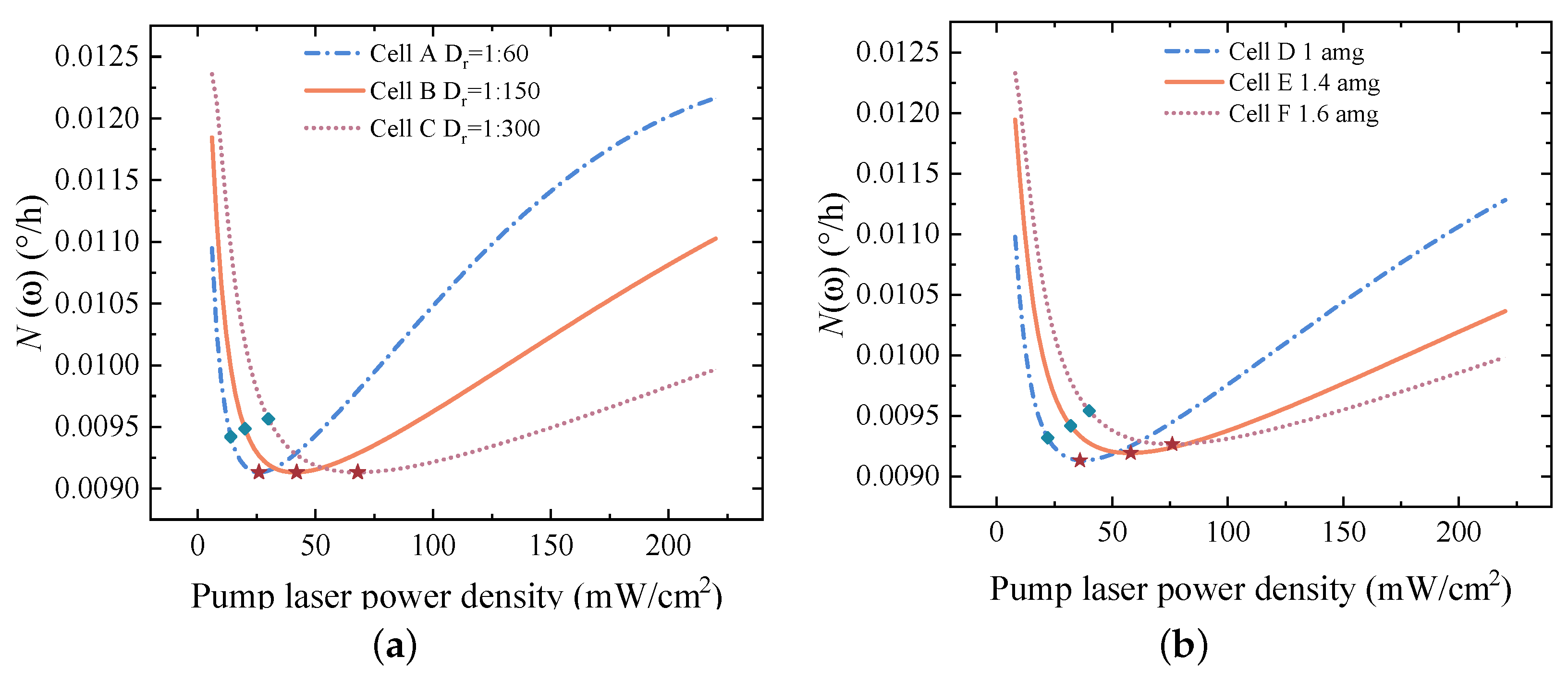
| Cell Number | Density Ratio at 180 °C | Cell Pressure (amg) |
|---|---|---|
| Cell A | 1:60 | 1 |
| Cell B | 1:150 | 1 |
| Cell C | 1:300 | 1 |
| Cell Number | Density Ratio at 180 °C | Cell Pressure (amg) |
|---|---|---|
| Cell D | 1:80 | 1 |
| Cell E | 1:80 | 1.4 |
| Cell F | 1:80 | 1.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Yuan, L.; Cai, Z.; Gao, H.; Wang, R.; Du, P.; Zhou, X. Analysis and Selection of the Optimal Pump Laser Power Density for SERF Co-Magnetometer Used in Rotation Sensing. Photonics 2024, 11, 835. https://doi.org/10.3390/photonics11090835
Zhang K, Yuan L, Cai Z, Gao H, Wang R, Du P, Zhou X. Analysis and Selection of the Optimal Pump Laser Power Density for SERF Co-Magnetometer Used in Rotation Sensing. Photonics. 2024; 11(9):835. https://doi.org/10.3390/photonics11090835
Chicago/Turabian StyleZhang, Kai, Linlin Yuan, Ze Cai, Hang Gao, Rui Wang, Pengcheng Du, and Xinxiu Zhou. 2024. "Analysis and Selection of the Optimal Pump Laser Power Density for SERF Co-Magnetometer Used in Rotation Sensing" Photonics 11, no. 9: 835. https://doi.org/10.3390/photonics11090835
APA StyleZhang, K., Yuan, L., Cai, Z., Gao, H., Wang, R., Du, P., & Zhou, X. (2024). Analysis and Selection of the Optimal Pump Laser Power Density for SERF Co-Magnetometer Used in Rotation Sensing. Photonics, 11(9), 835. https://doi.org/10.3390/photonics11090835





