Real-Time Massive Parallel Generation of Physical Random Bits Using Weak-Resonant-Cavity Fabry-Perot Laser Diodes
Abstract
1. Introduction
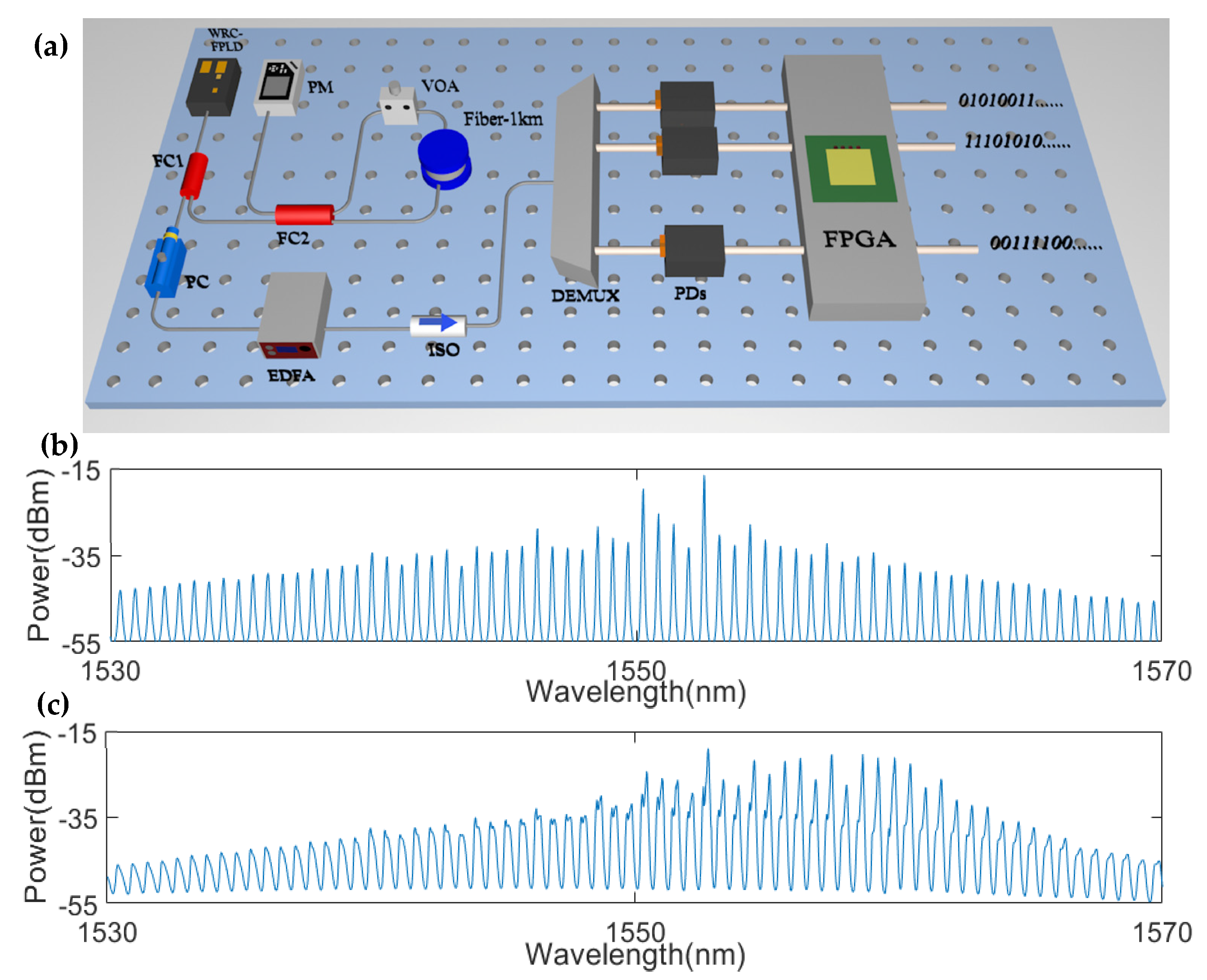
2. Experimental Setup
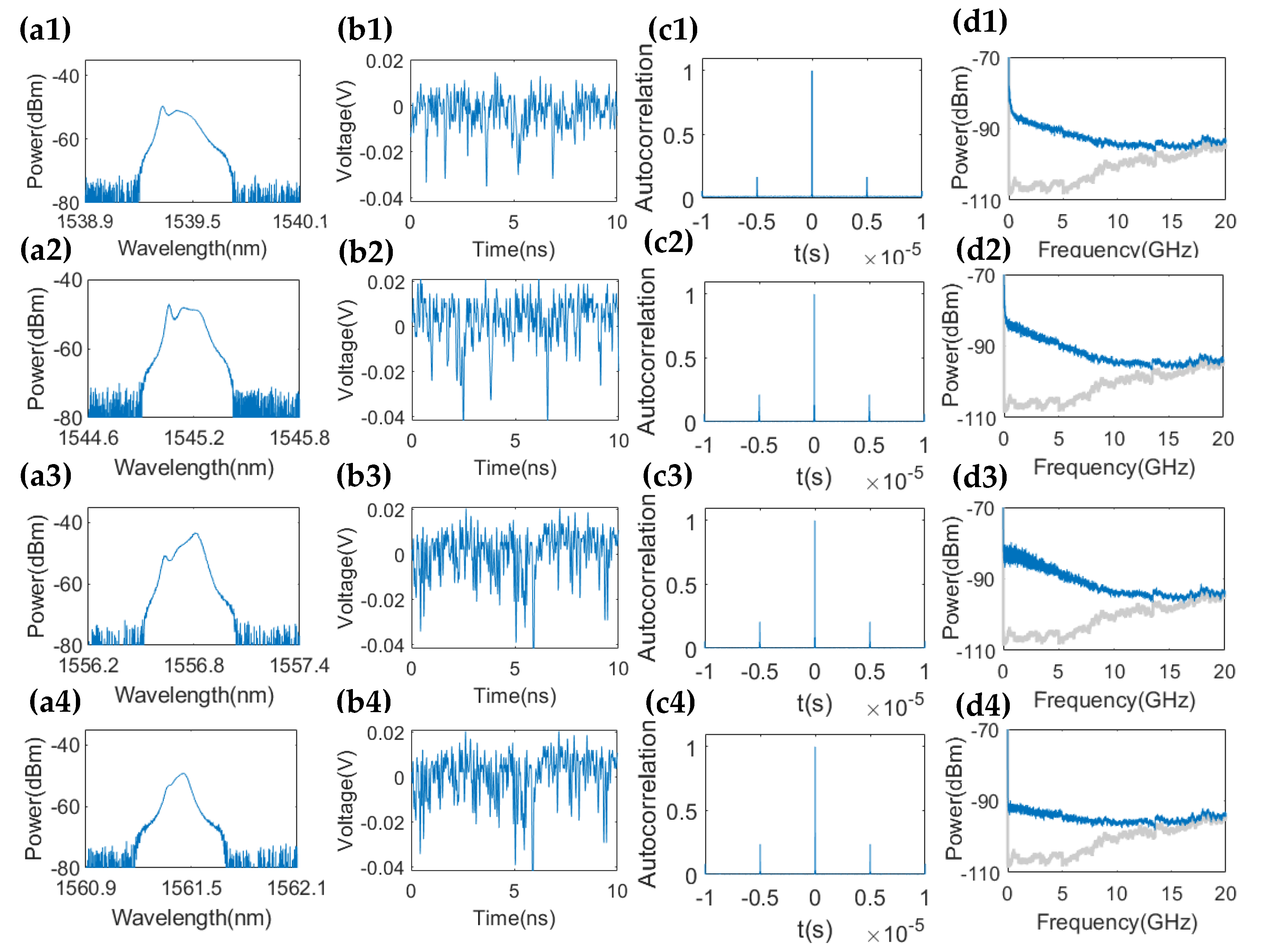
3. Analysis of Chaotic Characteristics of Chaotic Combs
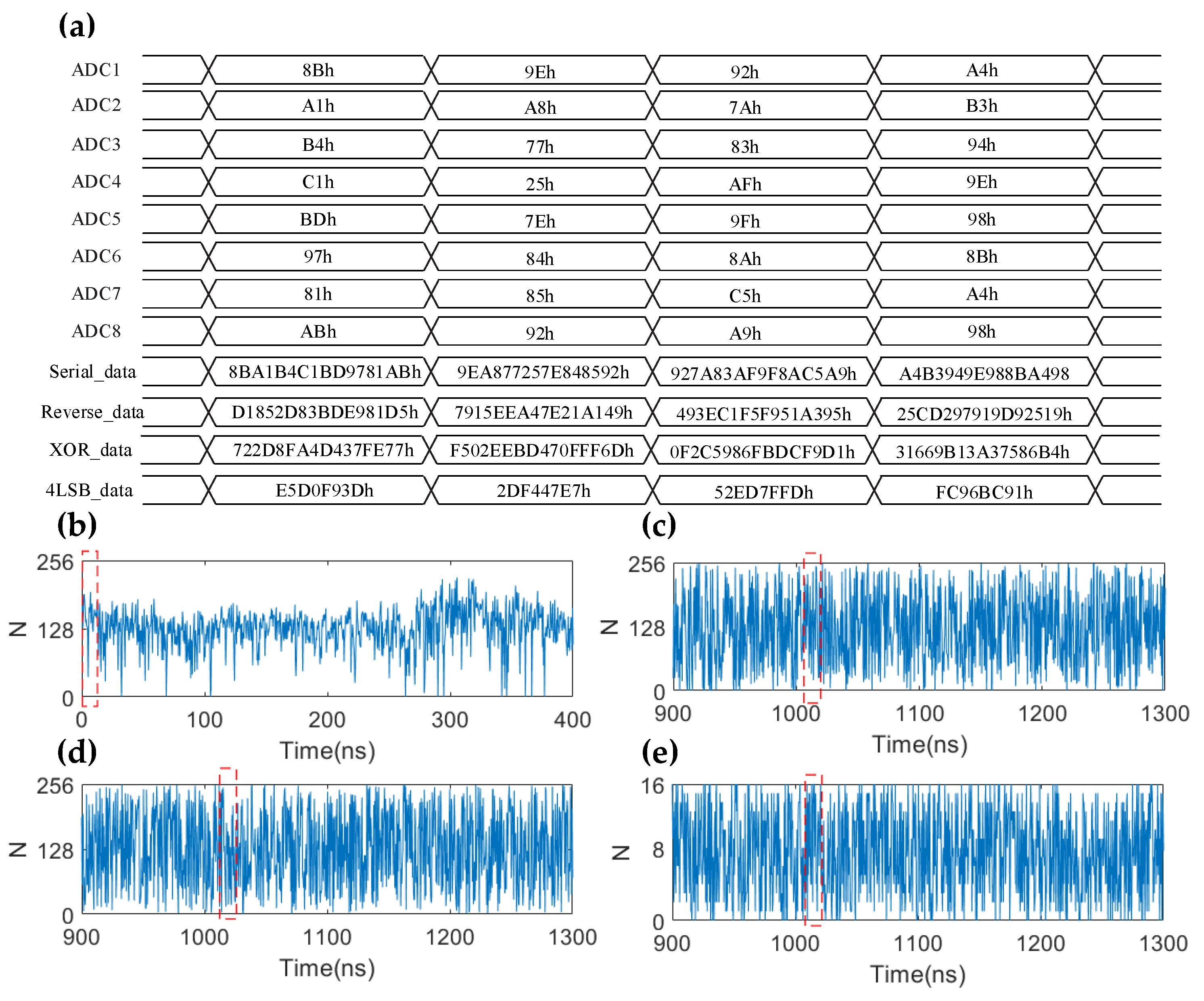
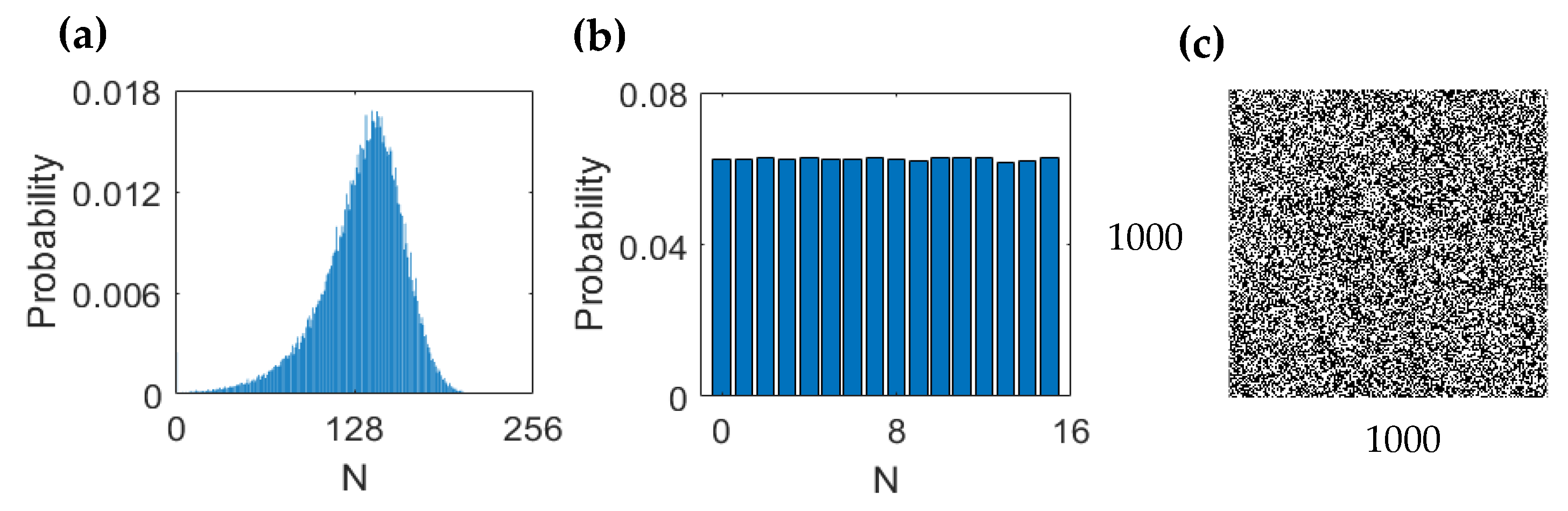
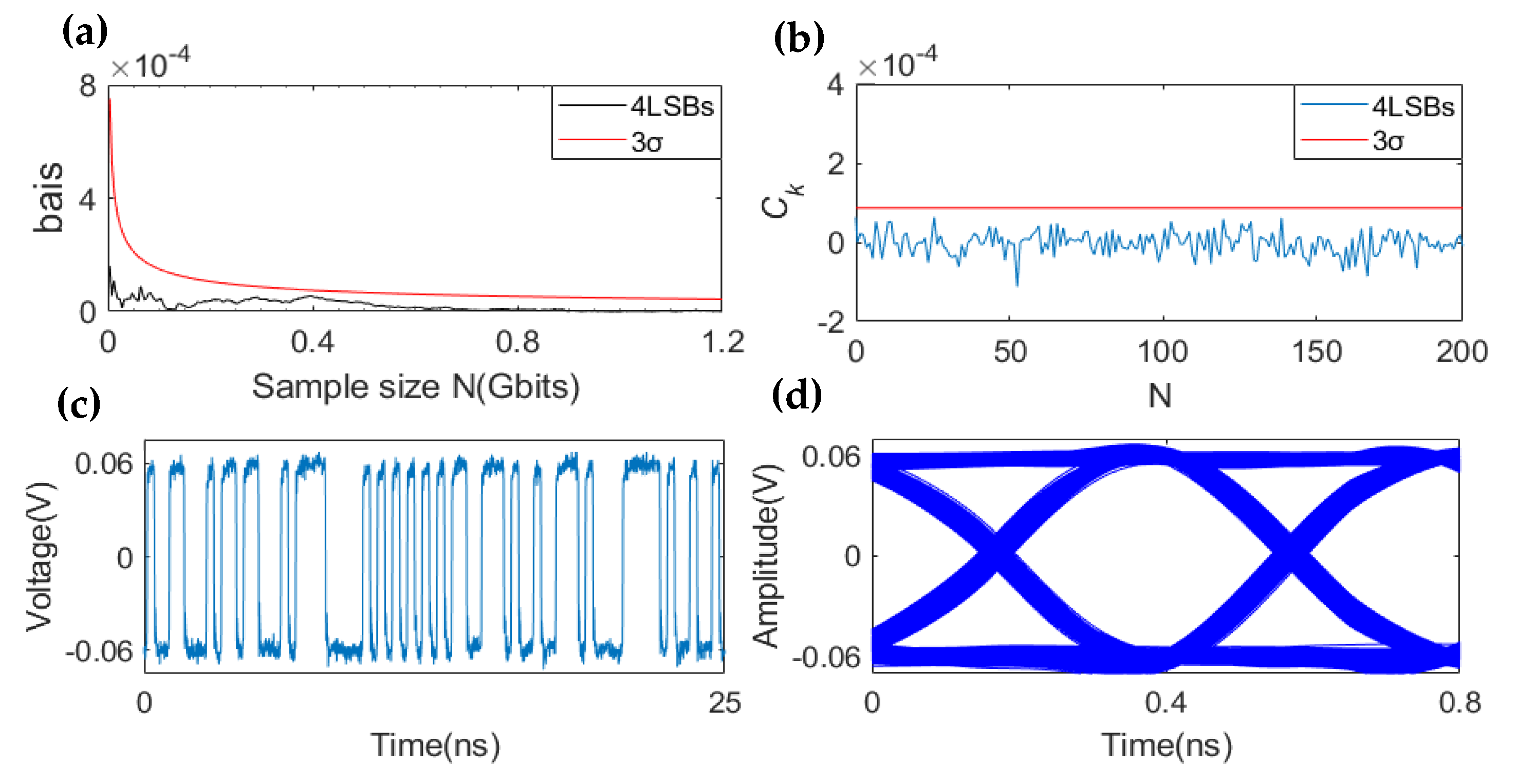
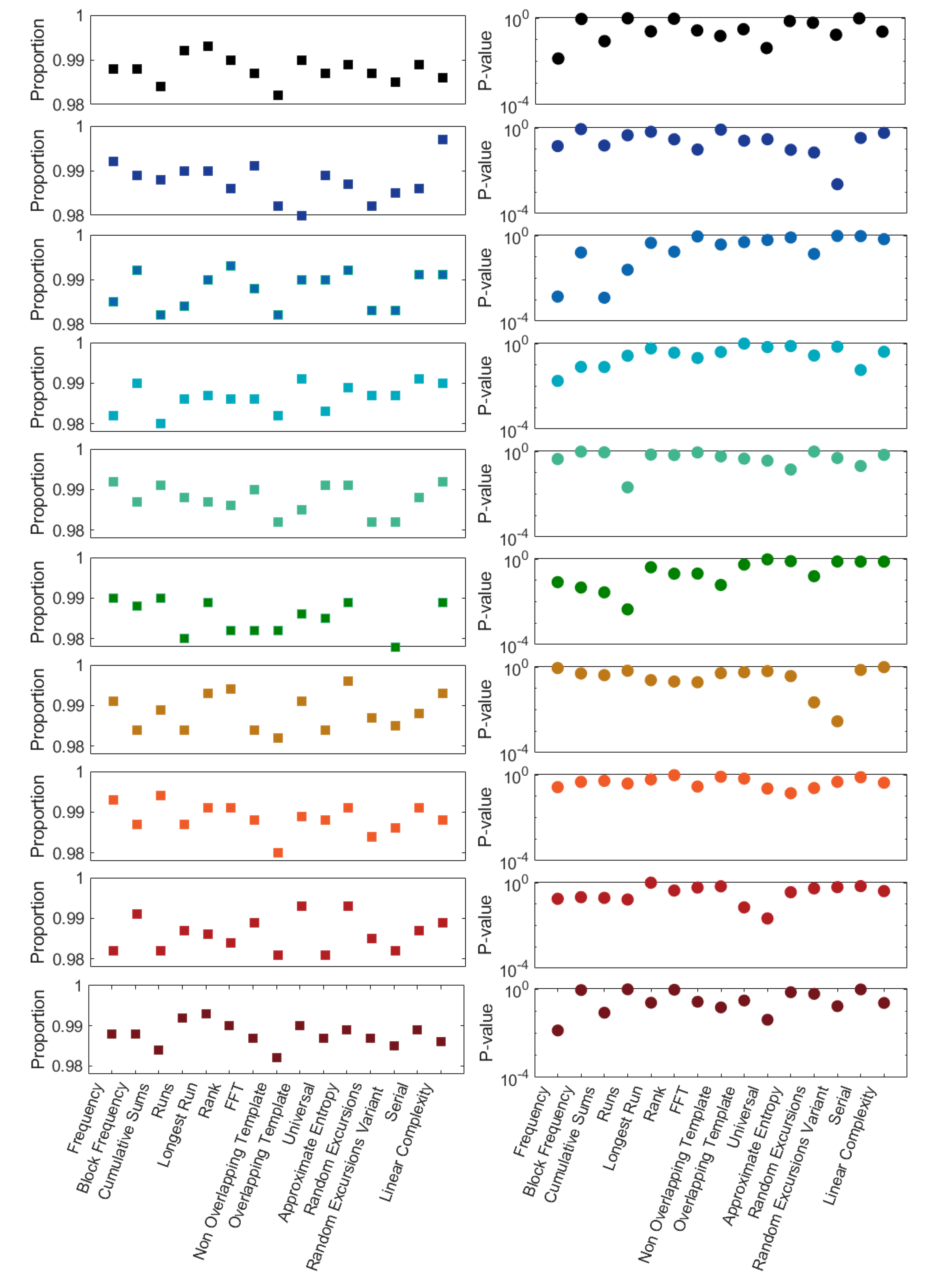
4. PRB Extraction and Evaluation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vicente, R.; Mirasso, C.-R.; Fischer, I. Simultaneous bidirectional message transmission in a chaos-based communication scheme. Opt. Lett. 2007, 32, 403–405. [Google Scholar] [CrossRef] [PubMed]
- Sciamanna, M.; Shore, K.-A. Physics and applications of laser diode chaos. Nat. Photonics 2015, 9, 151–162. [Google Scholar] [CrossRef]
- Li, P.; Li, Z.; Halang, W.-A.; Chen, G. A stream cipher based on a spatiotemporal chaotic system. Chaos Solitons Fractals 2007, 32, 1867–1876. [Google Scholar] [CrossRef]
- Blum, L.; Blum, M.; Shub, M. A simple unpredictable pseudo-random number generator. SIAM J. Comput. 1986, 15, 364–383. [Google Scholar] [CrossRef]
- Eichenaur, B.-J.; Lehn, J.; Topuzoglu, A. A nonlinera congruential pseudo-random number generator with power of two modulus. Math. Comput. 1998, 51, 757–759. [Google Scholar] [CrossRef]
- Uchida, A.; Amano, K.; Inoue, K.; Hirano, M.; Naito, S.; Someya, H.; Oowada, I.; Kurashige, T.; Shiki, M.; Yoshimori, S.; et al. Fast physical random bit generation with chaotic semiconductor lasers. Nat. Photonics 2008, 2, 728–732. [Google Scholar] [CrossRef]
- Reidler, I.; Aviad, Y.; Rosenbluh, M.; Kanter, I. Ultrahigh-speed random number generation based on a chaotic semiconductor laser. Phys. Rev. Lett. 2009, 103, 024102. [Google Scholar] [CrossRef]
- Hirano, K.; Yamazaki, T.; Morikatsu, S.; Okumura, H.; Aida, H.; Uchida, A.; Yoshimori, S.; Yoshimura, K.; Harayama, T.; Davis, P. Fast random bit generation with bandwidth-enhanced chaos in semiconductor lasers. Opt. Express 2010, 18, 5512–5524. [Google Scholar] [CrossRef] [PubMed]
- Kanter, I.; Aviad, Y.; Reidler, I.; Cohen, E.; Rosenbluh, M. An optical ultrafast random bit generator. Nat. Photonics 2010, 4, 58–61. [Google Scholar] [CrossRef]
- Sakuraba, R.; Iwakawa, K.; Kanno, K.; Uchida, A. Tb/s physical random bit generation with bandwidth-enhanced chaos in three-cascaded semiconductor lasers. Opt. Express 2015, 23, 1470–1490. [Google Scholar] [CrossRef]
- Zhang, L.; Pan, B.; Chen, G.; Guo, L.; Lu, D.; Zhao, L.; Wang, W. 640-Gbit/s fast physical random number generation using a broadband chaotic semiconductor laser. Sci. Rep. 2017, 7, 45900. [Google Scholar] [CrossRef]
- Li, P.; Wang, Y.-C.; Zhang, J.-Z. All-optical fast random number generator. Opt. Express 2010, 18, 20360–20369. [Google Scholar] [CrossRef] [PubMed]
- Li, X.-Z.; Chan, S.-C. Heterodyne random bit generation using an optically injected semiconductor laser in chaos. IEEE J. Quantum Electron. 2013, 49, 829–838. [Google Scholar] [CrossRef]
- Takahashi, R.; Akizawa, Y.; Uchida, A.; Harayama, T.; Tsuzuki, K.; Sunada, S.; Arai, K.; Yoshimura, K.; Davis, P. Fast physical random bit generation with photonic integrated circuits with different external cavity lengths for chaos generation. Opt. Express 2014, 22, 11727–11740. [Google Scholar] [CrossRef]
- Ran, C.; Tang, X.; Wu, Z.-M.; Xia, G.-Q. Dual-channel physical random bits generation by a master-slave vertical-cavity surface-emitting lasers chaotic system. Laser Phys. 2018, 28, 126202. [Google Scholar] [CrossRef]
- Li, X.-Z.; Chan, S.-C. Random bit generation using an optically injected semiconductor laser in chaos with oversampling. Opt. Lett. 2012, 37, 2163–2165. [Google Scholar] [CrossRef] [PubMed]
- Shi, B.; Luo, C.; Flores, J.-G.-F.; Lo, G.; Kwong, D.-L.; Wu, J.; Wong, C.-W. Gbps physical random bit generation based on the mesoscopic chaos of a silicon photonics crystal microcavity. Opt. Express 2020, 28, 36685–36695. [Google Scholar] [CrossRef] [PubMed]
- Ge, Z.; Xiao, T.; Hao, T.; Li, W.; Li, M. Tb/s fast random bit generation based on a broadband random optoelectronic oscillator. IEEE Photonics Technol. Lett. 2021, 33, 1223–1226. [Google Scholar] [CrossRef]
- Virte, M.; Mercier, E.; Thienpont, H.; Panajotov, K.; Sciamanna, M. Physical random bit generation from chaotic solitary laser diode. Opt. Express 2014, 22, 17271–17280. [Google Scholar] [CrossRef]
- Oliver, N.; Soriano, M.-C.; Sukow, D.-W.; Fischer, I. Dynamics of a semiconductor laser with polarization-rotated feedback and its utilization for random bit generation. Opt. Lett. 2011, 36, 4632–4634. [Google Scholar] [CrossRef]
- Akizawa, Y.; Yamazaki, T.; Uchida, A.; Harayama, T.; Sunada, S.; Arai, K.; Yoshimura, K.; Davis, P. Fast random number generation with bandwidth-enhanced chaotic semiconductor lasers at 8 × 50 Gb/s. IEEE Photonics Technol. Lett. 2012, 24, 1042–1044. [Google Scholar] [CrossRef]
- Argyris, A.; Deligiannidis, S.; Pikasis, E.; Bogris, A.; Syvridis, D. Implementation of 140 Gb/s true random bit generator based on a chaotic photonic integrated circuit. Opt. Express 2010, 18, 18763–18768. [Google Scholar] [CrossRef]
- Qiao, L.-J.; Lv, T.-S.; Xu, Y.; Zhang, M.-J.; Wang, T.; Zhou, R.-K.; Wang, Q.; Xu, H.-C. Generation of flat wideband chaos based on mutual injection of semiconductor lasers. Opt. Lett. 2019, 44, 5394–5397. [Google Scholar] [CrossRef]
- Wu, J.-G.; Tang, X.; Wu, Z.-M.; Xia, G.-Q.; Feng, G.-Y. Parallel generation of 10 Gbits/s physical random number streams using chaotic semiconductor lasers. Laser Phys. 2012, 22, 1476–1480. [Google Scholar] [CrossRef]
- Tang, X.; Wu, Z.-M.; Wu, J.-G.; Deng, T.; Chen, J.J.; Fan, L.; Zhong, Q.-Z.; Xia, G.-Q. Tbits/s physical random bit generation based on mutually coupled semiconductor laser chaotic entropy source. Opt. Express 2015, 23, 33130–33141. [Google Scholar] [CrossRef]
- Ji, X.-C.; Yao, X.-W.; Klenner, A.; Gan, Y.; Gaeta, A.-L.; Hendon, C.-P.; Lipson, M. Chip-based frequency comb sources for optical coherence tomography. Opt. Express 2019, 27, 19896–19905. [Google Scholar] [CrossRef] [PubMed]
- Marchand, P.-J.; Riemensberger, J.; Skehan, J.-C.; Ho, J.-J.; Pfeiffer, M.-H.-P.; Liu, J.-Q.; Hauger, C.; Lasser, T.; Kippenberg, T.-J. Soliton microcomb based spectral domain optical coherence tomography. Nat. Commun. 2021, 12, 427. [Google Scholar] [CrossRef] [PubMed]
- Shen, B.-T.; Shu, H.-W.; Xie, W.-Q.; Chen, R.-X.; Liu, Z.; Ge, Z.-F.; Zhang, X.-G.; Wang, Y.-M.; Zhang, Y.-H.; Cheng, B.-W.; et al. Harnessing microcomb-based parallel chaos for random number generation and optical decision making. Nat. Commun. 2023, 14, 4590. [Google Scholar] [CrossRef] [PubMed]
- Xiao, Z.-Y.; Li, T.; Cai, T.; Zhang, H.; Huang, Y.; Li, C.; Yao, B.; Wu, K.; Chen, J. Near-zero-dispersion soliton and broadband modulational instability Kerr microcombs in anomalous dispersion. Light Sci. Appl. 2023, 12, 33. [Google Scholar] [CrossRef]
- Hu, C.-X.; Xia, G.-Q.; Jiang, Z.-F.; Yue, D.-Z.; Yang, W.-Y.; Lin, G.-R.; Wu, Z.-M. Simultaneous Generation of Multi-Channel Broadband Chaotic Signals Based on Two Unidirectionally Coupled WRC-FPLDs. IEEE Photonics J. 2020, 12, 1504008. [Google Scholar] [CrossRef]
- Tang, X.; Xia, G.-Q.; Ran, C.; Deng, T.; Lin, X.-D.; Fan, L.; Gao, Z.-Y.; Lin, G.-R.; Wu, Z.-M. Fast Physical Random Bit Generation Based on a Broadband Chaotic Entropy Source Originated from a Filtered Feedback WRC-FPLD. IEEE Photonics J. 2019, 11, 7800710. [Google Scholar] [CrossRef]
- Yin, Z.-S.; Wang, F.; Deng, T.; Hu, D.-W.; Xia, G.-Q.; Wu, Z.-M. Multi-channel chaotic signal generation using a weak resonant cavity Fabry–Perot laser diode subject to self-phase-modulated feedback. Laser Phys. Lett. 2023, 20, 115802. [Google Scholar] [CrossRef]
- Wang, L.-S.; Zhao, T.; Wang, D.-M.; Wu, D.-Y.; Zhou, L.; Wu, J.; Liu, X.-Y.; Wang, Y.-C. Real-Time 14-Gbps Physical Random Bit Generator Based on Time-Interleaved Sampling of Broadband White Chaos. IEEE Photonics J. 2017, 9, 7201412. [Google Scholar] [CrossRef]
- Lin, Y.; Wang, F.; Liu, B. Random number generators for large-scale parallel Monte Carlo simulations on FPGA. J. Comput. Phys. 2018, 360, 93–103. [Google Scholar] [CrossRef]
- Yang, J.; Liu, J.-N.; Su, Q.; Li, Z.-Y.; Fan, F.; Xu, B.-J.; Guo, H. 5.4 Gbps real time quantum random number generator with simple implementation. Opt. Express 2016, 24, 27475–27481. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.-G.; Nie, Y.-Q.; Zhou, H.-Y.; Liang, H.; Ma, X.-F.; Zhang, J.; Pan, J.-W. Fully integrated 3.2 Gbps quantum random number generator with real-time extraction. Rev. Sci. Instrum. 2016, 87, 076102. [Google Scholar] [CrossRef]
- Xu, Y.-P.; Lu, P.; Mihailov, S.; Bao, X.-Y. Real-time physical random bit generation at Gbps based on random fiber lasers. Opt. Lett. 2017, 42, 4796–4799. [Google Scholar] [CrossRef] [PubMed]
- Ugagin, K.; Terashime, Y.; Iwakawa, K.; Uchida, A.; Harayama, T.; Yoshimura, K.; Inubushi, M. Real-time fast physical random number generator with a photonic integrated circuit. Opt. Express 2017, 25, 6511–6523. [Google Scholar] [CrossRef]
- Li, N.; Kim, B.; Chizhevsky, V.-N.; Locquet, A.; Bloch, M.; Citrin, D.-S.; Pan, W. Two approaches for ultrafast random bit generation based on the chaotic dynamics of a semiconductor laser. Opt. Express 2014, 22, 6634–6646. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Tang, X.; Wu, Z.; Wu, J.; Xia, G. Real-Time Massive Parallel Generation of Physical Random Bits Using Weak-Resonant-Cavity Fabry-Perot Laser Diodes. Photonics 2024, 11, 759. https://doi.org/10.3390/photonics11080759
Wang Y, Tang X, Wu Z, Wu J, Xia G. Real-Time Massive Parallel Generation of Physical Random Bits Using Weak-Resonant-Cavity Fabry-Perot Laser Diodes. Photonics. 2024; 11(8):759. https://doi.org/10.3390/photonics11080759
Chicago/Turabian StyleWang, Yongbo, Xi Tang, Zhengmao Wu, Jiagui Wu, and Guangqiong Xia. 2024. "Real-Time Massive Parallel Generation of Physical Random Bits Using Weak-Resonant-Cavity Fabry-Perot Laser Diodes" Photonics 11, no. 8: 759. https://doi.org/10.3390/photonics11080759
APA StyleWang, Y., Tang, X., Wu, Z., Wu, J., & Xia, G. (2024). Real-Time Massive Parallel Generation of Physical Random Bits Using Weak-Resonant-Cavity Fabry-Perot Laser Diodes. Photonics, 11(8), 759. https://doi.org/10.3390/photonics11080759




