Three-Shot Dual-Frequency Fringe Scheme Based on Spatial Computer-Generated Moiré Fringe
Abstract
1. Introduction
2. Principle
2.1. Principle of SCGMP
2.2. Principle of the Proposed Method
2.2.1. Absolute Phase Retrieval
2.2.2. Analysis of Basic Phase Acquisition Conditions
3. Experiments
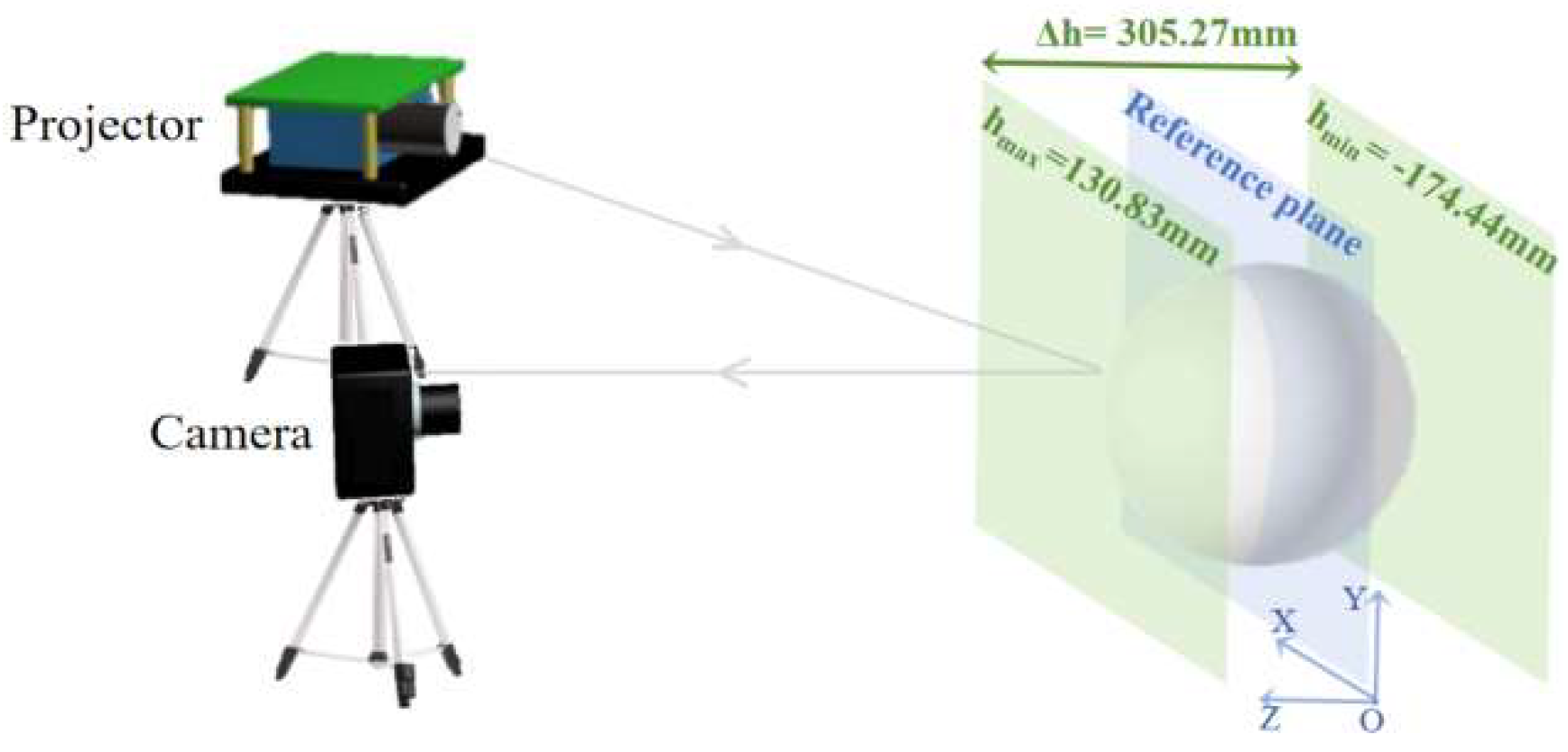
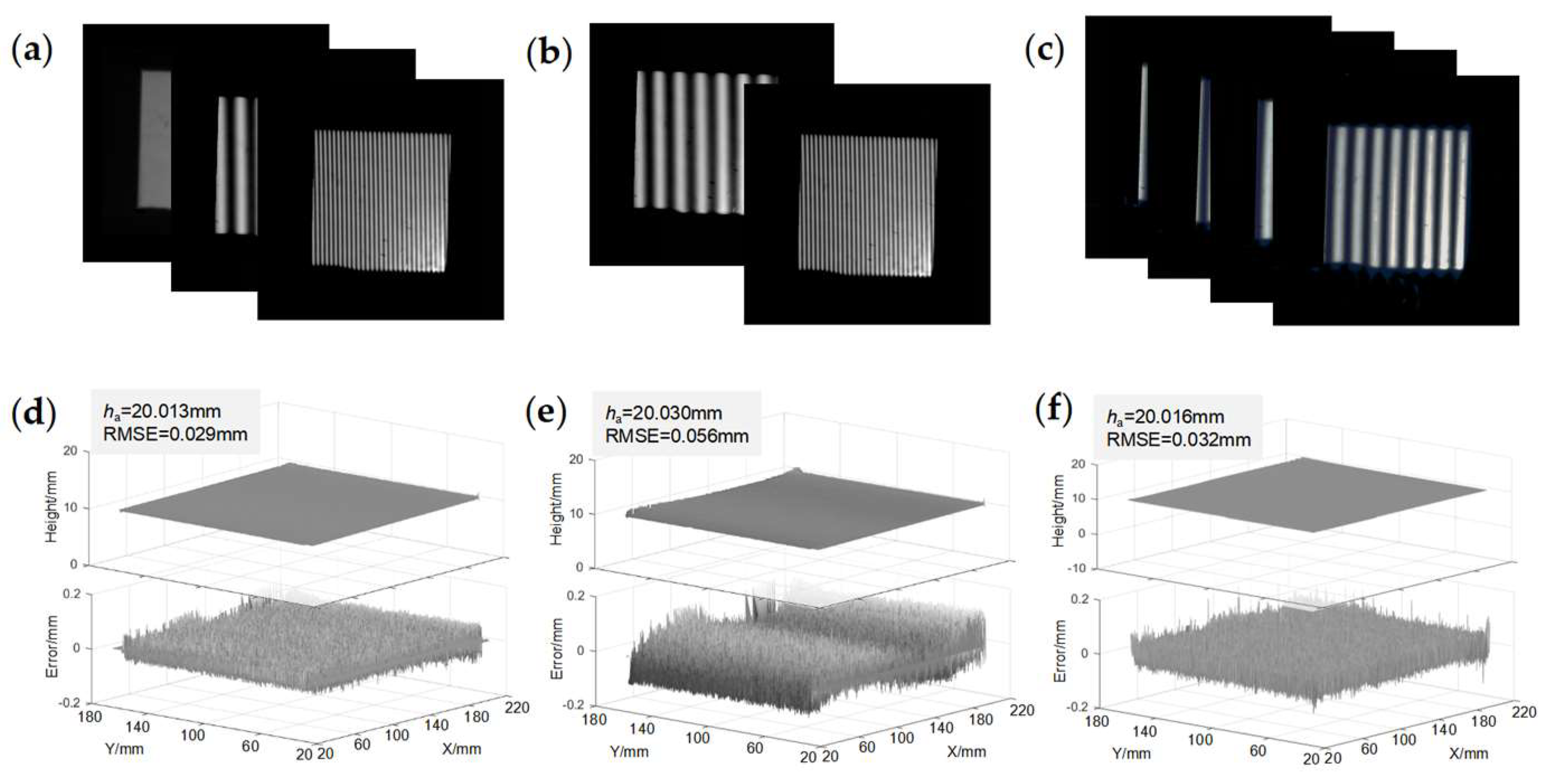
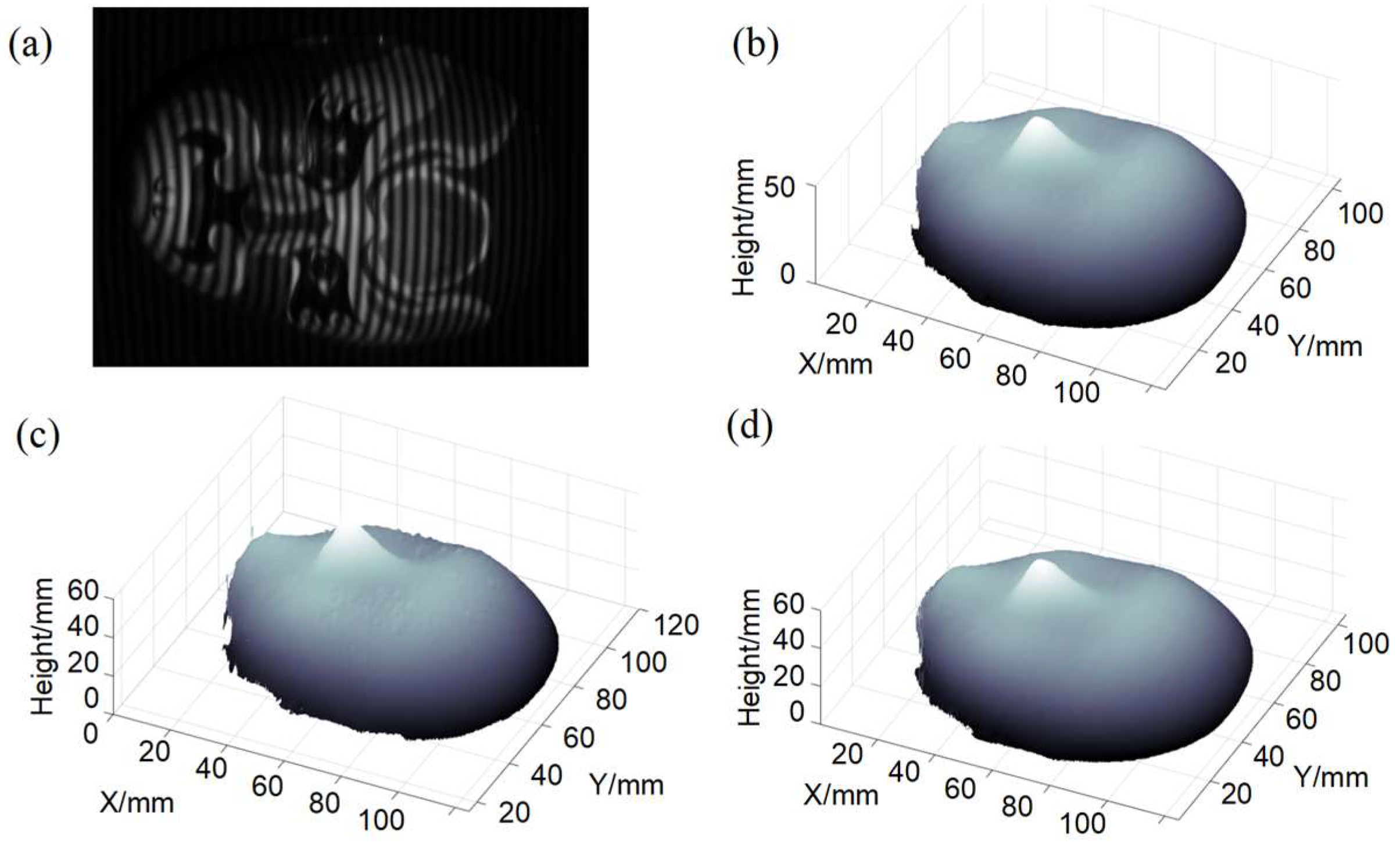
3.1. Accuracy Evaluation
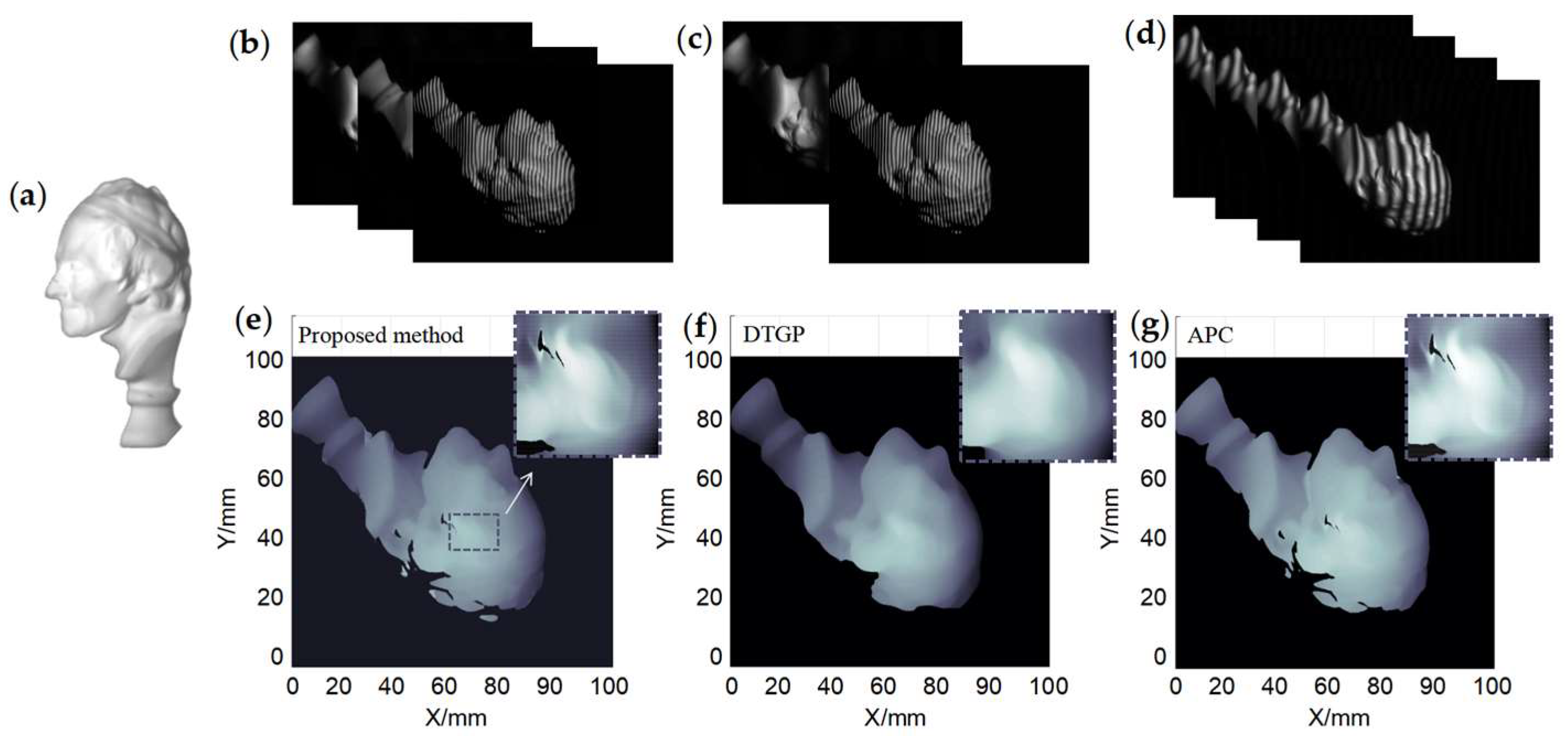
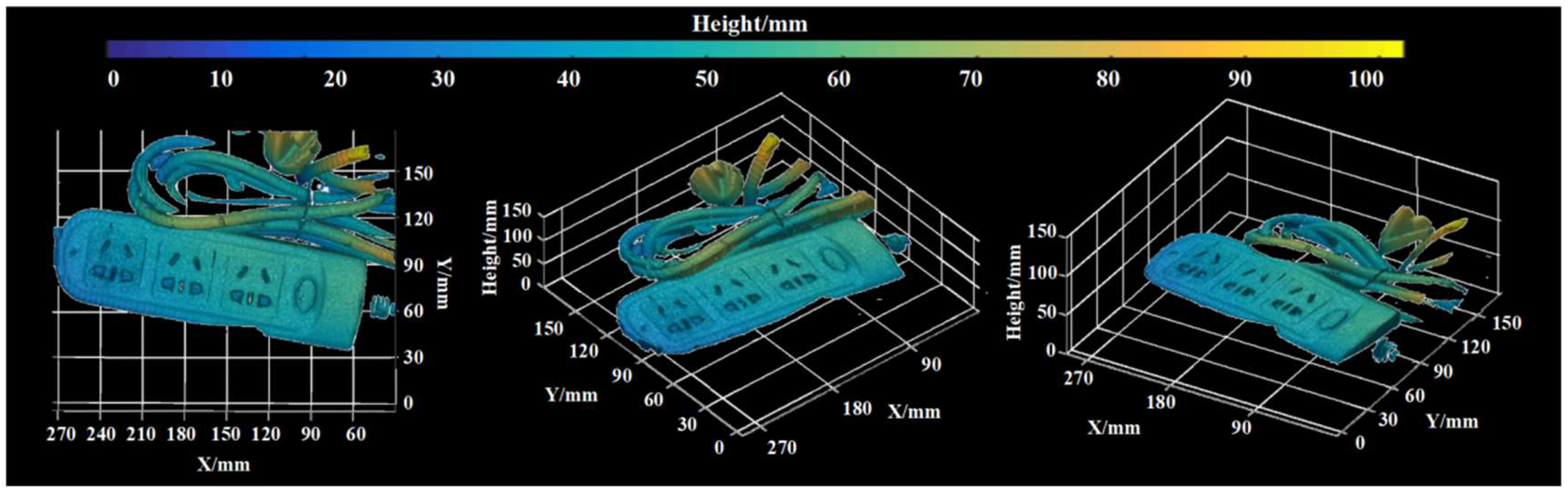
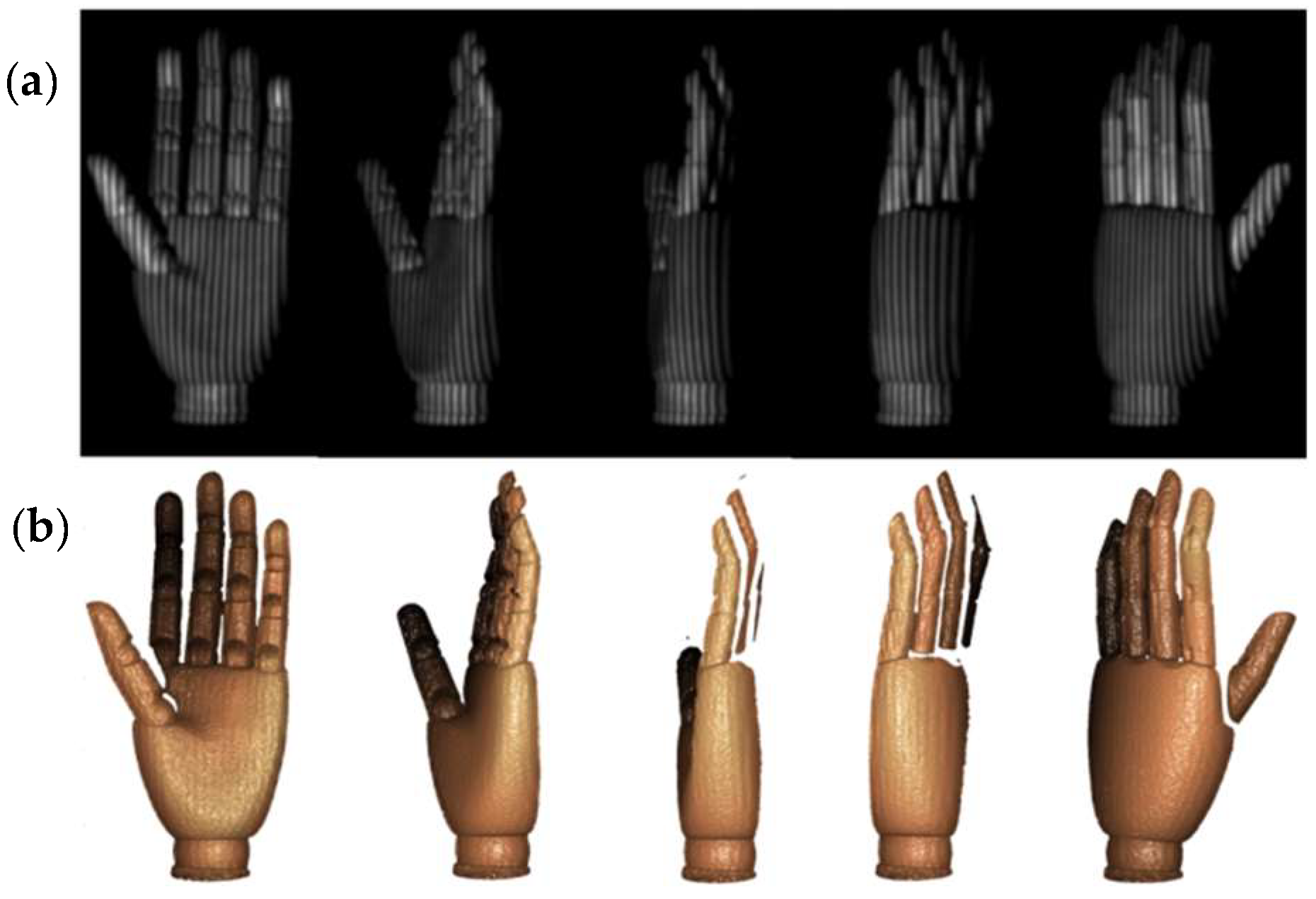
3.2. Robustness Verification
3.3. Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zuo, C.; Feng, S.J.; Huang, L.; Tao, T.Y.; Yin, W.; Chen, Q. Phase shifting algorithms for fringe projection profilometry: A review. Opt. Laser Eng. 2018, 109, 23–59. [Google Scholar] [CrossRef]
- Tan, J.; Su, W.; He, Z.; Bai, Y.; Dong, B.; Xie, S. Generic saturation-induced phase error correction for structured light 3D shape measurement. Opt. Lett. 2022, 47, 3387–3390. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.Y.; Yu, X.Y.; Wu, H.B.; Zhang, J.X.; Meng, X.L. Phase-unwrapping approach based on dual-frequency analog structured light. Opt. Commun. 2017, 382, 272–280. [Google Scholar] [CrossRef]
- Wu, Z.J.; Guo, W.B.; Zhang, Q.C. High-speed three-dimensional shape measurement based on shifting Gray-code light. Opt. Exp. 2019, 27, 22631–22644. [Google Scholar] [CrossRef]
- Zheng, Z.J.; Gao, J.; Mo, J.H.; Zhang, L.Y.; Zhang, Q.F. A fast self-correction method for nonlinear sinusoidal fringe images in 3-D measurement. IEEE Trans. Instrum. Meas. 2021, 70, 1–9. [Google Scholar] [CrossRef]
- Tan, J.; Liu, J.; Wang, X.; He, Z.; Su, W.; Huang, T.; Xie, S. Large depth range binary-focusing projection 3D shape reconstruction via unpaired data learning. Opt. Laser Eng. 2024, 181, 108442. [Google Scholar] [CrossRef]
- Takeda, M.; Mutoh, K. Fourier transform profilometry for the automatic measurement 3D object shapes. Appl. Opt. 1983, 22, 3977–3982. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.Q.; Chen, W.J.; Su, X.Y.; Chen, F. Fast Fourier transform profilometry based on two-frequency grating projection. Opto-Electron. Eng. 2004, 31, 39. [Google Scholar]
- Li, J.; Su, X.Y.; Guo, L.R. Improved fourier transform profilometry for the automatic measurement of 3-dimensional object shapes. Opt. Eng. 1990, 29, 1439–1444. [Google Scholar]
- Zuo, C.; Tao, T.Y.; Feng, S.J.; Huang, L.; Asundi, A.; Chen, Q. Micro fourier transform profilometry (μftp): 3D shape measurement at 10,000 frames per second. Opt. Laser Eng. 2018, 102, 70–91. [Google Scholar] [CrossRef]
- Hong, G.; Huang, P.S. 3-D shape measurement by use of a modified Fourier transform method. Proc. SPIE 2008, 7066, 104–111. [Google Scholar]
- Dai, M.Y.; Peng, K.; Zhao, J.; Wan, M.L.; Wang, W.F.; Cao, Y.P. Fast 3D measurement based on improved optical flow for dynamic objects. Opt. Exp. 2020, 28, 18969–18985. [Google Scholar] [CrossRef] [PubMed]
- Kuang, P.; Cao, Y.P.; Li, K. A new pixel matching method using the entire modulation of the measured object in online PMP. Optik 2014, 125, 137–140. [Google Scholar]
- Zheng, D.L.; Da, F.P.; Qian, K.M.; Seah, H.S. Phase-shifting profilometry combined with Gray-coded patterns projections: Unwrapping error removal by an adaptive median filter. Opt. Exp. 2017, 25, 4700–4713. [Google Scholar] [CrossRef] [PubMed]
- Takasaki, H. Moiré topography from its birth to practical application. Opt. Laser Eng. 1982, 3, 3–14. [Google Scholar] [CrossRef]
- Takasaki, H. Moiré topography. Appl. Opt. 1970, 9, 1467–1472. [Google Scholar] [CrossRef] [PubMed]
- Yoshizawa, T.; Tomisawa, T. Shadow moiré topography by means of the phase-shifted method. Opt. Eng. 1993, 32, 1668–1674. [Google Scholar] [CrossRef]
- Halioua, M.; Krishnamurthy, R.; Liu, H.; Chang, F. Projection moiré with moving gratings for automated 3-D topography. Appl. Opt. 1983, 22, 850. [Google Scholar] [CrossRef] [PubMed]
- Mohammadi, F.; Kofman, J. Single-frame digital phase-shifting 3D shape measurement using pixel-wise moiré-wavelength refinement. Opt. Laser Eng. 2016, 78, 196–204. [Google Scholar] [CrossRef]
- Mohammadi, F.; Kofman, J. Improved grid-noise removal in single frame digital moiré 3D shape measurement. Opt. Laser Eng. 2016, 86, 143–155. [Google Scholar] [CrossRef]
- Li, C.M.; Cao, Y.P.; Chen, C.; Wan, Y.Y.; Fu, G.K.; Wang, Y.P. Computer-generated Moiré profilometry. Opt. Exp. 2017, 25, 26815–26824. [Google Scholar] [CrossRef] [PubMed]
- Li, C.M.; Cao, Y.P.; Wang, L.; Wan, Y.Y.; Fu, G.K.; Wang, Y.P.; Chen, C. High precision computer-generated moiré profilometry. Sci. Rep. 2019, 9, 7804. [Google Scholar] [CrossRef] [PubMed]
- Li, C.M.; Cao, Y.P.; Wan, Y.Y.; Li, H.M.; Xu, C.; Zhang, H.C. Computer-generated frequency-carrier moiré profilometry. Opt. Commun. 2021, 501, 127381. [Google Scholar] [CrossRef]
- Li, H.; Cao, Y.; Wan, Y.; Li, C.; Xu, C.; Zhang, H.; An, H. A super-grayscale and real-time computer-generated Moiré profilometry using video grating projection. Sci. Rep. 2021, 11, 19882. [Google Scholar] [CrossRef]
- Zhang, H.C.; Cao, Y.P.; Li, C.M.; Wang, L.; Li, H.M.; Xu, C.; Wan, Y.Y. Color-encoded computer-generated Moiré profilometry with real-time 3D measurement and synchronous monitoring video collection. Opt. Eng. 2021, 60, 034109. [Google Scholar] [CrossRef]
- Zhang, H.C.; Cao, Y.P.; Li, C.M.; Wang, L.; Li, H.M.; Xu, C.; Wan, Y.Y. Color-encoded single-shot computer-generated Moiré profilometry. Sci. Rep. 2021, 1, 11020. [Google Scholar] [CrossRef]
- Zhang, H.C.; Cao, Y.P.; Li, H.M.; An, H.H.; Wu, H.T. Spatial computer-generated Moire profilometry. Sens. Actuators A Phys. 2024, 367, 115054. [Google Scholar] [CrossRef]
- Chen, K.; Xi, J.T.; Yu, Y.G. Quality-guided spatial phase unwrapping algorithm for fast three-dimensional measurement. Opt. Commun. 2013, 294, 139–147. [Google Scholar] [CrossRef]
- Liu, X.R.; Kofman, J. High-frequency background modulation fringe patterns based on a fringe-wavelength geometry-constraint model for 3D surface-shape measurement. Opt. Exp. 2017, 25, 16618–16628. [Google Scholar] [CrossRef]
- Cheng, T.; Du, Q.; Jiang, Y. Color fringe projection profilometry using geometric constraints. Opt. Commun. 2017, 398, 39–43. [Google Scholar] [CrossRef]
- Zuo, C.; Huang, L.; Zhang, M.L.; Chen, Q.; Asundi, A. Temporal phase unwrapping algorithms for fringe projection profilometry: A comparative review. Opt. Laser Eng. 2016, 85, 84–103. [Google Scholar] [CrossRef]
- Su, X.Y.; Chen, W.J. Reliability-guided phase unwrapping algorithm: A review. Opt. Lasers Eng. 2004, 42, 245–261. [Google Scholar] [CrossRef]
- Wang, J.; Yang, Y. Branch-cut algorithm with fast search ability for the shortest branch-cuts based on modified GA. J. Mod. Opt. 2019, 66, 473–485. [Google Scholar] [CrossRef]
- Arevalillo-Herraez, M.; Villatoro, F.R.; Gdeisat, M.A. A robust and simple measure for quality-guided 2D phase unwrapping algorithms. IEEE Trans. Image Process. 2016, 25, 2601–2609. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.J.; Guo, W.B.; Li, Y.Y.; Liu, Y.H.; Zhaang, Q.C. High-speed and high-efficiency three-dimensional shape measurement based on gray coded light. Photonics Res. 2020, 8, 819–829. [Google Scholar] [CrossRef]
- Zheng, D.L.; Kemao, Q.; Da, F.P.; Seah, H.S. Ternary gray code based phase unwrapping for 3D measurement using binary patterns with projector defocusing. Appl. Opt. 2017, 56, 3660–3665. [Google Scholar] [CrossRef]
- Wang, Y.J.; Zhang, S. Novel phase-coding method for absolute phase retrieval. Opt. Lett. 2012, 37, 2067–2069. [Google Scholar] [CrossRef] [PubMed]
- An, H.H.; Cao, Y.P.; Li, H.M.; Zhang, H.C. Temporal Phase Unwrapping Based on Unequal Phase-Shifting Code. IEEE Trans. Image Process. 2023, 32, 1432–1441. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.H.; Yang, Y.X. Phase extraction accuracy comparison based on multi-frequency phase-shifting method in fringe projection profilometry. Measurement 2022, 199, 111525. [Google Scholar] [CrossRef]
- Li, L.; Zheng, Y.; Yang, K.; Su, X.; Wang, Y.W.; Chen, X.C.; Wang, Y.J.; Li, B.W. Modified three-wavelength phase unwrapping algorithm for dynamic three-dimensional shape measurement. Opt. Commun. 2021, 480, 126409. [Google Scholar] [CrossRef]
- Wu, Z.J.; Guo, W.B.; Zhang, Q.C. Two-frequency phase-shifting method vs. Gray-coded-based method in dynamic fringe projection profilometry: A comparative review. Opt. Laser Eng. 2022, 153, 106995. [Google Scholar] [CrossRef]
- Li, J.L.; Su, H.J.; Su, X.Y. Two-frequency grating used in phase-measuring profilometry. Appl. Opt. 1997, 36, 277–280. [Google Scholar] [CrossRef] [PubMed]
- Guo, W.B.; Wu, Z.J.; Xu, R.C.; Zhang, Q.C.; Fujigaki, M. A fast reconstruction method for three-dimensional shape measurement using dual-frequency grating projection and phase-to-height lookup table. Opt. Laser Technol. 2019, 112, 269–277. [Google Scholar] [CrossRef]
- Liu, K.; Wang, Y.C.; Lau, D.; Hao, Q.; Hassebrook, L.G. Dual-frequency pattern scheme for high-speed 3-D shape measurement. Opt. Exp. 2010, 18, 5229–5244. [Google Scholar] [CrossRef] [PubMed]
- Dai, M.L.; Yang, F.J.; Liu, C.; He, X.Y. A dual-frequency fringe projection three-dimensional shape measurement system using a DLP 3D projector. Opt. Commun. 2017, 382, 294–301. [Google Scholar] [CrossRef]
- Qiao, N.; Quan, C. Dual-frequency fringe projection for 3D shape measurement based on correction of gamma nonliearity. Opt. Laser Technol. 2018, 106, 378–384. [Google Scholar] [CrossRef]
- Li, H.M.; Cao, Y.P.; Wu, H.T.; Xu, C.; Zhang, H.C.; An, H.H. A dual-frequency temporal-geometric phase unwrapping based on computer-generated frequency-carrier Moiré profilometry. Measurement 2022, 200, 111606. [Google Scholar] [CrossRef]
- Li, W.; Su, X.; Liu, Z. Large-scale three-dimensional object measurement: A practical coordinate mapping and image data-patching method. Appl. Opt. 2001, 40, 3326–3333. [Google Scholar] [CrossRef]
- Ma, Q.N.; Cao, Y.P.; Chen, C.; Wan, Y.Y.; Fu, G.K.; Wang, Y.P. Intrinsic feature revelation of phase-to-height mapping in phase measuring profilometry. Opt. Laser Technol. 2018, 108, 46–52. [Google Scholar] [CrossRef]


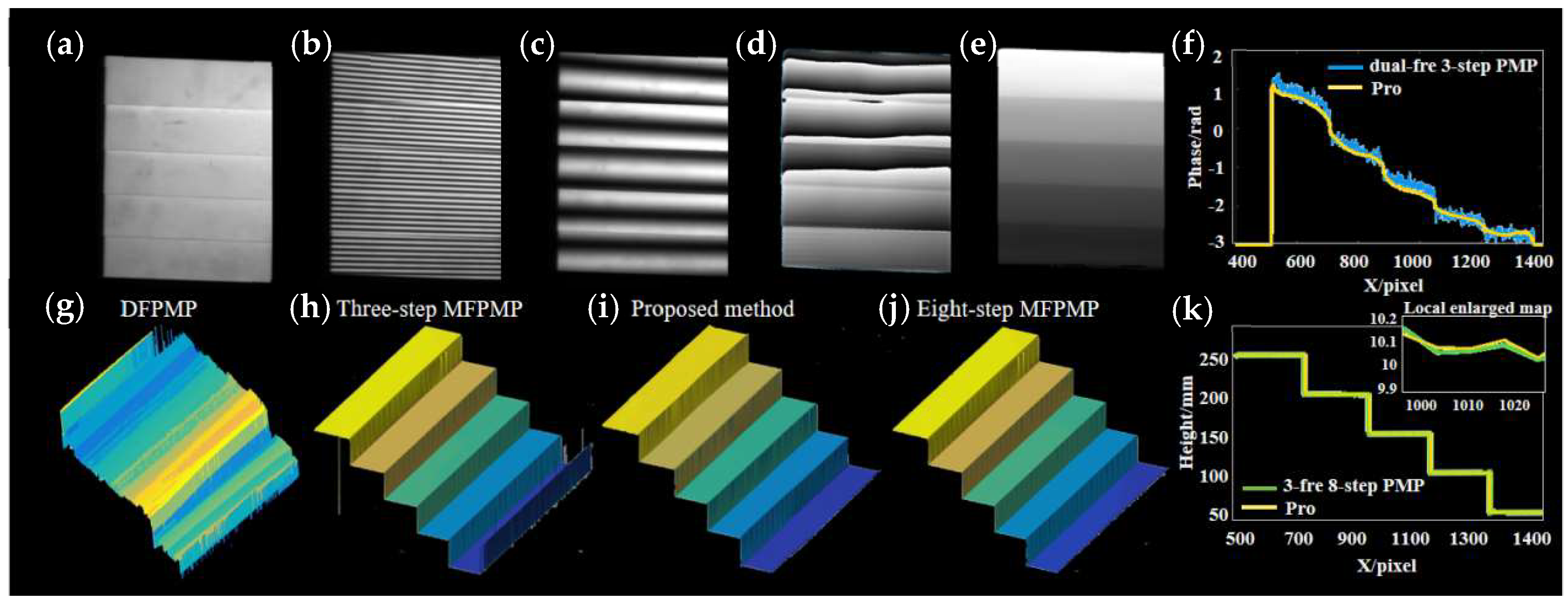

| Three-Step MFPMP | Proposed Method | Eight-Step MFPMP | |
|---|---|---|---|
| MAE (mm) | 0.027 | 0.015 | 0.012 |
| RMSE (mm) | 0.064 | 0.027 | 0.023 |
| Error pixel | 5371 | 1060 | 949 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Zhou, J.; Jia, D.; Huang, J.; Yuan, J. Three-Shot Dual-Frequency Fringe Scheme Based on Spatial Computer-Generated Moiré Fringe. Photonics 2024, 11, 758. https://doi.org/10.3390/photonics11080758
Zhang H, Zhou J, Jia D, Huang J, Yuan J. Three-Shot Dual-Frequency Fringe Scheme Based on Spatial Computer-Generated Moiré Fringe. Photonics. 2024; 11(8):758. https://doi.org/10.3390/photonics11080758
Chicago/Turabian StyleZhang, Hechen, Jin Zhou, Dan Jia, Jinlong Huang, and Jing Yuan. 2024. "Three-Shot Dual-Frequency Fringe Scheme Based on Spatial Computer-Generated Moiré Fringe" Photonics 11, no. 8: 758. https://doi.org/10.3390/photonics11080758
APA StyleZhang, H., Zhou, J., Jia, D., Huang, J., & Yuan, J. (2024). Three-Shot Dual-Frequency Fringe Scheme Based on Spatial Computer-Generated Moiré Fringe. Photonics, 11(8), 758. https://doi.org/10.3390/photonics11080758




