Theoretical Models for Performance Analysis of Spintronic THz Emitters
Abstract
1. Introduction
2. Basic Background of Spintronic THz Emitters
2.1. THz Time-Domain Spectroscopy Introduction
2.2. Basic Structure and Operation of STEs
3. THz Generation in the STE: Physical Phenomena
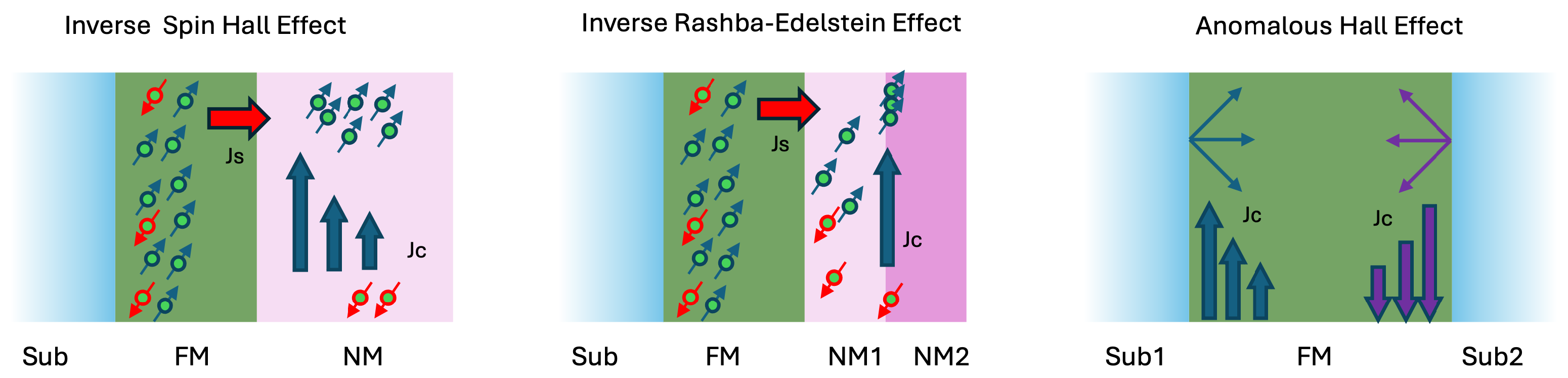
3.1. Spin Current Generation
3.2. Charge Current Generation
3.3. THz Emission
4. THz Outcoupling from the STE: Geometry Influence
4.1. Pump Laser Influence
4.2. Spin Diffusion Influence
4.3. THz Absorption Influence
5. Discussion and Comparison of the General Models
5.1. Typical Bilayer Structures of an STE
5.2. Secondary Enhancement to the THz Emission
5.3. AHE-Based THz Emission
5.4. Extension of Bilayer Samples
5.5. THz Bandwidth Study
6. THz Detection at the Detector: Response Functions
7. Conclusions and Future Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Neu, J.; Schmuttenmaer, C.A. Tutorial: An introduction to terahertz time domain spectroscopy (THz-TDS). J. Appl. Phys. 2018, 124, 231101. [Google Scholar] [CrossRef]
- Smith, R.M.; Arnold, M.A. Terahertz time-domain spectroscopy of solid samples: Principles, applications, and challenges. Appl. Spectrosc. Rev. 2011, 46, 636–679. [Google Scholar] [CrossRef]
- Tonouchi, M. Cutting-edge terahertz technology. Nat. Photonics 2007, 1, 97–105. [Google Scholar] [CrossRef]
- Federici, J.; Moeller, L. Review of terahertz and subterahertz wireless communications. J. Appl. Phys. 2010, 107, 111101. [Google Scholar] [CrossRef]
- Ferguson, B.; Zhang, X.C. Materials for terahertz science and technology. Nat. Mater. 2002, 1, 26–33. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Chen, Y.; Mao, J.; Yang, F.; Wang, N. Metasurface-assisted terahertz sensing. Sensors 2023, 23, 5902. [Google Scholar] [CrossRef] [PubMed]
- Kemp, M.C.; Taday, P.; Cole, B.E.; Cluff, J.; Fitzgerald, A.J.; Tribe, W.R. Security applications of terahertz technology. In Proceedings of the Terahertz for Military and Security Applications, SPIE, Orlando, FL, USA, 21 April 2003; Volume 5070, pp. 44–52. [Google Scholar]
- Seifert, T.S.; Cheng, L.; Wei, Z.; Kampfrath, T.; Qi, J. Spintronic sources of ultrashort terahertz electromagnetic pulses. Appl. Phys. Lett. 2022, 120, 180401. [Google Scholar] [CrossRef]
- Cheng, L.; Li, Z.; Zhao, D.; Chia, E.E. Studying spin–charge conversion using terahertz pulses. Appl. Mater. 2021, 9, 070902. [Google Scholar] [CrossRef]
- Walowski, J.; Münzenberg, M. Perspective: Ultrafast magnetism and THz spintronics. J. Appl. Phys. 2016, 120, 140901. [Google Scholar] [CrossRef]
- Huisman, T.J.; Rasing, T. THz emission spectroscopy for THz spintronics. J. Phys. Soc. Jpn. 2017, 86, 011009. [Google Scholar] [CrossRef]
- Seifert, T.; Jaiswal, S.; Martens, U.; Hannegan, J.; Braun, L.; Maldonado, P.; Freimuth, F.; Kronenberg, A.; Henrizi, J.; Radu, I.; et al. Efficient metallic spintronic emitters of ultrabroadband terahertz radiation. Nat. Photonics 2016, 10, 483–488. [Google Scholar] [CrossRef]
- Kampfrath, T.; Battiato, M.; Maldonado, P.; Eilers, G.; Nötzold, J.; Mährlein, S.; Zbarsky, V.; Freimuth, F.; Mokrousov, Y.; Blügel, S.; et al. Terahertz spin current pulses controlled by magnetic heterostructures. Nat. Nanotechnol. 2013, 8, 256–260. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Lee, K.; Yang, Y.; Li, Z.; Sharma, R.; Xi, L.; Salim, T.; Boothroyd, C.; Lam, Y.M.; Yang, H.; et al. Spintronic terahertz emitters in silicon-based heterostructures. Phys. Rev. Appl. 2022, 18, 034056. [Google Scholar] [CrossRef]
- Cheng, L.; Wang, X.; Yang, W.; Chai, J.; Yang, M.; Chen, M.; Wu, Y.; Chen, X.; Chi, D.; Goh, K.E.J.; et al. Far out-of-equilibrium spin populations trigger giant spin injection into atomically thin MoS2. Nat. Phys. 2019, 15, 347–351. [Google Scholar] [CrossRef]
- Wu, W.; Yaw Ameyaw, C.; Doty, M.F.; Jungfleisch, M.B. Principles of spintronic THz emitters. J. Appl. Phys. 2021, 130, 091101. [Google Scholar] [CrossRef]
- Battiato, M.; Carva, K.; Oppeneer, P.M. Superdiffusive Spin Transport as a Mechanism of Ultrafast Demagnetization. Phys. Rev. Lett. 2010, 105, 027203. [Google Scholar] [CrossRef] [PubMed]
- Choi, G.M.; Min, B.C.; Lee, K.J.; Cahill, D.G. Spin current generated by thermally driven ultrafast demagnetization. Nat. Commun. 2014, 5, 4334. [Google Scholar] [CrossRef] [PubMed]
- Battiato, M.; Carva, K.; Oppeneer, P.M. Theory of laser-induced ultrafast superdiffusive spin transport in layered heterostructures. Phys. Rev. B 2012, 86, 024404. [Google Scholar] [CrossRef]
- Kirilyuk, A.; Kimel, A.V.; Rasing, T. Ultrafast optical manipulation of magnetic order. Rev. Mod. Phys. 2010, 82, 2731–2784. [Google Scholar] [CrossRef]
- Rudolf, D.; La-O-Vorakiat, C.; Battiato, M.; Adam, R.; Shaw, J.M.; Turgut, E.; Maldonado, P.; Mathias, S.; Grychtol, P.; Nembach, H.T.; et al. Ultrafast magnetization enhancement in metallic multilayers driven by superdiffusive spin current. Nat. Commun. 2012, 3, 1037. [Google Scholar] [CrossRef] [PubMed]
- Zhang, G.P.; Hübner, W. Laser-Induced Ultrafast Demagnetization in Ferromagnetic Metals. Phys. Rev. Lett. 2000, 85, 3025–3028. [Google Scholar] [CrossRef] [PubMed]
- Eschenlohr, A.; Battiato, M.; Maldonado, P.; Pontius, N.; Kachel, T.; Holldack, K.; Mitzner, R.; Föhlisch, A.; Oppeneer, P.M.; Stamm, C. Ultrafast spin transport as key to femtosecond demagnetization. Nat. Mater. 2013, 12, 332–336. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Dal Forno, S.; Battiato, M. Transfer-matrix description of heterostructured spintronics terahertz emitters. Phys. Rev. B 2021, 104, 155437. [Google Scholar] [CrossRef]
- Zhou, C.; Liu, Y.; Wang, Z.; Ma, S.; Jia, M.W.; Wu, R.; Zhou, L.; Zhang, W.; Liu, M.; Wu, Y.; et al. Broadband terahertz generation via the interface inverse Rashba-Edelstein effect. Phys. Rev. Lett. 2018, 121, 086801. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Cheng, H.; Xu, Y.; Vallobra, P.; Eimer, S.; Zhang, X.; Wu, X.; Nie, T.; Zhao, W. Separation of emission mechanisms in spintronic terahertz emitters. Phys. Rev. B 2021, 104, 064419. [Google Scholar] [CrossRef]
- Zhang, Q.; Luo, Z.; Li, H.; Yang, Y.; Zhang, X.; Wu, Y. Terahertz emission from anomalous Hall effect in a single-layer ferromagnet. Phys. Rev. Appl. 2019, 12, 054027. [Google Scholar] [CrossRef]
- Mottamchetty, V.; Rani, P.; Brucas, R.; Rydberg, A.; Svedlindh, P.; Gupta, R. Direct evidence of terahertz emission arising from anomalous Hall effect. Sci. Rep. 2023, 13, 5988. [Google Scholar] [CrossRef] [PubMed]
- Seifert, T.S.; Martens, U.; Radu, F.; Ribow, M.; Berritta, M.; Nádvorník, L.; Starke, R.; Jungwirth, T.; Wolf, M.; Radu, I.; et al. Frequency-Independent Terahertz Anomalous Hall Effect in DyCo5, Co32Fe68, and Gd27Fe73 Thin Films from DC to 40 THz. Adv. Mater. 2021, 33, 2007398. [Google Scholar] [CrossRef] [PubMed]
- Beaurepaire, E.; Merle, J.C.; Daunois, A.; Bigot, J.Y. Ultrafast spin dynamics in ferromagnetic nickel. Phys. Rev. Lett. 1996, 76, 4250. [Google Scholar] [CrossRef] [PubMed]
- Gupta, R.; Cosco, F.; Malik, R.; Chen, X.; Saha, S.; Ghosh, A.; Pohlmann, T.; Mardegan, J.; Francoual, S.; Stefanuik, R.; et al. Element-resolved evidence of superdiffusive spin current arising from ultrafast demagnetization process. Phys. Rev. B 2023, 108, 064427. [Google Scholar] [CrossRef]
- Rouzegar, R.; Brandt, L.; Nádvorník, L.; Reiss, D.A.; Chekhov, A.L.; Gueckstock, O.; In, C.; Wolf, M.; Seifert, T.S.; Brouwer, P.W.; et al. Laser-induced terahertz spin transport in magnetic nanostructures arises from the same force as ultrafast demagnetization. Phys. Rev. B 2022, 106, 144427. [Google Scholar] [CrossRef]
- Seifert, T.S.; Jaiswal, S.; Barker, J.; Weber, S.T.; Razdolski, I.; Cramer, J.; Gueckstock, O.; Maehrlein, S.F.; Nadvornik, L.; Watanabe, S.; et al. Femtosecond formation dynamics of the spin Seebeck effect revealed by terahertz spectroscopy. Nat. Commun. 2018, 9, 2899. [Google Scholar] [CrossRef] [PubMed]
- Xiao, J.; Bauer, G.E.; Uchida, K.C.; Saitoh, E.; Maekawa, S. Theory of magnon-driven spin Seebeck effect. Phys. Rev. B 2010, 81, 214418. [Google Scholar] [CrossRef]
- Adachi, H.; Uchida, K.i.; Saitoh, E.; Maekawa, S. Theory of the spin Seebeck effect. Rep. Prog. Phys. 2013, 76, 036501. [Google Scholar] [CrossRef] [PubMed]
- Qiu, H.; Kato, K.; Hirota, K.; Sarukura, N.; Yoshimura, M.; Nakajima, M. Layer thickness dependence of the terahertz emission based on spin current in ferromagnetic heterostructures. Opt. Express 2018, 26, 15247–15254. [Google Scholar] [CrossRef] [PubMed]
- Sasaki, Y.; Suzuki, K.; Mizukami, S. Annealing effect on laser pulse-induced THz wave emission in Ta/CoFeB/MgO films. Appl. Phys. Lett. 2017, 111, 102401. [Google Scholar] [CrossRef]
- Seifert, T.S.; Tran, N.M.; Gueckstock, O.; Rouzegar, S.M.; Nadvornik, L.; Jaiswal, S.; Jakob, G.; Temnov, V.V.; Muenzenberg, M.; Wolf, M.; et al. Terahertz spectroscopy for all-optical spintronic characterization of the spin-Hall-effect metals Pt, W and Cu80Ir20. J. Phys. D Appl. Phys. 2018, 51, 364003. [Google Scholar] [CrossRef]
- Torosyan, G.; Keller, S.; Scheuer, L.; Beigang, R.; Papaioannou, E.T. Optimized spintronic terahertz emitters based on epitaxial grown Fe/Pt layer structures. Sci. Rep. 2018, 8, 1311. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Dal Forno, S.; Battiato, M. Modeling spintronic terahertz emitters as a function of spin generation and diffusion geometry. Phys. Rev. B 2023, 107, 144407. [Google Scholar] [CrossRef]
- Agarwal, P.; Yang, Y.; Medwal, R.; Asada, H.; Fukuma, Y.; Battiato, M.; Singh, R. Secondary spin current driven efficient THz spintronic emitters. Adv. Opt. Mater. 2023, 11, 2301027. [Google Scholar] [CrossRef]
- Papaioannou, E.T.; Torosyan, G.; Keller, S.; Scheuer, L.; Battiato, M.; Mag-Usara, V.K.; L’huillier, J.; Tani, M.; Beigang, R. Efficient terahertz generation using Fe/Pt spintronic emitters pumped at different wavelengths. IEEE Trans. Magnet. 2018, 54, 9100205. [Google Scholar] [CrossRef]
- Herapath, R.I.; Hornett, S.M.; Seifert, T.; Jakob, G.; Kläui, M.; Bertolotti, J.; Kampfrath, T.; Hendry, E. Impact of pump wavelength on terahertz emission of a cavity-enhanced spintronic trilayer. Appl. Phys. Lett. 2019, 114, 041107. [Google Scholar] [CrossRef]
- Adam, R.; Chen, G.; Bürgler, D.E.; Shou, T.; Komissarov, I.; Heidtfeld, S.; Hardtdegen, H.; Mikulics, M.; Schneider, C.M.; Sobolewski, R. Magnetically and optically tunable terahertz radiation from Ta/NiFe/Pt spintronic nanolayers generated by femtosecond laser pulses. Appl. Phys. Lett. 2019, 114, 212405. [Google Scholar] [CrossRef]
- Mag-Usara, V.K.; Escaño, M.C.; Petoukhoff, C.E.; Torosyan, G.; Scheuer, L.; Madéo, J.; Afalla, J.; Talara, M.L.; Muldera, J.E.; Kitahara, H.; et al. Optimum excitation wavelength and photon energy threshold for spintronic terahertz emission from Fe/Pt bilayer. Iscience 2022, 25, 104615. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Dal Forno, S.; Battiato, M. Perturbative transfer matrix method for optical-pump terahertz-probe spectroscopy of ultrafast dynamics in spintronic terahertz emitters. Phys. Rev. B 2024, 109, 024425. [Google Scholar] [CrossRef]
- Feng, Z.; Yu, R.; Zhou, Y.; Lu, H.; Tan, W.; Deng, H.; Liu, Q.; Zhai, Z.; Zhu, L.; Cai, J.; et al. Highly efficient spintronic terahertz emitter enabled by metal–dielectric photonic crystal. Adv. Opt. Mater. 2018, 6, 1800965. [Google Scholar] [CrossRef]
- Yang, D.; Liang, J.; Zhou, C.; Sun, L.; Zheng, R.; Luo, S.; Wu, Y.; Qi, J. Powerful and Tunable THz Emitters Based on the Fe/Pt Magnetic Heterostructure. Adv. Opt. Mater. 2016, 4, 1944–1949. [Google Scholar] [CrossRef]
- Yang, Y.; Dal Forno, S.; Battiato, M. Removal of Spectral Distortion Due to Echo for Ultrashort THz Pulses Propagating Through Multilayer Structures with Thick Substrate. J. Infrared Millim. Terahertz Waves 2021, 42, 1142–1152. [Google Scholar] [CrossRef]
- Wang, X.; Cheng, L.; Zhu, D.; Wu, Y.; Chen, M.; Wang, Y.; Zhao, D.; Boothroyd, C.B.; Lam, Y.M.; Zhu, J.X.; et al. Ultrafast spin-to-charge conversion at the surface of topological insulator thin films. Adv. Mater. 2018, 30, 1802356. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y.; Dal Forno, S.; Battiato, M. Theoretical Models for Performance Analysis of Spintronic THz Emitters. Photonics 2024, 11, 730. https://doi.org/10.3390/photonics11080730
Yang Y, Dal Forno S, Battiato M. Theoretical Models for Performance Analysis of Spintronic THz Emitters. Photonics. 2024; 11(8):730. https://doi.org/10.3390/photonics11080730
Chicago/Turabian StyleYang, Yingshu, Stefano Dal Forno, and Marco Battiato. 2024. "Theoretical Models for Performance Analysis of Spintronic THz Emitters" Photonics 11, no. 8: 730. https://doi.org/10.3390/photonics11080730
APA StyleYang, Y., Dal Forno, S., & Battiato, M. (2024). Theoretical Models for Performance Analysis of Spintronic THz Emitters. Photonics, 11(8), 730. https://doi.org/10.3390/photonics11080730






