Optical Rogue Waves in Fiber Lasers
Abstract
1. Introduction
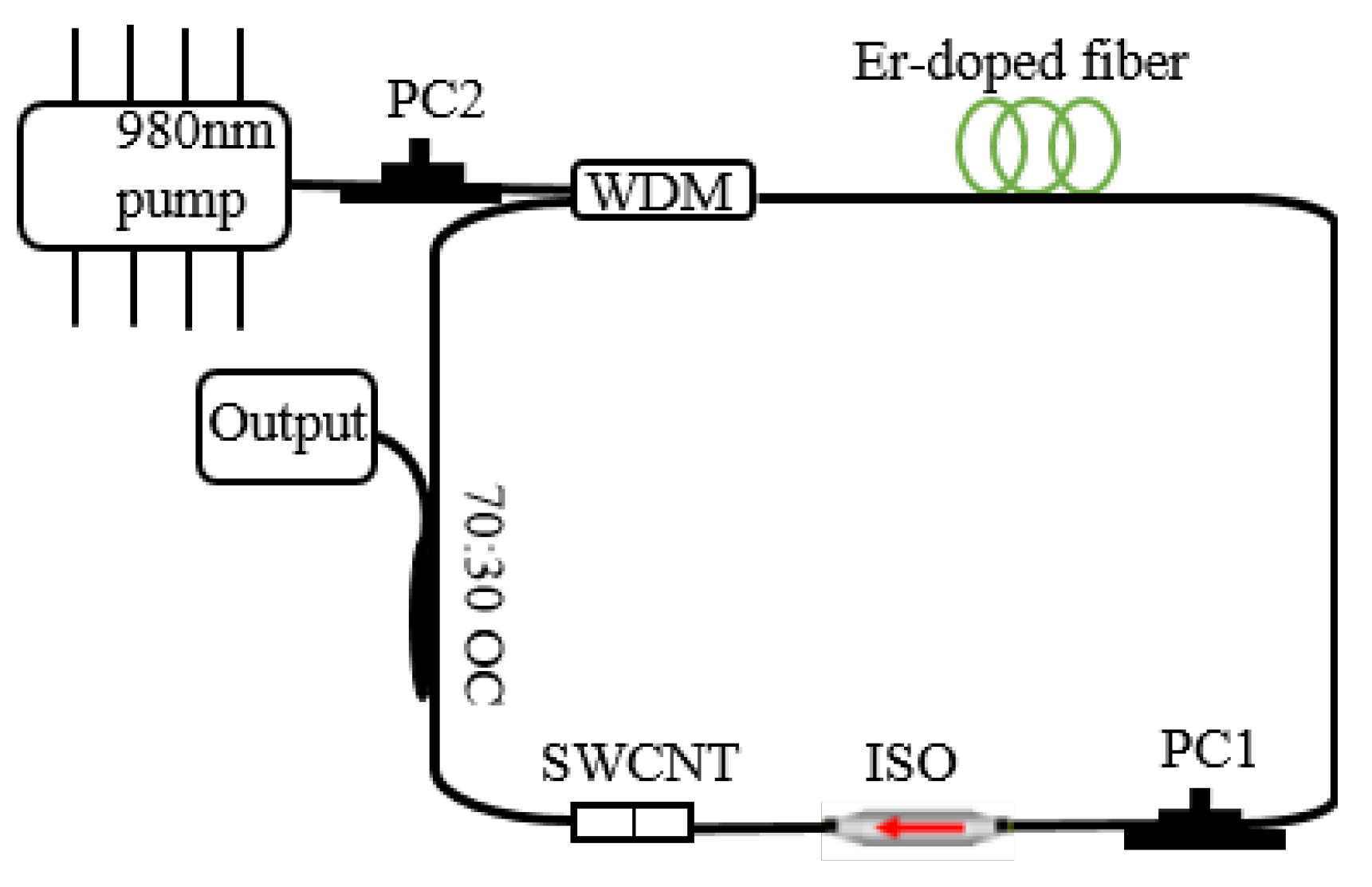
2. Testbed Experimental Setup
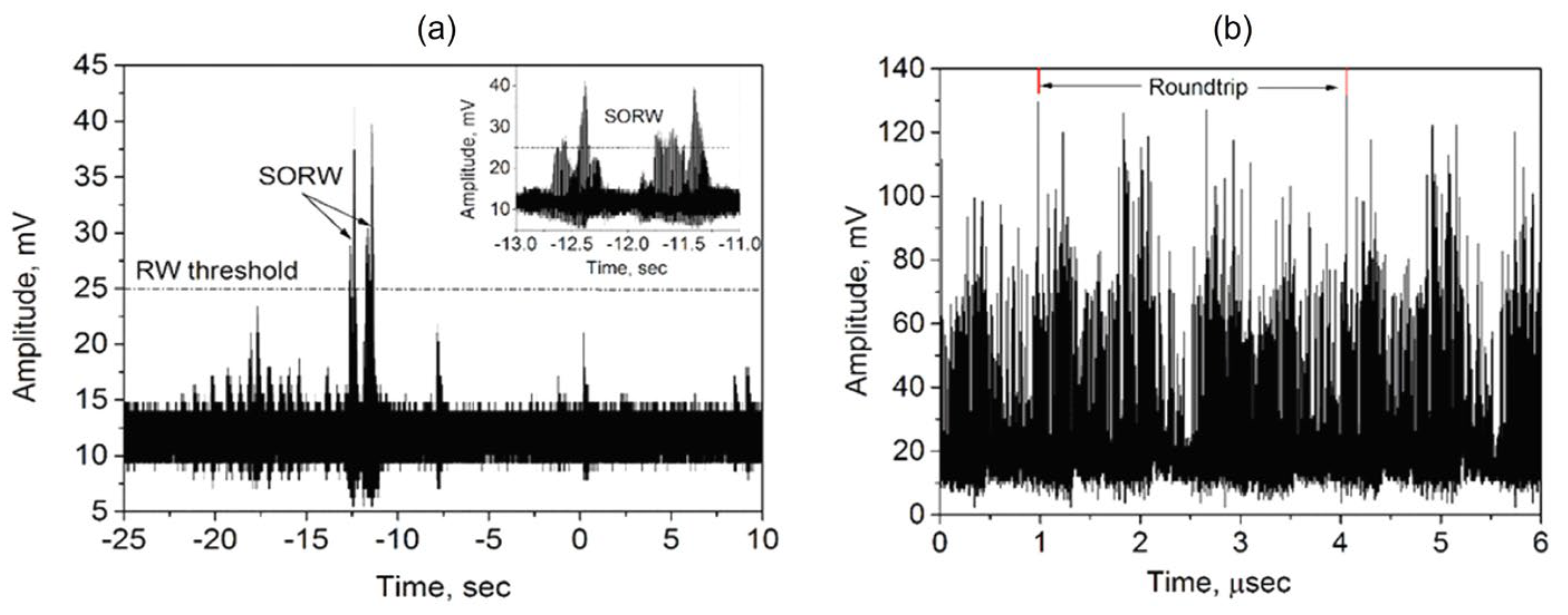
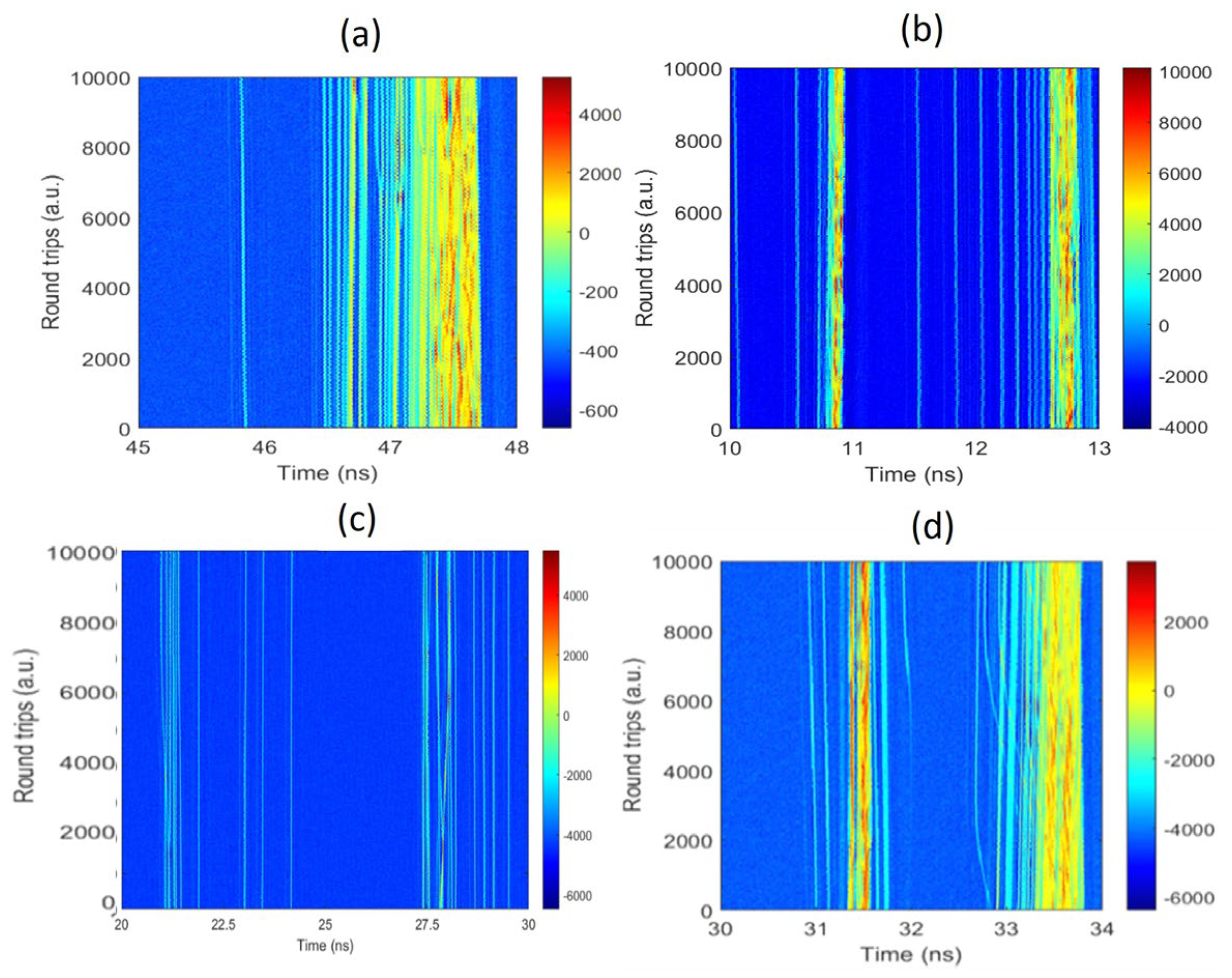
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Garrett, C.; Gemmrich, J. Rogue waves. Phys. Today 2009, 62, 62–63. [Google Scholar] [CrossRef]
- Hammani, K.; Finot, C.; Kibler, B.; Millot, G. Soliton generation and rogue-wave-like behavior through fourth-order scalar modulation instability. IEEE. Photonics J. 2009, 1, 205–212. [Google Scholar] [CrossRef]
- Arecchi, F.; Bortolozzo, U.; Montina, A.; Residori, S. Granularity and inhomogeneity are the joint generators of optical rogue waves. Phys. Rev. Lett. 2011, 106, 153901. [Google Scholar] [CrossRef]
- Onorato, M.; Residori, S.; Bortolozzo, U.; Montina, A.; Arecchi, F. Rogue waves and their generating mechanisms in different physical contexts. Phys. Rep. 2013, 528, 47–89. [Google Scholar] [CrossRef]
- Baronio, F.; Degasperis, A.; Conforti, M.; Wabnitz, S. Solutions of the vector nonlinear Schrödinger equations: Evidence for deterministic rogue waves. Phys. Rev. Lett. 2012, 109, 044102. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.-C.; Liu, J. Rogue-wave solutions of a three-component coupled nonlinear Schrödinger equation. Phys. Rev. E 2013, 87, 013201. [Google Scholar] [CrossRef] [PubMed]
- Guo, B.-L.; Ling, L.-M. Rogue wave, breathers and bright-dark-rogue solutions for the coupled Schrödinger equations. Chin. Phys. Lett. 2011, 28, 110202. [Google Scholar] [CrossRef]
- Montina, A.; Bortolozzo, U.; Residori, S.; Arecchi, F. Non-Gaussian statistics and extreme waves in a nonlinear optical cavity. Phys. Rev. Lett. 2009, 103, 173901. [Google Scholar] [CrossRef]
- Hammani, K.; Finot, C.; Millot, G. Emergence of extreme events in fiber-based parametric processes driven by a partially incoherent pump wave. Opt. Lett. 2009, 34, 1138–1140. [Google Scholar] [CrossRef]
- Bonatto, C.; Feyereisen, M.; Barland, S.; Giudici, M.; Masoller, C.; Leite, J.R.R.; Tredicce, J.R. Deterministic optical rogue waves. Phys. Rev. Lett. 2011, 107, 053901. [Google Scholar] [CrossRef] [PubMed]
- Kovalsky, M.G.; Hnilo, A.A.; Tredicce, J.R. Extreme events in the Ti: Sapphire laser. Opt. Lett. 2011, 36, 4449–4451. [Google Scholar] [CrossRef]
- Grelu, P.; Akhmediev, N. Dissipative solitons for mode-locked lasers. Nat. Photonics 2012, 6, 84. [Google Scholar] [CrossRef]
- Xu, C.; Wise, F. Recent advances in fiber lasers for nonlinear microscopy. Nat. Photonics 2013, 7, 875–882. [Google Scholar] [CrossRef] [PubMed]
- Solli, D.; Ropers, C.; Koonath, P.; Jalali, B. Optical rogue waves. Nature 2007, 450, 1054. [Google Scholar] [CrossRef]
- Solli, D.; Ropers, C.; Jalali, B. Active control of rogue waves for stimulated supercontinuum generation. Phys. Rev. Lett. 2008, 101, 233902. [Google Scholar] [CrossRef] [PubMed]
- Lafargue, C.; Bolger, J.; Genty, G.; Dias, F.; Dudley, J.; Eggleton, B. Direct detection of optical rogue wave energy statistics in supercontinuum generation. Electron. Lett. 2009, 45, 217–219. [Google Scholar] [CrossRef]
- Dudley, J.M.; Genty, G.; Dias, F.; Kibler, B.; Akhmediev, N. Modulation instability, Akhmediev Breathers and continuous wave supercontinuum generation. Opt. Express 2009, 17, 21497–21508. [Google Scholar] [CrossRef] [PubMed]
- Kibler, B.; Finot, C.; Dudley, J.M. Soliton and rogue wave statistics in supercontinuum generation in photonic crystal fiber with two zero dispersion wavelengths. Eur. Phys. J. Spec. Top. 2009, 173, 289–295. [Google Scholar] [CrossRef]
- Dudley, J.M.; Genty, G.; Eggleton, B.J. Harnessing and control of optical rogue waves in supercontinuum generation. Opt. Express 2008, 16, 3644–3651. [Google Scholar] [CrossRef]
- Hammani, K.; Finot, C.; Dudley, J.M.; Millot, G. Optical rogue-wave-like extreme value fluctuations in fiber Raman amplifiers. Opt. Express 2008, 16, 16467–16474. [Google Scholar] [CrossRef]
- Tarasov, N.; Sugavanam, S.; Churkin, D. Spatio-temporal generation regimes in quasi-CW Raman fiber lasers. Opt. Express 2015, 23, 24189–24194. [Google Scholar] [CrossRef]
- Hanzard, P.-H.; Talbi, M.; Mallek, D.; Kellou, A.; Leblond, H.; Sanchez, F. Brillouin scattering-induced rogue waves in self-pulsing fiber lasers. Sci. Rep. 2017, 7, 45868. [Google Scholar] [CrossRef] [PubMed]
- Soto-Crespo, J.; Grelu, P.; Akhmediev, N. Dissipative rogue waves: Extreme pulses generated by passively mode-locked lasers. Phys. Rev. E 2011, 84, 016604. [Google Scholar] [CrossRef]
- Zaviyalov, A.; Egorov, O.; Iliew, R.; Lederer, F. Rogue waves in mode-locked fiber lasers. Phys. Rev. A 2012, 85, 013828. [Google Scholar] [CrossRef]
- Finot, C.; Hammani, K.; Fatome, J.; Dudley, J.M.; Millot, G. Selection of extreme events generated in Raman fiber amplifiers through spectral offset filtering. IEEE J. Quantum Electron. 2010, 46, 205–213. [Google Scholar] [CrossRef]
- Sergeyev, S.V.; Kbashi, H.J.; Tarasov, N.; Loiko, Y.; Kolpakov, S.A. Vector-Resonance-Multimode Instability. Phys. Rev. Lett. 2017, 118, 033904. [Google Scholar] [CrossRef] [PubMed]
- Kolpakov, S.A.; Kbashi, H.J.; Sergeyev, S.V. Dynamics of vector rogue waves in a fiber laser with a ring cavity. Optica 2016, 3, 870–875. [Google Scholar] [CrossRef]
- Lecaplain, C.; Grelu, P.; Soto-Crespo, J.; Akhmediev, N. Dissipative rogue waves generated by chaotic pulse bunching in a mode-locked laser. Phys. Rev. Lett. 2012, 108, 233901. [Google Scholar] [CrossRef] [PubMed]
- Akhmediev, N.; Soto-Crespo, J.; Ankiewicz, A. Could rogue waves be used as efficient weapons against enemy ships? Eur. Phys. J. Spec. Top. 2010, 185, 259–266. [Google Scholar] [CrossRef]
- Demircan, A.; Amiranashvili, S.; Brée, C.; Mahnke, C.; Mitschke, F.; Steinmeyer, G. Rogue wave formation by accelerated solitons at an optical event horizon. Appl. Phys. B 2014, 115, 343–354. [Google Scholar] [CrossRef]
- Chouli, S.; Grelu, P. Soliton rains in a fiber laser: An experimental study. Phys. Rev. A 2010, 81, 063829. [Google Scholar] [CrossRef]
- Kbashi, H.J.; Sergeyev, S.V.; Al-Araimi, M.; Tarasov, N.; Rozhin, A. Vector soliton rain. Laser Phys. Lett. 2019, 16, 035103. [Google Scholar] [CrossRef]
- Sergeyev, S.V.; Eliwa, M.; Kbashi, H.J. Polarization attractors driven by vector soliton rain. Opt. Express 2022, 30, 35663–35670. [Google Scholar] [CrossRef]
- Runge, A.F.; Aguergaray, C.; Broderick, N.G.; Erkintalo, M. Raman rogue waves in a partially mode-locked fiber laser. Opt. Lett. 2014, 39, 319–322. [Google Scholar] [CrossRef]
- Liu, Z.W.; Zhang, S.M.; Wise, F.W. Rogue waves in a normal-dispersion fiber laser. Opt. Lett. 2015, 40, 1366–1369. [Google Scholar] [CrossRef]
- Teğin, U.; Wang, P.; Wang, L.V. Real-time observation of optical rogue waves in spatiotemporally mode-locked fiber lasers. Commun. Phys. 2023, 6, 60. [Google Scholar] [CrossRef]
- Laprel, C.; Billet, C.; Meng, F.; Ryczkowski, P.; Sylvestre, T.; Finot, C.; Genty, G.; Dudley, J.M. Real-time characterization of spectral instabilities in a modelocked fibre laser exhibiting soliton similariton dynamics. Sci. Rep. 2019, 9, 13950. [Google Scholar] [CrossRef]
- Kolpakov, S.; Kbashi, H.J.; Sergeyev, S.V. Slow optical rogue waves in a unidirectional fiber laser. In CLEO: QELS_Fundamental Science; Optica Publishing Group: Washington, DC, USA, 2016; JW2A-56. [Google Scholar]
- Kbashi, H.J.; Kolpakov, S.A.; Sergeyev, S.V. Fast and slow optical rogue waves in the fiber laser. Front. Phys. 2022, 10, 1048508. [Google Scholar] [CrossRef]
- Kbashi, H.J.; Sergeyev, S.V.; Al-Araimi, M.; Rozhin, A.; Korobko, D.; Fotiadi, A. High-frequency vector harmonic mode locking driven by acoustic resonances. Opt. Lett. 2019, 44, 5112–5115. [Google Scholar] [CrossRef]
- Kbashi, H.J.; Sharma, V.; Sergeyev, S.V. Phase-stable millimeter-wave generation using switchable dual-wavelength fiber laser. Opt. Lasers Eng. 2021, 137, 106390. [Google Scholar] [CrossRef]
- Kbashi, H.J.; Zajnulina, M.; Martinez, A.G.; Sergeyev, S.V. Mulitiscale spatiotemporal structures in mode-locked fiber lasers. Laser Phys. Lett. 2020, 17, 035103. [Google Scholar] [CrossRef]
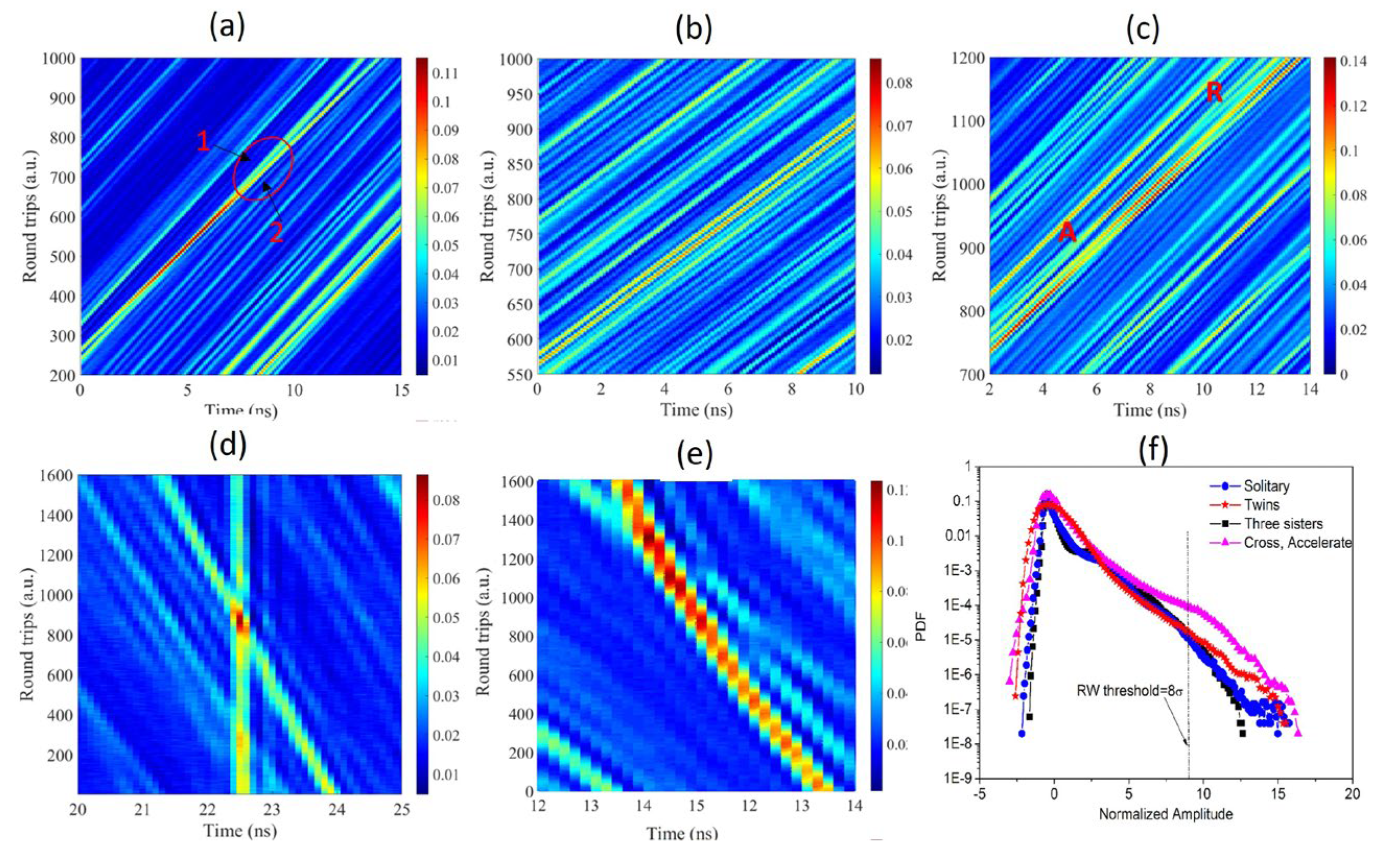
| Pattern | Lifetime (Round Trips) | Likelihood | Mechanism |
|---|---|---|---|
| FORW Lonely | Hundreds | ~0.6 | Pulse–pulse interaction |
| FORW Twins | Thousands | ~0.3 | Pulse–pulse interaction |
| FORW Three sisters | Hundreds | ~0.1 | Pulse–pulse interaction |
| FORW Accelerated | Hundreds | <0.01 | Pulse–pulse interaction |
| FORW Cross | <10−4 | <0.01 | Pulse–pulse collision |
| SORW | Hundreds of thousands | 0.195 | Polarization trapping |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kbashi, H.J.; Sergyev, S.V. Optical Rogue Waves in Fiber Lasers. Photonics 2024, 11, 657. https://doi.org/10.3390/photonics11070657
Kbashi HJ, Sergyev SV. Optical Rogue Waves in Fiber Lasers. Photonics. 2024; 11(7):657. https://doi.org/10.3390/photonics11070657
Chicago/Turabian StyleKbashi, Hani J., and Sergey V. Sergyev. 2024. "Optical Rogue Waves in Fiber Lasers" Photonics 11, no. 7: 657. https://doi.org/10.3390/photonics11070657
APA StyleKbashi, H. J., & Sergyev, S. V. (2024). Optical Rogue Waves in Fiber Lasers. Photonics, 11(7), 657. https://doi.org/10.3390/photonics11070657





