Efficient Optical Coupling between Dielectric Strip Waveguides and a Plasmonic Trench Waveguide
Abstract
1. Introduction
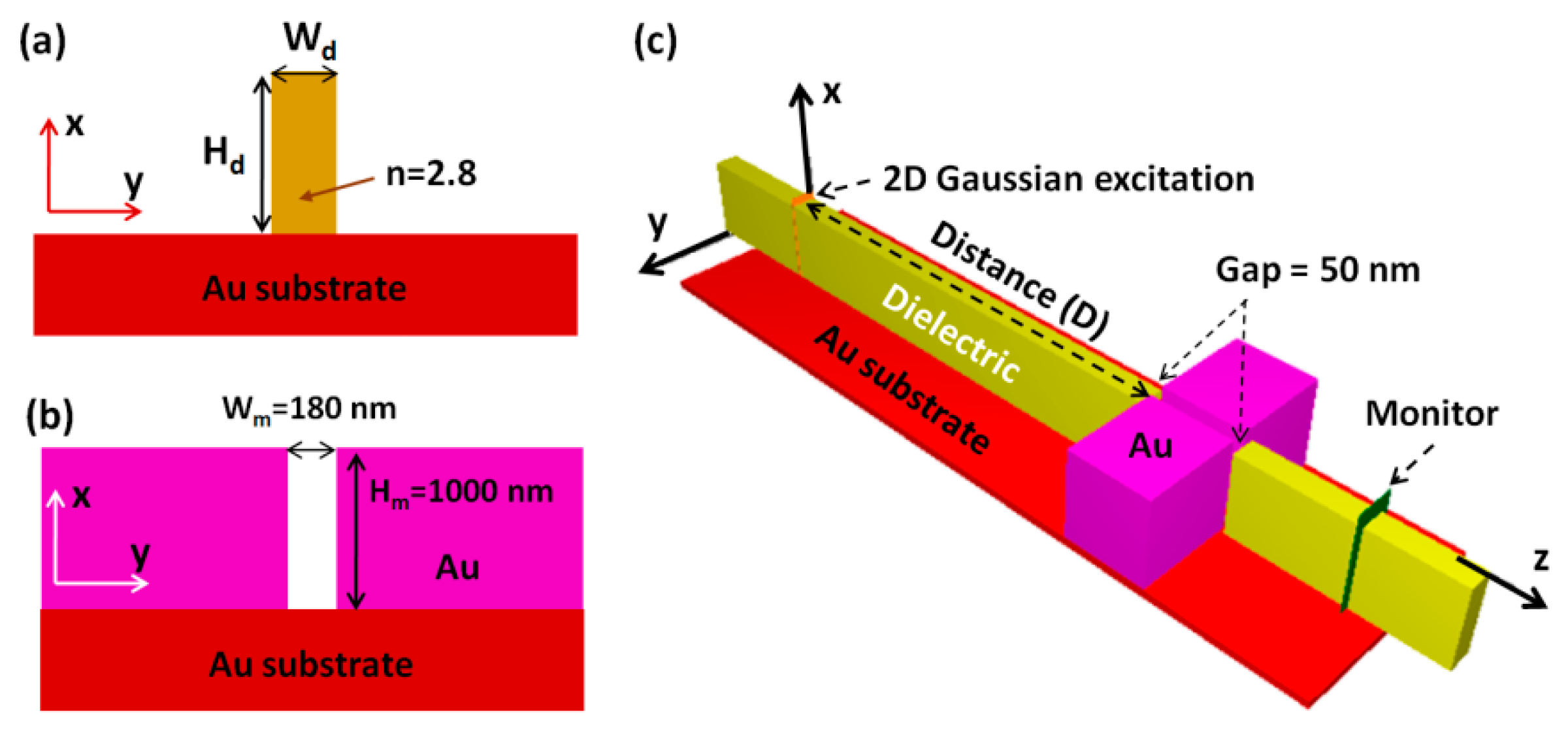
2. Simulation and Methodology
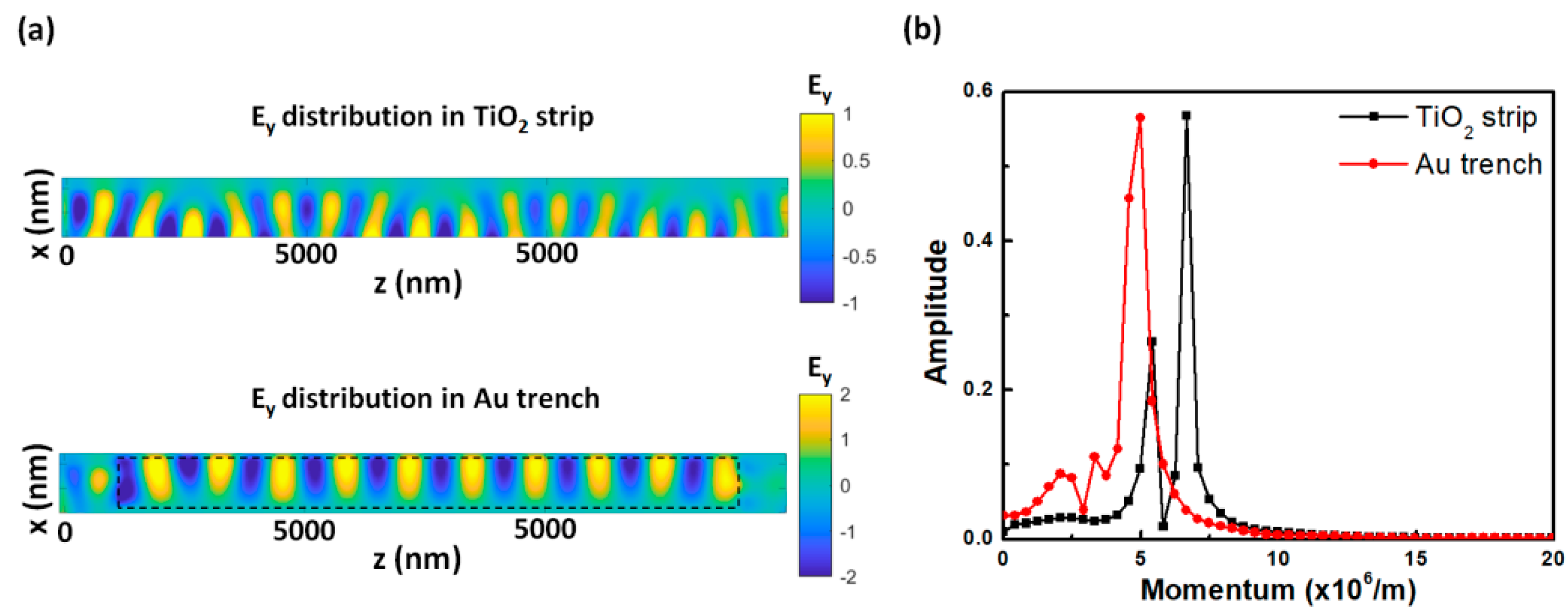
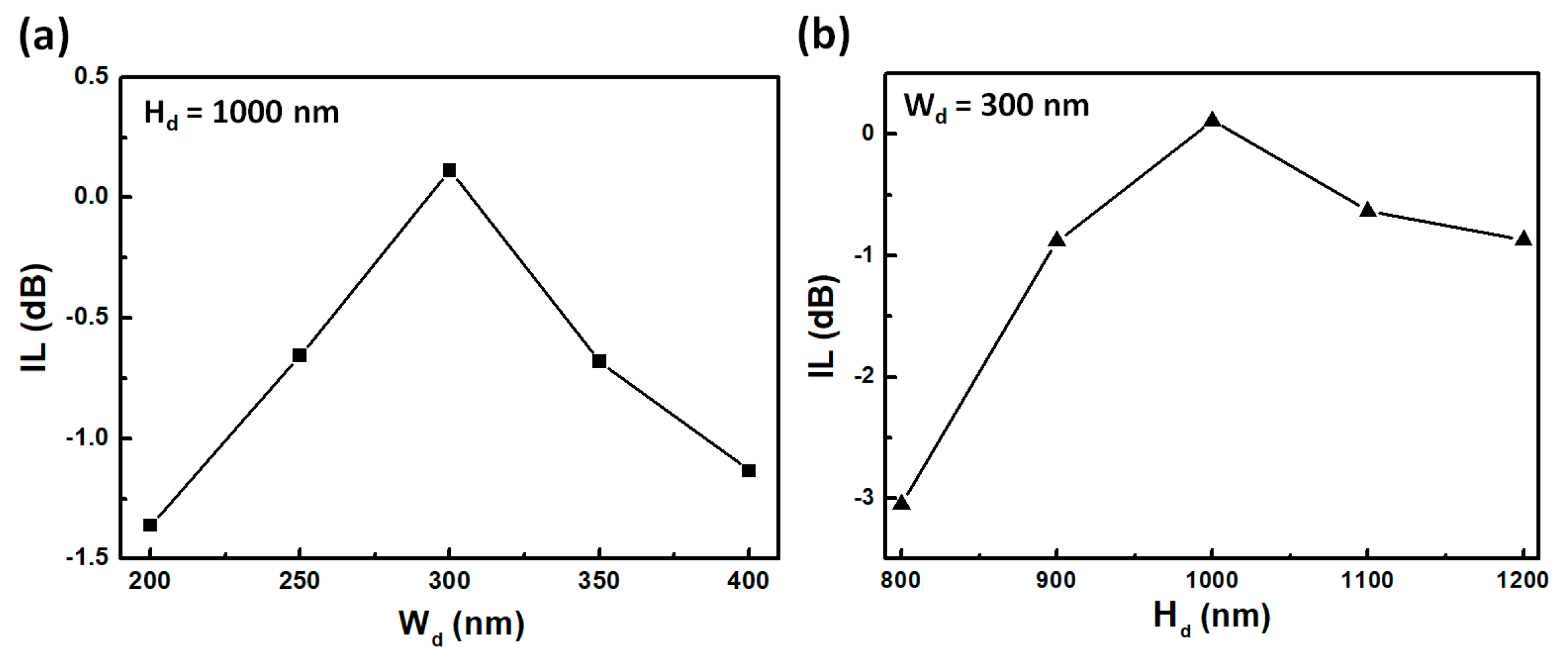
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Anwar, R.S.; Ning, H.; Mao, L. Recent advancements in surface plasmon polaritons-plasmonics in subwavelength structures in microwave and terahertz regimes. Digit. Commun. Netw. 2018, 4, 244–257. [Google Scholar] [CrossRef]
- Kim, J.T.; Park, S.; Ju, J.J.; Lee, S.; Kim, S. Low bending loss characteristics of hybrid plasmonic waveguide for flexible optical interconnect. Opt. Express 2010, 18, 24213–24220. [Google Scholar] [CrossRef]
- Tai, C.-Y.; Chang, S.H.; Chiu, T. Design and analysis of an ultra-compact and ultra-wideband polarization beam splitter based on coupled plasmonic waveguide arrays. IEEE Photonics Technol. Lett. 2007, 19, 1448–1450. [Google Scholar] [CrossRef]
- Liou, F.; Dai, D.; Wosinski, L. Ultracompact polarization beam splitter based on a dielectric-hybrid plasmonic-dielectric coupler. Opt. Lett. 2012, 37, 3372–3374. [Google Scholar] [CrossRef]
- Tai, C.-Y.; Yu, W.-H.; Chang, S.H. Giant angular dispersion mediated by plasmonic modal competition. Opt. Express 2010, 18, 24510–24515. [Google Scholar] [CrossRef]
- Jun, Y.C.; Huang, K.C.Y.; Brognersma, M.L. Plasmonic beaming and active control over fluorescent emission. Nat. Commun. 2011, 2, 283. [Google Scholar] [CrossRef]
- Gan, Q.; Gao, Y.; Bartoli, F.J. Vertical plasmonic Mach-Zehnder interferometer for sensitive optical sensing. Opt. Express 2009, 17, 20747–20755. [Google Scholar] [CrossRef]
- Gao, Y.; Gan, Q.; Xin, Z.; Cheng, X.; Bartoli, F.J. Plasmonic Mach-Zehnder interferometer for ultrasensitive on-chip biosensing. ACS Nano 2011, 5, 9836–9844. [Google Scholar] [CrossRef] [PubMed]
- Lu, H.; Liu, X.; Mao, D.; Wang, L.; Gong, Y. Tunable band-pass plasmonic waveguide filters with nanodisk resonators. Opt. Express 2010, 18, 17922–17927. [Google Scholar] [CrossRef]
- Zhuang, H.; Kong, F.; Li, K.; Sheng, S. Plasmonic bandpass filter based on graphene nanoribbon. Appl. Opt. 2015, 54, 2558–2564. [Google Scholar] [CrossRef]
- Chang, S.H.; Chiu, T.C.; Tai, C.-Y. Propagation characteristics of the supermode based on two coupled semi-infinite rib plasmonic waveguides. Opt. Express 2007, 15, 1755–1761. [Google Scholar] [CrossRef] [PubMed]
- Gramotnev, D.K.; Bozhevolnyi, S.I. Plasmonics beyond the diffraction limit. Nat. Photonics 2010, 4, 83–91. [Google Scholar] [CrossRef]
- Huong, N.T.; Chinh, N.V.; Hoang, C.M. Wedge surface Plasmon polariton waveguides based on wet-bulk micromachining. Photonics 2019, 6, 21. [Google Scholar] [CrossRef]
- Liao, Y.-S.; Wu, J.-R.; Thakur, D.; Hsu, J.-S.; Dwivedi, R.P.; Chang, S.H. Power loss reduction of angled metallic wedge plasmonic waveguides via the interplay between near-field optical coupling and modal coupling. Photonics 2022, 9, 663. [Google Scholar] [CrossRef]
- Kewes, G.; Schoengen, M.; Neitzke, O.; Lombardi, P.; Schonfeld, R.-S.; Mazzamuto, G.; Schell, A.W.; Probst, J.; Wolters, J.; Lochel, B.; et al. A realistic fabrication and design concept for quantum gates based on single emitters integrated in plasmonic dielectric waveguides structures. Sci. Rep. 2016, 6, 28877. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Hu, G.; Zhang, S.; Gao, D.; Sun, Y.; Wang, F. Gain characteristics of the hybrid solt waveguide amplifiers integrated with NaYF4:Er3+ NPs-PMMA covalently linked nanocomposites. RSC. Adv. 2020, 10, 11148–11155. [Google Scholar] [CrossRef] [PubMed]
- Veronis, G.; Fan, S. Theoretical investigation of compact couplers between dielectric slab waveguides and two-dimensional metal-dielectric-metal plasmonic waveguides. Opt. Express 2007, 15, 1211–1221. [Google Scholar] [CrossRef] [PubMed]
- Tian, J.; Yu, S.; Yan, W.; Qiu, M. Broadband high-efficiency surface-plasmon-polariton coupler with silicon-metal interface. Appl. Phys. Lett. 2009, 95, 013504. [Google Scholar] [CrossRef]
- Berini, P. Long-range surface Plasmon polaritons. Adv. Opt. Photonics 2009, 1, 484–588. [Google Scholar] [CrossRef]
- Chang, S.H.; Tai, C.-Y. Broadband energy conversion between off-plane Gaussian lightwave and in-plane surface Plasmon waves. IEEE Photonics Technol. Lett. 2011, 23, 1727–1729. [Google Scholar] [CrossRef]
- Manfrinato, V.R.; Zhang, L.; Su, D.; Duan, H.; Hobbs, R.G.; Stach, E.A.; Berggren, K.K. Resolution limits of electron-beam lithography toward atomic scale. Nano. Lett. 2013, 13, 1555–1558. [Google Scholar] [CrossRef] [PubMed]
- Wongwanitwattana, C.; Shah, V.A.; Myronov, M.; Parker, E.H.C.; Whall, T.; Leadley, D.R. Precision plasma etching of Si, Ge, and Ge:P by SF6 with added O2. J. Vac. Sci. Technol. A 2014, 32, 031302. [Google Scholar] [CrossRef]
- Mahmoodi, N.; Rushdi, A.I.; Bowen, J.; Sabouri, A.; Anthony, C.; Mendes, P.M.; Preece, J.A. Room temperature thermally evaporated thin Au film on Si suitable for application of thiol self-assembled monolayers in micro-nano-electro-mechanical-systems sensors. J. Vac. Sci. Technol. A 2017, 35, 041514. [Google Scholar] [CrossRef]
- Wright, J.T.; Carbaugh, D.J.; Haggerty, M.E.; Richard, A.L.; Ingram, D.C.; Kaya, S.; Jadwisienczak, W.W.; Rahman, F. Thermal oxidation of silicon in a residual oxygen atmosphere-the RESOX process-for self-limiting growth of thin silicon dioxide films. Semicond. Sci. Technol. 2016, 31, 105007. [Google Scholar] [CrossRef]
- Heo, C.H.H.; Lee, S.-B.; Boo, J.-H. Deposition of TiO2 thin films using RF magnetron sputtering method and study of their surface characteristics. Thin Solid Films 2005, 475, 183–188. [Google Scholar] [CrossRef]
- Dong, B.; Ma, Y.; Ren, Z.; Lee, C. Recent progress in nanoplasmonics-based integrated optical micro/nano-systems. J. Phys. D Appl. Phys. 2020, 53, 213001. [Google Scholar] [CrossRef]
- Udagedara, I.; Premaratne, M.; Rukhlenko, I.D.; Hattori, H.T.; Agrawal, G.P. Unified perfectly matched layer for finite-difference time-domain modeling of dispersive optical materials. Opt. Express 2009, 17, 21179–21190. [Google Scholar] [CrossRef]
- Jolivet, A.; Labbe, C.; Frilay, C.; Debieu, O.; Marie, P.; Horcholle, B.; Lemarie, F.; Portier, X.; Grygiel, C.; Duprey, S.; et al. Structural, optical, and electrical properties of TiO2 thin films deposited by ALD: Impact of the substrate, the deposited thickness and the deposition temperature. Appl. Surf. Sci. 2023, 608, 155214. [Google Scholar] [CrossRef]
- Johnson, P.B.; Christy, R.W. Optical constants of the noble metals. Phys. Rev. B 1972, 6, 4370–7379. [Google Scholar] [CrossRef]
- Qin, X.; He, Y.; Sun, W.; Fu, P.; Wang, S.; Zhou, Z.; Li, Y. Stepped waveguide metamaterials as low-loss effective replica of surface Plasmon polaritons. Nanophotonics 2023, 12, 1285–1293. [Google Scholar] [CrossRef]
- Shneen, W.F.; Ameen, S.M.M. Controllable surface Plasmon polariton propagation length using a suitable quantum dot material. Braz. J. Phys. 2024, 54, 59. [Google Scholar] [CrossRef]
- Chaparala, R.; Imamavali, S.; Tupakula, S. Enhancement of spoof surface plasmon polariton waveguide performance through modified groove width. Opt. Eng. 2024, 63, 055102. [Google Scholar] [CrossRef]
- Schouten, H.F.; Visser, T.D.; Lenstra, D.; Blok, H. Light transmission through a subwavelength slit: Waveguiding and optical vortices. Phys. Rev. E 2003, 67, 036608. [Google Scholar] [CrossRef] [PubMed]
- Sturman, B.; Podivilov, E.; Gorkunov, M. Theory of extraordinary light transmission through arrays of subwavelength slits. Phys. Rev. B 2008, 77, 075106. [Google Scholar] [CrossRef]

| Propagation Characteristics | TiO2 Strip on Au Substrate | Au Trench |
|---|---|---|
| kR(×106/m) | 6.648 | 4.706 |
| kI(×104/m) | 5.175 | 0.610 |
| kR/kI | 128.46 | 771.48 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.-R.; Chandel, A.; Chuang, C.; Chang, S.H. Efficient Optical Coupling between Dielectric Strip Waveguides and a Plasmonic Trench Waveguide. Photonics 2024, 11, 608. https://doi.org/10.3390/photonics11070608
Wu J-R, Chandel A, Chuang C, Chang SH. Efficient Optical Coupling between Dielectric Strip Waveguides and a Plasmonic Trench Waveguide. Photonics. 2024; 11(7):608. https://doi.org/10.3390/photonics11070608
Chicago/Turabian StyleWu, Jia-Ren, Anjali Chandel, Chiashain Chuang, and Sheng Hsiung Chang. 2024. "Efficient Optical Coupling between Dielectric Strip Waveguides and a Plasmonic Trench Waveguide" Photonics 11, no. 7: 608. https://doi.org/10.3390/photonics11070608
APA StyleWu, J.-R., Chandel, A., Chuang, C., & Chang, S. H. (2024). Efficient Optical Coupling between Dielectric Strip Waveguides and a Plasmonic Trench Waveguide. Photonics, 11(7), 608. https://doi.org/10.3390/photonics11070608







