Generating Optical Vortex Array Laser Beams of Superimposing Hermite–Gaussian Beams with a Dual–Phase Modulation Digital Laser System
Abstract
1. Introduction
2. Approach and Simulation Verification
2.1. Forming Vortex Array with Generating Superimposing Degenerated Hermite–Gaussian Modes
2.2. Laser Model and Simulation Verification
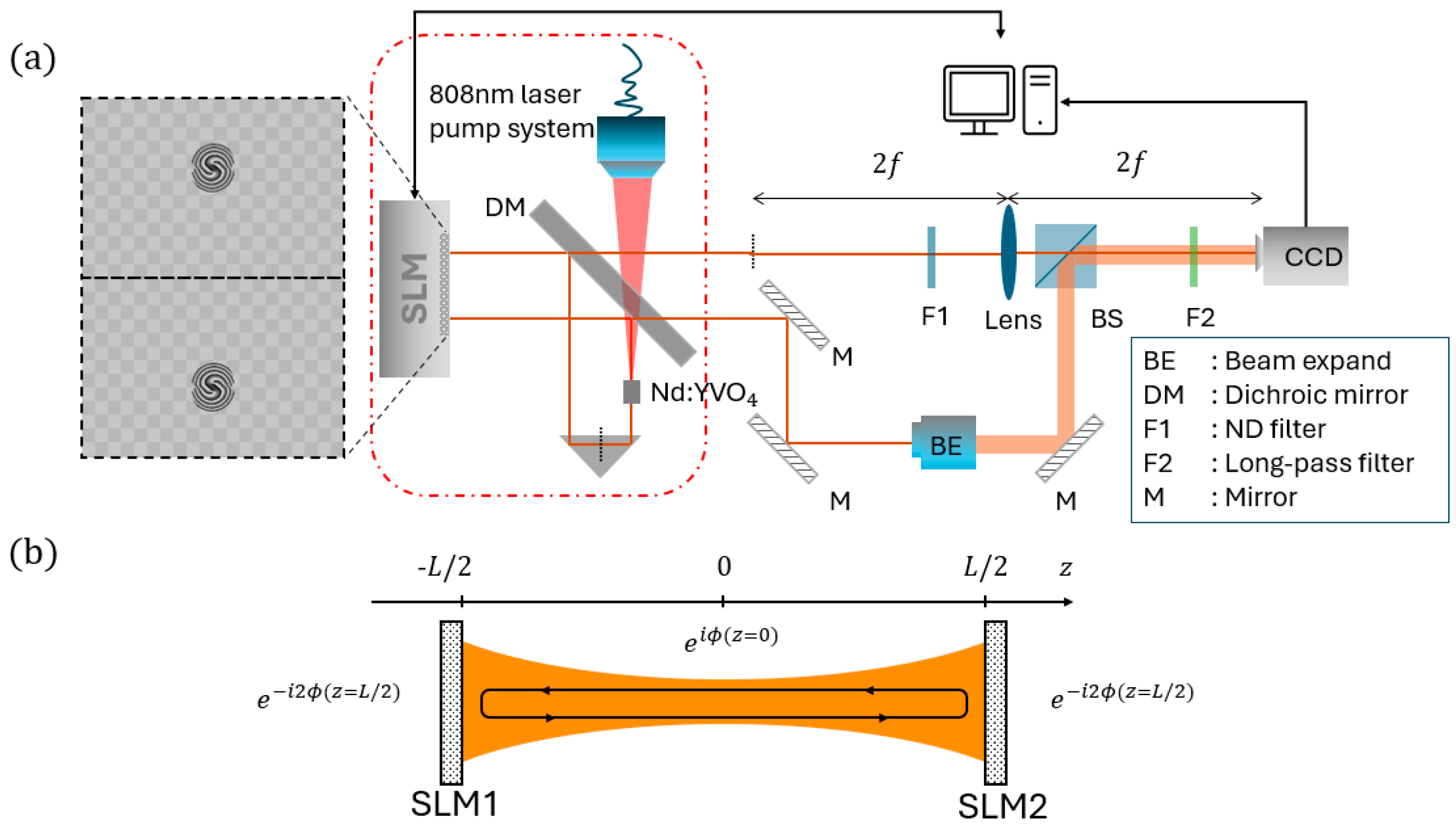
3. Experiment Setup
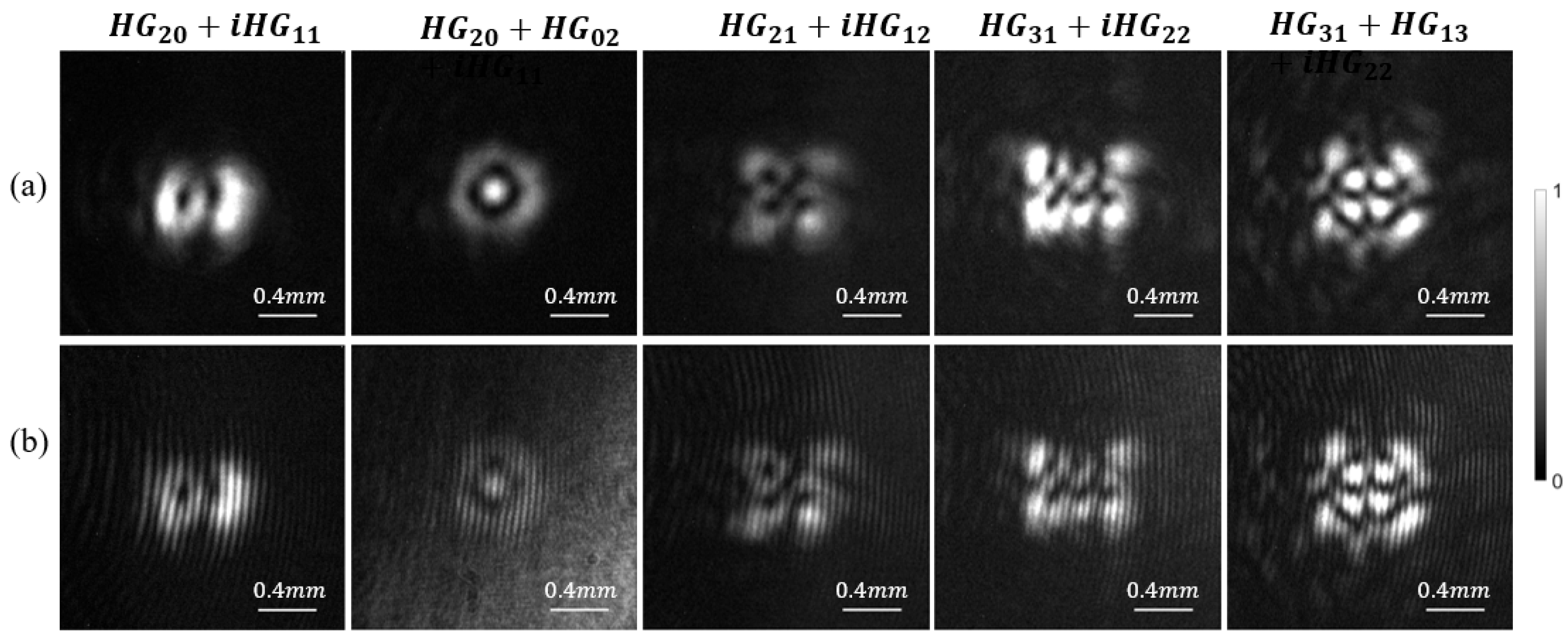
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef] [PubMed]
- Rubinsztein-Dunlop, H.; Forbes, A.; Berry, M.V.; Dennis, M.R.; Andrews, D.L.; Mansuripur, M.; Denz, C.; Alpmann, C.; Banzer, P.; Bauer, T. Roadmap on structured light. J. Opt. 2016, 19, 013001. [Google Scholar] [CrossRef]
- He, C.; Shen, Y.; Forbes, A. Towards higher-dimensional structured light. Light Sci. Appl. 2022, 11, 205. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.; Wang, X.; Xie, Z.; Min, C.; Fu, X.; Liu, Q.; Gong, M.; Yuan, X. Optical vortices 30 years on: OAM manipulation from topological charge to multiple singularities. Light Sci. Appl. 2019, 8, 90. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Wang, Y.; Yuan, Y.; Burokur, S.N. A Review of Orbital Angular Momentum Vortex Beams Generation: From Traditional Methods to Metasurfaces. Appl. Sci. 2020, 10, 1015. [Google Scholar] [CrossRef]
- Lian, Y.; Qi, X.; Wang, Y.; Bai, Z.; Wang, Y.; Lu, Z. OAM beam generation in space and its applications: A review. Opt. Lasers Eng. 2022, 151, 106923. [Google Scholar] [CrossRef]
- Forbes, A. Advances in Orbital Angular Momentum Lasers. J. Light. Technol. 2023, 41, 2079–2086. [Google Scholar] [CrossRef]
- Wang, X.; Nie, Z.; Liang, Y.; Wang, J.; Li, T.; Jia, B. Recent advances on optical vortex generation. Nanophotonics 2018, 7, 1533–1556. [Google Scholar] [CrossRef]
- Beijersbergen, M.W.; Coerwinkel, R.P.C.; Kristensen, M.; Woerdman, J.P. Helical-wavefront laser beams produced with a spiral phaseplate. Opt. Commun. 1994, 112, 321–327. [Google Scholar] [CrossRef]
- Beijersbergen, M.W.; Allen, L.; van der Veen, H.E.L.O.; Woerdman, J.P. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 1993, 96, 123–132. [Google Scholar] [CrossRef]
- Kumar, A.; Vaity, P.; Banerji, J.; Singh, R.P. Making an optical vortex and its copies using a single spatial light modulator. Phys. Lett. A 2011, 375, 3634–3640. [Google Scholar] [CrossRef]
- Gong, L.; Ren, Y.; Liu, W.; Wang, M.; Zhong, M.; Wang, Z.; Li, Y. Generation of cylindrically polarized vector vortex beams with digital micromirror device. J. Appl. Phys. 2014, 116, 183105. [Google Scholar] [CrossRef]
- Yi, J.; Li, D.; Feng, R.; Ratni, B.; Jiang, Z.H.; De Lustrac, A.; Werner, D.H.; Burokur, S.N. Design and validation of a metasurface lens for converging vortex beams. Appl. Phys. Express 2019, 12, 084501. [Google Scholar] [CrossRef]
- Chen, Q.; Qu, G.; Yin, J.; Wang, Y.; Ji, Z.; Yang, W.; Wang, Y.; Yin, Z.; Song, Q.; Kivshar, Y.; et al. Highly efficient vortex generation at the nanoscale. Nat. Nanotechnol. 2024. [Google Scholar] [CrossRef] [PubMed]
- Ito, A.; Kozawa, Y.; Sato, S. Generation of hollow scalar and vector beams using a spot-defect mirror. J. Opt. Soc. Am. A 2010, 27, 2072–2077. [Google Scholar] [CrossRef]
- Kim, D.J.; Kim, J.W. Direct generation of an optical vortex beam in a single-frequency Nd:YVO4 laser. Opt. Lett. 2015, 40, 399–402. [Google Scholar] [CrossRef]
- Naidoo, D.; Roux, F.S.; Dudley, A.; Litvin, I.; Piccirillo, B.; Marrucci, L.; Forbes, A. Controlled generation of higher-order Poincaré sphere beams from a laser. Nat. Photonics 2016, 10, 327–332. [Google Scholar] [CrossRef]
- Sroor, H.; Huang, Y.-W.; Sephton, B.; Naidoo, D.; Vallés, A.; Ginis, V.; Qiu, C.-W.; Ambrosio, A.; Capasso, F.; Forbes, A. High-purity orbital angular momentum states from a visible metasurface laser. Nat. Photonics 2020, 14, 498–503. [Google Scholar] [CrossRef]
- Oron, R.; Danziger, Y.; Davidson, N.; Friesem, A.A.; Hasman, E. Laser mode discrimination with intra-cavity spiral phase elements. Opt. Commun. 1999, 169, 115–121. [Google Scholar] [CrossRef]
- Eggleston, M.; Godat, T.; Munro, E.; Alonso, M.A.; Shi, H.; Bhattacharya, M. Ray transfer matrix for a spiral phase plate. J. Opt. Soc. Am. A 2013, 30, 2526–2530. [Google Scholar] [CrossRef]
- Miao, P.; Zhang, Z.; Sun, J.; Walasik, W.; Longhi, S.; Litchinitser, N.M.; Feng, L. Orbital angular momentum microlaser. Science 2016, 353, 464–467. [Google Scholar] [CrossRef] [PubMed]
- Ngcobo, S.; Litvin, I.; Burger, L.; Forbes, A. A digital laser for on-demand laser modes. Nat. Commun. 2013, 4, 2289. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.Y.; Li, Y.W. Spiral-Phase-Defect Resonator and Its Application in Vortex Laser of Controllable Topological Charges. IEEE Photonics J. 2022, 14, 1548307. [Google Scholar] [CrossRef]
- Thi, L.L.N.; Chang, K.-C.; Shu, S.-C. Dual modulation digital laser for generating vortex beams with tunable orbital angular momentum. Opt. Laser Technol. 2024, 176, 110928. [Google Scholar] [CrossRef]
- Du, J.; Quan, Z.; Li, K.; Wang, J. Optical vortex array: Generation and applications. Chin. Opt. Lett. 2024, 22, 020011. [Google Scholar]
- Zhu, L.; Tang, M.; Li, H.; Tai, Y.; Li, X. Optical vortex lattice: An exploitation of orbital angular momentum. Nanophotonics 2021, 10, 2487–2496. [Google Scholar] [CrossRef]
- Li, X.; Chu, J.; Smithwick, Q.; Chu, D. Automultiscopic displays based on orbital angular momentum of light. J. Opt. 2016, 18, 085608. [Google Scholar] [CrossRef]
- Lei, T.; Zhang, M.; Li, Y.; Jia, P.; Liu, G.N.; Xu, X.; Li, Z.; Min, C.; Lin, J.; Yu, C.; et al. Massive individual orbital angular momentum channels for multiplexing enabled by Dammann gratings. Light Sci. Appl. 2015, 4, e257. [Google Scholar] [CrossRef]
- Wang, J. Advances in communications using optical vortices. Photon. Res. 2016, 4, B14–B28. [Google Scholar] [CrossRef]
- Guo, C.-S.; Yu, Y.-N.; Hong, Z. Optical sorting using an array of optical vortices with fractional topological charge. Opt. Commun. 2010, 283, 1889–1893. [Google Scholar] [CrossRef]
- Curtis, J.E.; Koss, B.A.; Grier, D.G. Dynamic holographic optical tweezers. Opt. Commun. 2002, 207, 169–175. [Google Scholar] [CrossRef]
- Ladavac, K.; Grier, D.G. Microoptomechanical pumps assembled and driven by holographic optical vortex arrays. Opt. Express 2004, 12, 1144–1149. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.C.; Lu, T.H.; Huang, K.F.; Chen, Y.F. Generation of optical vortex array with transformation of standing-wave Laguerre-Gaussian mode. Opt. Express 2011, 19, 10293–10303. [Google Scholar] [CrossRef]
- Abramochkin, E.; Razueva, E.; Volostnikov, V. General astigmatic transform of Hermite–Laguerre–Gaussian beams. J. Opt. Soc. Am. A 2010, 27, 2506–2513. [Google Scholar] [CrossRef] [PubMed]
- Fu, S.; Wang, T.; Gao, C. Perfect optical vortex array with controllable diffraction order and topological charge. J. Opt. Soc. Am. A 2016, 33, 1836–1842. [Google Scholar] [CrossRef]
- Lu, Y.; Jiang, B.; Lü, S.; Liu, Y.; Li, S.; Cao, Z.; Qi, X. Arrays of Gaussian vortex, Bessel and Airy beams by computer-generated hologram. Opt. Commun. 2016, 363, 85–90. [Google Scholar] [CrossRef]
- Kapoor, A.; Kumar, M.; Senthilkumaran, P.; Joseph, J. Optical vortex array in spatially varying lattice. Opt. Commun. 2016, 365, 99–102. [Google Scholar] [CrossRef]
- Ma, H.; Li, X.; Tai, Y.; Li, H.; Wang, J.; Tang, M.; Tang, J.; Wang, Y.; Nie, Z. Generation of Circular Optical Vortex Array. Ann. Phys. 2017, 529, 1700285. [Google Scholar] [CrossRef]
- García-Martínez, P.; Sánchez-López, M.M.; Davis, J.A.; Cottrell, D.M.; Sand, D.; Moreno, I. Generation of Bessel beam arrays through Dammann gratings. Appl. Opt. 2012, 51, 1375–1381. [Google Scholar] [CrossRef]
- Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Planar Photonics with Metasurfaces. Science 2013, 339, 1232009. [Google Scholar] [CrossRef]
- Yu, N.; Capasso, F. Flat optics with designer metasurfaces. Nat. Mater. 2014, 13, 139–150. [Google Scholar] [CrossRef] [PubMed]
- Maguid, E.; Chriki, R.; Yannai, M.; Kleiner, V.; Hasman, E.; Friesem, A.A.; Davidson, N. Topologically Controlled Intracavity Laser Modes Based on Pancharatnam-Berry Phase. ACS Photonics 2018, 5, 1817–1821. [Google Scholar] [CrossRef]
- Jin, J.; Pu, M.; Wang, Y.; Li, X.; Ma, X.; Luo, J.; Zhao, Z.; Gao, P.; Luo, X. Multi-Channel Vortex Beam Generation by Simultaneous Amplitude and Phase Modulation with Two-Dimensional Metamaterial. Adv. Mater. Technol. 2017, 2, 1600201. [Google Scholar] [CrossRef]
- Piccardo, M.; Ambrosio, A. Recent twists in twisted light: A Perspective on optical vortices from dielectric metasurfaces. Appl. Phys. Lett. 2020, 117, 140501. [Google Scholar] [CrossRef]
- Shen, Y.; Wan, Z.; Fu, X.; Liu, Q.; Gong, M. Vortex lattices with transverse-mode-locking states switching in a large-aperture off-axis-pumped solid-state laser. J. Opt. Soc. Am. B 2018, 35, 2940–2944. [Google Scholar] [CrossRef]
- Huyet, G.; Martinoni, M.C.; Tredicce, J.R.; Rica, S. Spatiotemporal Dynamics of Lasers with a Large Fresnel Number. Phys. Rev. Lett. 1995, 75, 4027–4030. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Zhao, C. Spontaneous phase and frequency locking of transverse modes in different orders. Phys. Rev. Appl. 2020, 13, 024010. [Google Scholar] [CrossRef]
- Zhang, S.; Fu, Z.; Lai, L.; Jia, F.; Qiao, D.; Fan, Y.; Li, K.; Copner, N. Transverse mode locking of different frequency-degenerate families based on annular beam pumping. Opt. Lett. 2021, 46, 3195–3198. [Google Scholar] [CrossRef]
- Chen, K.; Xu, L.; Ni, A.; Tang, J.; Yi, K.; Jia, F.; Qiao, D.; Li, K.; Copner, N. Generation of a vortex point adjustable vortex array based on decentered annular beam pumping. Opt. Lett. 2023, 48, 2599–2602. [Google Scholar] [CrossRef]
- Zhang, Z.; Gao, Y.; Wang, X.; Zhao, S.; Jie, Y.; Zhao, C. Selective Generation of Laser Transverse Modes by Gain Regulation with a Digital Micromirror Device. IEEE Photonics Technol. Lett. 2022, 34, 420–423. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, Z.; Gao, Y.; Zhao, S.; Jie, Y.; Zhao, C. Investigation on the Formation of Laser Transverse Pattern Possessing Optical Lattices. Front. Phys. 2022, 9, 801916. [Google Scholar] [CrossRef]
- Chen, Y.F.; Lan, Y.P. Transverse pattern formation of optical vortices in a microchip laser with a large Fresnel number. Phys. Rev. A 2001, 65, 013802. [Google Scholar] [CrossRef]
- Piccardo, M.; de Oliveira, M.; Toma, A.; Aglieri, V.; Forbes, A.; Ambrosio, A. Vortex laser arrays with topological charge control and self-healing of defects. Nat. Photonics 2022, 16, 359–365. [Google Scholar] [CrossRef]
- Qiao, X.; Midya, B.; Gao, Z.; Zhang, Z.; Zhao, H.; Wu, T.; Yim, J.; Agarwal, R.; Litchinitser, N.M.; Feng, L. Higher-dimensional supersymmetric microlaser arrays. Science 2021, 372, 403–408. [Google Scholar] [CrossRef] [PubMed]
- Cai, X.; Wang, J.; Strain, M.J.; Johnson-Morris, B.; Zhu, J.; Sorel, M.; O’Brien, J.L.; Thompson, M.G.; Yu, S. Integrated Compact Optical Vortex Beam Emitters. Science 2012, 338, 363–366. [Google Scholar] [CrossRef] [PubMed]
- Porfirev, A.P.; Khonina, S.N. Simple method for efficient reconfigurable optical vortex beam splitting. Opt. Express 2017, 25, 18722–18735. [Google Scholar] [CrossRef]
- Goodman, J.W. Introduction to Fourier Optics; Roberts and Company Publishers: Greenwood Village, CO, USA, 2005. [Google Scholar]
- Fu, S.; Wang, T.; Gao, C. Generating perfect polarization vortices through encoding liquid-crystal display devices. Appl. Opt. 2016, 55, 6501–6505. [Google Scholar] [CrossRef]
- Chu, S.-C.; Otsuka, K. Numerical study for selective excitation of Ince-Gaussian modes in end-pumped solid-state lasers. Opt. Express 2007, 15, 16506–16519. [Google Scholar] [CrossRef] [PubMed]
- Endo, M.; Kawakami, M.; Nanri, K.; Takeda, S.; Fujioka, T. Two-dimensional simulation of an unstable resonator with a stable core. Appl. Opt. 1999, 38, 3298–3307. [Google Scholar] [CrossRef]
- Hu, A.; Lei, J.; Chen, P.; Wang, Y.; Li, S. Numerical investigation on the generation of high-order Laguerre-Gaussian beams in end-pumped solid-state lasers by introducing loss control. Appl. Opt. 2014, 53, 7845–7853. [Google Scholar] [CrossRef]
- Siegman, A.E. Lasers; University Science Books: Sausalito, CA, USA, 1986. [Google Scholar]
- Carter, W.H. Spot size and divergence for Hermite Gaussian beams of any order. Appl. Opt. 1980, 19, 1027–1029. [Google Scholar] [CrossRef] [PubMed]
- Tradonsky, C.; Mahler, S.; Cai, G.; Pal, V.; Chriki, R.; Friesem, A.A.; Davidson, N. High-resolution digital spatial control of a highly multimode laser. Optica 2021, 8, 880–884. [Google Scholar] [CrossRef]
- Song, L.-M.; Yang, Z.-J.; Li, X.-L.; Zhang, S.-M. Coherent superposition propagation of Laguerre–Gaussian and Hermite–Gaussian solitons. Appl. Math. Lett. 2020, 102, 106114. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen Thi, L.L.; Tsai, K.-F.; Chu, S.-C. Generating Optical Vortex Array Laser Beams of Superimposing Hermite–Gaussian Beams with a Dual–Phase Modulation Digital Laser System. Photonics 2024, 11, 563. https://doi.org/10.3390/photonics11060563
Nguyen Thi LL, Tsai K-F, Chu S-C. Generating Optical Vortex Array Laser Beams of Superimposing Hermite–Gaussian Beams with a Dual–Phase Modulation Digital Laser System. Photonics. 2024; 11(6):563. https://doi.org/10.3390/photonics11060563
Chicago/Turabian StyleNguyen Thi, Ly Ly, Ko-Fan Tsai, and Shu-Chun Chu. 2024. "Generating Optical Vortex Array Laser Beams of Superimposing Hermite–Gaussian Beams with a Dual–Phase Modulation Digital Laser System" Photonics 11, no. 6: 563. https://doi.org/10.3390/photonics11060563
APA StyleNguyen Thi, L. L., Tsai, K.-F., & Chu, S.-C. (2024). Generating Optical Vortex Array Laser Beams of Superimposing Hermite–Gaussian Beams with a Dual–Phase Modulation Digital Laser System. Photonics, 11(6), 563. https://doi.org/10.3390/photonics11060563




