Time–Frequency and Spectrum Analyses of All-Solid-State Self-Mode-Locked Burst Pulse Lasers
Abstract
1. Introduction
2. Theoretical Model
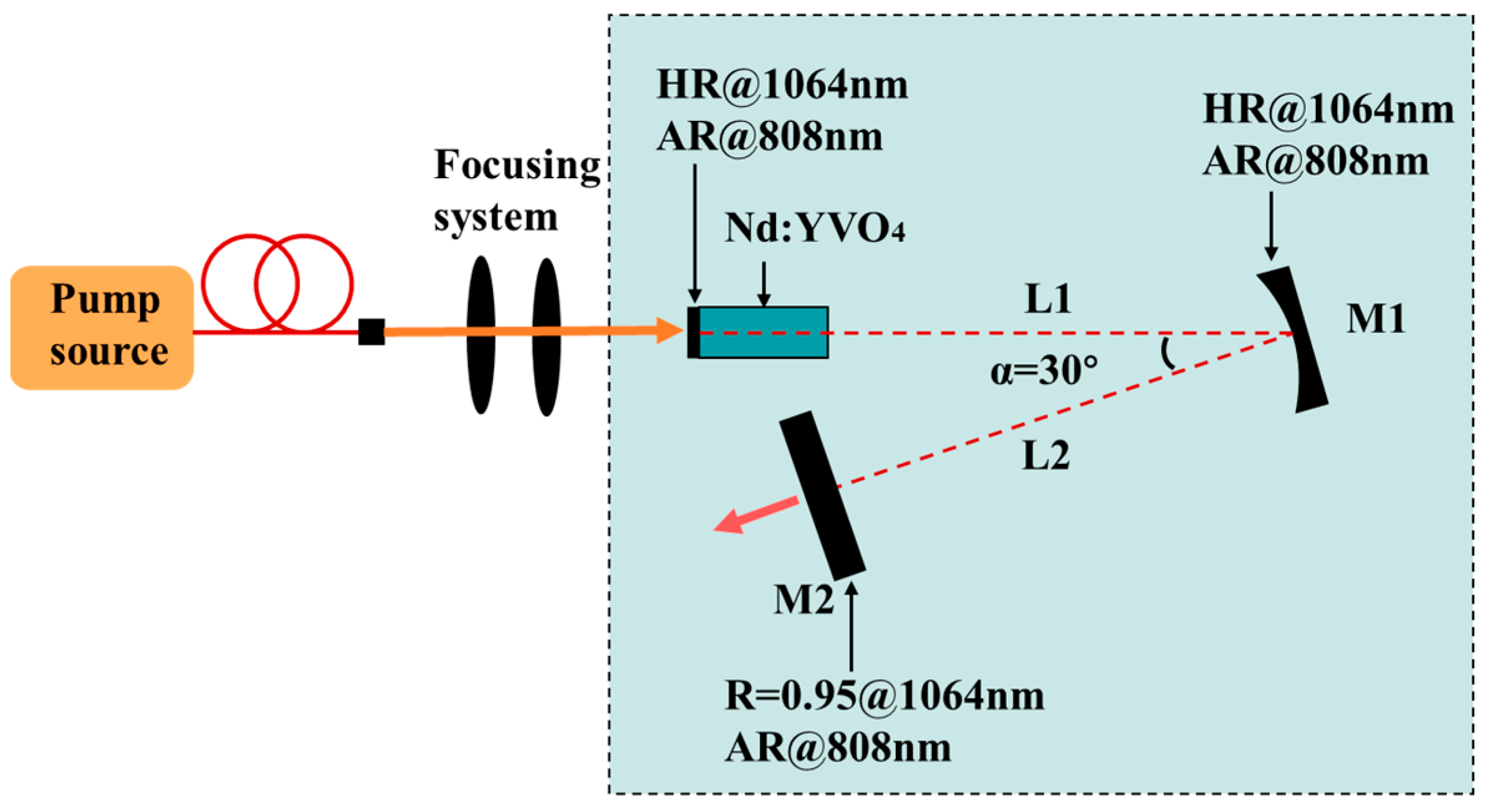
3. Experimental Setup
4. Experimental Results
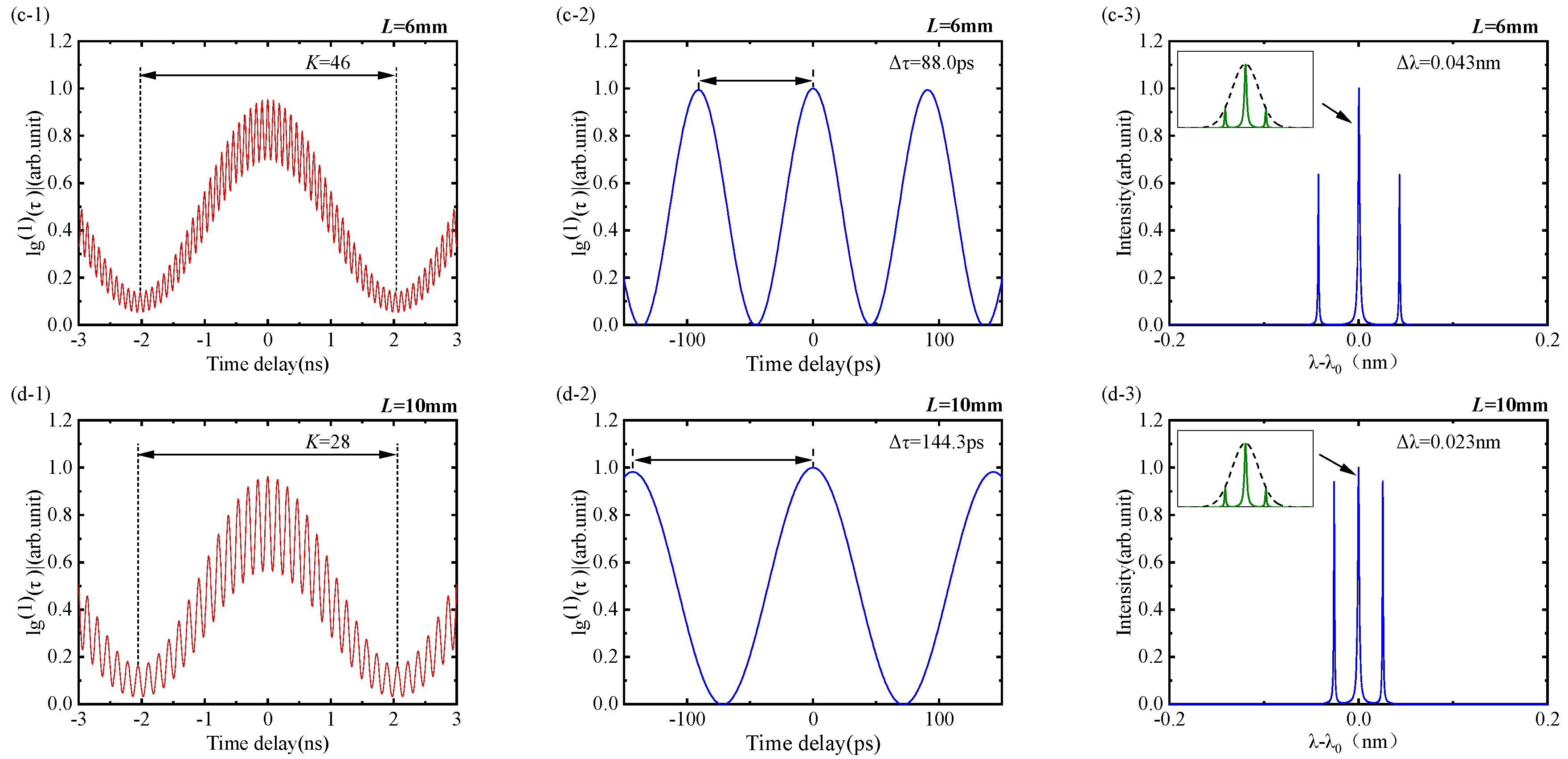
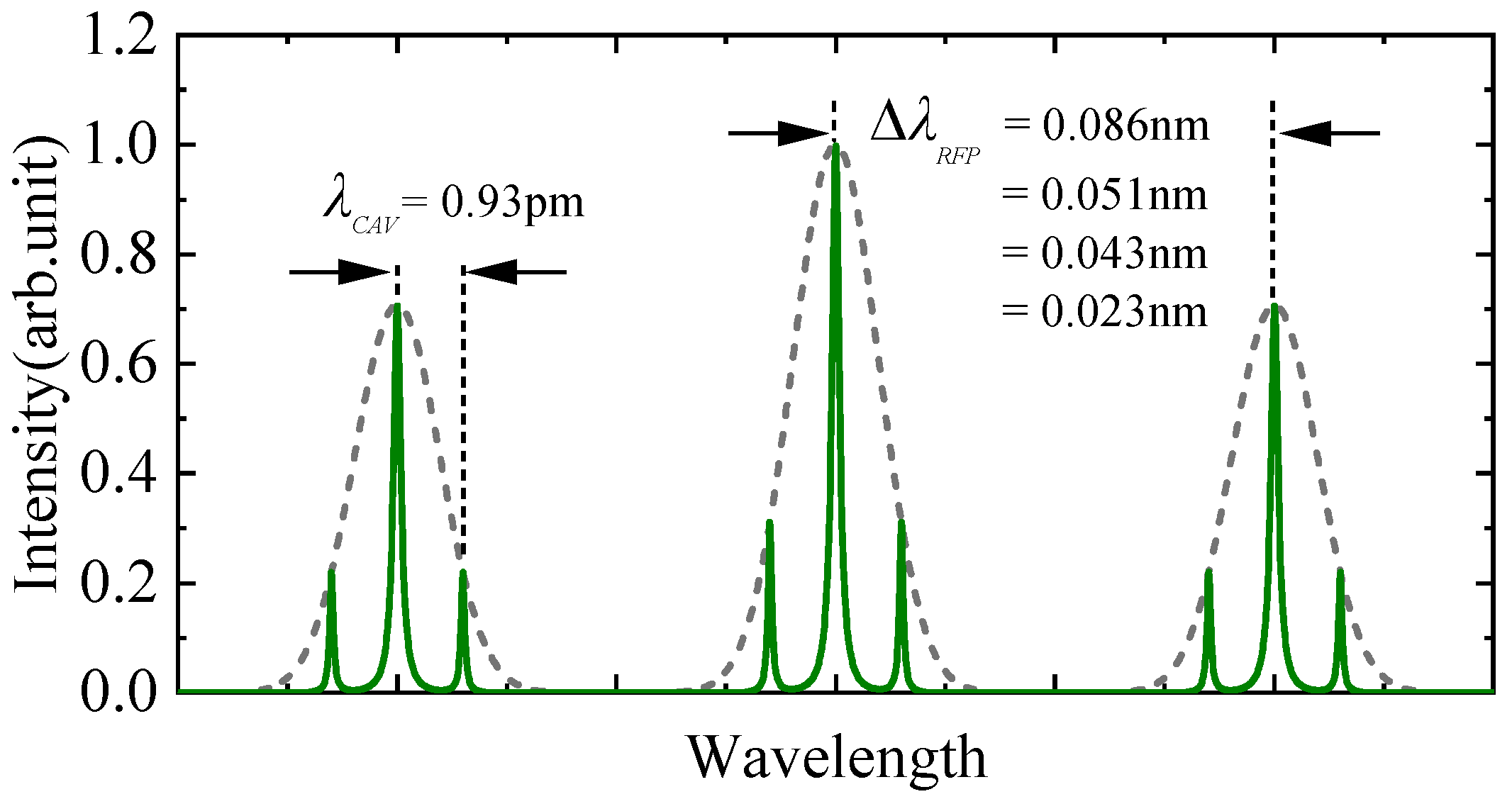
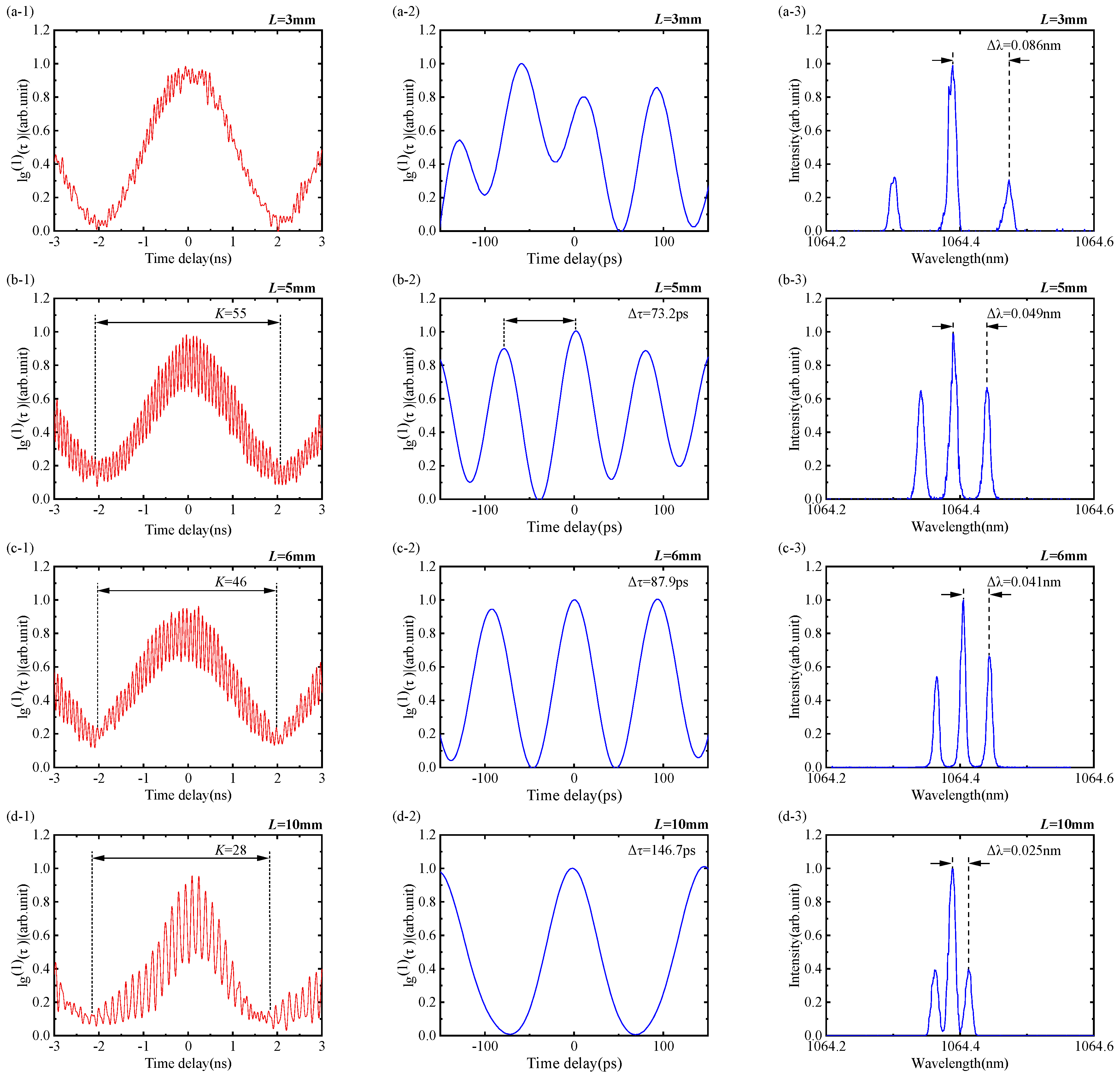
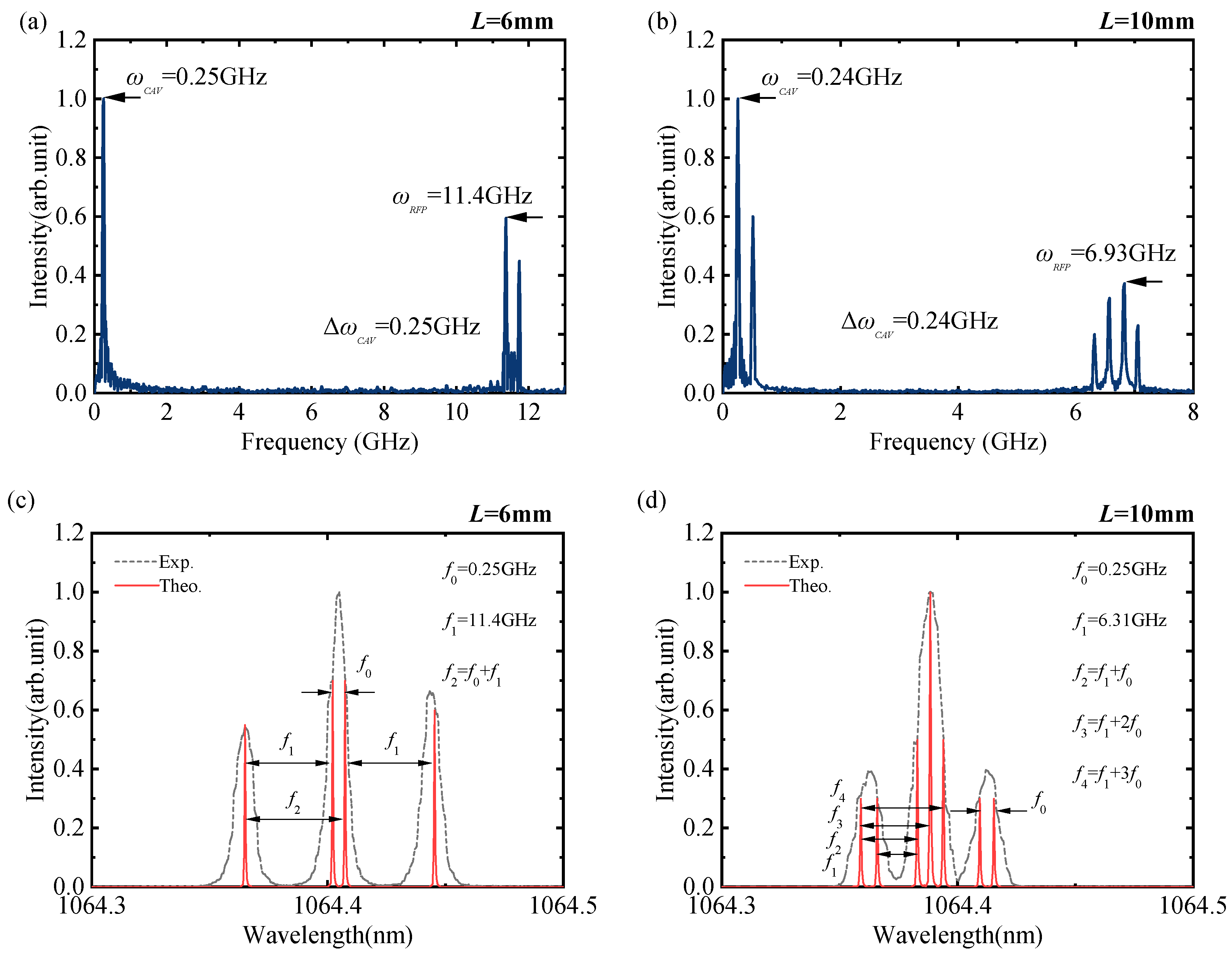
4.1. Influence on the Characteristics of Output Burst Pulses
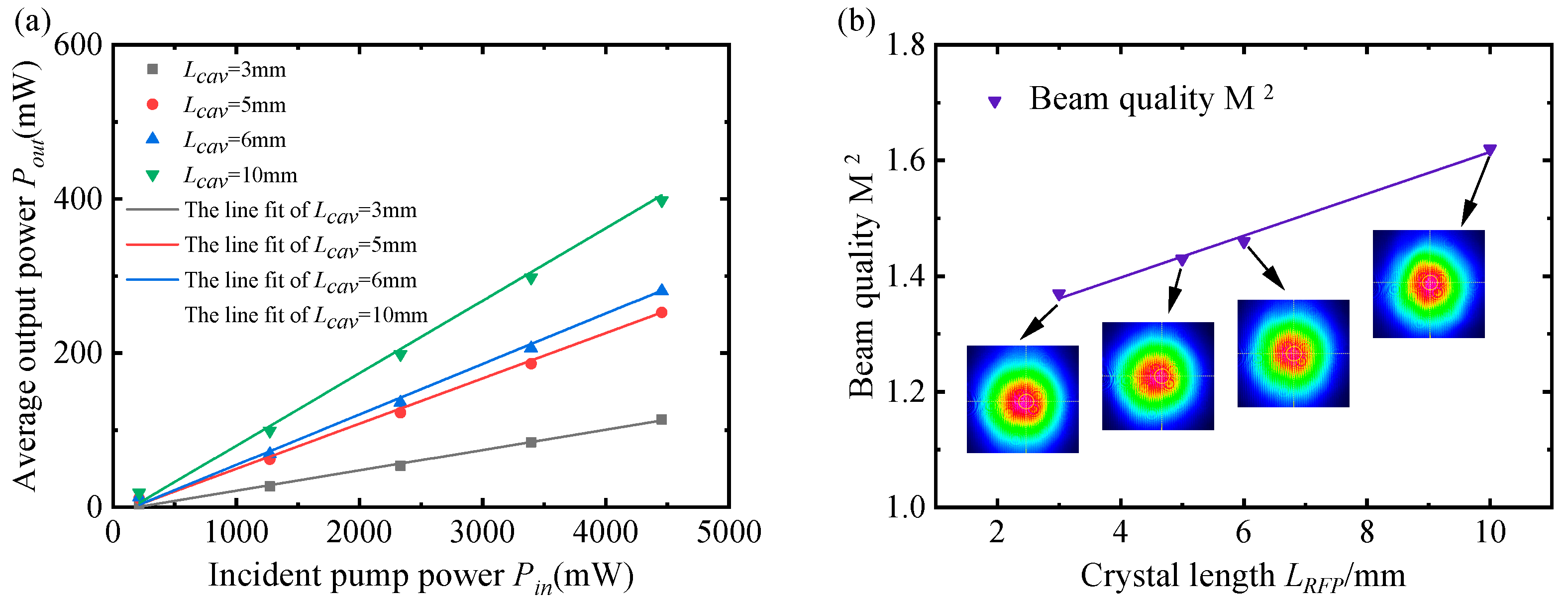
4.2. Study on Beam Quality and Output Power of the Burst Pulses
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Slipchenko, M.N.; Miller, J.D.; Roy, S.; Meyer, T.R.; Mance, J.G.; Gord, J.R. 100 kHz, 100 ms, 400 J burst-mode laser with dual-wavelength diode-pumped amplifiers. Opt. Lett. 2014, 39, 4735–4738. [Google Scholar] [CrossRef] [PubMed]
- Slipchenko, M.N.; Miller, J.D.; Roy, S.; Gord, J.R.; Danczyk, S.A.; Meyer, T.R. Quasi-continuous burst-mode laser for high-speed planar imaging. J. Opt. Lett. 2012, 37, 1346–1348. [Google Scholar] [CrossRef] [PubMed]
- Gattass, R.R.; Cerami, L.R.; Mazur, E. Micromachining of bulk glass with bursts of femtosecond laser pulses at variable repetition rates. J. Opt. Express 2006, 14, 5279–5284. [Google Scholar] [CrossRef] [PubMed]
- Dowling, K.; Dayel, M.J.; Lever, M.J.; French, P.M.W.; Hares, J.D.; Dymoke-Bradshaw, A.K.L. Fluorescence lifetime imaging with picosecond resolution for biomedical applications. J. Opt. Lett. 1998, 23, 810–812. [Google Scholar] [CrossRef] [PubMed]
- Halls, B.R.; Jiang, N.; Meyer, T.R.; Roy, S.; Slipchenko, M.N.; Gord, J.R. 4D spatiotemporal evolution of combustion intermediates in turbulent flames using burst-mode volumetric laser-induced fluorescence. J. Opt. Lett. 2017, 42, 2830–2833. [Google Scholar] [CrossRef] [PubMed]
- Ma, Y.; Li, X.; Yu, X.; Fan, R.; Yan, R.; Peng, J.; Xu, X.; Sun, R.; Chen, D. A novel miniaturized passively Q-switched pulse-burst laser for engine ignition. J. Opt. Express 2014, 22, 24655–24665. [Google Scholar] [CrossRef] [PubMed]
- Ma, Y.; He, Y.; Yu, X.; Li, X.; Li, J.; Yan, R.; Peng, J.; Zhang, X.; Sun, R.; Pan, Y.; et al. Multiple-beam, pulse-burst, passively Q-switched ceramic Nd:YAG laser under micro-lens array pumping. Opt. Express 2015, 23, 24955–24961. [Google Scholar] [CrossRef]
- Pan, H.; Yan, R.; Fa, X.; Yu, X.; Ma, Y.; Fan, R.; Li, X.; Chen, D.; Zhou, Z. High-peak-power, high-repetition-rate LD end-pumped Nd:YVO4 burst mode laser. J. Opt. Rev. 2016, 23, 386–390. [Google Scholar] [CrossRef]
- Li, X.; Xu, H.; Yan, R.; Jiang, Y.; Fan, R.; Dong, Z.; Chen, D. Burst-mode YVO4/Nd:YVO4 laser oscillator with pulse repetition rate up to 500 kHz. J. Optik 2021, 228, 165789. [Google Scholar] [CrossRef]
- He, C.; Yu, H.; Zhang, J.; Zou, S.; Zhao, P.; Lin, X. Flexible picosecond burst generation in a mode-locked Nd:YVO4 laser with a compound cavity. J. Appl. Phys. B 2019, 125, 147. [Google Scholar] [CrossRef]
- Wang, S.-M.; Lai, Y. Up to 400 GHz burst-mode pulse generation from a hybrid harmonic mode-locked Er-doped fibre laser. J. Laser Phys. Lett. 2017, 14, 025102. [Google Scholar] [CrossRef]
- Li, S.C.; Huang, T.L.; Hsieh, Y.H.; Liang, H.C.; Huang, K.F.; Chen, Y.F. Generation and characterization of burst modes in passively mode-locked lasers with internal Fabry–P Erot cavities. J. Opt. Lett. 2020, 45, 61–64. [Google Scholar] [CrossRef]
- Ruan, Q.; Zou, J.; Feng, C.; Chen, T.; Wang, H.; Dong, Z.; Luo, Z. Burst-mode pulse generation in passively mode-locked all-fiber green/orange lasers at 543 nm and 602 nm. J. Photonics Res. 2024, 12, 1231–1238. [Google Scholar] [CrossRef]
- Zhou, B.K. The Principles of Laser; National Defense Industry Press: Beijing, China, 2014; pp. 143–144. [Google Scholar]
- Sung, C.L.; Lee, C.Y.; Cho, H.H.; Huang, Y.J.; Chen, Y.F.; Pan, Z.B.; Yu, H.H.; Zhang, H.J.; Wang, J.Y. Theoretical and experimental studies for high-repetition-rate disordered crystal lasers with harmonic self-mode locking. J. Opt. Express 2016, 24, 3832–3838. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.T.; Huang, C.H. Effects of nonlinear phase in cascaded mode-locked Nd:YVO4 laser. J. Opt. Express 2019, 27, 504–511. [Google Scholar] [CrossRef] [PubMed]
- Lührmann, M.; Theobald, C.; Wallenstein, R.; L’huillier, J.A. Efficient generation of mode-locked pulses in Nd:YVO4 With a pulse duration adjustable between 34 ps and 1 ns. J. Opt. Express 2009, 17, 6177–6186. [Google Scholar] [CrossRef] [PubMed]
- Tuan, P.H.; Chang, C.C.; Lee, C.Y.; Cho, C.Y.; Liang, H.C.; Chen, Y.F. Exploiting concave-convex linear resonators to design end-pumped solid-state lasers with flexible cavity lengths: Application for exploring the self-mode-locked operation. J. Opt. Express 2016, 24, 26024. [Google Scholar] [CrossRef] [PubMed]
- Jin, Y.; Hu, M.; Xu, M.; Yan, H.; Liu, C.; Chen, L.; Li, H.; Bi, M.; Zhou, X. Exploiting the etalon effect to manipulate the pulse characteristics of a self-mode-locked Nd:YVO4 laser with a flexible cavity length. J. Opt. Commun. 2022, 517, 128331. [Google Scholar] [CrossRef]
- Jin, Y.; Xv, M.; Hu, M.; Zhang, Y.; Zhang, P.; Zhang, Z.; Zhang, H.; Liang, Q. High-repetition pulsed Nd:YVO4 laser based on the multi-longitudinal-mode beat note. J. Opt. Eng. 2021, 60, 066106. [Google Scholar] [CrossRef]
- Chen, Y.F.; Chang, M.T.; Huang, T.L.; Tung, J.C.; Huang, K.F.; Liang, H.C. Orthogonally Polarized Self-Mode-Locked Lasers With Repetition Rate Multiplication up to Hundreds of Gigahertz: Observation Of Temporal Carpet. J. IEEE J. Sel. Top. Quant. 2018, 24, 1101806. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, M.; Hu, M.; Li, Z.; Wang, J.; Fu, J.; Wang, S.; Ji, Y.; Li, H.; Bi, M.; Zhou, X.; et al. Time–Frequency and Spectrum Analyses of All-Solid-State Self-Mode-Locked Burst Pulse Lasers. Photonics 2024, 11, 558. https://doi.org/10.3390/photonics11060558
Xu M, Hu M, Li Z, Wang J, Fu J, Wang S, Ji Y, Li H, Bi M, Zhou X, et al. Time–Frequency and Spectrum Analyses of All-Solid-State Self-Mode-Locked Burst Pulse Lasers. Photonics. 2024; 11(6):558. https://doi.org/10.3390/photonics11060558
Chicago/Turabian StyleXu, Mengmeng, Miao Hu, Zerong Li, Jinxiu Wang, Jiaxin Fu, Shaokun Wang, Yingying Ji, Haozhen Li, Meihua Bi, Xuefang Zhou, and et al. 2024. "Time–Frequency and Spectrum Analyses of All-Solid-State Self-Mode-Locked Burst Pulse Lasers" Photonics 11, no. 6: 558. https://doi.org/10.3390/photonics11060558
APA StyleXu, M., Hu, M., Li, Z., Wang, J., Fu, J., Wang, S., Ji, Y., Li, H., Bi, M., Zhou, X., Pan, S., & Liu, C. (2024). Time–Frequency and Spectrum Analyses of All-Solid-State Self-Mode-Locked Burst Pulse Lasers. Photonics, 11(6), 558. https://doi.org/10.3390/photonics11060558



