Harnessing Multistability: A Novel Approach to Optical Logic Gate Construction Using Erbium-Doped Fiber Lasers

Abstract

We present an innovative method harnessing multistability within a diode-pumped erbium-doped fiber laser to construct logic gates. Our approach involves manipulating the intensity of external noise to regulate the probability of transitioning among four concurrent attractors. In this manner, we facilitate the realization of OR, AND, NOR, and NAND logic operations, aligning with the coexisting period-1, period-3, period-4, and period-5 orbits. Employing detrended fluctuation analysis, we establish equilibrium in the probability distributions of these states. The obtained results denote a substantial advancement in the field of optical logic gate development, representing a pivotal stride toward the seamless integration of an all-optical logic gate within laser oscillator-based systems.

1. Introduction

Systems exhibiting multiple coexisting attractors have been identified across various scientific disciplines and in natural phenomena [1,2]. Among many dynamical systems displaying coexisting attractors, the laser marked a pivotal milestone as the first system where multistability was experimentally observed [3]. Special attention is directed towards fiber lasers due to their pivotal roles in optical communications, reflectometry, sensing, and medicine [4,5]. In particular, erbium-doped fiber lasers (EDFLs) stand out as an optical device that has captured the significant interest of numerous researchers due to their versatility. These lasers are distinguished by their compact dimensions, high damage threshold, excellent heat dissipation, and superior efficiency. Beyond their practical applications, EDFLs hold significance in the realm of nonlinear systems. Their dynamics exhibit susceptibility to external perturbations, leading to the emergence of chaos and the coexistence of multiple attractors [2,6,7].

Multistability is an intriguing phenomenon not only from a scientific standpoint but also due to its important applications, including advancements in cognitive neuroscience [8,9,10], generation of giant pulses [11], secure communications [12,13], and digital computing [14,15,16]. Highlighting the last-mentioned advancements, it is noteworthy that experimental implementations of logic gates, rooted in a triple-well potential, have been successfully implemented in electronic systems [14] and cavity magnonic systems [15]. The intrinsic versatility of a triple-well potential system is demonstrated further, as it enables the attainment of all six logic operations through the combination of various states [16].

Considering the aforementioned advantages of EDFLs, coupled with their comparatively low implementation cost and straightforward experimental realization for future endeavors, the EDFL emerges as an exceptionally advantageous system for proposing and implementing optical logic gates [17,18]. In contrast to earlier logic gate implementations that relied on perturbing the laser with a digital signal, our current research delves into the influence of external noise. This enhancement streamlines the process, paving the way for the realization of four distinct logic operations. The convergence of diverse scientific domains, encompassing both attractor dynamics and logic operations, underscores the broad-reaching implications and interdisciplinary significance of these multifaceted systems.

The formulation of digital functions for characterizing logic gates within dynamical systems typically involves the integration of hybrid systems, where signals generated in a computer are translated into analog signals. The concept of “chaos computing” has gained considerable traction, leveraging the dynamic properties of chaotic systems. This approach predominantly relies on employing an analog chaotic oscillator that emulates the behavior of multiple logic gates. This unique feature enables seamless transitions between different modes of operation, facilitating the resolution of specific arithmetic operations [7]. The seminal work of Sinha et al. [19], introducing an SR (set–reset) flip-flop based on NOR gates implemented by a Chua circuit, marked the inception of extensive research and development in chaotic logic gates [18,20,21,22,23]. Noteworthy among these efforts are shared challenges, namely, (i) the utilization of systems with coexisting attractors, and (ii) the incorporation of the sum of two digital decorrelated and periodless signals as input to the dynamical system for generating the truth table associated with the logic gate. These commonalities form the foundational basis for exploring the potential and limitations of chaos-driven computing in logic gate applications.

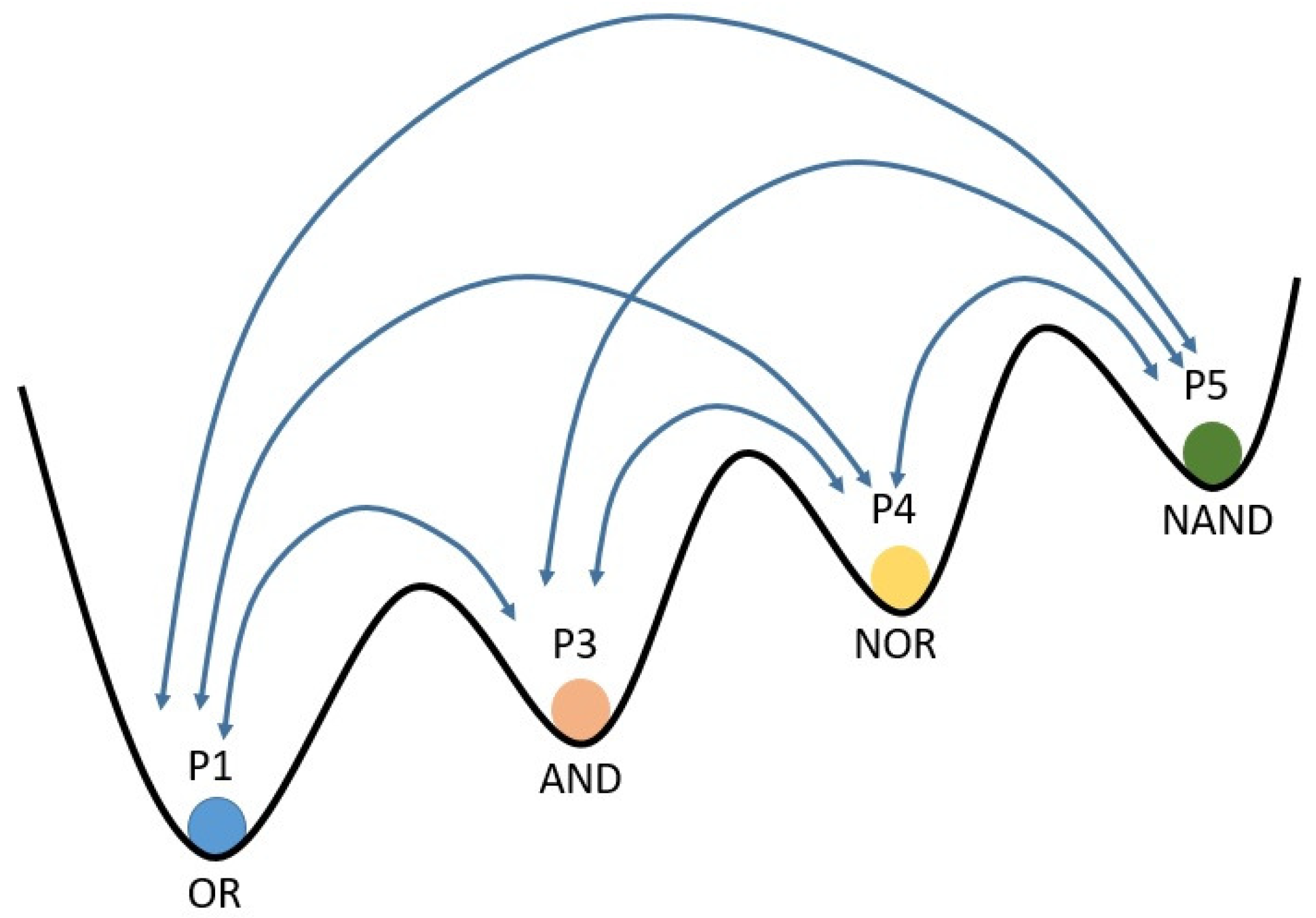
In this study, we introduce a novel approach to implementing logic gates using noise-induced multistate intermittency (or attractor hopping) in a laser system. Our work represents the first exploration of logic gate functionality controlled by external noise in such a system. Specifically, through comprehensive numerical simulations, we demonstrate the feasibility of realizing logic gates in a noisy EDFL coupled with a low-pass filter (LPF). In our system, each logic operation is uniquely associated with a specific periodic orbit. Notably, in the laser system featuring four coexisting periodic orbits, we assign the period 1 (P1) to OR operation, period 3 (P3) to AND, period 4 (P4) to NOR, and period 5 (P5) to NAND. The conceptual representation of the potential well for such a system is illustrated in Figure 1.

The introduction of noise in this system destabilizes coexisting attractors, making the system metastable and inducing transitions between them. This offers a dynamic and adaptable foundation for logic gate operations. In the presence of noise, a laser system featuring coexisting attractors undergoes multistate intermittency, wherein the laser switches between various coexisting states [24,25].

The subsequent sections of this paper are structured as follows. Section 2 provides an overview of the laser system employed, outlining its diverse behaviors and elucidating the method employed to induce attractor hopping within the system. In Section 3, we present the results achieved by equalizing the probability of occurrence for each coexisting period in the laser. This section also delves into the process of constructing a digital signal by activating logic operations when the EDFL exhibits equiprobable multistate intermittency. In Section 4, we explore a potential experimental implementation of the proposed method and elucidate it through a schematic representation of the corresponding laser device. The final section, Section 5, encapsulates the conclusions drawn from this work and offers a platform for discussions on its implications and potential future directions.

2. Numerical Model of Erbium-Doped Fiber Laser

The dynamics of an EDFL is described by two differential equations [26]

$$\begin{array}{c}{\displaystyle \frac{dP}{dt}}={\displaystyle \frac{2L}{T_r}}P\{r_w{\alpha }_0[N({\zeta }_1-{\zeta }_2)-1]-{\alpha }_{th}\}+P_{sp},\hfill \\ {\displaystyle \frac{dN}{dt}}={\displaystyle \frac{{\sigma }_1{r}_{w}P}{\pi r_0^2}}(N{\zeta }_1-1)-{\displaystyle \frac{N}{\tau }}+P_{pump},\hfill \end{array}$$

(1)

where P is the power of the laser in the cavity, $N=\frac{1}{n_{0}L}{\int }_0^L{N}^2\left(z\right)dz$ is the mean value of the upper level of the laser potential, where $n_0$ is the refractive index of the cold core of the erbium-doped fiber, ${\zeta }_1$ and ${\zeta }_2$ indicate the relationship between the effective cross sections and the fundamental absorption state, and $N_2$ is the upper level in the z coordinate (propagation direction of the laser emission). The element $T_r$ is the total time required for a photon to travel through the cavity, ${\alpha }_0$ is the absorption coefficient of the erbium-doped fiber, ${\alpha }_{th}$ is the threshold loss counter of the laser cavity, $\tau$ is the lifetime of an excited erbium ion, and $r_w$ is the quantity that determines how closely the fundamental mode of the laser and the volume of the erbium-doped core coincide. The spontaneous emission in the laser basic regime is described by

$$P_{sp}=N\frac{{10}^{-3}}{T_r\tau }{\left(\frac{{\lambda }_g}{{\omega }_0}\right)}^2\frac{r_0^2{\alpha }_{0}L}{4{\pi }^2{\sigma }_{1}2},$$

(2)

where ${\lambda }_g$ is the wavelength of the laser emission at 1.5 μm. The pump power

$$P_{pump}=P_p\frac{1-exp[-{\alpha }_0\beta L(1-N)]}{N_0\pi r_0^{2}L}$$

(3)

is periodically modulated as follows:

$$P_p=p\left[1-msin\left(2\pi f_{m}t\right)\right],$$

(4)

where m and $f_m$ are the modulation depth and frequency, respectively, $P_p$ is the dimensionless pumping power at the fiber inlet, and p is the constant power without modulation.

The system in Equation (1) is normalized to obtain the following dimensionless form [26]:

$$\begin{array}{c}\dot{x}=axy-bx+c(y+r_w),\hfill \\ \dot{y}=dxy-(y+r_w)+e\left\{1-exp\left[-\beta {\alpha }_{0}L\left(1-y+{\displaystyle \frac{r_w}{{\zeta }_2{r}_w}}\right)\right]\right\},\hfill \end{array}$$

(5)

where a, b, c, and d are coefficients given in

Table 1. Parameter values of the normalized system in Equation (5) for numerical simulations of the EDFL.

Parameter	Value	Parameter	Value
a	$6.620\times {10}^7$	b	$7.415\times {10}^6$
c	0.016	d	$4.076\times {10}^3$
e	506	$r_w$	0.307
${\zeta }_2{r}_w$	0.615	$-\beta {\alpha }_{0}L$	−18

.

These equations can be solved numerically by modulating the pump current of the laser diode with a periodic signal and analyzing the response of the laser to changes in modulation frequency and amplitude. Figure 2 shows the bifurcation diagram of the local maxima of the laser intensity obtained by varying modulation frequency $f_m$ for fixed modulation depth $m=1$. The frequency was changed in steps of 1 kHz, and a random initial condition was used for each change. The region of multistability explored in this paper are marked in red. One can see that at $f_m=80$ kHz, the laser exhibits the coexistence of four stable periodic orbits (P1, P3, P4, P5).

Remark 1. 

In this paper, we use the term “period” to denote the duration of a periodic orbit within the laser system. This refers to the repetitive oscillations induced by the harmonic modulation of the pump power. The numerical representation of the period aligns with the count of periods of the pump function in the EDFL emission.

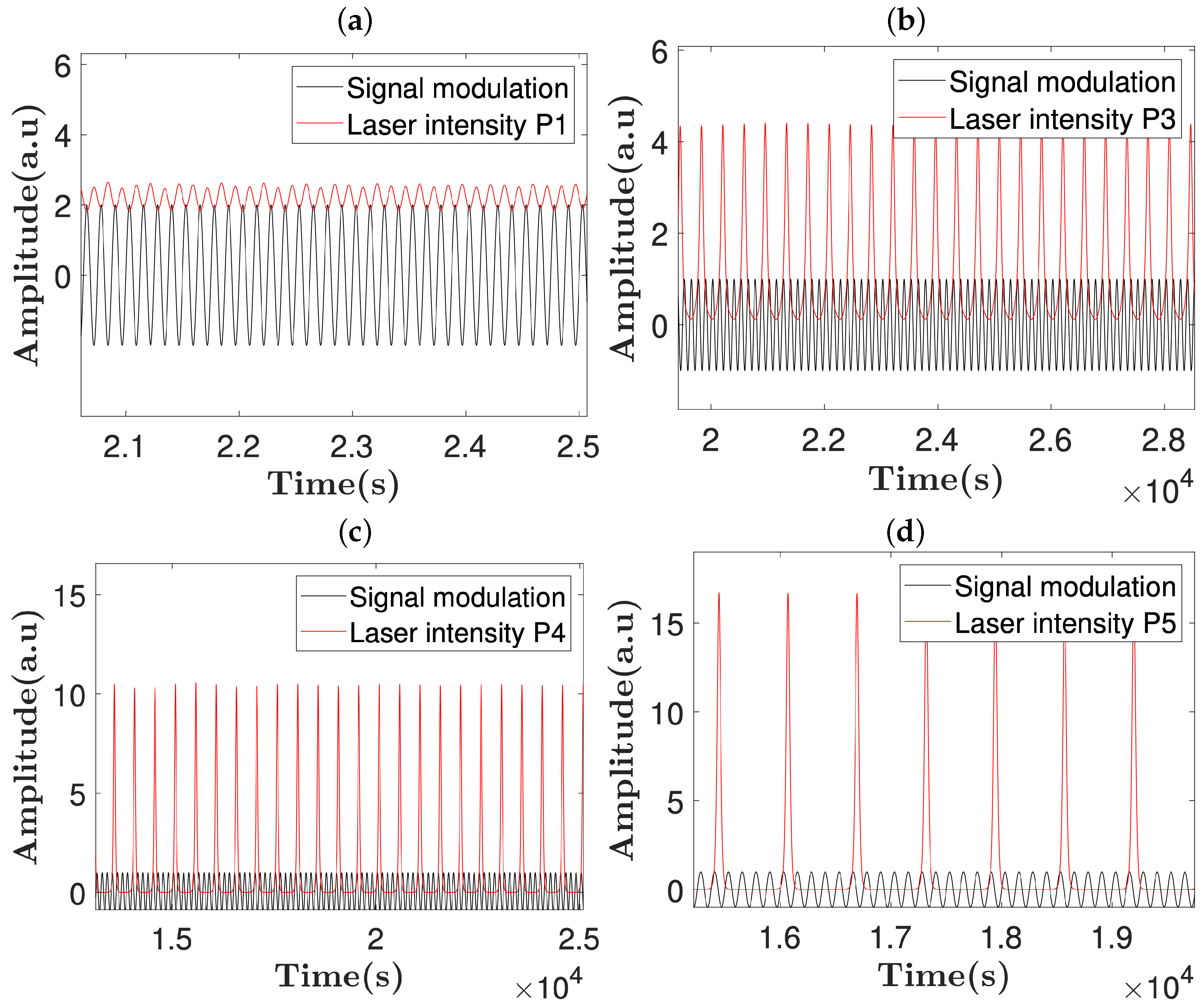
• If the laser oscillates in period 1, the modulation frequency is $f_m$.
• If the laser oscillates in period 3, the modulation frequency is $f_m/3$.
• If the laser oscillates in period 4, the modulation frequency is $f_m/4$.
• If the laser oscillates in period 5, the modulation frequency is $f_m/5$.

This relationship between the pumping frequency and the periodic response of the EDFL is illustrated with the time series in Figure 3.

2.1. System under Noise Addition

When the system described by Equation (5) is subjected to periodic modulation, the laser manifests remarkably intricate dynamics, as illustrated in Figure 2 and Figure 3. However, adding a stochastic component to Equation (4), we obtain

$$P_p=p[1-msin\left(2\pi f_{m}t\right)+\eta G(\xi ,f_n)],$$

(6)

where $\eta$ is the noise amplitude and $G(\eta ,f_n)$ is the noise function with zero mean between a random number $\eta \in [-1,1]$, and the frequency $f_n$ is the low-pass filtered (LPF) noise (white noise is filtered with a discrete fifth-order low-pass Butterworth filter). In the multistability region, noise triggers multistate intermittency, also known as attractor hopping, wherein the laser alternates among the coexisting states.

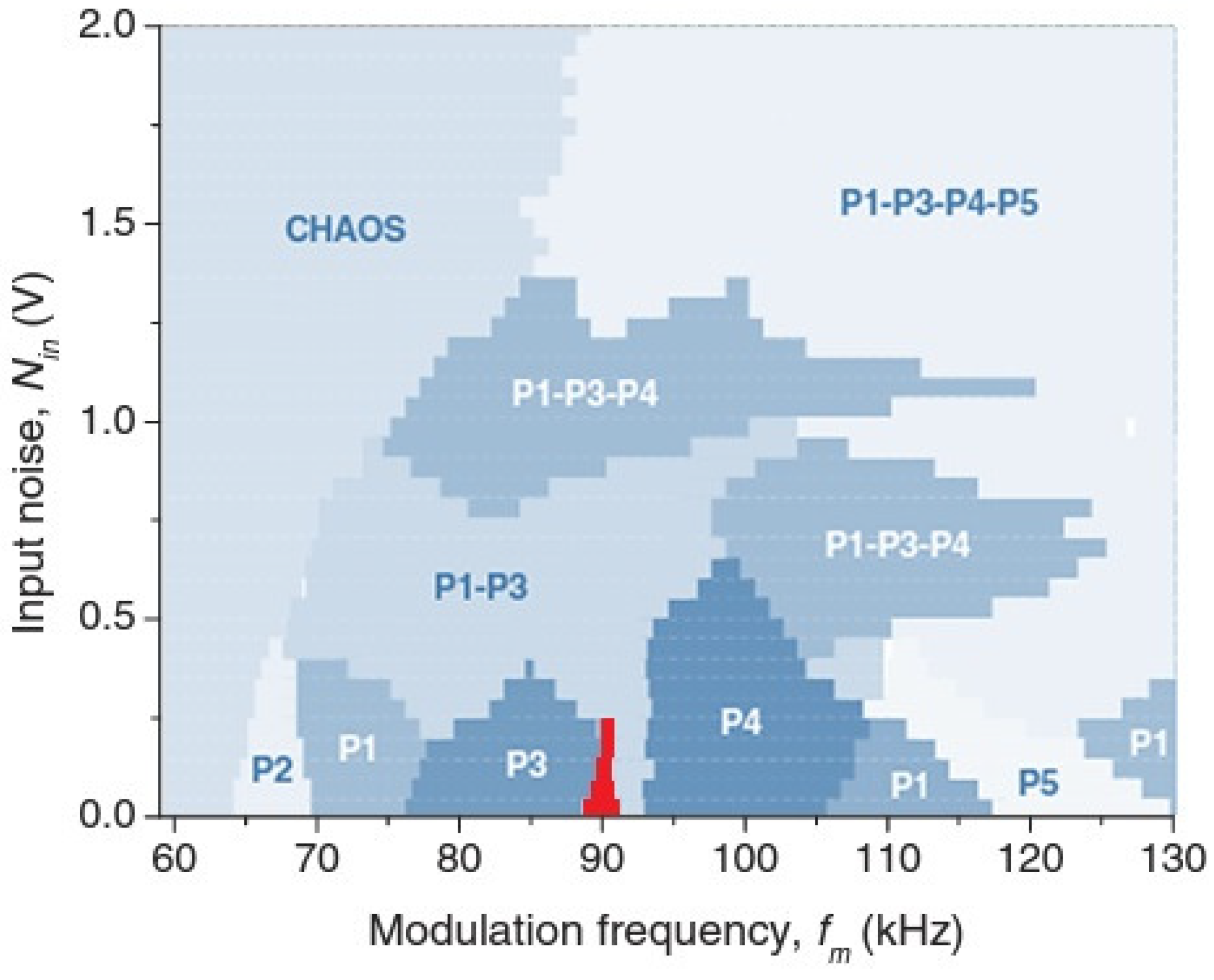
The diagram in Figure 4 displays the regions of various intermittency regimes experimentally observed in the EDFL in the parameter space of the modulation frequency $f_m$ and input noise intensity $N_{in}$ [27]. One can note the appearance of new intermittency attractors as the input noise intensity is increased. These attractors represent the mixture of P1 and P3 states; P1, P3, P4; and P1, P3, P4, P5; each state has different frequency, as indicated in Remark 1.

Remark 2. 

While the findings presented in Figure 2 and Figure 4 are derived from our earlier work [27], it is important to clarify that these results do not constitute the primary focus of this paper. Rather, they serve as foundational insights crucial for comprehending the contributions of the current study. As such, these results are retained within Remark 1 to provide essential context and background for the subsequent developments in this work.

2.2. Problem Statement

As discussed in the preceding sections, the development of logic gates within dynamical systems has garnered increasing attention due to its potential advantages in information processing speed, surpassing current achievements. This approach also enables the integration of various logic gates within a single oscillator. To accomplish this, existing systems rely on decorrelated digital signals as triggers to characterize system responses and construct associated truth tables for obtained logic gates. Typically, these digital signals are generated computationally, translated into analog signals, and then injected into chaotic dynamical systems, a paradigm known as chaos computing.

In this paper, we take a distinctive approach by generating random signals derived from an optical system, such as the EDFL, operating in the regime of multistate intermittency. By stabilizing the probability of occurrence for each coexisting state within this intermittency region, the laser signal exhibits amplitude variations characteristic of each period of oscillations. This variation is leveraged to activate and randomly deactivate logic operations, facilitating the creation of digital signals implementable in optical-system-based logic gates.

3. Results

Upon the addition of a filtered noisy signal, we observe multistate intermittency previously described [2]. This phenomenon unfolds as the external noise $\eta G(\xi ,f_n)$ is gradually increased. Figure 5 represent the time series of P1 (Figure 5a) and intermittent attractors (Figure 5b–d) illustrating switches between various coexisting states, derived from the LPF featuring a 60 Hz cut frequency.

While without noise ($\eta =0$) the laser stays in one of the coexisting attractors depending on the initial conditions, say P1 (Figure 5a), increasing noise intensity induces first into two-state intermittency (switches between P1 and P3), then tri-state intermittency (switches between P1, P3, and P4), and finally multistate intermittency (switches between P1, P3, P4, and P5 [27]. To facilitate the identification of distinct periodic states in the intermittent dynamics shown in Figure 5,

Table 2. Noise intensities for regimes illustrated in Figure 5.

State	Noise Intensity (a.u.)
$P_1$	1.5
$P_3$	4
$P_4$	8
$P_5$	>8

provides information about noise intensity for each intermittent state.

An analysis of multistate intermittency induced in the EDFL system under both periodic and stochastic modulation reveals an uneven distribution in the probability of attractor occurrences across varying noise levels. To address this discrepancy and align with the primary objective of the study—the random activation of logic operations through the laser system’s hopping between coexisting states—an investigation into the system’s response is undertaken for different noise intensities. This exploration aims to identify a parameter range wherein the probability of occurrence for coexisting states is comparable. By achieving a balanced distribution, any preferential bias in logic operation activation is eliminated, facilitating the generation of decorrelated digital signals by the optical system.

Remark 3. 

The assignment of logic operations to each of the coexisting states of the laser is as follows:

• If the system has oscillations of P1 type, the OR logic operation is performed.
• If the system has oscillations of P3 type, the AND logic operation is performed.
• If the system has oscillations of P4 type, the NOR logic operation is performed.
• If the system has oscillations of P5 type, the NAND logic operation is performed.

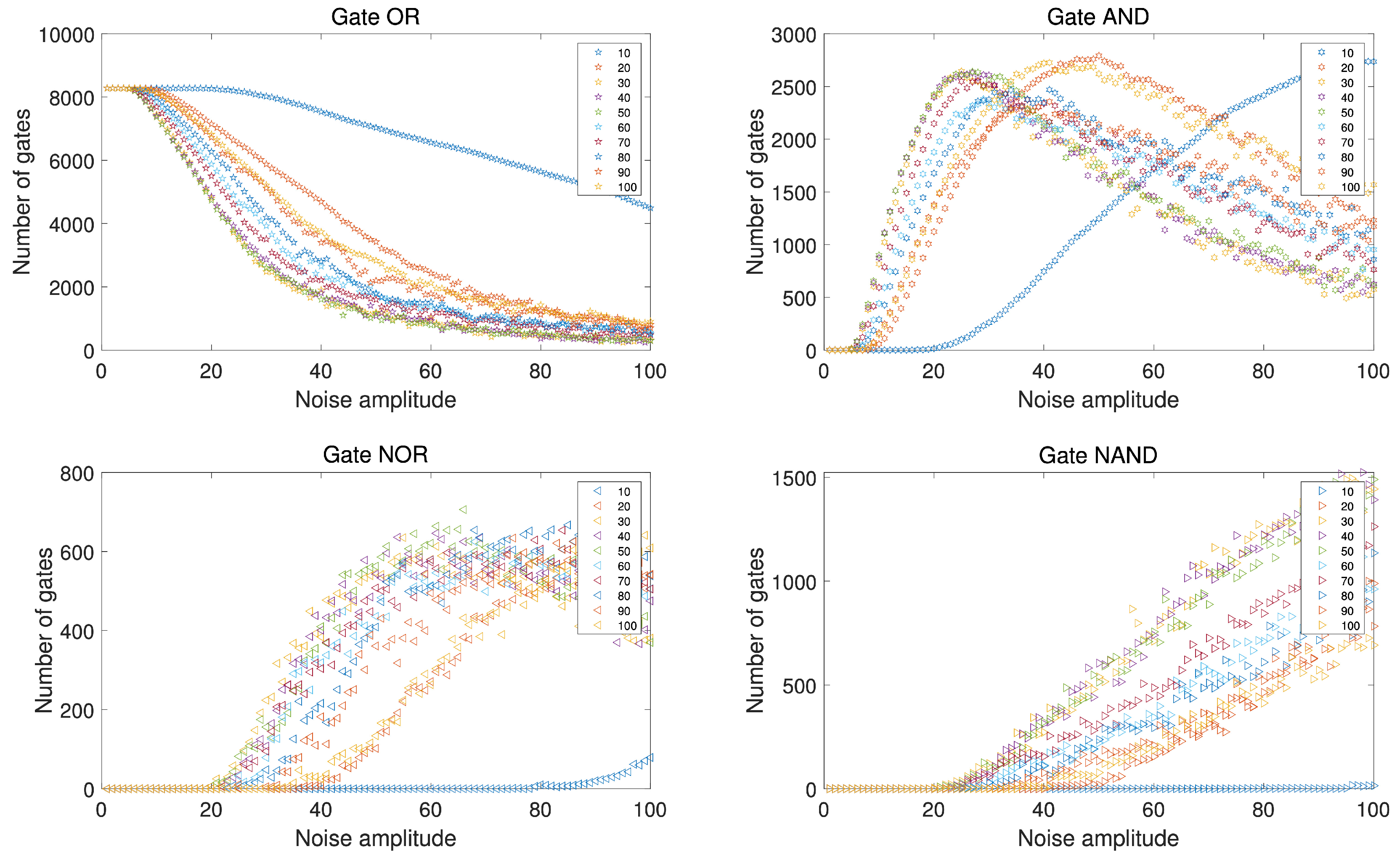
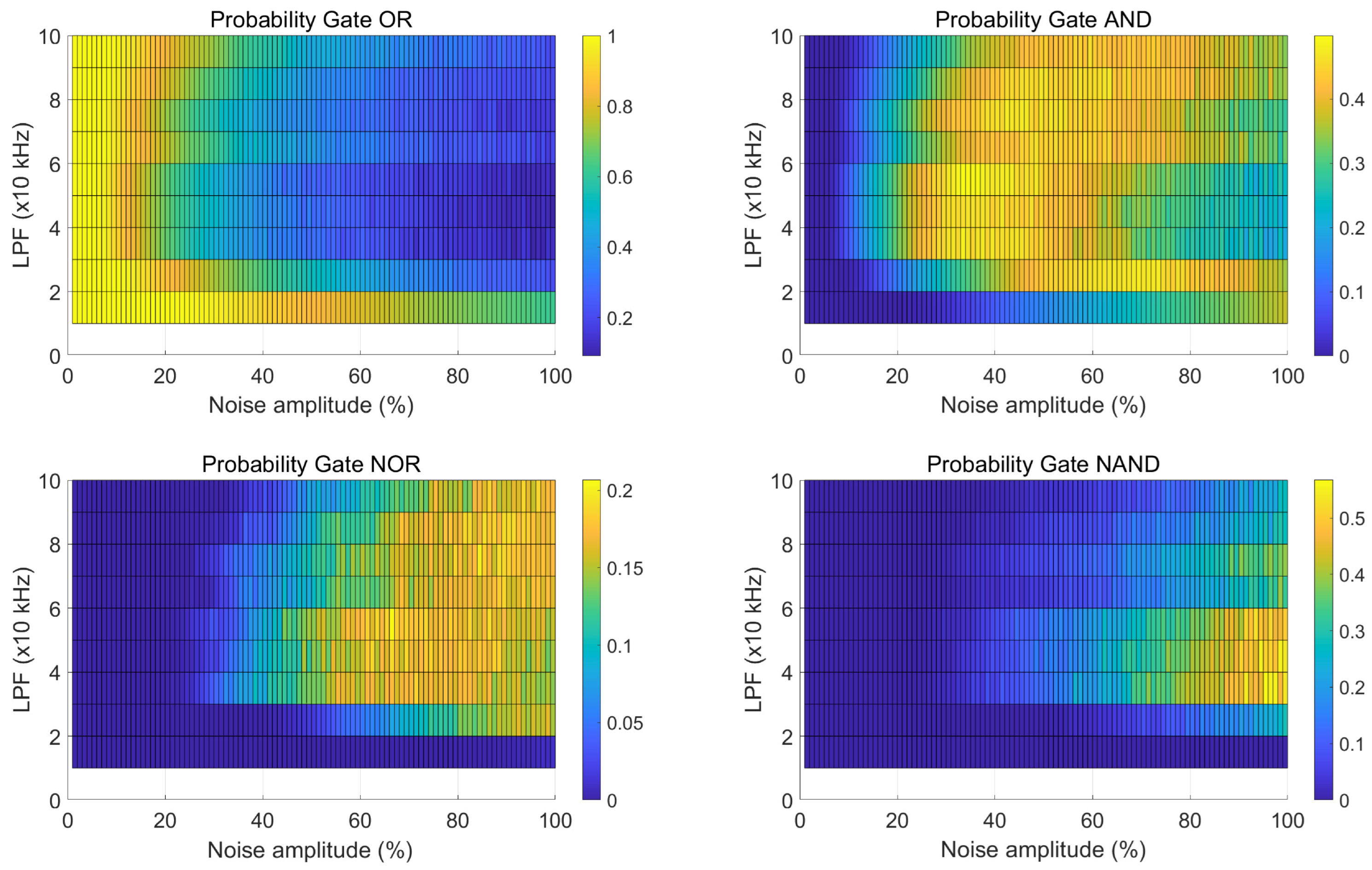
Figure 6 illustrates the assessment of each coexistent state in the EDFL for varying the noise intensity on the abscissa and LPF value on the ordinate. As outlined in Remark 3, the graphs in Figure 6 hold different logic gates. Notably, when the applied filter value $f_n$ is below 20 kHz, the observed behavior exhibits minimal variation compared to the system without LPF, consistent with previous observations [2].

Figure 7 presents the calculated probability of occurrence for each defined gate (period) based on each analyzed time series, with the color bar indicating the likelihood of a specific gate occurring in the time series for a given ($\eta ,f_n$) combination.

Upon identifying the optimal range for “stabilizing” the probabilities of occurrence for different periods in the system, each period is then assigned a logic operation. This assignment triggers the activation of the corresponding operation, leading to the generation of a digital signal, intricately associated with the EDFL dynamics. The operations allocated to each period, along with their respective truth tables, are detailed in

Table 3. Logic operations associated with each of the coexisting periods in multistate intermittency.

Inputs	OR	AND	NOR	NAND
0,0	0	0	1	1
1,0	1	0	0	1
0,1	1	0	0	1
1,1	1	1	0	0

.

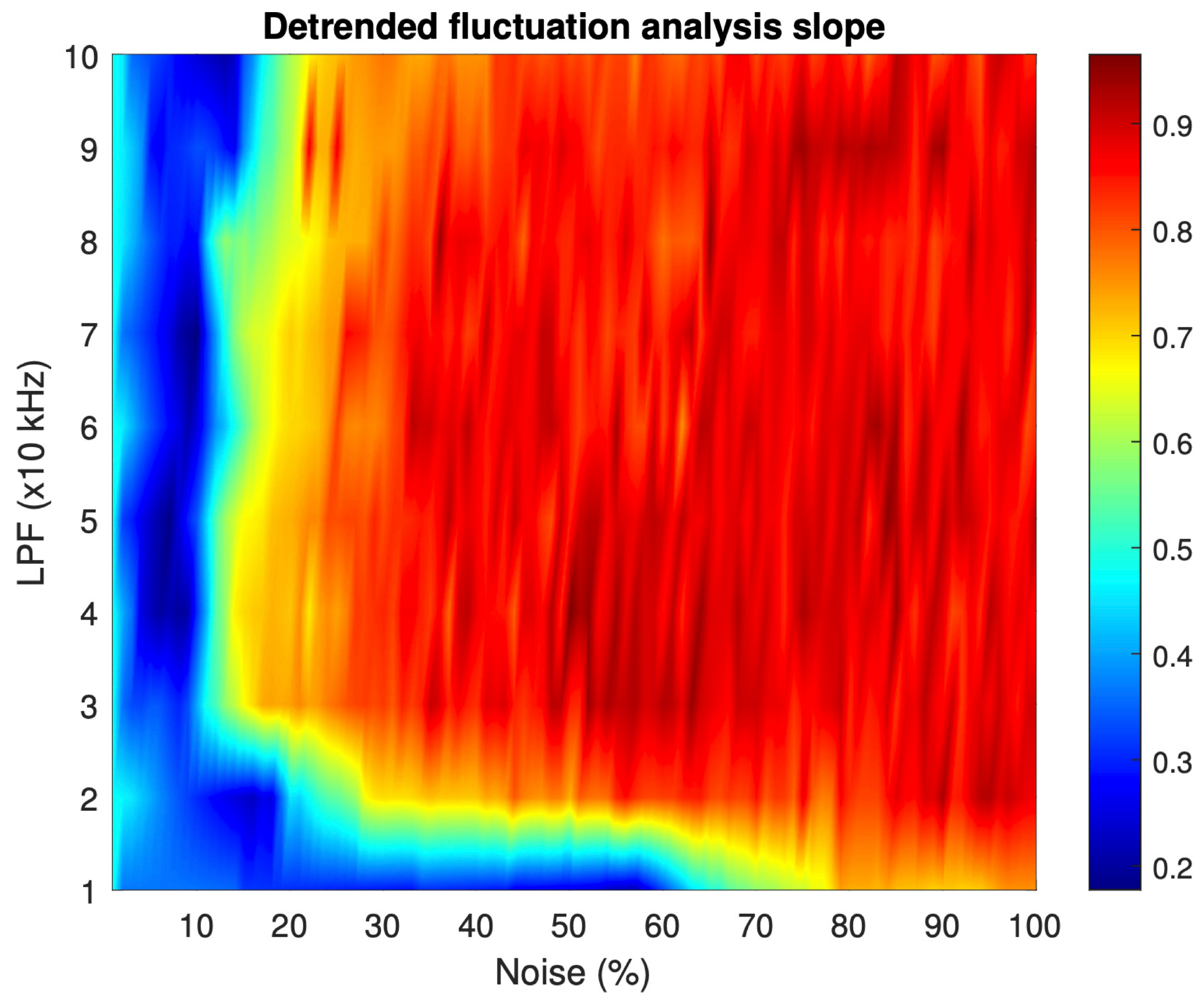
The depicted behavior in Figure 7 reveals that, for specific combinations of noise intensity and particular LPF frequency, achieving a comparable probability of activating different logic gates is attainable. In light of this, we incorporate detrended fluctuation analysis (DFA). Initially introduced by Peng et al. [28], DFA elucidates long-range power-law correlations, offering insights into the statistical self-affinity of specific behaviors. This method proves valuable for analyzing time series indicative of long-memory processes, characterized by correlations in dynamical nonlinear actions (e.g., power-law decaying autocorrelation functions). Importantly, DFA helps circumvent the inadvertent detection of spurious long-range correlations stemming from nonstationarity.

The DFA analysis employs fluctuation function $F(\nu ;s)$, adhering to the power-law scaling relation

$$F(\nu ;s)\sim s^{{\delta }_{\nu }},$$

(7)

where the time series is segmented into s pieces, each with length $\nu$. The scaling exponent or slope ${\delta }_{\nu }$ delineates distinct regimes, offering valuable insights into the laser dynamics, as follows.

• ${\delta }_{\nu }<1/2$: anti-correlated behavior;
• ${\delta }_{\nu }\simeq 1/2$: uncorrelated behavior or white noise;
• ${\delta }_{\nu }>1/2$: correlated behavior;
• ${\delta }_{\nu }\simeq 1$: $1/f$-noise or pink noise;
• ${\delta }_{\nu }>1$: non-stationary or unbounded behavior;
• ${\delta }_{\nu }\simeq 3/2$: Brownian motion.

Figure 8 presents the DFA results obtained for all analyzed time series across various noise intensity variations and applied LPF frequencies. The findings affirm that the diverse combinations of amplitude noise and LPF application reflect a correlated behavior among the different interacting states within the system. Additionally, certain regions indicate proximity to pink noise behavior, suggesting nuanced dynamics in specific conditions.

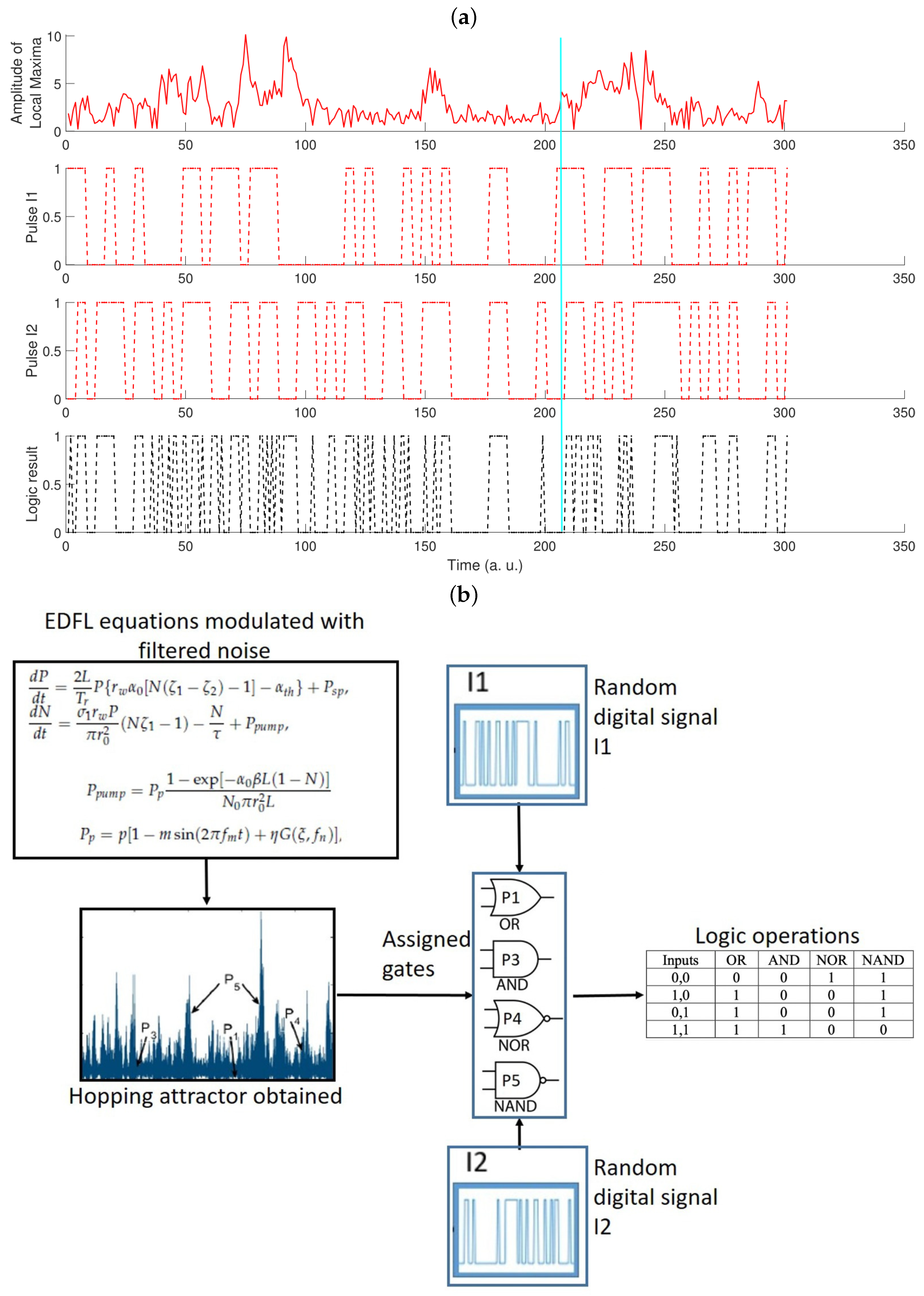
As an illustrative example of diverse applications, the upper trace in Figure 9a displays 300 red points that define a local maximum vector (LMV) derived from the EDFL time series with LPF at $f_n=60$ kHz and noise intensity $\eta =80$. The amplitude of each local maximum is employed to activate the corresponding logic gate, as outlined in Remark 4.

Remark 4. 

The logic gate is determined from the waveform of the local maxima according to the following rule:

• If the local maxima have intensity $I\le 1.5$, an OR gate is activated.
• If the local maxima have intensity $1.5<I\le 4$, an AND gate is activated.
• If the local maxima have intensity $4<I\le 8$, an NOR gate is activated.
• If the local maxima have intensity $I>8$, an NAND gate is activated.

Two red-square time series (Pulse I1 and Pulse I2) in the middle traces in Figure 9a depict randomly generated external digital signals, while the black-square time series (lower trace) represents the response to a logic operation (LO) between I1 and I2, defined by the LMV. To elaborate further, consider the time moment near 200 marked by a vertical blue line in Figure 9a. At this moment, the value of LMV corresponds to the AND gate, indicating the operation for I1 $=1$ and I2 $=0$. As expected, the result is 0, as demonstrated by the black time series of the LO in the lower trace. This example showcases the practical application of the proposed approach in executing logic operations based on the laser dynamics. Figure 9b shows a schematic representation of such a structure. It is worth mentioning that the presented result builds on earlier results of the research group that dealt with generating optical logic gates [18].

4. Possible Experimental Realization

The proposed method can be implemented through the experimental setup illustrated in Figure 10. A conceivable configuration incorporates key elements such as a function generator (FG) and a function noisy generator (FNG) facilitating modulation in a laser driver (LD). In the diagram, the erbium-doped fiber laser (EDFL) is delineated by black lines. An optical isolator (OI) is employed to prevent the ingress of reflected light, preserving the laser’s dynamical behavior. Subsequent to traversing the OI, the laser light impinges on a photo detector (PD), converting it into an electrical signal received by a data acquisition card (DAC).

As previously mentioned, the EDFL exhibits very rich dynamics contingent on the modulation frequency, as illustrated with the bifurcation diagram in Figure 2. For possible experimental realization, we can set the modulation frequency at 80 kHz, where four attractors (P1, P3, P4, and P5) coexist, while the introduction of external noise will induce a multistate intermittency regime, prompting the laser to transition among these coexisting states. Reiterating an earlier point, the amplitude of each attractor in the hopping attractor determines the optical gate selection. As digital squared signals I1 and I2 enter the DAC and subsequently reach the computer (COM), digital operations specified in Table 3 can be executed.

In addition, the proposed method holds significant potential for generating pseudorandom numbers, a capability applicable across various fields such as economics, engineering, meteorology, and biology. Within cryptography, pseudorandom number generators (PRNGs) find utility in creating cryptographic keys, initialization vectors (IVs), and other essential parameters for cryptographic algorithms. In the development of video games and entertainment applications, PRNGs play a crucial role in generating random scenarios, enemies, game elements, and other dynamic aspects. Additionally, within the realm of statistics, PRNGs are instrumental in executing random sampling and simulating probability distributions. Moreover, in the domain of modeling and optimization, PRNGs serve to model and optimize intricate systems. This application extends to the design of communication networks and the optimization of routes and schedules, showcasing the versatility and broad-reaching impact of the proposed method.

5. Conclusions

In this study, we numerically explored the potential of harnessing noise-induced multistate intermittency in a multistable EDFL to realize logic gates. The manipulation of noise intensity allowed for the attainment of specific logic operations. Additionally, employing a low-pass filter for each noise variation enabled an examination of how filters can influence the probability of a particular state’s occurrence. This activation of operations facilitated the generation of uncorrelated binary signals with variable periods, offering possibilities for implementation in logic gate generation schemes based on optical media.

Through the application of detrended fluctuation analysis, we revealed the correlated behavior resulting from various noise intensities combined with specific low-pass filters. This finding underscores the potential to achieve a more balanced equilibrium between different states exhibiting hopping intermittency in temporal series. Such equilibrium is valuable for applications, including encryption systems, where the generation of a pseudorandom number embedded in an optical system depends on a low-pass filter, offering enhanced security and unpredictability.

We also introduced a potential configuration for a laser device intended for the experimental implementation of optical logic gates, which we aim to carry out in the near future. An anticipated challenge in the experimental realization is the elevated level of internal noise. Nevertheless, we are optimistic about our ability to control it, drawing from our experience as demonstrated in [27].