Enhanced THz Circular-Polarization Detection in Miniaturized Chips with Chiral Antennas
Abstract
1. Introduction
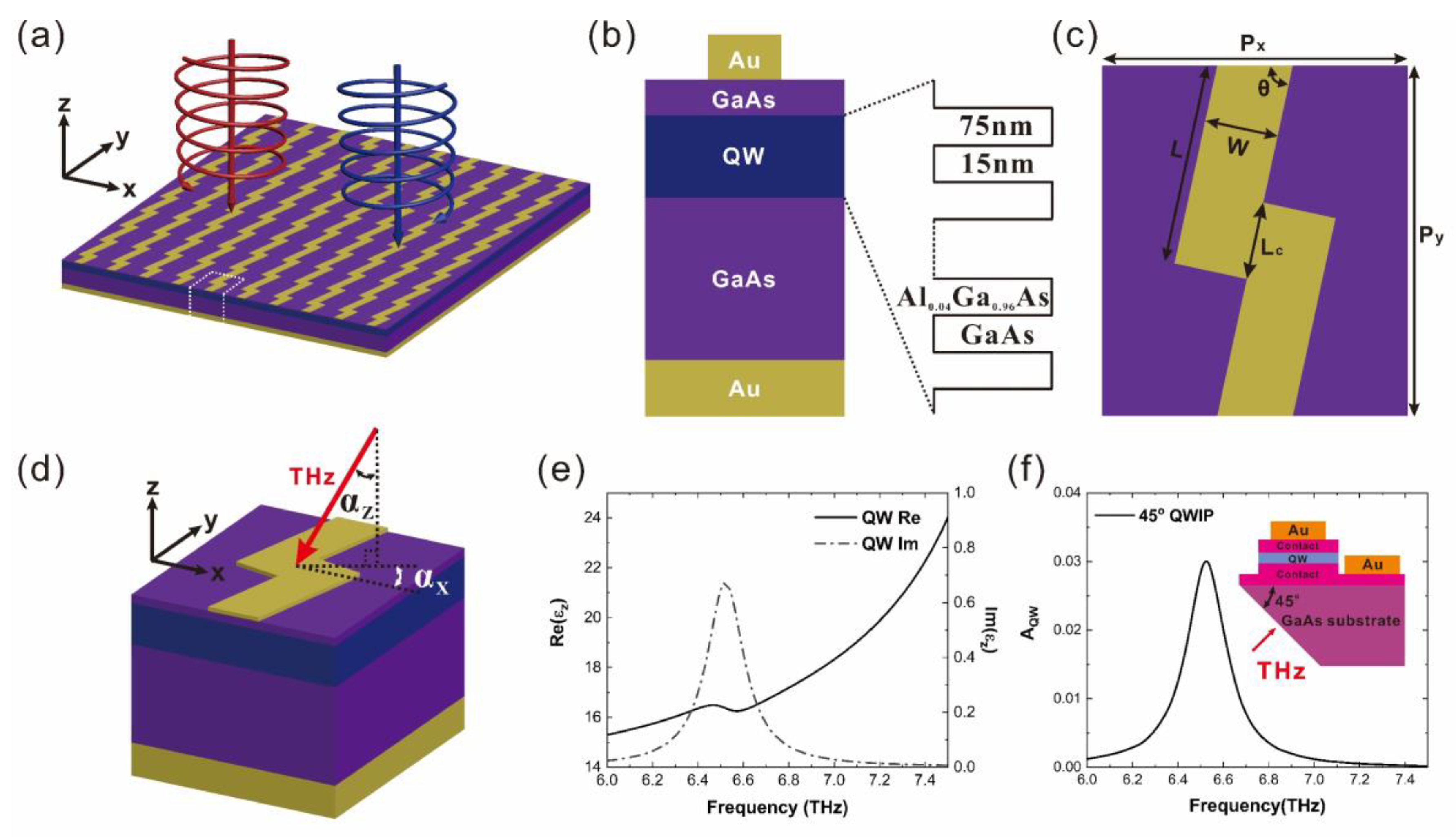
2. Device Structure
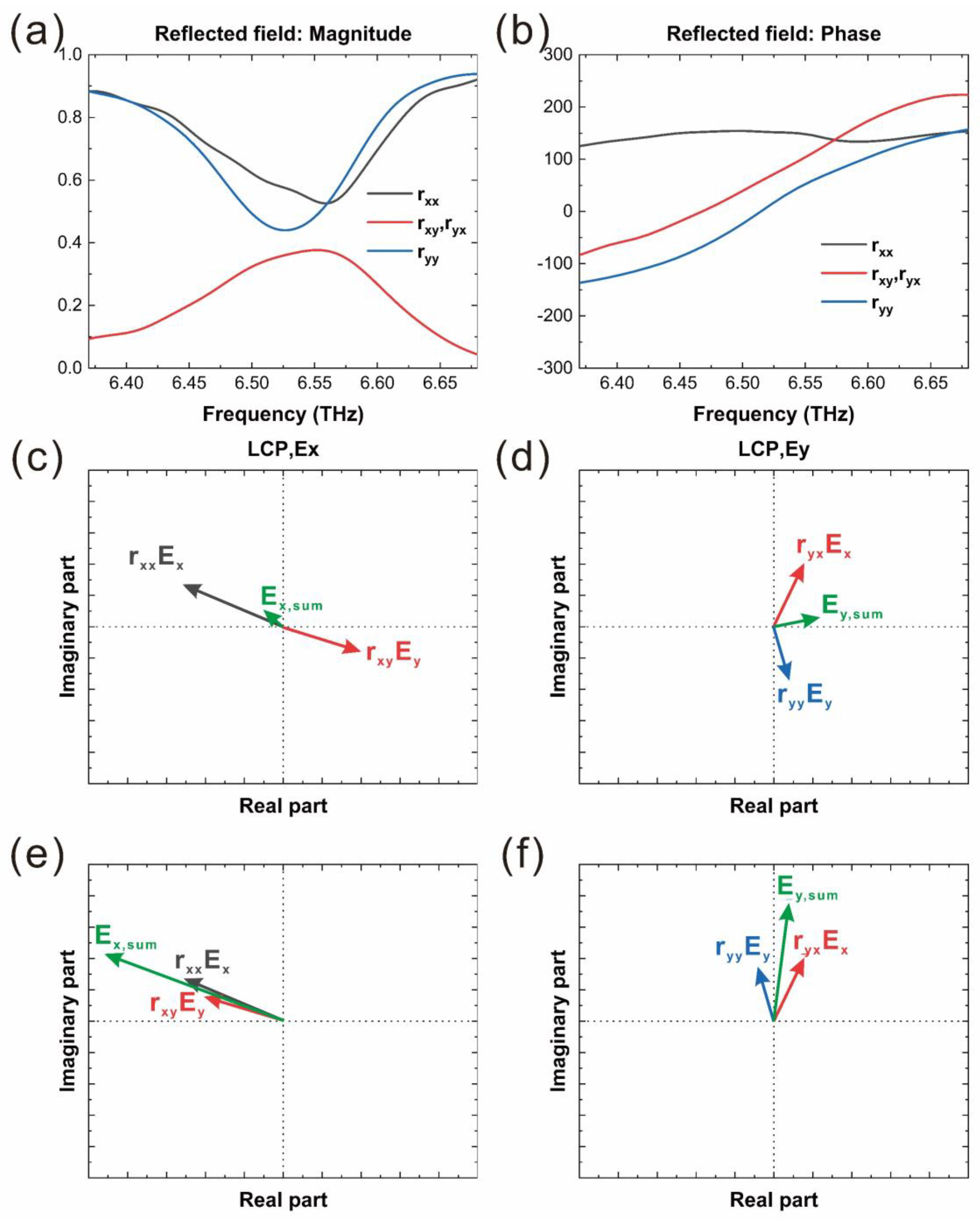
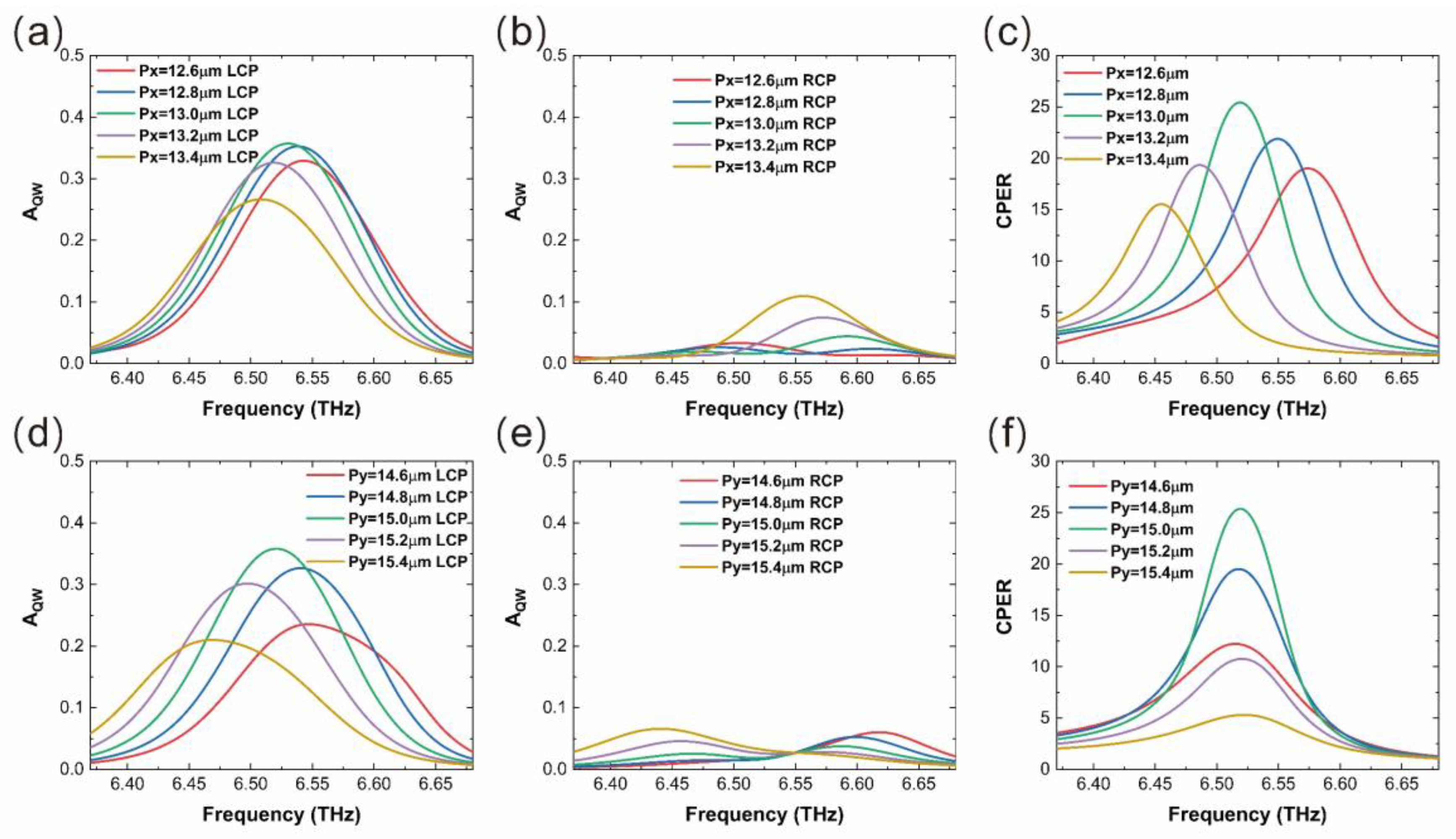
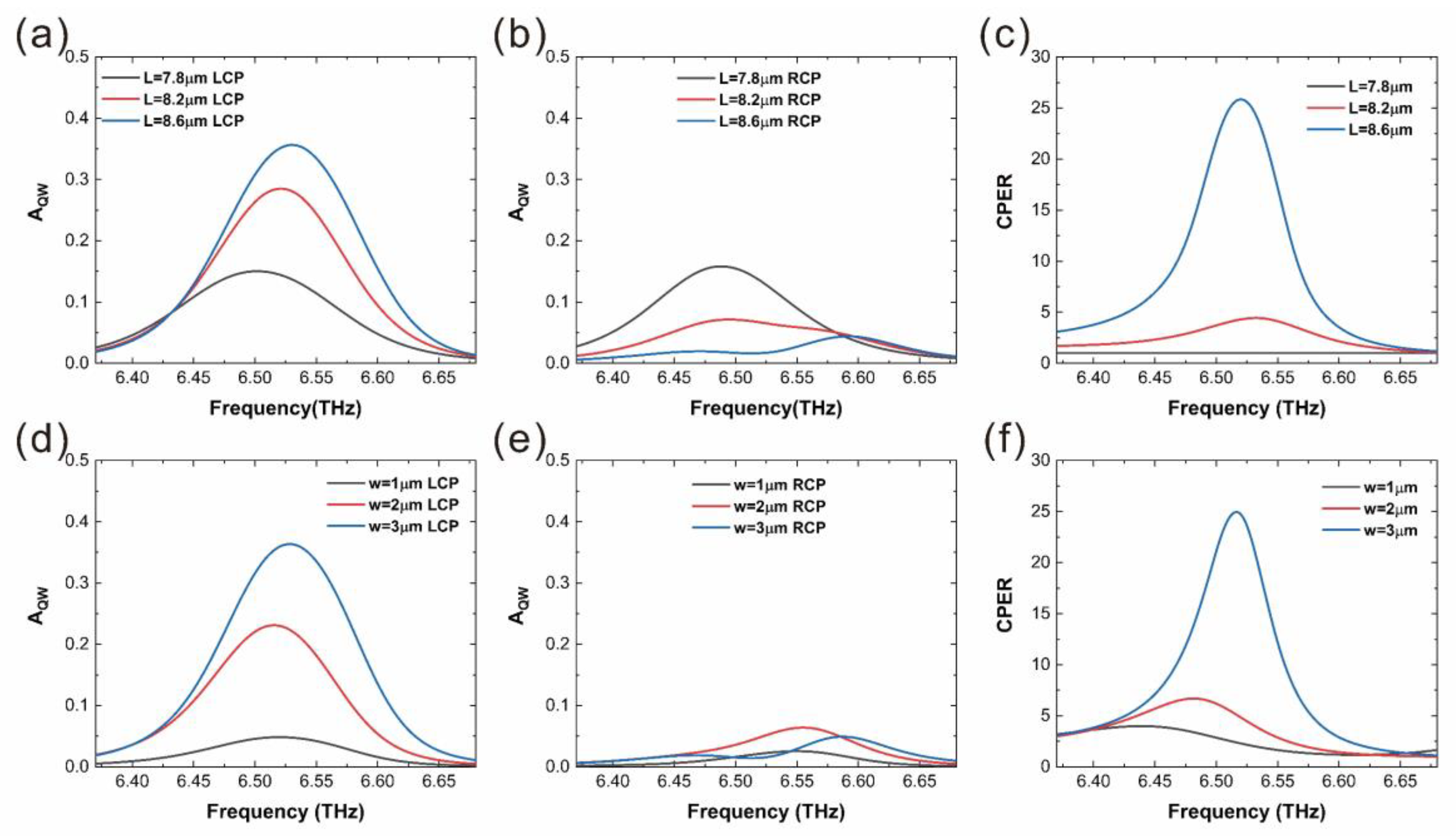
3. Result and Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chenault, D.B.; Pezzaniti, J.L. Polarization imaging through scattering media. In Proceedings of the Polarization Analysis, Measurement, and Remote Sensing III, San Diego, CA, USA, 2–4 August 2000; SPIE: Bellingham, WA, USA, 2000; Volume 4133, pp. 124–133. [Google Scholar]
- Feng, W.; Kim, J.Y.; Wang, X.; Calcaterra, H.A.; Qu, Z.; Meshi, L.; Kotov, N.A. Assembly of mesoscale helices with near-unity enantiomeric excess and light-matter interactions for chiral semiconductors. Sci. Adv. 2017, 3, e1601159. [Google Scholar] [CrossRef] [PubMed]
- Sherson, J.F.; Krauter, H.; Olsson, R.K.; Julsgaard, B.; Hammerer, K.; Cirac, I.; Polzik, E.S. Quantum teleportation between light and matter. Nature 2006, 443, 557–560. [Google Scholar] [CrossRef] [PubMed]
- Farshchi, R.; Ramsteiner, M.; Herfort, J.; Tahraoui, A.; Grahn, H.T. Optical communication of spin information between light emitting diodes. Appl. Phys. Lett. 2011, 98, 162508. [Google Scholar] [CrossRef]
- Kwon, J.; Tamura, M.; Lucas, P.W.; Hashimoto, J.; Kusakabe, N.; Kandori, R.; Nakajima, Y.; Nagayama, T.; Nagata, T.; Hough, J.H. Near-infrared circular polarization images of NGC, 6.3.3.4.-V. Astrophys. J. Lett. 2013, 765, L6. [Google Scholar] [CrossRef]
- Tang, Y.; Cohen, A.E. Enhanced enantioselectivity in excitation of chiral molecules by superchiral light. Science 2011, 332, 333–336. [Google Scholar] [CrossRef] [PubMed]
- Nagali, E.; Sciarrino, F.; De Martini, F.; Marrucci, L.; Piccirillo, B.; Karimi, E.; Santamato, E. Quantum information transfer from spin to orbital angular momentum of photons. Phys. Rev. Lett. 2009, 103, 013601. [Google Scholar] [CrossRef] [PubMed]
- Fujita, T.; Morimoto, K.; Kiyama, H.; Allison, G.; Larsson, M.; Ludwig, A.; Valentin, S.R.; Wieck, A.W.; Oiwa, A.; Tarucha, S. Angular momentum transfer from photon polarization to an electron spin in a gate-defined quantum dot. Nat. Commun. 2019, 10, 2991. [Google Scholar] [CrossRef] [PubMed]
- Togan, E.; Chu, Y.; Trifonov, A.S.; Jiang, L.; Maze, J.; Childress, L.; Dutt, M.V.G.; Sørensen, A.S.; Hemmer, P.R.; Zibrov, A.S.; et al. Quantum entanglement between an optical photon and a solid-state spin qubit. Nature 2010, 466, 730–734. [Google Scholar] [CrossRef]
- Sayed, M.; Yu, J.; Liu, G.; Jaroniec, M. Non-noble plasmonic metal-based photocatalysts. Chem. Rev. 2022, 122, 10484–10537. [Google Scholar] [CrossRef]
- Weisman, D.; Carmesin, C.M.; Rozenman, G.G.; Efremov, M.A.; Shemer, L.; Schleich, W.P.; Arie, A. Diffractive guiding of waves by a periodic array of slits. Phys. Rev. Lett. 2021, 127, 014303. [Google Scholar] [CrossRef]
- Wang, L.; Hasanzadeh Kafshgari, M.; Meunier, M. Optical properties and applications of plasmonic-metal nanoparticles. Adv. Funct. Mater. 2020, 30, 2005400. [Google Scholar] [CrossRef]
- Jauffred, L.; Samadi, A.; Klingberg, H.; Bendix, P.M.; Oddershede, L.B. Plasmonic heating of nanostructures. Chem. Rev. 2019, 119, 8087–8130. [Google Scholar] [CrossRef]
- Zhou, J.; Deng, J.; Shi, M.; Chu, Z.; Li, H.; Dong, R.; Chen, X. Cavity coupled plasmonic resonator enhanced infrared detectors. Appl. Phys. Lett. 2021, 119, 160504. [Google Scholar] [CrossRef]
- Mackay, T.G.; Lakhtakia, A. Negatively refracting chiral metamaterials: A review. SPIE Rev. 2010, 1, 018003. [Google Scholar] [CrossRef]
- Liu, W.; Mei, L.; Li, Y.; Yu, L.; Lai, Z.; Yu, T.; Chen, H. Controlling the spin-selective absorption with two-dimensional chiral plasmonic gratings. Opt. Lett. 2019, 44, 5868–5871. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Coppens, Z.J.; Besteiro, L.V.; Wang, W.; Govorov, A.O.; Valentine, J. Circularly polarized light detection with hot electrons in chiral plasmonic metamaterials. Nat. Commun. 2015, 6, 8379. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Jia, H.; Yao, K.; Cai, W.; Chen, H.; Liu, Y. Circular dichroism metamirrors with near-perfect extinction. ACS Photonics 2016, 3, 2096–2101. [Google Scholar] [CrossRef]
- Plum, E.; Zheludev, N.I. Chiral mirrors. Appl. Phys. Lett. 2015, 106, 221901. [Google Scholar] [CrossRef]
- Kang, L.; Rodrigues, S.P.; Taghinejad, M.; Lan, S.; Lee, K.T.; Liu, Y.; Werner, D.H.; Urbas, A.; Cai, W. Preserving spin states upon reflection: Linear and nonlinear responses of a chiral meta-mirror. Nano Lett. 2017, 17, 7102–7109. [Google Scholar] [CrossRef]
- Collin, S.; Pardo, F.; Bardou, N.; Lemaître, A.; Averin, S.; Pelouard, J.L. Harvesting light at the nanoscale by GaAs-gold nanowire arrays. Opt. Express 2011, 19, 17293–17297. [Google Scholar] [CrossRef]
- Assefa, S.; Xia, F.; Vlasov, Y.A. Reinventing germanium avalanche photodetector for nanophotonic on-chip optical interconnects. Nature 2010, 464, 80–84. [Google Scholar] [CrossRef] [PubMed]
- Green, M.A.; Zhao, J.; Wang, A.; Wenham, S.R. Very high efficiency silicon solar cells-science and technology. IEEE Trans. Electron Devices 1999, 46, 1940–1947. [Google Scholar] [CrossRef]
- Zhang, M.; Yeow, J.T.W. Nanotechnology-Based Terahertz Biological Sensing: A review of its current state and things to come. IEEE Nanotechnol. Mag. 2016, 10, 30–38. [Google Scholar] [CrossRef]
- Giles, R.H.; Doradla, F.; Martin, J.; Joseph, C.S. Applications of terahertz frequency technologies in biology. In Proceedings of the 2017 42nd International Conference on Infrared, Millimeter, and Terahertz Waves (IRMMW-THz), Cancun, Mexico, 27 August–1 September 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–2. [Google Scholar]
- AlNabooda, M.O.; Shubair, R.M.; Rishani, N.R.; Aldabbagh, G. Terahertz spectroscopy and imaging for the detection and identification of illicit drugs. In Proceedings of the 2017 Sensors Networks Smart and Emerging Technologies (SENSET), Beiriut, Lebanon, 12–14 September 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–4. [Google Scholar]
- Bin, L.; Liping, C. Exploration on precision farming pollution detection using THz technology. Infrared Laser Eng. 2016, 45, 425003. [Google Scholar] [CrossRef]
- Yazgan, A.; Jofre, L.; Romeu, J. Comparative efficiency and power assessment of optical photoconductive material-based terahertz sources for wireless communication systems. J. Circuits Syst. Comput. 2019, 28, 1950005. [Google Scholar] [CrossRef]
- Hossain, Z.; Xia, Q.; Jornet, J.M. TeraSim: An ns-3 extension to simulate Terahertz-band communication networks. Nano Commun. Netw. 2018, 17, 36–44. [Google Scholar] [CrossRef]
- Tuovinen, J. Method for testing reflector antennas at THz frequencies. IEEE Antennas Propag. Mag. 1993, 35, 7–13. [Google Scholar] [CrossRef]
- Mueller, E.R.; Henschke, R.; Robotham, W.E., Jr.; Newman, L.A.; Laughman, L.M.; Hart, R.A.; Kennedy, J.; Pickett, H.M. Terahertz local oscillator for the Microwave Limb Sounder on the Aura satellite. Appl. Opt. 2007, 46, 4907–4915. [Google Scholar] [CrossRef]
- Gaidis, M.C.; Pickett, H.M.; Smith, C.D.; Martin, S.C.; Smith, R.P.; Siegel, P.H. A 2.5-THz receiver front end for spaceborne applications. IEEE Trans. Microw. Theory Tech. 2000, 48, 733–739. [Google Scholar] [CrossRef]
- Shalini, M. A compact antenna structure for circular polarized terahertz radiation. Optik 2021, 231, 166393. [Google Scholar]
- Chu, Z.; Zhou, J.; Dai, X.; Li, F.; Lan, M.; Ji, Z.; Lu, W.; Chen, X. Circular polarization discrimination enhanced by anisotropic media. Adv. Opt. Mater. 2020, 8, 1901800. [Google Scholar] [CrossRef]
- Li, J.; Li, J.; Yang, Y.; Li, J.; Zhang, Y.; Wu, L.; Zhang, Z.; Yang, M.; Zheng, C.; Li, J.; et al. Metal-graphene hybrid active chiral metasurfaces for dynamic terahertz wavefront modulation and near field imaging. Carbon 2020, 163, 34–42. [Google Scholar] [CrossRef]
- Seilmeier, A.; Plödereder, U.; Baier, J.; Weimann, G. Quantum Well Intersubband Transition Physics and Devices; Liu, H.C., Levine, B.F., Anderson, J.Y., Eds.; NATO ASI Series E 270; Kluwer: Dordrecht, The Netherlands, 1994; p. 421. [Google Scholar]
- Zhen, T.; Zhou, J.; Li, Z.; Chen, X. Realization of both high absorption of active materials and low ohmic loss in plasmonic cavities. Adv. Opt. Mater. 2019, 7, 1801627. [Google Scholar] [CrossRef]
- Tang, W.; Zhou, J.; Zheng, Y.; Zhou, Y.; Hao, J.; Chen, X.; Lu, W. All-dielectric resonant waveguide based quantum well infrared photodetectors for hyperspectral detection. Optics Commun. 2018, 427, 196–201. [Google Scholar] [CrossRef]
- Chu, Z.; Zhou, Y.; Zhou, J.; Chen, P.; Li, Z.; Lu, W.; Chen, X. Quantum well infrared detectors enhanced by faceted plasmonic cavities. Infrared Phys. Technol. 2021, 116, 103746. [Google Scholar] [CrossRef]
- Dai, X.; Chu, Z.; Deng, J.; Li, F.; Zhou, J.; Xiong, D.; Zhou, X.; Chen, X.; Li, N.; Li, Z.; et al. Detection band expansion by independently tunable double resonances in a long-wavelength dual-color QWIP. Opt. Express 2022, 30, 43579–43589. [Google Scholar] [CrossRef]
- Helm, M. The basic physics of intersubband transitions. In Semiconductors and Semimetals; Elsevier: Amsterdam, The Netherlands, 1999; Volume 62, pp. 1–99. [Google Scholar]
- Lee, S.J.; Ku, Z.; Barve, A.; Montoya, J.; Jang, W.Y.; Brueck, S.R.J.; Sundaram, M.; Reisinger, A.; Krishna, S.; Noh, S.K. A monolithically integrated plasmonic infrared quantum dot camera. Nat. Commun. 2011, 2, 286. [Google Scholar] [CrossRef]
- Levine, B.F. Quantum-well infrared photodetectors. J. Appl. Phys. 1993, 74, R1–R81. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants of Solids; Academic Press: New York, NY, USA, 1998; Volume 3. [Google Scholar]
- Rehm, R.; Schneider, H.; Schönbein, C.S.; Walther, M. Noise current investigations of g-r noise limited and shot noise limited QWIPs. Phys. E Low-Dimens. Syst. Nanostructures 2000, 7, 124–129. [Google Scholar] [CrossRef]
- Zheng, Y. Research on Material Growth and Optical Coupling Devices of Terahertz Quantum Well Photodetectors. Ph.D. Dissertation, University of Chinese Academy of Sciences, Beijing, China, 2020. Available online: https://kns.cnki.net/kcms2/article/abstract?v=MwumK-qo9AANfBclwM2M5OjAZAEaIVuTPy-laiTHRSeyZzM-Kzp3Jtp5MZKoBq5eEXrXOEQyklWOq7P5rlJsB_f0_f7yvkAPgJMwG6qfAvi4p0avCEF3RYHhuli8iz8eeZG6rsg1IhM=&uniplatform=NZKPT&language=CHS (accessed on 1 June 2019).
- Collett, E. Field Guide to Polarization; SPIE: Bellingham, WA, USA, 2005. [Google Scholar]
- Shen, J.; Zhou, J.; Zhu, T.; Deng, J.; Wang, B.; Jing, W.; Ma, J.; Qin, X.; Liu, H.; Li, J.; et al. On-chip long-wavelength infrared polarimeter for full-Stokes polarization detection. Opt. Mater. Express 2023, 13, 2475–2488. [Google Scholar] [CrossRef]
- De Abajo, F.G. Colloquium: Light scattering by particle and hole arrays. Rev. Mod. Phys. 2007, 79, 1267. [Google Scholar] [CrossRef]
- Sihvola, A.H. Electromagnetic Mixing Formulas and Applications; IET: London, UK, 1999. [Google Scholar]
- Zhen, Y.; Deng, J.; Bu, Y.; Dai, X.; Yu, Y.; Shi, M.; Wang, R.; Ye, T.; Chen, G.; Zhou, J. Recent advances in on-chip infrared polarization detection. J. Infrared Millim. Waves 2024, 43, 52–62. [Google Scholar]
- Wang, W.; Besteiro, L.V.; Liu, T.; Wu, C.; Sun, J.; Yu, P.; Chang, L.; Wang, Z.; Govorov, A.O. Generation of hot electrons with chiral metamaterial perfect absorbers: Giant optical chirality for polarization-sensitive photochemistry. ACS Photonics 2019, 6, 3241–3252. [Google Scholar] [CrossRef]
- Mahmud, M.S.; Rosenmann, D.; Czaplewski, D.A.; Gao, J.; Yang, X. Chiral plasmonic metasurface absorbers in the mid-infrared wavelength range. Opt. Lett. 2020, 45, 5372–5375. [Google Scholar] [CrossRef] [PubMed]
- Peng, J.; Cumming, B.P.; Gu, M. Direct detection of photon spin angular momentum by a chiral graphene mid-infrared photodetector. Opt. Lett. 2019, 44, 2998–3001. [Google Scholar] [CrossRef]
- Ouyang, L.; Wang, W.; Rosenmann, D.; Czaplewski, D.A.; Gao, J.; Yang, X. Near-infrared chiral plasmonic metasurface absorbers. Opt. Express 2018, 26, 31484–31489. [Google Scholar] [CrossRef]
- Pan, M.; Li, Q.; Hong, Y.; Cai, L.; Lu, J.; Qiu, M. Circular-polarization-sensitive absorption in refractory metamaterials composed of molybdenum zigzag arrays. Opt. Express 2018, 26, 17772–17780. [Google Scholar] [CrossRef]
- Chen, Y.; Gao, J.; Yang, X. Chiral metamaterials of plasmonic slanted nanoapertures with symmetry breaking. Nano Lett. 2018, 18, 520–527. [Google Scholar] [CrossRef] [PubMed]
- Ouyang, L.; Rosenmann, D.; Czaplewski, D.A.; Gao, J.; Yang, X. Broadband infrared circular dichroism in chiral metasurface absorbers. Nanotechnology 2020, 31, 295203. [Google Scholar] [CrossRef]
- Fang, Y.; Verre, R.; Shao, L.; Nordlander, P.; Käll, M. Hot electron generation and cathodoluminescence nanoscopy of chiral split ring resonators. Nano Lett. 2016, 16, 5183–5190. [Google Scholar] [CrossRef]
- Han, C.; Tam, W.Y. Broadband optical magnetism in chiral metallic nanohole arrays by shadowing vapor deposition. Appl. Phys. Lett. 2016, 109, 251102. [Google Scholar] [CrossRef]
- Li, Y.; Jing, Z.; Bai, Y.; Ullah, H.; Zhang, Z. Effects of electric field coupling on the circular dichroism of composite nanostructures. J. Opt. 2020, 22, 055002. [Google Scholar] [CrossRef]
- Lumerical Inc. Available online: http://www.lumerical.com/products/ (accessed on 1 January 2022).
- COMSOL. Multiphysics v. 6.0. cn.comsol.com; COMSOL AB: Stockholm, Sweden, 2023. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, F.; Zhou, J.; Deng, J.; Shen, J.; Zhu, T.; Jing, W.; Dai, X.; Ye, J.; Zhang, Y.; Huang, J.; et al. Enhanced THz Circular-Polarization Detection in Miniaturized Chips with Chiral Antennas. Photonics 2024, 11, 162. https://doi.org/10.3390/photonics11020162
Li F, Zhou J, Deng J, Shen J, Zhu T, Jing W, Dai X, Ye J, Zhang Y, Huang J, et al. Enhanced THz Circular-Polarization Detection in Miniaturized Chips with Chiral Antennas. Photonics. 2024; 11(2):162. https://doi.org/10.3390/photonics11020162
Chicago/Turabian StyleLi, Fangzhe, Jing Zhou, Jie Deng, Jinyong Shen, Tianyun Zhu, Wenji Jing, Xu Dai, Jiexian Ye, Yujie Zhang, Junwei Huang, and et al. 2024. "Enhanced THz Circular-Polarization Detection in Miniaturized Chips with Chiral Antennas" Photonics 11, no. 2: 162. https://doi.org/10.3390/photonics11020162
APA StyleLi, F., Zhou, J., Deng, J., Shen, J., Zhu, T., Jing, W., Dai, X., Ye, J., Zhang, Y., Huang, J., & Chen, X. (2024). Enhanced THz Circular-Polarization Detection in Miniaturized Chips with Chiral Antennas. Photonics, 11(2), 162. https://doi.org/10.3390/photonics11020162





