The Origin of Threshold Reduction in Random Lasers Based on MoS2/Au NPs: Charge Transfer
Abstract
1. Introduction
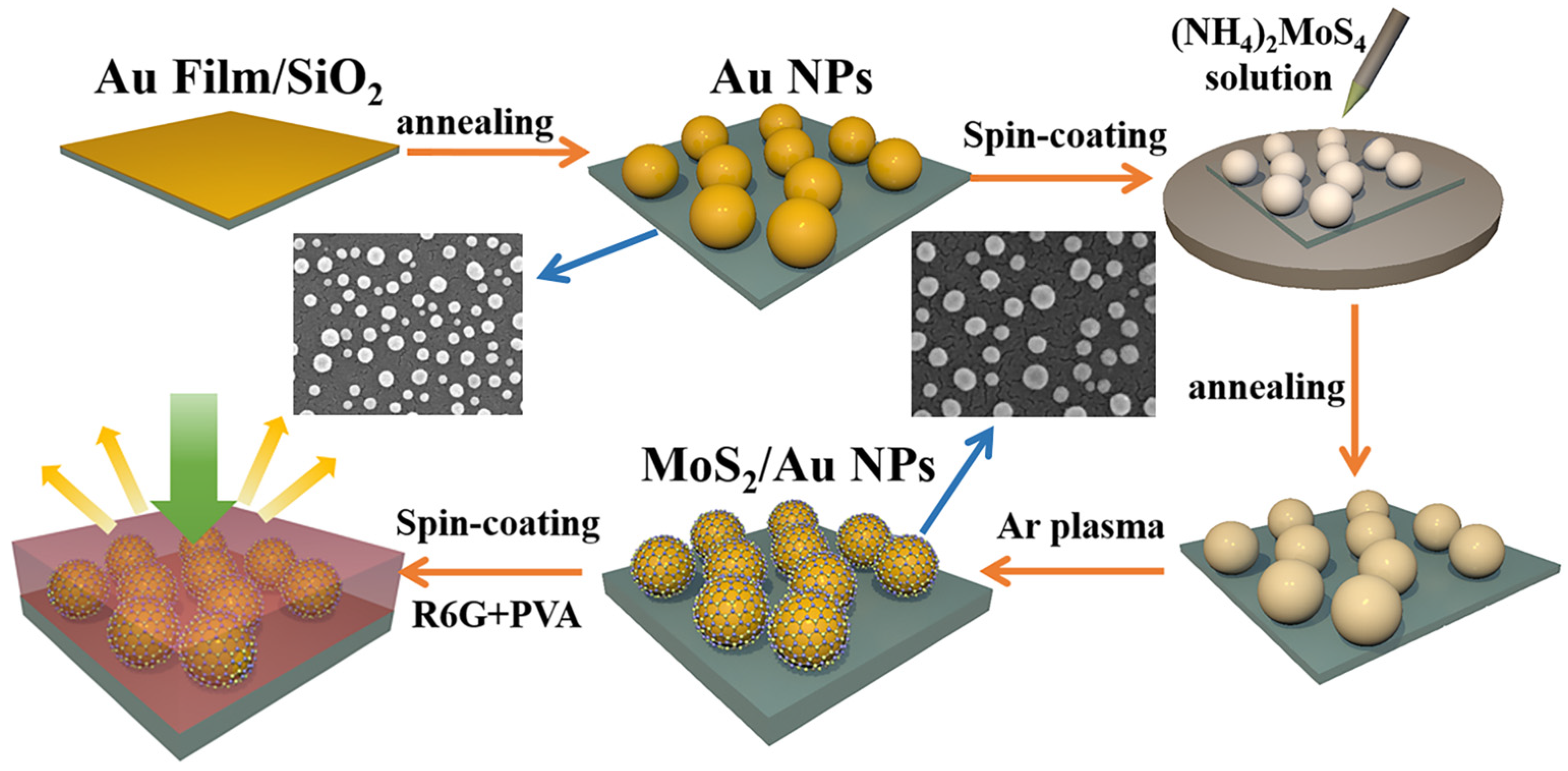
2. Preparation of Experimental Sample
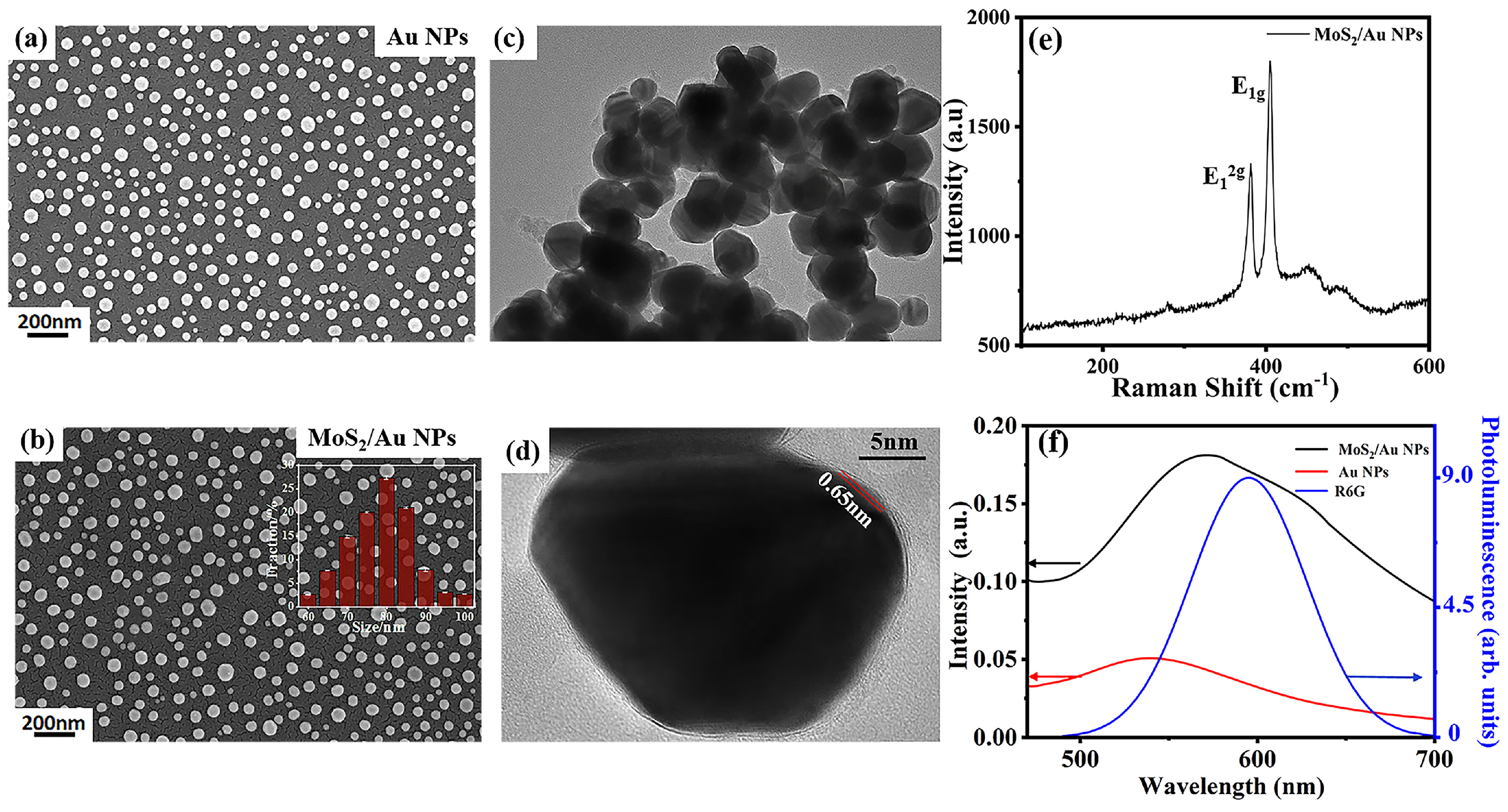
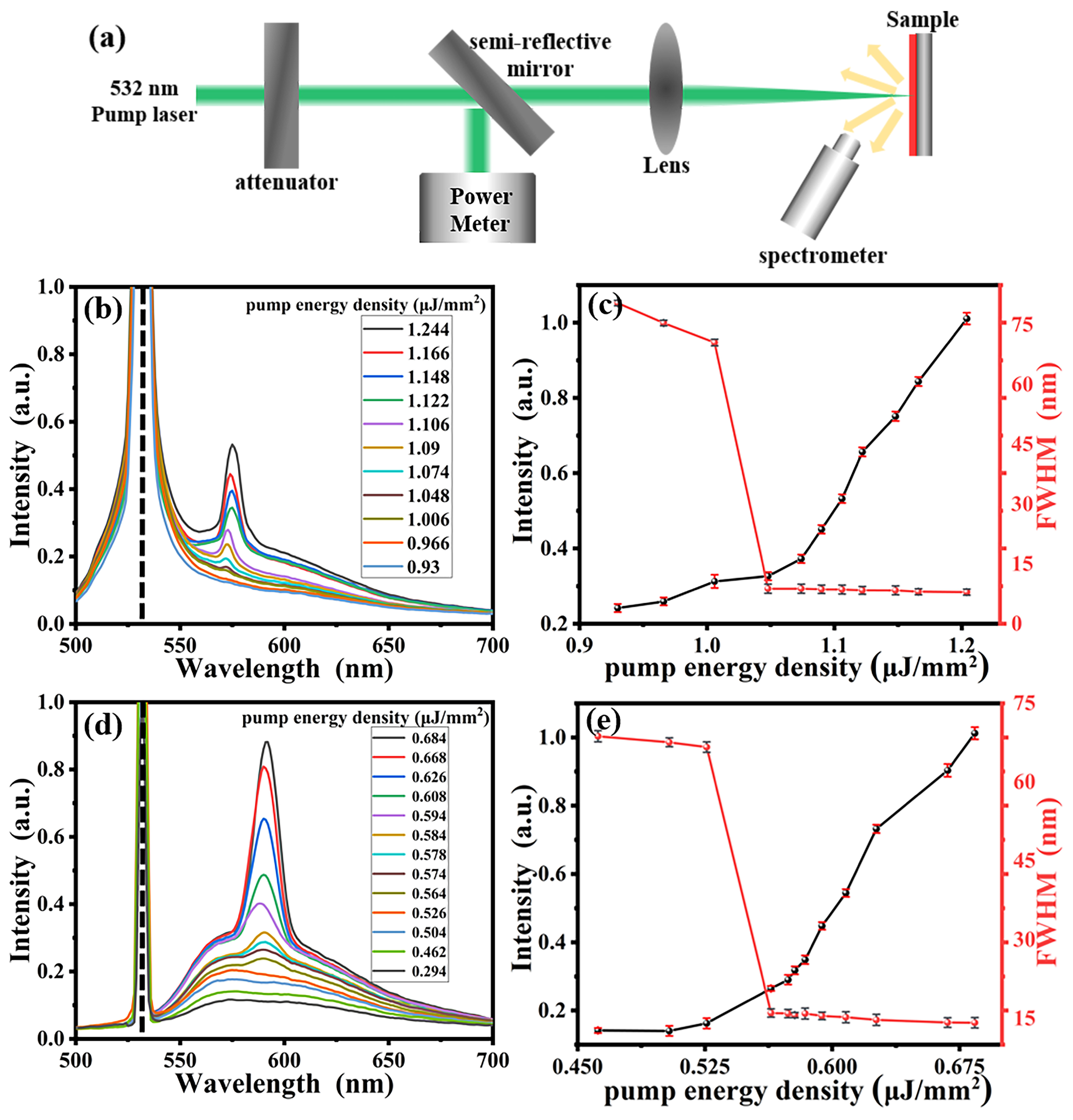
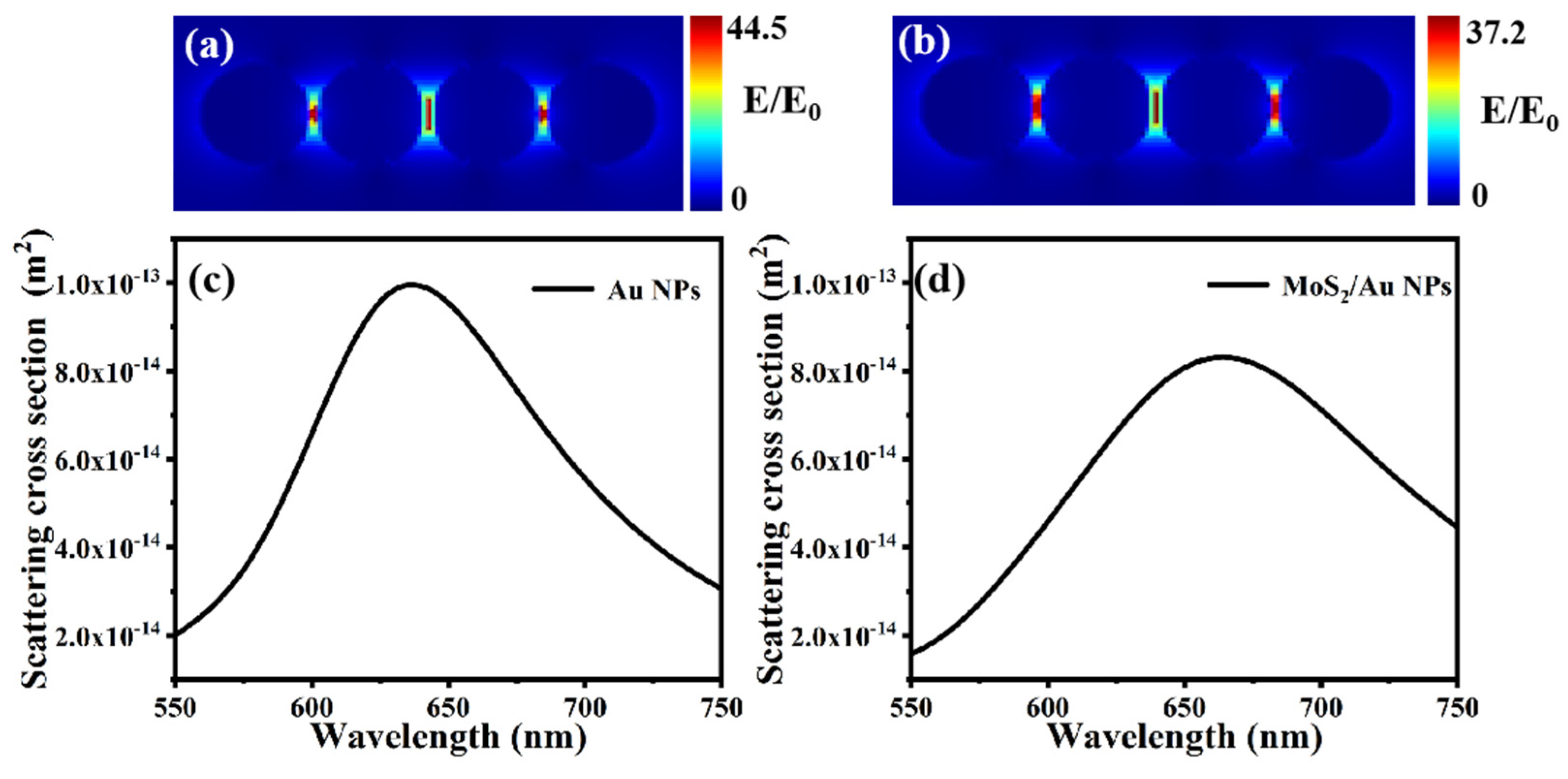
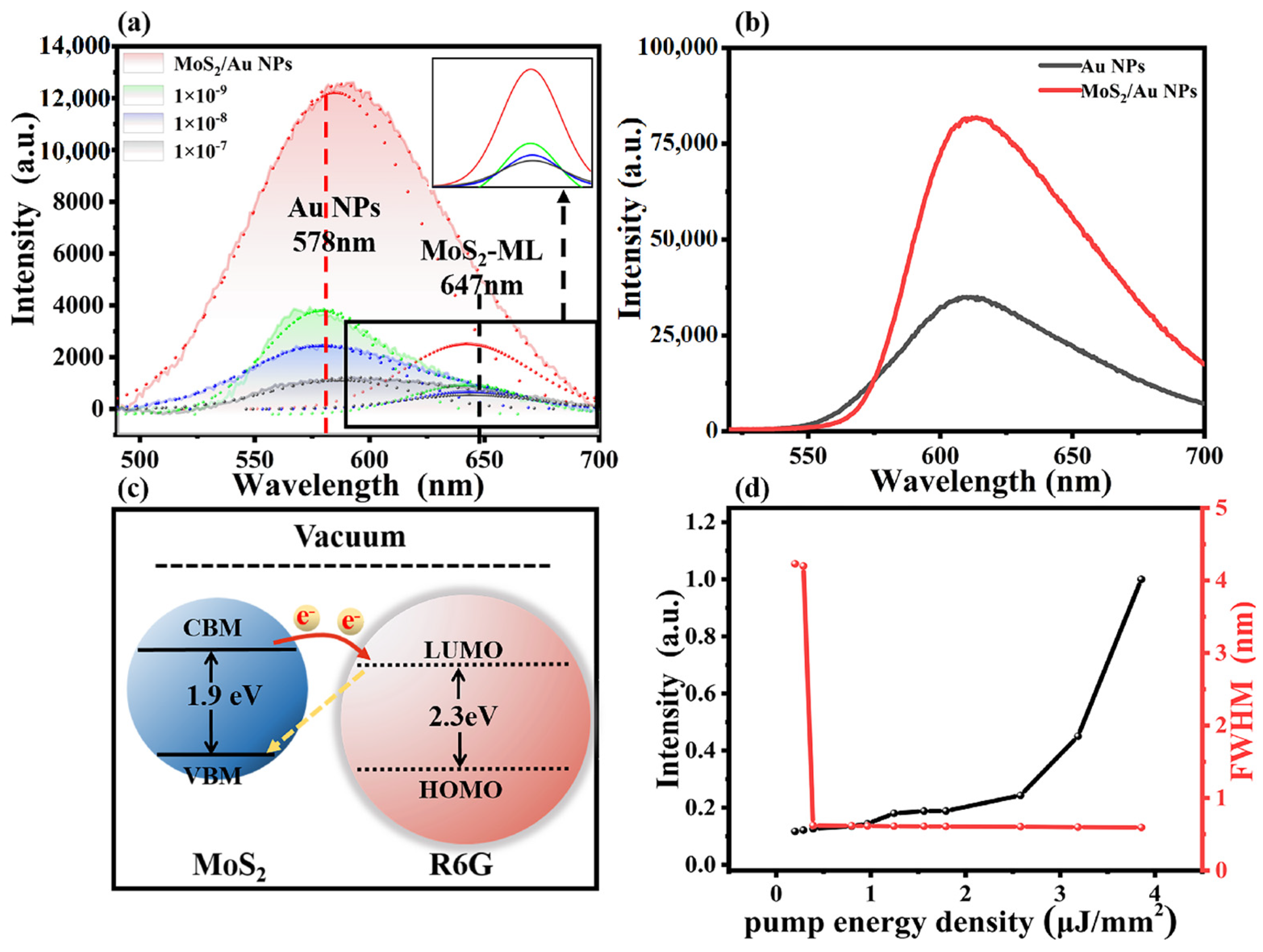
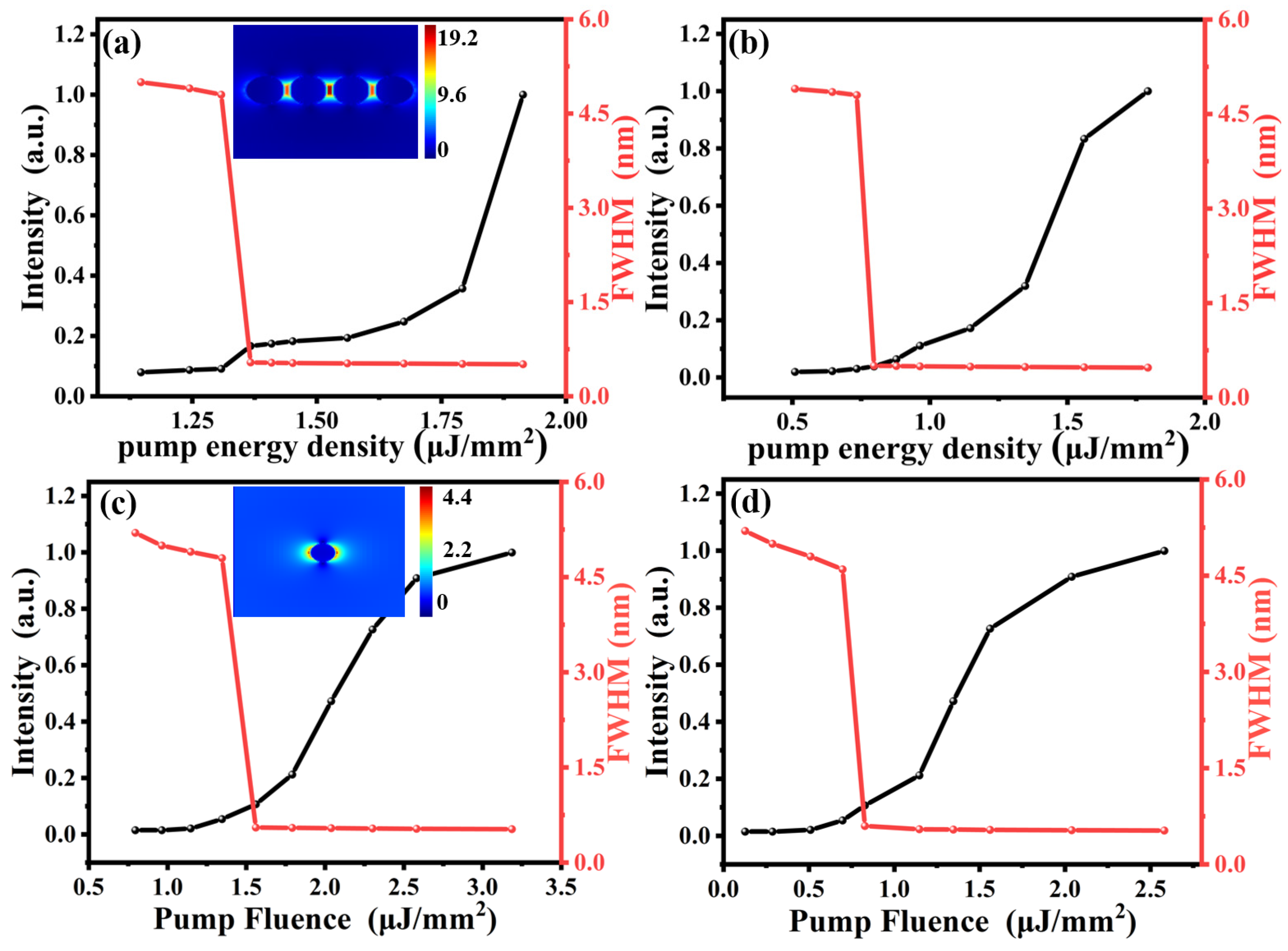
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bian, Y.; Xue, H.; Wang, Z. Programmable Random Lasing Pluses Based on Waveguide-Assisted Random Scattering Feedback. Laser Photonics Rev. 2021, 15, 2000506. [Google Scholar] [CrossRef]
- Consoli, A.; Caselli, N.; López, C. Electrically driven random lasing from a modified Fabry–Pérot laser diode. Nat. Photonics 2022, 16, 219–225. [Google Scholar] [CrossRef]
- Luan, F.; Gu, B.; Gomes, A.S.; Yong, K.T.; Wen, S.; Prasad, P.N. Lasing in nanocomposite random media. Nano Today 2015, 10, 168–192. [Google Scholar] [CrossRef]
- Azmi, A.N.; Wan Ismail, W.Z.; Abu Hassan, H.; Halim, M.M.; Zainal, N.; Muskens, O.L.; Wan Ahmad Kamil, W.M. Review of open cavity random lasers as laser-based sensors. ACS Sens. 2022, 7, 914–928. [Google Scholar] [CrossRef] [PubMed]
- Gomes, A.S.; Moura, A.L.; de Araújo, C.B.; Raposo, E.P. Recent advances and applications of random lasers and random fiber lasers. Prog. Quantum Electron. 2021, 78, 100343. [Google Scholar] [CrossRef]
- Gummaluri, V.S.; Nair, R.V.; Krishnan, S.R.; Vijayan, C. Femtosecond laser-pumped plasmonically enhanced near-infrared random laser based on engineered scatterers. Opt. Lett. 2017, 42, 5002–5005. [Google Scholar] [CrossRef]
- Haddawi, S.F.; Humud, H.R.; Hamidi, S.M. Tunable low power piezo-plasmonic random laser under external voltage. Optik 2020, 207, 164482. [Google Scholar] [CrossRef]
- Xia, J.Y.; He, J.J.; Xie, K.; Zhang, X.J.; Hu, L.; Li, Y.X.; Chen, X.; Ma, J.; Wen, J.; Chen, J.; et al. Replica symmetry breaking in FRET-assisted random laser based on electrospun polymer fiber. Ann. Phys. 2019, 531, 1900066. [Google Scholar] [CrossRef]
- Li, Y.; Xie, K.; Zhang, X.; Hu, Z.; Ma, J.; Chen, X.; Zhang, J.; Liu, Z.; Chen, D. Coherent Random Lasing Realized in Polymer Vesicles. Photonic Sens. 2019, 10, 254–264. [Google Scholar] [CrossRef]
- Goldberg, I.; Annavarapu, N.; Leitner, S.; Elkhouly, K.; Han, F.; Verellen, N.; Kuna, T.; Qiu, W.; Rolin, C.; Genoe, J.; et al. Multimode Lasing in All-Solution-Processed UV-Nanoimprinted Distributed Feedback MAPbI3 Perovskite Waveguides. ACS Photonics 2023, 10, 1591–1600. [Google Scholar] [CrossRef]
- Shi, X.; Chang, Q.; Bian, Y.; Cui, H.; Wang, Z. Line Width-Tunable Random Laser Based on Manipulating Plasmonic Scattering. ACS Photonics 2019, 6, 2245–2251. [Google Scholar] [CrossRef]
- Ejbarah, R.A.; Jassim, J.M.; Hamidi, S.M. The effect of dye concentration and cell thickness on dye–polymer random laser action. Opt. Quantum Electron. 2021, 53, 116. [Google Scholar] [CrossRef]
- Gayathri, R.; Monika, K.; Murukeshan, V.M.; Vijayan, C. Low threshold incoherent random lasing with spectral overlap optimization of size-tuned plasmonic nanorods. Opt. Laser Technol. 2021, 139, 106959. [Google Scholar] [CrossRef]
- Zhao, Q.; Ye, L.; Cheng, Z.; Hong, S.; Penty, R.; White, I. Random lasing action from PMMA waveguide doped with CdSe/ZnS CQDs plasmonically enhanced by Ag nanoislands. Opt. Laser Technol. 2020, 131, 106358. [Google Scholar] [CrossRef]
- Wan, Y.; Wang, H.; Li, H.; Ye, R.; Zhang, X.; Lyu, J.; Cai, Y. Low-threshold random lasers enhanced by titanium nitride nanoparticles suspended randomly in gain solutions. Opt. Express 2022, 30, 8222–8233. [Google Scholar] [CrossRef] [PubMed]
- Zhang, N.; Ning, S.; Dai, K.; Zhang, Y.; Wu, Y.; Yuan, F.; Zhang, F. Random lasing based on a nanoplasmonic hybrid structure composed of (Au core)-(Ag shell) nanorods with Ag film. Opt. Mater. Express 2020, 10, 1204–1214. [Google Scholar] [CrossRef]
- Xu, L.; Li, F.; Wei, L.; Zhou, J.; Liu, S. Design of Surface Plasmon Nanolaser Based on MoS2. Appl. Sci. 2018, 8, 2110. [Google Scholar] [CrossRef]
- Wan, Y.; Li, X.; Wang, Y.; Li, Z.; Liu, X.; Cai, Y. Low-threshold random lasers based on the DCM-DEG gain system with graphene nanosheets. Opt. Express 2023, 31, 6713–6721. [Google Scholar] [CrossRef] [PubMed]
- Shen, Y.L.; Shi, B.R.; Lv, H.; Zhang, S.Y.; Wang, X. Graphene-based Au nanoparticle enhanced dye random laser. Acta Phys. Sin. 2022, 71, 034206. [Google Scholar] [CrossRef]
- Roy, P.K.; Haider, G.; Lin, H.I.; Liao, Y.M.; Lu, C.H.; Chen, K.H.; Chen, L.C.; Shih, W.H.; Liang, C.T.; Chen, Y.F. Multicolor Ultralow-Threshold Random Laser Assisted by Vertical-Graphene Network. Adv. Opt. Mater. 2018, 6, 1800382. [Google Scholar] [CrossRef]
- Yang, F.; Cheng, S.; Zhang, X.; Ren, X.; Li, R.; Dong, H.; Hu, W. 2D organic materials for optoelectronic applications. Adv. Mater. 2018, 30, 1702415. [Google Scholar] [CrossRef]
- Shangguan, Q.; Zhao, Y.; Song, Z.; Wang, J.; Yang, H.; Chen, J.; Yi, Z. High sensitivity active adjustable graphene absorber for refractive index sensing applications. Diam. Relat. Mater. 2022, 128, 109273. [Google Scholar] [CrossRef]
- Xu, X.; Liu, L. MoS2 with Controlled Thickness for Electrocatalytic Hydrogen Evolution. Nanoscale Res. Lett. 2021, 16, 137. [Google Scholar] [CrossRef]
- Zhou, Y.; Liu, Y.; Zhang, M.; Han, Q.; Wang, Y.; Sun, X.; Zhang, X.; Dong, C.; Sun, J.; Tang, Z.; et al. Rationally designed hierarchical N, P co-doped carbon connected 1T/2H-MoS2 heterostructures with cooperative effect as ultrafast and durable anode materials for efficient sodium storage. Chem. Eng. J. 2022, 433, 133778. [Google Scholar] [CrossRef]
- Nalwa, H.S. A review of molybdenum disulfide (MoS2) based photodetectors: From ultra-broadband, self-powered to flexible devices. RSC Adv. 2020, 10, 30529–30602. [Google Scholar] [CrossRef]
- Chen, Y.; Sun, M. Two-dimensional WS2/MoS2 heterostructures: Properties and applications. Nanoscale 2021, 13, 5594–5619. [Google Scholar] [CrossRef]
- An, K.; Chen, M.; He, B.; Ai, H.; Wang, W.; Zhang, Z.; Pan, Z.; Chen, S.; Ip, W.F.; Lo, K.H.; et al. Wafer-Scale 2H-MoS2 Monolayer for High Surface-enhanced Raman Scattering Performance: Charge-Transfer Coupled with Molecule Resonance. Adv. Mater. Technol. 2022, 7, 2200217. [Google Scholar] [CrossRef]
- Sun, L.; Hu, H.; Zhan, D.; Yan, J.; Liu, L.; Teguh, J.S.; Yeow, E.K.L.; Lee, P.S.; Shen, Z. Plasma Modified MoS2 Nanoflakes for Surface Enhanced Raman Scattering. Small 2014, 10, 1090–1095. [Google Scholar] [CrossRef]
- Li, Z.; Jiang, S.; Xu, S.; Zhang, C.; Qiu, H.; Li, C.; Sheng, Y.; Huo, Y.; Yang, C.; Man, B. Few-layer MoS2-encapsulated Cu nanoparticle hybrids fabricated by two-step annealing process for surface enhanced Raman scattering. Sens. Actuators B Chem. 2016, 230, 645–652. [Google Scholar] [CrossRef]
- Jayasekara, C.; Premaratne, M.; Gunapala, S.D.; Stockman, M.I. MoS2 spaser. J. Appl. Phys. 2016, 13, 133101. [Google Scholar] [CrossRef]
- Dixit, T.; Arora, A.; Krishnan, A.; Ganapathi, K.L.; Nayak, P.K.; Rao, M.S.R. Near Infrared Random Lasing in Multilayer MoS2. ACS Omega 2018, 3, 14097–14102. [Google Scholar] [CrossRef]
- Zhai, T.; Zhang, X.; Pang, Z.; Su, X.; Liu, H.; Feng, S.; Wang, L. Random Laser Based on Waveguided Plasmonic Gain Channels. Nano Lett. 2011, 11, 4295–4298. [Google Scholar] [CrossRef]
- Cui, Y.; Wang, J.; Li, Y.; Wu, Y.; Been, E.; Zhang, Z.; Cui, Y. Twisted epitaxy of gold nanodisks grown between twisted substrate layers of molybdenum disulfide. Science 2024, 383, 212–219. [Google Scholar] [CrossRef]
- Bornacelli, J.; Torres-Torres, C.; Silva-Pereyra, H.; Labrada-Delgado, G.; Crespo-Sosa, A.; Cheang-Wong, J.; Oliver, A. Superlinear photoluminescence by ultrafast laser pulses in dielectric matrices with metal nanoclusters. Sci. Rep. 2019, 9, 5699. [Google Scholar] [CrossRef]
- Ziegler, J.; Djiango, M.; Vidal, C.; Hrelescu, C.; Klar, T.A. Gold nanostars for random lasing enhancement. Opt. Express 2015, 23, 15152–15159. [Google Scholar] [CrossRef]
- Chang, S.H.; Taflove, A. Finite-difference time-domain model of lasing action in a four-level two-electron atomic system. Opt. Express 2004, 16, 3827–3833. [Google Scholar] [CrossRef]
- Yan, M.; Sun, K.; Ning, T.; Zhao, L.; Ren, Y.; Huo, Y. Numerical study of low threshold nanolasers based on quasi continuous beam binding of resonant waveguide grating structures. Acta Phys. Sin. 2023, 4, 044202. [Google Scholar] [CrossRef]
- Hakala, T.K.; Rekola, H.T.; Väkeväinen, A.I.; Martikainen, J.P.; Nečada, M.; Moilanen, A.J.; Törmä, P. Lasing in dark and bright modes of a finite-sized plasmonic lattice. Nat. Commun. 2017, 8, 13687. [Google Scholar] [CrossRef]
- Sun, H.; Yao, M.; Song, Y.; Zhu, L.; Dong, J.; Liu, R.; Li, P.; Zhao, B.; Liu, B. Pressure-induced SERS enhancement in a MoS2/Au/R6G system by a two-step charge transfer process. Nanoscale 2019, 11, 21493–21501. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huo, Y.; Sun, K.; Zhang, Y.; Liu, W.; Wang, J.; Wan, Y.; Zhao, L.; Ning, T.; Li, Z.; Ren, Y. The Origin of Threshold Reduction in Random Lasers Based on MoS2/Au NPs: Charge Transfer. Photonics 2024, 11, 168. https://doi.org/10.3390/photonics11020168
Huo Y, Sun K, Zhang Y, Liu W, Wang J, Wan Y, Zhao L, Ning T, Li Z, Ren Y. The Origin of Threshold Reduction in Random Lasers Based on MoS2/Au NPs: Charge Transfer. Photonics. 2024; 11(2):168. https://doi.org/10.3390/photonics11020168
Chicago/Turabian StyleHuo, Yanyan, Ke Sun, Yuqian Zhang, Weihao Liu, Junkun Wang, Yuan Wan, Lina Zhao, Tingyin Ning, Zhen Li, and Yingying Ren. 2024. "The Origin of Threshold Reduction in Random Lasers Based on MoS2/Au NPs: Charge Transfer" Photonics 11, no. 2: 168. https://doi.org/10.3390/photonics11020168
APA StyleHuo, Y., Sun, K., Zhang, Y., Liu, W., Wang, J., Wan, Y., Zhao, L., Ning, T., Li, Z., & Ren, Y. (2024). The Origin of Threshold Reduction in Random Lasers Based on MoS2/Au NPs: Charge Transfer. Photonics, 11(2), 168. https://doi.org/10.3390/photonics11020168




