Active Optical Tuning of Azopolymeric Whispering Gallery Mode Microresonators for Filter Applications
Abstract
1. Introduction
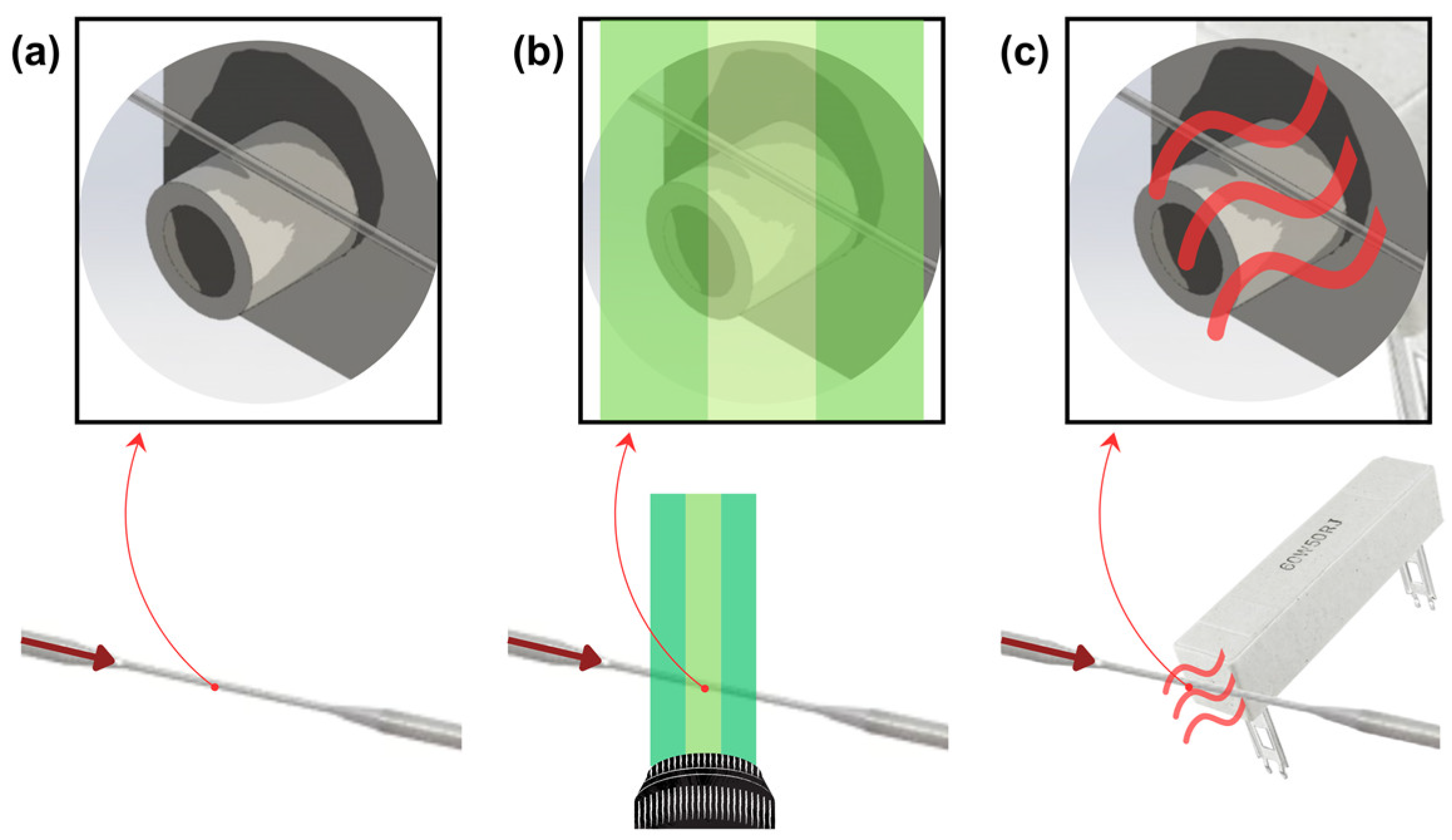
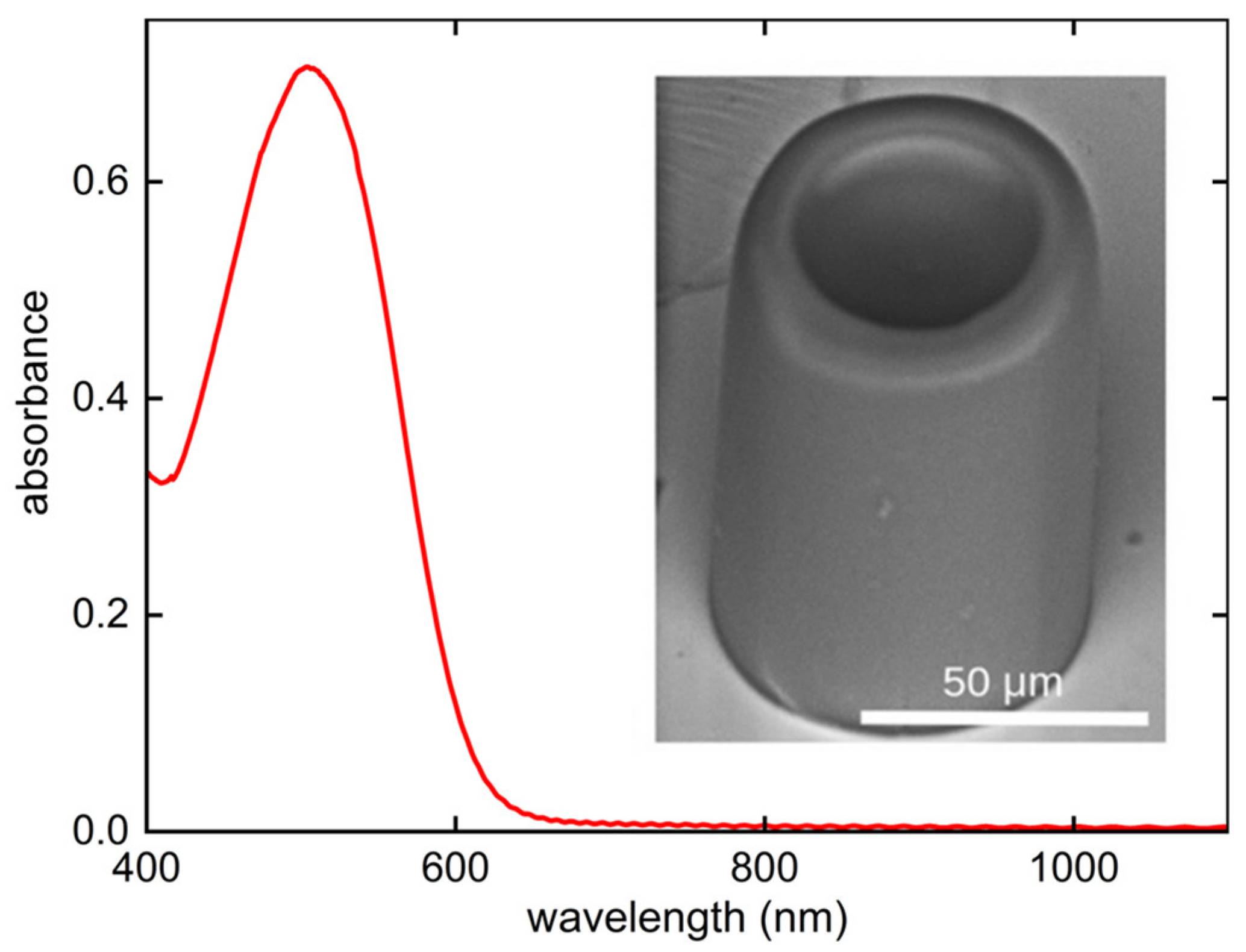
2. Materials and Methods
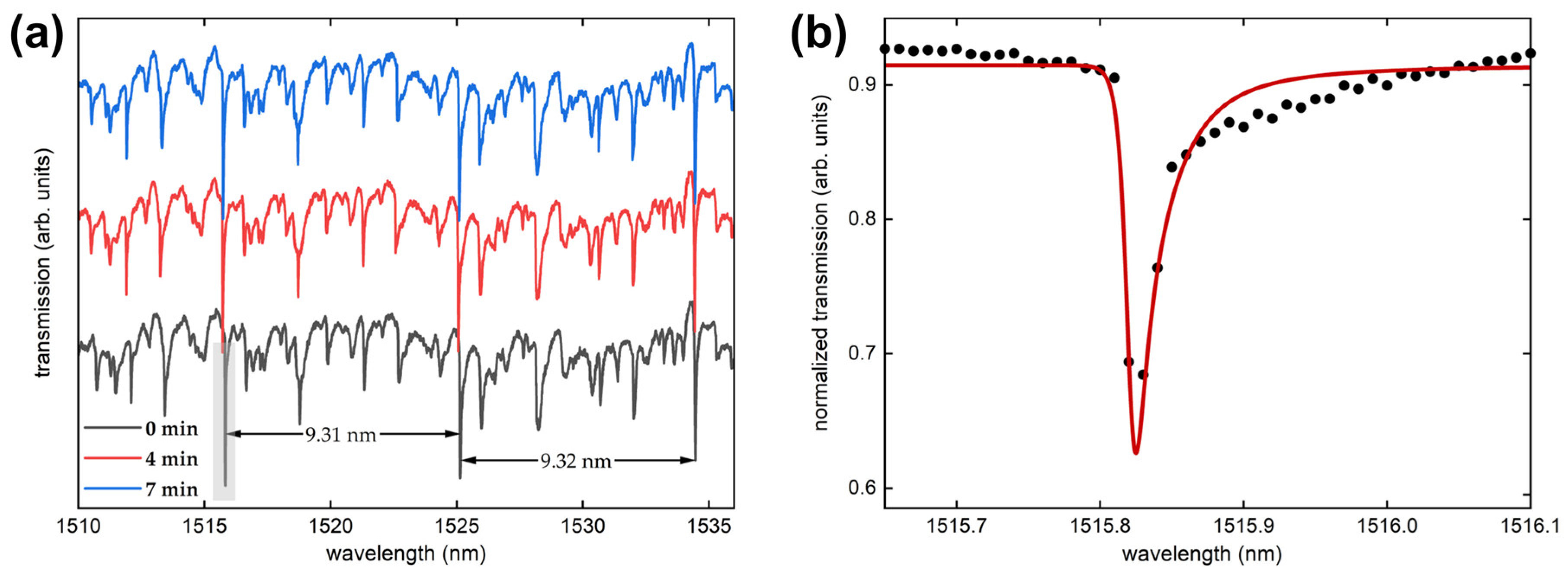
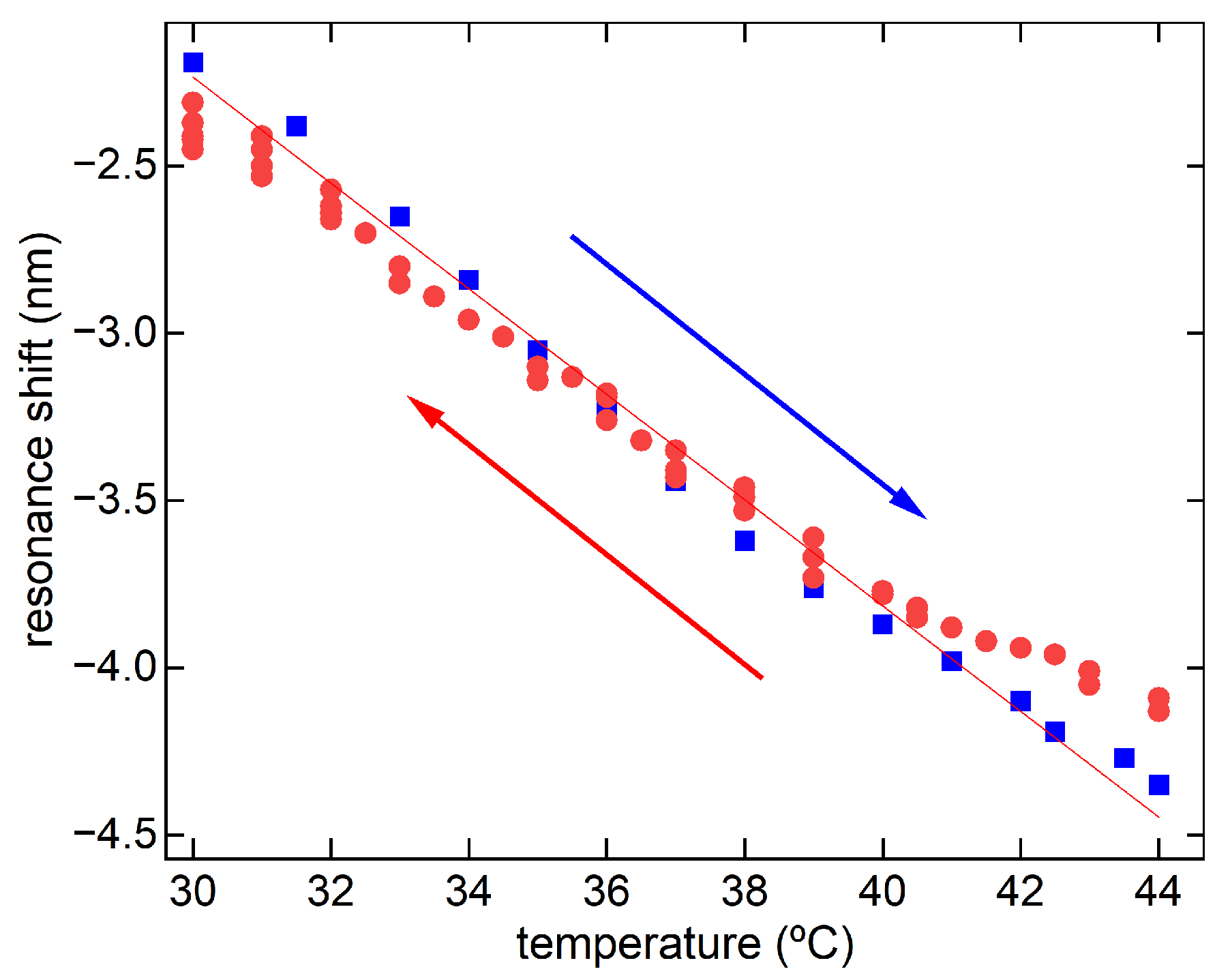
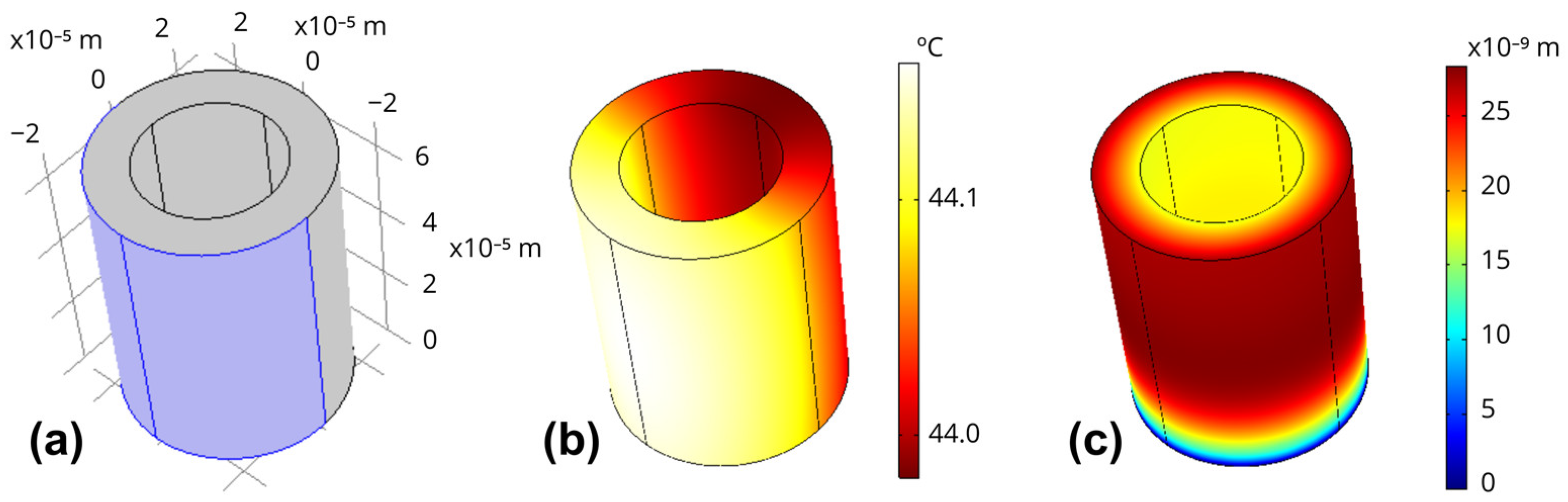
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Malinauskas, M.; Farsari, M.; Piskarskas, A.; Juodkazis, S. Ultrafast laser nanostructuring of photopolymers: A decade of advances. Phys. Rep. 2013, 533, 1–31. [Google Scholar] [CrossRef]
- LaFratta, C.N.; Fourkas, J.T.; Baldacchini, T.; Farrer, R.A. Multiphoton fabrication. Angew. Chem.—Int. Ed. 2007, 46, 6238–6258. [Google Scholar] [CrossRef] [PubMed]
- Maruo, S.; Nakamura, O.; Kawata, S. Three-dimensional microfabrication with two-photon-absorbed photopolymerization. Opt. Lett. 1997, 22, 132–134. [Google Scholar] [CrossRef] [PubMed]
- Kawata, S.; Sun, H.-B.; Tanaka, T.; Takada, K. Finer features for functional microdevices. Nature 2001, 412, 697–698. [Google Scholar] [CrossRef] [PubMed]
- Boyd, R.W. Nonlinear Optics; Elsevier: Amsterdam, The Netherlands, 2020. [Google Scholar] [CrossRef]
- Otuka, A.J.G.; Tomazio, N.B.; Paula, K.T.; Mendonça, C.R. Two-Photon Polymerization: Functionalized Microstructures, Micro-Resonators, and Bio-Scaffolds. Polymers 2021, 13, 1994. [Google Scholar] [CrossRef] [PubMed]
- Sun, H.B.; Kawata, S. Two-photon photopolymerization and 3D lithographic microfabrication. Adv. Polym. Sci. 2004, 170, 169–273. [Google Scholar] [CrossRef]
- Carlotti, M.; Mattoli, V. Functional Materials for Two-Photon Polymerization in Microfabrication. Small 2019, 15, 1902687. [Google Scholar] [CrossRef] [PubMed]
- Dietrich, P.I.; Blaicher, M.; Reuter, I.; Billah, M.; Hoose, T.; Hofmann, A.; Caer, C.; Dangel, R.; Offrein, B.; Troppenz, U.; et al. In situ 3D nanoprinting of free-form coupling elements for hybrid photonic integration. Nat. Photonics 2018, 12, 241–247. [Google Scholar] [CrossRef]
- Tomazio, N.B.; Otuka, A.J.G.; Almeida, G.F.B.; Roselló-Mechó, X.; Andrés, M.V.; Mendonça, C.R. Femtosecond laser fabrication of high-Q whispering gallery mode microresonators via two-photon polymerization. J. Polym. Sci. B Polym. Phys. 2017, 55, 569–574. [Google Scholar] [CrossRef]
- Lindenmann, N.; Dottermusch, S.; Goedecke, M.L.; Hoose, T.; Billah, M.R.; Onanuga, T.P.; Hofmann, A.; Freude, W.; Koos, C. Connecting silicon photonic circuits to multicore fibers by photonic wire bonding. J. Light. Technol. 2015, 33, 755–760. [Google Scholar] [CrossRef]
- Jimenez Gordillo, O.A.; Chaitanya, S.; Chang, Y.-C.; Dave, U.D.; Mohanty, A.; Lipson, M. Plug-and-play fiber to waveguide connector. Opt. Express 2019, 27, 20305. [Google Scholar] [CrossRef] [PubMed]
- Pitts, J.D.; Campagnola, P.J.; Epling, G.A.; Goodman, S.L. Submicron multiphoton free-form fabrication of proteins and polymers: Studies of reaction efficiencies and applications in sustained release. Macromolecules 2000, 33, 1514–1523. [Google Scholar] [CrossRef]
- Tayalia, P.; Mendonca, C.R.; Baldacchini, T.; Mooney, D.J.; Mazur, E. 3D cell-migration studies using two-photon engineered polymer scaffolds. Adv. Mater. 2008, 20, 4494–4498. [Google Scholar] [CrossRef]
- Gittard, S.D.; Ovsianikov, A.; Akar, H.; Chichkov, B.; Monteiro-Riviere, N.A.; Stafslien, S.; Chisholm, B.; Shin, C.-C.; Shih, C.-M.; Lin, S.-J.; et al. Two photon polymerization-micromolding of polyethylene glycol-gentamicin sulfate microneedles. Adv. Eng. Mater. 2010, 12, B77–B82. [Google Scholar] [CrossRef]
- Maruo, S.; Ikuta, K.; Korogi, H. Force-controllable, optically driven micromachines fabricated by single-step two-photon microstereolithography. J. Microelectromechanical Syst. 2003, 12, 533–539. [Google Scholar] [CrossRef]
- Galajda, P.; Ormos, P. Complex micromachines produced and driven by light. Appl. Phys. Lett. 2001, 78, 249–251. [Google Scholar] [CrossRef]
- Von Freymann, G.; Ledermann, A.; Thiel, M.; Staude, I.; Essig, S.; Busch, K.; Wegener, M. Three-dimensional nanostructures for photonics. Adv. Funct. Mater. 2010, 20, 1038–1052. [Google Scholar] [CrossRef]
- Klein, S.; Barsella, A.; Leblond, H.; Bulou, H.; Fort, A.; Andraud, C.; Lemercier, G.; Mulatier, J.C.; Dorkenoo, K. One-step waveguide and optical circuit writing in photopolymerizable materials processed by two-photon absorption. Appl. Phys. Lett. 2005, 86, 211118. [Google Scholar] [CrossRef]
- Lindenmann, N.; Balthasar, G.; Hillerkuss, D.; Schmogrow, R.; Jordan, M.; Leuthold, J.; Freude, W.; Koos, C. Photonic wire bonding: A novel concept for chip-scale interconnects. Opt. Express 2012, 20, 17667. [Google Scholar] [CrossRef]
- Zhang, S.; Tang, S.-J.; Feng, S.; Xiao, Y.-F.; Cui, W.; Wang, X.; Sun, W.; Ye, J.; Han, P.; Zhang, X.; et al. High-Q Polymer Microcavities Integrated on a Multicore Fiber Facet for Vapor Sensing. Adv. Opt. Mater. 2019, 7, 1900602. [Google Scholar] [CrossRef]
- Ams, M.; Marshall, G.D.; Dekker, P.; Piper, J.A.; Withford, M.J. Ultrafast laser written active devices. Laser Photonics Rev. 2009, 3, 535–544. [Google Scholar] [CrossRef]
- Tomazio, N.B.; De Boni, L.; Mendonca, C.R. Low threshold Rhodamine-doped whispering gallery mode microlasers fabricated by direct laser writing. Sci. Rep. 2017, 7, 8559. [Google Scholar] [CrossRef]
- Li, L.; Gershgoren, E.; Kumi, G.; Chen, W.-Y.; Ho, P.-T.; Herman, W.N.; Fourkas, J.T. High-performance microring resonators fabricated with multiphoton absorption polymerization. Adv. Mater. 2008, 20, 3668–3671. [Google Scholar] [CrossRef]
- Liu, Z.P.; Li, Y.; Xiao, Y.-F.; Li, B.-B.; Jiang, X.-F.; Qin, Y.; Feng, X.-B.; Yang, H.; Gong, Q. Direct laser writing of whispering gallery microcavities by two-photon polymerization. Appl. Phys. Lett. 2010, 97, 211105. [Google Scholar] [CrossRef]
- Grossmann, T.; Schleede, S.; Hauser, M.; Beck, T.; Thiel, M.; von Freymann, G.; Mappes, T.; Kalt, H. Direct laser writing for active and passive high-Q polymer microdisks on silicon. Opt. Express 2011, 19, 11451. [Google Scholar] [CrossRef] [PubMed]
- Tomazio, N.B.; Paula, K.T.; Henrique, F.R.; Andrade, M.B.; Roselló-Mechó, X.; Delgado-Pinar, M.; Andrés, M.V.; Mendonca, C.R. Mode cleaning in graphene oxide-doped polymeric whispering gallery mode microresonators. J. Mater. Chem. C Mater. 2020, 8, 9707–9713. [Google Scholar] [CrossRef]
- Pöllinger, M.; O’Shea, D.; Warken, F.; Rauschenbeutel, A. Ultrahigh-Q Tunable Whispering-Gallery-Mode Microresonator. Phys. Rev. Lett. 2009, 103, 053901. [Google Scholar] [CrossRef]
- Savchenkov, A.A.; Ilchenko, V.S.; Matsko, A.B.; Maleki, L. Tunable filter based on whispering gallery modes. Electron. Lett. 2003, 39, 389. [Google Scholar] [CrossRef]
- Ioppolo, T.; Ayaz, U.; Ötügen, M.V. Tuning of whispering gallery modes of spherical resonators using an external electric field. Opt. Express 2009, 17, 16465. [Google Scholar] [CrossRef] [PubMed]
- Adolphson, M.; Qavi, A.J.; Shmuylovich, L.; Liao, J.; Amarasinghe, G.K.; Yang, L. Reverse tuning of whispering gallery mode microresonators. In Optics and Biophotonics in Low-Resource Settings IX; Levitz, D., Ozcan, A., Eds.; SPIE: Bellingham, WA, USA, 2023; p. 38. [Google Scholar] [CrossRef]
- Wang, Z.; Mallik, A.K.; Wei, F.; Wang, Z.; Rout, A.; Wu, Q.; Semenova, Y. Enhancing the thermo-optic tuning performance of whispering gallery modes in a microcapillary resonator filled with nematic liquid crystal. Opt. Commun. 2023, 537, 129442. [Google Scholar] [CrossRef]
- Kovach, A.; He, J.; Saris, P.J.G.; Chen, D.; Armani, A.M. Optically tunable microresonator using an azobenzene monolayer. AIP Adv. 2020, 10, 045117. [Google Scholar] [CrossRef]
- Weber, M.J. Handbook of Optical Materials; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar] [CrossRef]
- Barreda, A.; Zou, C.; Sinelnik, A.; Menshikov, E.; Sinev, I.; Pertsch, T.; Staude, I. Tuning and switching effects of quasi-BIC states combining phase change materials with all-dielectric metasurfaces. Opt. Mater Express 2022, 12, 3132. [Google Scholar] [CrossRef]
- Izdebskaya, Y.V.; Yang, Z.; Shvedov, V.G.; Neshev, D.N.; Shadrivov, I.V. Multifunctional Metasurface Tuning by Liquid Crystals in Three Dimensions. Nano Lett. 2023, 23, 9825–9831. [Google Scholar] [CrossRef] [PubMed]
- Izdebskaya, Y.V.; Yang, Z.; Liu, M.; Choi, D.-Y.; Komar, A.; Neshev, D.N.; Shadrivov, I.V. Magnetic tuning of liquid crystal dielectric metasurfaces. Nanophotonics 2022, 11, 3895–3900. [Google Scholar] [CrossRef]
- Birks, T.A.; Li, Y.W. The shape of fiber tapers. J. Light. Technol. 1992, 10, 432–438. [Google Scholar] [CrossRef]
- Arkema Sartomer Americas. SR399. Available online: https://americas.sartomer.arkema.com/en/product-finders/product/f/sartomer_MonomerAcrylates_US/p/sr399/ (accessed on 31 January 2024).
- Derkowska-Zielinska, B.; Krupka, O.; Smokal, V.; Grabowski, A.; Naparty, M.; Skowronski, L. Optical properties of disperse dyes doped poly(methyl methacrylate). Mol. Cryst. Liq. Cryst. 2016, 639, 87–93. [Google Scholar] [CrossRef]
- Couto, F.A.; Andrade, M.B.; Otuka, A.J.G.; Pratavieira, S.; Muniz, S.R.; Mendonça, C.R. Integrating Fluorescent Nanodiamonds into Polymeric Microstructures Fabricated by Two-Photon Polymerization. Nanomaterials 2023, 13, 2571. [Google Scholar] [CrossRef] [PubMed]
- Carmon, T.; Yang, L.; Vahala, K.J. Dynamical thermal behavior and thermal self-stability of microcavities. Opt. Express 2004, 12, 4742. [Google Scholar] [CrossRef]
- Dong, C.-H.; He, L.; Xiao, Y.-F.; Gaddam, V.R.; Ozdemir, S.K.; Han, Z.-F.; Guo, G.-C.; Yang, L. Fabrication of high-Q polydimethylsiloxane optical microspheres for thermal sensing. Appl. Phys. Lett. 2009, 94, 231119. [Google Scholar] [CrossRef]
- Deng, Y.; Liu, F.; Leseman, Z.C.; Hossein-Zadeh, M. Thermo-optomechanical oscillator for sensing applications. Opt. Express 2013, 21, 4653. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Veluthandath, A.V.; Murugan, G.S.; Bisht, P.B. Temperature dependence of whispering gallery modes of quantum dot-doped microbottle resonators. J. Lumin. 2020, 221, 117050. [Google Scholar] [CrossRef]
- Goods, S. Thermal Expansion and Hydration Behavior of PMMA Moulding Materials for LIGA Applications; Sandia National Lab.: Albuquerque, NM, USA; Livermore, CA, USA, 2003. [Google Scholar] [CrossRef]
- Bednarski, H.; Hajduk, B. Dispersion of thermo-optic coefficient of optical polymers. Polymer 2021, 230, 124074. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jorge, G.H.A.; Couto, F.A.; Almeida, J.M.P.; Marques, V.A.S.; Andrade, M.B.; Mendonça, C.R. Active Optical Tuning of Azopolymeric Whispering Gallery Mode Microresonators for Filter Applications. Photonics 2024, 11, 167. https://doi.org/10.3390/photonics11020167
Jorge GHA, Couto FA, Almeida JMP, Marques VAS, Andrade MB, Mendonça CR. Active Optical Tuning of Azopolymeric Whispering Gallery Mode Microresonators for Filter Applications. Photonics. 2024; 11(2):167. https://doi.org/10.3390/photonics11020167
Chicago/Turabian StyleJorge, Gabriel H. A., Filipe A. Couto, Juliana M. P. Almeida, Victor A. S. Marques, Marcelo B. Andrade, and Cleber R. Mendonça. 2024. "Active Optical Tuning of Azopolymeric Whispering Gallery Mode Microresonators for Filter Applications" Photonics 11, no. 2: 167. https://doi.org/10.3390/photonics11020167
APA StyleJorge, G. H. A., Couto, F. A., Almeida, J. M. P., Marques, V. A. S., Andrade, M. B., & Mendonça, C. R. (2024). Active Optical Tuning of Azopolymeric Whispering Gallery Mode Microresonators for Filter Applications. Photonics, 11(2), 167. https://doi.org/10.3390/photonics11020167





