Effect of Polarization on Cross-Spectral Density Matrix
Abstract
1. Introduction
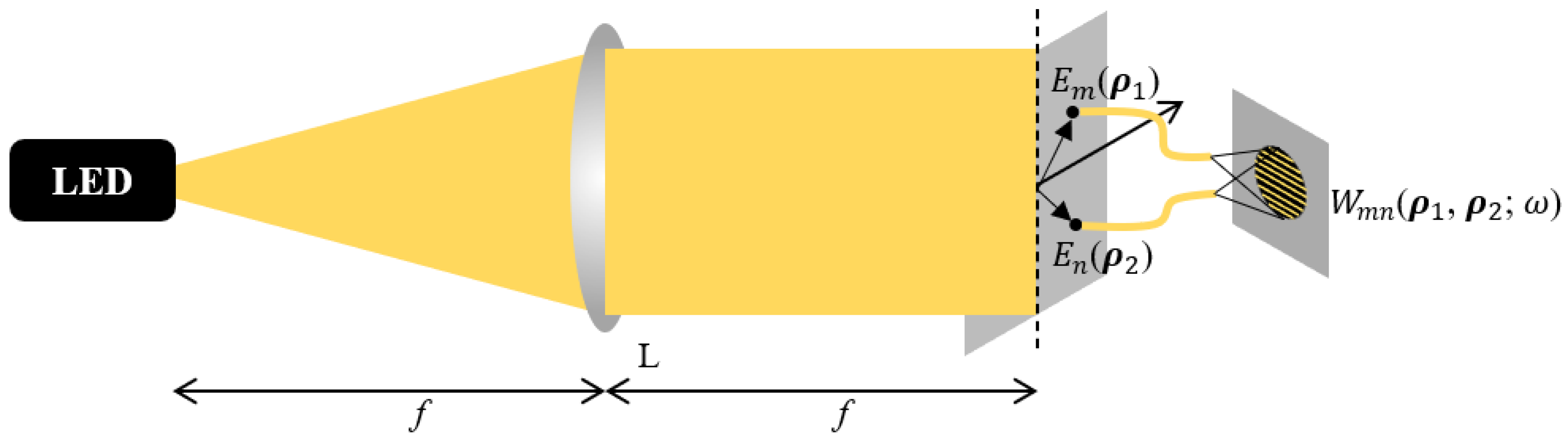
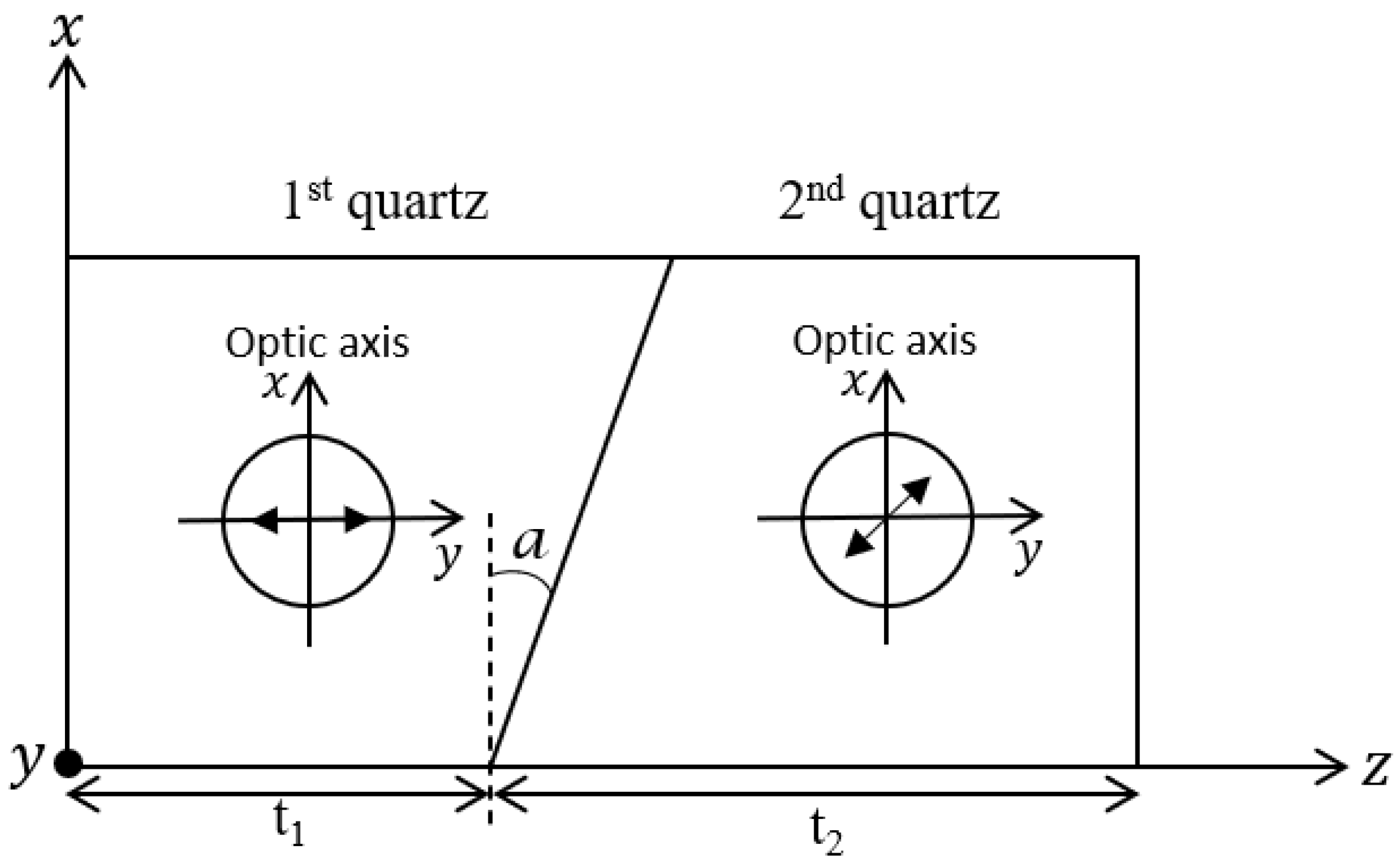
2. Principle
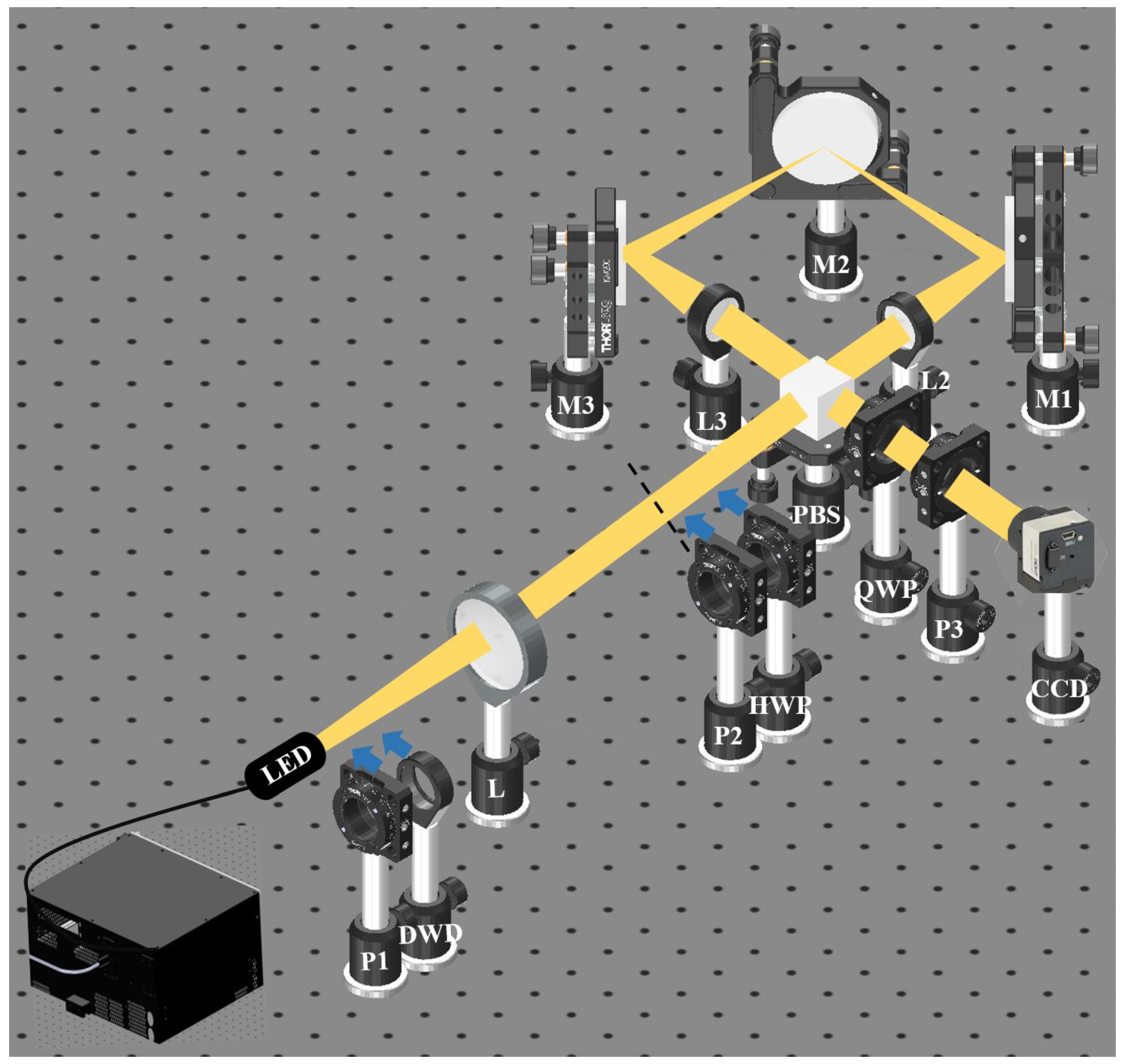
3. Experimental Setup and Description
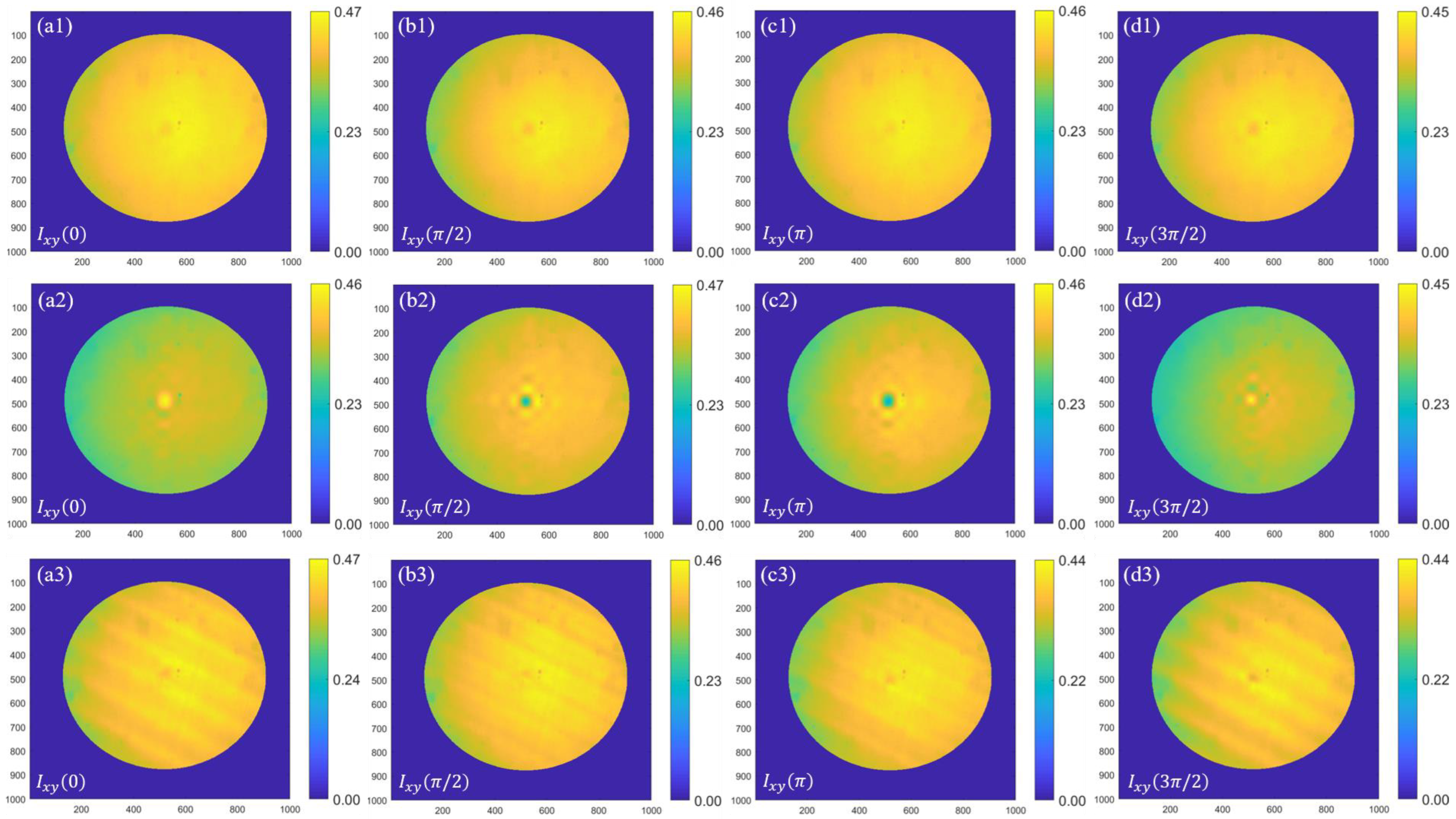
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rubinsztein-Dunlop, H.; Forbes, A.; Berry, M.V.; Dennis, M.R.; Andrews, D.L.; Mansuripur, M.; Denz, C.; Alpmann, C.; Banzer, P.; Bauer, T.; et al. Roadmap on structured light. J. Opt. 2016, 19, 013001. [Google Scholar] [CrossRef]
- Forbes, A.; de Oliveira, M.; Dennis, M.R. Structured light. Nat. Photonics 2021, 15, 253–262. [Google Scholar] [CrossRef]
- Korotkova, O.; Gbur, G. Applications of Optical Coherence Theory. Prog. Opt. 2020, 65, 43–104. [Google Scholar]
- Forbes, A.; Dudley, A.; McLaren, M. Creation and detection of optical modes with spatial light modulators. Adv. Opt. Photonics 2016, 8, 200–227. [Google Scholar] [CrossRef]
- Ren, Y.X.; Lu, R.D.; Gong, L. Tailoring light with a digital micromirror device. Ann. Phys. 2015, 527, 447–470. [Google Scholar] [CrossRef]
- Yu, N.; Capasso, F. Flat optics with designer metasurfaces. Nat. Mater. 2014, 13, 139–150. [Google Scholar] [CrossRef] [PubMed]
- Keren-Zur, S.; Michaeli, L.; Suchowski, H.; Ellenbogen, T. Shaping light with nonlinear metasurfaces. Adv. Opt. Photonics 2018, 10, 309–353. [Google Scholar] [CrossRef]
- Rumand, A.; Cardano, F.; Piccirillo, B.; Marrucci, L. Q-plate technology: A progress review. J. Opt. Soc. Am. B 2019, 36, D70–D87. [Google Scholar]
- Forbes, A. Structured light from lasers. Laser Photonics Rev. 2019, 13, 1900140. [Google Scholar] [CrossRef]
- Andrews, L.C.; Phillips, R.L. Laser Beam Propagation through Random Media; SPIE: Bellingham, DC, USA, 2005. [Google Scholar]
- Goodman, J.W. Speckle Phenomena in Optics: Theory and Applications; Roberts and Company Publishers: Greenwood Village, CO, USA, 2007. [Google Scholar]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Chen, Y.; Wang, F.; Zhao, C.; Cai, Y. Experimental demonstration of a Laguerre-Gaussian correlated Schell-model vortex beam. Opt. Express 2014, 22, 5826–5838. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.-F.; Zhou, Y.; Wu, H.-W.; Chen, H.-J.; Sheng, Z.-Q.; Qu, J. Focus shaping of the radially polarized Laguerre-Gaussian-correlated Schell-model vortex beams. Opt. Express 2018, 26, 20076–20088. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Yao, M.; Qiu, Z.; Yi, X.; Liu, Z. Evolution properties of Bessel-Gaussian Schell-model beams in non-Kolmogorov turbulence. Opt. Express 2015, 23, 12508–12523. [Google Scholar] [CrossRef]
- Peng, X.; Lu, X.; Liu, X.; Zhao, C.; Lin, R.; Liu, L.; Cai, Y. Generation and propagation of a Hermite-Gaussian correlated Schell-model LG0l Beam. Appl. Sci. 2019, 9, 610. [Google Scholar] [CrossRef]
- Korotkova, O.; Sahin, S.; Shchepakina, E. Multi-Gaussian Schell-model beams. J. Opt. Soc. Am. A 2012, 29, 2159–2164. [Google Scholar] [CrossRef] [PubMed]
- Cai, Y.; Chen, Y.; Wang, F. Generation and propagation of partially coherent beams with nonconventional correlation functions: A review. J. Opt. Soc. Am. A 2014, 31, 2083–2096. [Google Scholar] [CrossRef] [PubMed]
- Cui, S.; Chen, Z.; Zhang, L.; Pu, J. Experimental generation of nonuniformly correlated partially coherent light beams. Opt. Lett. 2013, 38, 4821–4824. [Google Scholar] [CrossRef] [PubMed]
- Takeda, M.; Wang, W.; Duan, Z.; Miyamoto, Y. Coherence holography. Opt. Express 2005, 13, 9629–9635. [Google Scholar] [CrossRef]
- Naik, D.N.; Singh, R.K.; Ezawa, T.; Miyamoto, Y.; Takeda, M. Photon correlation holography. Opt. Express 2011, 19, 1408–1421. [Google Scholar] [CrossRef]
- Wang, F.; Liu, X.; Yuan, Y.; Cai, Y. Experimental generation of partially coherent beams with different complex degrees of coherence. Opt. Lett. 2013, 38, 1814–1816. [Google Scholar] [CrossRef]
- Santarsiero, M.; Martínez-Herrero, R.; Maluenda, D.; De Sande, J.C.; Piquero, G.; Gori, F. Partially coherent sources with circular coherence. Opt. Lett. 2017, 42, 1512–1515. [Google Scholar] [CrossRef]
- Singh, R.K.; Sharma, A.M.; Senthilkumaran, P. Vortex array embedded in a partially coherent beam. Opt. Lett. 2015, 40, 2751–2754. [Google Scholar] [CrossRef]
- Mei, Z.; Korotkova, O.; Zhao, D.; Mao, Y. Self-focusing vortex beams. Opt. Lett. 2021, 46, 2384–2387. [Google Scholar] [CrossRef]
- Wolf, E. Introduction to the Theory of Coherence and Polarization of Light; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Wolf, E. Unified theory of coherence and polarization of random electromagnetic beams. Phys. Lett. A 2003, 312, 263–267. [Google Scholar] [CrossRef]
- Friberg, A.T.; Setälä, T. Electromagnetic theory of optical coherence (invited). J. Opt. Soc. Am. A 2016, 33, 2431–2442. [Google Scholar] [CrossRef]
- James, D.F.V. Changes of polarization of light beams on propagation in free space. J. Opt. Soc. Am. A 1994, 11, 1641–1643. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Wolf, E. Propagation-induced polarization changes in partially coherent optical beams. J. Opt. Soc. Am. A 2000, 17, 2019–2023. [Google Scholar] [CrossRef] [PubMed]
- Brown, D.P.; Brown, T.G. Partially correlated azimuthal vortex illumination: Coherence and correlation measurements and effects in imaging. Opt. Express 2008, 16, 20418–20426. [Google Scholar] [CrossRef] [PubMed]
- Lerman, G.M.; Levy, U. Effect of radial polarization and apodization on spot size under tight focusing conditions. Opt. Express 2008, 16, 4567–4581. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Guo, H.; Li, J.; Ling, L.; Feng, B.; Li, Z.Y. Optical trapping of gold nanoparticles by cylindrical vector beam. Opt. Lett. 2012, 37, 1694–1696. [Google Scholar] [CrossRef] [PubMed]
- Gataric, M.; Gordon, G.S.; Renna, F.; Ramos, A.G.; Alcolea, M.P.; Bohndiek, S.E. Reconstruction of optical vector-fields with applications in endoscopic imaging. IEEE Trans. Med. Imaging 2018, 38, 955–967. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.G.; Kihm, H.W.; Kihm, J.E.; Choi, W.J.; Kim, H.; Ropers, C.; Park, D.J.; Yoon, Y.C.; Choi, S.B.; Woo, D.H.; et al. Vector field microscopic imaging of light. Nat. Photonics 2007, 1, 53–56. [Google Scholar] [CrossRef]
- Bautista, G.; Kauranen, M. Vector-field nonlinear microscopy of nanostructures. ACS Photonics 2016, 3, 1351–1370. [Google Scholar] [CrossRef]
- Ouyang, J.; Perrie, W.; Allegre, O.J.; Heil, T.; Jin, Y.; Fearon, E.; Eckford, D.; Edwardson, S.P.; Dearden, G. Tailored optical vector fields for ultrashort-pulse laser induced complex surface plasmon structuring. Opt. Express 2015, 23, 12562–12572. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Huang, Y.; Chen, Y.; Guo, L.; Cai, Y. Orbital angular moment of an electromagnetic Gaussian Schell-model beam with a twist phase. Opt. Express 2015, 23, 30283–30296. [Google Scholar] [CrossRef]
- Korotkova, O.; Chen, X.; Setälä, T. Electromagnetic Schell-model beams with arbitrary complex correlation states. Opt. Lett. 2019, 44, 4945–4948. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Wang, F.; Liu, L.; Zhao, C.; Cai, Y.; Korotkova, O. Generation and propagation of a partially coherent vector beam with special correlation functions. Phys. Rev. A 2014, 89, 013801. [Google Scholar] [CrossRef]
- Singh, R.K.; Naik, D.N.; Itou, H.; Miyamoto, Y.; Takeda, M. Vectorial coherence holography. Opt. Express 2011, 19, 11558–11567. [Google Scholar] [CrossRef] [PubMed]
- Mishra, S.; Gautam, S.K.; Naik, D.N.; Chen, Z.; Pu, J.; Singh, R.K. Tailoring and analysis of vectorial coherence. J. Opt. 2018, 20, 125605. [Google Scholar] [CrossRef]
- Shirai, T.; Wolf, E. Coherence and polarization of electromagnetic beams modulated by random phase screens and their changes on propagation in free space. J. Opt. Soc. Am. A 2004, 21, 1907–1916. [Google Scholar] [CrossRef]
- Liu, Y.; Dong, Z.; Wang, F.; Cai, Y.; Chen, Y. Experimental synthesis of higher-order Poincaré sphere beam array with spatial coherence engineering. Appl. Phys. Lett. 2023, 122, 161106. [Google Scholar] [CrossRef]
- Chen, L.; Chen, Z.; Singh, R.K.; Pu, J. Imaging of polarimetric-phase object through scattering medium by phase shifting. Opt. Express 2020, 28, 8145–8155. [Google Scholar] [CrossRef]
- Kanseri, B.; Kandpal, H.C. Experimental determination of electric cross-spectral density matrix and generalized Stokes parameters for a laser beam. Opt. Lett. 2008, 33, 2410–2412. [Google Scholar] [CrossRef]
- Partanen, H.; Hoenders, B.J.; Friberg, A.T.; Setälä, T. Young’s interference experiment with electromagnetic narrowband light. J. Opt. Soc. Am. A 2018, 35, 1379–1384. [Google Scholar] [CrossRef]
- Lu, X.; Shao, Y.; Zhao, C.; Konijnenberg, S.; Zhu, X.; Tang, Y.; Cai, Y.; Urbach, H.P. Noniterative spatially partially coherent diffractive imaging using pinhole array mask. Adv. Photonics 2019, 1, 016005. [Google Scholar] [CrossRef]
- Turunen, J.; Halder, A.; Koivurova, M.; Setälä, T. Measurement of spatial coherence of light [Invited]. J. Opt. Soc. Am. A 2022, 39, C214–C239. [Google Scholar] [CrossRef]
- Hassinen, T.; Tervo, J.; Setälä, T.; Friberg, A.T. Hanbury Brown–Twiss effect with electromagnetic waves. Opt. Express 2011, 19, 15188–15195. [Google Scholar] [CrossRef] [PubMed]
- Singh, R.K.; Sharma, M.A. Recovery of complex valued objects from two-point intensity correlation measurement. Appl. Phys. Lett. 2014, 104, 111108. [Google Scholar] [CrossRef]
- Vinu, R.V.; Singh, R.K. Experimental determination of generalized Stokes parameters. Opt. Lett. 2015, 40, 1227–1230. [Google Scholar] [CrossRef] [PubMed]
- Yoneda, N.; Quan, X.; Matoba, O. Single-shot generalized Hanbury Brown–Twiss experiments using a polarization camera for target intensity reconstruction in scattering media. Opt. Lett. 2023, 48, 632–635. [Google Scholar] [CrossRef]
- Dong, Z.; Huang, Z.; Chen, Y.; Wang, F.; Cai, Y. Measuring complex correlation matrix of partially coherent vector light via a generalized Hanbury Brown–Twiss experiment. Opt. Express 2020, 28, 20634–20644. [Google Scholar] [CrossRef]
- Wang, Z.; Lu, X.; Huang, W.; Konijnenberg, A.P.; Zhang, H.; Zhao, C.; Cai, Y. Measuring the complete complex correlation matrix of a partially coherent vector beam via self-referencing holography. Appl. Phys. Lett. 2021, 119, 111101. [Google Scholar] [CrossRef]
- Gautam, A.; Athira, T.S.; Naik, D.N.; Singh, R.; Narayanamurthy, C.S.; Singh, R.K. Recording of incoherent vector holograms using elements of the spatial cross-spectral density matrix. Opt. Lasers Eng. 2023, 169, 107687. [Google Scholar] [CrossRef]
- Chandra, S.; Gautam, A.; Singh, R.K. Folded interferometer to measure coherence-polarization matrix. Opt. Lett. 2023, in press. [Google Scholar] [CrossRef]
- Tervo, J.; Turunen, J. Transverse and longitudinal periodicities in fields produced by polarization gratings. Opt. Commun. 2001, 190, 51–57. [Google Scholar] [CrossRef]
- De Sande, J.C.G.; Santarsiero, M.; Piquero, G.; Gori, F. Longitudinal polarization periodicity of unpolarized light passing through a double wedge depolarizer. Opt. Express 2012, 20, 27348–27360. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Singh, R.K.; Naik, D.N.; Itou, H.; Miyamoto, Y.; Takeda, M. Stokes holography. Opt. Lett. 2012, 37, 966–968. [Google Scholar] [CrossRef] [PubMed]
- Tervo, J. Azimuthal polarization and partial coherence. J. Opt. Soc. Am. A 2003, 20, 1974–1980. [Google Scholar] [CrossRef] [PubMed]
- Piquero, G.; Vargas-Balbuena, J. Non-uniformly polarized beams across their transverse profiles: An introductory study for undergraduate optics courses. Eur. J. Phys. 2004, 25, 793. [Google Scholar] [CrossRef]
- Beckley, A.M.; Brown, T.G.; Alonso, M.A. Full Poincaré beams. Opt. Express 2010, 18, 10777–10785. [Google Scholar] [CrossRef]
- Chandra, S.; Singh, R.; Singh, R.K. Poincare vector correlations to estimate polarization dynamics in the laser speckle. Phys. Scr. 2023, 98, 065504. [Google Scholar] [CrossRef]
- Korotkova, O.; Wolf, E. Changes in the state of polarization of a random electromagnetic beam on propagation. Opt. Commun. 2005, 246, 35–43. [Google Scholar] [CrossRef]
- Sarkar, T.; Chandra, S.; Singh, R.K. Phase recovery with intensity and polarization correlation. Prog. Opt. 2023, 68, 101–190. [Google Scholar]
- De Sande, J.C.G.; Piquero, G.; Santarsiero, M.; Gori, F. Partially coherent electromagnetic beams propagating through double-wedge depolarizers. J. Opt. 2014, 16, 035708. [Google Scholar] [CrossRef]
- Santarsiero, M.; De Sande, J.C.G.; Piquero, G.; Gori, F. Coherence–polarization properties of fields radiated from transversely periodic electromagnetic sources. J. Opt. 2013, 15, 055701. [Google Scholar] [CrossRef]
- Partanen, H.; Friberg, A.T.; Setälä, T.; Turunen, J. Spectral measurement of coherence Stokes parameters of random broadband light beams. Photonics Res. 2019, 7, 669–677. [Google Scholar] [CrossRef]
- Helen, S.S.; Kothiyal, M.P.; Sirohi, R.S. Achromatic phase shifting by a rotating polarizer. Opt. Commun. 1998, 154, 249–254. [Google Scholar] [CrossRef]
- Gori, F.; Ramírez-Sánchez, V.; Santarsiero, M.; Shirai, T. On genuine cross-spectral density matrices. J. Opt. A Pure Appl. Opt. 2009, 11, 085706. [Google Scholar] [CrossRef]
- Singh, R.K.; Naik, D.N.; Itou, H.; Brundabanam, M.M.; Miyamoto, Y.; Takeda, M. Vectorial van Cittert–Zernike theorem based on spatial averaging: Experimental demonstrations. Opt. Lett. 2013, 38, 4809–4812. [Google Scholar] [CrossRef] [PubMed]
- Gerrard, A.; Burch, J.M. Introduction to Matrix Methods in Optics; Courier Corporation: Chelmsford, MA, USA, 1994. [Google Scholar]
- Roy, M.; Svahn, P.; Cherel, L.; Sheppard, C.J.R. Geometric phase-shifting for low-coherence interference microscopy. Opt. Lasers Eng. 2002, 37, 631–641. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gautam, A.; Naik, D.N.; Narayanamurthy, C.S.; Singh, R.K. Effect of Polarization on Cross-Spectral Density Matrix. Photonics 2024, 11, 142. https://doi.org/10.3390/photonics11020142
Gautam A, Naik DN, Narayanamurthy CS, Singh RK. Effect of Polarization on Cross-Spectral Density Matrix. Photonics. 2024; 11(2):142. https://doi.org/10.3390/photonics11020142
Chicago/Turabian StyleGautam, Akanksha, Dinesh N. Naik, C. S. Narayanamurthy, and Rakesh Kumar Singh. 2024. "Effect of Polarization on Cross-Spectral Density Matrix" Photonics 11, no. 2: 142. https://doi.org/10.3390/photonics11020142
APA StyleGautam, A., Naik, D. N., Narayanamurthy, C. S., & Singh, R. K. (2024). Effect of Polarization on Cross-Spectral Density Matrix. Photonics, 11(2), 142. https://doi.org/10.3390/photonics11020142




