Scalable Optical Frequency Rulers with the Faraday Effect
Abstract
1. Introduction
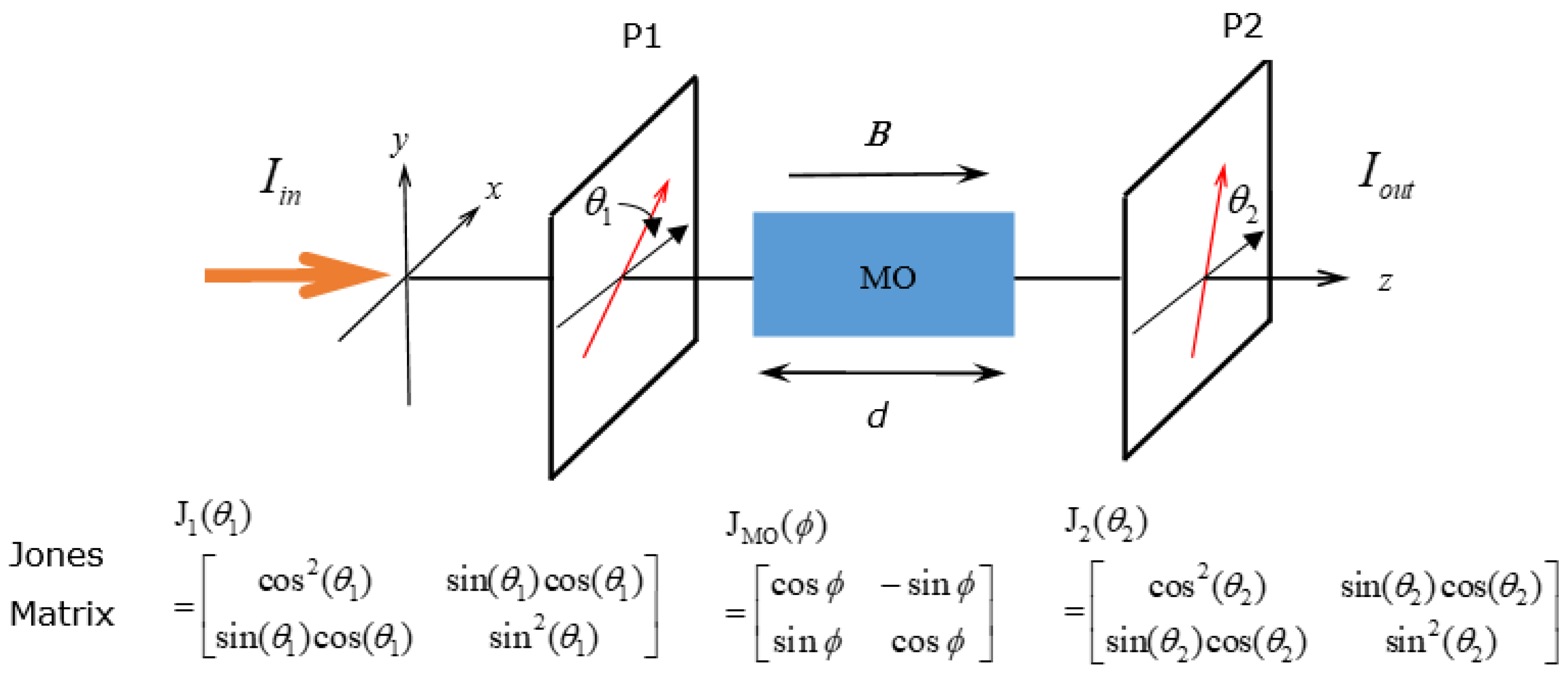
2. Theory and Numerical Results
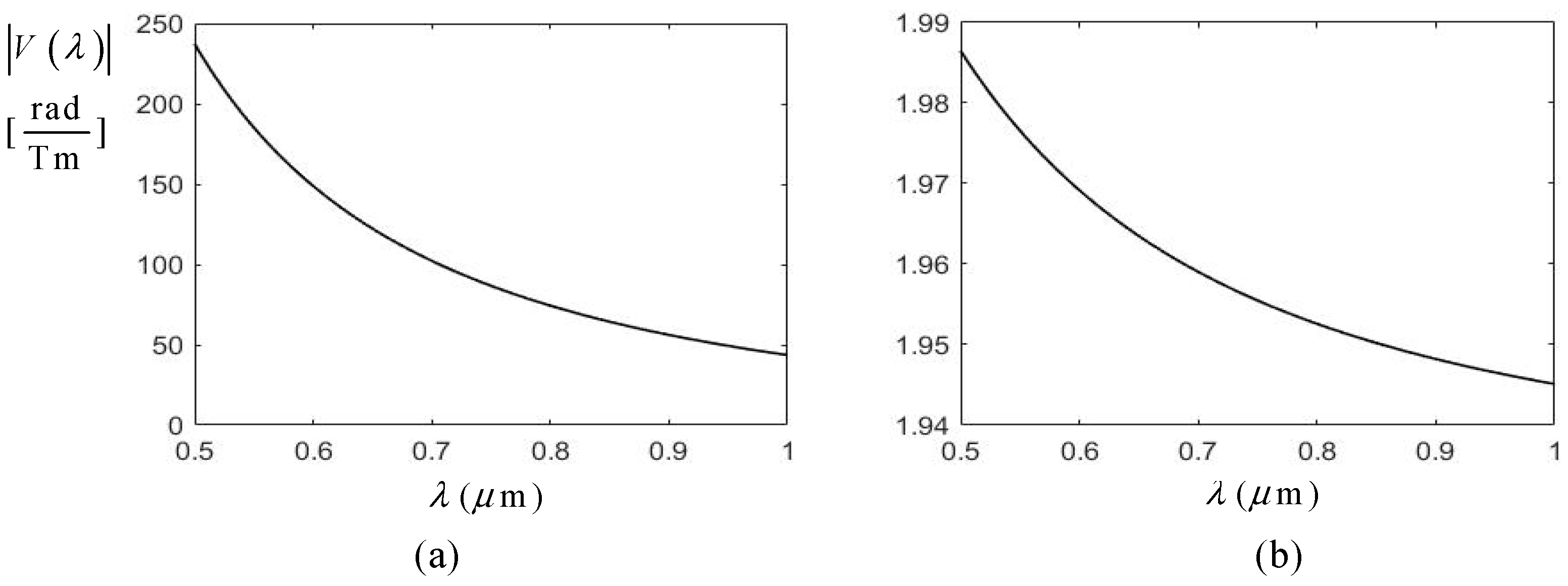
2.1. Monochromatic Wave Situation
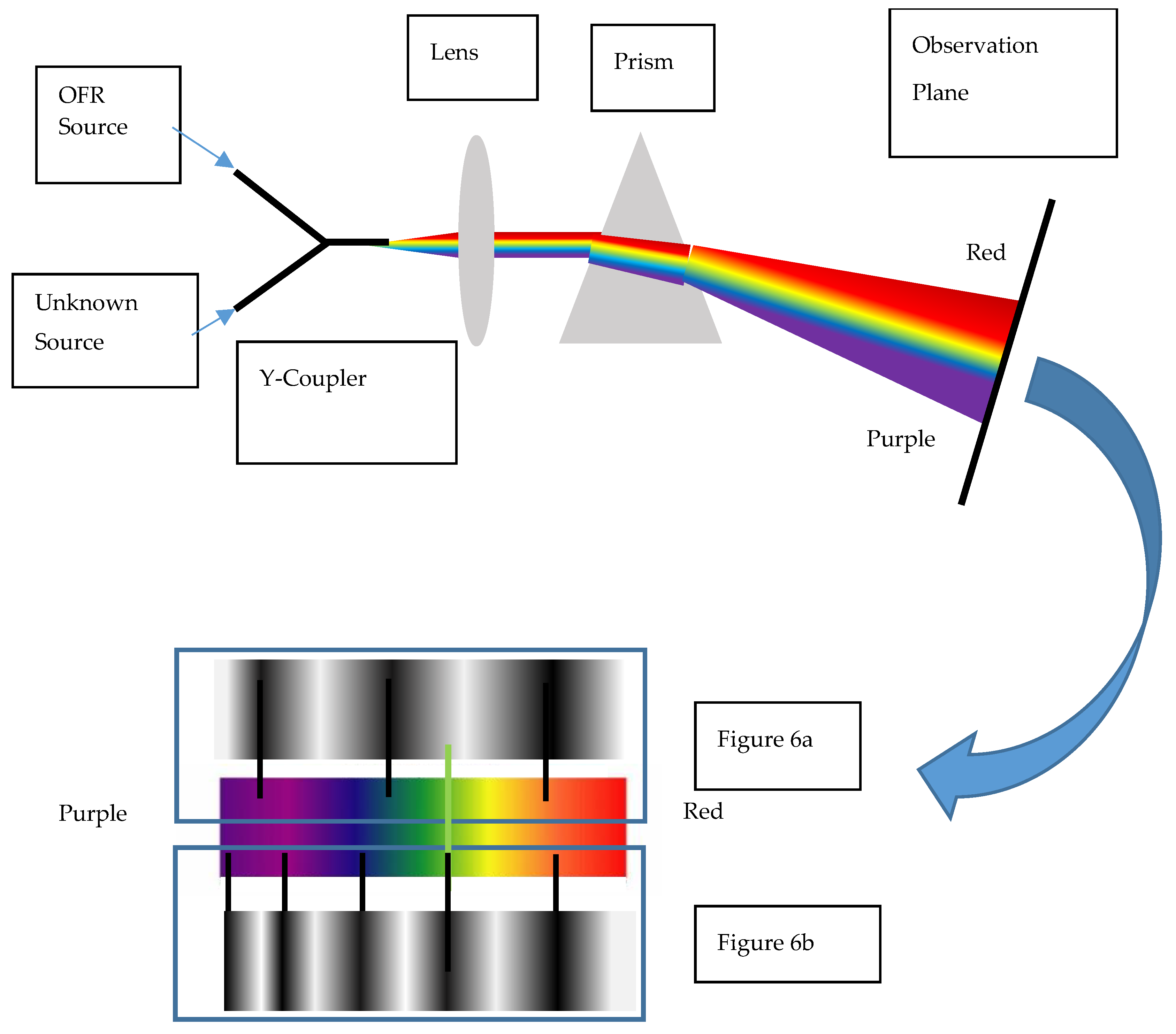
2.2. Polychromatic Wave Situation
3. Results
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wolf, E. Invariance of the spectrum of light on propagation. Phys. Rev. Lett. 1986, 56, 1370–1372. [Google Scholar] [CrossRef] [PubMed]
- Wolf, E. Red shifts and blue shifts of spectral lines emitted by two correlated sources. Phys. Rev. Lett. 1987, 58, 2646–2648. [Google Scholar] [CrossRef] [PubMed]
- Visser, T.D.; Wolf, E. Spectral anomalies near phase singularities in partially coherent focused wavefields. J. Opt. A Pure Appl. Opt. 2003, 5, 371–373. [Google Scholar] [CrossRef]
- Soskin, M.S.; Vasnetov, M.V. Singular optics. In Progress in Optics; Wolf, E., Ed.; Elsevier: Amsterdam, The Netherlands, 2001; Volume 42, pp. 219–276. [Google Scholar]
- Han, P. Spatial–Spectral Correspondence Relationship for Mono—Poly chromatic Light Diffraction. In Progress in Optics; Visser, T.D., Ed.; Elsevier: Amsterdam, The Netherlands, 2018; Volume 63, pp. 33–87. [Google Scholar]
- Chang, J.P.; Weng, J.H.; Hsu, H.C.; Lee, P.Y.; Han, P. A Data Transmission Method with Spectral Switches via Electroabsorption. Appl. Sci. 2022, 12, 979. [Google Scholar] [CrossRef]
- Ding, P.; Pu, J.; Weng, J.; Han, P. Spectral anomalies by superposition of polychromatic Gaussian beam and Gaussian vortex beam. Opt. Express 2014, 22, 213037. [Google Scholar] [CrossRef] [PubMed]
- Foley, J.T.; Wolf, E. Phenomenon of spectral switches as a new effect in singular optics with polychromatic light. J. Opt. Soc. Am. A 2002, 19, 2510–2516. [Google Scholar] [CrossRef] [PubMed]
- Rasouli, S.; Khazaei, A.M.; Hebri, D. Talbot carpet at the transverseplane produced in the diffraction of plane wave from amplituderadial gratings. J. Opt. Soc. Am. A 2018, 35, 55–64. [Google Scholar] [CrossRef] [PubMed]
- Born, M.; Wolf, E. Principles of Optics, 7th ed.; Cambridge University: Cambridge, UK, 1999; p. 587. [Google Scholar]
- Bartels, A.; Heinecke, D.; Diddams, S.A. 10-GHz self-referenced optical frequency comb. Science 2009, 326, 681. [Google Scholar] [CrossRef] [PubMed]
- Kuhn, K.J. Laser Engineering; Prentice-Hall Inc.: Hoboken, NJ, USA, 1998; Chapter 3. [Google Scholar]
- Han, P. Optical frequency ruler with moving fluid. Chin. Opt. Lett. 2013, 11, 122601. [Google Scholar] [CrossRef]
- Kanseri, B.; Rathi, A.K. Broadband spectral shaping using nematic liquid crystal. Results Phys. 2019, 12, 531–534. [Google Scholar] [CrossRef]
- Ding, P.F.; Hsu, H.C.; Han, P. Spectral manipulation and tunable optical frequency ruler using liquid crystal’s birefringence. OPTIK 2019, 179, 115–121. [Google Scholar] [CrossRef]
- Tsai, C.M.; Weng, J.H.; Lin, K.W.; Han, P. Movable optical frequency ruler with optical activity. Appl. Sci. 2023, 10, 206. [Google Scholar] [CrossRef]
- Mihailovic, P.; Petricevic, S. Fiber Optic Sensors Based on the Faraday Effect. Sensors 2021, 21, 6564. [Google Scholar] [CrossRef] [PubMed]
- Yoshino, T. Theory for the Faraday effect in optical fiber. J. Opt. Soc. Am. B 2005, 22, 1856–1860. [Google Scholar] [CrossRef]
- Teich, M.; Saleh, B. Fundamentals of Photonics, 2nd ed.; Wiley: Hoboken, NJ, USA, 2007; pp. 228–230. [Google Scholar]
- Iizuka, K. Elements of Photonics; Wiley: Hoboken, NJ, USA, 2002; Volume 1, p. 421. [Google Scholar]
- Slezak, O.; Yasuhara, R.; Lucianetti, A.; Mocek, T. Wavelength dependence of magneto-optic properties of terbium gallium garnet ceramics. Opt. Express 2015, 23, 013641. [Google Scholar] [CrossRef] [PubMed]
- Vojna, D.; Slezak, O.; Lucianetti, A.; Mocek, T. Verdet constant of magneto-active materials developed for high-power Faraday devices. Appl. Sci. 2019, 9, 3160. [Google Scholar] [CrossRef]
- Schlarb, U.; Sugg, B. Refractive index of Terbium Gallium Garnet. Phys. Stat. Sol. 1994, 182, K91. [Google Scholar] [CrossRef]
- Jones, R.C. New calcules for the treatment of optical systems. J. Opt. Soc. Am. 1947, 37, 107–110. [Google Scholar] [CrossRef]
- Gil, J.J. Characteristic properties of Mueller matrices. J. Opt. Soc. Am. A 2000, 17, 328–334. [Google Scholar] [CrossRef] [PubMed]
- Gil, J.J.; Ossikovski, R. Polarized Light and the Mueller Matrix Approach; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Glushkov, A.V. Optical Communication Systems: Signal Processing and Cybersecurity; Astroprint: San Diego, CA, USA, 2011. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, J.-P.; Tsai, C.-M.; Ding, P.; Pu, J.; Han, P. Scalable Optical Frequency Rulers with the Faraday Effect. Photonics 2024, 11, 127. https://doi.org/10.3390/photonics11020127
Chang J-P, Tsai C-M, Ding P, Pu J, Han P. Scalable Optical Frequency Rulers with the Faraday Effect. Photonics. 2024; 11(2):127. https://doi.org/10.3390/photonics11020127
Chicago/Turabian StyleChang, Jyun-Ping, Cheng-Mu Tsai, Panfeng Ding, Jixiong Pu, and Pin Han. 2024. "Scalable Optical Frequency Rulers with the Faraday Effect" Photonics 11, no. 2: 127. https://doi.org/10.3390/photonics11020127
APA StyleChang, J.-P., Tsai, C.-M., Ding, P., Pu, J., & Han, P. (2024). Scalable Optical Frequency Rulers with the Faraday Effect. Photonics, 11(2), 127. https://doi.org/10.3390/photonics11020127






