Design of High-Q-Gradient Dielectric Nanoparticle Chain Surface Plasmonic Cavities
Abstract
1. Introduction
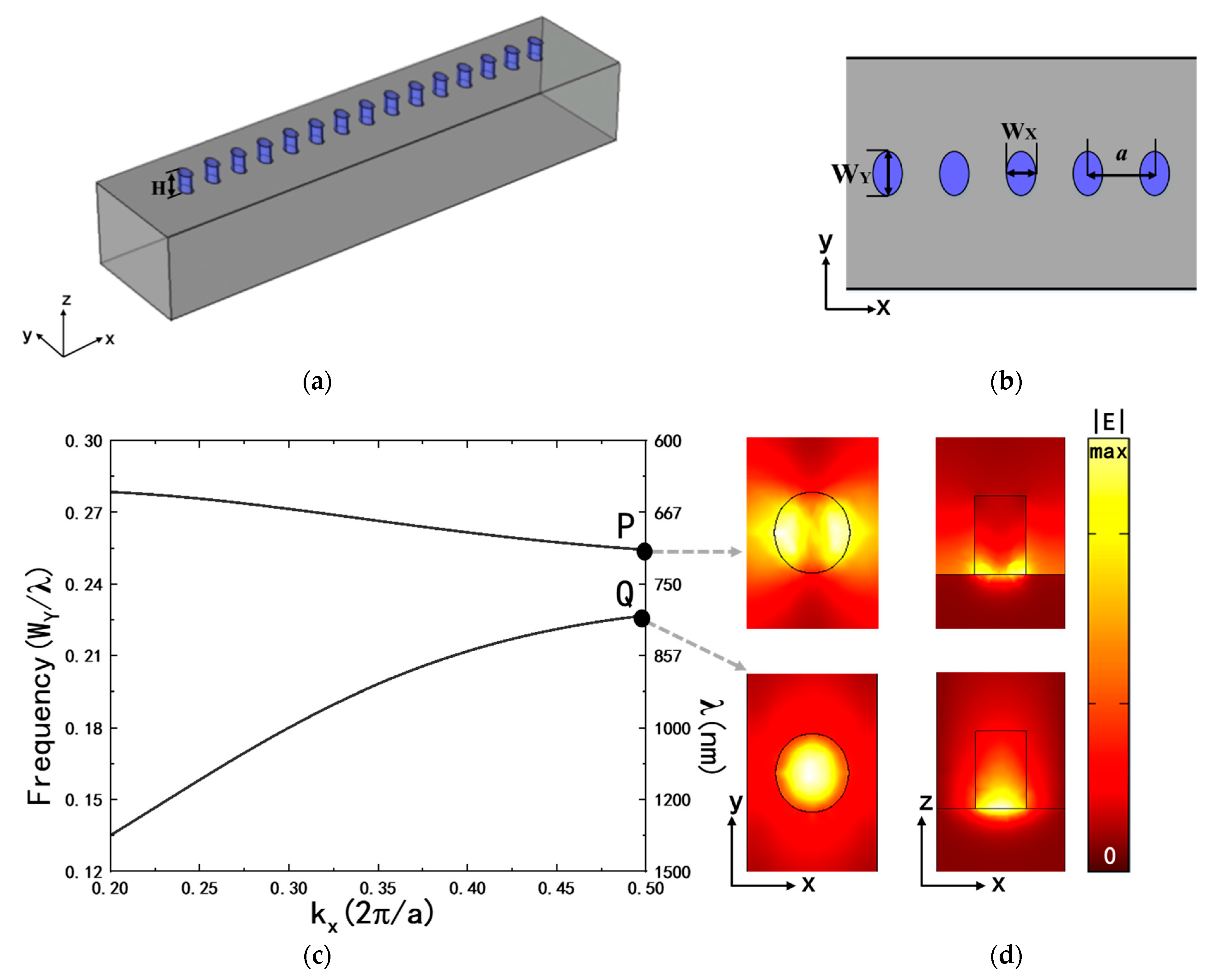
2. Band Edge Mode of Periodic Nanoparticle Chain Structure
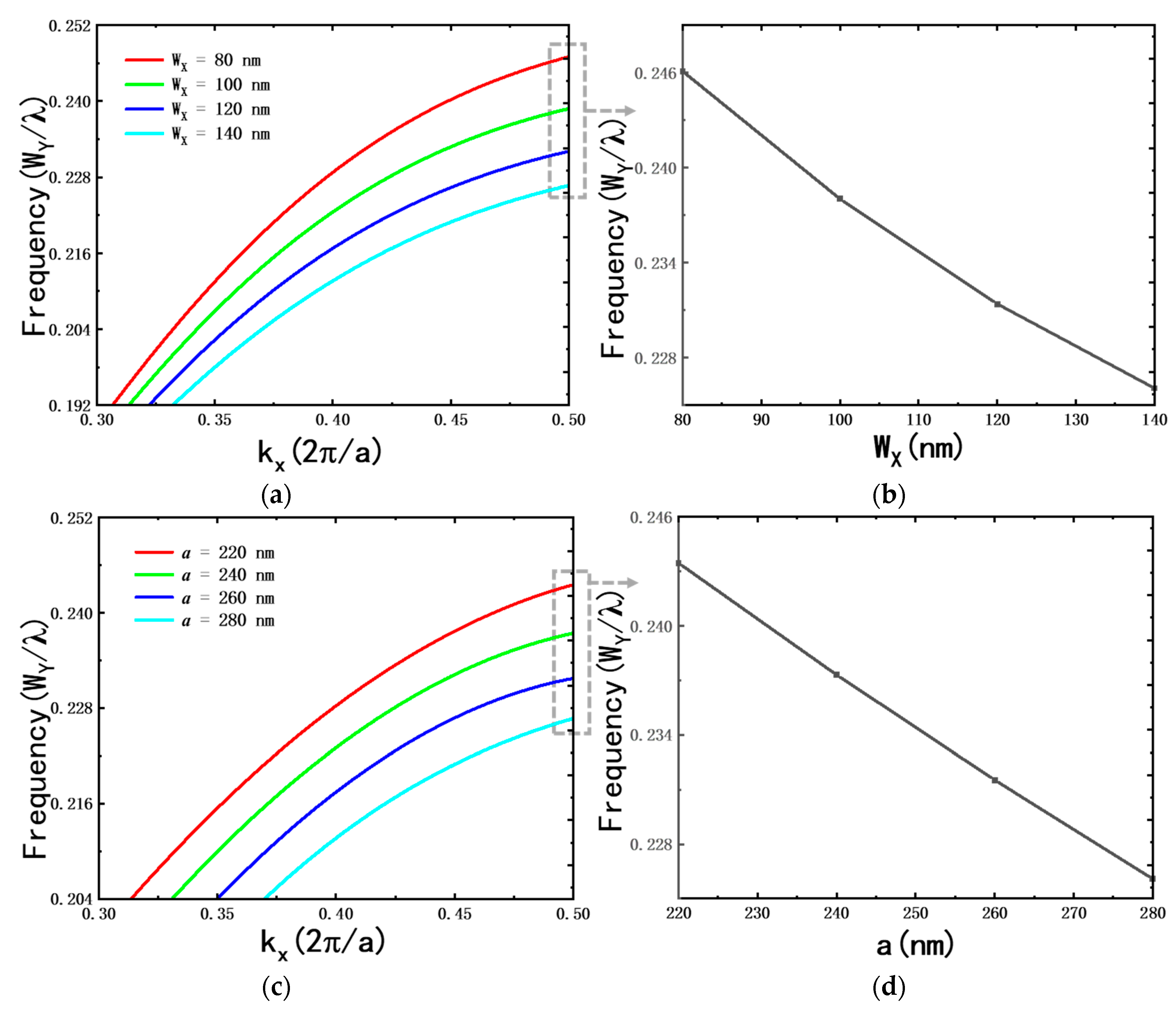
3. Three Surface Plasmon Trap Cavities Made by Engineering Particle Sizes
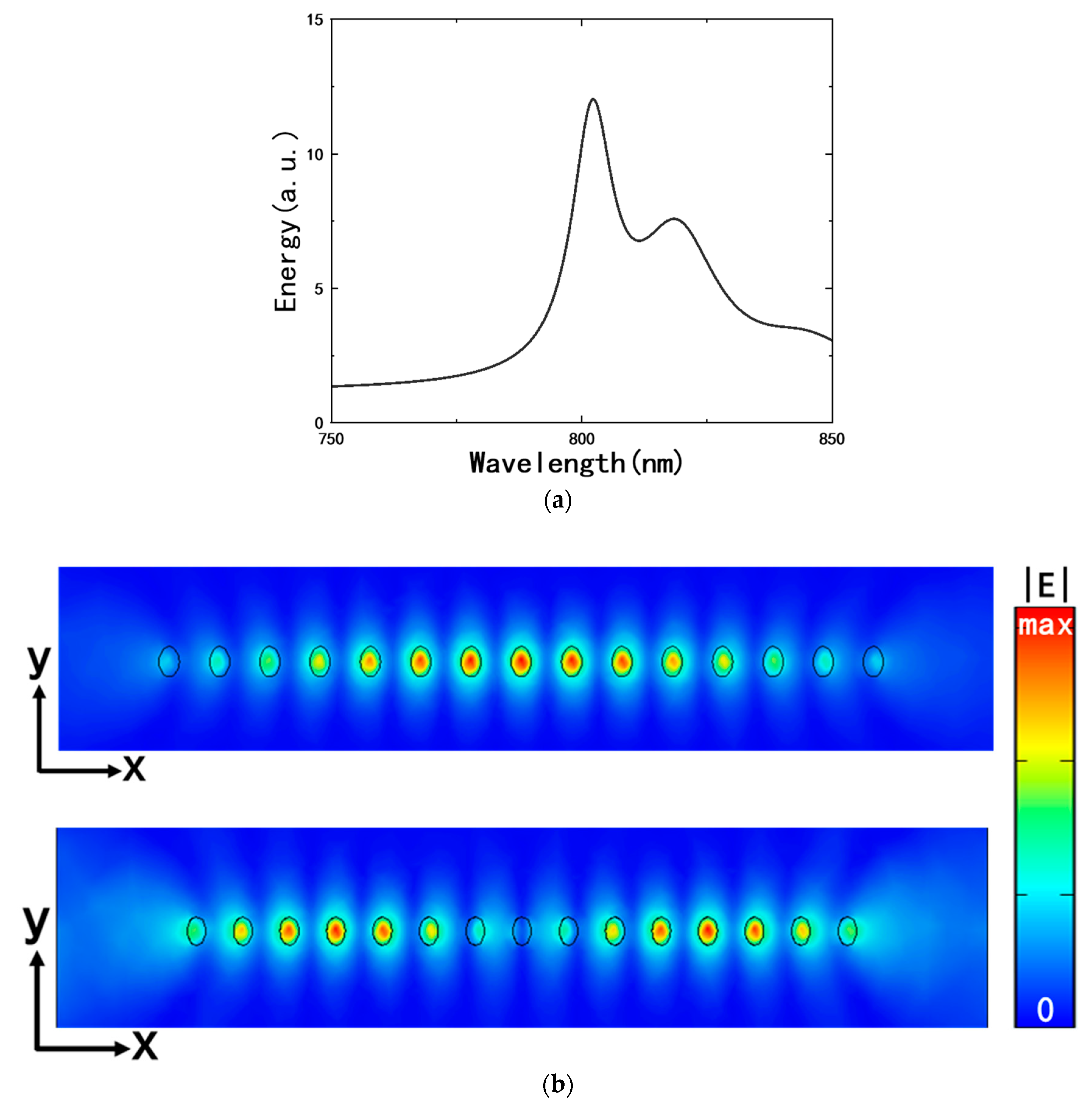
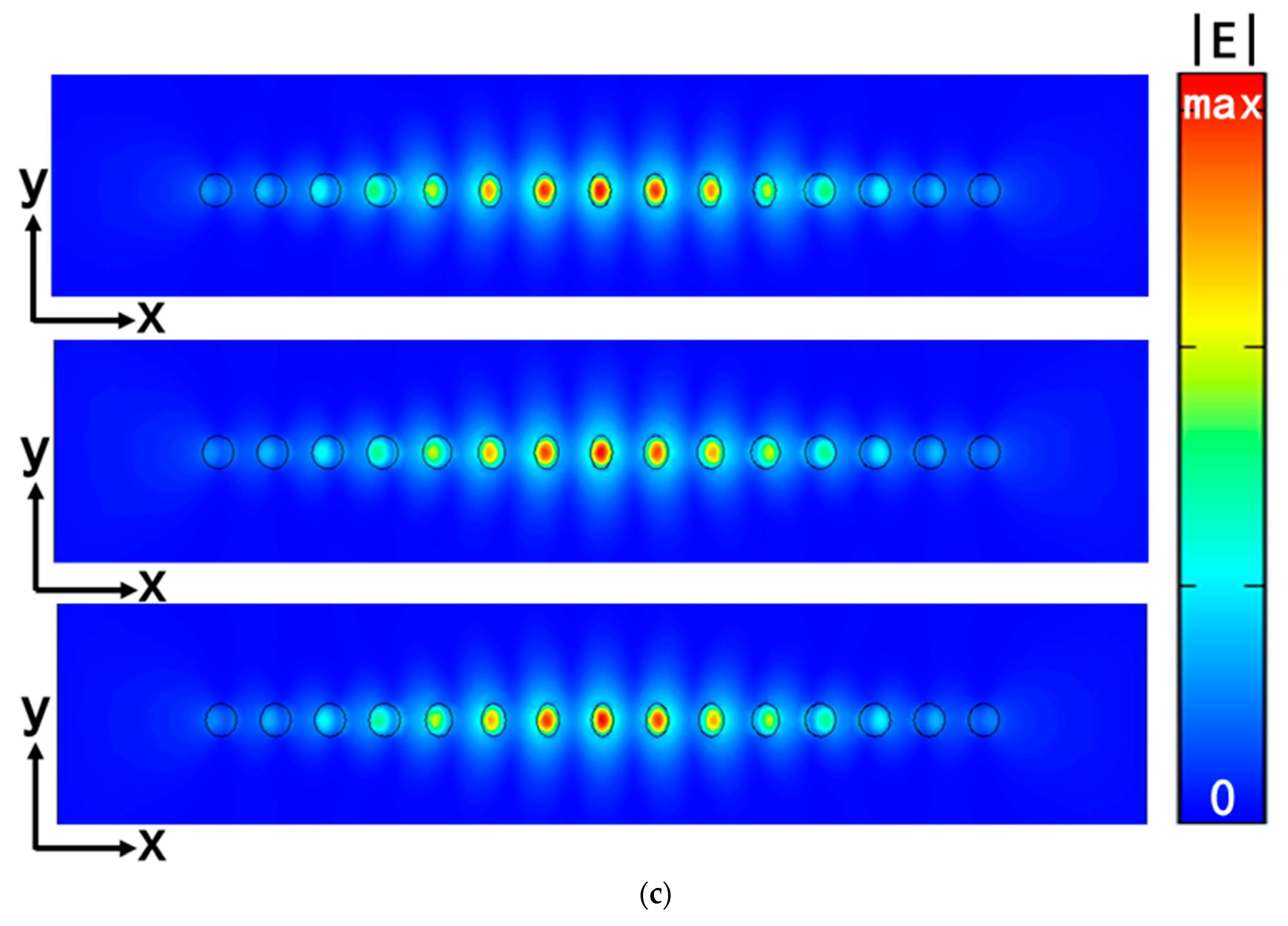
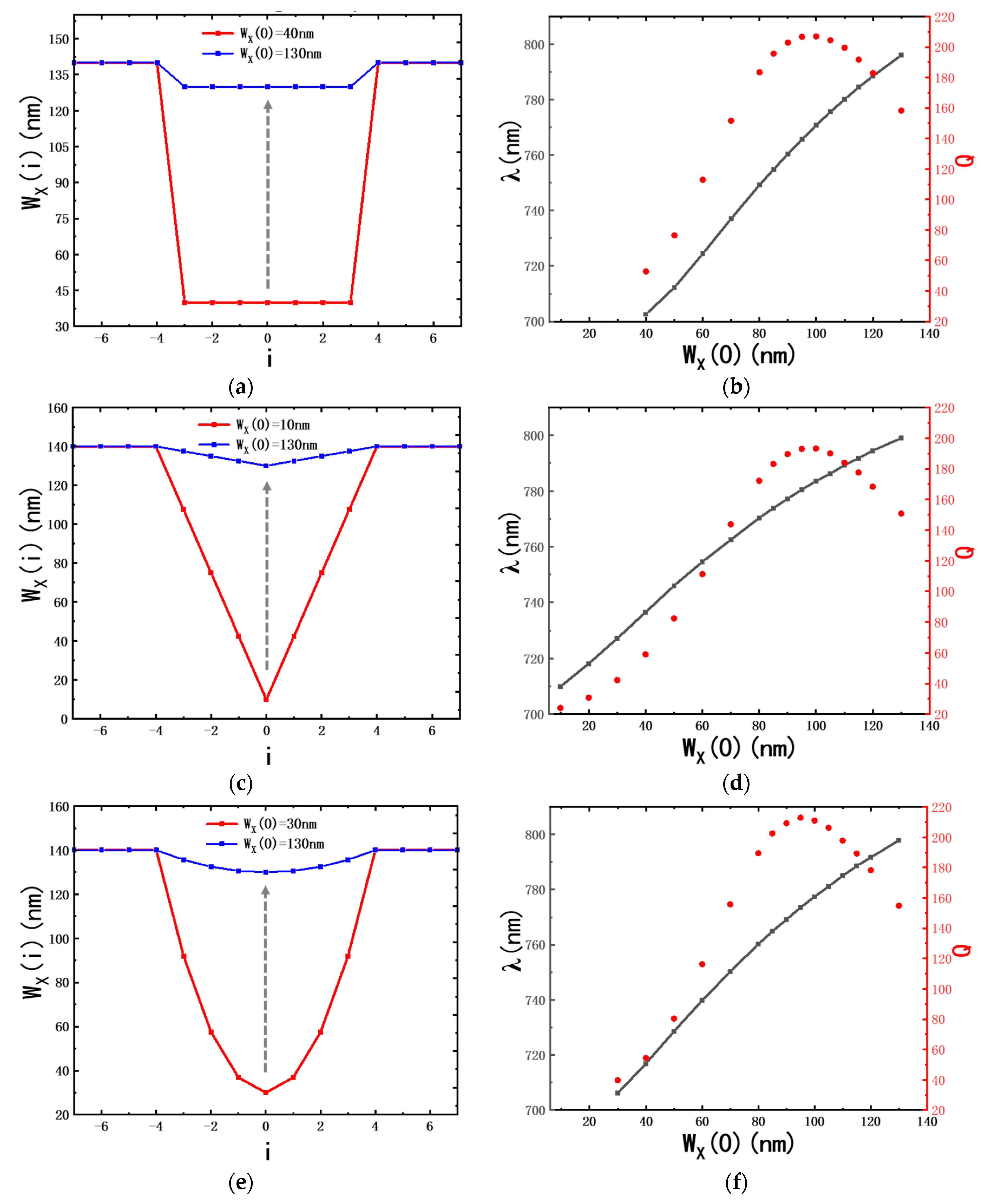
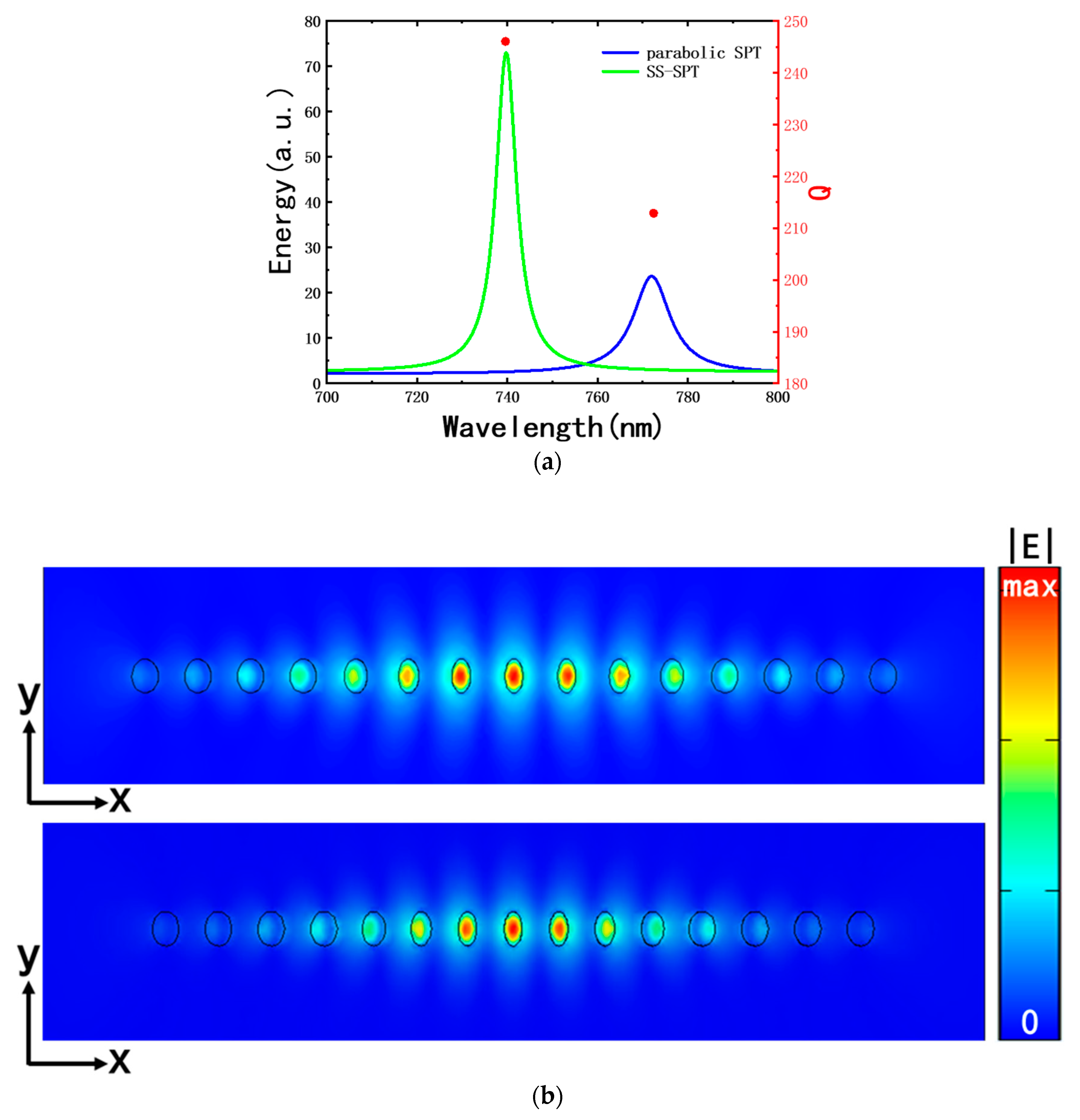
4. Size-gradient and Spacing-gradient Surface Plasmon Trap Cavity
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bergman, D.J.; Stockman, M.I. Surface Plasmon Amplification by Stimulated Emission of Radiation: Quantum Generation of Coherent Surface Plasmons in Nanosystems. Phys. Rev. Lett. 2003, 90, 027402. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zhao, B.; Min, C.; Zhang, Y.; Yang, J.; Guo, C.; Yuan, X. Research progress of femtosecond surface plasmon polariton. Chin. Phys. B 2020, 29, 027302. [Google Scholar] [CrossRef]
- Berini, P.; De Leon, I. Surface plasmon–polariton amplifiers and lasers. Nat. Photonics 2012, 6, 16–24. [Google Scholar] [CrossRef]
- Noginov, M.A.; Zhu, G.; Belgrave, A.M.; Bakker, R.; Shalaev, V.M.; Narimanov, E.E.; Stout, S.; Herz, E.; Suteewong, T.; Wiesner, U. Demonstration of a spaser-based nanolaser. Nature 2009, 460, 1110–1112. [Google Scholar] [CrossRef] [PubMed]
- Meng, X.; Kildishev, A.V.; Fujita, K.; Tanaka, K.; Shalaev, V.M. Wavelength-Tunable Spasing in the Visible. Nano Lett. 2013, 13, 4106–4112. [Google Scholar] [CrossRef]
- Zhou, W.; Dridi, M.; Suh, J.Y.; Kim, C.H.; Co, D.T.; Wasielewski, M.R.; Schatz, G.C.; Odom, T.W. Lasing action in strongly coupled plasmonic nanocavity arrays. Nat. Nanotechnol. 2013, 8, 506–511. [Google Scholar] [CrossRef]
- Wang, D.; Bourgeois, M.R.; Lee, W.-K.; Li, R.; Trivedi, D.; Knudson, M.P.; Wang, W.; Schatz, G.C.; Odom, T.W. Stretchable Nanolasing from Hybrid Quadrupole Plasmons. Nano Lett. 2018, 18, 4549–4555. [Google Scholar] [CrossRef]
- Yang, A.; Hoang, T.B.; Dridi, M.; Deeb, C.; Mikkelsen, M.H.; Schatz, G.C.; Odom, T.W. Real-time tunable lasing from plasmonic nanocavity arrays. Nat. Commun. 2015, 6, 6939. [Google Scholar] [CrossRef] [PubMed]
- Huang, Z.-T.; Yin, C.-W.; Hong, Y.-H.; Li, H.; Hong, K.-B.; Kao, T.S.; Shih, M.-H.; Lu, T.-C. Hybrid Plasmonic Surface Lattice Resonance Perovskite Lasers on Silver Nanoparticle Arrays. Adv. Opt. Mater. 2021, 9, 2100299. [Google Scholar] [CrossRef]
- Wang, J.; Jia, X.; Wang, Z.; Liu, W.; Zhu, X.; Huang, Z.; Yu, H.; Yang, Q.; Sun, Y.; Wang, Z.; et al. Ultrafast plasmonic lasing from a metal/semiconductor interface. Nanoscale 2020, 12, 16403–16408. [Google Scholar] [CrossRef] [PubMed]
- Tao, T.; Zhi, T.; Liu, B.; Dai, J.; Zhuang, Z.; Xie, Z.; Chen, P.; Ren, F.; Chen, D.; Zheng, Y.; et al. Manipulable and Hybridized, Ultralow-Threshold Lasing in a Plasmonic Laser Using Elliptical InGaN/GaN Nanorods. Adv. Funct. Mater. 2017, 27, 1703198. [Google Scholar] [CrossRef]
- Repp, D.; Barreda, A.; Vitale, F.; Staude, I.; Peschel, U.; Ronning, C.; Pertsch, T. Lasing modes in ZnO nanowires coupled to planar metals. Opt. Express 2023, 31, 3364. [Google Scholar] [CrossRef]
- Wang, J.; Jia, X.; Guan, Y.; Ren, K.; Yu, H.; Wang, Z.; Qu, S.; Yang, Q.; Lin, J.; Wang, Z.; et al. The Electron–Hole Plasma Contributes to Both Plasmonic and Photonic Lasing from CH3NH3PbBr3 Nanowires at Room Temperature. Laser Photonics Rev. 2021, 15, 2000512. [Google Scholar] [CrossRef]
- Chou, Y.-H.; Wu, Y.-M.; Hong, K.-B.; Chou, B.-T.; Shih, J.-H.; Chung, Y.-C.; Chen, P.-Y.; Lin, T.-R.; Lin, C.-C.; Lin, S.-D.; et al. High-Operation-Temperature Plasmonic Nanolasers on Single-Crystalline Aluminum. Nano Lett. 2016, 16, 3179–3186. [Google Scholar] [CrossRef]
- Wu, Z.; Chen, J.; Mi, Y.; Sui, X.; Zhang, S.; Du, W.; Wang, R.; Shi, J.; Wu, X.; Qiu, X.; et al. All-Inorganic CsPbBr 3 Nanowire Based Plasmonic Lasers. Adv. Opt. Mater. 2018, 6, 1800674. [Google Scholar] [CrossRef]
- Huang, C.; Sun, W.; Fan, Y.; Wang, Y.; Gao, Y.; Zhang, N.; Wang, K.; Liu, S.; Wang, S.; Xiao, S.; et al. Formation of Lead Halide Perovskite Based Plasmonic Nanolasers and Nanolaser Arrays by Tailoring the Substrate. ACS Nano 2018, 12, 3865–3874. [Google Scholar] [CrossRef] [PubMed]
- Kwon, S.-H.; Kang, J.-H.; Seassal, C.; Kim, S.-K.; Regreny, P.; Lee, Y.-H.; Lieber, C.M.; Park, H.-G. Subwavelength Plasmonic Lasing from a Semiconductor Nanodisk with Silver Nanopan Cavity. Nano Lett. 2010, 10, 3679–3683. [Google Scholar] [CrossRef]
- Stockman, M.I. The spaser as a nanoscale quantum generator and ultrafast amplifier. J. Opt. 2010, 12, 024004. [Google Scholar] [CrossRef]
- Zhang, L.; Ge, C.; Zhang, K.; Tian, C.; Fang, X.; Zhai, W.; Tao, L.; Li, Y.; Ran, G. Lattice plasmons in dielectric nanoparticle arrays arranged on metal film. J. Opt. 2016, 18, 125002. [Google Scholar] [CrossRef]
- Qi, D.; Tang, S.; Wang, L.; Dai, S.; Shen, X.; Wang, C.; Chen, S. Pulse laser-induced size-controllable and symmetrical ordering of single-crystal Si islands. Nanoscale 2018, 10, 8133–8138. [Google Scholar] [CrossRef]
- Shen, B.; Huang, Y.; Duan, X.; Ren, X.; Zhang, X.; Wang, Q. Subwavelength Energy Transport Along a Dielectric Nanoparticle Chain in a Metal Slot. IEEE Photonics J. 2013, 5, 4500309. [Google Scholar] [CrossRef]
- Krasnok, A.; Li, S.; Lepeshov, S.; Savelev, R.; Baranov, D.G.; Alu, A. All-Optical Switching and Unidirectional Plasmon Launching with Nonlinear Dielectric Nanoantennas. Phys. Rev. Appl. 2018, 9, 14015. [Google Scholar] [CrossRef]
- Sun, Y.; Zhao, D.; Zhang, Z.; Garg, N.; Bogdanov, B.V.; Senyushkin, P.R.; Su, M.; Zuev, D.A.; Kumar, S.; Ganguli, A.K.; et al. Green printed hybrid optical dielectric nanostructures on a mirror. Photonics Nanostruct. Fundam. Appl. 2023, 55, 101147. [Google Scholar] [CrossRef]
- Oudich, M.; El-Jallal, S.; Pennec, Y.; Djafari-Rouhani, B.; Gomis-Bresco, J.; Navarro-Urrios, D.; Sotomayor Torres, C.M.; Martínez, A.; Makhoute, A. Optomechanic interaction in a corrugated phoxonic nanobeam cavity. Phys. Rev. B 2014, 89, 245122. [Google Scholar] [CrossRef]
- Bordas, F.; Steel, M.J.; Seassal, C.; Rahmani, A. Confinement of band-edge modes in a photonic crystal slab. Opt. Express 2007, 15, 10890. [Google Scholar] [CrossRef]
- Goncharov, A.P.; Gorelik, V.S. Probe Raman spectroscopy in photon traps. Bull. Lebedev Phys. Inst. 2008, 35, 257–263. [Google Scholar] [CrossRef]
- Dong, P.; Dai, D.; Shi, Y. Low-index-mode photonic crystal nanobeam cavity for refractive index sensing at the 2 μm wavelength band. Appl. Opt. 2019, 58, 3059. [Google Scholar] [CrossRef]
- Alagappan, G.; Krivitsky, L.A.; Png, C.E. Purcell enhancement of light emission in nanodiamond using a trenched nanobeam cavity. J. Opt. 2020, 22, 025401. [Google Scholar] [CrossRef]
- Cheng, Z.; Dong, J.; Zhang, X. Ultracompact optical switch using a single semisymmetric Fano nanobeam cavity. Opt. Lett. 2020, 45, 2363. [Google Scholar] [CrossRef]
- He, Z.; Chen, B.; Hua, Y.; Liu, Z.; Wei, Y.; Liu, S.; Hu, A.; Shen, X.; Zhang, Y.; Gao, Y.; et al. CMOS Compatible High-Performance Nanolasing Based on Perovskite–SiN Hybrid Integration. Adv. Opt. Mater. 2020, 8, 2000453. [Google Scholar] [CrossRef]
- Yang, D.-Q.; Duan, B.; Liu, X.; Wang, A.-Q.; Li, X.-G.; Ji, Y.-F. Photonic Crystal Nanobeam Cavities for Nanoscale Optical Sensing: A Review. Micromachines 2020, 11, 72. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.-H.; Xu, X.-S. High- Q cavity based on gradated one-dimensional photonic crystal. Chin. Phys. B 2014, 23, 114205. [Google Scholar] [CrossRef]
- Quan, Q.; Burgess, I.B.; Tang, S.K.Y.; Floyd, D.L.; Loncar, M. High-Q, low index-contrast polymeric photonic crystal nanobeam cavities. Opt. Express 2011, 19, 22191–22197. [Google Scholar] [CrossRef] [PubMed]
- Palik, E.D.; Ghosh, G. (Eds.) Handbook of Optical Constants of Solids; Academic Press: San Diego, CA, USA, 1998; ISBN 978-0-12-544420-0. [Google Scholar]
- Yu, H.; Ren, K.; Wu, Q.; Wang, J.; Lin, J.; Wang, Z.; Xu, J.; Oulton, R.F.; Qu, S.; Jin, P. Organic-inorganic perovskite plasmonic nanowire lasers with a low threshold and a good thermal stability. Nanoscale 2016, 8, 19536–19540. [Google Scholar] [CrossRef] [PubMed]
- Segovia, R.; Qu, G.; Peng, M.; Sun, X.; Shi, H.; Gao, B. Evolution of Photoluminescence, Raman, and Structure of CH3NH3PbI3 Perovskite Microwires Under Humidity Exposure. Nanoscale Res. Lett. 2018, 13, 79. [Google Scholar] [CrossRef] [PubMed]
- Löper, P.; Stuckelberger, M.; Niesen, B.; Werner, J.; Filipič, M.; Moon, S.-J.; Yum, J.-H.; Topič, M.; De Wolf, S.; Ballif, C. Complex Refractive Index Spectra of CH3NH3PbI3 Perovskite Thin Films Determined by Spectroscopic Ellipsometry and Spectrophotometry. J. Phys. Chem. Lett. 2015, 6, 66–71. [Google Scholar] [CrossRef]
- Li, W.; Peng, X.; Jia, L.; Yan, W.; Yang, F.; Li, Z. Designs for improving plasmonic nanowire microcavity quality factor. In Proceedings of the 5th Optics Young Scientist Summit (OYSS 2022); Lu, C.-Y., Chen, F., Li, Z., Cai, Y., Eds.; SPIE: Fuzhou, China, 2022; p. 74. [Google Scholar]
- Kim, S.; Ahn, B.-H.; Kim, J.-Y.; Jeong, K.-Y.; Soo, K.; Lee, Y.-H. Nanobeam photonic bandedge lasers. Opt. Express 2011, 19, 24055–24060. [Google Scholar] [CrossRef]
- Yang, D.; Tian, H.; Ji, Y. High-Q and high-sensitivity width-modulated photonic crystal single nanobeam air-mode cavity for refractive index sensing. Appl. Opt. 2015, 54, 1. [Google Scholar] [CrossRef]
- Yu, P.; Qi, B.; Xu, C.; Hu, T.; Jiang, X.; Wang, M.; Yang, J. An improved surface-plasmonic nanobeam cavity for higher Q and smaller V. Chin. Sci. Bull. 2012, 57, 3371–3374. [Google Scholar] [CrossRef]
- Yao, K.; Shi, Y. High-Q width modulated photonic crystal stack mode-gap cavity and its application to refractive index sensing. Opt. Express 2012, 20, 27039. [Google Scholar] [CrossRef]
- Zhu, H.; Fu, Y.; Meng, F.; Wu, X.; Gong, Z.; Ding, Q.; Gustafsson, M.V.; Trinh, M.T.; Jin, S.; Zhu, X.-Y. Lead halide perovskite nanowire lasers with low lasing thresholds and high quality factors. Nat. Mater. 2015, 14, 636–642. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Li, J.; Zhang, S.; Yi, C.; Xu, Z. Novel CH3NH3PbI3/polyimide composites with enhanced film-forming and electrical conductive properties. High Perform. Polym. 2018, 30, 847–855. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Peng, X.; Kang, Y.; Mao, X.; Yan, W.; Zhao, Y.; Liu, K.; Yang, F.; Li, Z. Design of High-Q-Gradient Dielectric Nanoparticle Chain Surface Plasmonic Cavities. Photonics 2024, 11, 117. https://doi.org/10.3390/photonics11020117
Liu J, Peng X, Kang Y, Mao X, Yan W, Zhao Y, Liu K, Yang F, Li Z. Design of High-Q-Gradient Dielectric Nanoparticle Chain Surface Plasmonic Cavities. Photonics. 2024; 11(2):117. https://doi.org/10.3390/photonics11020117
Chicago/Turabian StyleLiu, Jing, Xuanran Peng, Yaru Kang, Xu Mao, Wei Yan, Yongmei Zhao, Kong Liu, Fuhua Yang, and Zhaofeng Li. 2024. "Design of High-Q-Gradient Dielectric Nanoparticle Chain Surface Plasmonic Cavities" Photonics 11, no. 2: 117. https://doi.org/10.3390/photonics11020117
APA StyleLiu, J., Peng, X., Kang, Y., Mao, X., Yan, W., Zhao, Y., Liu, K., Yang, F., & Li, Z. (2024). Design of High-Q-Gradient Dielectric Nanoparticle Chain Surface Plasmonic Cavities. Photonics, 11(2), 117. https://doi.org/10.3390/photonics11020117





