A Method of Laser Frequency Stabilization Based on the Effect of Linear Dichroism in Alkali Metal Vapors in a Modulated Transverse Magnetic Field

Abstract

We present a method of laser frequency stabilization based on the linear dichroism signal in a transverse magnetic field. This method is similar to the DAVLL (Dichroic Atomic Vapor Laser Lock) method. It differs from DAVLL and from its existing modifications primarily by the fact that it uses signals of linearly polarized light caused by alignment, rather than circular refraction caused by orientation, and therefore allows us to obtain error signals at the magnetic field modulation frequency (or its second harmonic) by extremely simple means. The method allows the laser frequency to be stabilized in the vicinity of the low-frequency transition in the D1 line of Cs; it does not require strong magnetic fields or careful shielding of cells containing cesium atoms. Although the absorption line in a gas-filled cell is typically gigahertz wide, the achievable resolution, limited by the signal-to-noise ratio of photon shot noise, can reach units or tens of kilohertz in a one hertz bandwidth.

1. Introduction

The development of new precise spectroscopic methods and closely related methods of quantum optics and spintronics has led to the emergence of new areas of applied physics. New optical methods of controlling the spin states of atomic ensembles, cooling of atoms and molecules [1], and interferometry of cold atoms [2] have resulted in the creation of new secure communication systems [3,4], optical [5] and radio frequency standards using thermal and cold atoms, and classes of quantum sensors (e.g., optical sensors of magnetic field [6,7,8,9,10], electric field [11], rotation [12], temperature [13], and so on). The vast majority of the above methods and devices are based on the use of a highly coherent laser stabilized in the vicinity of a narrow spectral absorption line in the atomic medium. As a rule, such a medium consists of hydrogen-like structures characterized by the presence of one electron on the outer shell, namely alkali metal atoms (in most cases—rubidium or cesium) in the gaseous phase or isolated positive ions of alkali earth metals. The field of applications of quantum optics is continuously expanding—an example is the wide class of sensors based on nitrogen-vacancy centers in diamond [14,15,16] or devices based on rare earth atoms [17] and their ions [18]. Nevertheless, alkali metals undoubtedly remain the most commonly used medium in all of the above methods and tasks.

The aforementioned advanced methods in quantum optics rely on stabilized single-mode lasers. While various techniques exist for stabilizing laser frequency—including stabilization to optical resonators, beat frequency stabilization using a reference laser, and many others—long-term frequency stabilization is invariably achieved by referencing to atomic or molecular transitions. Atomic optical transitions, as natural frequency references, offer unparalleled accuracy and long-term stability, making them indispensable for quantum optics instrumentation. Therefore, many applications, including laser cooling, quantum communication, and atomic interferometry, require lasers stabilized to transitions in separate atomic ensembles. A typical stabilization module consists of a cell with reference atoms, a thermostat, a magnetic shield, and an optical system for beam shaping and detection. A well-known example is the COSY unit from Thorlabs, Inc., which utilizes saturated absorption spectroscopy resonances.

It should be noted, however, that simpler frequency stabilization methods often require laser frequency modulation, with the modulation amplitude being comparable to the width of the absorption line [19]. To stabilize the laser frequency by atomic transitions, the absorption (or saturated absorption) signal is typically used, which requires light frequency modulation and subsequent lock-in amplification [20]. This method has a significant disadvantage: the laser radiation line is split into a number of spectral components that together form an anti-symmetric linear spectrum. At the same time, the most precise methods in both quantum optics (e.g., cold atom interferometry) and laser spectroscopy (in particular, nonlinear sub-Doppler or Raman spectroscopy) impose stringent requirements on the laser spectrum that are incompatible with such modulation.

For a long time, this contradiction was resolved (and continues to be resolved) by using complex, unreliable, and expensive external acousto-optic modulators. These devices not only shift the laser frequency but also alter its direction, necessitating relatively intricate optical schemes to compensate for this effect.

The situation changed dramatically with the invention of the Dichroic Atomic Vapor Laser Lock (DAVLL) technique, which, for the first time, enabled laser stabilization to an atomic transition without frequency modulation. Within a few years, DAVLL schemes became ubiquitous in laboratories, and several modifications of this method emerged, overcoming its inherent limitations to varying degrees.

This work presents a stabilization method akin to DAVLL, but based on a distinct physical principle: detecting the absorption signal of linearly polarized light caused by alignment, rather than circular refraction caused by orientation. This approach avoids the main drawbacks of DAVLL and its modifications.

To date, many schemes without modulation have been developed; all of them are based on polarization spectroscopy methods. In the 1970s, it was shown [21] that the circular anisotropy signal of saturated absorption in a weak magnetic field can be used to stabilize the laser frequency. This method was applied in [22,23]; its advantage is its high stability, while its disadvantages include a complex two-beam scheme, the impossibility of frequency locking outside the narrow line, and dependence on the stability of the magnetic field.

1.1. The DAVLL Method

The DAVLL method [24,25] uses the circular dichroism signal in a strong magnetic field. The main advantage of the method is its simplicity, the compactness of its single-beam optical scheme, and the possibility of suppressing laser intensity noise in a balanced way. Its main disadvantages are signal registration at DC frequency and the necessity of creating a strong magnetic field.

The DAVLL scheme can be easily modified to detect the circular birefringence signal or the sum of birefringence and dichroism signals [22,26]. The laser frequency can be tuned within the optical linewidth by rotating the balanced polarization-sensitive detector and the quarter-wave plate. Disadvantages of this scheme include the drift of the DAVLL reference frequency caused by any instability in the temperature of the reference cell [27], the polarization instability of the optical scheme, and the temperature instability of the magnetic field.

In applications where higher stability is required, the DAVLL circuit may use saturated absorption resonances [28,29]. The saturated absorption signals have a high conversion slope and small width, ensuring minimal frequency drift. However, the two-beam scheme cannot provide compactness; in addition, its range of continuous frequency tuning is extremely small.

The development of the DAVLL method using both saturated absorption resonances and saturated reflection signals is described in [28,29,30]. The use of millimeter-sized compact cells in [31,32] allowed the development of compact devices. In [33], a three-beam scheme for the detection of sub-Doppler circular dichroism resonances is presented; it is characterized by high complexity while showing high stability and low drift of the reference frequency (0.25 MHz and 0.02 MHz over a time of 10 h, respectively).

Among the unsolved problems is the task of tuning the frequency of the reference scheme within wide limits. This task is somewhat self-contradictory since a wide frequency tuning range means a large width of the reference frequency line, and therefore a low conversion slope, which corresponds to a low sensitivity to detuning and a high level of drift. Attempts have been made to solve this problem by using an acousto-optical modulator (AOM) in the DAVLL circuit to lock to the frequency harmonics [34], or by increasing the width of the absorption line by increasing the cell temperature [35] or reducing its size [36,37].

In [38], linearly polarized light was directed transversely to a strong magnetic field. The linear birefringence signal, which has a dispersive form suitable for stabilization, was recorded with a balance detector (t-DAVLL method). Further, in [39], the saturated absorption signal of birefringence in a magnetic field of the order of millitesla units was registered by this method. The disadvantages of this approach are its strong magnetic field and DC signal detection.

To the best of our knowledge, the work closest to ours is [40], where linear dichroism signals in a cesium vacuum cell were observed in a transverse magnetic field of about 0.68 mT under D₂ line excitation. The polarization direction was modulated along and across the magnetic field, allowing the absorption coefficients of orthogonal polarizations to be compared. The absorption signal at the modulation frequency was recorded. The polarization modulation was carried out within very small limits, of the order of milliradian units. The use of the obtained linear dichroism signal to stabilize the laser frequency was not proposed.

Note that all methods based on the observation of dichroism in the longitudinal field allow one to obtain the error signal only at a low (conditionally “zero” or “DC”) frequency, at which the laser noise is particularly high. To transfer the signal to the region of non-zero frequencies, additional devices must be used—e.g., a mechanical optical chopper. This severely limits the scope of application of such methods—as the use of strong magnetic fields does in the original DAVLL method.

1.2. TL-DAVLL Method

In contrast to the above works, the present work considers a method of polarization spectroscopy in a small, but non-zero, transverse magnetic field, which allows the stabilization of the laser frequency without its modulation. We propose our own variant of the DAVLL scheme in a modulated transverse magnetic field—Transverse Linear Dichroic Atomic Vapor Laser Lock (TL-DAVLL) (Since the method involves detecting an absorption signal, this abbreviation can also stand for Transmission-Loss DAVLL). As will be shown below, this method is free from the abovementioned drawbacks, while being (i) extremely simple and (ii) potentially providing both high resolution at the level of hundreds or even tens of kilohertz and high resilience to variations in parameters (such as cell temperature, laser intensity, etc.). This method can be used in MW atomic clocks. First of all, this applies to laser-pumped beam frequency standards, in which the optical density of the atomic beam is too low to stabilize the laser directly by absorption in the beam. The TL-DAVLL method will allow the stabilization of the laser at the optical transition frequency without modulating the laser frequency (in contrast to standard absorption stabilization methods) and without using strong magnetic fields (in contrast to the DAVLL method). It appears that this method can also be successfully used in cesium cold atom clocks to stabilize cooling and pumping lasers, since, in addition to the advantages noted above, it allows the smooth tuning of the laser radiation frequency.

This article is organized as follows: in Section 2, we list Materials and Methods, outline the basic principles of the effect of linear dichroism in the optically pumped atomic media, and define the direction of the research; we also describe the experimental setup. In Section 3, we present the results of our investigation of the parameters of the proposed TL-DAVLL method and estimate its achievable resolution and sensitivity; we also present the results of a laser frequency locking demo experiment. In Section 4, we discuss the main advantages and disadvantages of the method.

2. Materials and Methods

2.1. General Description

The main method used in this work is the method of polarization laser spectroscopy. The object is a cell containing saturated vapor of an alkali metal (cesium) in a weak (<10 µT) magnetic field transverse to the incoming resonant laser beam (standard Voigt configuration). The goal is to stabilize the single-mode narrow-line laser on the absorption line of the alkali metal without modulating the light parameters and without using strong magnetic fields. Methods for shielding the external magnetic field, stabilizing the temperature of the cell, and amplifying lock-in are employed.

2.2. Basic Principles

Let us consider the interaction of an alkali atom characterized by nuclear spin I with linearly polarized light directed across a constant magnetic field B₀. The ground state of the hydrogen-like atom is split into two hyperfine sublevels, F = I − 1/2 and F = I + 1/2 (Figure 1a). Each hyperfine level, in turn, is split into 2F + 1 magnetic, or Zeeman, sublevels, characterized by a magnetic number m_F (m_F = –F… + F).

Let the linearly polarized light propagate along the axis z (Figure 1b–e). When the polarization vector is directed parallel to B₀ (Figure 1b,d), light causes transitions at which the magnetic quantum numbers of the ground m_F and excited m_F’ states do not change (Δm_F = 0). Light polarized perpendicularly to B₀ excites transitions with Δm_F = ±1 (Figure 1c,e). For the case of excitation of the F = 4 level of cesium by linearly polarized D₁ line light, the relative probabilities of optical transitions are shown in Figure 1b–e. The populations of Zeeman sublevels, determined by the balance between the optical pumping and relaxation rates, are also schematically shown. In the case of linear light polarization, the distribution of populations is symmetric. Let us represent the population distribution as a sum of 2F + 1 multipole moments A_l:

$$A_{\mathrm{l}}={{\displaystyle \sum _{m_F}\left(-1\right)}}^{F-m_F}\langle F,m_F,F,-m_F;l,0\rangle n_{m_F}$$

(1)

where the value in angle brackets is the Clebsch–Gordon coefficient, n_mF is the population of the Zeeman m_F level of the state F. Multipole moments (1) represent a particular case of the decomposition of the medium density matrix by irreducible tensor components in the so-called (κq)-representation in the case of q = 0 [41]. Linearly polarized light produces a symmetric population distribution described by the sum of moments with even values of l, the smallest of which is the quadrupole (l = 2) moment, called the alignment. The non-isotropic absorption of light is proportional to the product of the alignment and the corresponding moment of the polarization matrix of the light [41].

In cells filled with buffer gas at pressures of several torr and higher, pumping occurs by the mechanism of “depopulation” [42]: during the typical time (~30 ns) that an atom spends in the excited state, the populations of the excited-state sublevels almost completely equalize due to frequent collisions with buffer gas atoms (or molecules). In this simplest case, the changes in the population of the ground-state levels are inversely proportional to the optical transitions probabilities (indicated by the numbers in the circles in Figure 1) [42].

Figure 1 shows that (i) under identical conditions, the signs of the alignment at each of the two transitions F = 4 → F′ = 4 and F = 4 → F′ = 3 are opposite; (ii) the alignment at each transition changes its sign when the polarization orientation with respect to the magnetic field changes. The absorption coefficients of such an optically oriented (more precisely, aligned) medium appear to be different for the detecting light under different polarizations. When the pumping light also acts as the detecting light, it turns out that regardless of the sign of the alignment (and, hence, of the polarization direction), the light bleaches the medium, and the sign of this effect is always the same—the absorption coefficient of the medium decreases with increasing pump efficiency. But if we consider the alignment signal (i.e., the difference ΔI of the intensities of the beam passed through the medium at E||B₀ and E⊥B₀) in the system, it turns out to be sign-variable under certain conditions. As shown, one of these conditions is the sufficient overlap of optical profiles corresponding to the transitions F = 4 → F′ = 4 and F = 4 → F′ = 3. The alignment signal parameters are determined by many factors, but above all by the laser frequency f and the light intensity. Periodically switching the direction of the light polarization with respect to the magnetic field allows this signal to be shifted to a non-zero frequency.

A significant simplification of the optical scheme can be achieved by switching the direction of the transverse magnetic field (Figure 2b) in the plane perpendicular to the laser beam to a direction perpendicular to the original one. If such modulation is carried out at a rate not much higher than the relaxation rate of the ground-state sublevels, the intensity of the radiation passing through the cell is modulated at the field-switching frequency. The transverse dichroism signal S_T1 can be extracted from the time dependence of the intensity by lock-in amplification of ΔI at the field-switching frequency.

It follows from the above that both the value of S_T1 and its sign depend on the laser frequency f. This makes it possible to use the difference signal S_T1 to stabilize the frequency f, which will be demonstrated below. In particular, when pumping a low-frequency pair of optical D₁ transitions of the Cs line in a cell with a buffer gas, the value of S_T1 goes through zero and changes its sign, while the laser frequency sweeps from the transition F = 4 → F′ = 3 to F = 4 → F′ = 4. Note that the overlapping of the optical profiles of the interrogated transitions is essential for this purpose: in a vacuum cell where very strong alignment signals can be observed, the change in the sign of S_T1 does not occur.

The physical implementation of the proposed method is as follows (Figure 2): a cell containing an alkali metal and a buffer gas is placed inside a magnetic shield and heated to a temperature providing an optimal optical density of atomic vapor. A two- or three-dimensional system of Helmholtz coils is placed around the cell inside the shield. Stabilized laser radiation is passed through the cell (we assume that the beam direction coincides with the z-axis and the polarization direction of the beam coincides with the x-axis). A voltage is alternately applied to the x and y Helmholtz coils, creating a magnetic field of the order of units or tens of microtesla (the larger the field, the more stable the system is to external perturbations, and the lower the shielding requirements). The x, y, and z coils can also be used to compensate for external magnetic fields. The intensity of the light transmitted through the cell is measured by a photodetector; thus, this method uses a single-beam scheme, which is its additional advantage. As shown above, different directions of the transverse magnetic field correspond to different absorption coefficients (the so-called linear dichroism effect); this difference is measured by lock-in amplification of the transmitted radiation signal, with the signal controlling one of the coils used as a reference signal. The magnitude and sign of this difference depends on the detuning of the laser radiation frequency relative to the atomic transition, so a feedback loop can be used to lock the laser frequency to the frequency approximately corresponding to the maximum of the absorption profile in the cell. An additional photodetector can be used to detect laser intensity noise for subsequent subtraction from the signal.

As will be shown below, the time dependence of the intensity contains not only the first harmonic but also a strong second harmonic (the transverse dichroism signal S_T2), which can also be used as the error signal. In our experiment, we used cesium as the alkali metal, but the proposed method can be extended to other alkali metals, primarily rubidium.

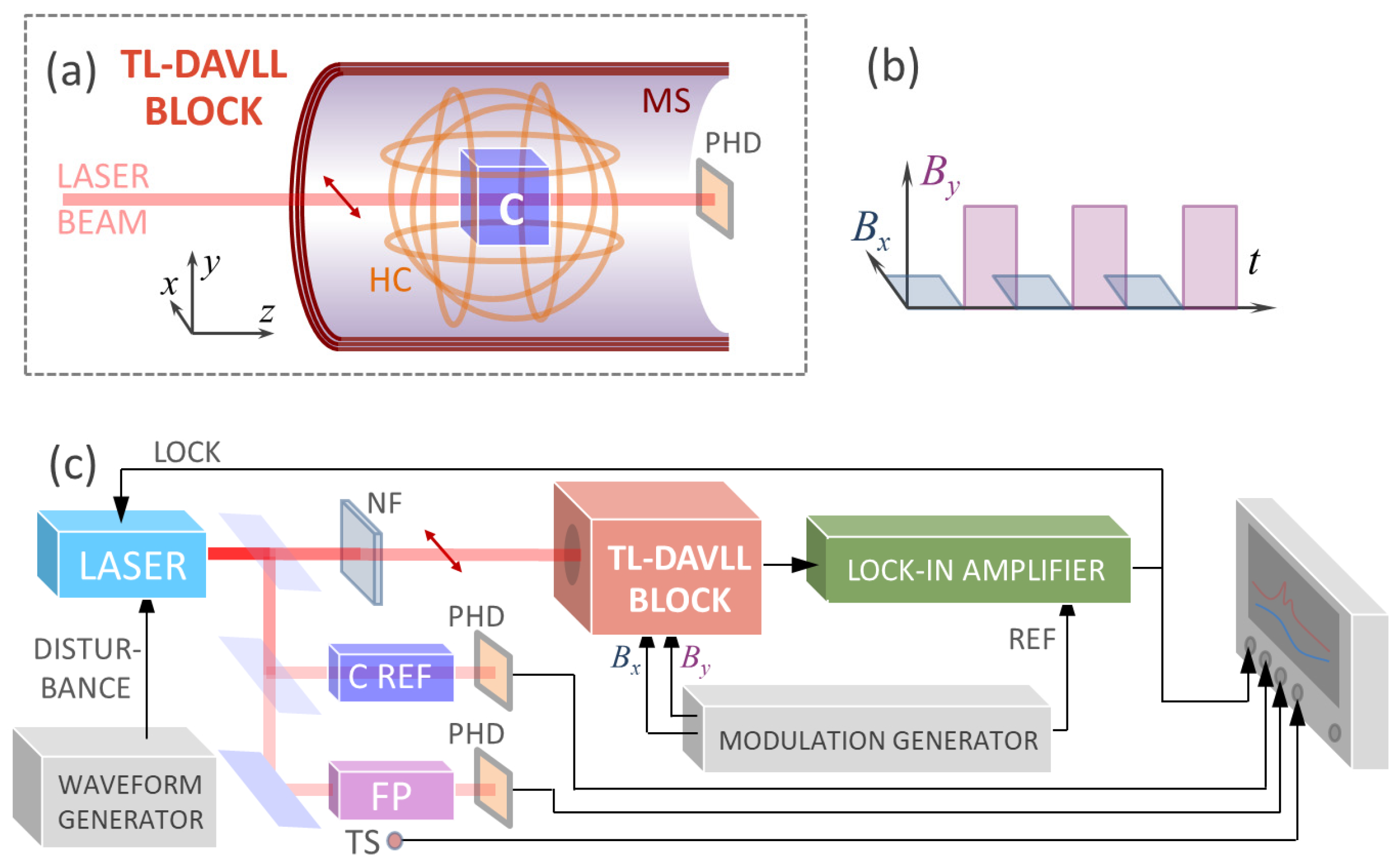
2.3. Experimental Setup

A scheme of the experimental setup is shown in Figure 2. A cell with an internal size of 5 × 5 × 5 mm³ containing a few milligrams of Cs and nitrogen at a pressure of 200 torr was placed in a thermostat (not shown in the scheme) and in a cylindrical three-layer magnetic shield [43,44]. The dependences of the cell’s transmittance signal ΔI on the detuning ∆f of the laser radiation from the Cs absorption lines were studied. The laser power P_p, the cell temperature T, the frequency f_mod, and the amplitude B_mod of modulation, as well as the value of the longitudinal field B_z, were varied in the wide range. At each parameter’s value, the laser frequency was scanned in the vicinity of the absorption lines F = 4 ↔ F′ = 3, F′ = 4 (low-frequency pair) or F = 3 ↔ F′ = 3, F′ = 4 (high-frequency pair), and the dependence ΔI (∆f) was recorded. Simultaneously, the intensity of the light that passed through the reference vacuum cell was recorded. The range of continuous frequency tuning of the laser radiation was 8–10 GHz. The signal from the reference cell was approximated by the sum of two Gaussian profiles on a nonlinear substrate; thus, the frequency scale of each recording was determined.

Linearly polarized light with a wavelength of 895 nm (D₁ line of Cs) generated by an external cavity semiconductor laser (VitaWave Company—Moscow, Russia) was transmitted through the cell in the direction of the shield axis (z-axis). The beam cross-section was ~3 mm². A part of the laser beam was split off by a semi-transparent mirror and directed to a Cs vapor reference cell. The light passed through the cells was registered by photodiodes.

The transverse field with modulated orientation (x–y) was generated by a Helmholtz coil 3D system; the modulation frequency f_mod was varied between 20 Hz and 20 kHz. The longitudinal (z) pair of coils was used to compensate the residual longitudinal field and to create a test field along the z axis.

The main cell transmittance signal was amplified by a low-noise amplifier and detected and filtered by two Stanford Research SR830 lock-in amplifiers at the first and second harmonics of f_mod. The presence of the second harmonic in the transmission signal is due to the physical nature of the alignment effect, which corresponds to a symmetrical distribution of atomic moments (see Section 2.1). Lock-in amplification (also called “synchronous detection”) allowed us not only to extract both these harmonics, but also to suppress excessive noise by limiting the frequency band [45,46].

Next, the resulting error signal was numerically filtered; the locking frequency (at which the signal takes a zero value) and the steepness of the signal at this point were calculated. All the signals were recalculated to the photocurrent level at the photodetector (Hamamatsu photodiode, efficiency of conversion of radiation power into photocurrent ~0.7 A/W).

Measurements (unless otherwise specified) were performed at B_mod = 3 μT and f_mod = 130 Hz.

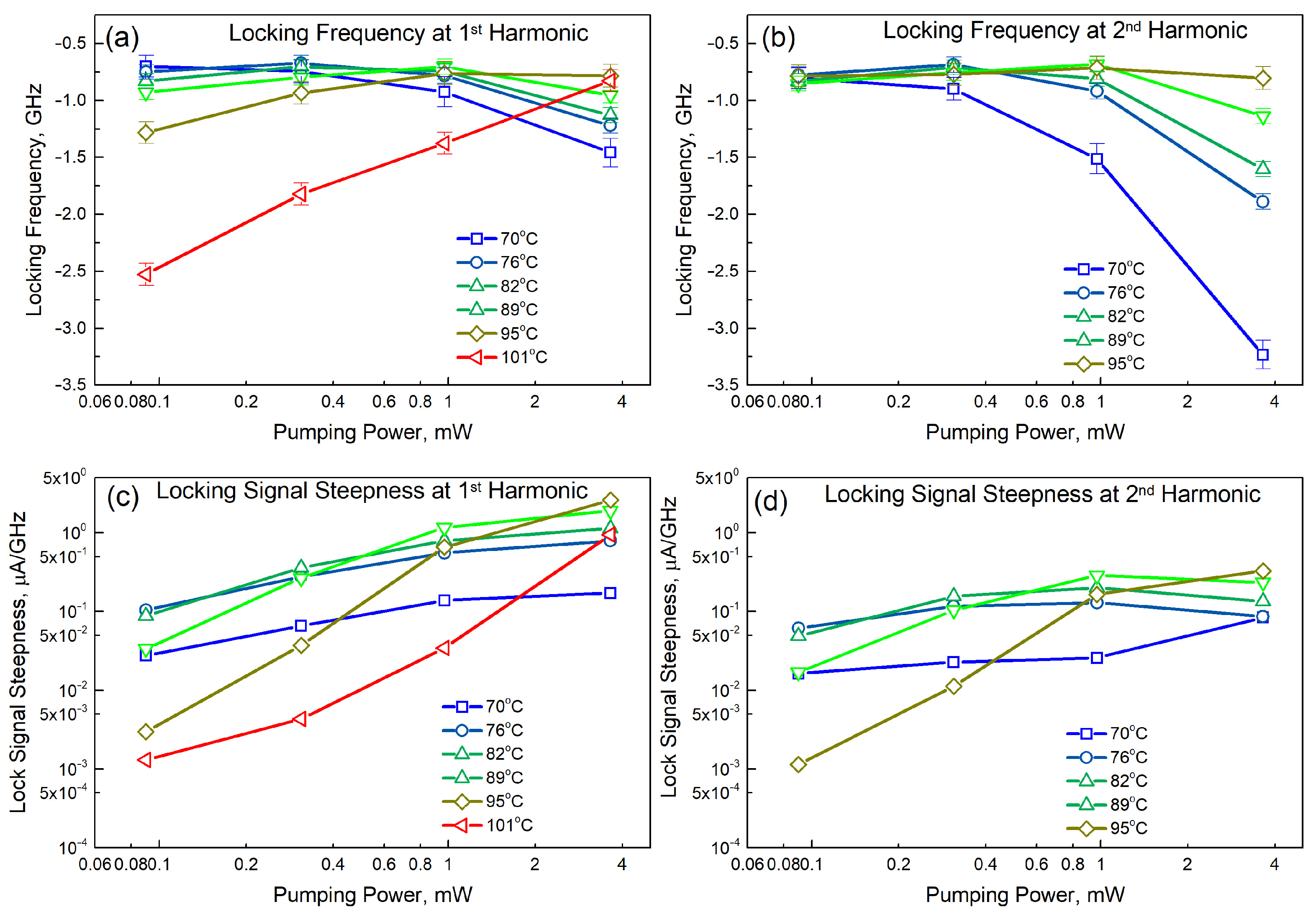
3. Results

3.1. The Linear Dichroism Signal in the Cs Cell

Figure 3 shows examples of signal dependences on the laser frequency, detuning from the F = 4 ↔ F’ = 3 transition both in the reference vacuum cell (taken at room temperature) (a) and in the TL-DAVLL cell (taken at T = 95 °C) (b,c). Figure 3c also shows the calculated absorption profiles, taking into account the broadening and shift by the buffer gas (nitrogen) at a pressure of 200 torr. The data on the broadening and shift of the alkali metal lines are given in [47]. At the nitrogen pressure of 200 Torr used in the experiment, the broadening of the cesium D1 line is 1624 MHz (which exceeds the hyperfine splitting of the excited state, which is 1167 MHz), and the shift is of −1542 MHz.

As can be seen from Figure 3b, at this temperature, the light absorption in the center of the line is very high at low incident light intensities; a strong decrease in the intensity of the transmitted light leads to the dominance of the technical noise in the center of the absorption line. But as the pumping power increases, the medium bleaches and the dichroism signals S_T1 (Figure 3c) at the first harmonic of the modulation frequency are detected with a very good signal-to-noise ratio (signals S_T2 at the second harmonic demonstrate similar frequency dependences with an amplitude that is almost an order of magnitude smaller). As expected, the dichroism signals change their sign in the region between the transition frequencies F = 4 ↔ F’ = 3 and F = 4 ↔ F’ = 4 in the gas cell (a collisional shift of the optical transitions in the gas cell is calculated using the data from [47]; the positions of optical profiles displaced by collisions are shown in Figure 3c with dotted lines).

It should be noted that the width of the linear part of the S_T1’s dependence on the frequency tuning (Figure 3c) allows, with some loss of accuracy, a controlled tuning of the laser frequency within the profile of the absorption line. This can be achieved by introducing an artificial offset to the value of the locking threshold. Let the signal S_T1(ν) steepness be K = dS_T1/dν. When stabilizing with a DC offset of magnitude A, the frequency shift Δν relative to the zero point of the S_T1(ν) signal is Δν = A/K. When the slope changes by dK, the relative change in frequency will be dΔν/Δν ≈ dK/K. In other words, the relative change in the frequency shift is equal to the relative change in the slope of the frequency dependence of the signal. Thus, even with Δν = 100 MHz and dK/K = 0.1 (a clearly overestimated value), the frequency variation will be 10 MHz, which is quite acceptable in many applications.

The parametric dependences of the frequency at which the laser is stabilized (in the absence of an artificial offset) using the first and second harmonics are shown in Figure 4a,b. The corresponding values of the steepness of the dichroism signals in the vicinity of the transition F = 4 ↔ F′ = 3 are shown in Figure 4c,d. It can be seen that the above dependences have extrema both in temperature and pumping power.

Now, we evaluate the ultimate parameters of the method assuming that the technical noise of the laser at the modulation frequency is suppressed to the level of the shot noise of the photocurrent (this is easier to achieve if the modulation frequency is high enough):

$$\delta f=\sqrt{2e\cdot I_{PH}}\cdot {\left({{\displaystyle \frac{d{S}_d}{df}}|}_{S_D=0}\right)}^{-1}.$$

(2)

In Figure 5a,b, the dependences given in Figure 4a,c are presented in the form of contour maps. Figure 5c shows the estimated value of the maximum achievable resolution limited by the shot noise. It was calculated by dividing the shot noise value calculated from the experimentally measured photocurrent by the experimentally measured steepness value. The maximum value of the achievable resolution in Figure 5c is ~2 kHz/√Hz, but it is realized under sub-optimal conditions in terms of stability.

The white crosses in Figure 5a–c mark the point where the minima of the temperature and pumping power dependence of the reference frequency are reached and the corresponding first derivatives are zeroed. In this case, the quadratic coefficients of the dependence of the stabilization frequency on the cell temperature and the power of the incoming radiation measured at T = 90 °C, P_p = 1.0 mW, were as follows:

d²f/dI² = –180 ± 30 MHz/mW²,
d²f/dT² = –0.61 ± 0.29 MHz/°C².

(3)

All similar dependences were measured for the dichroism signals in the vicinity of transitions F = 3 ↔ F’ = 3, F’ = 4. It turned out that the steepness of these signals is about an order of magnitude less than the steepness of the signals near the F = 4 ↔ F’ = 3, F’ = 4 transitions. Furthermore, the zero-crossing of the dichroism signal is not reached in all pumping regimes. Nevertheless, the local extrema of the frequency dependence on temperature and pumping power are also observed for the high-frequency pair of transitions.

The dependence of the steepness of the dichroism signals on the modulation frequency was somewhat unexpected (Figure 6). One could expect that the frequency decay of the steepness starts at f_mod > Γ/2π, where Γ/2π is the relaxation rate of the Cs ground-state sublevels in the cell under study (in our experiment, Γ/2π varied from 350 Hz to 2 kHz, depending on the pumping parameters), but the dependences we obtained turned out to be much more complicated.

Obviously, at high (>Γ/2π) frequencies, the dynamics of the system when switching the direction of the magnetic field are determined not by relaxation but by the re-distribution of the second-order magnetic moments to new magnetic field directions. In our case, the system proved to be operable at frequencies up to f_mod ≈ 10 kHz. At the frequency f_mod ≈ 3.5 kHz, the phase inversion of the signals occurs; it is noteworthy that these frequencies are almost independent of the magnitude of the field generated by the transverse coils. All these effects require further investigation, but it is beyond the scope of this article, devoted to the description of the method and demonstration of its performance.

Another parameter that can affect the performance of the circuit is the presence of the residual longitudinal magnetic field. We studied its influence and found that as long as the longitudinal field does not exceed ~20% of the transverse field (0.8 μT in our case), it has no noticeable effect on the reference frequency (Figure 7a). The steepness of the signal decreases by a factor of less than 1/√2 in this case (Figure 7b). This result is very significant for practical applications: it means that the proposed method not only does not use strong magnetic fields, but also does not require particularly good magnetic shielding.

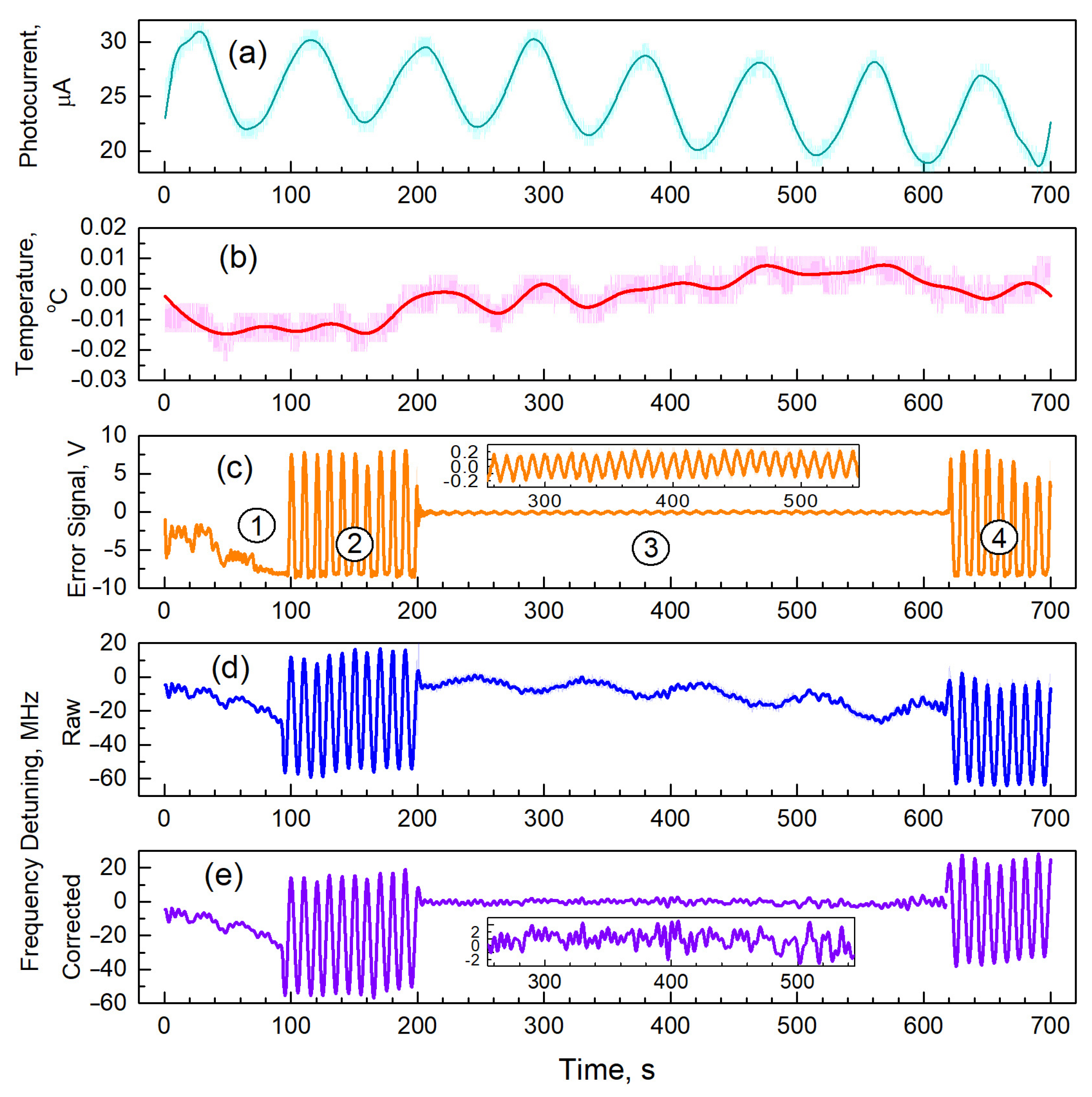
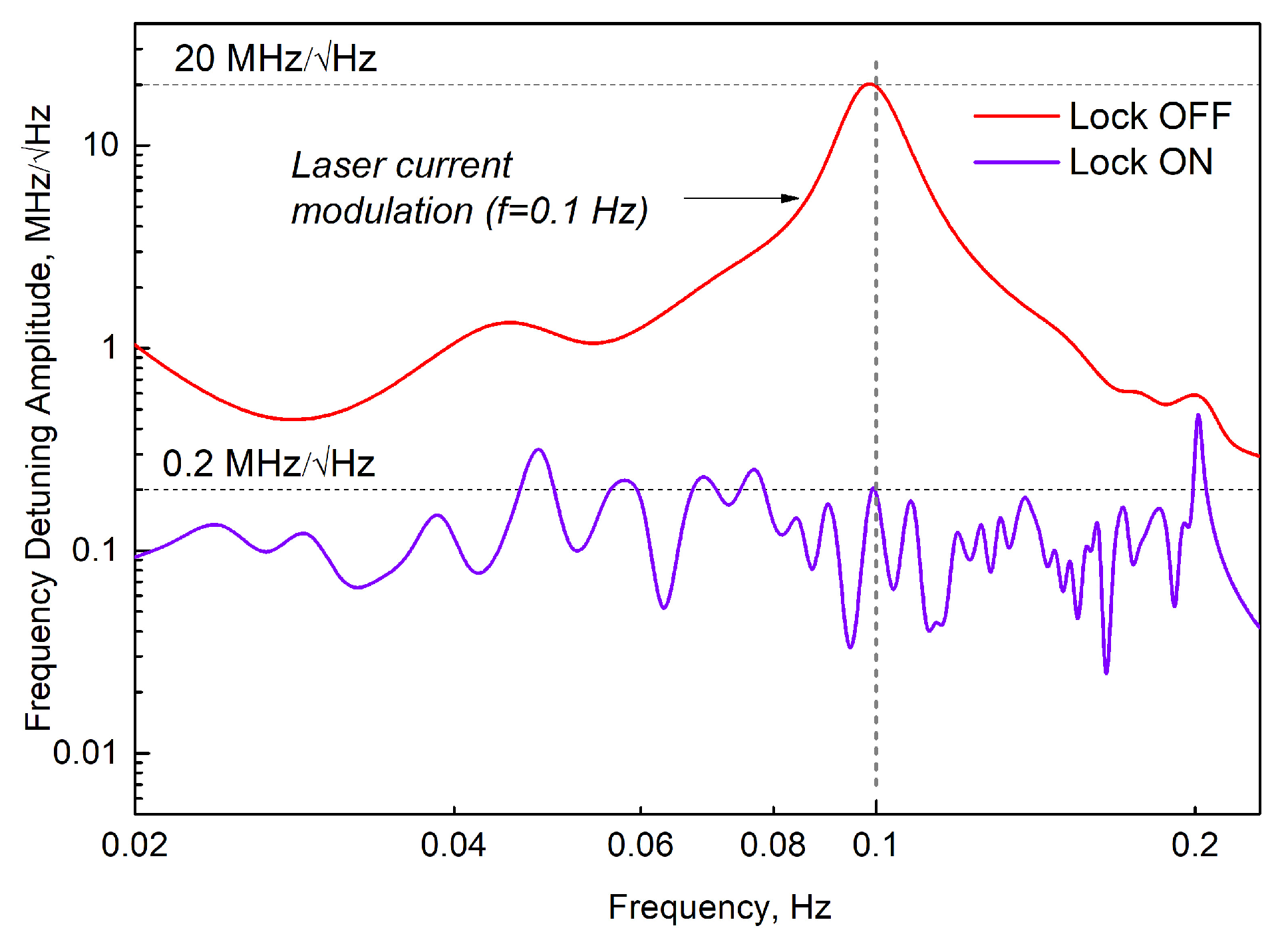
3.2. Experiment Demonstrating Laser Frequency Stabilization

We tried to perform a demo stabilization of the laser using the transverse dichroism signal. To monitor the laser frequency, we used a Fabry–Perot SA-200 interferometer (Thorlabs, Inc.—Newton, NJ, USA). It should be noted that temperature variations of the interferometer frequency dominate in the scheme—the temperature coefficient of invar expansion (1.2 × 10⁻⁶ K⁻¹) corresponds to the temperature coefficient of the interferometer frequency ~0.3 GHz/K, or 30 MHz at temperature change dT = 0.1 °C.

Therefore, we first artificially expanded the interferometer bandwidth (~7.5 MHz) by switching the interferometer photodiode into a significantly nonlinear photovoltaic mode—in this case, the width of the quasi-linear part of the interferometer output signal characteristic was 75 MHz. Second, we had to add independent temperature control of the interferometer body to the circuit. Third, we had to demonstrate the performance of the laser frequency stabilization scheme by introducing artificial perturbations into the laser frequency, similar to what was conducted in [37]. In our case, a sinusoidal perturbation at a frequency of 0.1 Hz was introduced into the laser diode current, and the detected error signal from the TL-DAVLL block was fed to the piezoceramic control input of the laser external resonator.

Figure 8 shows the results of a demonstration experiment performed at f_mod = 1 kHz. No balanced photodetector was used in the experiment, in order to demonstrate the performance of the simplest version of the TL-DAVLL block. Therefore, the modulation frequency was increased to reduce the influence of low-frequency laser noise. For stabilization, we chose the points marked in Figure 5a–c by white crosses (P = 1.2 mW, T = 88.5 °C). In this case, the stabilization frequency was shifted from the frequency of the unperturbed transition F = 4 ↔ F′ = 3 by Δf = −0.7 GHz.

As can be seen from Figure 8a, the temperature controller of the TL-DAVLL cell operated in the oscillating mode; the magnitude of slow oscillations was ~1.2 °C, which, given the circuit parameters, resulted in frequency variations of ~7.7 MHz (Figure 8d). The interferometer temperature drifted slowly within 0.02 °C (Figure 8b), corresponding to a frequency drift of the interferometer of about 6 MHz (Figure 8d).

At the interval 0–200 s, the stabilization scheme was switched off. At t = 100 s, slow modulation of the laser current with a frequency of 0.1 Hz was turned on. At t = 200 s, the stabilization scheme was turned on, and it was switched off again at t = 610 s. At this interval, the 0.1 Hz component disappears from the interferometer signal but appears in the error signal of the TL-DAVLL circuit (Figure 8c).

During processing, we subtracted long-term drifts, specifically, the component correlated with the cell temperature (Figure 8a) and the quadratic baseline representing the total drift from the interferometer signal (Figure 8b). The result is shown in Figure 8e. Note that subtracting slow components did not affect the calculation of variations at 0.1 Hz. Figure 9 demonstrates the suppression of external perturbations by comparing the spectra of the interferometer signal with the stabilization loop turned off and on.

It can be seen that the perturbation is suppressed by at least two orders of magnitude. The time constant of the Stanford Research SR830 lock-in amplifier was 10 s at a gain drop of 6 dB/oct. Taking into consideration a suppression factor in the feedback loop of K ≈ 100 (Figure 8c and Figure 9), it corresponds to a time constant τ ≈ 0.1 s at closed-loop feedback. A further increase in the control coefficient is possible by using a PID controller.

4. Discussion

We investigated the parameters of our proposed TL-DAVLL method, evaluated its ultimate parameters, and performed an experiment demonstrating the efficiency of the method. The suppression of artificially introduced interference up to 0.1–0.2 MHz/√Hz was achieved in a demonstration experiment (Figure 9).

Based on the ultimate parameters of the scheme obtained above, we can estimate the achievable level of its drift. Let us assume that the uncontrolled variations in the parameters in the system are dI/I = 0.01 and dT = 0.1 °C. Substituting these values into (3), we obtain an ultimate total frequency instability of 24 kHz. At the same time, with a constant temperature-setting error of only ΔT = 1 °C and the same level of variation dT = 0.1 °C, the total frequency instability reaches 100 kHz, and this value grows quadratically with ΔT.

When assessing long-term stability, the effect of the aging of the gas mixture should also be taken into account. Based on the values given in [47,48], we may calculate the relative frequency shifts of the optical D₁ and hyperfine transitions by buffer gas (nitrogen), ~2.3 × 10⁻⁸ torr⁻¹ and ~2.9 × 10⁻⁸ torr⁻¹, correspondingly. Since the relative shifts for both types of transitions are close in magnitude, their drifts associated with changes in the gas pressure in the cell should also be close. For the hyperfine transition used in MW gas cell atomic clocks, the typical value of this drift is ~10⁻¹¹ per year [49]. Therefore, we can expect that the relative frequency shift of the optical transition due to cell aging will be approximately the same, and the absolute shift should not exceed 4 kHz/year. Obviously, this type of shift is not dominant.

The ultimate (shot noise-limited) frequency resolution under the conditions mentioned above is 3.9 kHz/√Hz. Thus, the ultimate relative short-term resolution of the method is 1.2 × 10⁻¹¹ in a one-hertz bandwidth, and the expected relative instability is about 3 × 10⁻¹⁰.

The ultimate resolution related to the width of the optical profile (~3.2 GHz in our experiment) is 1.2 × 10⁻⁶ in a one-hertz bandwidth, from which it follows that the required signal-to-noise ratio is about 120 dB.

Both the short-term instability level of 0.1–0.2 MHz/√Hz (Figure 9) and the long-term (300 s) instability level of about 1 MHz (Figure 8e) shown in the demonstration experiment significantly exceed the achievable levels. They can be reduced, provided that PID controllers optimized for the task are used in the laser frequency and thermostat temperature stabilization circuits.

In addition, it should be taken into account that, due to the complicated dynamics of the alignment momentum, the scheme parameters change with the modulation frequency; thus, in our demonstration experiment, changing f_mod from 130 Hz to 1 kHz led to a shift of the extremum of the temperature dependence, and to the appearance of a linear temperature coefficient of ~6.5 MHz/°C (as can be clearly seen from the time interval 3 in Figure 8c). However, the modulation frequency is a very well-controlled scheme parameter and its variations can be ignored.

The obtained results allow us to draw a number of conclusions regarding both the proposed method in general and its specific application for the stabilization of laser radiation by the D₁ absorption line of Cs.

General conclusions:

• The method is extremely simple: the setup for the error signal detection consists of a magnetic shield, a 2D or 3D Helmholtz coil, a cell, a heater, and a photodetector;
• The method does not require any type of modulation of the laser beam;
• The modulation of the magnetic field allows the dichroism signal at a non-zero frequency to be registered;
• The method does not require strong magnetic fields; it is realized in transverse fields in the μT range;
• The resilience of the parameters to residual longitudinal fields at the level of tenths of μT allows the use of minimal shielding, like a single-layer shield or even a system of compensating coils;
• The registration of the dichroism signal is possible on the second harmonic of the modulation frequency, which even in the most compact version of the scheme allows for avoiding the influence of the magnetic field generated by the coil system on the photodetector, cables, and elements of electrical circuits;
• The laser stabilization frequency in this method is determined by the collisional shift of transition frequencies in the used cell; therefore, the choice of the locking frequency can be made not only by introducing the locking level’s artificial offset but also by selecting the gas filling of the cell.

Below, we present conclusions with respect to the concrete application of the method for stabilization of laser radiation by the Cs D₁ absorption line in the vicinity of the F = 4 ↔ F′ = 3 transition:

8.

The stabilization of the laser using the zero point of the dichroism signal is possible at the frequency corresponding approximately to the top of the absorption profile in the gas cell;

9.

A high achievable signal-to-noise ratio (~10⁶ with respect to the shot noise) makes it possible to realize a frequency resolution at the level of kilohertz or tens of kilohertz in a one-hertz bandwidth;

10.

The presence of a smooth extremum of the dependence of the locking frequency on the cell temperature and light intensity makes it possible to achieve a high (at the level of hundreds or even tens of kilohertz) stability of the locking frequency;

11.

The width of the linear part of the dependence of the dichroism signal on the frequency tuning allows (with a corresponding loss of accuracy of up to ~10 MHz) a controlled tuning of the laser light frequency within the profile of the absorption line (depending on the cell gas filling, from hundreds of MHz to units of GHz);

12.

The magnetic field modulation frequency can reach ten kilohertz.

The measured shape of the dichroism signal dependence (Figure 3c) also allows the stabilization of the laser outside the absorption line at a distance of up to several line widths from the lowest-frequency transition of the D₁ line, which can be used for quantum nondestructive measurements.

5. Conclusions

We have presented a method similar to the DAVLL method—Transverse Linear Dichroic Atomic Vapor Laser Lock (TL-DAVLL)—which, as far as can be judged from the results of our studies, allows us to avoid the main disadvantages of the existing schemes. This method is based on the dichroism signals in the transverse magnetic field, which were studied with a laser tuned to the D₁ line of Cs in a gas-filled cell. We have demonstrated the applicability of this scheme for the stabilization of the laser frequency in the vicinity of a pair of low-frequency transitions. In this case, parameters of the signals were obtained which illustrate the high achievable stability and resolving power of the method. Based on the similarity of the optical pumping processes in Cs and ⁸⁷Rb, we believe that there is every reason to expect that the method will also be applicable to the D₁ line of ⁸⁷Rb.