Implementation or Suppression of the Collective Lasing of the Laser Channels at the Intracavity Spectral Beam Combining
Abstract
:1. Introduction
2. Experimental Setup
3. Results
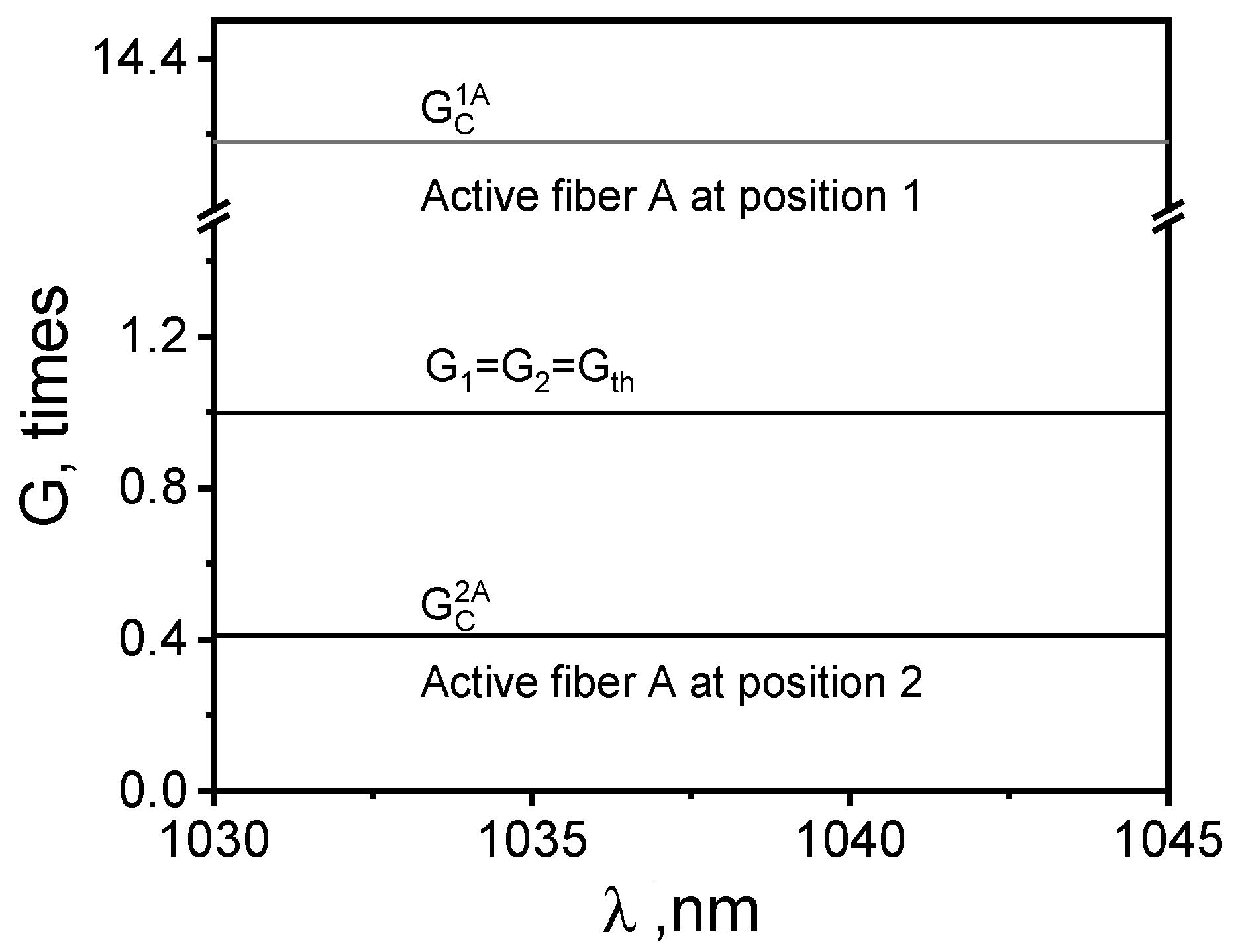
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Strecker, M.; Plötner, M.; Stutzki, F.; Walbaum, T.; Ehrhardt, S.; Benkenstein, T.; Zeitner, U.; Schreiber, T.; Eberhardt, R.; Tünnermann, A.; et al. Highly Efficient Dual-Grating 3-Channel Spectral Beam Combining of Narrow-Linewidth Monolithic CwYb-Doped Fiber Amplifiers up to 5.5 kW. In Proceedings of the Fiber Lasers XVI: Technology and Systems, San Francisco, CA, USA, 7 March 2019. [Google Scholar] [CrossRef]
- Chen, F.; Ma, J.; Wei, C.; Zhu, R.; Zhou, W.; Yuan, Q.; Pan, S.; Zhang, J.; Wen, Y.; Dou, J. 10 kW-Level Spectral Beam Combination of Two High Power Broad-Linewidth Fiber Lasers by Means of Edge Filters. Opt. Express 2017, 25, 32783. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhu, Z.; Liu, X.; Yu, M.; Li, S.; Zhang, L.; Ni, Q.; Wang, J.; Wang, X. High-Power, High-Beam-Quality Spectral Beam Combination of Six Narrow-Linewidth Fiber Amplifiers with Two Transmission Diffraction Gratings. Appl. Opt. 2019, 58, 8339. [Google Scholar] [CrossRef]
- Honea, E.; Afzal, R.S.; Savage-Leuchs, M.; Henrie, J.; Brar, K.; Kurz, N.; Jander, D.; Gitkind, N.; Hu, D.; Robin, C.; et al. Advances in Fiber Laser Spectral Beam Combining for Power Scaling. In Proceedings of the Components and Packaging for Laser Systems II, San Francisco, CA, USA, 22 April 2016. [Google Scholar] [CrossRef]
- Fan, T.Y. Laser Beam Combining for High-Power, High-Radiance Sources. IEEE J. Select. Top. Quantum Electron. 2005, 11, 567–577. [Google Scholar] [CrossRef]
- Huang, R.K.; Chann, B.; Missaggia, L.J.; Donnelly, J.P.; Harris, C.T.; Turner, G.W.; Goyal, A.K.; Fan, T.Y.; Sanchez-Rubio, A. High-Brightness Wavelength Beam Combined Semiconductor Laser Diode Arrays. IEEE Photon. Technol. Lett. 2007, 19, 209–211. [Google Scholar] [CrossRef]
- Bochove, E.J. Theory of Spectral Beam Combining of Fiber Lasers. IEEE J. Quantum Electron. 2002, 38, 432–445. [Google Scholar] [CrossRef]
- Zhu, Z.; Gou, L.; Jiang, M.; Hui, Y.; Lei, H.; Li, Q. High Beam Quality in Two Directions and High Efficiency Output of a Diode Laser Array by Spectral-Beam-Combining. Opt. Express 2014, 22, 17804. [Google Scholar] [CrossRef]
- Chann, B.; Huang, R.K.; Missaggia, L.J.; Harris, C.T.; Liau, Z.L.; Goyal, A.K.; Donnelly, J.P.; Fan, T.Y.; Sanchez-Rubio, A.; Turner, G.W. Near-Diffraction-Limited Diode Laser Arrays by Wavelength Beam Combining. Opt. Lett. 2005, 30, 2104. [Google Scholar] [CrossRef] [PubMed]
- Cook, C.C.; Fan, T.Y. Spectral Beam Combining of Yb-Doped Fiber Lasers in an External Cavity. In Advanced Solid State Lasers; Optica Publishing Group: Washington, DC, USA, 2001. [Google Scholar] [CrossRef]
- Likhanskiĭ, V.V.; Napartovich, A.P. Radiation Emitted by Optically Coupled Lasers. Sov. Phys. Usp. 1990, 33, 228–252. [Google Scholar] [CrossRef]
- Glova, A.F. Phase Locking of Optically Coupled Lasers. Quantum Electron. 2003, 33, 283–306. [Google Scholar] [CrossRef]
- Goodno, G.D.; Komine, H.; McNaught, S.J.; Weiss, S.B.; Redmond, S.; Long, W.; Simpson, R.; Cheung, E.C.; Howland, D.; Epp, P.; et al. Coherent Combination of High-Power, Zigzag Slab Lasers. Opt. Lett. 2006, 31, 1247. [Google Scholar] [CrossRef]
- Glova, A.F.; Kurchatov, S.Y.; Likhanskii, V.V.; Lysikov, A.Y.; Napartovich, A.P. Coherent Emission of a Linear Array of CO2Waveguide Lasers with a Spatial Filter. Quantum Electron. 1996, 26, 500–502. [Google Scholar] [CrossRef]
- Guryev, D.A.; Nikolaev, D.A.; Tsvetkov, V.B. Nd:GGG Disk Laser with Multipoint Spatially Periodic Optical Pumping. Laser Phys. Lett. 2016, 13, 045003. [Google Scholar] [CrossRef]
- Guryev, D.A.; Nikolaev, D.A.; Tsvetkov, V.B. Nd:YVO4 Disk Laser with Multipoint Diode Pumping and Diffraction Limited Output. Laser Phys. Lett. 2019, 16, 075002. [Google Scholar] [CrossRef]
- Kamynin, V.A.; Kashin, V.V.; Nikolaev, D.A.; Trikshev, A.I.; Tsvetkov, V.B.; Yakunin, V.P. Appearance of Collective Lasing of Laser Channels at the Intracavity Spectral Beam Combining. Opt. Commun. 2022, 506, 127591. [Google Scholar] [CrossRef]
- Desurvire, E. Erbium-Doped Fiber Amplifiers: Principles and Applications; John Wiley & Sons. Inc.: Hoboken, NJ, USA, 2002; ISBN 978-0-471-26434-7. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kamynin, V.A.; Kashin, V.V.; Nikolaev, D.A.; Tsvetkov, V.B. Implementation or Suppression of the Collective Lasing of the Laser Channels at the Intracavity Spectral Beam Combining. Photonics 2023, 10, 1022. https://doi.org/10.3390/photonics10091022
Kamynin VA, Kashin VV, Nikolaev DA, Tsvetkov VB. Implementation or Suppression of the Collective Lasing of the Laser Channels at the Intracavity Spectral Beam Combining. Photonics. 2023; 10(9):1022. https://doi.org/10.3390/photonics10091022
Chicago/Turabian StyleKamynin, Vladimir A., Vitalii V. Kashin, Dmitri A. Nikolaev, and Vladimir B. Tsvetkov. 2023. "Implementation or Suppression of the Collective Lasing of the Laser Channels at the Intracavity Spectral Beam Combining" Photonics 10, no. 9: 1022. https://doi.org/10.3390/photonics10091022
APA StyleKamynin, V. A., Kashin, V. V., Nikolaev, D. A., & Tsvetkov, V. B. (2023). Implementation or Suppression of the Collective Lasing of the Laser Channels at the Intracavity Spectral Beam Combining. Photonics, 10(9), 1022. https://doi.org/10.3390/photonics10091022



