Partially Coherent Cylindrical Vector Sources
Abstract
1. Introduction
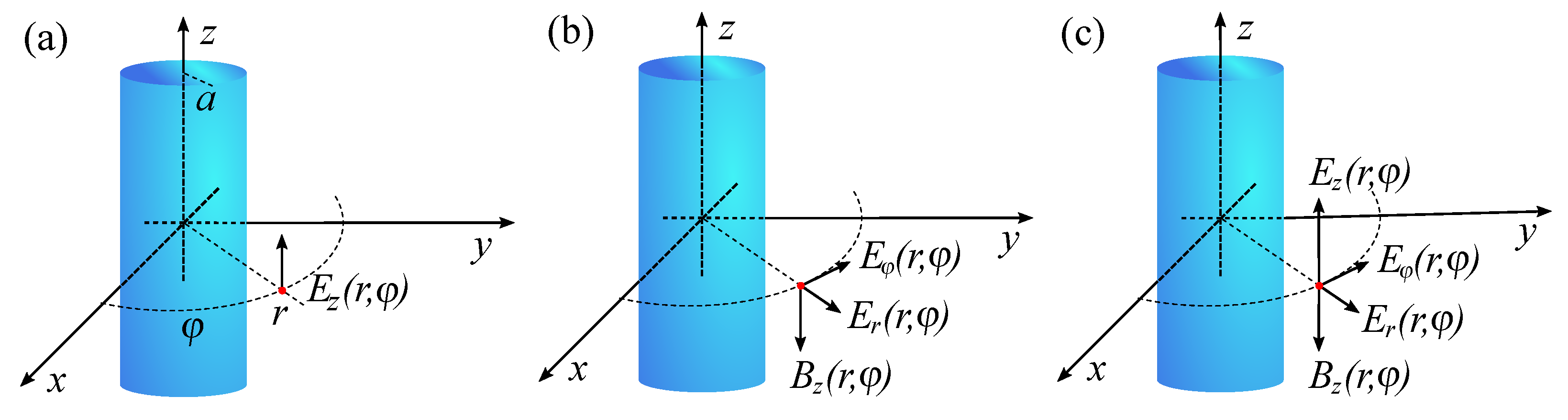
2. Preliminaries
3. The Basis Functions
4. Evolution of the Expansion Coefficients
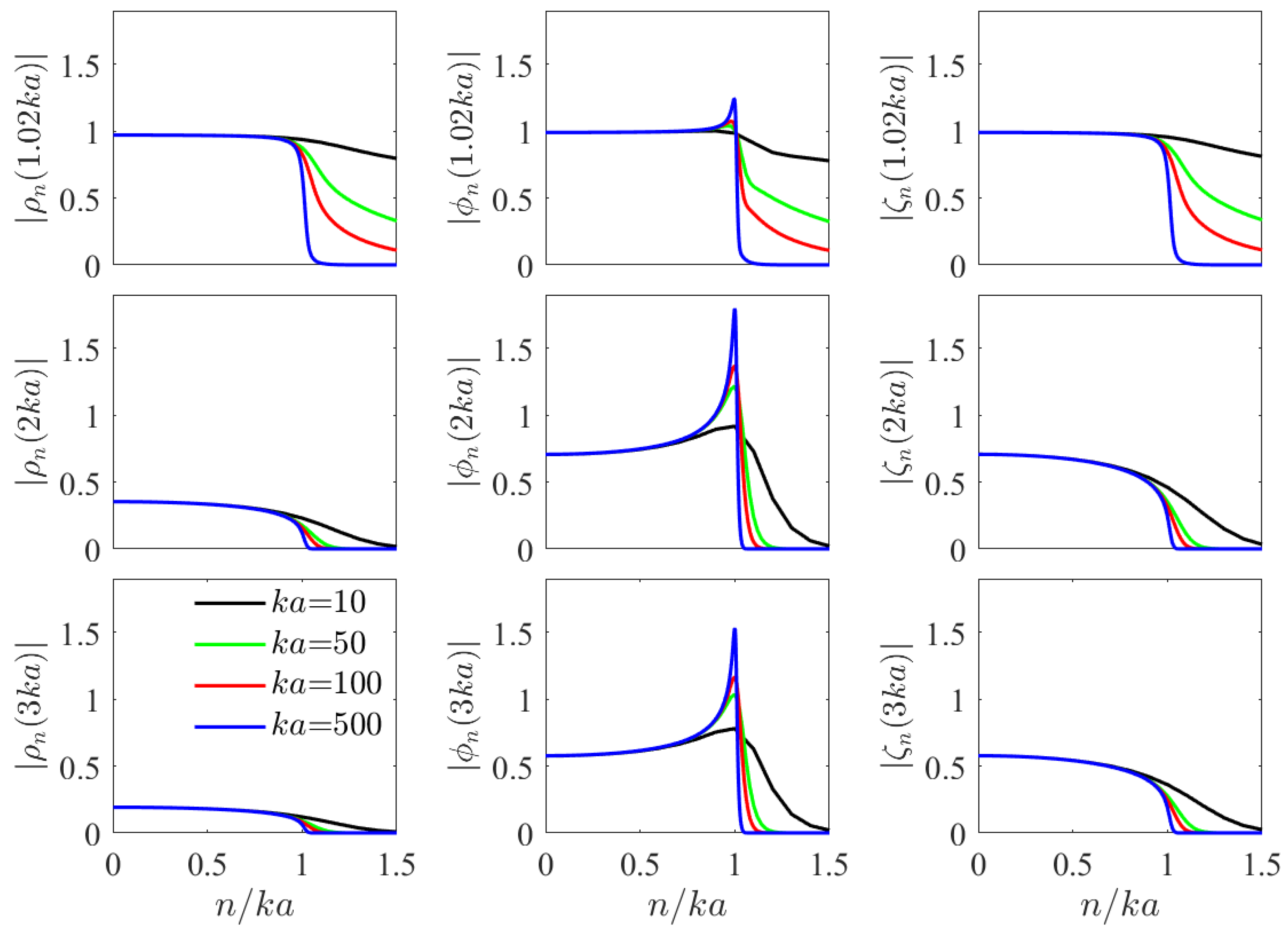
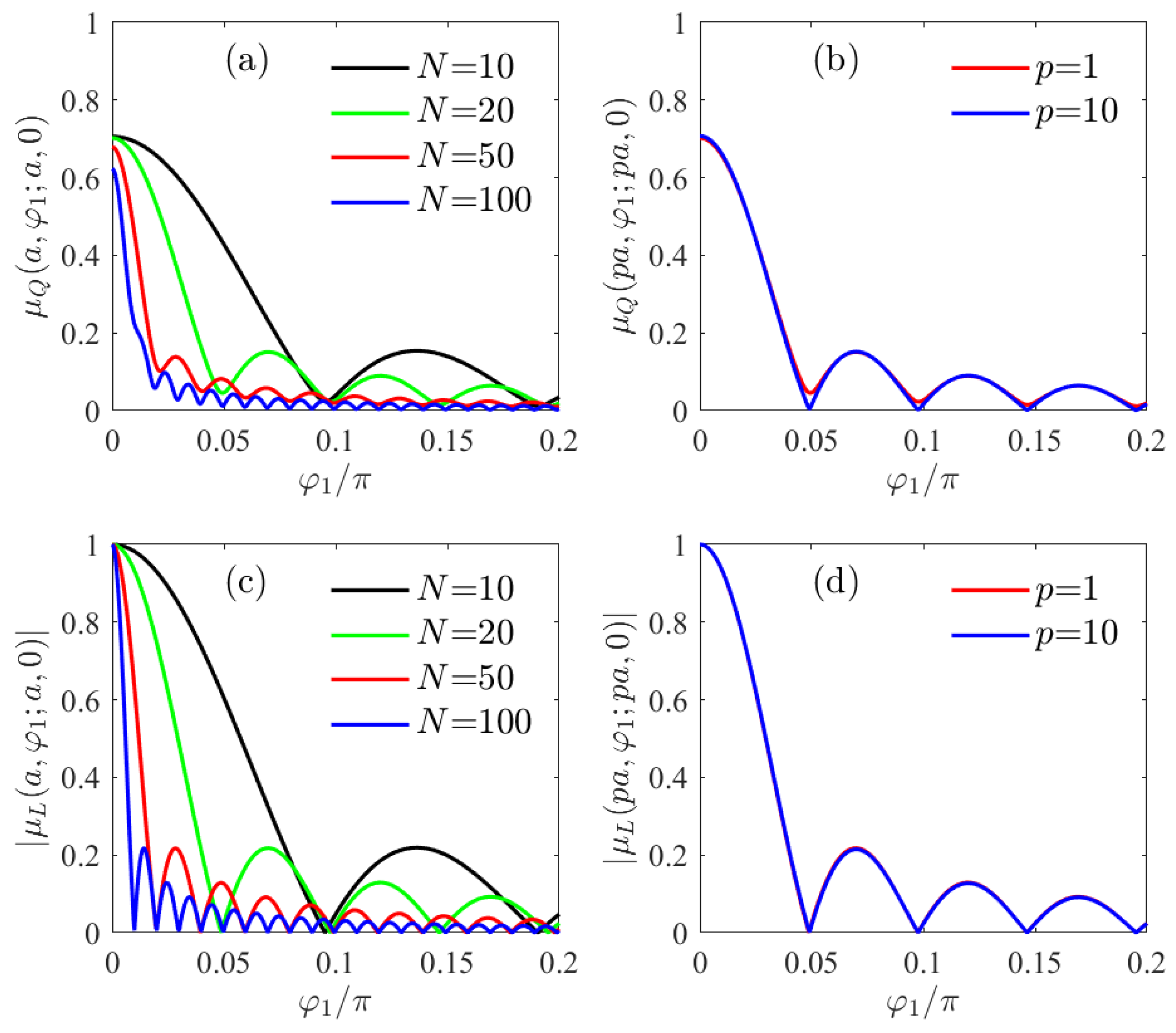
5. The Basis Functions as Coherent Vector Modes
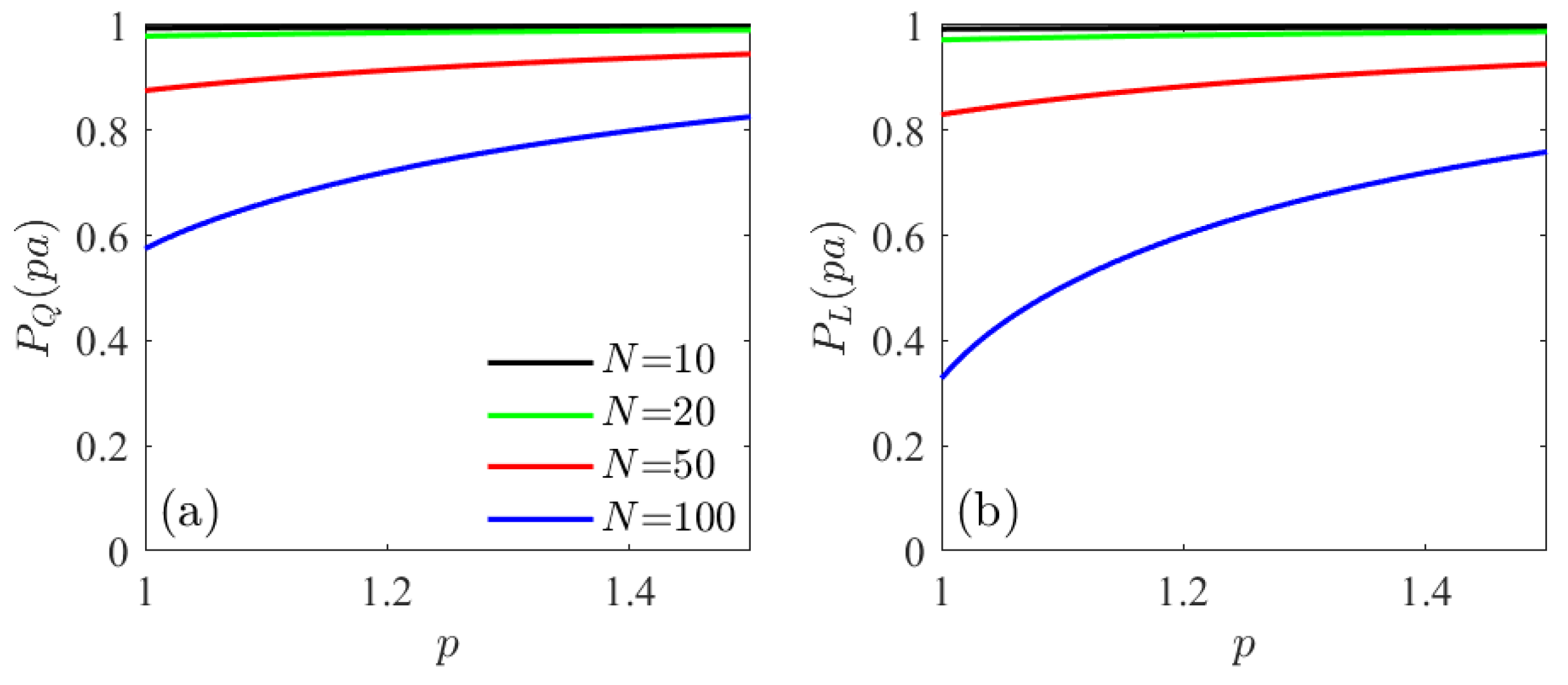
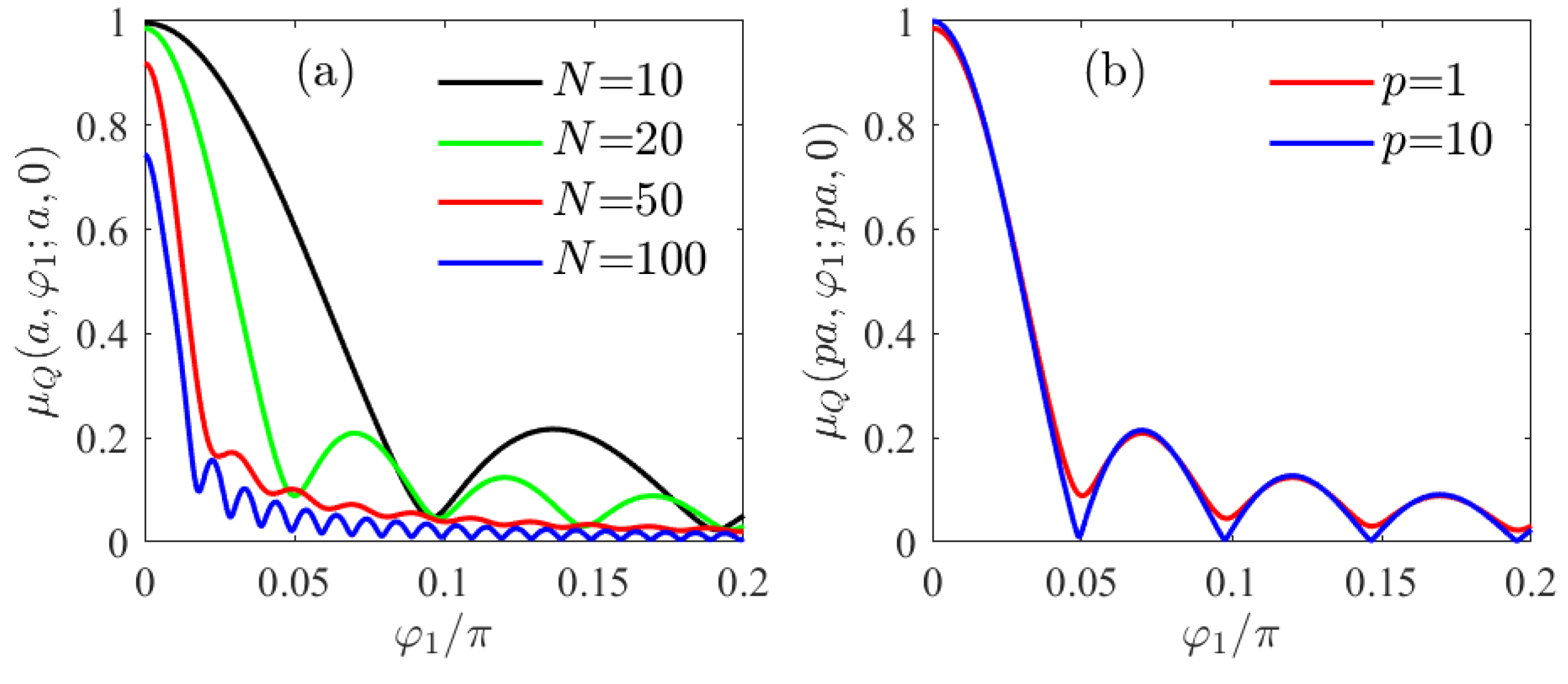
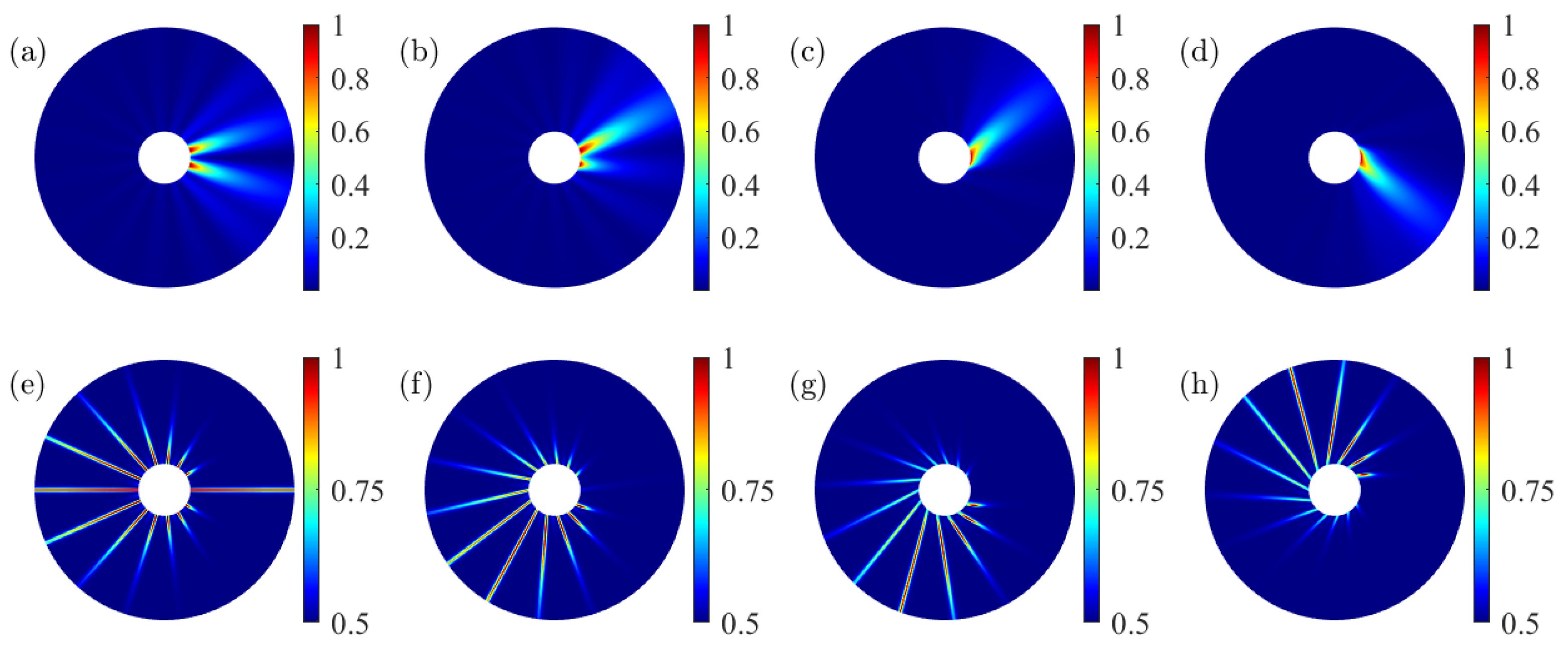
6. Discussion
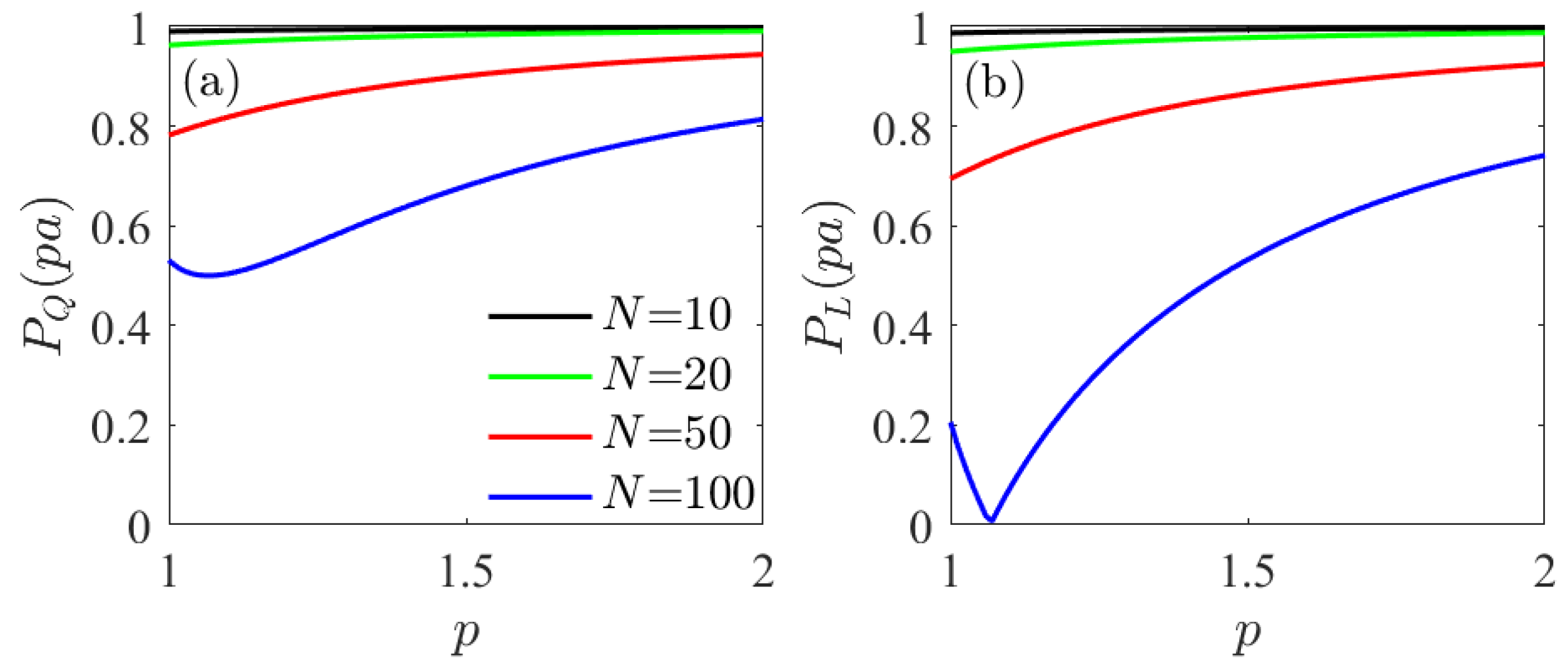
6.1. E-Polarization
6.2. H-Polarization
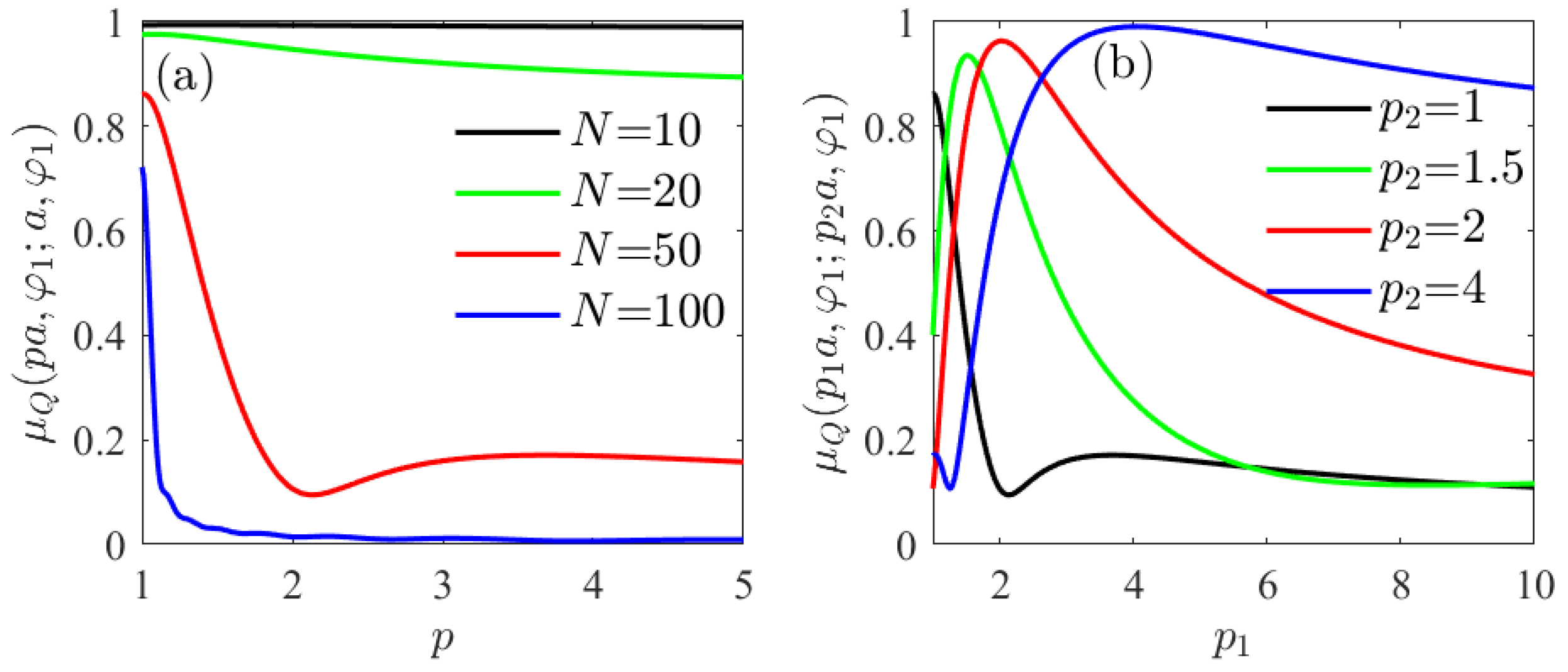
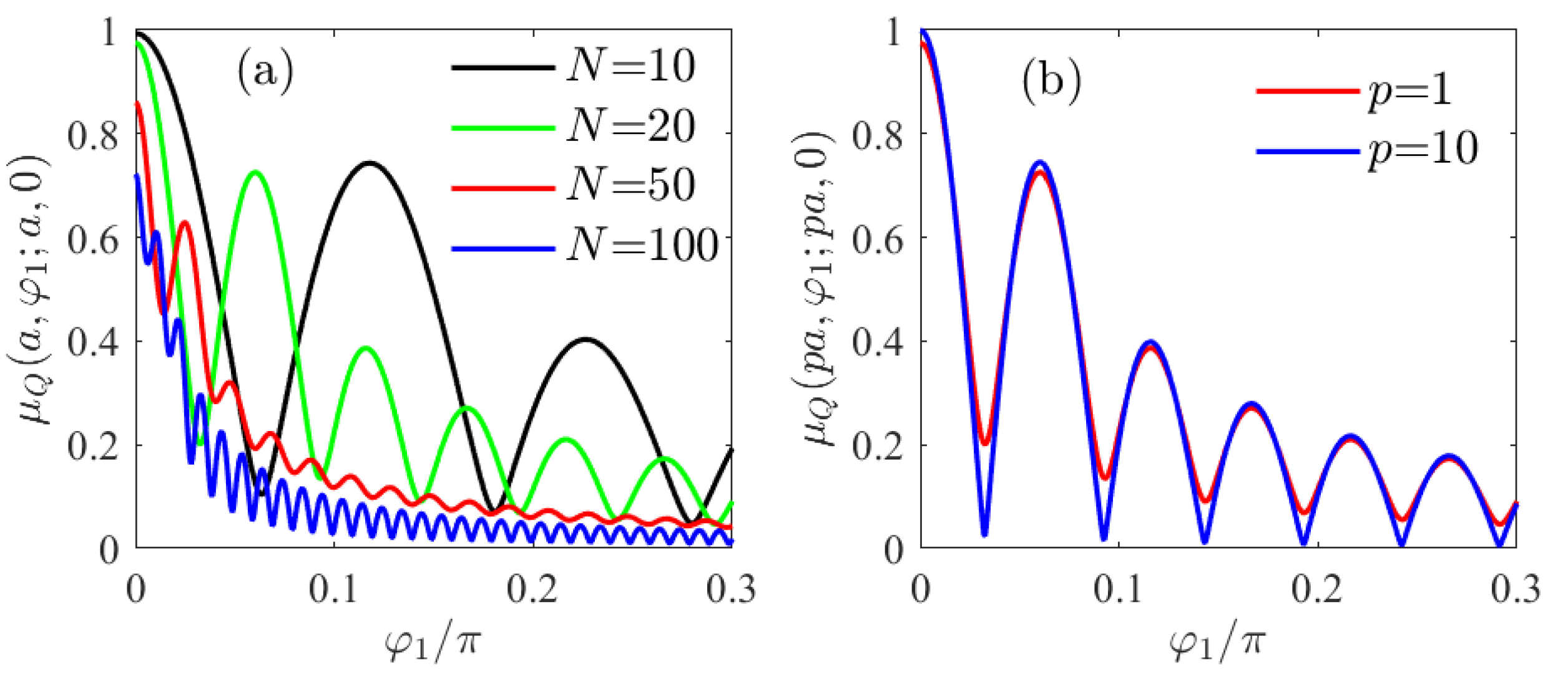
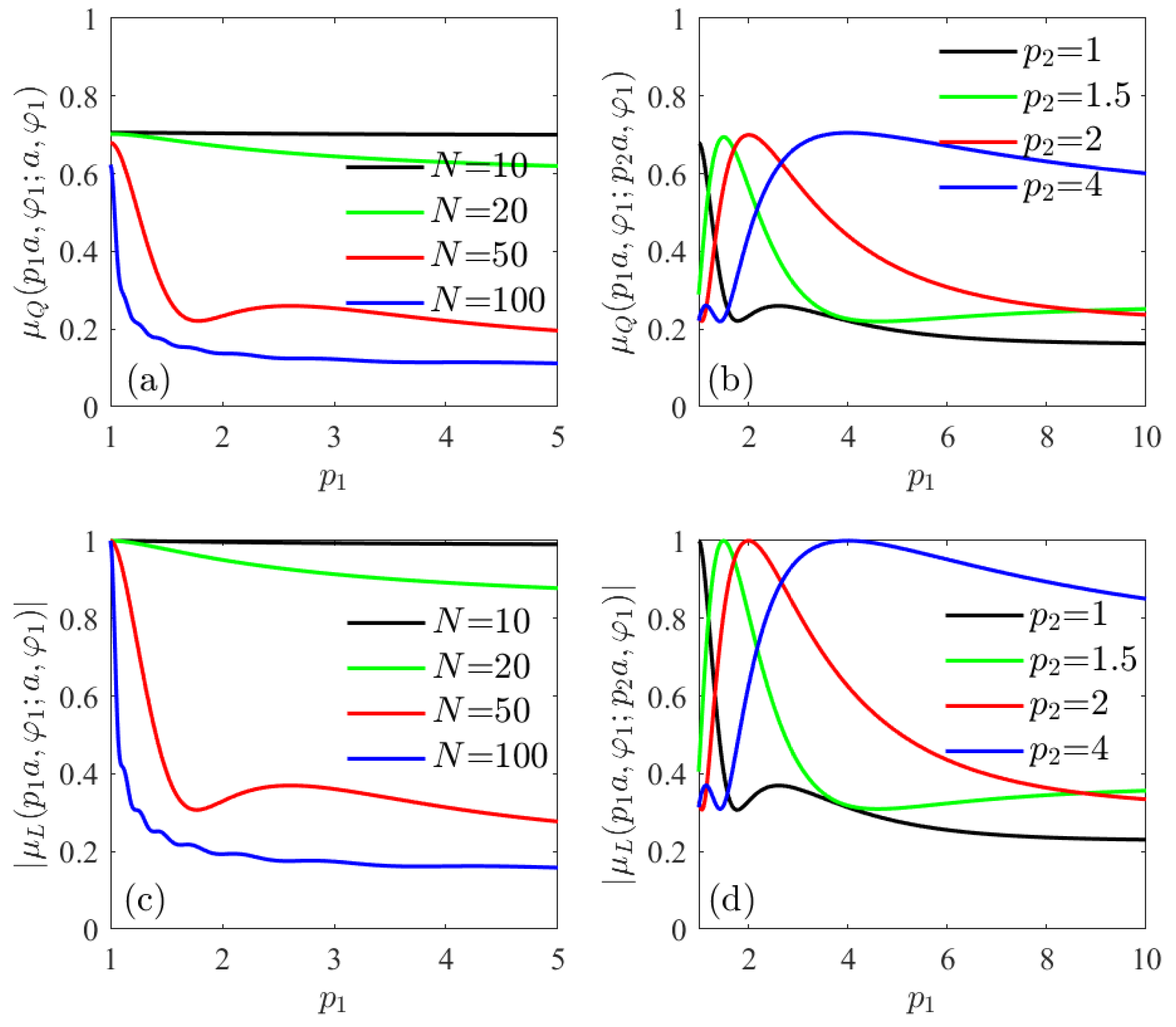
6.3. E- and H-Polarization
6.4. Mutually Correlated Modes
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| CSD | Cross-spectral density |
| DOP | Degree of polarization |
References
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar] [CrossRef]
- James, D.F.V. Change of polarization of light beams on propagation in free space. J. Opt. Soc. Am. A 1994, 11, 1641–1643. [Google Scholar] [CrossRef]
- Gori, F.; Santarsiero, M.; Vicalvi, S.; Borghi, R.; Guattari, G. Beam coherence-polarization matrix. Pure Appl. Opt. J. Eur. Opt. Soc. Part A 1998, 7, 941. [Google Scholar] [CrossRef]
- Tervo, J. Azimuthal polarization and partial coherence. J. Opt. Soc. Am. A 2003, 20, 1974–1980. [Google Scholar] [CrossRef]
- Ramírez-Sánchez, V.; Piquero, G.; Santarsiero, M. Synthesis and characterization of partially coherent beams with propagation-invariant transverse polarization pattern. Opt. Commun. 2010, 283, 4484–4489. [Google Scholar] [CrossRef]
- Santarsiero, M.; Ramírez-Sánchez, V.; Borghi, R. Partially correlated thin annular sources: The vectorial case. J. Opt. Soc. Am. A 2010, 27, 1450–1456. [Google Scholar] [CrossRef] [PubMed]
- Guo, L.; Tang, Z.; Liang, C.; Tan, Z. Intensity and spatial correlation properties of tightly focused partially coherent radially polarized vortex beams. Opt. Laser Technol. 2011, 43, 895–898. [Google Scholar] [CrossRef]
- de Sande, J.C.G.; Santarsiero, M.; Piquero, G.; Gori, F. Longitudinal polarization periodicity of unpolarized light passing through a double wedge depolarizer. Opt. Express 2012, 20, 27348–27360. [Google Scholar] [CrossRef]
- Santarsiero, M.; de Sande, J.C.G.; Piquero, G.; Gori, F. Coherence-polarization properties of fields radiated from transversely periodic electromagnetic sources. J. Opt. 2013, 15, 055701. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, F.; Liu, L.; Zhao, C.; Cai, Y.; Korotkova, O. Generation and propagation of a partially coherent vector beam with special correlation functions. Phys. Rev. A 2014, 89, 013801. [Google Scholar] [CrossRef]
- Mei, Z.; Korotkova, O. Electromagnetic Schell-model sources generating far fields with stable and flexible concentric rings profiles. Opt. Express 2016, 24, 5572–5583. [Google Scholar] [CrossRef]
- Xu, H.F.; Zhou, Y.; Wu, H.W.; Chen, H.J.; Sheng, Z.Q.; Qu, J. Focus shaping of the radially polarized Laguerre–Gaussian-correlated Schell-model vortex beams. Opt. Express 2018, 26, 20076–20088. [Google Scholar] [CrossRef] [PubMed]
- Senthilkumar, M.; Rajesh, K.; Udhayakumar, M.; Jaroszewicz, Z.; Mahadevan, G. Focusing properties of spirally polarized sinh Gaussian beam. Opt. Laser Technol. 2019, 111, 623–628. [Google Scholar] [CrossRef]
- Hyde, M.W.; Xiao, X.; Voelz, D.G. Generating electromagnetic nonuniformly correlated beams. Opt. Lett. 2019, 44, 5719–5722. [Google Scholar] [CrossRef] [PubMed]
- Yu, J.; Zhu, X.; Lin, S.; Wang, F.; Gbur, G.; Cai, Y. Vector partially coherent beams with prescribed non-uniform correlation structure. Opt. Lett. 2020, 45, 3824–3827. [Google Scholar] [CrossRef] [PubMed]
- Hyde IV, M.W. Synthesizing General Electromagnetic Partially Coherent Sources from Random, Correlated Complex Screens. Optics 2020, 1, 8. [Google Scholar] [CrossRef]
- Tong, R.; Dong, Z.; Chen, Y.; Wang, F.; Cai, Y.; Setälä, T. Fast calculation of tightly focused random electromagnetic beams: Controlling the focal field by spatial coherence. Opt. Express 2020, 28, 9713–9727. [Google Scholar] [CrossRef]
- Zhu, X.; Yu, J.; Wang, F.; Chen, Y.; Cai, Y.; Korotkova, O. Synthesis of vector nonuniformly correlated light beams by a single digital mirror device. Opt. Lett. 2021, 46, 2996–2999. [Google Scholar] [CrossRef]
- Martínez-Herrero, R.; Piquero, G.; Santarsiero, M.; Gori, F.; González de Sande, J.C. A class of vectorial pseudo-Schell model sources with structured coherence and polarization. Opt. Laser Technol. 2022, 152, 108079. [Google Scholar] [CrossRef]
- Agarwal, G.S.; Gbur, G.; Wolf, E. Coherence properties of sunlight. Opt. Lett. 2004, 29, 459–461. [Google Scholar] [CrossRef]
- Gori, F.; Korotkova, O. Modal expansion for spherical homogeneous sources. Opt. Commun. 2009, 282, 3859–3861. [Google Scholar] [CrossRef]
- de Sande, J.C.G.; Korotkova, O.; Martínez-Herrero, R.; Santarsiero, M.; Piquero, G.; Failla, A.V.; Gori, F. Partially coherent spherical sources with spherical harmonic modes. J. Opt. Soc. Am. A 2022, 39, C21–C28. [Google Scholar] [CrossRef]
- Martínez-Herrero, R.; Korotkova, O.; Santarsiero, M.; Piquero, G.; de Sande, J.C.G.; Failla, A.V.; Gori, F. Cylindrical partially coherent scalar sources. Opt. Lett. 2022, 47, 5224–5227. [Google Scholar] [CrossRef] [PubMed]
- Santarsiero, M.; Sande, J.C.G.D.; Korotkova, O.; Martínez-Herrero, R.; Piquero, G.; Gori, F. Three-dimensional polarization of fields radiated by partially coherent electromagnetic cylindrical sources. Opt. Lett. 2023, 48, 2476–2479. [Google Scholar] [CrossRef] [PubMed]
- Hyde, M.W.; Bogle, A.E.; Havrilla, M.J. Scattering of a partially-coherent wave from a material circular cylinder. Opt. Express 2013, 21, 32327–32339. [Google Scholar] [CrossRef]
- Petrov, E.Y.; Kudrin, A.V. Exact Axisymmetric Solutions of the Maxwell Equations in a Nonlinear Nondispersive Medium. Phys. Rev. Lett. 2010, 104, 190404. [Google Scholar] [CrossRef]
- Xiong, H.; Si, L.G.; Huang, P.; Yang, X. Analytic description of cylindrical electromagnetic wave propagation in an inhomogeneous nonlinear and nondispersive medium. Phys. Rev. E 2010, 82, 057602. [Google Scholar] [CrossRef] [PubMed]
- Panofsky, W.K.H.; Philips, M. Classical Electricity and Magnetism; Addison-Wesley: Mineola, NY, USA, 1962. [Google Scholar]
- Gbur, G.J. Mathematical Methods for Optical Physics and Engineering; Cambridge University Press: New York, NY, USA, 2011. [Google Scholar]
- Arfken, G.B.; Weberr, H.J. Mathematical Methods for Physicists, 6th ed.; Elsevier Academic Press: New York, NY, USA, 2005. [Google Scholar]
- Setälä, T.; Tervo, J.; Friberg, A.T. Complete electromagnetic coherence in the space–frequency domain. Opt. Lett. 2004, 29, 328–330. [Google Scholar] [CrossRef]
- Wolf, E. Introduction to the Theory of Coherence and Polarization of Light; Cambridge University Press: Cambridge, UK, 2007; p. 222. [Google Scholar]
- Setälä, T.; Shevchenko, A.; Kaivola, M.; Friberg, A.T. Degree of polarization for optical near fields. Phys. Rev. E 2002, 66, 016615. [Google Scholar] [CrossRef]
- Ellis, J.; Dogariu, A.; Ponomarenko, S.; Wolf, E. Degree of polarization of statistically stationary electromagnetic fields. Opt. Commun. 2005, 248, 333–337. [Google Scholar] [CrossRef]
- Auñón, J.M.; Nieto-Vesperinas, M. On two definitions of the three-dimensional degree of polarization in the near field of statistically homogeneous partially coherent sources. Opt. Lett. 2013, 38, 58–60. [Google Scholar] [CrossRef]
- Luis, A. Degree of polarization for three-dimensional fields as a distance between correlation matrices. Opt. Commun. 2005, 253, 10–14. [Google Scholar] [CrossRef]
- Gil, J.J.; Ossikovski, R. Polarized Light and the Mueller Matrix Approach; CRC Press Taylor & Francis Group: Boca Raton, FL, USA, 2016. [Google Scholar] [CrossRef]
- Tervo, J.; Setälä, T.; Friberg, A.T. Degree of coherence for electromagnetic fields. Opt. Express 2003, 11, 1137–1143. [Google Scholar] [CrossRef]
- Korotkova, O.; Wolf, E. Spectral degree of coherence of a random three-dimensional electromagnetic field. J. Opt. Soc. Am. A 2004, 21, 2382–2385. [Google Scholar] [CrossRef]
- Gori, F.; Santarsiero, M.; Borghi, R. Maximizing Young’s fringe visibility through reversible optical transformations. Opt. Lett. 2007, 32, 588–590. [Google Scholar] [CrossRef]
- Martínez-Herrero, R.; Mejías, P.M. Maximum visibility under unitary transformations in two-pinhole interference for electromagnetic fields. Opt. Lett. 2007, 32, 1471–1473. [Google Scholar] [CrossRef] [PubMed]
- Luis, A. Degree of coherence for vectorial electromagnetic fields as the distance between correlation matrices. J. Opt. Soc. Am. A 2007, 24, 1063–1068. [Google Scholar] [CrossRef]
- Martínez-Herrero, R.; Mejías, P.M.; Piquero, G. Characterization of Partially Polarized Light Fields; Springer Series in Optical Science; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Erdelyi, A. Higher Trascendental Functions; McGraw-Hill: New York, NY, USA, 1953; Vollume II. [Google Scholar]
- Borghi, R.; Gori, F.; Korotkova, O.; Santarsiero, M. Propagation of cross-spectral densities from spherical sources. Opt. Lett. 2012, 37, 3183–3185. [Google Scholar] [CrossRef]
- Gori, F.; Santarsiero, M.; Simon, R.; Piquero, G.; Borghi, R.; Guattari, G. Coherent-mode decomposition of partially polarized, partially coherent sources. J. Opt. Soc. Am. A 2003, 20, 78–84. [Google Scholar] [CrossRef]
- Tervo, J.; Setälä, T.; Friberg, A.T. Theory of partially coherent electromagnetic fields in the space–frequency domain. J. Opt. Soc. Am. A 2004, 21, 2205–2215. [Google Scholar] [CrossRef] [PubMed]
- Korotkova, O.; Chen, X. Phase structuring of the complex degree of coherence. Opt. Lett. 2018, 43, 4727–4730. [Google Scholar] [CrossRef] [PubMed]
- Korotkova, O.; Chen, X.; Setälä, T. Electromagnetic Schell-model beams with arbitrary complex correlation states. Opt. Lett. 2019, 44, 4945–4948. [Google Scholar] [CrossRef] [PubMed]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santarsiero, M.; de Sande, J.C.G.; Korotkova, O.; Martínez-Herrero, R.; Piquero, G.; Gori, F. Partially Coherent Cylindrical Vector Sources. Photonics 2023, 10, 831. https://doi.org/10.3390/photonics10070831
Santarsiero M, de Sande JCG, Korotkova O, Martínez-Herrero R, Piquero G, Gori F. Partially Coherent Cylindrical Vector Sources. Photonics. 2023; 10(7):831. https://doi.org/10.3390/photonics10070831
Chicago/Turabian StyleSantarsiero, Massimo, Juan Carlos González de Sande, Olga Korotkova, Rosario Martínez-Herrero, Gemma Piquero, and Franco Gori. 2023. "Partially Coherent Cylindrical Vector Sources" Photonics 10, no. 7: 831. https://doi.org/10.3390/photonics10070831
APA StyleSantarsiero, M., de Sande, J. C. G., Korotkova, O., Martínez-Herrero, R., Piquero, G., & Gori, F. (2023). Partially Coherent Cylindrical Vector Sources. Photonics, 10(7), 831. https://doi.org/10.3390/photonics10070831





