Design and Simulation Analysis of Piezoelectric Ceramic Tube-Based Fiber Optic Nutator Applied to an Intersatellite Laser Communication System
Abstract
1. Introduction
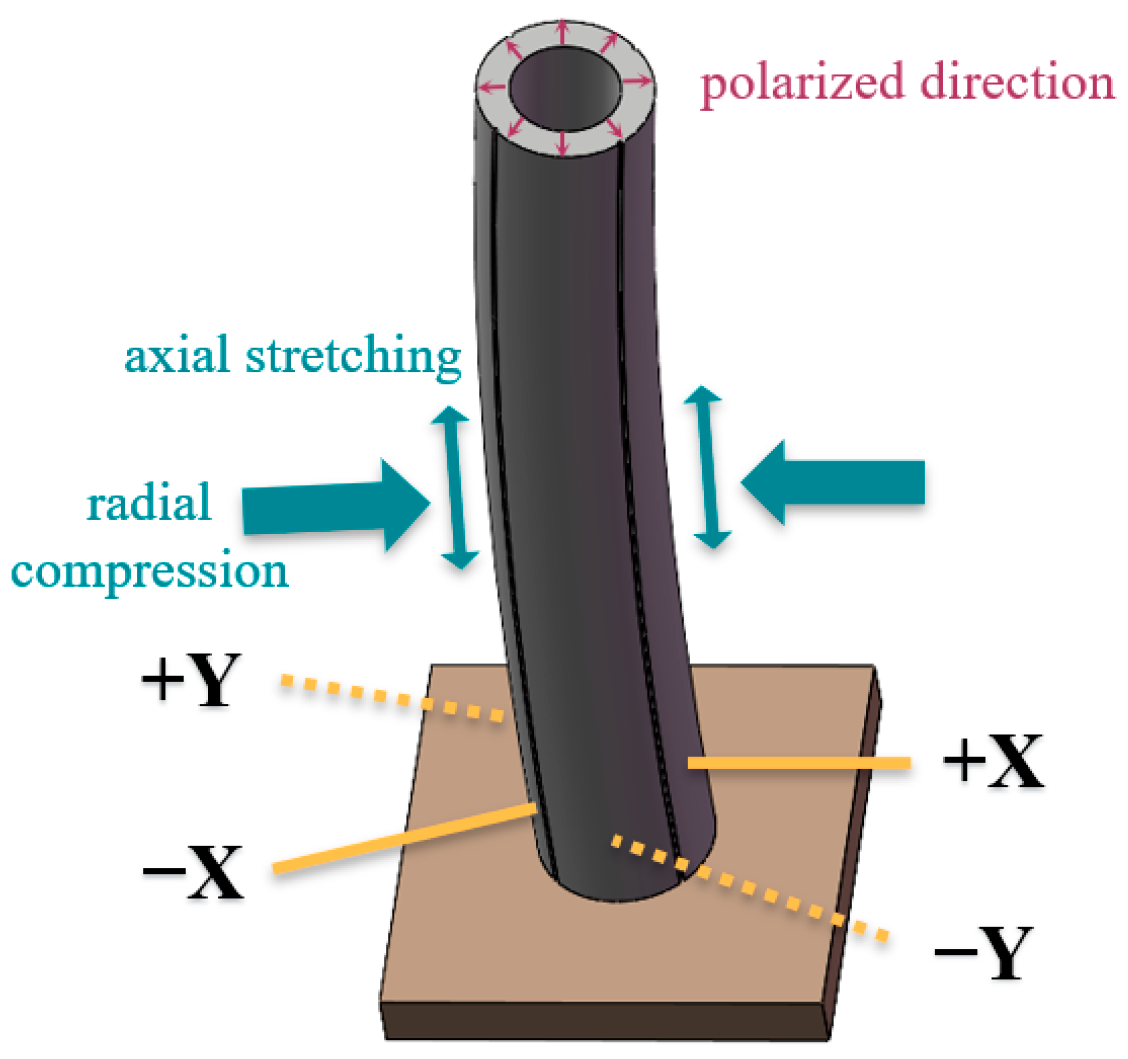
2. Structural Design of the PCT-Based Fiber Optic Nutator
2.1. Principle of Operation of PCT-Based Fiber Optic Nutator
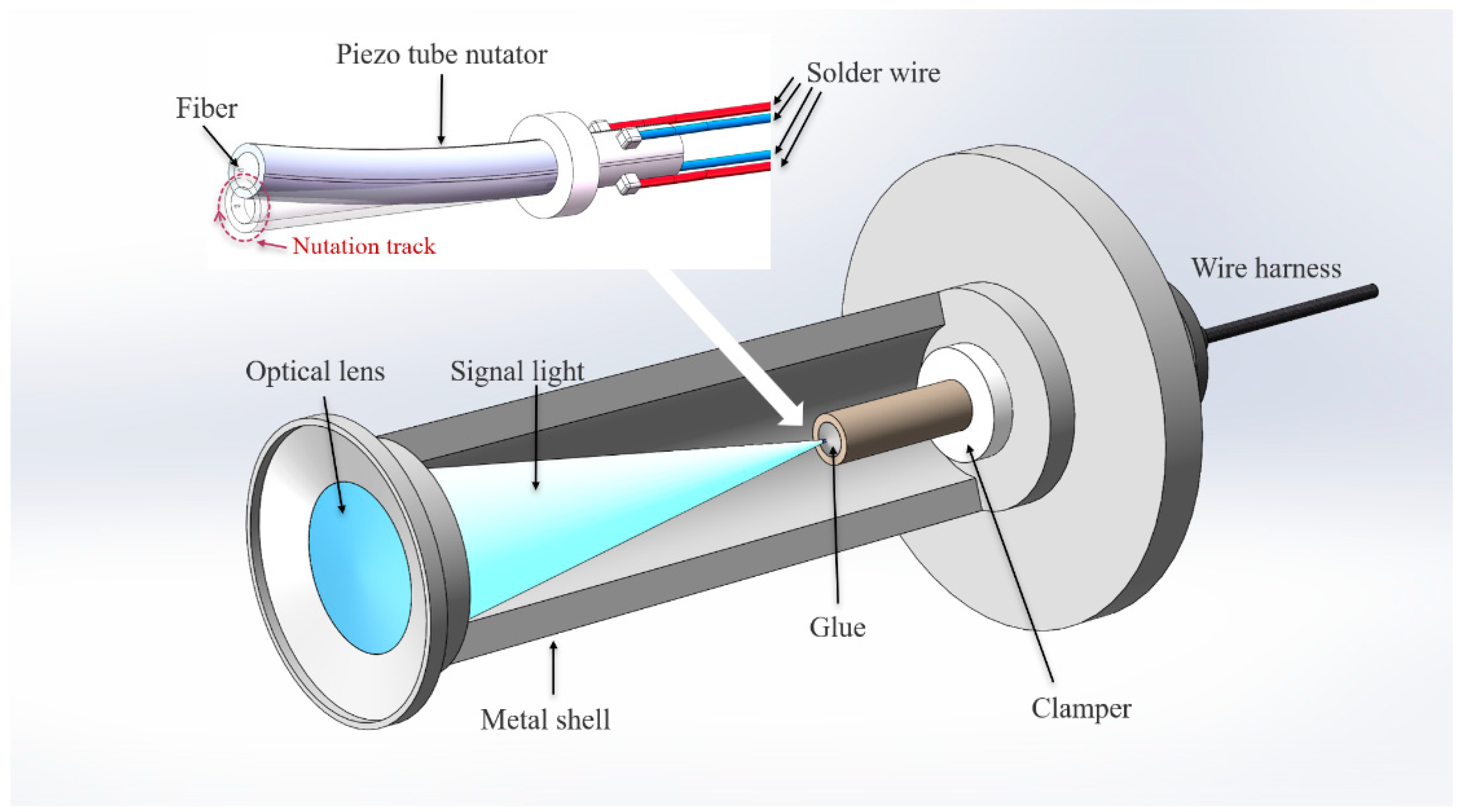
2.2. Mechanical Structural Design
3. Simulation and Experimental Verification of the PCT-Based Fiber Optic Nutator
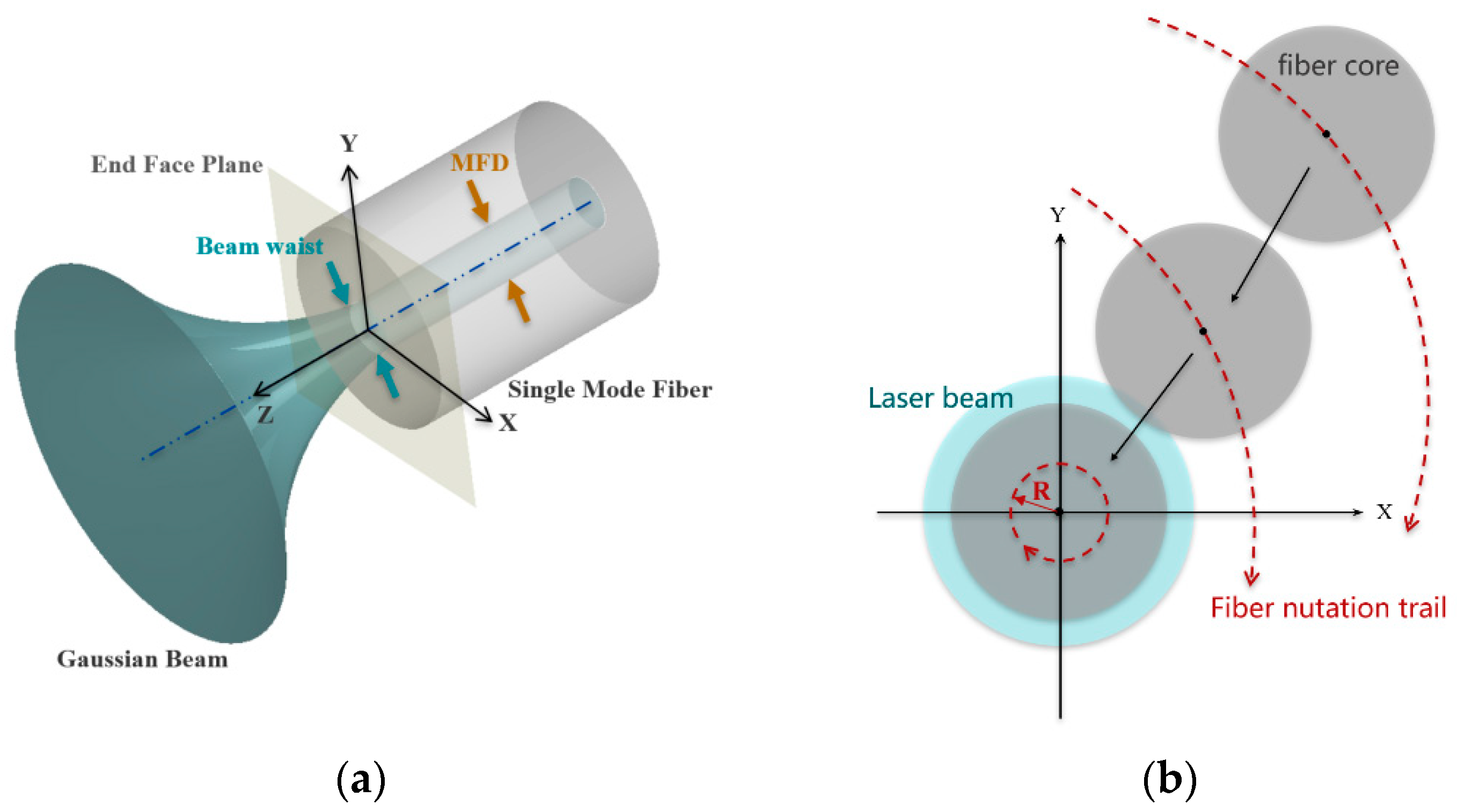
3.1. Simulation Theory of the PCT
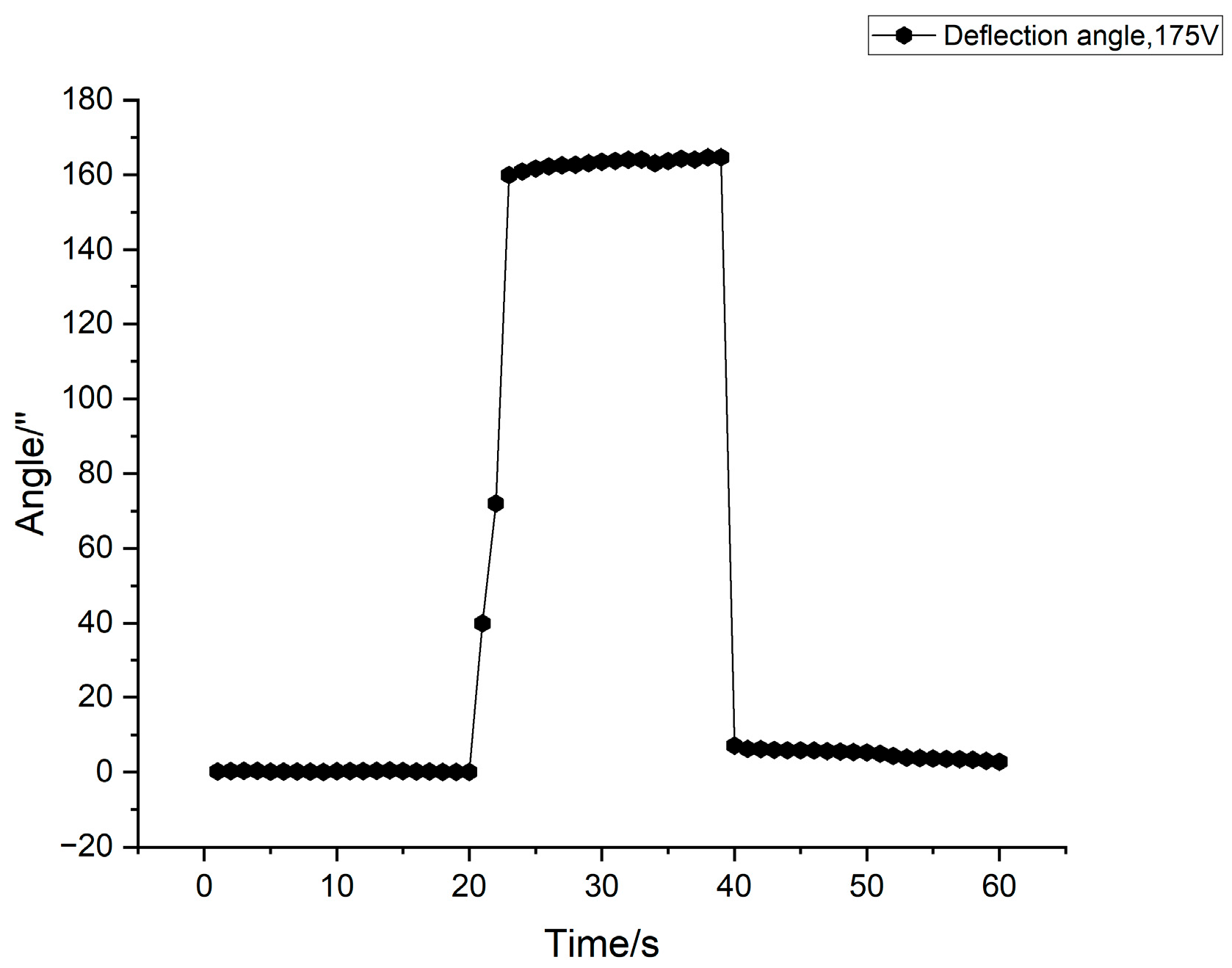
3.2. Experimental Verification of the Simulation Model
3.3. Simulation of Natural Frequency and Maximum Nutation Radius of the PCT-Based Fiber Optic Nutator
4. Design Parameter Criteria of the PCT-Based Fiber Optic Nutator
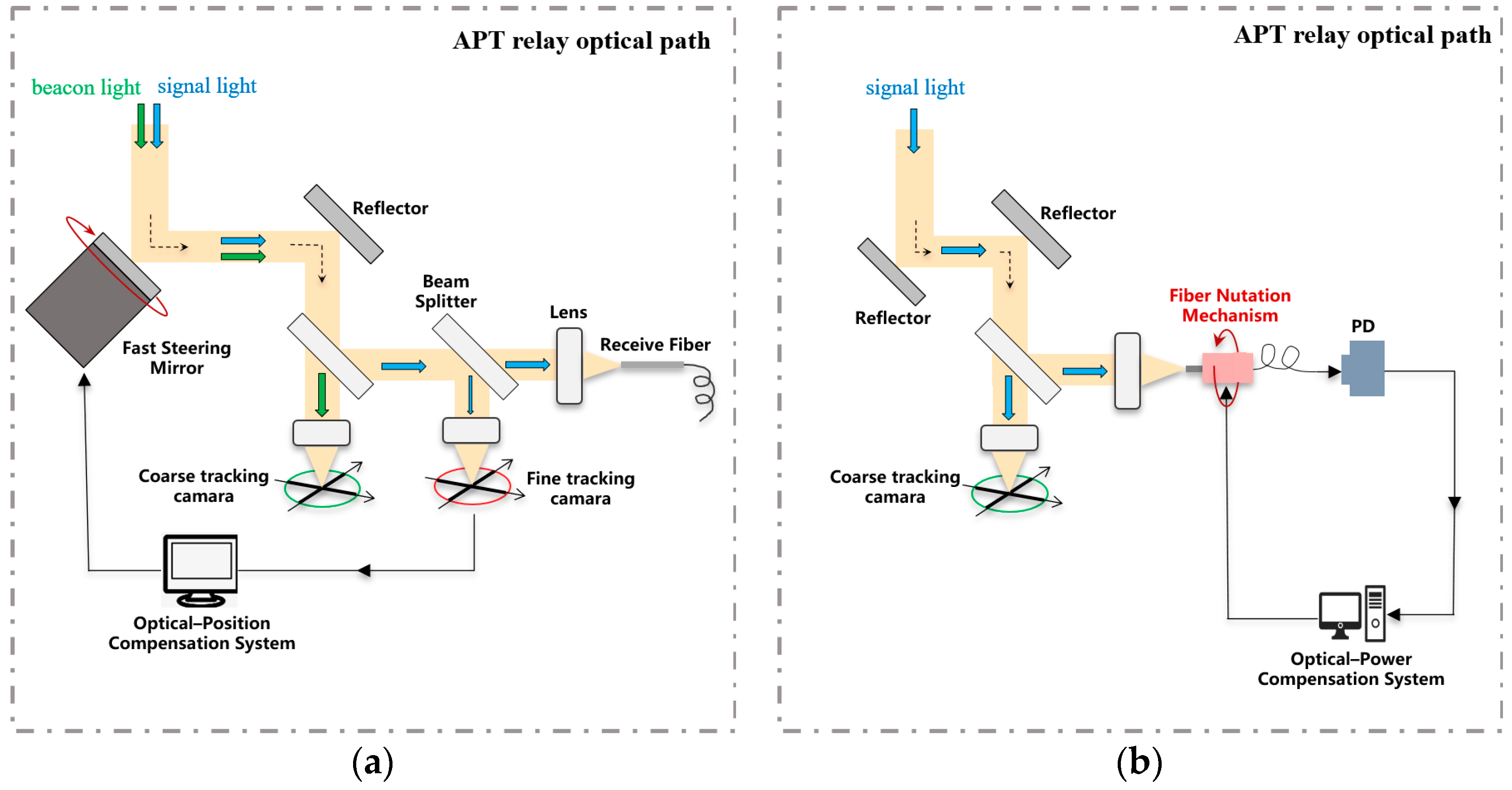
4.1. Design of the Relay Optical Path for the APT System
4.2. Design Parameters
5. Conclusions
- Based on the nutation principle of the PCT, the mechanical structure design process of the fiber optic nutator was described in detail, and the design scheme of the fiber optic nutator was presented.
- A finite element simulation model based on the PCT was established, and the theoretical analysis and simulation calculations were carried out to obtain the maximum chapter radius (R) of 20.09 μm, the minimum nutation accuracy (η) of 0.145 μm, and the maximum operating bandwidth (F) of 20 kHz for the fiber optic nutator. The 1st–6th order intrinsic frequencies of the mechanism were 2144.3, 2144.5, 2501.1, 2501.3, 10,481.0, and 11,345.0 Hz.
- PCT deflection angle measurement experiments based on a parallel light collimator at different steady-state voltages were designed, and the results were compared with the PCT deflection angle obtained by simulation under the same boundary conditions. The relative error between the final simulation and experimental results was <5%, which proved the accuracy of the PCT simulation model.
- A design for the relay optical path of the APT system with energy feedback compensation through the PCT-based fiber optic nutator was proposed, and the design parameters of the nutator based on the relay optical path of the APT system were calculated. Through comparison, all the structural parameters of the PCT-based fiber optic mechanism designed in this study met the requirements of the design parameters.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yamac, D.; Frederic, M.D. Fiber-coupling efficiency for free-space optical communication through atmospheric turbulence. Appl. Opt. 2005, 44, 4946–4952. [Google Scholar]
- Fidler, F.K.; Knapek, M.; Horwath, J.; Leeb, W.R. Optical communications for high-altitude platforms. IEEE J. Sel. Top. Quantum Electron. 2010, 16, 1058–1070. [Google Scholar] [CrossRef]
- Arnon, S.; Rotman, R.; Kopeika, N.S. The performance limitations of free space optical communication satellite networks due to vibrations-analog case. Opt. Eng. 1997, 36, 3148–3157. [Google Scholar] [CrossRef]
- Hu, D.J.J.; Liu, L.; Dong, H.; Zhang, H. Design of a broadband fiber optic mode coupler for multimode optical coherence tomography. Photonics 2023, 10, 162. [Google Scholar] [CrossRef]
- Song, W.; Xie, Y.; Hao, W.; Han, J.; Yan, P.; Li, X.; Wang, Y.; Li, X.; Sun, C.; Li, Z. Spatial coupling efficiency of collimators based on gradient-index lens with an angle polish. Opt. Laser Technol. 2023, 162, 109245. [Google Scholar] [CrossRef]
- Wang, J.; Song, Y.; Jiang, H.; Dong, K.; Liu, Y. Prototype development of multi-target tracking system for space multi-node laser communication network. Optik 2023, 274, 170552. [Google Scholar] [CrossRef]
- Li, Z.; Liu, B.; Wang, H.; Chen, Z.; Zhang, Q.; Hua, K.; Yang, J. Target tracking and ranging based on single photon detection. Photonics 2021, 8, 278. [Google Scholar] [CrossRef]
- Zhang, F.; Han, J.; Ruan, P. Beam pointing analysis and a novel coarse pointing assembly design in space laser communication. Optik 2019, 189, 130–147. [Google Scholar] [CrossRef]
- Yu, S.; Wu, F.; Tan, L.; Ma, J. Static position errors correction on the satellite optical communication terminal. Opt. Eng. 2017, 56, 026112. [Google Scholar] [CrossRef]
- Heine, F.; Mühlnikel, G.; Zech, H.; Tröndle, D.; Seel, S.; Motzigemba, M.; Meyer, R.; Philipp-May, S.; Benzi, E. LCT for the European Data Relay System: In Orbit Commissioning of the Alphasat and Sentinel 1A LCTs. SPIE 2015, 9354, 133–138. [Google Scholar]
- Hu, S.; Yu, H.; Duan, Z.; Zhu, Y.; Cao, C.; Zhou, M.; Li, G.; Liu, H. Multi-parameter influenced acquisition model with an in-orbit jitter for inter-satellite laser communication of the LCES system. Opt. Express 2022, 30, 34362–34377. [Google Scholar] [CrossRef]
- Deng, K.; Wang, B.-Z.; Zhao, G.-H.; Huang, J.; Zhang, P.; Jiang, D.-G.; Yao, Z.-S. Principle and performance analysis of coherent tracking sensor based on local oscillator beam nutation. Opt. Express 2014, 22, 23528. [Google Scholar] [CrossRef] [PubMed]
- Geng, C.; Li, X.; Zhang, X.; Rao, C. Coherent beam combination of an optical array using adaptive fiber optics collimators. Opt. Commun. 2011, 284, 5531–5536. [Google Scholar] [CrossRef]
- Zhi, D.; Ma, Y.; Ma, P.; Si, L.; Wang, X.; Zhou, P. Adaptive fiber optics collimator based on flexible hinges. Appl. Opt. 2014, 53, 5434–5438. [Google Scholar] [CrossRef] [PubMed]
- Lachinova, S.L.; Vorontsov, M.A. Exotic laser beam engineering with coherent fiber-array systems. J. Opt. 2013, 15, 105501. [Google Scholar] [CrossRef]
- Zhang, L.; Yu, X.; Zhao, B.; Wang, T.; Tong, S. Method for 10 Gbps near-ground quasi-static free-space laser transmission by nutation mutual coupling. Opt. Express 2022, 30, 33465–33478. [Google Scholar] [CrossRef] [PubMed]
- Yan, X.; Cao, C.; Zhang, W.; Zeng, X.; Feng, Z.; Wu, Z.; Wang, T. Wavefront detection and compensation technology based on signal light nutation under atmospheric turbulence. IEEE Commun. Lett. 2021, 25, 3340–3344. [Google Scholar] [CrossRef]
- Swanson, E.A.; Bondurant, R.S. A Space-Based Optical Communication System Utilizing Fiber Optics; Technical Report; MIT Lincoln Laboratory: Lexington, MA, USA, 1989. [Google Scholar]
- Swanson, E.A.; Bondurant, R.S. Using fiber optics to simplify free-space lasercom systems. SPIE 1990, 1218, 70–82. [Google Scholar]
- Knibbe, T.E.; Swanson, E.A.; Roberge, J.K. Spatial tracking using an electro-optic nutator and a single-mode optical fiber. SPIE 1992, 1635, 309–317. [Google Scholar]
- Knibbe, T.E. Spatial Tracking Using an Electro-Optic Nutator and a Single-Mode Optical Fiber. Master’s Dissertation, Massachusetts Institute of Technology, Cambridge, MA, USA, 1993. [Google Scholar]
- Geng, C.; Luo, W.; Tan, Y.; Liu, H.; Mu, J.; Li, X. Experimental Demonstration of Using Divergence Cost-Function in SPGD Algorithm for Coherent Beam Combining with Tip/Tilt Control. Opt. Express 2013, 21, 25045. [Google Scholar] [CrossRef]
- Luo, W.; Geng, C.; Wu, Y.Y.; Tan, Y.; Luo, Q.; Liu, H.M.; Li, X.Y. Experimental demonstration of single-mode fiber coupling using adaptive fiber coupler. Chin. Phys. B 2014, 23, 014207. [Google Scholar] [CrossRef]
- Zhang, Q.M.; Wang, H.; Cross, L.E. Piezoelectric tubes and tubular composites for actuator and sensor applications. J. Mater. Sci. 1993, 28, 3962–3968. [Google Scholar] [CrossRef]
- Saito, Y.; Takao, H.; Tani, T.; Nonoyama, T.; Takatori, K.; Homma, T.; Nagaya, T.; Nakamura, M. Lead-free piezoceramics. Nature 2004, 432, 84–87. [Google Scholar] [CrossRef] [PubMed]
- Li, E.Q.; Xu, Q.; Sun, J.; Fuh, J.Y.H.; Wong, Y.S.; Thoroddsen, S.T. Design and fabrication of a PET/PTFE-based piezoelectric squeeze mode drop-on-demand inkjet printhead with interchangeable nozzle. Sens. Actuators A Phys. 2010, 163, 315–322. [Google Scholar] [CrossRef]
- Weibin, R.; Zhenguang, W.; Lining, S. Design and testing of one-dimensional nano-positioning stage with large travel range and high resolution. Nanotechnol. Precis. Eng. 2012, 10, 384–389. [Google Scholar]
- Fauzi, N.I.M.; Fen, Y.W.; Abdullah, J.; Kamarudin, M.A.; Omar, N.A.S.; Eddin, F.B.K.; Ramdzan, N.S.M.; Daniyal, W.M.E.M.M. Evaluation of structural and optical properties of graphene oxide-polyvinyl alcohol thin film and its potential for pesticide detection using an optical method. Photonics 2022, 9, 300. [Google Scholar] [CrossRef]
- Lee, C.M.; Engelbrecht, C.J.; Soper, T.D.; Helmchen, F.; Seibel, E.J. Scanning fiber endoscopy with highly flexible, 1 mm catheterscopes for wide-field, full-color imaging. J. Biophoton. 2010, 3, 385–407. [Google Scholar] [CrossRef]
- Pshenay-Severin, E.; Bae, H.; Reichwald, K.; Matz, G.; Bierlich, J.; Kobelke, J.; Lorenz, A.; Schwuchow, A.; Meyer-Zedler, T.; Schmitt, M.; et al. Multimodal nonlinear endomicroscopic imaging probe using a double-core double-clad fiber and focus-combining micro-optical concept. Light Sci. Appl. 2021, 10, 207. [Google Scholar] [CrossRef]
- Dreischer, T.; Maerki, A.; Weigel, T.; Baister, G.C.; Haupt, C.; Wehrle, K. Operating in sub-arc seconds: High-precision laser terminals for intersatellite communications. SPIE 2002, 4902, 87–98. [Google Scholar]
- Zhao, X.; Hou, X.; Zhu, F.; Li, T.; Sun, J.; Zhu, R.; Gao, M.; Yang, Y.; Chen, W. Experimental verification of coherent tracking system based on fiber nutation. Opt. Express 2019, 27, 23996. [Google Scholar]
- Wang, Z.L.; Wu, W. Piezotronics and piezo-phototronics: Fundamentals and applications. Natl. Sci. Rev. 2013, 1, 29. [Google Scholar] [CrossRef]
- Shang, Y.; Jiao, Z.; Wang, S.; Wang, X. Dynamic robust compensation control to inherent high-frequency motion disturbance on the electro-hydraulic load simulator. Int. J. Comput. Appl. Technol. 2009, 36, 117. [Google Scholar] [CrossRef]
- Drossel, W.G.; Hensel, S.; Kranz, B.; Nestler, M.; Goeschel, A. Sheet metal forming of piezoceramic–metal-laminar structures—Simulation and experimental analysis. CIRP Ann.-Manuf. Technol. 2009, 58, 279–282. [Google Scholar] [CrossRef]
- Wang, S.L.; Cao, W.H.; Fan, Y.J. The performance analysis and research of a new type of piezoelectric ceramic tube drivers based on ANASYS. Mater. Rep. 2012, 26, 154–157. [Google Scholar]
- Yang, M.M.; Wang, Y.E.; Wei, S.M. Modeling and simulation of radial polarized piezo tube. Electron. Compon. Mater. 2018, 37, 23–27+44. [Google Scholar]
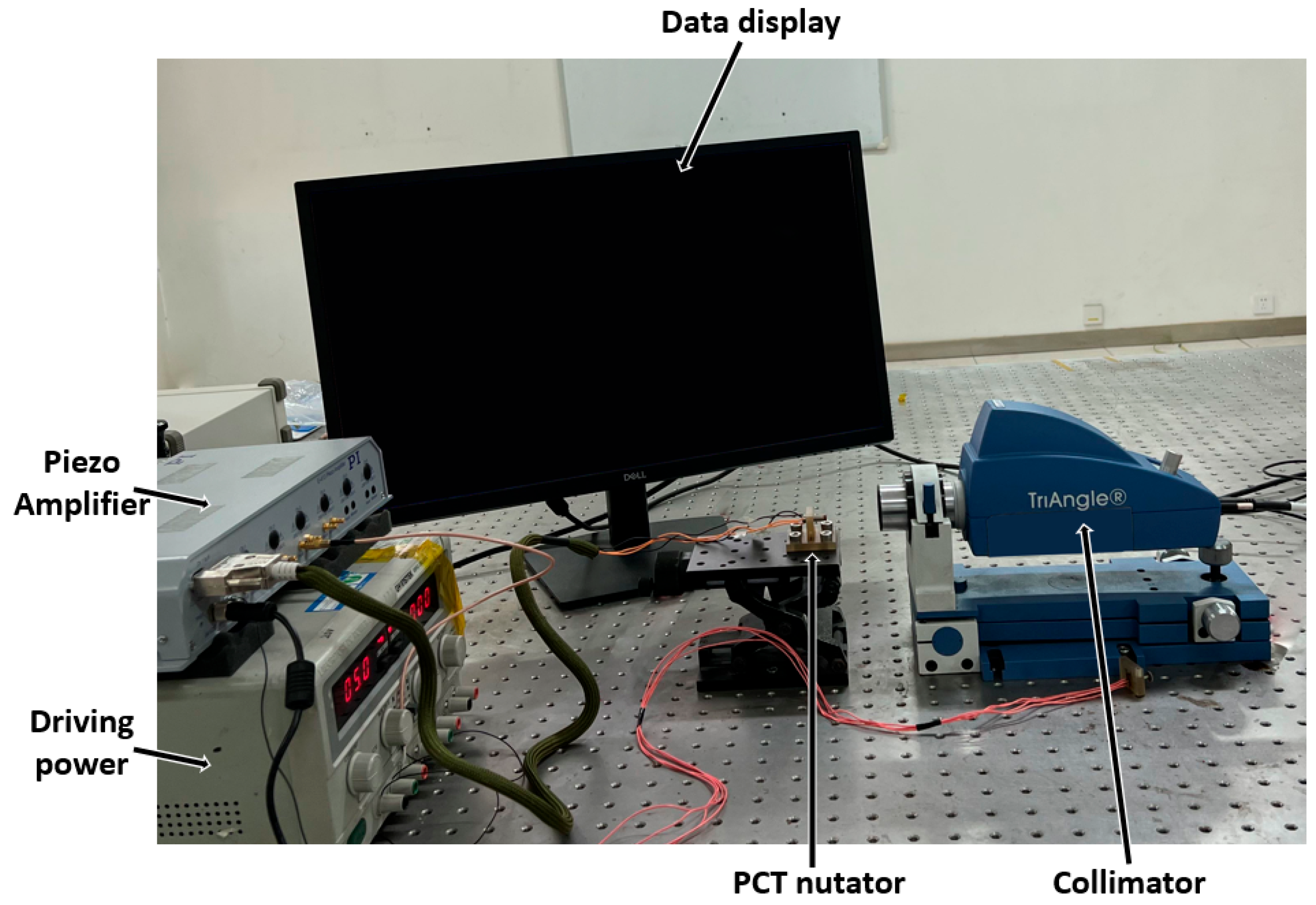





| Device | Type | Main Parameters |
|---|---|---|
| PCT | PI PT230.94 | 3.2 mm × 2.2 mm × 30 mm, PIC255 |
| Deflection mirror | YZ-GFSJP-X | Full band, 5 mm × 1.2 mm |
| Piezo amplifier | TriAngle TA100-38 | Input range: −10–10 V, gain: 25 times |
| Autocollimator | PI E-413.30 | Resolution: 1″, sampling interval: 1 s |
| Input power | GW GPC-30600 | Input range: −10–10 V, resolution: 0.1 V |
| Parameter Type | Symbol | Value |
|---|---|---|
| Signal wavelength | λ | 1556.15 nm |
| Mode-field radius of SMF | ω0 | 4.0 μm |
| Focal length of fine tracking optical system | fj | 2615.7 mm |
| Focal length of communication receiving branch | fb | 38.13 mm |
| Entry pupil diameter of fine tracking branch | D | 10 mm |
| Coarse tracking bandwidth | Fc | 10.01 Hz |
| Field angle of fine tracking branch | αj | 0.8 mrad |
| Maximum Nutation Radius R | Minimum Nutation Accuracy η | Maximum Bandwidth F | |
| Required design parameters | ≥15.25 μm | ≤0.91 μm | ≥359.69 Hz |
| Actual design parameters | 20.15 μm | 0.145 μm | 20 kHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, B.; Ruan, P.; Han, J.; Li, X.; Chang, Z.; Wang, Y.; Wang, X. Design and Simulation Analysis of Piezoelectric Ceramic Tube-Based Fiber Optic Nutator Applied to an Intersatellite Laser Communication System. Photonics 2023, 10, 769. https://doi.org/10.3390/photonics10070769
Peng B, Ruan P, Han J, Li X, Chang Z, Wang Y, Wang X. Design and Simulation Analysis of Piezoelectric Ceramic Tube-Based Fiber Optic Nutator Applied to an Intersatellite Laser Communication System. Photonics. 2023; 10(7):769. https://doi.org/10.3390/photonics10070769
Chicago/Turabian StylePeng, Bo, Ping Ruan, Junfeng Han, Xiangyu Li, Zhiyuan Chang, Yifan Wang, and Xuan Wang. 2023. "Design and Simulation Analysis of Piezoelectric Ceramic Tube-Based Fiber Optic Nutator Applied to an Intersatellite Laser Communication System" Photonics 10, no. 7: 769. https://doi.org/10.3390/photonics10070769
APA StylePeng, B., Ruan, P., Han, J., Li, X., Chang, Z., Wang, Y., & Wang, X. (2023). Design and Simulation Analysis of Piezoelectric Ceramic Tube-Based Fiber Optic Nutator Applied to an Intersatellite Laser Communication System. Photonics, 10(7), 769. https://doi.org/10.3390/photonics10070769





