Polarization Controller Based on Variable-Step Greedy Linear Descent for Self-Homodyne Coherent Transmission Systems
Abstract
1. Introduction
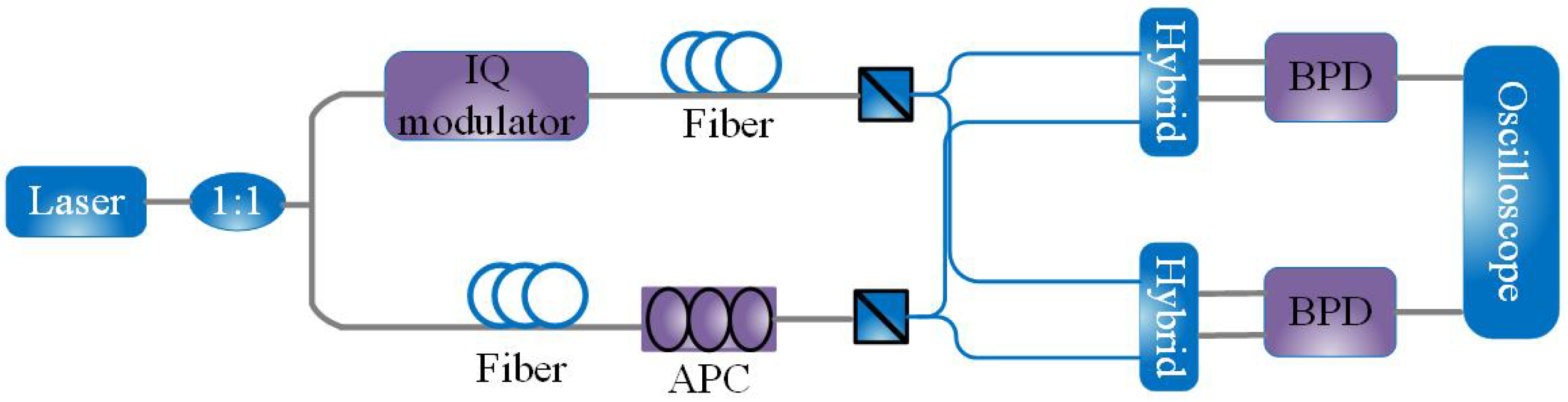
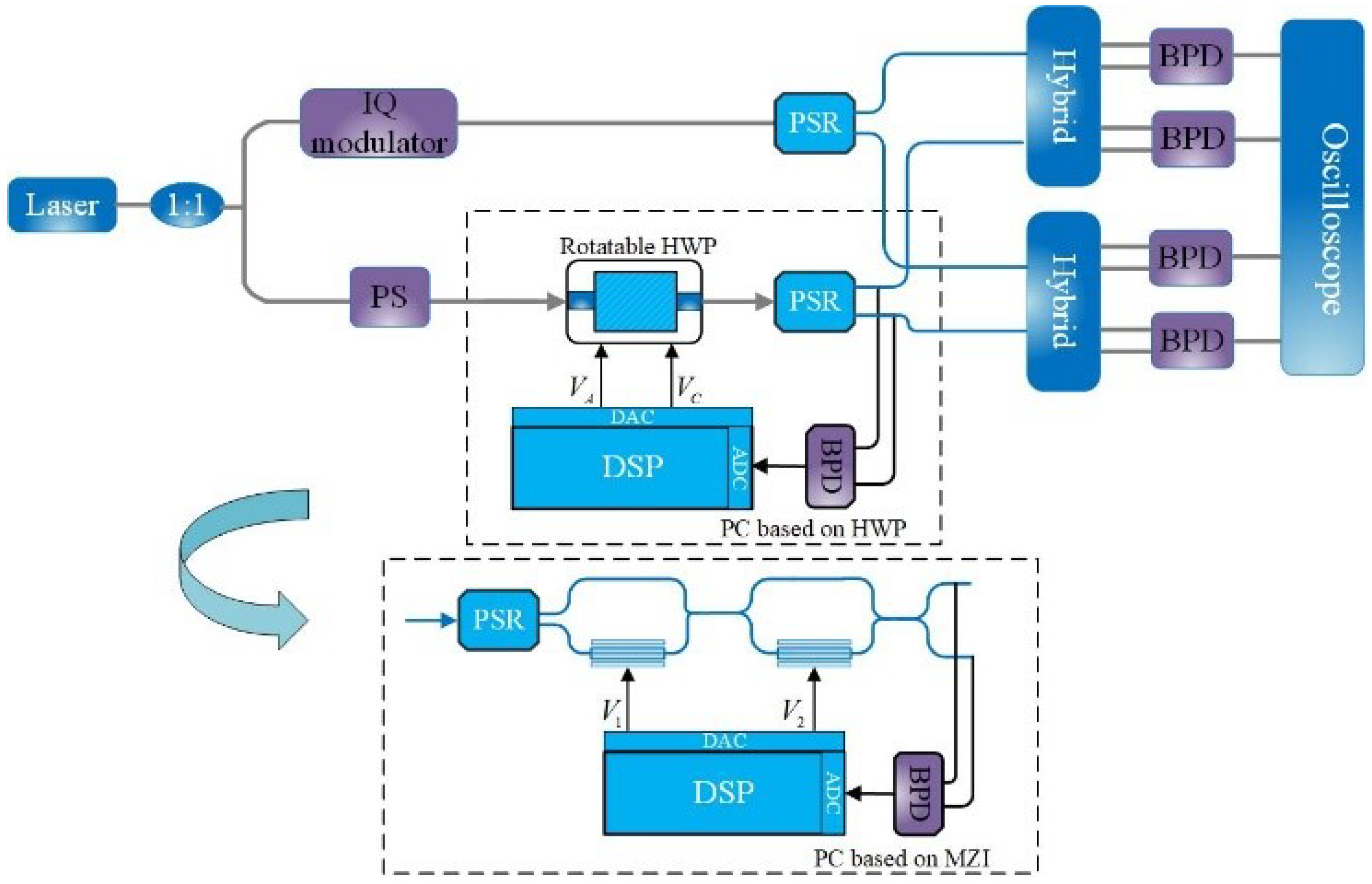
2. Polarization Control Structure Furthermore, Principle
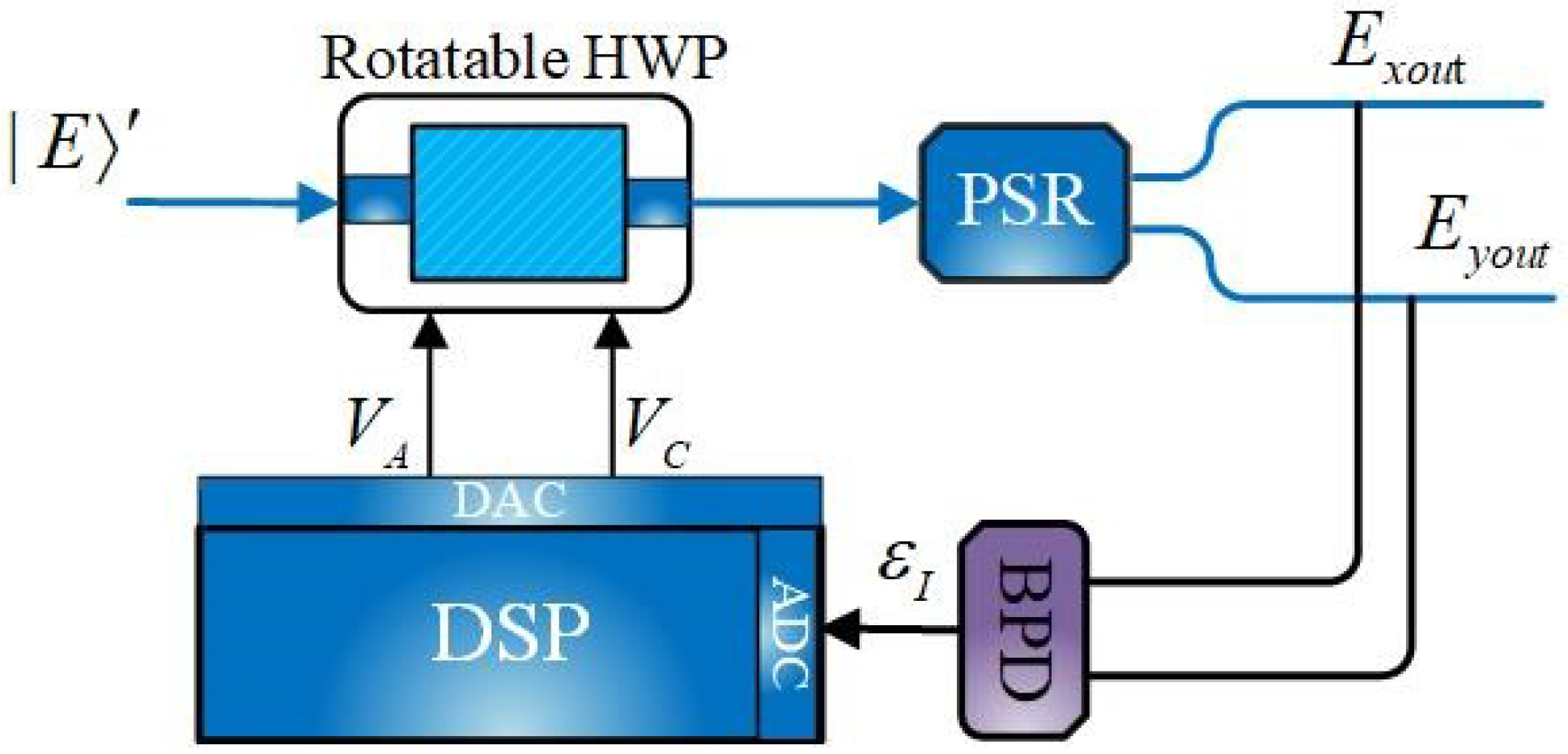
2.1. Waveplate-Based Polarization Control Structure
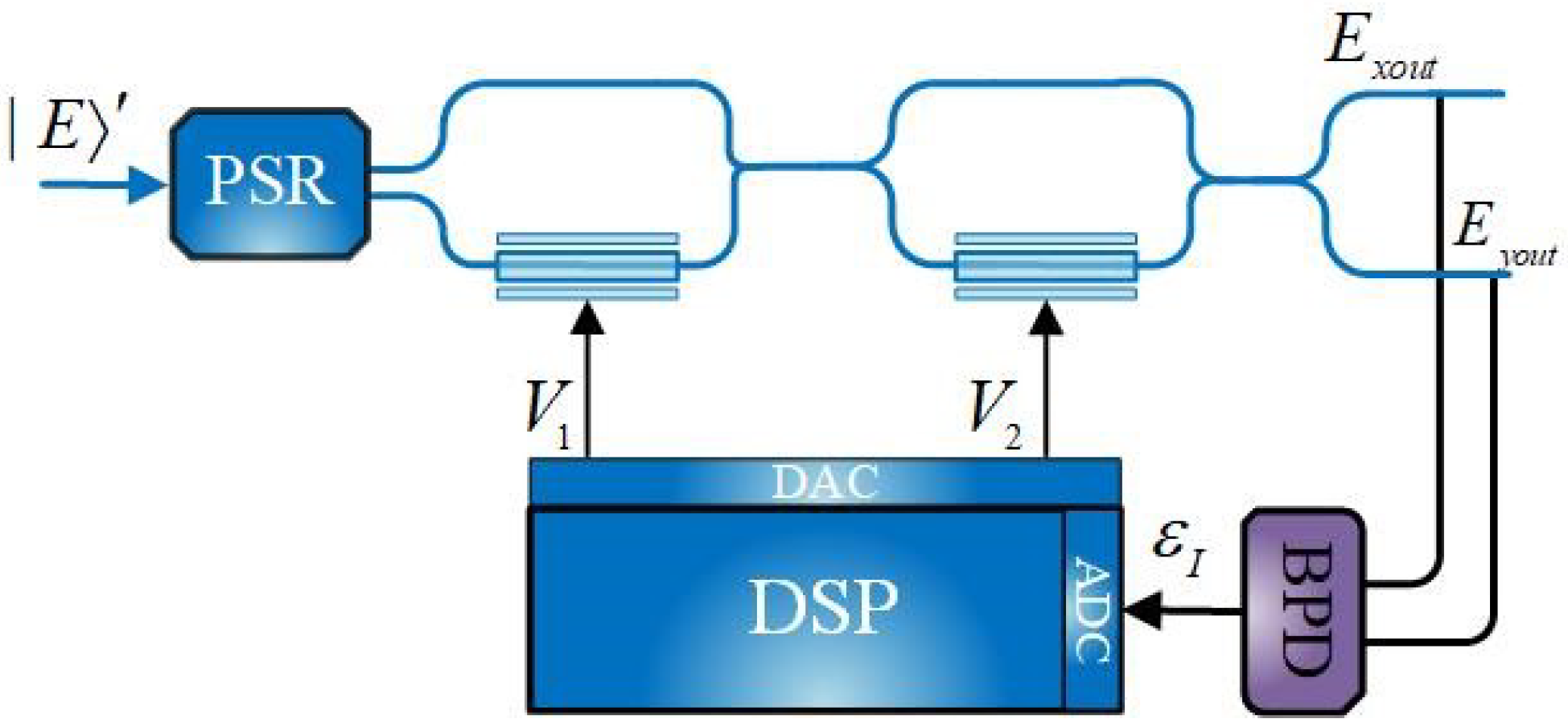
2.2. Mach–Zehnder Interferometer-Based Polarization Control Structure
2.3. Comparisons of These Two Control Structures
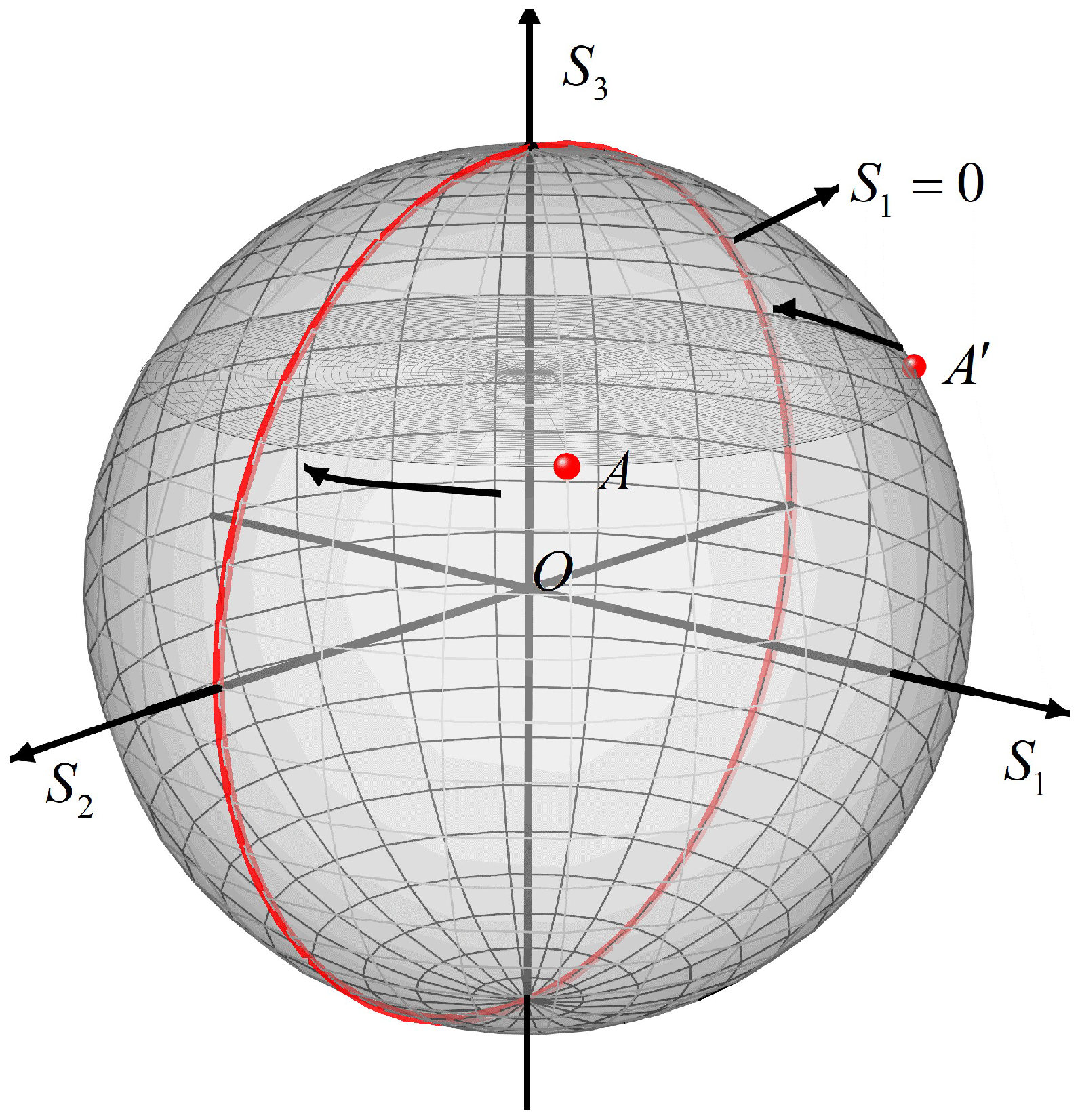
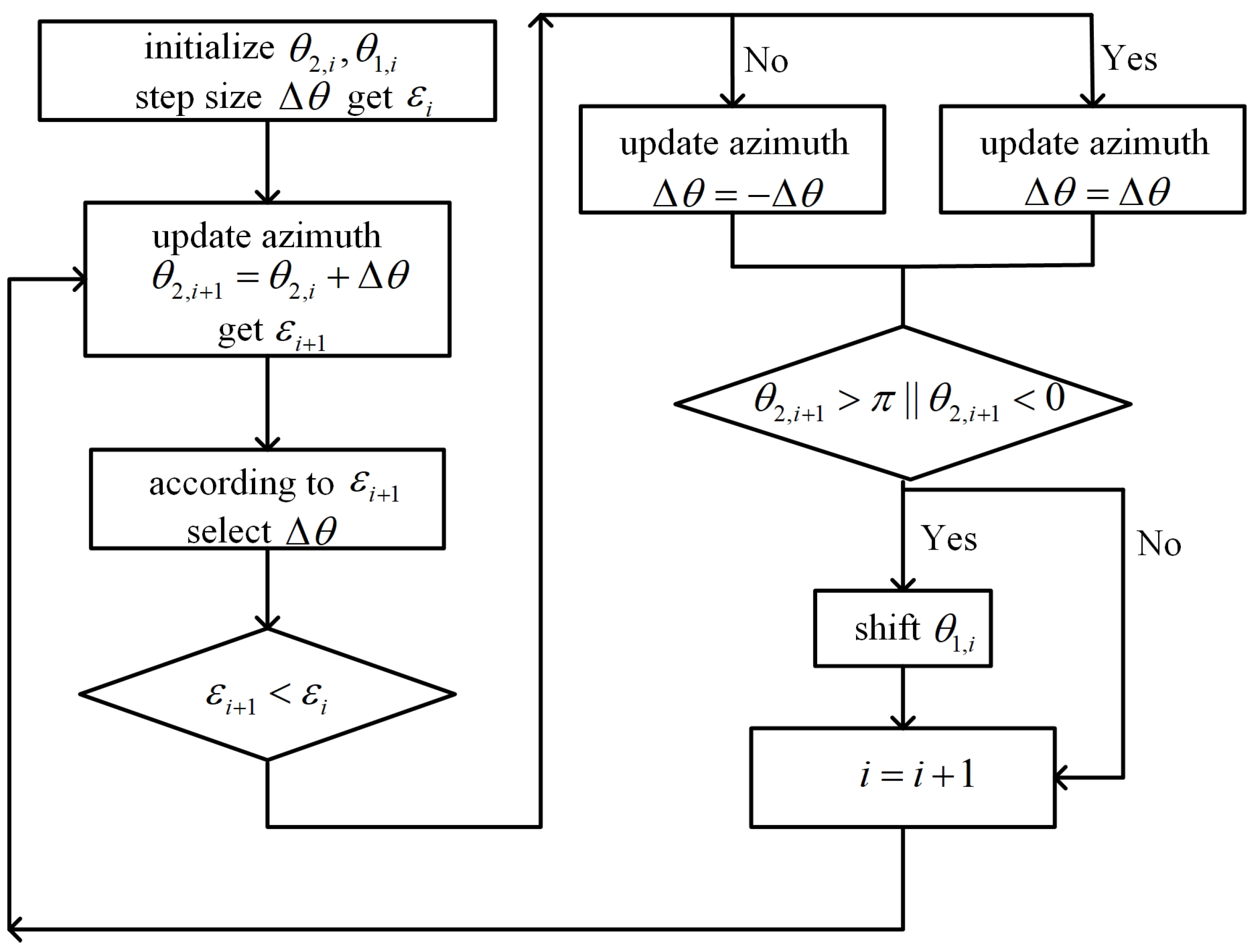
3. Polarization Control Algorithm
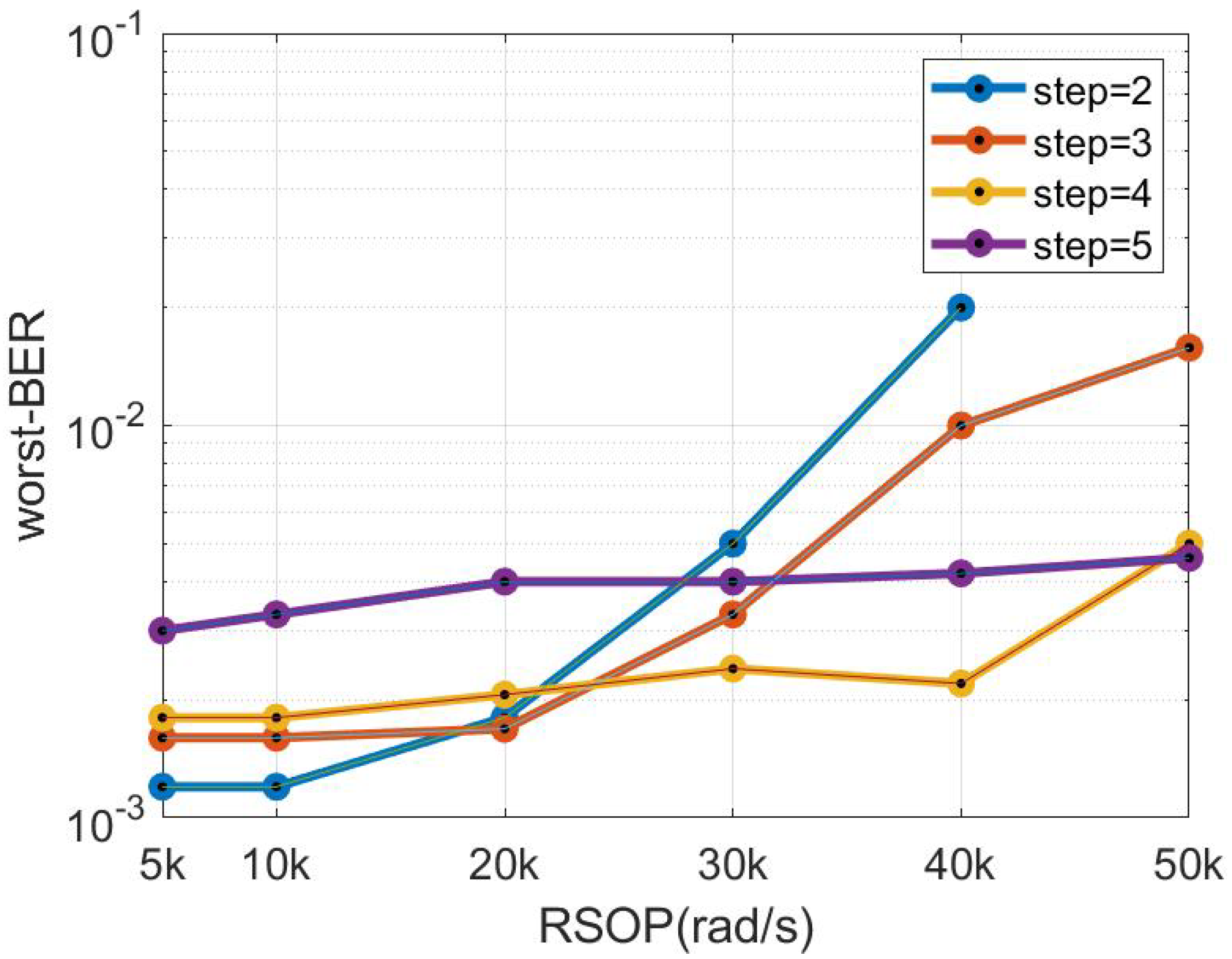
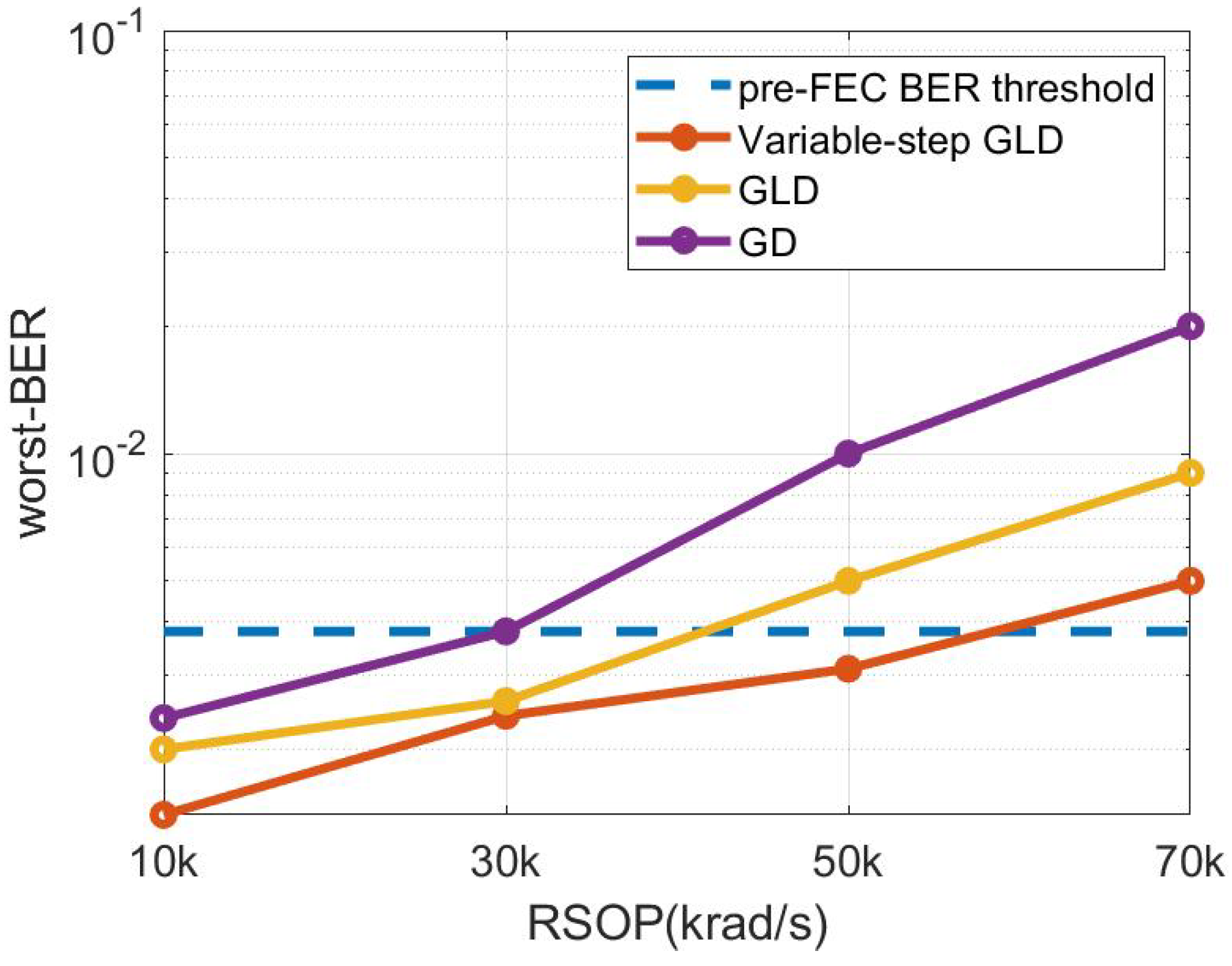
4. System Algorithm Simulation Furthermore, Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Perin, J.K.; Shastri, A.; Kahn, J.M. Coherent data center links. J. Light. Technol. 2020, 39, 730–741. [Google Scholar] [CrossRef]
- Gui, T.; Wang, X.; Tang, M.; Yu, Y.; Lu, Y.; Li, L. Real-time demonstration of homodyne coherent bidirectional transmission for next-generation data center interconnects. J. Light. Technol. 2021, 39, 1231–1238. [Google Scholar] [CrossRef]
- Xiao, L.; Wang, Y.; Li, Y.; Bai, Q.; Liu, X.; Jin, B. Polarization Fading Suppression for Optical Fiber Sensing: A Review. IEEE Sens. J. 2022, 22, 8295–8312. [Google Scholar] [CrossRef]
- Ito, M.; Okawa, K.; Suganuma, T.; Fukui, T.; Kato, E.; Tanemura, T.; Nakano, Y. Efficient InGaAsP MQW-based polarization controller without active-passive integration. Opt. Express 2021, 29, 10538–10545. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Zeng, Y.; Liao, R.; Shen, L.; Zhao, C.; Wu, H.; Tang, M. Half-Wave-Plate based Adaptive Polarization Controller. In CLEO: QELS_Fundamental Science, Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CA, USA, 9–14 May 2021; Optica Publishing Group: Washington, DC, USA, 2021; p. JW1A-49. [Google Scholar]
- Noe, R.; Heidrich, H.; Hoffmann, D. Endless polarization control systems for coherent optics. J. Light. Technol. 1988, 6, 1199–1208. [Google Scholar] [CrossRef]
- Madsen, C.K.; Oswald, P.; Cappuzzo, M.; Chen, E.; Gomez, L.; Griffin, A.; Kasper, A.; Laskowski, E.; Stulz, L.; Wong-Foy, A. Reset-free integrated polarization controller using phase shifters. IEEE J. Sel. Top. Quantum Electron. 2005, 11, 431–438. [Google Scholar] [CrossRef]
- Ji, H.; Li, J.; Li, X.; Dong, S.; Xu, Z.; Su, Y.; Shieh, W. Complementary polarization-diversity coherent receiver for self-coherent homodyne detection with rapid polarization tracking. J. Light. Technol. 2022, 40, 2773–2779. [Google Scholar] [CrossRef]
- Zheng, Z.; Lu, L.; Li, C.; Wang, W.; Zhang, S.; Fan, L.; Zhang, T.; Zuo, T.; Liu, L.; Lau, A.P.T.; et al. High speed, low voltage polarization controller based on heterogeneous integration of silicon and lithium niobate. In Proceedings of the Optical Fiber Communication Conference, Washington, DC, USA, 6–11 June 2021; p. Th1A-12. [Google Scholar]
- Heismann, F. Analysis of a reset-free polarization controller for fast automatic polarization stabilization in fiber-optic transmission systems. J. Light. Technol. 1994, 12, 690–699. [Google Scholar] [CrossRef]
- Garcia, J.D.; Amaral, G.C. An optimal polarization tracking algorithm for Lithium-Niobate-based polarization controllers. In Proceedings of the 2016 IEEE Sensor Array and Multichannel Signal Processing Workshop (SAM), Rio de Janeiro, Brazil, 10–13 July 2016; pp. 1–5. [Google Scholar]
- Su, R.; Liu, Y.; Yang, B.; Ma, P.; Wang, X.; Zhou, P.; Xu, X. Active polarization control of a 1.43 kW narrow linewidth fiber amplifier based on SPGD algorithm. J. Opt. 2017, 19, 045802. [Google Scholar] [CrossRef]
- Sha, Z.; Feng, H.; Shi, Y.; Zeng, Z. Polarization control for dual mach-zehnder fiber vibration sensor using simulated annealing. In Proceedings of the 2019 IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Auckland, New Zealand, 20–23 May 2019; pp. 1–6. [Google Scholar]
- Hou, Q.; Yuan, X.; Zhang, Y.; Zhang, J. Endless polarization stabilization control for optical communication systems. Chin. Opt. Lett. 2014, 12, 110603. [Google Scholar]
- Puttnam, B.J.; Luís, R.S.; Delgado Mendinueta, J.M. Self-homodyne detection in optical communication systems. Photonics 2014, 1, 110–130. [Google Scholar] [CrossRef]
- Gui, T.; Cao, J.; Chen, X.; Zheng, K.; Yuan, S.; Fang, X.; Lei, Y.; Zhan, Q.; Wang, D.; Sui, Q.; et al. Real-time Single-Carrier 800 Gb/s DP-64QAM Demonstration using Bi-Directional Self-homodyne Coherent Transceivers with 200 krad/s Endless Active Polarization Controller. In Proceedings of the Optoelectronics and Communications Conference, Hong Kong, China, 3–7 July 2021; p. T5A-5. [Google Scholar]
- Wang, X.; Zeng, Y.; Liao, R. High-performance polarization management devices based on thin-film lithium niobate. In Proceedings of the 2021 Optical Fiber Communications Conference and Exhibition (OFC), Online, 6–10 June 2021; pp. 1–3. [Google Scholar]
- Lin, Z. Endless polarization stabilization control for optical communication systems. Light. Sci. Appl. 2022, 11, 93. [Google Scholar] [CrossRef] [PubMed]
- Martinelli, M.; Martelli, P.; Pietralunga, S.M. Polarization stabilization in optical communications systems. J. Light. Technol. 2006, 24, 4172–4183. [Google Scholar] [CrossRef]
- Sirmaci, Y.D.; Barreda Gomez, A.; Pertsch, T.; Schmid, J.H.; Cheben, P.; Staude, I. All-dielectric Huygens’ meta-waveguides for resonant integrated photonics. Laser Photonics Rev. 2023, 17, 2200860. [Google Scholar] [CrossRef]
- Polarization Controllers—EOSPACE, INC. Available online: https://www.eospace.com/polarization-controller (accessed on 18 May 2023).
- Ma, M.; Shoman, H.; Tang, K.; Shekhar, S.; Jaeger, N.A.; Chrostowski, L. Automated control algorithms for silicon photonic polarization receiver. Opt. Express 2020, 28, 1885–1896. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Lu, L.; Gao, W.; Li, X.; Chen, J.; Zhou, L. Silicon non-blocking 4 × 4 optical switch with automated polarization adjustment. Chin. Opt. Lett. 2021, 19, 101302. [Google Scholar] [CrossRef]
- Novoptel: Polarization Scramblers: Technology at Work. Available online: https://novoptel.de/Scrambling/Scrambling_en.php (accessed on 26 June 2023).







| Parameter | HWP [21] | MZI [18] |
|---|---|---|
| Half-wave voltage | 10 vs. | 2.4 v |
| Length | 6.2 cm | 1.5 cm |
| Response time | <100 ns | <5 ns |
| Optical insertion loss | 2.4–3.0 dB | 0.92 dB |
| Operating wavelength | 1.5–1.6 μm | 0.4–5 μm |
| Reset | NO | YES |
| Parameter | Value |
|---|---|
| Modulation format | 16 QAM |
| Polarization | Dual-polarization |
| Baudrate | 28 GBaud |
| Laser power | 13.8 dBm |
| Laser linewidth | 1 MHz |
| Responsivity of PD | 0.65 |
| Dark current of PD | 10 nA |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, Q.; Zhou, X.; Li, R.; Gao, Y.; Wang, S.; Li, F.; Long, K. Polarization Controller Based on Variable-Step Greedy Linear Descent for Self-Homodyne Coherent Transmission Systems. Photonics 2023, 10, 770. https://doi.org/10.3390/photonics10070770
Fang Q, Zhou X, Li R, Gao Y, Wang S, Li F, Long K. Polarization Controller Based on Variable-Step Greedy Linear Descent for Self-Homodyne Coherent Transmission Systems. Photonics. 2023; 10(7):770. https://doi.org/10.3390/photonics10070770
Chicago/Turabian StyleFang, Qianwen, Xian Zhou, Rui Li, Yuyuan Gao, Shiyao Wang, Feiyu Li, and Keping Long. 2023. "Polarization Controller Based on Variable-Step Greedy Linear Descent for Self-Homodyne Coherent Transmission Systems" Photonics 10, no. 7: 770. https://doi.org/10.3390/photonics10070770
APA StyleFang, Q., Zhou, X., Li, R., Gao, Y., Wang, S., Li, F., & Long, K. (2023). Polarization Controller Based on Variable-Step Greedy Linear Descent for Self-Homodyne Coherent Transmission Systems. Photonics, 10(7), 770. https://doi.org/10.3390/photonics10070770






