Terahertz Bessel Beams Formed by Binary and Holographic Axicons
Abstract
1. Introduction
2. Materials and Methods
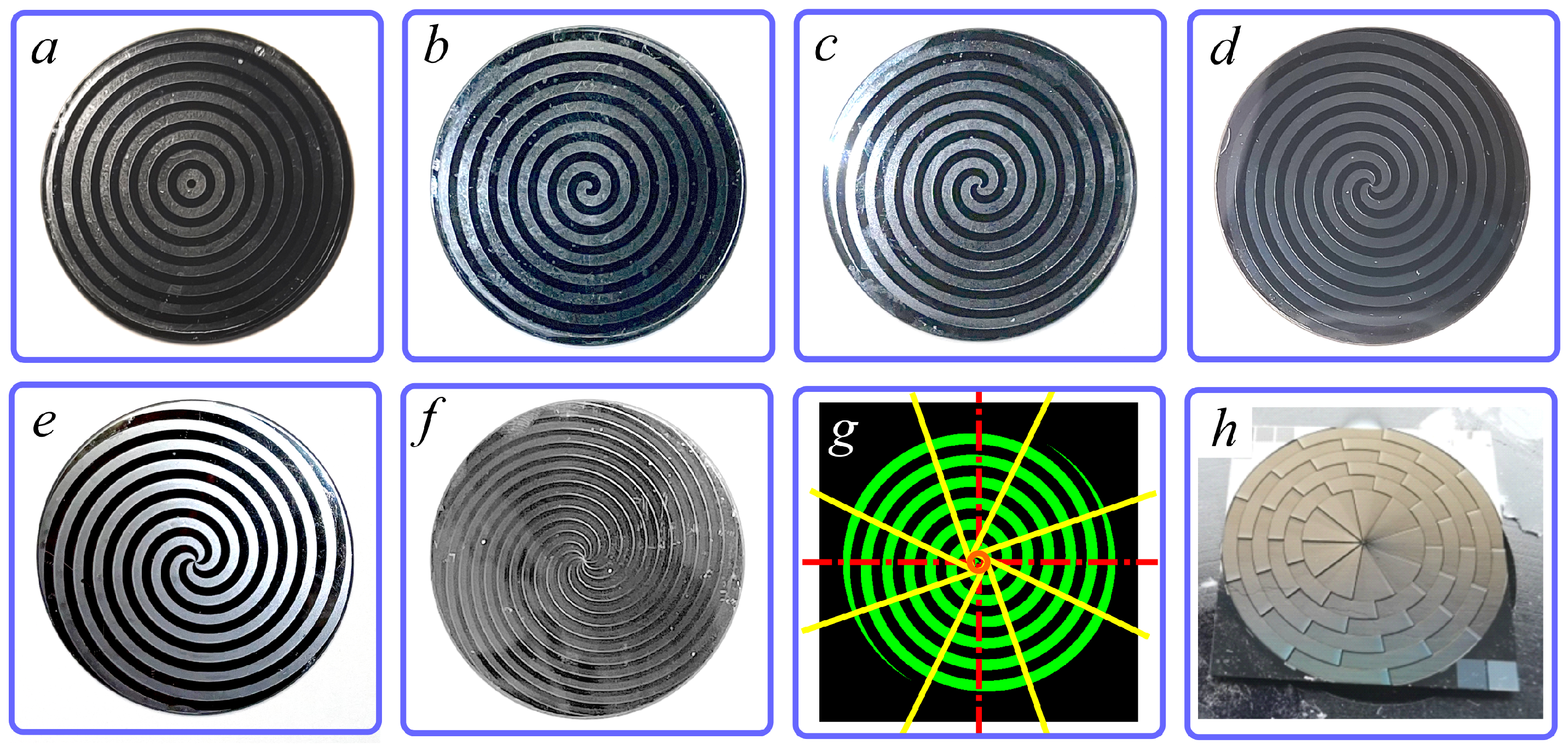
2.1. Diffractive Axicons
| Axicon | BA0 | BA1 | BA2 | BA3 | BA4 | BA3-2 | BA9 | HA9 |
|---|---|---|---|---|---|---|---|---|
| l | 0 | 1 | 2 | 3 | 4 | 3 | 9 | 9 |
| p, mm | 3.1 | 3.1 | 3.1 | 3.1 | 3.1 | 2.03 | 2.03 | N/A |
| R, mm | 22 | 22 | 22 | 22 | 22 | 22 | 22 | 10 |
| Z, mm | 550 | 550 | 550 | 550 | 550 | 360 | 360 | 144 |
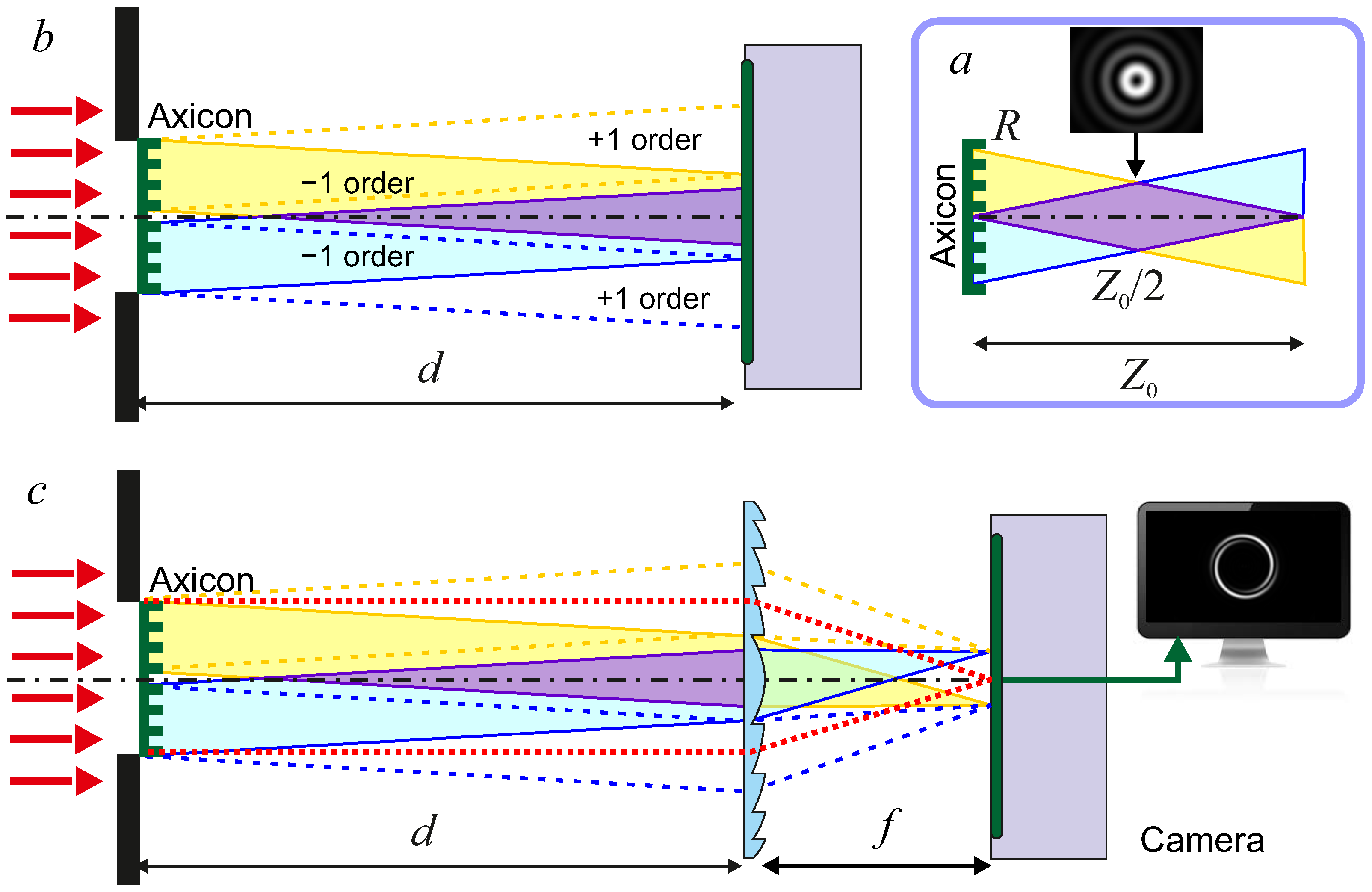
2.2. Experimental Setup
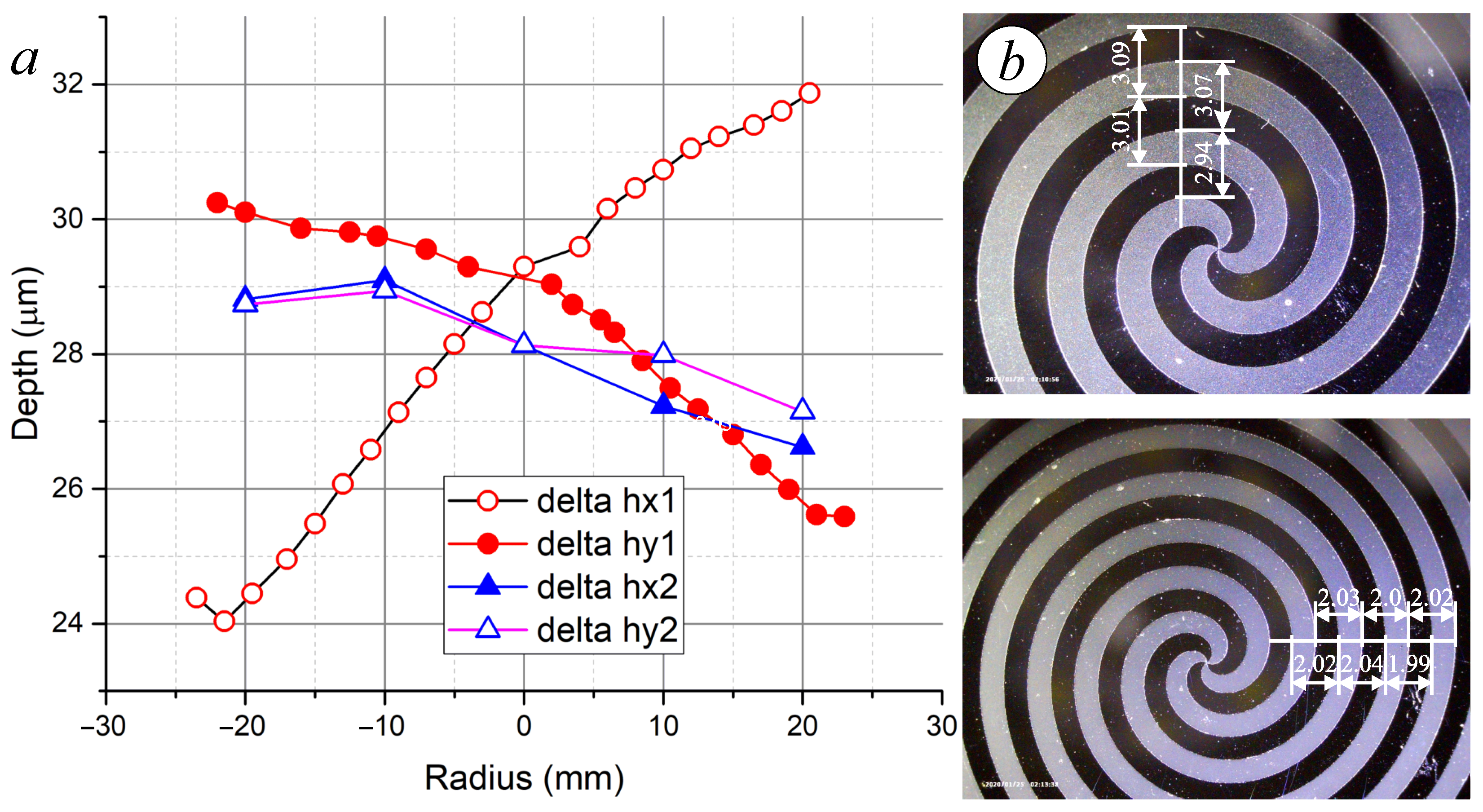
2.3. Axicons: Fabrication and Profile Measurements
2.4. Analytical Calculations and Numerical Simulations
3. Results and Discussion
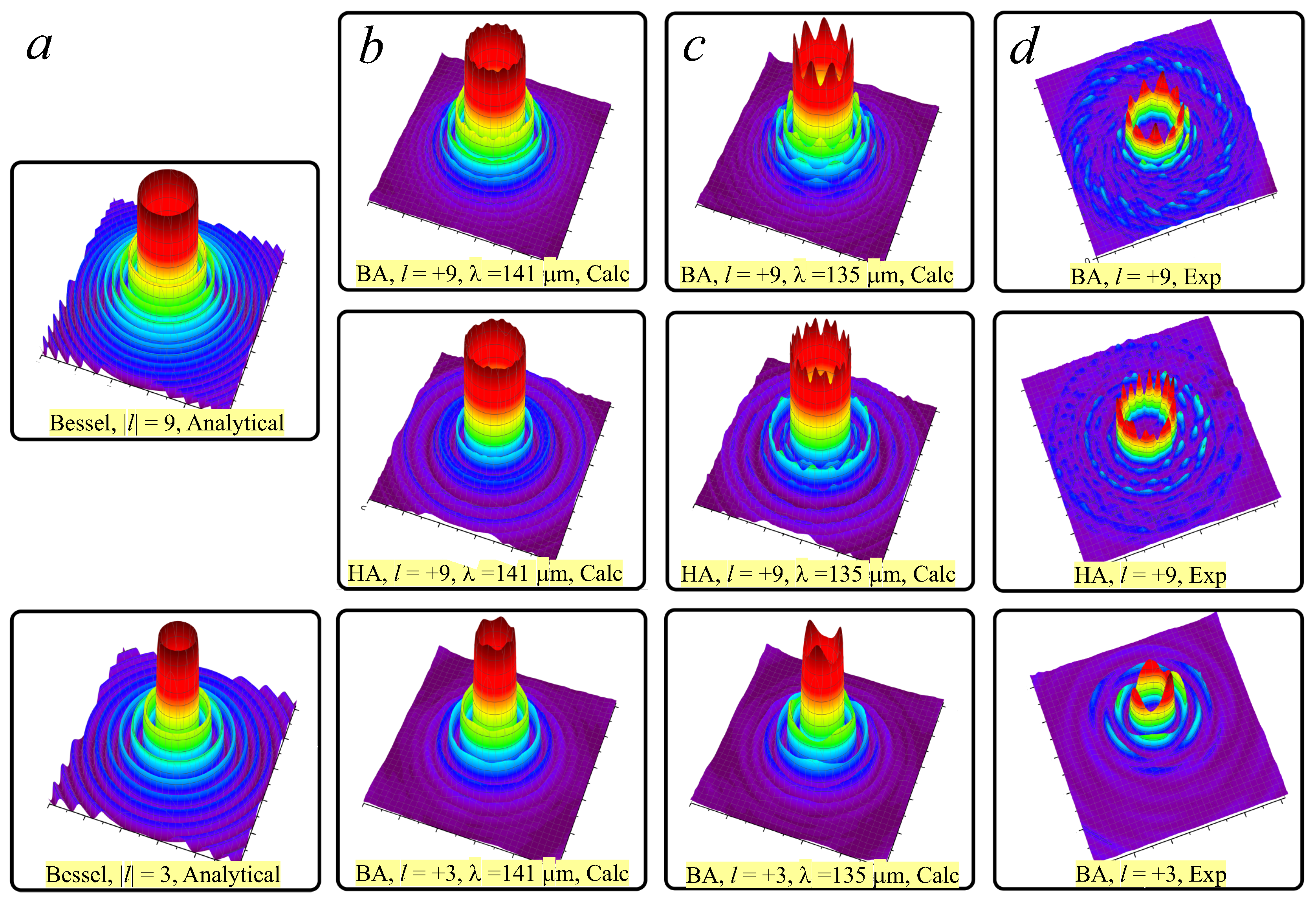
3.1. Bessel Beams Obtained Experimentally
3.2. Numerical Simulations: Effect of Wavelength Change and Phase Profile Inaccuracy
3.3. Perfect Beams Formed with Binary and Holographic Axicons
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.; Woerdman, J. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185. [Google Scholar] [CrossRef]
- Padgett, M.J. Orbital angular momentum 25 years on. Opt. Express 2017, 25, 11265–11274. [Google Scholar] [CrossRef]
- Shen, Y.; Wang, X.; Xie, Z.; Min, C.; Fu, X.; Liu, Q.; Gong, M.; Yuan, X. Optical vortices 30 years on: OAM manipulation from topological charge to multiple singularities. Light. Sci. Appl. 2019, 8, 90. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, J. A review of multiple optical vortices generation: Methods and applications. Front. Optoelectron. 2019, 12, 52–68. [Google Scholar] [CrossRef]
- Pavlov, D.; Porfirev, A.; Khonina, S.; Pan, L.; Kudryashov, S.; Kuchmizhak, A. Coaxial hole array fabricated by ultrafast femtosecond-laser processing with spatially multiplexed vortex beams for surface enhanced infrared absorption. Appl. Surf. Sci. 2021, 541, 148602. [Google Scholar] [CrossRef]
- Brasselet, E.; Murazawa, N.; Misawa, H.; Juodkazis, S. Optical vortices from liquid crystal droplets. Phys. Rev. Lett. 2009, 103, 103903. [Google Scholar] [CrossRef] [PubMed]
- Shen, Z.; Hu, Z.; Yuan, G.; Min, C.; Fang, H.; Yuan, X.C. Visualizing orbital angular momentum of plasmonic vortices. Opt. Lett. 2012, 37, 4627–4629. [Google Scholar] [CrossRef] [PubMed]
- Zhuang, X. Unraveling DNA condensation with optical tweezers. Science 2004, 305, 188–190. [Google Scholar] [CrossRef] [PubMed]
- Brullot, W.; Vanbel, M.K.; Swusten, T.; Verbiest, T. Resolving enantiomers using the optical angular momentum of twisted light. Sci. Adv. 2016, 2, e1501349. [Google Scholar] [CrossRef]
- Jeffries, G.D.; Edgar, J.S.; Zhao, Y.; Shelby, J.P.; Fong, C.; Chiu, D.T. Using polarization-shaped optical vortex traps for single-cell nanosurgery. Nano Lett. 2007, 7, 415–420. [Google Scholar] [CrossRef]
- Tamburini, F.; Anzolin, G.; Umbriaco, G.; Bianchini, A.; Barbieri, C. Overcoming the Rayleigh criterion limit with optical vortices. Phys. Rev. Lett. 2006, 97, 163903. [Google Scholar] [CrossRef]
- Fürhapter, S.; Jesacher, A.; Bernet, S.; Ritsch-Marte, M. Spiral phase contrast imaging in microscopy. Opt. Express 2005, 13, 689–694. [Google Scholar] [CrossRef]
- Xie, G.; Song, H.; Zhao, Z.; Milione, G.; Ren, Y.; Liu, C.; Zhang, R.; Bao, C.; Li, L.; Wang, Z.; et al. Using a Complex Optical Orbital-Angular-Momentum Spectrum to Measure Object Parameters: A Spatial Domain Approach. arXiv 2017, arXiv:1705.09051. [Google Scholar]
- Fu, S.; Gao, C. Influences of atmospheric turbulence effects on the orbital angular momentum spectra of vortex beams. Photonics Res. 2016, 4, B1–B4. [Google Scholar] [CrossRef]
- Li, Y.; Yu, L.; Zhang, Y. Influence of anisotropic turbulence on the orbital angular momentum modes of Hermite-Gaussian vortex beam in the ocean. Opt. Express 2017, 25, 12203–12215. [Google Scholar] [CrossRef] [PubMed]
- Lavery, M.P.; Barnett, S.M.; Speirits, F.C.; Padgett, M.J. Observation of the rotational Doppler shift of a white-light, orbital-angular-momentum-carrying beam backscattered from a rotating body. Optica 2014, 1, 1–4. [Google Scholar] [CrossRef]
- Cheng, W.; Polynkin, P. Micromachining of borosilicate glass surfaces using femtosecond higher-order Bessel beams. JOSA B 2014, 31, C48–C52. [Google Scholar] [CrossRef]
- Oosterbeek, R.N.; Ashforth, S.; Bodley, O.; Simpson, M.C. Measuring the ablation threshold fluence in femtosecond laser micromachining with vortex and Bessel pulses. Opt. Express 2018, 26, 34558–34568. [Google Scholar] [CrossRef]
- Baltrukonis, J.; Ulčinas, O.; Orlov, S.; Jukna, V. High-order vector bessel-gauss beams for laser micromachining of transparent materials. Phys. Rev. Appl. 2021, 16, 034001. [Google Scholar] [CrossRef]
- Lutz, C.; Schwarz, S.; Marx, J.; Esen, C.; Hellmann, R. Multi-Bessel Beams Generated by an Axicon and a Spatial Light Modulator for Drilling Applications. Photonics 2023, 10, 413. [Google Scholar] [CrossRef]
- Krenn, M.; Fickler, R.; Fink, M.; Handsteiner, J.; Malik, M.; Scheidl, T.; Ursin, R.; Zeilinger, A. Communication with spatially modulated light through turbulent air across Vienna. New J. Phys. 2014, 16, 113028. [Google Scholar] [CrossRef]
- Willner, A.E.; Huang, H.; Yan, Y.; Ren, Y.; Ahmed, N.; Xie, G.; Bao, C.; Li, L.; Cao, Y.; Zhao, Z.; et al. Optical communications using orbital angular momentum beams. Adv. Opt. Photonics 2015, 7, 66–106. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.Y.; Fazal, I.M.; Ahmed, N.; Yan, Y.; Huang, H.; Ren, Y.; Yue, Y.; Dolinar, S.; Tur, M.; et al. Terabit free-space data transmission employing orbital angular momentum multiplexing. Nat. Photonics 2012, 6, 488–496. [Google Scholar] [CrossRef]
- Yan, Y.; Xie, G.; Lavery, M.P.; Huang, H.; Ahmed, N.; Bao, C.; Ren, Y.; Cao, Y.; Li, L.; Zhao, Z.; et al. High-capacity millimetre-wave communications with orbital angular momentum multiplexing. Nat. Commun. 2014, 5, 4876. [Google Scholar] [CrossRef]
- Wei, X.; Liu, C.; Zhang, Z.; Zhu, L.; Wang, J.; Wang, K.; Yang, Z.; Liu, J. Orbit angular momentum encoding at 0.3 THz via 3D printed spiral phase plates. In Infrared, Millimeter-Wave, and Terahertz Technologies III; SPIE: Manhattan, NY, USA, 2014; Volume 9275, pp. 260–265. [Google Scholar]
- Zhan, Q. Cylindrical vector beams: From mathematical concepts to applications. Adv. Opt. Photonics 2009, 1, 1–57. [Google Scholar] [CrossRef]
- Yang, Y.; Qiu, C.W. Generation of Optical Vortex Beams. In Electromagnetic Vortices: Wave Phenomena and Engineering Applications; Wiley-IEEE Press: Manhattan, NY, USA, 2021; pp. 223–244. [Google Scholar]
- Yang, Y.; Li, Y.; Wang, C. Generation and expansion of Laguerre–Gaussian beams. J. Opt. 2022, 51, 910–926. [Google Scholar] [CrossRef]
- Pereiro-García, J.; García-de Blas, M.; Geday, M.A.; Quintana, X.; Caño-García, M. Flat variable liquid crystal diffractive spiral axicon enabling perfect vortex beams generation. Sci. Rep. 2023, 13, 2385. [Google Scholar] [CrossRef]
- Litvin, I.A.; Dudley, A.; Roux, F.S.; Forbes, A. Azimuthal decomposition with digital holograms. Opt. Express 2012, 20, 10996–11004. [Google Scholar] [CrossRef]
- Flamm, D.; Naidoo, D.; Schulze, C.; Forbes, A.; Duparré, M. Mode analysis with a spatial light modulator as a correlation filter. Opt. Lett. 2012, 37, 2478–2480. [Google Scholar] [CrossRef]
- Mazilu, M.; Mourka, A.; Vettenburg, T.; Wright, E.M.; Dholakia, K. Simultaneous determination of the constituent azimuthal and radial mode indices for light fields possessing orbital angular momentum. Appl. Phys. Lett. 2012, 100, 231115. [Google Scholar] [CrossRef]
- Mourka, A.; Mazilu, M.; Wright, E.M.; Dholakia, K. Modal characterization using principal component analysis: Application to Bessel, higher-order Gaussian beams and their superposition. Sci. Rep. 2013, 3, 1422. [Google Scholar] [CrossRef] [PubMed]
- Dudley, A.; Mhlanga, T.; Lavery, M.; McDonald, A.; Roux, F.S.; Padgett, M.; Forbes, A. Efficient sorting of Bessel beams. Opt. Express 2013, 21, 165–171. [Google Scholar] [CrossRef] [PubMed]
- Trichili, A.; Mhlanga, T.; Ismail, Y.; Roux, F.S.; McLaren, M.; Zghal, M.; Forbes, A. Detection of Bessel beams with digital axicons. Opt. Express 2014, 22, 17553–17560. [Google Scholar] [CrossRef] [PubMed]
- Forbes, A.; Dudley, A.; McLaren, M. Creation and detection of optical modes with spatial light modulators. Adv. Opt. Photonics 2016, 8, 200–227. [Google Scholar] [CrossRef]
- Guan, S.; Cheng, J.; Chang, S. Recent Progress of Terahertz Spatial Light Modulators: Materials, Principles and Applications. Micromachines 2022, 13, 1637. [Google Scholar] [CrossRef]
- Petrov, N.V.; Sokolenko, B.; Kulya, M.S.; Gorodetsky, A.; Chernykh, A.V. Design of broadband terahertz vector and vortex beams: I. Review of materials and components. Light. Adv. Manuf. 2022, 3, 54. [Google Scholar] [CrossRef]
- Shen, Y.; Shen, Z.; Zhao, G.; Hu, W. Photopatterned liquid crystal mediated terahertz Bessel vortex beam generator. Chin. Opt. Lett. 2020, 18, 080003. [Google Scholar] [CrossRef]
- Shen, Z.X.; Tang, M.J.; Chen, P.; Zhou, S.H.; Ge, S.J.; Duan, W.; Wei, T.; Liang, X.; Hu, W.; Lu, Y.Q. Planar terahertz photonics mediated by liquid crystal polymers. Adv. Opt. Mater. 2020, 8, 1902124. [Google Scholar] [CrossRef]
- Glyavin, M.Y.; Luchinin, A.G.; Golubiatnikov, G.Y. Generation of 1.5-kW, 1-THz Coherent Radiation from a Gyrotron with a Pulsed Magnetic Field. Phys. Rev. Lett. 2008, 100, 015101. [Google Scholar] [CrossRef]
- Glyavin, M. Development and applications of THz gyrotrons. EPJ Web Conf. 2017, 149, 01008. [Google Scholar] [CrossRef]
- Tan, P.; Huang, J.; Liu, K.; Xiong, Y.; Fan, M. Terahertz radiation sources based on free electron lasers and their applications. Sci. China Inf. Sci. 2012, 55, 1–15. [Google Scholar] [CrossRef]
- Kulipanov, G.N.; Bagryanskaya, E.G.; Chesnokov, E.N.; Choporova, Y.Y.; Gerasimov, V.V.; Getmanov, Y.V.; Kiselev, S.L.; Knyazev, B.A.; Kubarev, V.V.; Peltek, S.E.; et al. Novosibirsk free electron laser—facility description and recent experiments. IEEE Trans. Terahertz Sci. Technol. 2015, 5, 798–809. [Google Scholar] [CrossRef]
- Petrillo, V.; Andreone, A.; Bacci, A.; Bosotti, A.; Broggi, F.; Drebot, I.; Galzerano, G.; Giannotti, D.; Giove, D.; Koral, C.; et al. High brilliance Free-Electron Laser Oscillator operating at multi-MegaHertz repetition rate in the short-TeraHertz emission range. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2022, 1040, 167289. [Google Scholar] [CrossRef]
- Helm, M.; Winnerl, S.; Pashkin, A.; Klopf, J.; Deinert, J.C.; Kovalev, S.; Evtushenko, P.; Lehnert, U.; Xiang, R.; Arnold, A.; et al. The ELBE infrared and THz facility at Helmholtz-Zentrum Dresden-Rossendorf. Eur. Phys. J. Plus 2023, 138, 158. [Google Scholar] [CrossRef]
- Soifer, V.A. Methods for Computer Design of Diffractive Optical Elements; John Wiley & Sons, Inc.: New York, NY, USA, 2001; p. 784. [Google Scholar]
- Tudor, R.; Bulzan, G.A.; Kusko, M.; Kusko, C.; Avramescu, V.; Vasilache, D.; Gavrila, R. Multilevel Spiral Axicon for High-Order Bessel–Gauss Beams Generation. Nanomaterials 2023, 13, 579. [Google Scholar] [CrossRef] [PubMed]
- Kononenko, T.V.; Knyazev, B.A.; Sovyk, D.N.; Pavelyev, V.S.; Komlenok, M.S.; Komandin, G.A.; Konov, V.I. Silicon kinoform cylindrical lens with low surface roughness for high-power terahertz radiation. Opt. Laser Technol. 2020, 123, 105953. [Google Scholar] [CrossRef]
- Komlenok, M.S.; Kononenko, T.V.; Konov, V.I.; Choporova, Y.Y.; Osintseva, N.D.; Knyazev, B.A.; Pavelyev, V.S.; Tukmakov, K.N.; Soifer, V.A. Silicon diffractive optical element with piecewise continuous profile to focus high-power terahertz radiation into a square area. J. Opt. Soc. Am. B 2021, 38, B9. [Google Scholar] [CrossRef]
- Komlenok, M.; Kononenko, T.; Sovyk, D.; Pavelyev, V.; Knyazev, B.; Ashkinazi, E.; Reshetnikov, A.; Komandin, G.; Pashinin, V.; Ralchenko, V.; et al. Diamond diffractive lens with a continuous profile for powerful terahertz radiation. Opt. Lett. 2021, 46, 340. [Google Scholar] [CrossRef]
- Pavelyev, V.; Choporova, Y.; Osintseva, N.; Tukmakov, K.; Knyazev, B. Control of transverse mode content and polarization structure of terahertz coherent beams. Comput. Opt. 2019, 43, 1103–1108. [Google Scholar] [CrossRef]
- Agafonov, A.N.; Volodkin, B.O.; Volotovsky, S.G.; Kaveev, A.K.; Knyazev, B.A.; Kropotov, G.I.; Tykmakov, K.N.; Pavelyev, V.S.; Tsygankova, E.V.; Tsypishka, D.I.; et al. Optical elements for focusing of terahertz laser radiation in a given two-dimensional domain. Opt. Mem. Neural Netw. 2014, 23, 185–190. [Google Scholar] [CrossRef]
- Agafonov, A.N.; Volodkin, B.O.; Kachalov, D.G.; Knyazev, B.A.; Kropotov, G.I.; Tukmakov, K.N.; Pavelyev, V.S.; Tsypishka, D.I.; Choporova, Y.Y.; Kaveev, A.K. Focusing of Novosibirsk Free Electron Laser (NovoFEL) radiation into paraxial segment. J. Mod. Opt. 2016, 63, 1051–1054. [Google Scholar] [CrossRef]
- Pavelyev, V.S.; Degtyarev, S.A.; Tukmakov, K.N.; Reshetnikov, A.S.; Knyazev, B.A.; Choporova, Y.Y. Silicon subwavelength axicons for terahertz beam polarization transformation. J. Phys. Conf. Ser. 2021, 1745, 012022. [Google Scholar] [CrossRef]
- Pavelyev, V.; Khonina, S.; Degtyarev, S.; Tukmakov, K.; Reshetnikov, A.; Gerasimov, V.; Osintseva, N.; Knyazev, B. Subwavelength Diffractive Optical Elements for Generation of Terahertz Coherent Beams with Pre-Given Polarization State. Sensors 2023, 23, 1579. [Google Scholar] [CrossRef]
- Choporova, Y.Y.; Knyazev, B.A.; Kulipanov, G.N.; Pavelyev, V.S.; Scheglov, M.A.; Vinokurov, N.A.; Volodkin, B.O.; Zhabin, V.N. High-power Bessel beams with orbital angular momentum in the terahertz range. Phys. Rev. A 2017, 96, 023846. [Google Scholar] [CrossRef]
- Choporova, Y.; Knyazev, B.; Pavelyev, V. Holography with high-power CW coherent terahertz source: Optical components, imaging, and applications. Light. Adv. Manuf. 2022, 3, 525–541. [Google Scholar] [CrossRef]
- Knyazev, B.; Choporova, Y.Y.; Mitkov, M.; Pavelyev, V.; Volodkin, B. Generation of terahertz surface plasmon polaritons using nondiffractive Bessel beams with orbital angular momentum. Phys. Rev. Lett. 2015, 115, 163901. [Google Scholar] [CrossRef] [PubMed]
- Choporova, Y.Y.; Knyazev, B.A.; Mitkov, M.S. Holography as imaging technique for the THz range. In Proceedings of the 2016 41st International Conference on Infrared, Millimeter, and Terahertz Waves (IRMMW-THz), Copenhagen, Denmark, 25–30 September 2016; pp. 1–2. [Google Scholar] [CrossRef]
- Knyazev, B.A.; Serbo, V. Beams of photons with nonzero projections of orbital angular momenta: New results. Physics-Uspekhi 2018, 61, 449. [Google Scholar] [CrossRef]
- Knyazev, B.; Kameshkov, O.; Vinokurov, N.; Cherkassky, V.; Choporova, Y.; Pavelyev, V. Quasi-Talbot effect with vortex beams and formation of vortex beamlet arrays. Opt. Express 2018, 26, 14174. [Google Scholar] [CrossRef]
- Kotelnikov, I.A.; Kameshkov, O.E.; Knyazev, B.A. Diffraction of bessel beams on 2D amplitude gratings—A new branch in the talbot effect study. J. Opt. 2020, 22, 065603. [Google Scholar] [CrossRef]
- Gerasimov, V.; Kameshkov, O.; Knyazev, B.; Osintseva, N.; Pavelyev, V. Vortex surface plasmon polaritons on a cylindrical waveguide: Generation, propagation, and diffraction. J. Opt. 2021, 23, 10LT01. [Google Scholar] [CrossRef]
- Ostrovsky, A.S.; Rickenstorff-Parrao, C.; Arrizón, V. Generation of the “perfect” optical vortex using a liquid-crystal spatial light modulator. Opt. Lett. 2013, 38, 534–536. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Fang, Z.X.; Ren, Y.X.; Gong, L.; Lu, R.D. Generation and characterization of a perfect vortex beam with a large topological charge through a digital micromirror device. Appl. Opt. 2015, 54, 8030–8035. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Yang, Q.; Xu, Q.; Jiang, X.; Wu, T.; Gu, J.; Han, J.; Zhang, W. Multichannel terahertz quasi-perfect vortex beams generation enabled by multifunctional metasurfaces. Nanophotonics 2022, 11, 3631–3640. [Google Scholar] [CrossRef]
- Ahmed, H.; Intaravanne, Y.; Ming, Y.; Ansari, M.A.; Buller, G.S.; Zentgraf, T.; Chen, X. Multichannel superposition of grafted perfect vortex beams. Adv. Mater. 2022, 34, 2203044. [Google Scholar] [CrossRef]
- Stegeman, G.; Wallis, R.; Maradudin, A. Excitation of surface polaritons by end-fire coupling. Opt. Lett. 1983, 8, 386–388. [Google Scholar] [CrossRef]
- Edelmann, A.; Moeller, L.; Jahns, J. Coupling of terahertz radiation to metallic wire using end-fire technique. Electron. Lett. 2013, 49, 884–886. [Google Scholar] [CrossRef]
- Fisher, C.; Botten, L.C.; Poulton, C.G.; McPhedran, R.C.; de Sterke, C.M. Efficient end-fire coupling of surface plasmons in a metal waveguide. JOSA B 2015, 32, 412–425. [Google Scholar] [CrossRef]
- Knyazev, B.; Cherkassky, V.; Kameshkov, O. “Perfect” Terahertz Vortex Beams Formed Using Diffractive Axicons and Prospects for Excitation of Vortex Surface Plasmon Polaritons. Appl. Sci. 2021, 11, 717. [Google Scholar] [CrossRef]
- Simon, D.S. Bessel beams, self-healing, and diffraction-free propagation. In A Guided Tour of Light Beams (Second Edition); 2053–2563; IOP Publishing: Bristol, UK, 2020; pp. 5-1–5-22. [Google Scholar] [CrossRef]
- Khonina, S.; Kotlyar, V.; Shinkaryev, M.; Soifer, V.; Uspleniev, G. The phase rotor filter. J. Mod. Opt. 1992, 39, 1147–1154. [Google Scholar] [CrossRef]
- Cheong, W.; Lee, W.; Yuan, X.C.; Zhang, L.S.; Dholakia, K.; Wang, H. Direct electron-beam writing of continuous spiral phase plates in negative resist with high power efficiency for optical manipulation. Appl. Phys. Lett. 2004, 85, 5784–5786. [Google Scholar] [CrossRef]
- Oemrawsingh, S.; Van Houwelingen, J.; Eliel, E.; Woerdman, J.; Verstegen, E.; Kloosterboer, J.; ’t Hooft, G.W. Production and characterization of spiral phase plates for optical wavelengths. Appl. Opt. 2004, 43, 688–694. [Google Scholar] [CrossRef] [PubMed]
- Sundbeck, S.; Gruzberg, I.; Grier, D.G. Structure and scaling of helical modes of light. Opt. Lett. 2005, 30, 477–479. [Google Scholar] [CrossRef]
- Heckenberg, N.; McDuff, R.; Smith, C.; White, A. Generation of optical phase singularities by computer-generated holograms. Opt. Lett. 1992, 17, 221–223. [Google Scholar] [CrossRef] [PubMed]
- Goodman, J.W. Introduction to Fourier Optics; Roberts and Company Publishers: Greenwood Village, CO, USA, 2005. [Google Scholar]
- Voelz, D.G. Computational Fourier Optics: A MATLAB Tutorial; SPIE Press: Bellingham, WA, USA, 2011; Volume 534. [Google Scholar]
- Kameshkov, O.; Knyazev, B. Simulating diffraction on a series of amplitude-phase masks for experiments at the Novosibirsk free electron laser. Bull. Russ. Acad. Sci. Phys. 2019, 83, 184–189. [Google Scholar] [CrossRef]
- Dem’yanenko, M.A.; Esaev, D.G.; Knyazev, B.A.; Kulipanov, G.N.; Vinokurov, N.A. Imaging with a 90 frames/s microbolometer focal plane array and high-power terahertz free electron laser. Appl. Phys. Lett. 2008, 92, 131116. [Google Scholar] [CrossRef]
- Dem’yanenko, M.A.; Esaev, D.G.; Ovsyuk, V.N.; Fomin, B.I.; Aseev, A.L.; Knyazev, B.A.; Kulipanov, G.N.; Vinokurov, N.A. Microbolometer detector arrays for the infrared and terahertz ranges. J. Opt. Technol. 2009, 76, 739. [Google Scholar] [CrossRef]
- Osintseva, N.; Gerasimov, V.; Knyazev, B.; Komlenok, M.; Pavelyev, V.; Yablokov, D. Terahertz Bessel and “perfect” vortex beams generated with a binary axicon and axicon with continuous relief. Comput. Opt. 2022, 46, 375–380. [Google Scholar] [CrossRef]
- Chen, M.; Mazilu, M.; Arita, Y.; Wright, E.M.; Dholakia, K. Dynamics of microparticles trapped in a perfect vortex beam. Opt. Lett. 2013, 38, 4919–4922. [Google Scholar] [CrossRef]
- Vaity, P.; Rusch, L. Perfect vortex beam: Fourier transformation of a Bessel beam. Opt. Lett. 2015, 40, 597–600. [Google Scholar] [CrossRef]
- Pinnell, J.; Rodríguez-Fajardo, V.; Forbes, A. How perfect are perfect vortex beams? Opt. Lett. 2019, 44, 5614–5617. [Google Scholar] [CrossRef]
- Li, H.; Liu, J.; Ding, W.; Bai, L.; Wu, Z. Propagation of arbitrarily polarized terahertz Bessel vortex beam in inhomogeneous unmagnetized plasma slab. Phys. Plasmas 2018, 25, 123505. [Google Scholar] [CrossRef]
- Durrani, I. Photon orbital angular momentum in a plasma vortex. Bull. Pure Appl. Sci.-Phys. 2012, 31, 103–111. [Google Scholar]
- Li, H.; Honary, F.; Wu, Z.; Shang, Q.; Bai, L. Reflection, transmission, and absorption of vortex beams propagation in an inhomogeneous magnetized plasma slab. IEEE Trans. Antennas Propag. 2018, 66, 4194–4201. [Google Scholar] [CrossRef]
- Li, J.S.; Cheng, J.; Zhang, D.P. Terahertz Bessel beam generator. Appl. Opt. 2023, 62, 4197–4202. [Google Scholar] [CrossRef]
- Zhang, K.; Yuan, Y.; Zhang, D.; Ding, X.; Ratni, B.; Burokur, S.N.; Lu, M.; Tang, K.; Wu, Q. Phase-engineered metalenses to generate converging and non-diffractive vortex beam carrying orbital angular momentum in microwave region. Opt. Express 2018, 26, 1351–1360. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Knyazev, B.; Osintseva, N.; Komlenok, M.; Pavelyev, V.; Gerasimov, V.; Kameshkov, O.; Choporova, Y.; Tukmakov, K. Terahertz Bessel Beams Formed by Binary and Holographic Axicons. Photonics 2023, 10, 700. https://doi.org/10.3390/photonics10060700
Knyazev B, Osintseva N, Komlenok M, Pavelyev V, Gerasimov V, Kameshkov O, Choporova Y, Tukmakov K. Terahertz Bessel Beams Formed by Binary and Holographic Axicons. Photonics. 2023; 10(6):700. https://doi.org/10.3390/photonics10060700
Chicago/Turabian StyleKnyazev, Boris, Natalya Osintseva, Maxim Komlenok, Vladimir Pavelyev, Vasily Gerasimov, Oleg Kameshkov, Yulia Choporova, and Konstantin Tukmakov. 2023. "Terahertz Bessel Beams Formed by Binary and Holographic Axicons" Photonics 10, no. 6: 700. https://doi.org/10.3390/photonics10060700
APA StyleKnyazev, B., Osintseva, N., Komlenok, M., Pavelyev, V., Gerasimov, V., Kameshkov, O., Choporova, Y., & Tukmakov, K. (2023). Terahertz Bessel Beams Formed by Binary and Holographic Axicons. Photonics, 10(6), 700. https://doi.org/10.3390/photonics10060700






