High-Precision Laser Self-Mixing Displacement Sensor Based on Orthogonal Signal Phase Multiplication Technique
Abstract
:1. Introduction
2. Measurement Principle
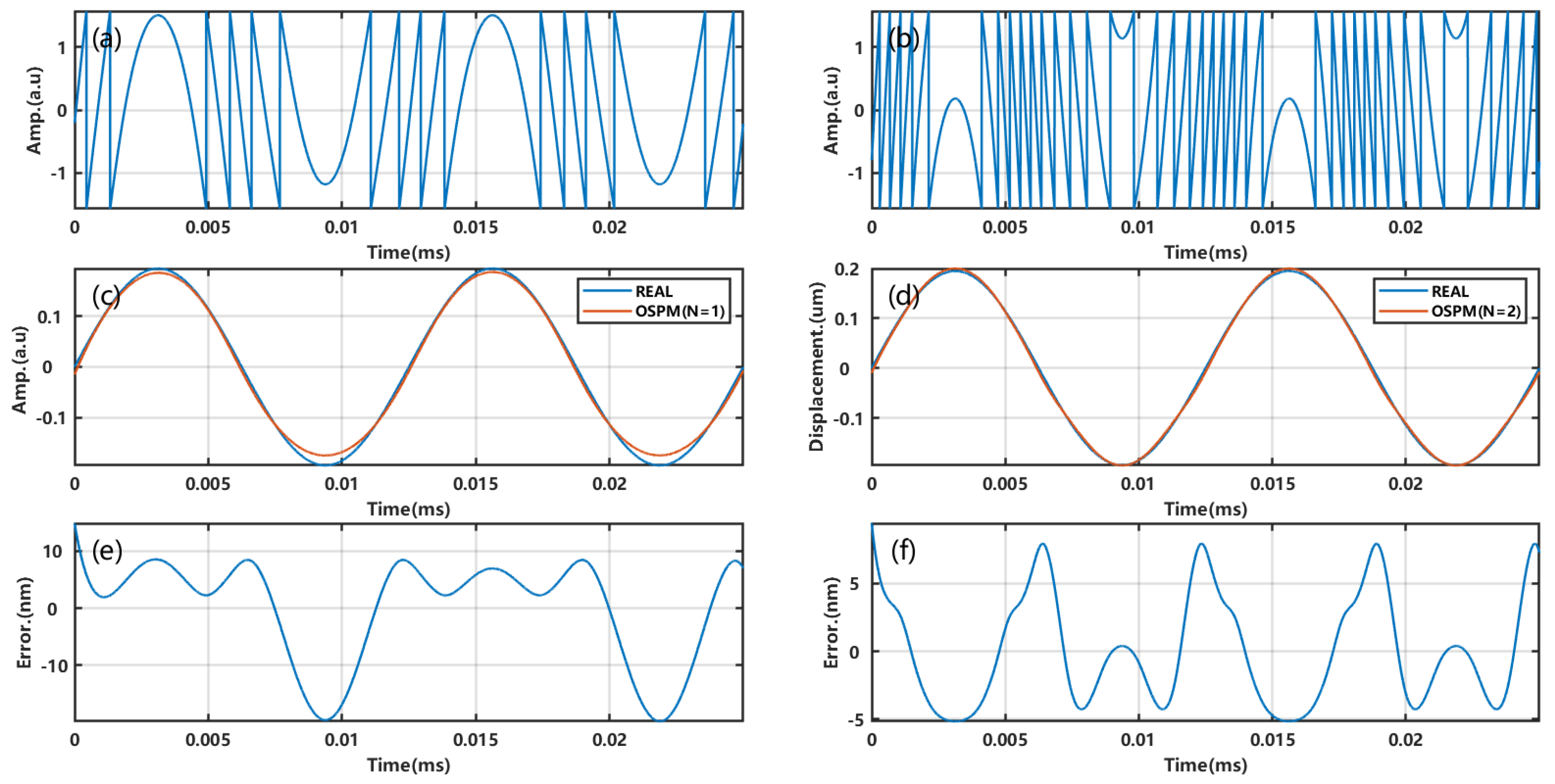
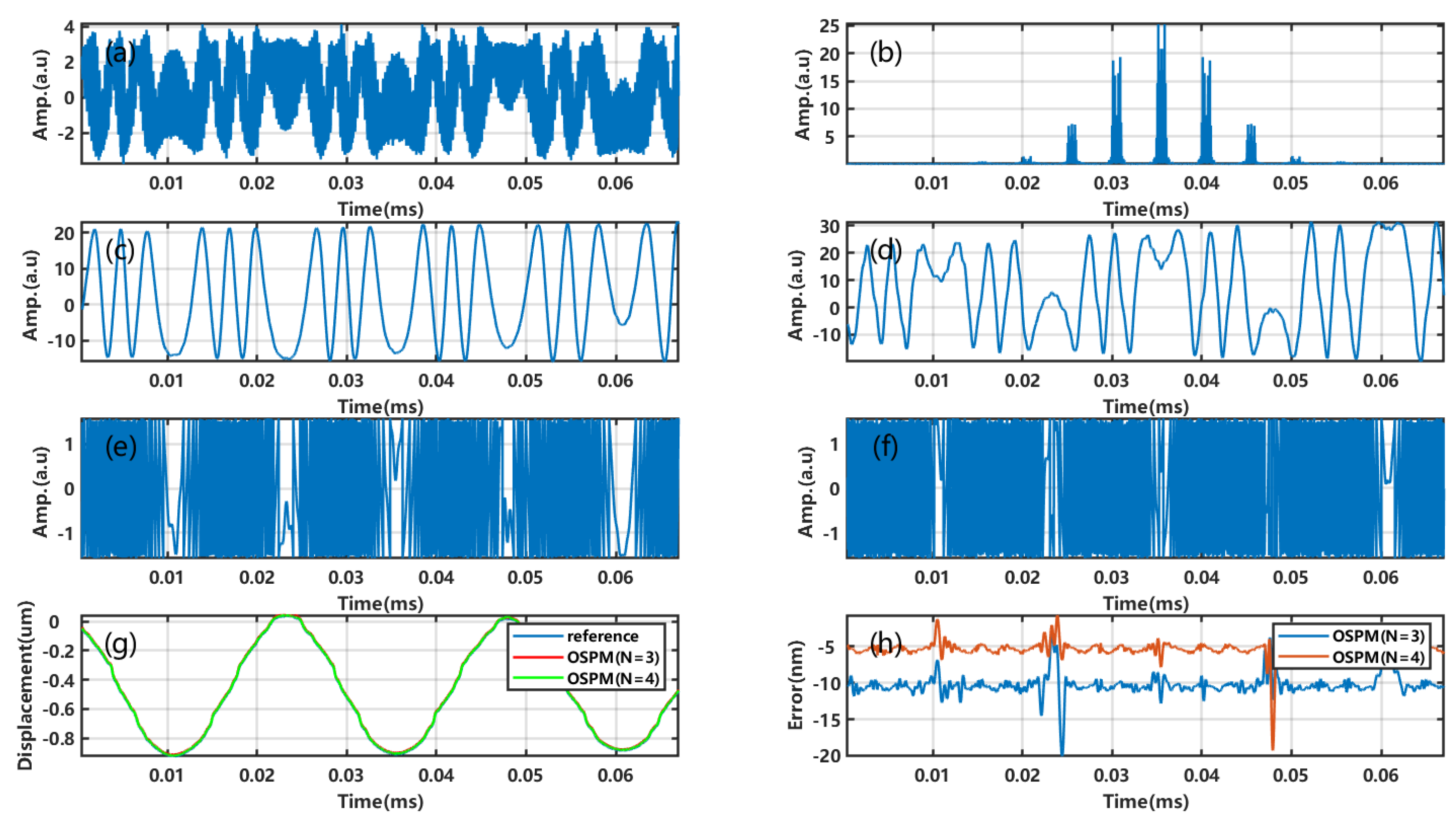
3. Simulations and Experiments
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, H.-J.; Deibel, J.; Nyberg, S.; Riles, K. High-precision absolute distance and vibration measurement with frequency scanned interferometry. Appl. Opt. 2005, 44, 3937–3944. [Google Scholar] [CrossRef] [PubMed]
- Pfister, T.; Buttner, L.; Czarske, J.; Krain, H.; Schodl, R. Turbo machine tip clearance and vibration measurements using a fibre optic laser Doppler position sensor. Meas. Sci. Technol. 2006, 17, 1693–1705. [Google Scholar] [CrossRef]
- Donati, S. Developing self-mixing interferometry for instrumentation and measurements. Laser Photonics Rev. 2012, 6, 393–417. [Google Scholar] [CrossRef]
- Chen, W.; Zhang, S.; Long, X. Polarisation control through an optical feedback technique and its application in precise measurements. Sci. Rep. 2013, 3, 1992. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wang, R.; Wei, Z.; Wang, X.; Xu, H.; Sun, H.; Huang, W. Broad Range and High Precision Self-Mixing Interferometer Based on Spectral Analysis with Multiple Reflections. IEEE Sens. J. 2018, 19, 926–932. [Google Scholar] [CrossRef]
- Guo, D.; Wang, M. Self-mixing interferometry based on a double-modulation technique for absolute distance measurement. Appl. Opt. 2007, 46, 1486–1491. [Google Scholar] [CrossRef]
- Duan, Z.; Yu, Y.; Gao, B.; Jiang, C. Absolute distance measurement based on multiple self-mixing interferometry. Opt. Commun. 2017, 389, 270–274. [Google Scholar] [CrossRef]
- Kou, K.; Wang, C.; Liu, Y. All-phase FFT based distance measurement in laser self-mixing interferometry. Opt. Lasers Eng. 2021, 142, 106611. [Google Scholar] [CrossRef]
- Li, L.; Zhang, Y.; Zhu, Y.; Dai, Y.; Zhang, X.; Liang, X. Absolute Distance Measurement Based on Self-Mixing Interferometry Using Compressed Sensing. Appl. Sci. 2022, 12, 8635. [Google Scholar] [CrossRef]
- Donati, S.; Rossi, D.; Norgia, M. Single Channel Self-Mixing Interferometer Measures Simultaneously Displace0ment and Tilt and Yaw Angles of a Reflective Target. IEEE J. Quantum Electron. 2015, 51, 1–8. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, H. Angle measurement method based on speckle affected laser self-mixing interference signal. Opt. Commun. 2021, 482, 126569. [Google Scholar] [CrossRef]
- Xu, X.; Dai, Z.; Wang, Y.; Li, M.; Tan, Y. High Sensitivity and Full-Circle Optical Rotary Sensor for Non-Cooperatively Tracing Wrist Tremor with Nanoradian Resolution. IEEE Trans. Ind. Electron. 2021, 69, 9605–9612. [Google Scholar] [CrossRef]
- Wang, X.; Yuan, Y.; Chen, P.; Gao, B. Laser self-mixing based on peak–valley point detection algorithm for displacement reconstruction. Opt. Quantum Electron. 2020, 52, 34. [Google Scholar] [CrossRef]
- Amin, S.; Zabit, U.; Bernal, O.D.; Hussain, T. High Resolution Laser Self-Mixing Displacement Sensor Under Large Variation in Optical Feedback and Speckle. IEEE Sens. J. 2020, 20, 9140–9147. [Google Scholar] [CrossRef]
- Wang, Y.; Li, Y.; Xu, X.; Tian, M.; Zhu, K.; Tan, Y. All-fiber laser feedback interferometry with 300 m transmission distance. Opt. Lett. 2021, 46, 821–824. [Google Scholar] [CrossRef]
- Ge, S.; Lin, Y.; Chen, H.; Kong, X.; Zhu, D.; Dong, Z.; Wang, X.; Huang, W. Signal extraction method based on spectral processing for a dual-channel SMI vibration sensor. Opt. Lasers Eng. 2023, 164, 107531. [Google Scholar] [CrossRef]
- Wu, S.; Wang, D.; Xiang, R.; Zhou, J.; Ma, Y.; Gui, H.; Liu, J.; Wang, H.; Lu, L.; Yu, B. All-Fiber Configuration Laser Self-Mixing Doppler Velocimeter Based on Distributed Feedback Fiber Laser. Sensors 2016, 16, 1179. [Google Scholar] [CrossRef]
- Jiang, C.; Geng, Y.; Liu, Y.; Liu, Y.; Chen, P.; Yin, S. Rotation velocity measurement based on self-mixing interference with a dual-external-cavity single-laser diode. Appl. Opt. 2019, 58, 604–608. [Google Scholar] [CrossRef]
- Wang, X.; Yang, H.; Hu, L.; Li, Z.; Chen, H.; Huang, W. Single Channel Instrument for Simultaneous Rotation Speed and Vibration Measurement Based on Self-Mixing Speckle Interference. IEEE Photonics J. 2021, 14, 6802405. [Google Scholar] [CrossRef]
- Tan, Y.; Zhang, S.; Xu, C.; Zhao, S. Inspecting and locating foreign body in biological sample by laser confocal feedback technology. Appl. Phys. Lett. 2013, 103, 101909. [Google Scholar] [CrossRef]
- Zhao, Y.; Xu, G.; Zhang, C.; Liu, K.; Lu, L. Vibration displacement immunization model for measuring the free spectral range by means of a laser self-mixing velocimeter. Appl. Opt. 2019, 58, 5540–5546. [Google Scholar] [CrossRef] [PubMed]
- Zhou, B.; Wang, Z.; Shen, X.; Zhang, L.; Tan, Y. High-sensitivity laser confocal tomography based on frequency-shifted feedback technique. Opt. Lasers Eng. 2020, 129, 106059. [Google Scholar] [CrossRef]
- Liu, B.; Ruan, Y.; Yu, Y. Determining System Parameters and Target Movement Directions in a Laser Self-Mixing Interferometry Sensor. Photonics 2022, 9, 612. [Google Scholar] [CrossRef]
- Donati, S.; Giuliani, G.; Merlo, S. Laser diode feedback interferometer for measurement of displacements without ambiguity. IEEE J. Quantum Electron. 1995, 31, 113–119. [Google Scholar] [CrossRef]
- Bes, C.; Plantier, G.; Bosch, T. Displacement Measurements Using a Self-Mixing Laser Diode Under Moderate Feedback. IEEE Trans. Instrum. Meas. 2006, 55, 1101–1105. [Google Scholar] [CrossRef]
- Guo, D.; Wang, M.; Tan, S. Self-mixing interferometer based on sinusoidal phase modulating technique. Opt. Express 2005, 13, 1537–1543. [Google Scholar] [CrossRef]
- Guo, D. Quadrature demodulation technique for self-mixing interferometry displacement sensor. Opt. Commun. 2011, 284, 5766–5769. [Google Scholar] [CrossRef]
- Xia, W.; Wang, M.; Yang, Z.; Guo, W.; Hao, H.; Guo, D. High-accuracy sinusoidal phase-modulating self-mixing interferometer using an electro-optic modulator: Development and evaluation. Appl. Opt. 2013, 52, B52–B59. [Google Scholar] [CrossRef]
- Ali, N.; Zabit, U.; Bernal, O.D. Nanometric Vibration Sensing Using Spectral Processing of Laser Self-Mixing Feedback Phase. IEEE Sens. J. 2021, 21, 17766–17774. [Google Scholar] [CrossRef]
- Lu, L.; Hu, L.; Li, Z.; Qiu, L.; Huang, W.; Wang, X. High Precision Self-Mixing Interferometer Based on Reflective Phase Modulation Method. IEEE Access 2020, 8, 204153–204159. [Google Scholar] [CrossRef]
- De Groot, P.J.; Gallatin, G.M.; Macomber, S.H. Ranging and velocimetry signal generation in a backscatter-modulated laser diode. Appl. Opt. 1988, 27, 4475–4480. [Google Scholar] [CrossRef] [PubMed]
- Cui, X.; Liu, Y.; Chen, P.; Li, C. Measuring two vibrations using dual-external-cavity structure in a self-mixing system. Opt. Lasers Eng. 2021, 141, 106557. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Zhong, Z.; Chen, H.; Zhu, D.; Zheng, T.; Huang, W. High-Precision Laser Self-Mixing Displacement Sensor Based on Orthogonal Signal Phase Multiplication Technique. Photonics 2023, 10, 575. https://doi.org/10.3390/photonics10050575
Wang X, Zhong Z, Chen H, Zhu D, Zheng T, Huang W. High-Precision Laser Self-Mixing Displacement Sensor Based on Orthogonal Signal Phase Multiplication Technique. Photonics. 2023; 10(5):575. https://doi.org/10.3390/photonics10050575
Chicago/Turabian StyleWang, Xiulin, Zhengjian Zhong, Hanqiao Chen, Desheng Zhu, Tongchang Zheng, and Wencai Huang. 2023. "High-Precision Laser Self-Mixing Displacement Sensor Based on Orthogonal Signal Phase Multiplication Technique" Photonics 10, no. 5: 575. https://doi.org/10.3390/photonics10050575
APA StyleWang, X., Zhong, Z., Chen, H., Zhu, D., Zheng, T., & Huang, W. (2023). High-Precision Laser Self-Mixing Displacement Sensor Based on Orthogonal Signal Phase Multiplication Technique. Photonics, 10(5), 575. https://doi.org/10.3390/photonics10050575




