Method for the Quantum Metric Tensor Measurement in a Continuous Variable System
Abstract
1. Introduction
2. Quantum Metric Tensorl (QMT)
3. The KNPO as the Continuous Variable System
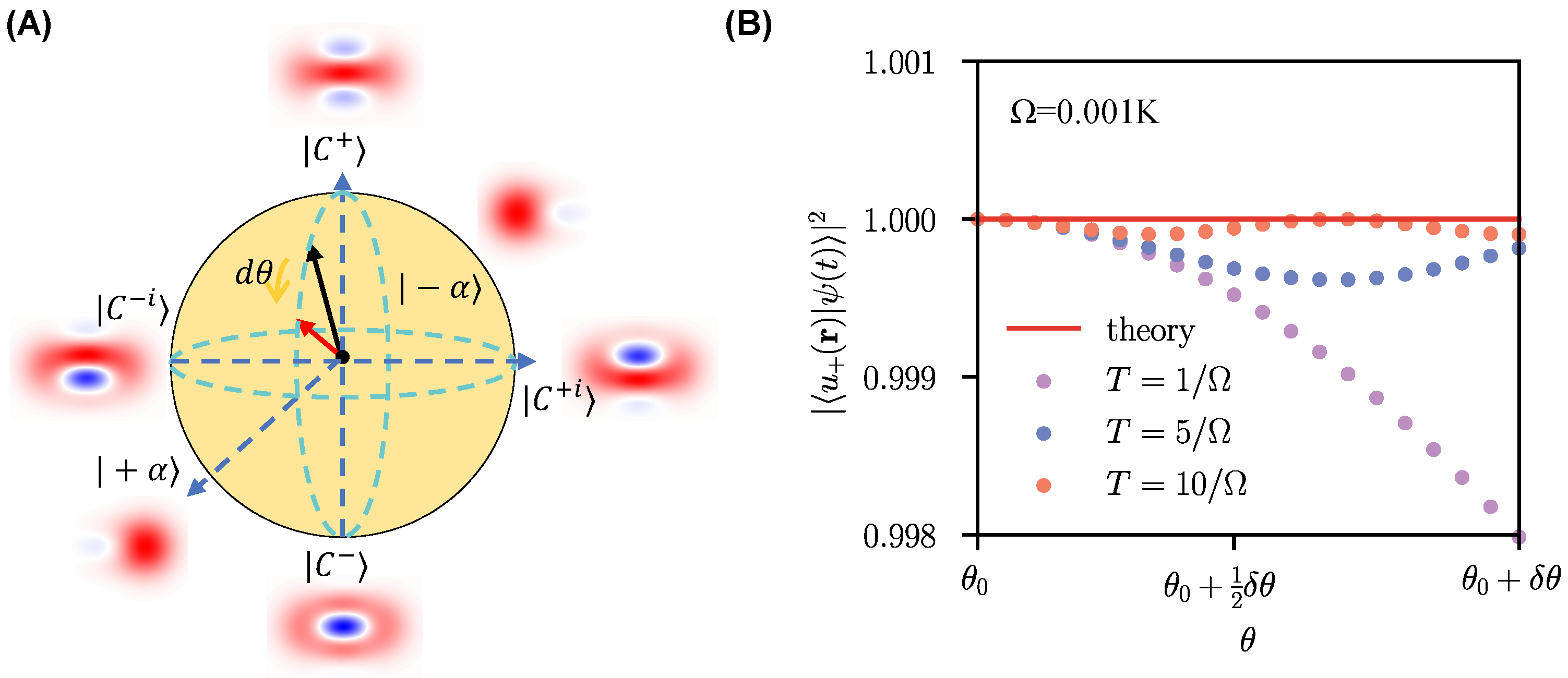
4. Arbitrary Manipulation of the Continuous Variable System
5. Method for the Measurement of the QMT
6. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| QGT | quantum geometric tensor |
| KNPO | Kerr nonlinear parametric oscillator |
| SQUID | superconducting quantum interference device |
| ECN | Euler characteristic number |
Appendix A. Quantum Geometric Tensor
Appendix B. Kerr Nonlinear Parametric Oscillator
References
- Kibble, T.W.B. Geometrization of quantum mechanics. Commun. Math. Phys. 1979, 65, 189–201. [Google Scholar] [CrossRef]
- Anandan, J.; Aharonov, Y. Geometry of quantum evolution. Phys. Rev. Lett. 1990, 65, 1697–1700. [Google Scholar] [CrossRef] [PubMed]
- Brody, D.C.; Hughston, L.P. Geometric quantum mechanics. J. Geom. Phys. 2001, 38, 19–53. [Google Scholar] [CrossRef]
- Kolodrubetz, M.; Sels, D.; Mehta, P.; Polkovnikov, A. Geometry and non-adiabatic response in quantum and classical systems. Phys. Rep. 2017, 697, 1–87. [Google Scholar] [CrossRef]
- Campos Venuti, L.; Zanardi, P. Quantum Critical Scaling of the Geometric Tensors. Phys. Rev. Lett. 2007, 99, 095701. [Google Scholar] [CrossRef]
- Ma, Y.Q.; Chen, S.; Fan, H.; Liu, W.M. Abelian and non-Abelian quantum geometric tensor. Phys. Rev. B 2010, 81, 245129. [Google Scholar] [CrossRef]
- Cheng, R. Quantum Geometric Tensor (Fubini-Study Metric) in Simple Quantum system. arXiv 2013, arXiv:1502.03551. [Google Scholar]
- Provost, J.P.; Vallee, G. Riemannian structure on manifolds of quantum states. Commun. Math. Phys. 1980, 76, 289–301. [Google Scholar] [CrossRef]
- Aharonov, Y.; Bohm, D. Significance of Electromagnetic Potentials in the Quantum Theory. Phys. Rev. 1959, 115, 485–491. [Google Scholar] [CrossRef]
- Xiao, D.; Chang, M.C.; Niu, Q. Berry phase effects on electronic properties. Rev. Mod. Phys. 2010, 82, 1959–2007. [Google Scholar] [CrossRef]
- Bohm, A.R.; Mostafazadeh, A.; Koizumi, H.; Niu, Q.; Zwanziger, J. The Geometric Phase in Quantum Systems: Foundations, Mathematical Concepts, and Applications in Molecular and Condensed Matter Physics; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Pachos, J.; Zanardi, P.; Rasetti, M. Non-Abelian Berry connections for quantum computation. Phys. Rev. A 1999, 61, 010305. [Google Scholar] [CrossRef]
- Zanardi, P.; Rasetti, M. Holonomic quantum computation. Phys. Lett. A 1999, 264, 94–99. [Google Scholar] [CrossRef]
- Berry, M.V. Quantal phase factors accompanying adiabatic changes. Proc. R. Soc. Lond. A Math. Phys. Sci. 1984, 392, 45–57. [Google Scholar] [CrossRef]
- Hasan, M.Z.; Kane, C.L. Colloquium: Topological insulators. Rev. Mod. Phys. 2010, 82, 3045–3067. [Google Scholar] [CrossRef]
- Wen, X.G. Mean-field theory of spin-liquid states with finite energy gap and topological orders. Phys. Rev. B 1991, 44, 2664–2672. [Google Scholar] [CrossRef]
- Wen, X.G. Colloquium: Zoo of quantum-topological phases of matter. Rev. Mod. Phys. 2017, 89, 041004. [Google Scholar] [CrossRef]
- Zhang, D.W.; Zhu, Y.Q.; Zhao, Y.X.; Yan, H.; Zhu, S.L. Topological quantum matter with cold atoms. Adv. Phys. 2018, 67, 253–402. [Google Scholar] [CrossRef]
- Qi, X.L.; Zhang, S.C. Topological insulators and superconductors. Rev. Mod. Phys. 2011, 83, 1057–1110. [Google Scholar] [CrossRef]
- Armitage, N.; Mele, E.; Vishwanath, A. Weyl and Dirac semimetals in three-dimensional solids. Rev. Mod. Phys. 2018, 90, 015001. [Google Scholar] [CrossRef]
- Wootters, W.K. Statistical distance and Hilbert space. Phys. Rev. D 1981, 23, 357–362. [Google Scholar] [CrossRef]
- Braunstein, S.L.; Caves, C.M. Statistical distance and the geometry of quantum states. Phys. Rev. Lett. 1994, 72, 3439–3443. [Google Scholar] [CrossRef]
- Souza, I.; Wilkens, T.; Martin, R.M. Polarization and localization in insulators: Generating function approach. Phys. Rev. B 2000, 62, 1666–1683. [Google Scholar] [CrossRef]
- Ozawa, T.; Goldman, N. Probing localization and quantum geometry by spectroscopy. Phys. Rev. Res. 2019, 1, 032019. [Google Scholar] [CrossRef]
- Roy, R. Band geometry of fractional topological insulators. Phys. Rev. B 2014, 90, 165139. [Google Scholar] [CrossRef]
- Lim, L.K.; Fuchs, J.N.; Montambaux, G. Geometry of Bloch states probed by Stückelberg interferometry. Phys. Rev. A 2015, 92, 063627. [Google Scholar] [CrossRef]
- Palumbo, G.; Goldman, N. Revealing Tensor Monopoles through Quantum-Metric Measurements. Phys. Rev. Lett. 2018, 121, 170401. [Google Scholar] [CrossRef]
- Zanardi, P.; Paunković, N. Ground state overlap and quantum phase transitions. Phys. Rev. E 2006, 74, 031123. [Google Scholar] [CrossRef]
- Carollo, A.; Valenti, D.; Spagnolo, B. Geometry of quantum phase transitions. Phys. Rep. 2020, 838, 1–72. [Google Scholar] [CrossRef]
- Zanardi, P.; Giorda, P.; Cozzini, M. Information-Theoretic Differential Geometry of Quantum Phase Transitions. Phys. Rev. Lett. 2007, 99, 100603. [Google Scholar] [CrossRef]
- Rezakhani, A.T.; Abasto, D.F.; Lidar, D.A.; Zanardi, P. Intrinsic geometry of quantum adiabatic evolution and quantum phase transitions. Phys. Rev. A 2010, 82, 012321. [Google Scholar] [CrossRef]
- Ma, Y.Q.; Gu, S.J.; Chen, S.; Fan, H.; Liu, W.M. The Euler number of Bloch states manifold and the quantum phases in gapped fermionic systems. Europhys. Lett. 2013, 103, 10008. [Google Scholar] [CrossRef]
- Kolodrubetz, M.; Gritsev, V.; Polkovnikov, A. Classifying and measuring geometry of a quantum ground state manifold. Phys. Rev. B 2013, 88, 064304. [Google Scholar] [CrossRef]
- Neupert, T.; Chamon, C.; Mudry, C. Measuring the quantum geometry of Bloch bands with current noise. Phys. Rev. B 2013, 87, 245103. [Google Scholar] [CrossRef]
- Ozawa, T. Steady-state Hall response and quantum geometry of driven-dissipative lattices. Phys. Rev. B 2018, 97, 041108. [Google Scholar] [CrossRef]
- Bleu, O.; Malpuech, G.; Gao, Y.; Solnyshkov, D. Effective Theory of Nonadiabatic Quantum Evolution Based on the Quantum Geometric Tensor. Phys. Rev. Lett. 2018, 121, 020401. [Google Scholar] [CrossRef] [PubMed]
- Bleu, O.; Solnyshkov, D.D.; Malpuech, G. Measuring the quantum geometric tensor in two-dimensional photonic and exciton-polariton systems. Phys. Rev. B 2018, 97, 195422. [Google Scholar] [CrossRef]
- Klees, R.; Rastelli, G.; Cuevas, J.; Belzig, W. Microwave Spectroscopy Reveals the Quantum Geometric Tensor of Topological Josephson Matter. Phys. Rev. Lett. 2020, 124, 197002. [Google Scholar] [CrossRef]
- Tan, X.; Zhang, D.W.; Yang, Z.; Chu, J.; Zhu, Y.Q.; Li, D.; Yang, X.; Song, S.; Han, Z.; Li, Z.; et al. Experimental Measurement of the Quantum Metric Tensor and Related Topological Phase Transition with a Superconducting Qubit. Phys. Rev. Lett. 2019, 122, 210401. [Google Scholar] [CrossRef] [PubMed]
- Zheng, W.; Xu, J.; Ma, Z.; Li, Y.; Dong, Y.; Zhang, Y.; Wang, X.; Sun, G.; Wu, P.; Zhao, J.; et al. Measuring Quantum Geometric Tensor of Non-Abelian System in Superconducting Circuits. Chin. Phys. Lett. 2022, 39, 100202. [Google Scholar] [CrossRef]
- Yu, M.; Yang, P.; Gong, M.; Cao, Q.; Lu, Q.; Liu, H.; Zhang, S.; Plenio, M.B.; Jelezko, F.; Ozawa, T.; et al. Experimental measurement of the quantum geometric tensor using coupled qubits in diamond. Natl. Sci. Rev. 2019, 7, 254–260. [Google Scholar] [CrossRef] [PubMed]
- Chamberland, C.; Noh, K.; Arrangoiz-Arriola, P.; Campbell, E.T.; Hann, C.T.; Iverson, J.; Putterman, H.; Bohdanowicz, T.C.; Flammia, S.T.; Keller, A.; et al. Building a Fault-Tolerant Quantum Computer Using Concatenated Cat Codes. PRX Quantum 2022, 3, 010329. [Google Scholar] [CrossRef]
- Mirrahimi, M.; Leghtas, Z.; Albert, V.V.; Touzard, S.; Schoelkopf, R.J.; Jiang, L.; Devoret, M.H. Dynamically protected cat-qubits: A new paradigm for universal quantum computation. New J. Phys. 2014, 16, 045014. [Google Scholar] [CrossRef]
- Nigg, S.E. Deterministic Hadamard gate for microwave cat-state qubits in circuit QED. Phys. Rev. A 2014, 89, 022340. [Google Scholar] [CrossRef]
- Yi, X.; Yang, Q.F.; Zhang, X.; Yang, K.Y.; Li, X.; Vahala, K. Single-mode dispersive waves and soliton microcomb dynamics. Nat. Commun. 2017, 8, 14869. [Google Scholar] [CrossRef]
- Yang, C.P.; Su, Q.P.; Zheng, S.B.; Nori, F.; Han, S. Entangling two oscillators with arbitrary asymmetric initial states. Phys. Rev. A 2017, 95, 052341. [Google Scholar] [CrossRef]
- Wang, Z.; Pechal, M.; Wollack, E.A.; Arrangoiz-Arriola, P.; Gao, M.; Lee, N.R.; Safavi-Naeini, A.H. Quantum Dynamics of a Few-Photon Parametric Oscillator. Phys. Rev. X 2019, 9, 021049. [Google Scholar] [CrossRef]
- Puri, S.; Boutin, S.; Blais, A. Engineering the quantum states of light in a Kerr-nonlinear resonator by two-photon driving. NPJ Quantum Inf. 2017, 3, 18. [Google Scholar] [CrossRef]
- Goto, H. Universal quantum computation with a nonlinear oscillator network. Phys. Rev. A 2016, 93, 50301. [Google Scholar] [CrossRef]
- Grimm, A.; Frattini, N.E.; Puri, S.; Mundhada, S.O.; Touzard, S.; Mirrahimi, M.; Girvin, S.M.; Shankar, S.; Devoret, M.H. Stabilization and operation of a Kerr-cat qubit. Nature 2020, 584, 205–209. [Google Scholar] [CrossRef] [PubMed]
- Yurke, B.; Stoler, D. The dynamic generation of Schrödinger cats and their detection. Phys. B+C 1988, 151, 298–301. [Google Scholar] [CrossRef]
- Leghtas, Z.; Touzard, S.; Pop, I.M.; Kou, A.; Vlastakis, B.; Petrenko, A.; Sliwa, K.M.; Narla, A.; Shankar, S.; Hatridge, M.J.; et al. Confining the state of light to a quantum manifold by engineered two-photon loss. Science 2015, 347, 853–857. [Google Scholar] [CrossRef] [PubMed]
- Sugawa, S.; Salces-Carcoba, F.; Perry, A.R.; Yue, Y.; Spielman, I.B. Second Chern number of a quantum-simulated non-Abelian Yang monopole. Science 2018, 360, 1429–1434. [Google Scholar] [CrossRef] [PubMed]
- Weber, T.; Fobes, D.M.; Waizner, J.; Steffens, P.; Tucker, G.S.; Böhm, M.; Beddrich, L.; Franz, C.; Gabold, H.; Bewley, R.; et al. Topological magnon band structure of emergent Landau levels in a skyrmion lattice. Science 2022, 375, 1025–1030. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, L.-S.; Zhang, H.-L.; Yang, Z.-B. Method for the Quantum Metric Tensor Measurement in a Continuous Variable System. Photonics 2023, 10, 256. https://doi.org/10.3390/photonics10030256
Lin L-S, Zhang H-L, Yang Z-B. Method for the Quantum Metric Tensor Measurement in a Continuous Variable System. Photonics. 2023; 10(3):256. https://doi.org/10.3390/photonics10030256
Chicago/Turabian StyleLin, Ling-Shan, Hao-Long Zhang, and Zhen-Biao Yang. 2023. "Method for the Quantum Metric Tensor Measurement in a Continuous Variable System" Photonics 10, no. 3: 256. https://doi.org/10.3390/photonics10030256
APA StyleLin, L.-S., Zhang, H.-L., & Yang, Z.-B. (2023). Method for the Quantum Metric Tensor Measurement in a Continuous Variable System. Photonics, 10(3), 256. https://doi.org/10.3390/photonics10030256




