Dependence of the Registered Blood Flow in Incoherent Optical Fluctuation Flowmetry on the Mean Photon Path Length in a Tissue
Abstract
1. Introduction
2. Materials and Methods
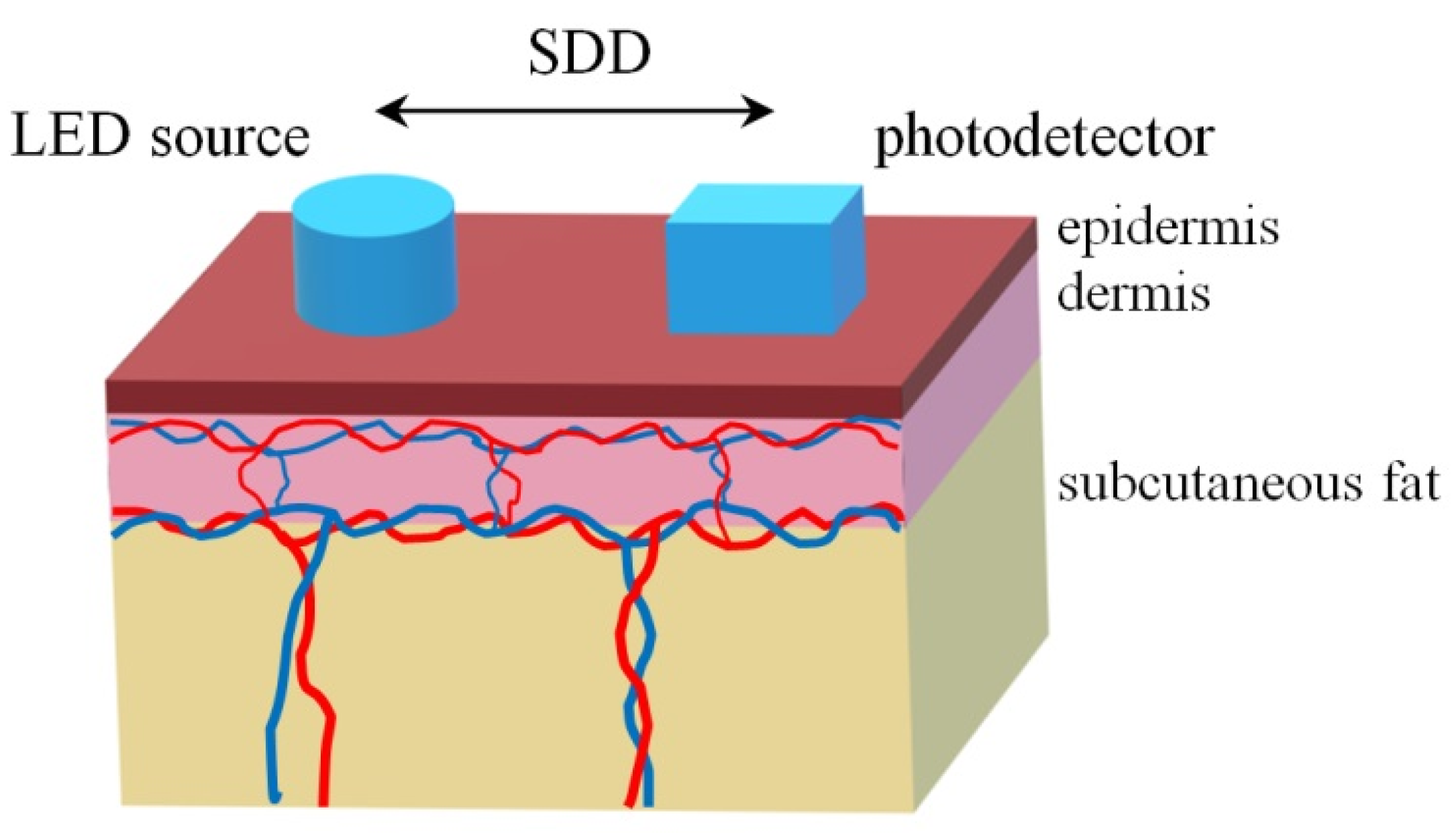
2.1. Optical Model of the IOFF Signal Formation
2.2. Monte Carlo Simulation
2.3. Optical Properties of Skin Layers
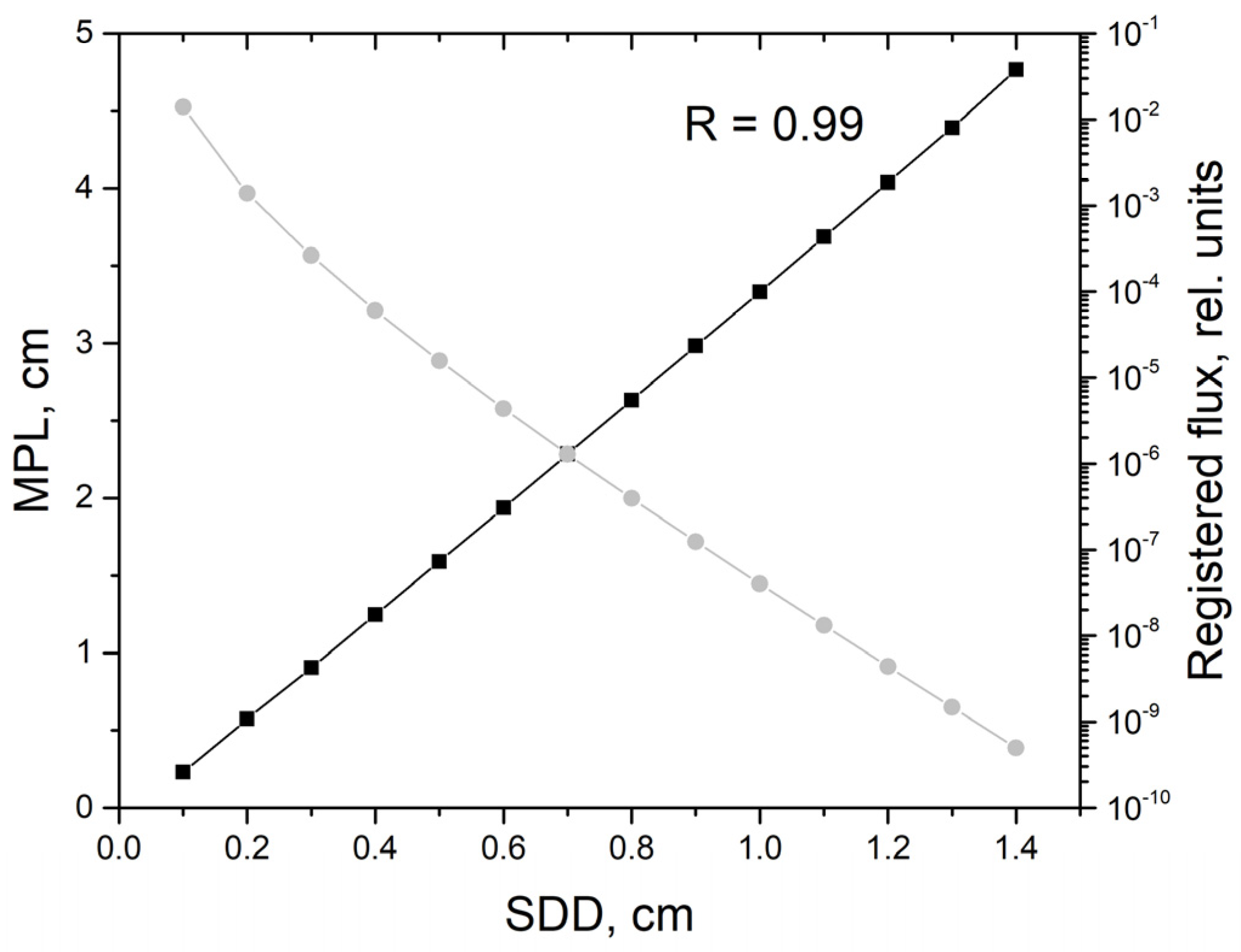
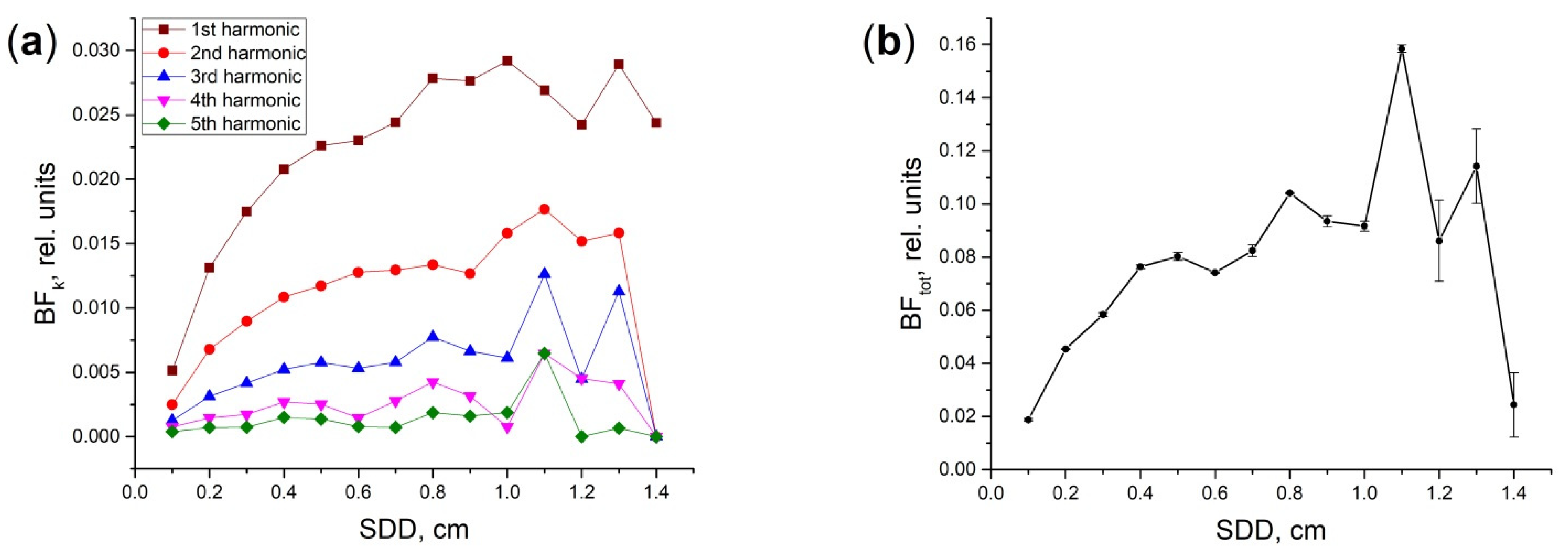
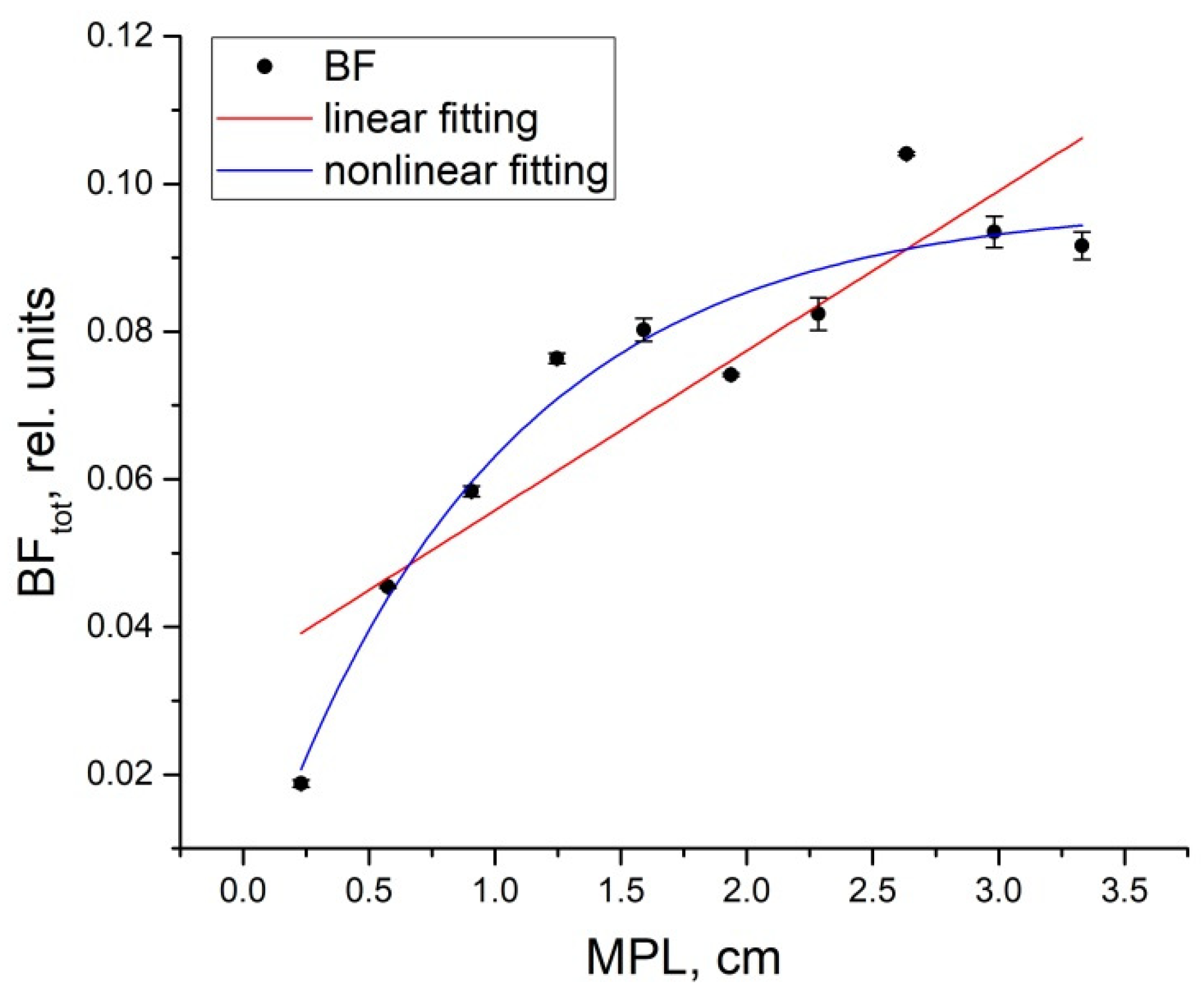
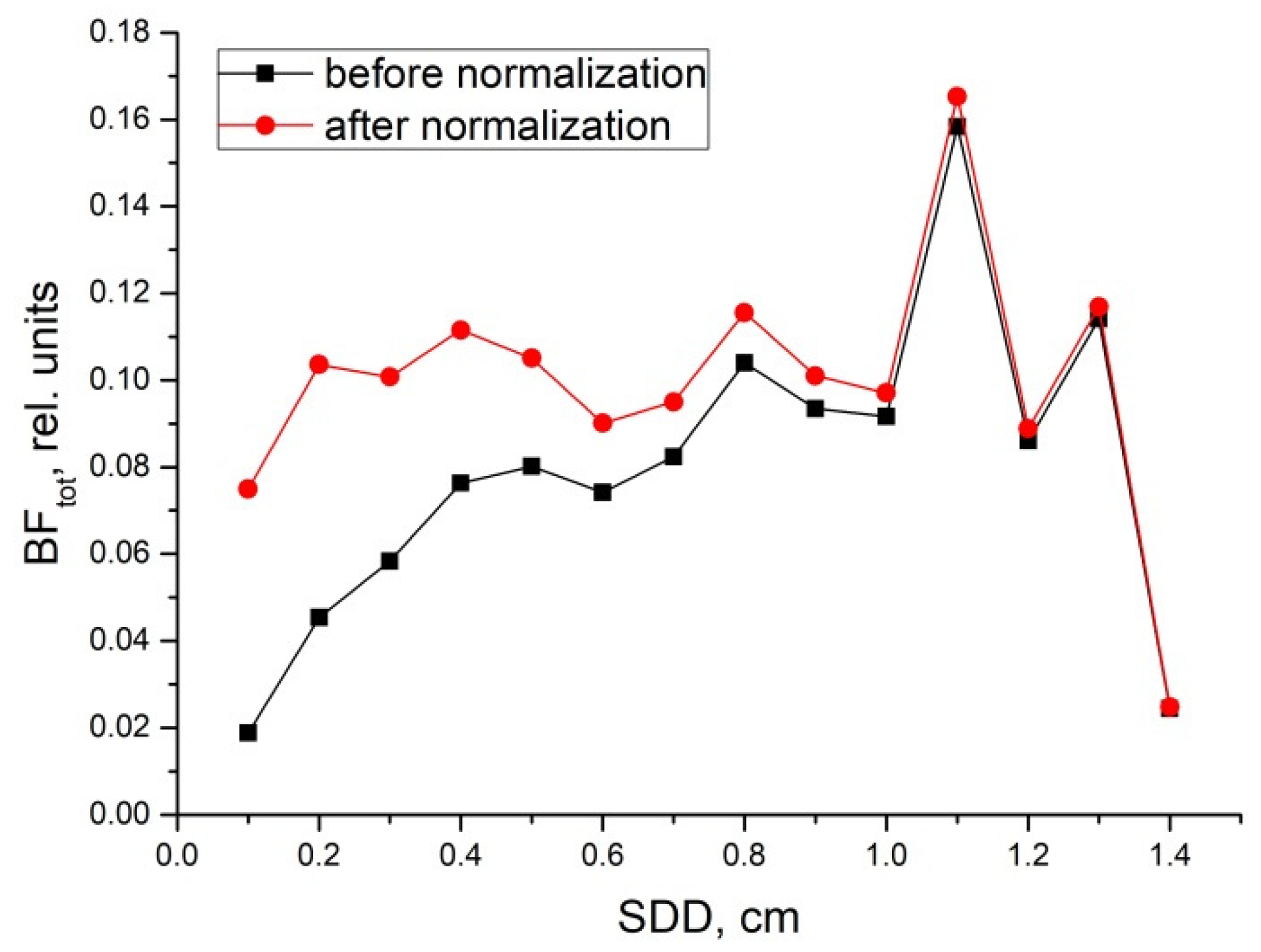
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Briers, J.D. Laser Doppler, speckle and related techniques for blood perfusion mapping and imaging. Physiol. Meas. 2001, 22, R35. [Google Scholar] [CrossRef] [PubMed]
- Yu, G. Diffuse correlation spectroscopy (DCS): A diagnostic tool for assessing tissue blood flow in vascular-related diseases and therapies. Curr. Med. Imaging 2012, 8, 194–210. [Google Scholar] [CrossRef]
- Rajan, V.; Varghese, B.; van Leeuwen, T.G.; Steenbergen, W. Review of methodological developments in laser Doppler flowmetry. Lasers Med. Sci. 2009, 24, 269–283. [Google Scholar] [CrossRef]
- Lapitan, D.G.; Rogatkin, D.A.; Tarasov, A.P. Model of Doppler scattering with variable blood volume in laser Doppler flowmetry. Proc. SPIE 2017, 10417, 104170V. [Google Scholar] [CrossRef]
- Lapitan, D.G.; Rogatkin, D.A. Functional studies on blood microcirculation system with laser Doppler flowmetry in clinical medicine: Problems and prospects. Alm. Clin. Med. 2016, 44, 249–259. [Google Scholar] [CrossRef]
- Kulikov, D.; Glazkov, A.; Dreval, A.; Kovaleva, Y.; Rogatkin, D.; Kulikov, A.; Molochkov, A. Approaches to improve the predictive value of laser Doppler flowmetry in detection of microcirculation disorders in diabetes mellitus. Clin. Hemorheol. Microcirc. 2018, 70, 173–179. [Google Scholar] [CrossRef]
- Rogatkin, D.A.; Lapaeva, L.G.; Bychenkov, O.A.; Tereshchenko, S.G.; Shumskii, V.I. Principal sources of errors in noninvasive medical spectrophotometry. Part 1. Physicotechnical sources and factors of errors. Meas. Technol. 2013, 56, 201–210. [Google Scholar] [CrossRef]
- Rogatkin, D.A.; Lapaeva, L.G.; Bychenkov, O.A.; Tereshchenko, S.G.; Shumskii, V.I. Principal sources of errors in noninvasive medical spectrophotometry. Part 2. Medicobiological factors of errors. Meas. Technol. 2013, 56, 455–463. [Google Scholar] [CrossRef]
- Reisner, A.; Shaltis, P.A.; McCombie, D.; Asada, H.H.; Warner, D.S.; Warner, M.A. Utility of the photoplethysmogram in circulatory monitoring. Anesthesiology 2008, 108, 950–958. [Google Scholar] [CrossRef]
- Allen, J. Photoplethysmography and its application in clinical physiological measurement. Physiol. Meas. 2007, 28, R1–R39. [Google Scholar] [CrossRef]
- Lapitan, D.; Rogatkin, D. Optical incoherent technique for noninvasive assessment of blood flow in tissues: Theoretical model and experimental study. J. Biophotonics 2021, 14, e202000459. [Google Scholar] [CrossRef] [PubMed]
- Lima, A.; Bakker, J. Noninvasive monitoring of peripheral perfusion. Intensive Care Med. 2005, 31, 1316–1326. [Google Scholar] [CrossRef]
- Thomas, D.L.; Lythgoe, M.F.; Pell, G.S.; Calamante, F.; Ordidge, R.J. The measurement of diffusion and perfusion in biological systems using magnetic resonance imaging. Phys. Med. Biol. 2000, 45, R97–R138. [Google Scholar] [CrossRef] [PubMed]
- Tarasov, A.; Lapitan, D.; Rogatkin, D. Combined non-invasive optical oximeter and flowmeter with basic metrological equipment. Photonics 2022, 9, 392. [Google Scholar] [CrossRef]
- Lapitan, D.G.; Raznitsyn, O.A. A method and a device prototype for noninvasive measurements of blood perfusion in a tissue. Instrum. Exp. Technol. 2018, 61, 745–750. [Google Scholar] [CrossRef]
- Liebert, A.; Leahy, M.; Maniewski, R. Multichannel laser-Doppler probe for blood perfusion measurements with depth discrimination. Med. Biol. Eng. Comput. 1998, 36, 740–747. [Google Scholar] [CrossRef] [PubMed]
- Fredriksson, I.; Larsson, M.; Strömberg, T. Measurement depth and volume in laser Doppler flowmetry. Microvasc. Res. 2009, 78, 4–13. [Google Scholar] [CrossRef]
- Han, G.; Feng, H.; Chen, S.; Guo, Q.; Wang, H. Optimization of source-detector separation for non-invasive regional cerebral blood flow sensing. Infrared Phys. Technol. 2021, 117, 103843. [Google Scholar] [CrossRef]
- Rogers, D.W.O. Fifty years of Monte Carlo simulations for medical physics. Phys. Med. Biol. 2006, 51, R287. [Google Scholar] [CrossRef]
- Tuchin, V.V. Tissue Optics; Society of Photo-Optical Instrumentation Engineers (SPIE): Bellingham, WA, USA, 2015. [Google Scholar]
- Lapitan, D.G.; Tarasov, A.P.; Rogatkin, D.A. Justification of the photoplethysmography sensor configuration by Monte Carlo modeling of the pulse waveform. J. Biomed. Photonics Eng. 2022, 8, 030306. [Google Scholar] [CrossRef]
- Reuss, J.L. Multilayer modeling of reflectance pulse oximetry. IEEE Trans. Biomed. Eng. 2005, 52, 153–159. [Google Scholar] [CrossRef]
- Dunaev, A.V.; Zherebtsov, E.A.; Rogatkin, D.A.; Stewart, N.A.; Sokolovski, E.U. Substantiation of medical and technical requirements for noninvasive spectrophotometric diagnostic devices. J. Biomed. Opt. 2013, 18, 107009. [Google Scholar] [CrossRef] [PubMed]
- Jacques, S.L. Skin Optics Summary. 1998. Available online: https://omlc.org/news/jan98/skinoptics.html (accessed on 30 November 2022).
- Saidi, I.S. Transcutaneous Optical Measurement of Hyperbilirubinemia in Neonates. Ph.D. Thesis, Rice University, Houston, TX, USA, 1992. [Google Scholar]
- Jacques, S.L. Origins of tissue optical properties in the UVA, visible, and NIR regions. OSA TOPS Adv. Opt. Imaging Phot. Migr. 1996, 2, 364–369. [Google Scholar]
- Chatterjee, S.; Budidha, K.; Kyriacou, P.A. Investigating the origin of photoplethysmography using a multiwavelength Monte Carlo model. Physiol. Meas. 2020, 41, 084001. [Google Scholar] [CrossRef] [PubMed]
- Meglinski, I.V.; Matcher, S.J. Computer simulation of the skin reflectance spectra. Comput. Methods Programs Biomed. 2003, 70, 179–186. [Google Scholar] [CrossRef]
- Prahl, S.A.; Keijzer, M.; Jacques, S.L.; Welch, A.J. A Monte Carlo model of light propagation in tissue. In Dosimetry of Laser Radiation in Medicine and Biology; Müller, G., Sliney, D., Eds.; SPIE Institute Series; SPIE: Bellingham, WA, USA, 1989; pp. 102–111. [Google Scholar]
- Wang, L.; Jacques, S.L.; Zheng, L. MCML—Monte Carlo modeling of light transport in multi-layered tissues. Comput. Meth. Programs Biomed. 1995, 47, 131–146. [Google Scholar] [CrossRef]
- Tarasov, A.P. Acceleration of Monte Carlo simulation of light transport in tissues using disk-detector geometry in the backscattering problem. In Proceedings of the 2020 International Conference Laser Optics (ICLO), Saint Petersburg, Russia, 2–6 November 2020. [Google Scholar] [CrossRef]
- Tarasov, A.P. Using disk-detector geometry for calculation of optical path length by Monte Carlo simulation of light transport in turbid media. In Proceedings of the 2022 International Conference Laser Optics (ICLO), St. Petersburg, Russia, 20–24 June 2022. [Google Scholar] [CrossRef]
- Bosschaart, N.; Edelman, G.J.; Aalders, M.C.; van Leeuwen, T.G.; Faber, D.J. A literature review and novel theoretical approach on the optical properties of whole blood. Lasers Med. Sci. 2014, 29, 453–479. [Google Scholar] [CrossRef]
- Hale, G.M.; Querry, M.R. Optical constants of water in the 200-nm to 200-m wavelength region. Appl. Opt. 1973, 2, 555–563. [Google Scholar] [CrossRef]
- Simpson, C.R.; Kohl, M.; Essenpreis, M.; Cope, M. Near-infrared optical properties of ex vivo human skin and subcutaneous tissues measured using the Monte Carlo inversion technique. Phys. Med. Biol. 1998, 43, 2465–2478. [Google Scholar] [CrossRef]
- Bashkatov, A.N.; Genina, E.A.; Tuchin, V.V. Optical properties of skin, subcutaneous, and muscle tissues: A review. J. Innov. Opt. Health Sci. 2011, 4, 9–38. [Google Scholar] [CrossRef]
- Moço, A.V.; Stuijk, S.; de Haan, G. New insights into the origin of remote PPG signals in visible light and infrared. Sci. Rep. 2018, 8, 8501. [Google Scholar] [CrossRef]
- Jacques, S.L. Optical properties of biological tissues: A review. Phys. Med. Biol. 2013, 58, R37–R61. [Google Scholar] [CrossRef] [PubMed]
- Salomatina, E.; Jiang, B.; Novak, J.; Yaroslavsky, A.N. Optical properties of normal and cancerous human skin in the visible and near-infrared spectral range. J. Biomed. Opt. 2006, 11, 064026. [Google Scholar] [CrossRef] [PubMed]
- Weiss, G.H.; Nossal, R.; Bonner, R.F. Statistics of penetration depth of photons re-emitted from irradiated tissue. J. Mod. Opt. 1989, 36, 349–359. [Google Scholar] [CrossRef]
| Layer | Thickness, mm | Vmel, rel. Units | Vw, rel. Units | Vb,A, rel. Units | Vb,V, rel. Units |
|---|---|---|---|---|---|
| Epidermis | 0.2 | 0.1 | 0.2 | – | – |
| Dermis | 0.7 | – | 0.6 | 0.05 | 0.05 |
| Subcutaneous fat | ∞ | – | 0.15 | 0.025 | 0.025 |
| Optical Parameter | Chromophore | ||||
|---|---|---|---|---|---|
| Melanin | Water | Arterial Blood (Hct = 45%) | Venous Blood (Hct = 45%) | Fat | |
| , cm−1 | 136.2 | 0.267 | 4.02 | 4.17 | 1.38 |
| Layer | |||||
| Epidermis | Dermis | Subcutaneous fat | |||
| , cm−1 | 183.9 | 111.1 | 102.7 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lapitan, D.G.; Tarasov, A.P.; Rogatkin, D.A. Dependence of the Registered Blood Flow in Incoherent Optical Fluctuation Flowmetry on the Mean Photon Path Length in a Tissue. Photonics 2023, 10, 190. https://doi.org/10.3390/photonics10020190
Lapitan DG, Tarasov AP, Rogatkin DA. Dependence of the Registered Blood Flow in Incoherent Optical Fluctuation Flowmetry on the Mean Photon Path Length in a Tissue. Photonics. 2023; 10(2):190. https://doi.org/10.3390/photonics10020190
Chicago/Turabian StyleLapitan, Denis G., Andrey P. Tarasov, and Dmitry A. Rogatkin. 2023. "Dependence of the Registered Blood Flow in Incoherent Optical Fluctuation Flowmetry on the Mean Photon Path Length in a Tissue" Photonics 10, no. 2: 190. https://doi.org/10.3390/photonics10020190
APA StyleLapitan, D. G., Tarasov, A. P., & Rogatkin, D. A. (2023). Dependence of the Registered Blood Flow in Incoherent Optical Fluctuation Flowmetry on the Mean Photon Path Length in a Tissue. Photonics, 10(2), 190. https://doi.org/10.3390/photonics10020190





