Introduction of Research Work on Laser Proton Acceleration and Its Application Carried out on Compact Laser–Plasma Accelerator at Peking University
Abstract
1. Introduction
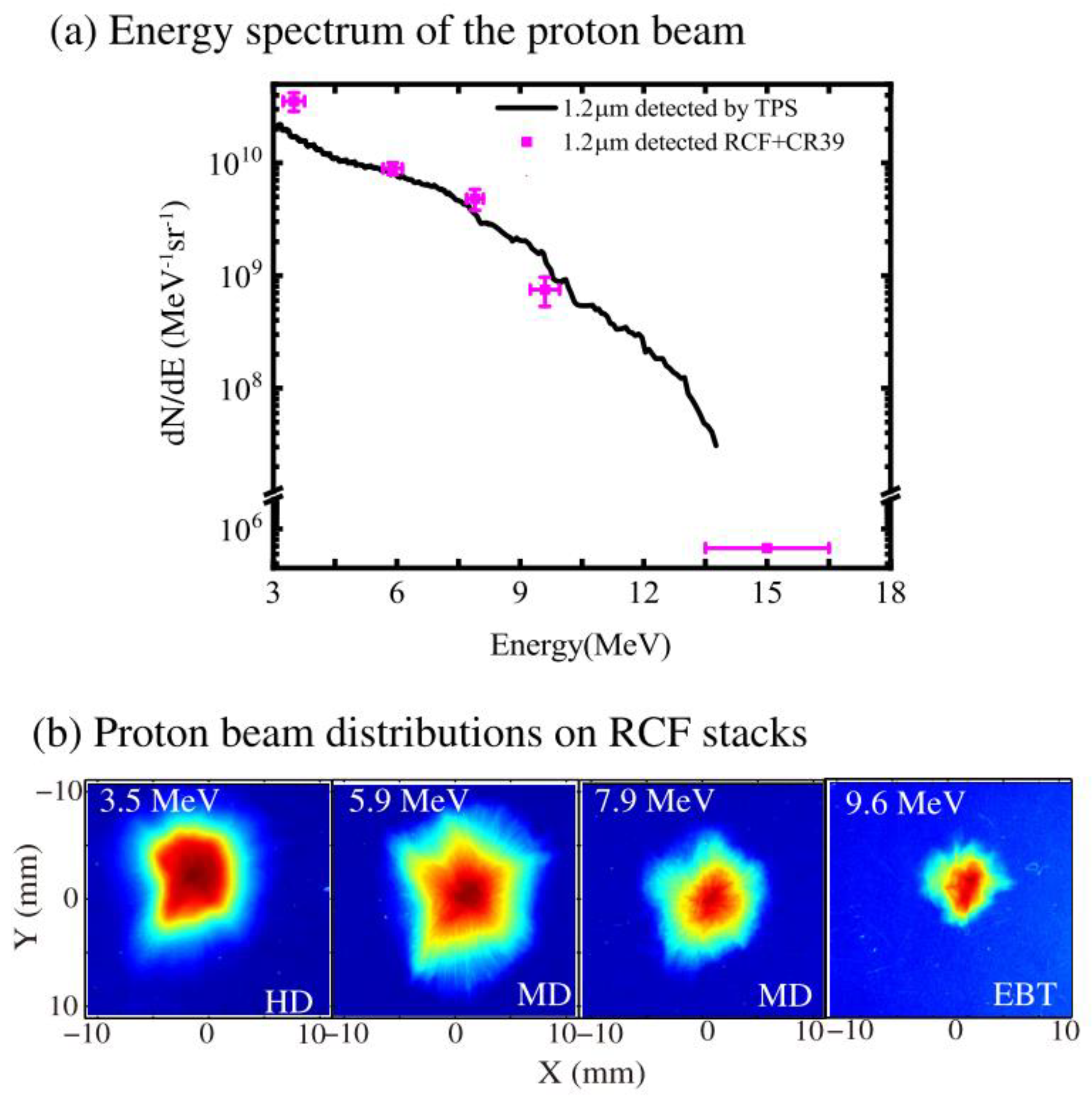
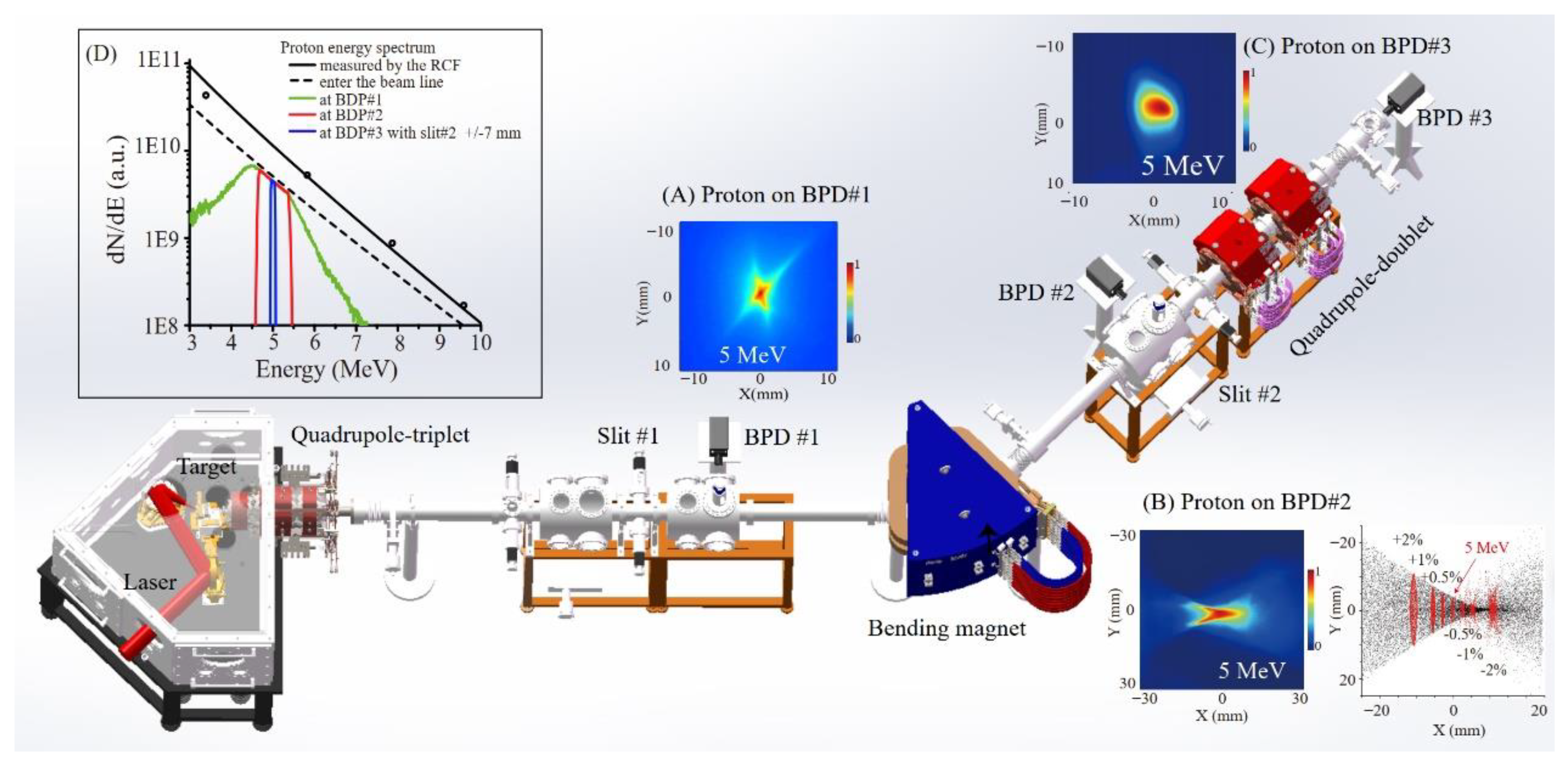
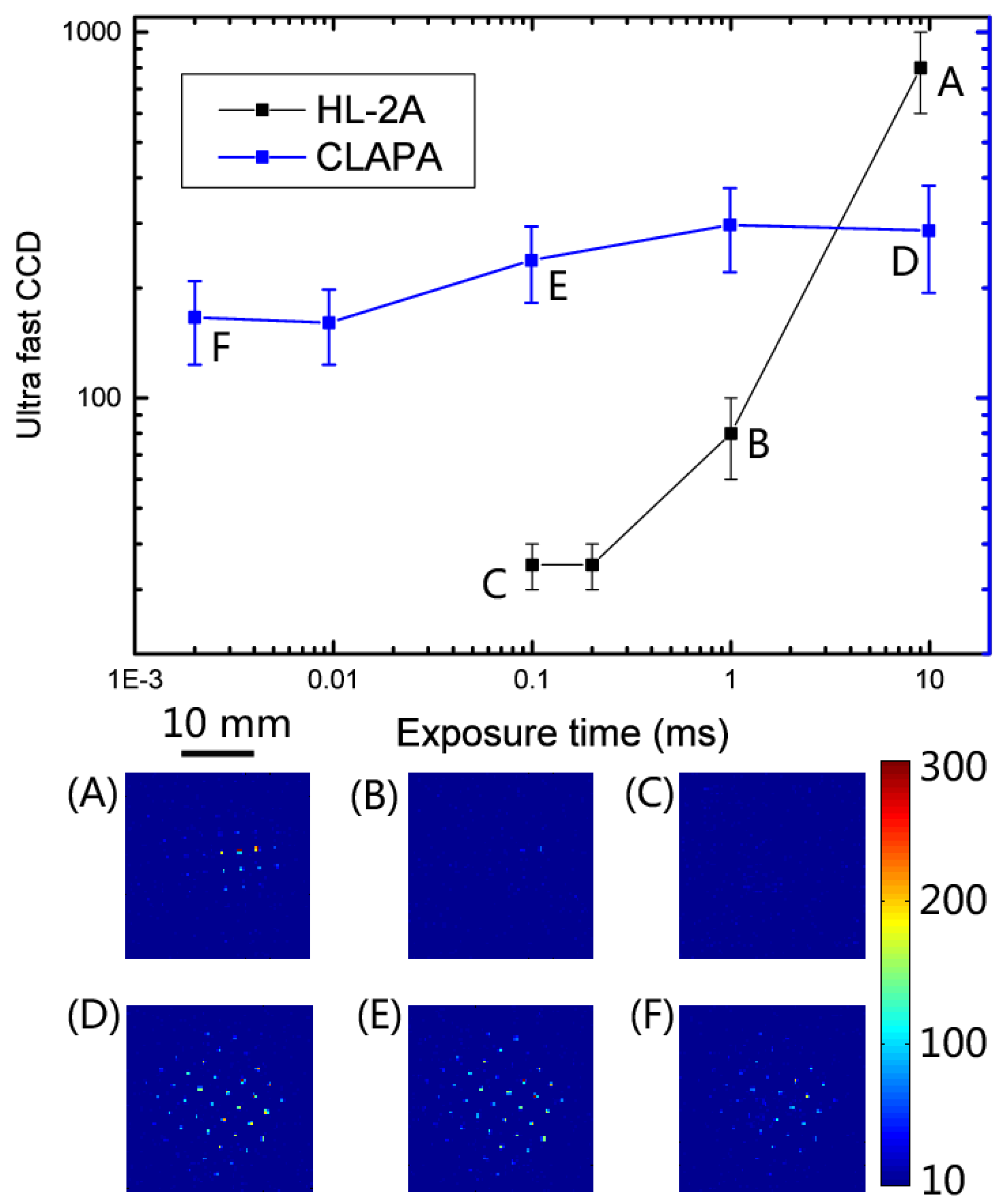
2. Experimental Demonstration of a Laser Proton Accelerator with Accurate Beam Control through Image-Relaying Transport
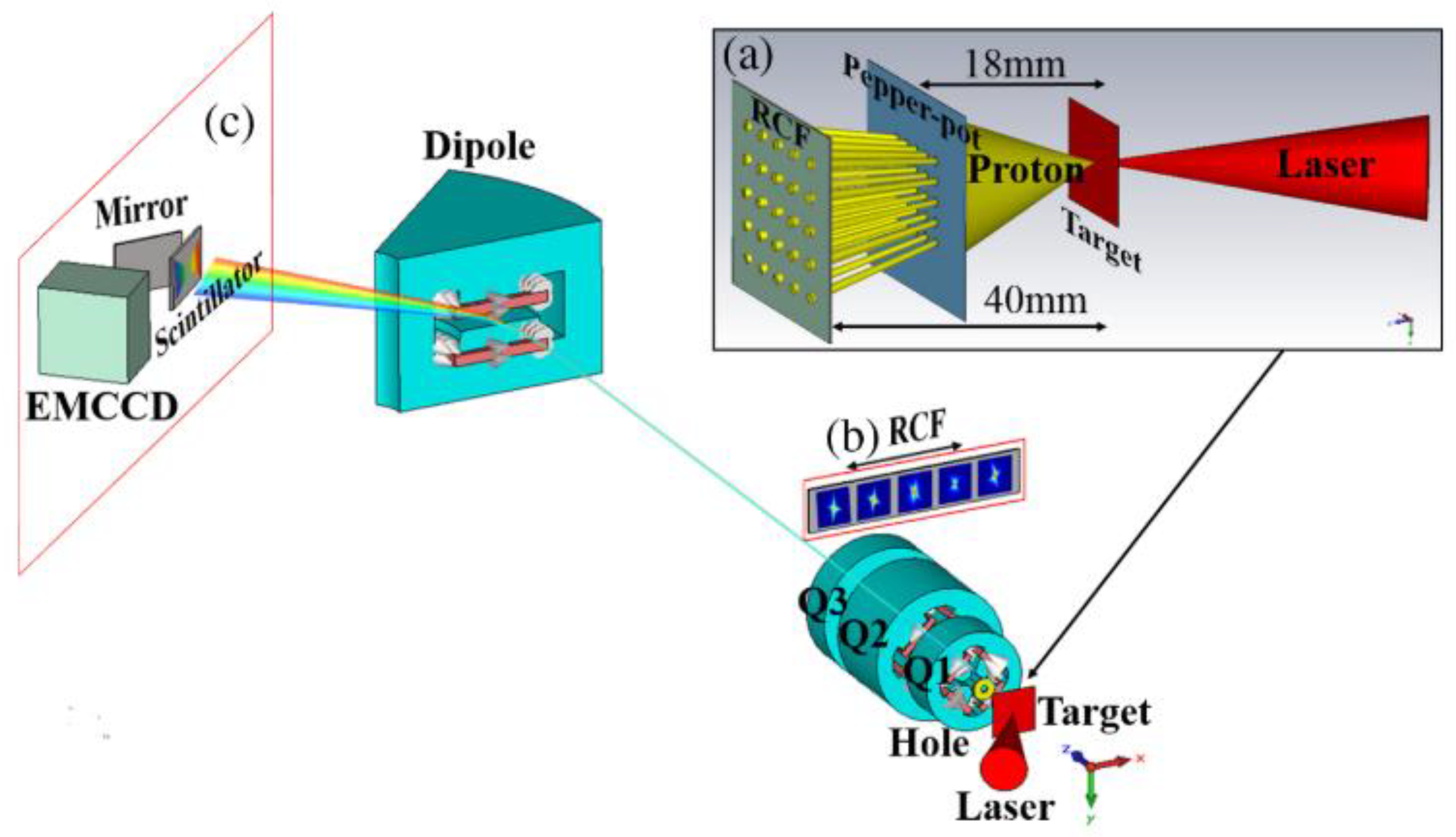
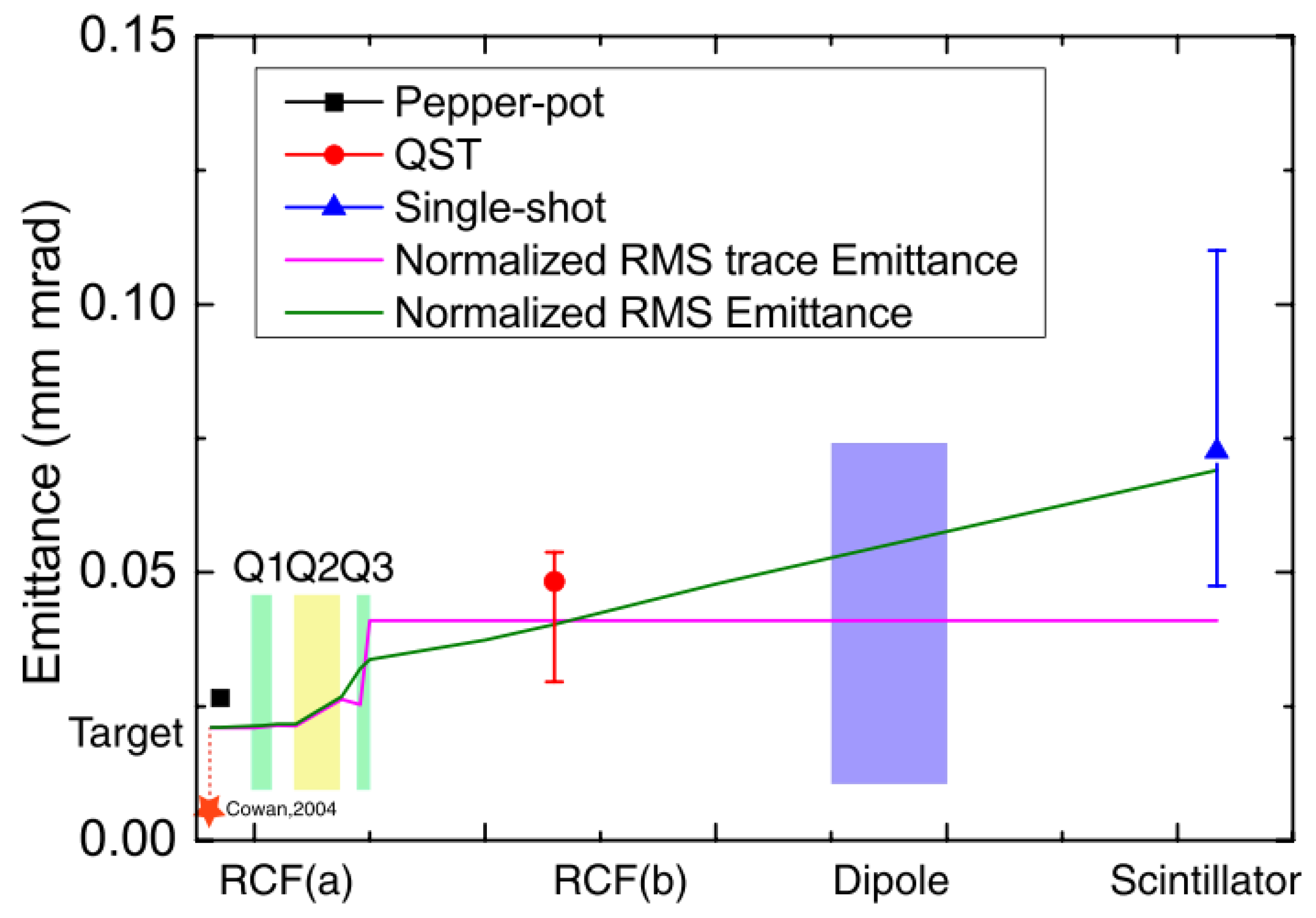
3. Emittance Measurement along Transport Beamline for Laser-Driven Protons
4. Production of the Spread-Out Bragg Peak (SOBP) by a Tailored Energy Deposition
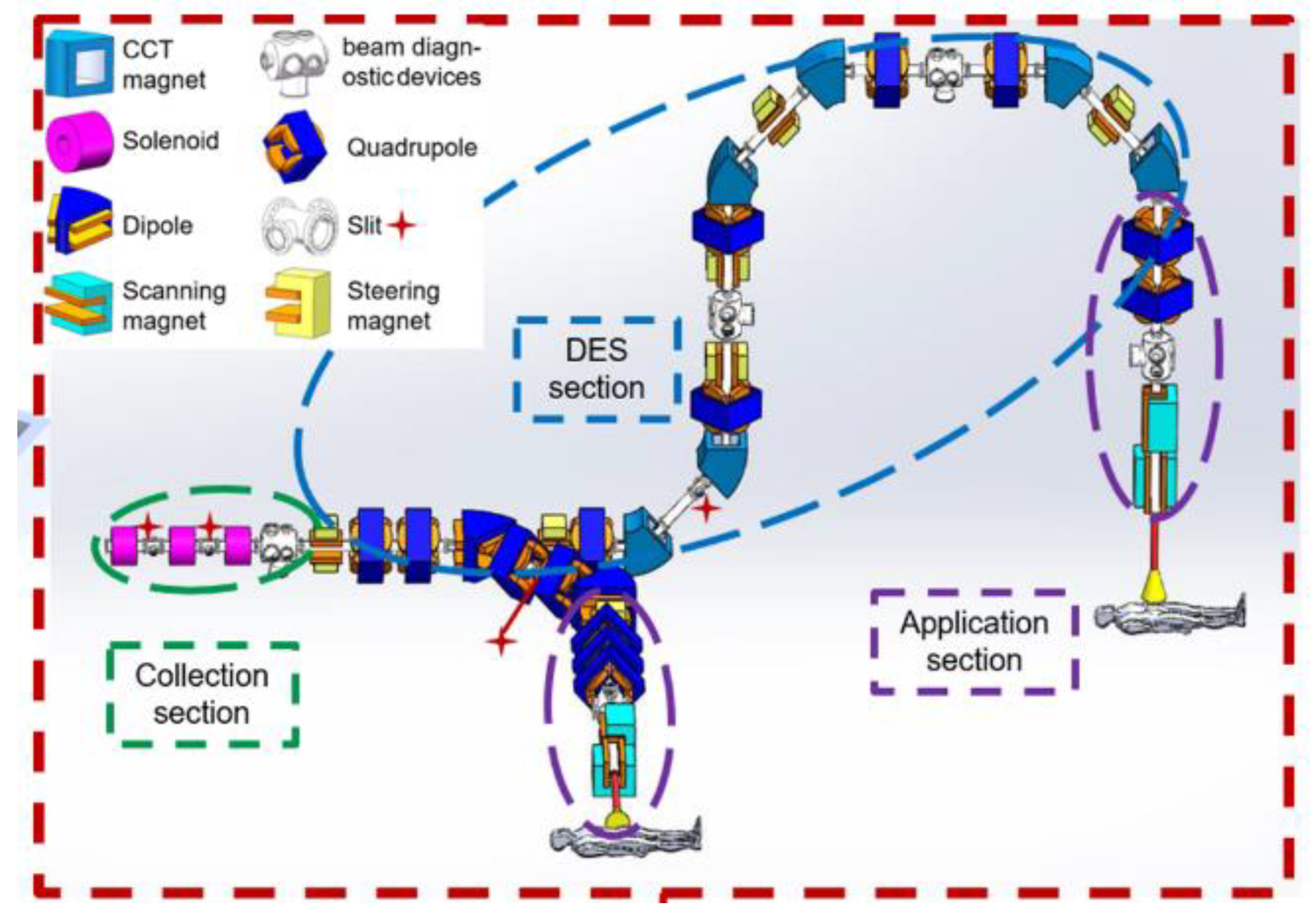
5. The Beamline Design for CLAPA Ⅱ
5.1. Achromatic Beamline Design for CLAPA Ⅱ
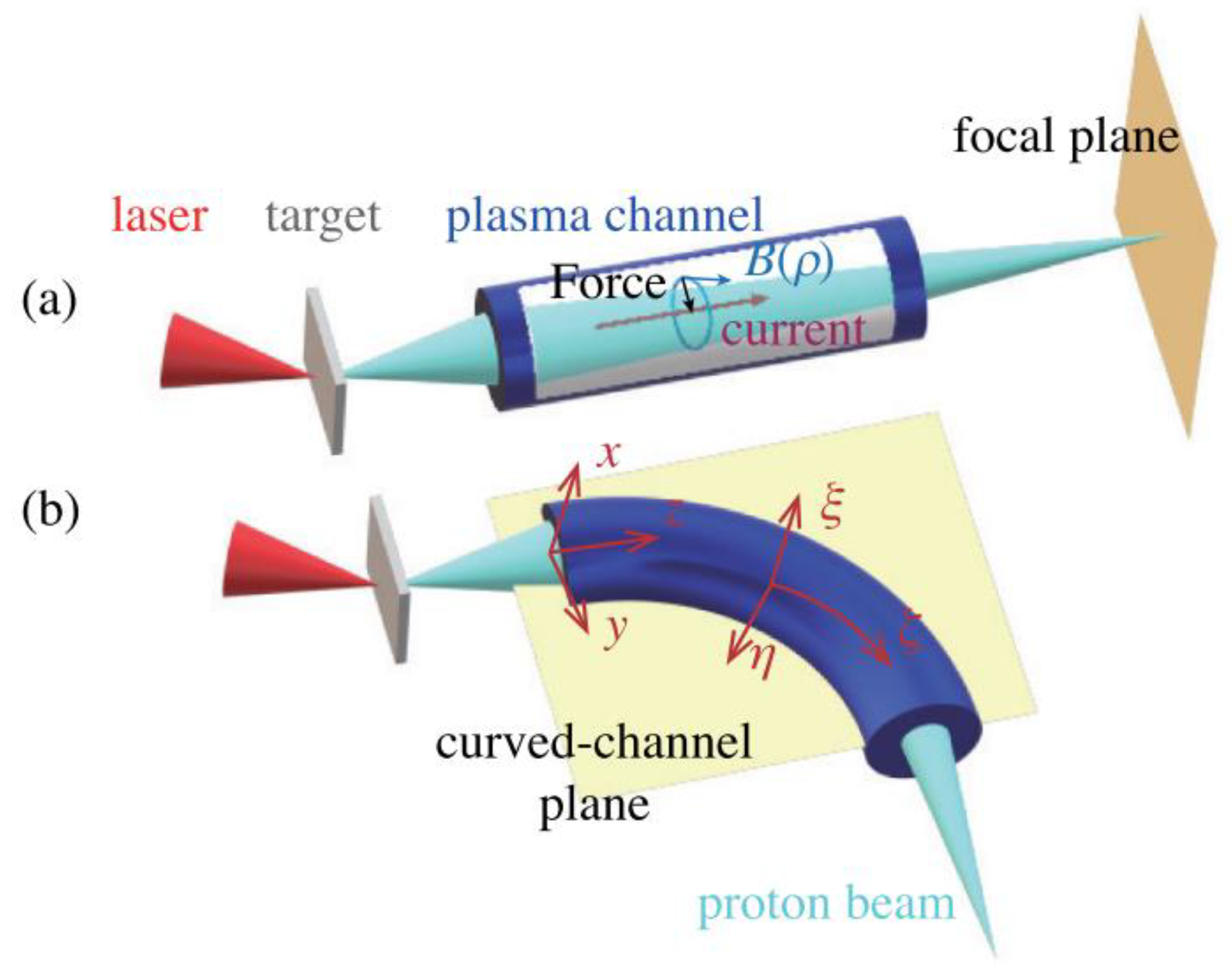
5.2. Designing of Active Plasma Lens for CLAPA Ⅱ
6. Application of Laser Proton Acceleration
6.1. Laser-Accelerated Proton Radiography of Biological Samples
6.2. Laser-Accelerated Ultra-High Dose Rate FLASH Radiation of Normal Cells and Tumor-Cells
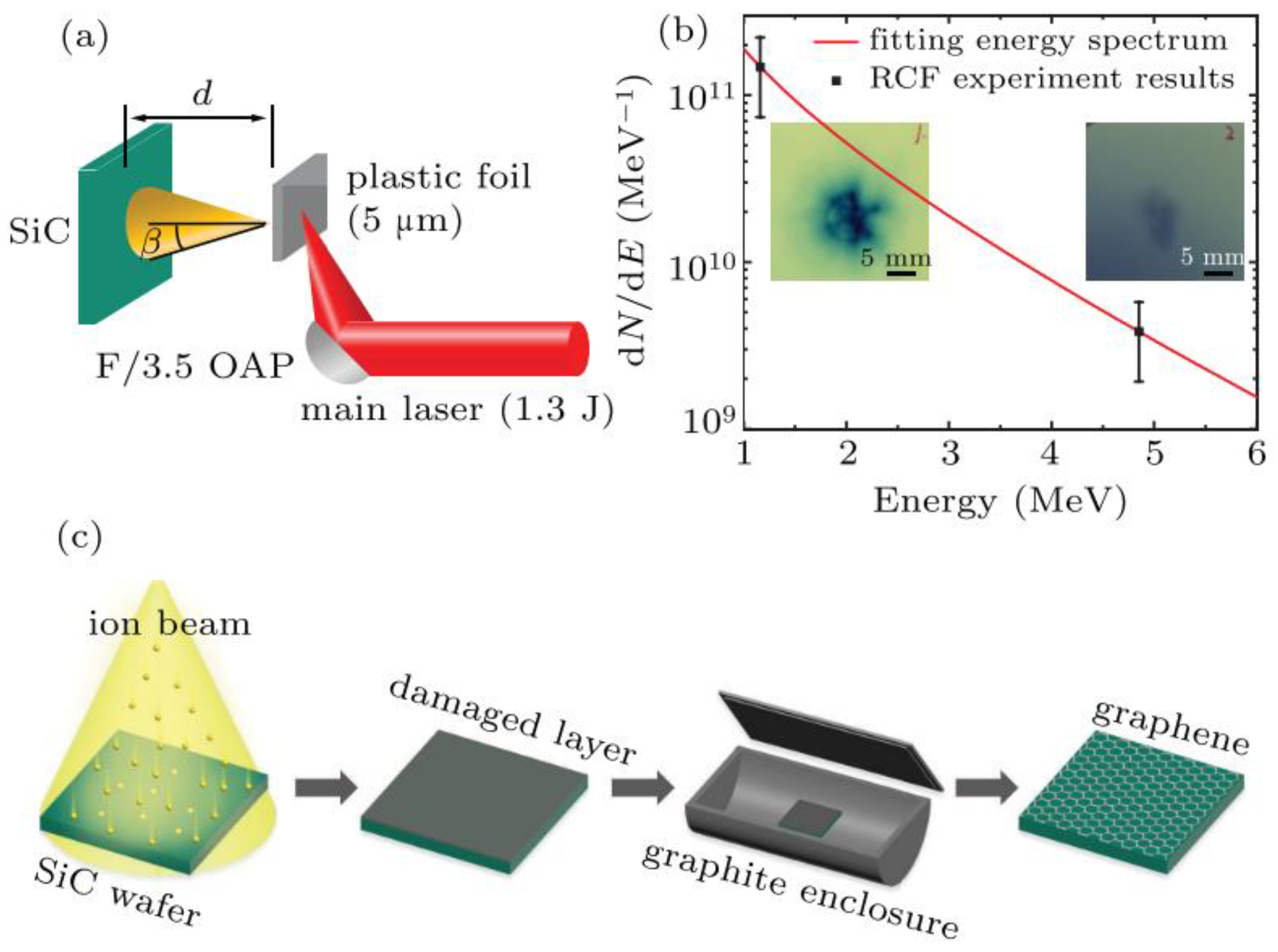
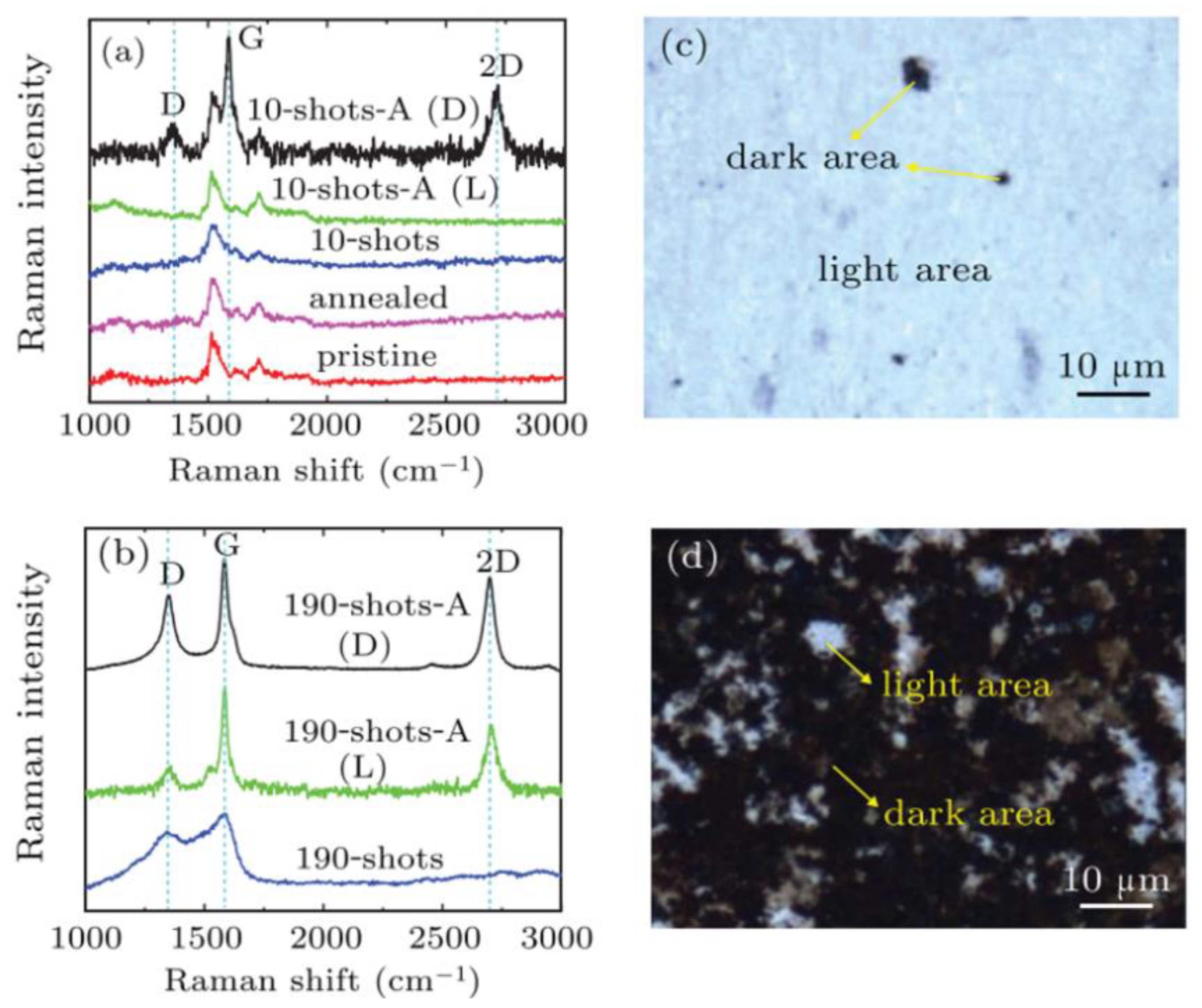
6.3. Preparation of Graphene on SiC by Laser-Accelerated Pulsed Ion Beams
6.4. Laser-Accelerated Ion-Beam Trace Probe (LITP) for Measuring the Electromagnetic Fields in a Magnetic Confinement Fusion Device
7. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tajima, T.; Dawson, J.M. Laser electron accelerator. Phys. Rev. Lett. 1979, 43, 267. [Google Scholar] [CrossRef]
- Danson, C.; Haefner, C.; Bromage, J.; Butcher, T.; Chanteloup, J.-C.; Chowdhury, E.; Galvanauskas, A.; Gizzi, L.; Hein, J.; Hillier, D.; et al. Petawatt and exawatt class lasers worldwide. High Power Laser Sci. Eng. 2019, 7, e54. [Google Scholar] [CrossRef]
- Mourou, G. Nobel lecture: Extreme light physics and application. Rev. Mod. Phys. 2019, 91, 030501. [Google Scholar] [CrossRef]
- Esarey, E.; Schroeder, C.B.; Leemans, W.P. Physics of laser-driven plasma-based electron accelerators. Rev. Mod. Phys. 2009, 81, 1229. [Google Scholar] [CrossRef]
- Macchi, A.; Borghesi, M.; Passoni, M. Ion acceleration by superintense laser-plasma interaction. Rev. Mod. Phys. 2013, 85, 751–793. [Google Scholar] [CrossRef]
- Gonsalves, A.J.; Nakamura, K.; Daniels, J.; Benedetti, C.; Pieronek, C.; de Raadt, T.C.H.; Steinke, S.; Bin, J.H.; Bulanov, S.S.; van Tilborg, J.; et al. Petawatt laser guiding and electron beam acceleration to 8 GeV in a laser-heated capillary discharge waveguide. Phys. Rev. Lett. 2019, 122, 084801. [Google Scholar] [CrossRef]
- Higginson, A.; Gray, R.J.; King, M.; Dance, R.J.; Williamson, S.D.; Butler, N.M.; Wilson, R.; Capdessus, R.; Armstrong, C.; Green, J.S.; et al. Near-100 MeV protons via a laser-driven transparency-enhanced hybrid acceleration scheme. Nat. Commun. 2018, 9, 724. [Google Scholar] [CrossRef] [PubMed]
- Daido, H.; Nishiuchi, M.; Pirozhkov, A.S. Review of laser-driven ion sources and their applications. Rep. Prog. Phys. 2012, 75, 056401. [Google Scholar] [CrossRef]
- Raffestin, D.; Lecherbourg, L.; Lantuéjoul, I.; Vauzour, B.; Masson-Laborde, P.E.; Davoine, X.; Blanchot, N.; Dubois, J.L.; Vaisseau, X.; d’Humières, E.; et al. Enhanced ion acceleration using the high-energy petawatt PETAL laser. Matter Radiat. Extrem. 2021, 6, 056901. [Google Scholar] [CrossRef]
- Okamura, M. Laser ion source for heavy ion inertial fusion. Matter Radiat. Extrem. 2018, 3, 61. [Google Scholar] [CrossRef]
- Martinez, B.; Chen, S.N.; Bolaños, S.; Blanchot, N.; Boutoux, G.; Cayzac, W.; Courtois, C.; Davoine, X.; Duval, A.; Horny, V.; et al. Numerical investigation of spallation neutrons generated from petawatt-scale laser-driven proton beams. Matter Radiat. Extrem. 2022, 7, 024401. [Google Scholar] [CrossRef]
- Romano, F.; Schillaci, F.; Cirrone, G.A.P.; Cuttone, G.; Scuderi, V.; Allegra, L.; Amato, A.; Amico, A.; Candiano, G.; De Luca, G.; et al. The ELIMED transport and dosimetry beamline for laser-driven ion beams. Nucl. Instrum. Methods Phys. Res. Sect. A 2016, 829, 153. [Google Scholar] [CrossRef]
- Masood, U.; Bussmann, M.; Cowan, T.E.; Enghardt, W.; Karsch, L.; Kroll, F. A compact solution for ion beam therapy with laser accelerated protons. Appl. Phys. B 2014, 117, 41. [Google Scholar] [CrossRef]
- Pommarel, L.; Vauzour, B.; Mégnin-Chanet, F.; Bayart, E.; Delmas, O.; Goudjil, F.; Nauraye, C.; Letellier, V.; Pouzoulet, F.; Schillaci, F.; et al. Spectral and spatial shaping of a laser-produced ion beam for radiation-biology experiments. Phys. Rev. Accel. Beams 2017, 20, 032801. [Google Scholar] [CrossRef]
- Mora, P. Plasma expansion into a vacuum. Phys. Rev. Lett. 2003, 90, 185002. [Google Scholar] [CrossRef] [PubMed]
- Pukhov, A. Three-dimensional simulations of ion acceleration from a foil irradiated by a short-pulse laser. Phys. Rev. Lett. 2001, 86, 3562. [Google Scholar] [CrossRef] [PubMed]
- Palmer, C.A.J.; Schreiber, J.; Nagel, S.R.; Dover, N.P.; Bellei, C.; Beg, F.N.; Bott, S.; Clarke, R.J.; Dangor, A.E.; Hassan, S.M.; et al. Rayleigh–Taylor instability of an ultrathin foil accelerated by the radiation pressure of an intense laser. Phys. Rev. Lett. 2012, 108, 225002. [Google Scholar] [CrossRef]
- Wan, Y.; Andriyash, I.A.; Lu, W.; Mori, W.B.; Malka, V. Effects of the transverse instability and wave breaking on the laser-driven thin foil acceleration. Phys. Rev. Lett. 2020, 125, 104801. [Google Scholar] [CrossRef]
- Zhu, J.G.; Wu, M.J.; Liao, Q.; Geng, Y.X.; Zhu, K.; Li, C.C.; Xu, X.H.; Li, D.Y.; Shou, Y.R.; Yang, T.; et al. Experimental demonstration of a laser proton accelerator with accurate beam control through image-relaying transport. Phys. Rev. Accel. Beams 2019, 22, 061302. [Google Scholar] [CrossRef]
- Geng, Y.X.; Liao, Q.; Shou, Y.R.; Zhu, J.G.; Xu, X.H.; Wu, M.J.; Wang, P.J.; Li, D.Y.; Yang, T.; Hu, R.H.; et al. Generating proton beams exceeding 10 MeV using high contrast 60 TW laser. Chin. Phys. Lett. 2018, 35, 092901. [Google Scholar] [CrossRef]
- Geng, Y.X.; Wu, D.; Yu, W.; Sheng, Z.M.; Fritzsche, S.; Liao, Q.; Wu, M.J.; Xu, X.H.; Li, D.Y.; Ma, W.J.; et al. Proton beams from intense laser-solid interaction: Effects of the target materials. Matter Radiat. Extrem. 2020, 5, 064402. [Google Scholar] [CrossRef]
- Zhu, J.G.; Zhu, K.; Tao, L.; Geng, Y.X.; Lin, C.; Ma, W.J.; Lu, H.Y.; Zhao, Y.Y.; Lu, Y.R.; Chen, J.E.; et al. Beam line design of compact laser plasma accelerator. Chin. Phys. Lett. 2017, 34, 054101. [Google Scholar] [CrossRef]
- Zhu, J.G.; Zhu, K.; Tao, L.; Xu, X.H.; Lin, C.; Ma, W.J.; Lu, H.Y.; Zhao, Y.Y.; Lu, Y.R.; Chen, J.E.; et al. Distribution uniformity of laser-accelerated proton beams. Chin. Phys. C 2017, 41, 097001. [Google Scholar] [CrossRef]
- Wilks, S.C.; Langdon, A.B.; Cowan, T.E.; Roth, M.; Singh, M.; Hatchett, S.; Key, M.H.; Pennington, D.; MacKinnon, A.; Snavely, R.A. Energetic proton generation in ultra-intense laser–solid interactions. Phys. Plasmas 2001, 8, 542–549. [Google Scholar] [CrossRef]
- Xu, X.H.; Liao, Q.; Wu, M.J.; Geng, Y.X.; Li, D.Y.; Zhu, J.G.; Li, C.C.; Hu, R.H.; Shou, Y.R.; Chen, Y.H.; et al. Detection and analysis of laser driven proton beams by calibrated Gafchromic HD-V2 and MD-V3 radiochromic films. Rev. Sci. Instrum. 2019, 90, 033306. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.; Zhu, J.; Li, D.; Yang, T.; Liao, Q.; Geng, Y.; Xu, X.; Li, C.; Shou, Y.; Zhao, Y.; et al. Collection and focusing of laser accelerated proton beam by an electromagnetic quadrupole triplet lens. Nucl. Instrum. Methods Phys. Res. Sect. A 2020, 955, 163249. [Google Scholar] [CrossRef]
- Yan, X.Q.; Lin, C.; Sheng, Z.M.; Guo, Z.Y.; Liu, B.C.; Lu, Y.R.; Fang, J.X.; Chen, J.E. Generating high-current monoenergetic proton beams by a circularly polarized laser pulse in the phase-stable acceleration regime. Phys. Rev. Lett. 2008, 100, 135003. [Google Scholar] [CrossRef]
- Esirkepov, T.; Borghesi, M.; Bulanov, S.V.; Mourou, G.; Tajima, T. Highly efficient relativistic-ion generation in the laser-piston regime. Phys. Rev. Lett. 2004, 92, 175003. [Google Scholar] [CrossRef]
- Kar, S.; Ahmed, H.; Prasad, R.; Cerchez, M.; Brauckmann, S.; Aurand, B.; Cantono, G.; Hadjisolomou, P.; Lewis, C.L.S.; Macchi, A.; et al. Guided post-acceleration of laser-driven ions by a miniature modular structure. Nat. Commun. 2016, 7, 10792. [Google Scholar] [CrossRef]
- Bardon, M.; Moreau, J.G.; Romagnani, L.; Rousseaux, C.; Ferri, M.; Lefévre, F.; Lantuéjoul, I.; Etchessahar, B.; Bazzoli, S.; Farcage, D.; et al. Physics of chromatic focusing, post-acceleration and bunching of laser-driven proton beams in helical coil targets. Plasma Phys. Control. Fusion 2020, 62, 125019. [Google Scholar] [CrossRef]
- Floettmann, K. Some basic features of the beam emittance. Phys. Rev. ST Accel. Beams 2003, 6, 034202. [Google Scholar] [CrossRef]
- Cowan, T.E.; Fuchs, J.; Ruhl, H.; Kemp, A.; Audebert, P.; Roth, M.; Stephens, R.; Barton, I.; Blazevic, A.; Brambrink, E.; et al. Ultralow emittance, multi-MeV proton beams from a laser virtual-cathode plasma accelerator. Phys. Rev. Lett. 2004, 92, 204801. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.J.; Li, D.Y.; Zhu, J.G.; Yang, T.; Hu, X.Y.; Geng, Y.X.; Zhu, K.; Easton, M.J.; Zhao, Y.Y.; Zhang, A.L.; et al. Emittance measurement along transport beam line for laser driven protons. Phys. Rev. Accel. Beams 2020, 23, 031302. [Google Scholar] [CrossRef]
- Zhu, J.G.; Wu, M.J.; Zhu, K.; Geng, Y.X.; Liao, Q.; Li, D.Y.; Yang, T.; Eatson, M.J.; Li, C.C.; Xu, X.H.; et al. Demonstration of tailored energy deposition in a laser proton accelerator. Phys. Rev. Accel. Beams 2020, 23, 121304. [Google Scholar] [CrossRef]
- Tajima, T.; Habs, D.; Yan, X. Laser Acceleration of Ions for Radiation Therapy; World Scientific: Singapore, 2009; pp. 201–228. [Google Scholar]
- Yan, X.Q.; Tajima, T.; Hegelich, M.; Yin, L.; Habs, D. Theory of laser ion acceleration from a foil target of nanometer thickness. Appl. Phys. B 2009, 98, 711–721. [Google Scholar] [CrossRef]
- Wang, H.Y.; Yan, X.Q.; Chen, J.E.; He, X.T.; Ma, W.J.; Bin, J.H.; Schreiber, J.; Tajima, T.; Habs, D. Efficient and stable acceleration by irradiating a two-layer target with a linearly polarized laser pulse. Phys. Plasmas 2013, 20, 013101. [Google Scholar] [CrossRef]
- Esirkepov, T.; Bulanov, S.V.; Nishihara, K.; Tajima, T.; Pegoraro, F.; Khoroshkov, V.S.; Mima, K.; Daido, H.; Kato, Y.; Kitagawa, Y.; et al. Proposed double-layer target for the generation of high-quality laser-accelerated ion beams. Phys. Rev. Lett. 2002, 89, 175003. [Google Scholar] [CrossRef]
- Necas, A.; Tajima, T.; Mourou, G.; Osvay, K. Laser ion acceleration in a near critical density trap. Photonics 2022, 9, 453. [Google Scholar] [CrossRef]
- Ma, W.J.; Kim, I.J.; Yu, J.Q.; Choi, I.W.; Singh, P.K.; Lee, H.W.; Sung, J.H.; Lee, S.K.; Lin, C.; Liao, Q.; et al. Laser Acceleration of highly energetic carbon ions using a double-layer target composed of slightly underdense plasma and ultrathin foil. Phys. Rev. Lett. 2019, 122, 014803. [Google Scholar] [CrossRef] [PubMed]
- Esirkepov, T.; Yamagiwa, M.; Tajima, T. Laser ion-acceleration scaling laws seen in multiparametric particle-in-cell simulations. Phys. Rev. Lett. 2006, 96, 105001. [Google Scholar] [CrossRef]
- Henig, A.; Steinke, S.; Schnürer, M.; Sokollik, T.; Hörlein, R.; Kiefer, D.; Jung, D.; Schreiber, J.; Hegelich, B.M.; Yan, X.Q.; et al. Radiation-pressure acceleration of ion beams driven by circularly polarized laser pulses. Phys. Rev. Lett. 2009, 103, 245003. [Google Scholar] [CrossRef]
- Kar, S.; Kakolee, K.F.; Qiao, B.; Macchi, A.; Cerchez, M.; Doria, D.; Geissler, M.; McKenna, P.; Neely, D.; Osterholz, J.; et al. Ion acceleration in multispecies targets driven by intense laser radiation pressure. Phys. Rev. Lett. 2012, 109, 185006. [Google Scholar] [CrossRef]
- Kim, I.J.; Pae, K.H.; Choi, I.W.; Lee, C.L.; Kim, H.T.; Singhal, H.; Sung, J.H.; Lee, S.K.; Lee, H.W.; Nickles, P.V.; et al. Radiation pressure acceleration of protons to 93 MeV with circularly polarized petawatt laser pulses. Phys. Plasmas 2016, 23, 070701. [Google Scholar] [CrossRef]
- Fuchs, J.; Antici, P.; d’Humières, E.; Lefebvre, E.; Borghesi, M.; Brambrink, E.; Cecchetti, C.A.; Kaluza, M.; Malka, V.; Manclossi, M.; et al. Laser-driven proton scaling laws and new paths towards energy increase. Nat. Phys. 2008, 2, 28. [Google Scholar] [CrossRef]
- Wang, K.D.; Zhu, K.; Easton, M.J.; Li, Y.J.; Lin, C.; Yan, X.Q. Achromatic beamline design for a laser-driven proton therapy accelerator. Phys. Rev. Accel. Beams 2020, 23, 111302. [Google Scholar] [CrossRef]
- Brouwer, L.; Caspi, S.; Edwards, K.; Godeke, A.; Prestemon, S. Design and test of a curved superconducting dipole magnet for proton therapy. Nucl. Instrum. Methods Phys. Res. Sect. A 2020, 957, 163414. [Google Scholar] [CrossRef]
- Leemans, W.P.; Nagler, B.; Gonsalves, A.J.; Toth, C.; Nakamura, K.; Geddes, C.G.R.; Esarey, E.; Schroeder, C.B.; Hooker, S.M. GeV electron beams from a centimetre-scale accelerator. Nat. Phys. 2006, 2, 696–699. [Google Scholar] [CrossRef]
- Steinke, S.; van Tilborg, J.; Benedetti, C.; Geddes, C.G.R.; Schroeder, C.B.; Daniels, J.; Swanson, K.K.; Gonsalves, A.J.; Nakamura, K.; Matlis, N.H.; et al. Multistage coupling of independent laser-plasma accelerators. Nature 2016, 530, 190. [Google Scholar] [CrossRef]
- Van Tilborg, J.; Steinke, S.; Geddes, C.G.R.; Matlis, N.H.; Shaw, B.H.; Gonsalves, A.J.; Huijts, J.V.; Nakamura, K.; Daniels, J.; Schroeder, C.B.; et al. Active plasma lensing for relativistic laser-plasma-accelerated electron beams. Phys. Rev. Lett. 2015, 115, 184802. [Google Scholar] [CrossRef]
- Pompili, R.; Anania, M.P.; Bellaveglia, M.; Biagioni, A.; Bini, S.; Bisesto, F.; Brentegani, E.; Castorina, G.; Chiadroni, E.; Cianchi, A.; et al. Experimental characterization of active plasma lensing for electron beams. Appl. Phys. Lett. 2017, 110, 104101. [Google Scholar] [CrossRef]
- Lindstrøm, C.A.; Adli, E.; Boyle, G.; Corsini, R.; Dyson, A.E.; Farabolini, W.; Hooker, S.M.; Meisel, M.; Osterhoff, J.; Röckemann, J.-H.; et al. Emittance preservation in an aberration-free active plasma lens. Phys. Rev. Lett. 2018, 121, 194801. [Google Scholar] [CrossRef] [PubMed]
- Yang, T.; Cheng, H.; Yan, Y.; Wu, M.; Li, D.; Li, Y.; Xia, Y.; Lin, C.; Yan, X. Designing of active plasma lens for focusing laser-plasma-accelerated pulsed proton beams. Phys. Rev. Accel. Beams 2021, 24, 031301. [Google Scholar] [CrossRef]
- Hershcovitch, A. High-pressure arcs as vacuum-atmosphere interface and plasma lens for nonvacuum electron beam welding machines, electron beam melting, and nonvacuum ion material modification. J. Appl. Phys. 1995, 78, 5283. [Google Scholar] [CrossRef]
- Lu, L.; Ma, W.; Li, C.; He, T.; Yang, L.; Sun, L.; Xu, X.; Wang, W.; Shi, L. New developments of HIF injector. Matter Radiat. Extrem. 2018, 3, 50. [Google Scholar] [CrossRef]
- Hofmann, I. Review of accelerator driven heavy ion nuclear fusion. Matter Radiat. Extrem. 2018, 3, 1. [Google Scholar] [CrossRef]
- Yao, W.; Fazzini, A.; Chen, S.N.; Burdonov, K.; Antici, P.; Béard, J.; Bolaños, S.; Ciardi, A.; Diab, R.; Filippov, E.D.; et al. Detailed characterization of a laboratory magnetized supercritical collisionless shock and of the associated proton energization. Matter Radiat. Extrem. 2022, 7, 014402. [Google Scholar] [CrossRef]
- Kroll, F.; Brack, F.; Bernert, C.; Bock, S.; Bodenstein, E.; Brüchner, K.; Cowan, T.E.; Gaus, L.; Gebhardt, R.; Helbig, U.; et al. Tumour irradiation in mice with a laser-accelerated proton beam. Nat. Phys. 2022, 18, 316. [Google Scholar] [CrossRef]
- Scott, W.T. The theory of small-angle multiple scattering of fast charged particles. Rev. Mod. Phys. 1963, 35, 231. [Google Scholar] [CrossRef]
- Faenov, A.Y.; Pikuz, T.A.; Fukuda, Y.; Kando, M.; Kotaki, H.; Homma, T.; Kawase, K.; Kameshima, T.; Pirozhkov, A.; Yogo, A.; et al. Submicron iconography of nanostructures using a femtosecond-laser-driven-cluster-based source. Appl. Phys. Lett. 2009, 95, 101107. [Google Scholar] [CrossRef]
- Son, J.; Lee, C.H.; Kang, J.; Jang, D.Y.; Park, J.; Kim, Y.H.; Kim, Y.K.; Choi, C.I.; Kim, I.J.; Choi, I.W.; et al. Fine phantom image from laser-induced proton radiography with a spatial resolution of several μm. J. Korean Phys. Soc. 2014, 65, 6. [Google Scholar] [CrossRef]
- Wang, W.; Shen, B.; Zhang, H.; Lu, X.; Wang, C.; Liu, Y.; Yu, L.; Chu, Y.; Li, Y.; Xu, T.; et al. Large-scale proton radiography with micrometer spatial resolution using femtosecond petawatt laser system. AIP Adv. 2015, 5, 107214. [Google Scholar]
- Li, D.Y.; Xu, X.H.; Yang, T.; Wu, M.J.; Zhang, Y.F.; Cheng, H.; Hu, X.Y.; Geng, Y.X.; Zhu, J.G.; Zhao, Y.Y.; et al. Influence factors of resolution in laser accelerated proton radiography and image deblurring. AIP Adv. 2021, 11, 085316. [Google Scholar] [CrossRef]
- Bourhis, J.; Sozzi, W.J.; Jorge, P.G.; Gaide, O.; Bailat, C.; Duclos, F.; Patin, D.; Ozsahin, M.; Bochud, F.; Germond, J.; et al. Treatment of a first patient with FLASH-radiotherapy. Radiother. Oncol. 2019, 139, 18–22. [Google Scholar] [CrossRef] [PubMed]
- Bin, J.; Obst-Huebl, L.; Mao, J.; Nakamura, K.; Geulig, L.D.; Chang, H.; Ji, Q.; He, L.; Chant, J.D.; Kober, Z.; et al. A new platform for ultra-high dose rate radiobiological research using the BELLA PW laser proton beamline. Sci. Rep. 2022, 12, 1484. [Google Scholar] [CrossRef] [PubMed]
- Bayart, E.; Flacco, A.; Delmas, O.; Pommarel, L.; Levy, D.; Cavallone, M.; Megnin-Chanet, F.; Deutsch, E.; Malka, V. Fast dose fractionation using ultra-short laser accelerated proton pulses can increase cancer cell mortality, which relies on functional PARP1 protein. Sci. Rep. 2019, 9, 10132. [Google Scholar] [CrossRef]
- Yang, G.; Lu, C.; Mei, Z.; Sun, X.; Han, J.; Qian, J.; Liang, Y.; Pan, Z.; Kong, D.; Xu, S.; et al. Association of cancer stem cell radio-resistance under ultra-high dose rate FLASH irradiation with lysosome-mediated autophagy. Front. Cell Dev. Biol. 2021, 9, 1032. [Google Scholar] [CrossRef]
- Han, J.; Mei, Z.; Lu, C.; Qian, J.; Liang, Y.; Sun, X.; Pan, Z.; Kong, D.; Xu, S.; Liu, Z.; et al. Ultra-high dose rate FLASH irradiation induced radio-resistance of normal fibroblast cells can be enhanced by hypoxia and mitochondrial dysfunction resulting from loss of cytochrome C. Front. Cell Dev. Biol. 2021, 9, 1089. [Google Scholar] [CrossRef]
- Patel, P.K.; Mackinnon, A.J.; Key, M.H.; Cowan, T.E.; Foord, M.E.; Allen, M.; Price, D.F.; Ruhl, H.; Springer, P.T.; Stephens, R. Isochoric heating of solid-density matter with an ultrafast proton beam. Phys. Rev. Lett. 2003, 91, 125004. [Google Scholar] [CrossRef]
- Dyer, G.M.; Bernstein, A.C.; Cho, B.I.; Osterholz, J.; Grigsby, W.; Dalton, A.; Shepherd, R.; Ping, Y.; Chen, H.; Widmann, K.; et al. Equation-of-state measurement of dense plasmas heated with fast protons. Phys. Rev. Lett. 2008, 101, 015002. [Google Scholar] [CrossRef]
- Barberio, M.; Scisciò, M.; Vallières, S.; Cardelli, F.; Chen, S.N.; Famulari, G.; Gangolf, T.; Revet, G.; Schiavi, A.; Senzacqua, M.; et al. Laser-accelerated particle beams for stress testing of materials. Nat. Commun. 2018, 9, 372. [Google Scholar] [CrossRef]
- Alvarez, J.; Mima, K.; Tanaka, K.A.; Fernandez, J.; Garoz, D.; Habara, H.; Kikuyama, K.; Kondo, K.; Perlado, J.M. Ultraintense lasers as a promising research tool for fusion material testing: Production of ions, X-rays and neutrons. Plasma Fusion Res. 2013, 8, 3404055. [Google Scholar] [CrossRef]
- Barberio, M.; Vallières, S.; Scisciò, M.; Kolhatkar, G.; Ruediger, A.; Antici, P. Graphitization of diamond by laser-accelerated proton beams. Carbon 2018, 139, 531. [Google Scholar] [CrossRef]
- Barberio, M.; Scisciò, M.; Vallières, S.; Veltri, S.; Morabito, A.; Antici, P. Laser-generated proton beams for high-precision ultra-fast crystal synthesis. Sci. Rep. 2017, 7, 12522. [Google Scholar] [CrossRef]
- Barberio, M.; Giusepponi, S.; Vallières, S.; Scisció, M.; Celino, M.; Antici, P. Ultra-fast high-precision metallic nanoparticle synthesis using laser-accelerated protons. Sci. Rep. 2020, 10, 9570. [Google Scholar] [CrossRef]
- Zhou, D.; Li, D.; Chen, Y.; Wu, M.; Yang, T.; Cheng, H.; Li, Y.; Chen, Y.; Li, Y.; Geng, Y.; et al. Preparation of graphene on SiC by laser-accelerated pulsed ion beams. Chin. Phys. B 2021, 30, 116106. [Google Scholar] [CrossRef]
- Yang, X.Y.; Chen, Y.H.; Lin, C.; Wang, L.; Xu, M.; Wang, X.G.; Xiao, C.J. A new method of measuring the poloidal magnetic and radial electric fields in a tokamak using a laser-accelerated ion-beam trace probe. Rev. Sci. Instrum. 2014, 85, 11E429. [Google Scholar] [CrossRef] [PubMed]
- Yang, X.; Xiao, C.; Chen, Y.; Xu, T.; Lin, C.; Wang, L.; Xu, M.; Yu, Y. 2D profile of poloidal magnetic field diagnosed by a laser-driven ion-beam trace probe (LITP). Rev. Sci. Instrum. 2016, 87, 11D608. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.H.; Yang, X.Y.; Lin, C.; Wang, L.; Xu, M.; Wang, X.G.; Xiao, C.J. 2D electron density profile measurement in tokamak by laser-accelerated ion-beam probe. Rev. Sci. Instrum. 2014, 85, 11D860. [Google Scholar] [CrossRef]
- Yang, X.; Xiao, C.; Chen, Y.; Xu, T.; Yu, Y.; Xu, M.; Wang, L.; Wang, X.; Lin, C. Theoretical study on the laser-driven ion-beam trace probe in toroidal devices with large poloidal magnetic field. JINST 2018, 13, C03034. [Google Scholar] [CrossRef]
- Wu, M.J.; Yang, X.Y.; Xu, T.C.; Li, D.Y.; Chen, Y.H.; Zhu, J.G.; Yang, T.; Hu, X.Y.; Ma, W.J.; Zhao, Y.Y.; et al. Calibration and test of CsI scintillator ion detection system for tokamak magnetic field diagnosis based on laser-driven ion-beam trace probe (LITP). Nucl. Fusion 2022, 62, 106028. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Yang, T.; Wu, M.; Mei, Z.; Wang, K.; Lu, C.; Zhao, Y.; Ma, W.; Zhu, K.; Geng, Y.; et al. Introduction of Research Work on Laser Proton Acceleration and Its Application Carried out on Compact Laser–Plasma Accelerator at Peking University. Photonics 2023, 10, 132. https://doi.org/10.3390/photonics10020132
Li D, Yang T, Wu M, Mei Z, Wang K, Lu C, Zhao Y, Ma W, Zhu K, Geng Y, et al. Introduction of Research Work on Laser Proton Acceleration and Its Application Carried out on Compact Laser–Plasma Accelerator at Peking University. Photonics. 2023; 10(2):132. https://doi.org/10.3390/photonics10020132
Chicago/Turabian StyleLi, Dongyu, Tang Yang, Minjian Wu, Zhusong Mei, Kedong Wang, Chunyang Lu, Yanying Zhao, Wenjun Ma, Kun Zhu, Yixing Geng, and et al. 2023. "Introduction of Research Work on Laser Proton Acceleration and Its Application Carried out on Compact Laser–Plasma Accelerator at Peking University" Photonics 10, no. 2: 132. https://doi.org/10.3390/photonics10020132
APA StyleLi, D., Yang, T., Wu, M., Mei, Z., Wang, K., Lu, C., Zhao, Y., Ma, W., Zhu, K., Geng, Y., Yang, G., Xiao, C., Chen, J., Lin, C., Tajima, T., & Yan, X. (2023). Introduction of Research Work on Laser Proton Acceleration and Its Application Carried out on Compact Laser–Plasma Accelerator at Peking University. Photonics, 10(2), 132. https://doi.org/10.3390/photonics10020132






