Polarization-Dependent Absorption and Transmission Metasurfaces for Linearly and Circularly Polarized Light in Terahertz Band
Abstract
1. Introduction
2. Results and Discussion
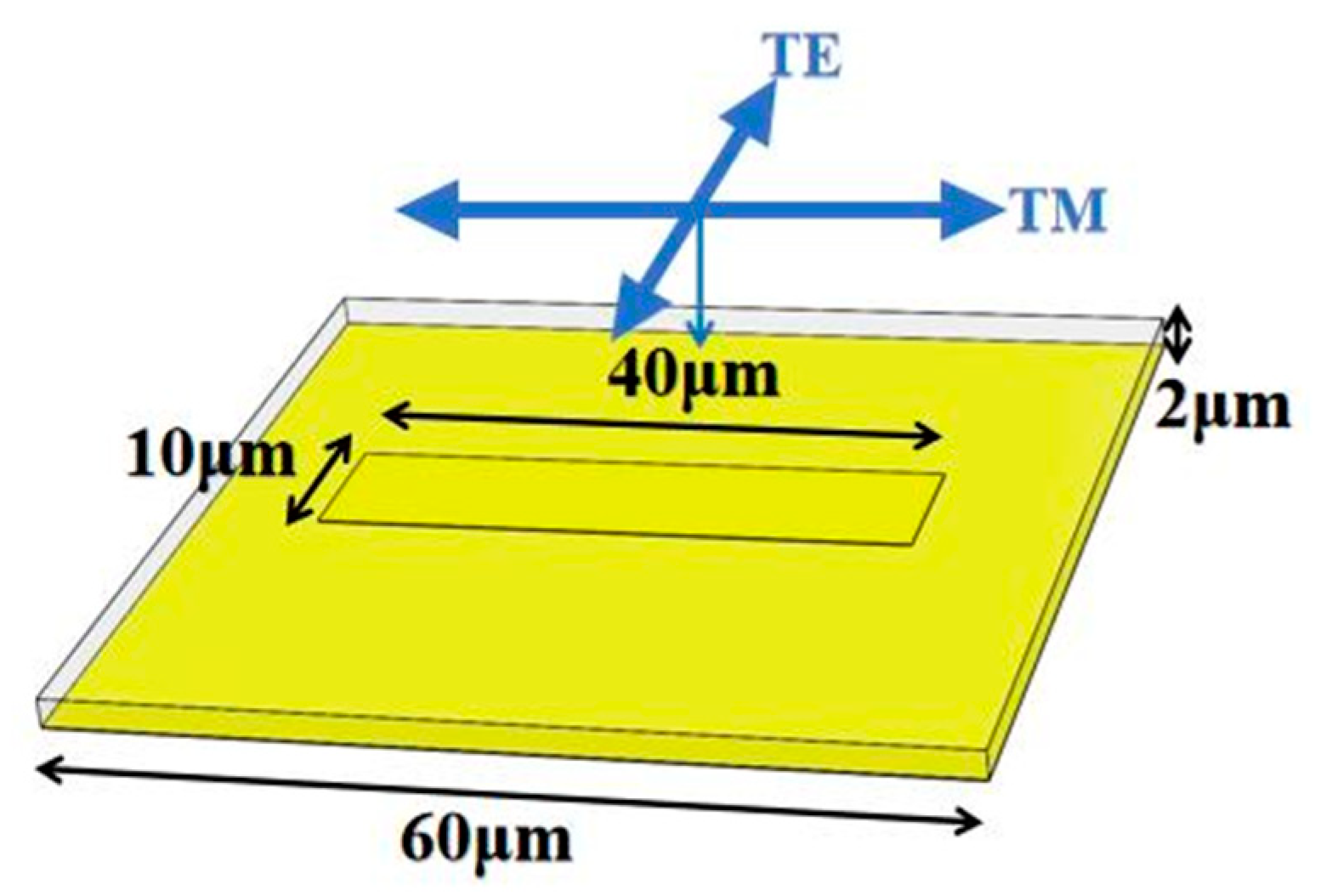
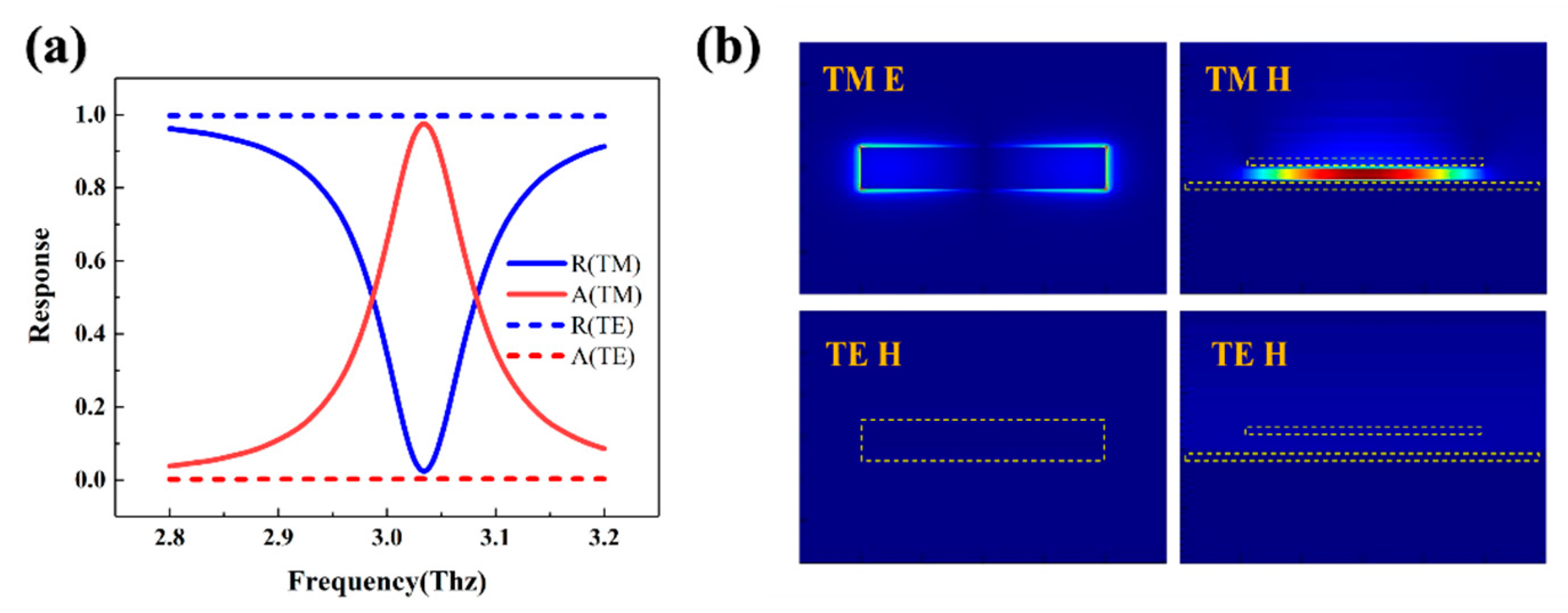
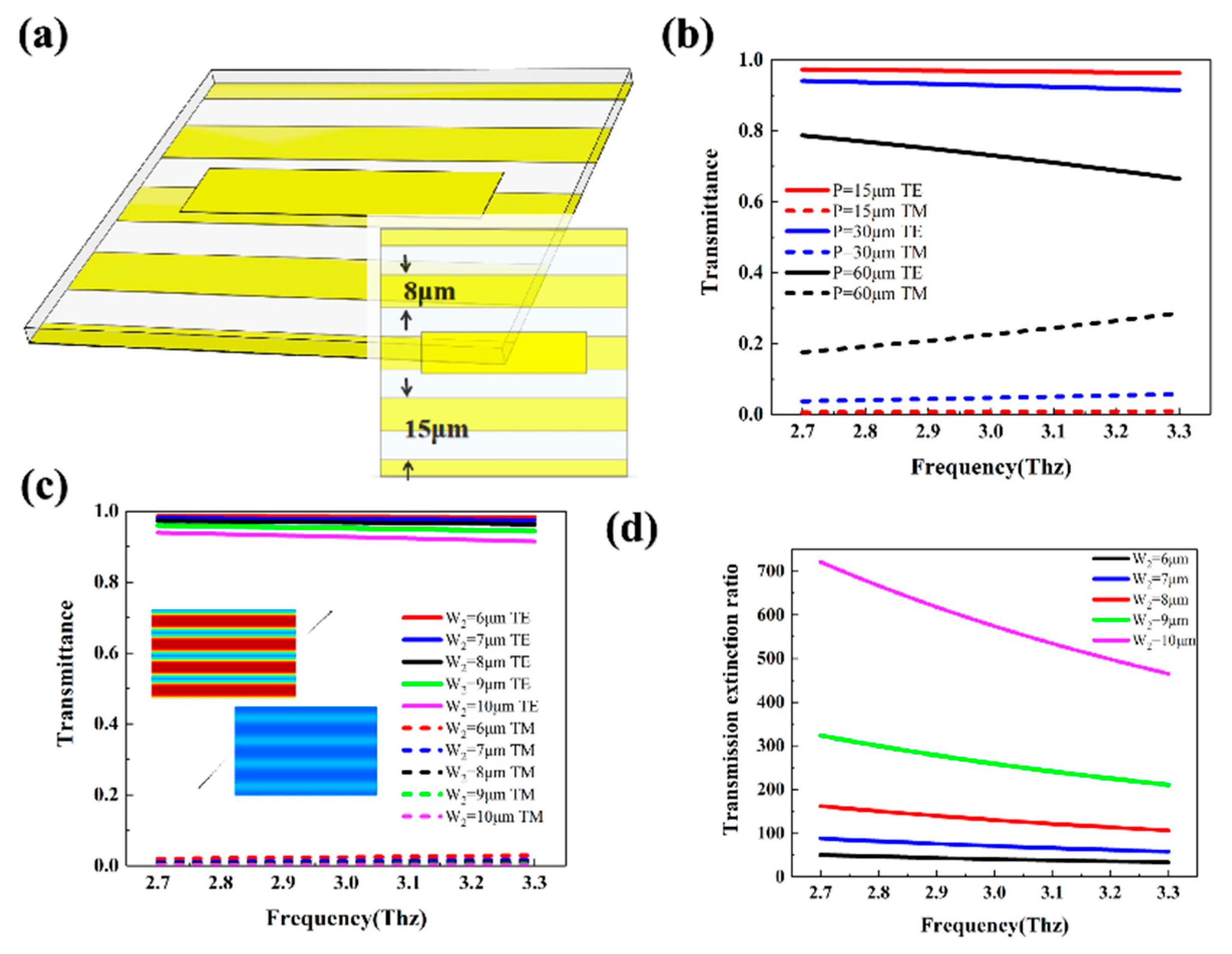
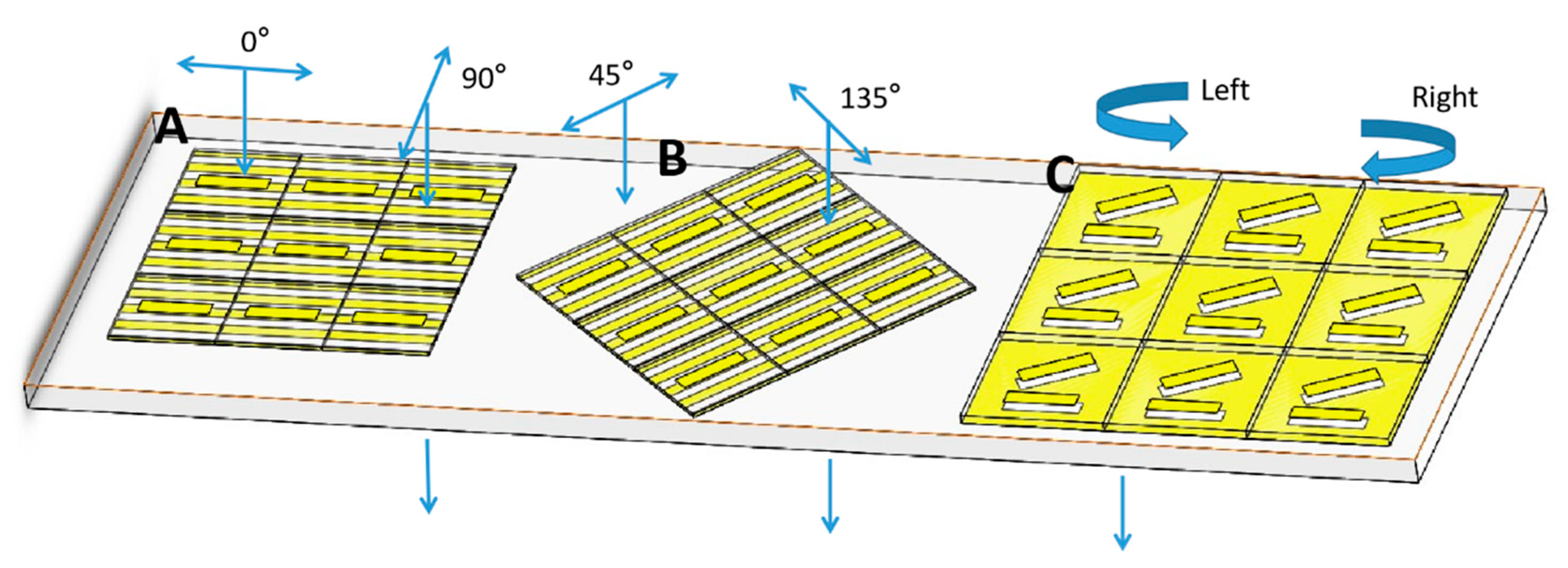
2.1. Polarization Dependent Transmission and Absorption Structure for Linearly Polarized Light
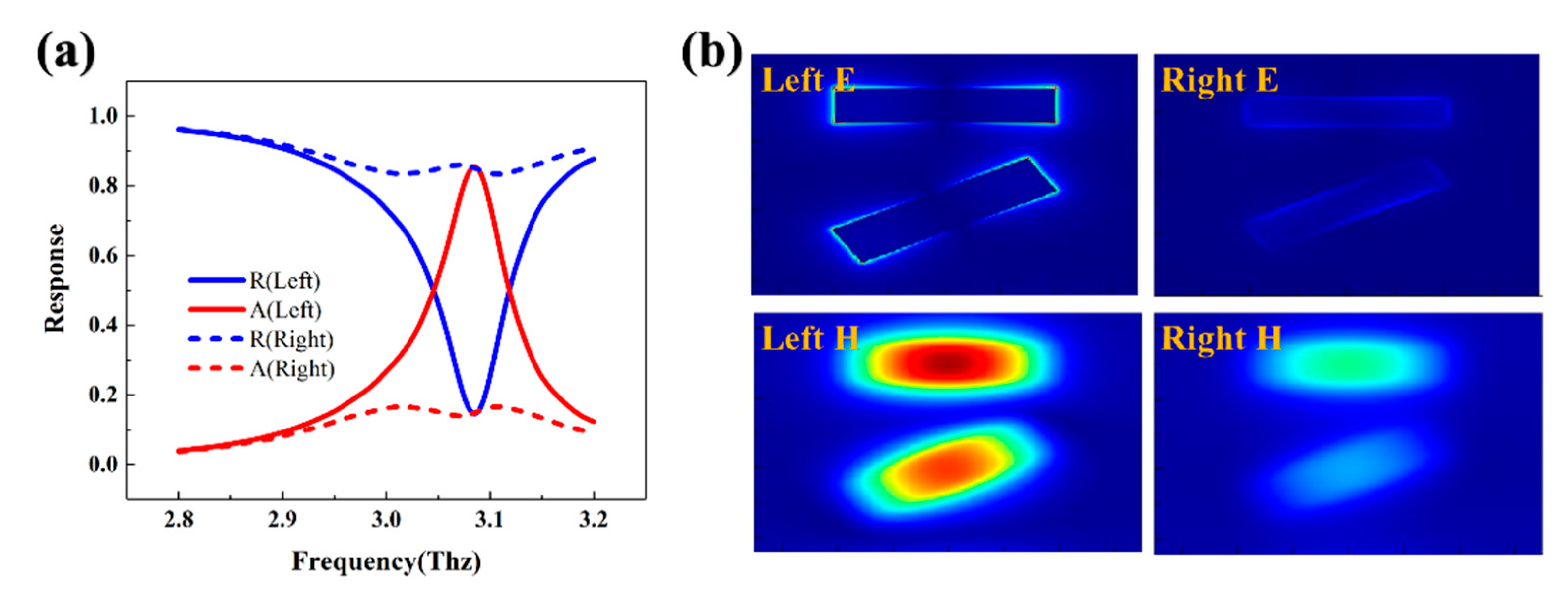
2.2. Polarization-Dependent Transmission and Absorption Structure for Circularly Polarized Light
3. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhong, S. Progress in terahertz nondestructive testing: A review. Front. Mech. Eng. 2019, 14, 273–281. [Google Scholar] [CrossRef]
- Amenabar, I.; Lopez, F.; Mendikute, A. In introductory review to THz non-destructive testing of composite mater. J. Infrared Millim. Terahertz Waves 2013, 34, 152–169. [Google Scholar] [CrossRef]
- Goyal, R. Vishwakarma D K. Design of a graphene-based patch antenna on glass substrate for high-speed terahertz communications. Microw. Opt. Technol. Lett. 2018, 60, 1594–1600. [Google Scholar] [CrossRef]
- Jia, S.; Wang, S.; Liu, K.; Pang, X.; Zhang, H.; Jin, X.; Zheng, S.; Chi, H.; Zhang, X.; Yu, X. A unified system with integrated generation of high-speed communication and high-resolution sensing signals based on THz photonics. J. Light. Technol. 2018, 36, 4549–4556. [Google Scholar] [CrossRef]
- Withington, S. Terahertz astronomical telescopes and instrumentation. Philosophical Transactions of the Royal Society of London. Ser. A Math. Phys. Eng. Sci. 2004, 362, 395–402. [Google Scholar]
- Kulesa, C. Terahertz spectroscopy for astronomy: From comets to cosmology. IEEE Trans. Terahertz Sci. Technol. 2011, 1, 232–240. [Google Scholar] [CrossRef]
- Shangguan, Q.; Chen, Z.; Yang, H.; Cheng, S.; Yang, W.; Yi, Z.; Wu, X.; Wang, S.; Yi, Y.; Wu, P. Design of ultra-narrow band graphene refractive index sensor. Sensors 2022, 22, 6483. [Google Scholar] [CrossRef]
- Wang, X.; Lin, J.; Yan, Z.; Yi, Z.; Yu, J.; Zhang, W.; Qin, F.; Wu, X.; Zhang, J.; Wu, P. Tunable high-sensitivity sensing detector based on Bulk Dirac semimetal. RSC Adv. 2022, 12, 32583–32591. [Google Scholar] [CrossRef]
- Escorcia, I.; Grant, J.; Gough, J.; Cumming, D.R.S. Uncooled CMOS terahertz imager using a metamaterial absorber and pn diode. Opt. Lett. 2016, 41, 3261–3264. [Google Scholar] [CrossRef]
- Carranza, I.E.; Grant, J.P.; Gough, J.; Cumming, D. Terahertz metamaterial absorbers implemented in CMOS technology for imaging applications: Scaling to large format focal plane arrays. IEEE J. Sel. Top. Quantum Electron. 2016, 23, 2630307. [Google Scholar]
- Jornet, J.M.; Akyildiz, I.F. Graphene-based plasmonic nano-antenna for terahertz band communication in nanonetworks. IEEE J. Sel. Areas Commun. 2013, 31, 685–694. [Google Scholar] [CrossRef]
- Pan, W.; Shen, T.; Ma, Y.; Zhang, Z.; Yang, H.; Wang, X.; Zhang, X.; Li, Y.; Yang, L. Dual-band and polarization-independent metamaterial terahertz narrowband absorber. Appl. Opt. 2021, 60, 2235–2241. [Google Scholar] [CrossRef]
- Zheng, Z.; Zheng, Y.; Luo, Y.; Yi, Z.; Zhang, J.; Liu, Z.; Yang, W.; Yu, Y.; Wu, X.; Wu, P. A switchable terahertz device combining ultra-wideband absorption and ultra-wideband complete reflection. Phys. Chem. Chem. Phys. 2022, 24, 2527–2533. [Google Scholar] [CrossRef] [PubMed]
- Ning, R.; Bao, J.; Jiao, Z.; Xu, Y. Omnidirectional polarization-insensitive tunable absorption in graphene metamaterial of nanodisk structure. J. Appl. Phys. 2015, 118, 203101. [Google Scholar] [CrossRef]
- Chen, H.; Chen, Z.; Yang, H.; Wen, L.; Yi, Z.; Zhou, Z.; Dai, B.; Zhang, J.; Wu, X.; Wu, P. Multi-mode surface plasmon resonance absorber based on dart-type single-layer graphene. RSC Adv. 2022, 12, 7821–7829. [Google Scholar] [CrossRef] [PubMed]
- Hokmabadi, M.P.; Wilbert, D.S.; Kung, P.; Kim, S.M. Polarization-dependent, frequency-selective THz stereometamaterial perfect absorber. Phys. Rev. Appl. 2014, 1, 044003. [Google Scholar] [CrossRef]
- Hu, F.; Zou, T.; Quan, B.; Xu, X.; Bo, S.; Chen, T.; Wang, L.; Gu, C.; Li, J. Polarization-dependent terahertz metamaterial absorber with high absorption in two orthogonal directions. Opt. Commun. 2014, 332, 321–326. [Google Scholar] [CrossRef]
- Yu, H.; Meng, D.; Liang, Z.; Xu, H.; Qin, Z.; Su, X.; Smith, D.R.; Liu, Y. Polarization-dependent broadband absorber based on composite metamaterials in the long-wavelength infrared range. Opt. Express 2021, 29, 36111–36120. [Google Scholar] [CrossRef]
- Ouyang, L.; Wang, W.; Rosenmann, D.; Czaplewski, D.A.; Gao, J.; Yang, X. Near-infrared chiral plasmonic metasurface absorbers. Opt. Express 2018, 26, 31484–31489. [Google Scholar] [CrossRef]
- Mahmud, M.S.; Rosenmann, D.; Czaplewski, D.A.; Gao, J.; Yang, X. Chiral plasmonic metasurface absorbers in the mid-infrared wavelength range. Opt. Lett. 2020, 45, 5372–5375. [Google Scholar] [CrossRef]
- Tang, H.; Rosenmann, D.; Czaplewski, D.A.; Yang, X.; Gao, J. Dual-band selective circular dichroism in mid-infrared chiral metasurfaces. Opt. Express 2022, 30, 20063–20075. [Google Scholar] [CrossRef] [PubMed]
- Tang, B.; Li, Z.; Palacios, E.; Liu, Z.; Butun, S.; Aydin, K. Chiral-selective plasmonic metasurface absorbers operating at visible frequencies. IEEE Photonics Technol. Lett. 2017, 29, 295–298. [Google Scholar] [CrossRef]
- Wu, P.C.; Chen, J.W.; Yin, C.W.; Lai, Y.C.; Chung, T.L.; Liao, C.Y.; Chen, B.H.; Lee, K.-W.; Chuang, C.-J.; Wang, C.-M.; et al. Visible metasurfaces for on-chip polarimetry. ACS Photonics 2017, 5, 2568–2573. [Google Scholar] [CrossRef]
- Arbabi, E.; Kamali, S.M.; Arbabi, A.; Faraon, A. Full-Stokes imaging polarimetry using dielectric metasurfaces. ACS Photonics 2018, 5, 3132–3140. [Google Scholar] [CrossRef]
- Brand, G.F. The strip grating as a circular polarizer. Am. J. Phys. 2003, 71, 452–456. [Google Scholar] [CrossRef]
- Basiri, A.; Chen, X.; Bai, J.; Amrollahi, P.; Carpenter, J.; Holman, Z.; Wang, C.; Yao, Y. Nature-inspired chiral metasurfaces for circular polarization detection and full-Stokes polarimetric measurements. Light Sci. Appl. 2019, 8, 78. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, B.; Ouyang, M.; Ren, H.; Wu, J.; Zhang, Y.; Fu, Y. Polarization-Dependent Absorption and Transmission Metasurfaces for Linearly and Circularly Polarized Light in Terahertz Band. Photonics 2023, 10, 100. https://doi.org/10.3390/photonics10020100
Yang B, Ouyang M, Ren H, Wu J, Zhang Y, Fu Y. Polarization-Dependent Absorption and Transmission Metasurfaces for Linearly and Circularly Polarized Light in Terahertz Band. Photonics. 2023; 10(2):100. https://doi.org/10.3390/photonics10020100
Chicago/Turabian StyleYang, Bowei, Mingzhao Ouyang, Hang Ren, Jinshuang Wu, Yixin Zhang, and Yuegang Fu. 2023. "Polarization-Dependent Absorption and Transmission Metasurfaces for Linearly and Circularly Polarized Light in Terahertz Band" Photonics 10, no. 2: 100. https://doi.org/10.3390/photonics10020100
APA StyleYang, B., Ouyang, M., Ren, H., Wu, J., Zhang, Y., & Fu, Y. (2023). Polarization-Dependent Absorption and Transmission Metasurfaces for Linearly and Circularly Polarized Light in Terahertz Band. Photonics, 10(2), 100. https://doi.org/10.3390/photonics10020100




