The Proposal of a Photon–Photon Resonance Control Scheme by Using an Active MMI Laser Diode

Abstract

The modulation bandwidth in the direct modulation of a laser diode is limited by relaxation oscillation. To achieve an even higher frequency response, photon–photon resonance has been investigated to extend this modulation bandwidth. Thus far, several reports have demonstrated a higher modulation performance being achieved when utilizing PPR; however, to our knowledge, the PPR control scheme has not been comprehensively discussed. In this paper, we discuss the theory of PPR in regard to the PPR frequency, intensity, and width control scheme. We propose to utilize waveguide configuration, specifically an active multimode interferometer, for optimization. The simulation results offer an approximately 8 GHz improvement in the frequency response.

1. Introduction

It is forecasted that the interconnection speed in supercomputers will exceed 1 Tbps in the near future [1]. For this reason, optical interconnection has been already deployed in supercomputers [2,3,4]. A similar performance to that of supercomputers will be required in personal computers (PCs) within a few decades, according to past research [5]. Optical interconnection, therefore, may be also introduced into mobile devices, including PCs, smartphones, and others. For these applications, a directly modulated laser diode (DML) is one candidate, with the merits of a small footprint and relatively low energy consumption [6].

The DML’s modulation bandwidth is restricted, in principle, by the so-called relaxation oscillation frequency (or, in other words, the carrier photon resonance (CPR) frequency). Here, we write this frequency as $f_{CPR}$. It is well known that damping occurs in the frequency response in regions with a higher frequency than $f_{CPR}$. Consequently, the response degrades by less than −3 dB at around $f_{CPR}$ (typically a slightly higher frequency than $f_{CPR}$). Because of this damping, a response at a much higher frequency is not available, which prevents potential high-speed direct modulation.

One possible means to overcome the restriction of relaxation oscillation is to utilize photon–photon resonance (PPR) [7,8,9,10,11,12,13,14], which is known to display a higher-frequency resonance compared to CPR. Thus far, all the theoretical works focused on laser diodes (LDs) have reported that PPR phenomena appear with the existence of an additional oscillation path, besides the main oscillation path, in an LD cavity [15,16,17,18,19,20,21,22,23,24]. PPR at 45 GHz was calculated in a DBR laser with a complex cavity [20]. A coupled cavity injection grating laser [21] was proposed to extend the modulation bandwidth to 37 GHz. An active multimode interferometer (MMI) laser diode (LD) with multiple PPRs at 24 GHz and 60 GHz was reported [19]. A DFB+R laser exhibited a bandwidth over 65 GHz [22]. A novel dual DFB laser increased the modulation bandwidth to 57.6 GHz [23]. Furthermore, a bandwidth of 108 GHz was reported in a DFB laser combined with a DBR structure [24]. This is because another resonance peak, in addition to $f_{CPR}$, improves the damping at higher frequencies than $f_{CPR}$. Therefore, the so-called −3 dB bandwidth is extended compared to the case without PPR. Compared to other reports on PPR in DBR and DFB lasers, there are two advantages of the active MMI laser. The first is that there are no grating structures, which eases the fabrication. The second is that the active MMI LD configuration offers the potential to exploit multiple PPRs. To achieve a higher-frequency response without −3 dB degradation in the bandwidth in an active MMI LD, one important point is to optimize the peak position, width, and height (i.e., using a control scheme for the PPR peak). However, it seems that the investigation of the control scheme is not sufficient. In this paper, we propose a control scheme for the PPR peak in terms of the frequency position, peak intensity height, and width, using an active MMI laser diode configuration. Based on a theoretical analysis, coupling efficiency is a key parameter of PPR phenomena. Previously, PPR control with coupling efficiency in VCSEL lasers was reported by changing the aperture between two sections [25]. In this work, we utilize a unique active MMI LD that enables the tuning of the coupling efficiency. In this active MMI LD, two spatial lasing modes are employed. The unique active MMI LD configuration enables two different lasing paths sharing the same output port. In addition, the proposed active MMI LD consists of separated identical waveguides. By considering the length balance of the output port waveguide and the separated identical waveguides, the coupling efficiency is designed as the optimum portion. As a result, we demonstrate a substantial improvement of 8 GHz in the frequency response when the coupling ratio is set at 75%.

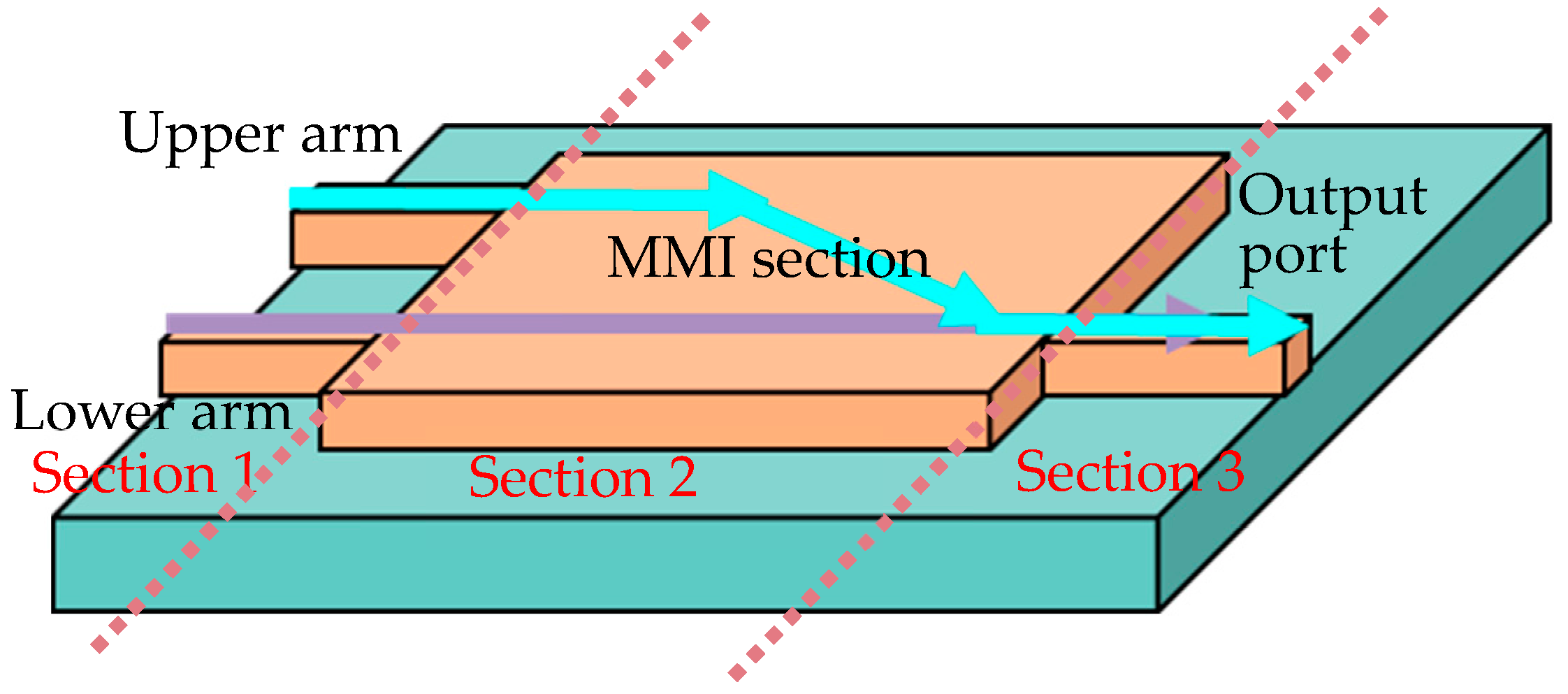
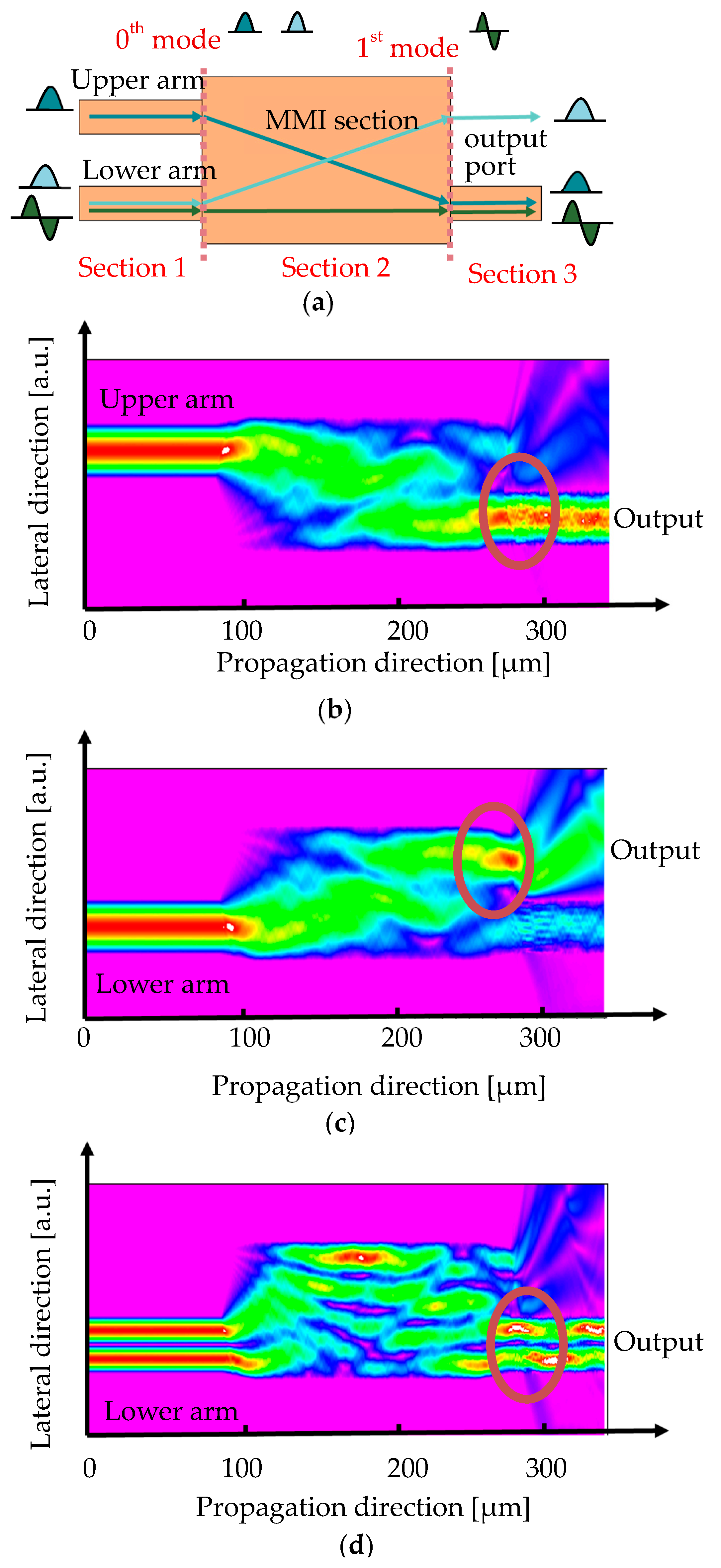
2. PPR Numerical Model in Proposed Active MMI LD

It has been reported that PPR phenomena are related to the interaction between multiple longitudinal lasing modes in LD [15,16,17,18,19,20,21,22,23,24,25]. To generate multiple lasing modes, multiple cavities assembled in one device are required. For the purpose of achieving and controlling the PPR phenomenon, we propose utilizing a unique, mode-selectable active MMI configuration in this paper. This is because this configuration enables a common output port, whereas it secures different lasing paths independently in a single laser diode, which is explained as follows. Figure 1 illustrates the waveguide configuration of this active MMI LD. It is divided into three sections. Section 1 is a separated identical waveguide in LHS. Section 2 is the MMI section, which lies in the middle as the main pumping area. Section 3 is the output waveguide section, which is connected to the RHS of the MMI section. Section 3 is the modulation region. Cleaved waveguide facets are present in Section 1 and Section 3. Facet reflection is used in this design. All the sections in the active MMI LD are active areas. An active MMI high-power laser was reported previously [26] and the direct-monitored light field had a mostly decentral profile, in contrast to the case of hole burning attributed to filamentation. Hence, we assume that the gain is relatively uniform in this paper. In this configuration, one route supports the lateral fundamental order oscillation mode for one wavelength, and the other one is the lateral first-order oscillation mode at a different wavelength [27]. In this case, the two different routes merge to form a single output.

As explained above, this configuration supports two distinct oscillation modes and, consequently, two different lasing wavelengths. In the case of two different lasing wavelengths, it is known that the following rate equation is exploitable. Using the following equations, it is possible to analyze PPR phenomena in the proposed active MMI configuration. All four rate equations are derived from electro-magnetic wave theory in lasers [28,29,30,31,32,33,34,35,36,37,38]. Unlike the traditional one [39], two lasing modes are included in the rate equation. One is referred to as the main lasing mode and the other is referred to as the sub-lasing mode.

The four differential equations for the PPR phenomena are summarized in the following (1)–(4) (The detail of the rate equations are explained in the Appendix A):

$$\frac{d{S}_1\left(t\right)}{dt}=G_1\left. [N\left(t\right)-N_{th}\right]\times S_1\left(t\right)$$

(1)

$$\frac{\mathrm{d}{S}_2\left(t\right)}{\mathrm{d}t}=G_2\left. [N\left(t\right)-N_{th}\right]\times S_2\left(t\right)+2\kappa \sqrt{{(S}_1\left(t\right)\times \left(t\right)}\cos \left({\theta }_2\left(t\right)-{\theta }_1\left(t\right)\right)$$

(2)

$$\frac{d{\theta }_2(t)}{dt}=\frac{\alpha }{2}{[G}_2\left(N\left(t\right)-N_{th}\right)]+\kappa \sqrt{\frac{S_1\left(t\right)}{S_2\left(t\right)}}\mathit{sin}({\theta }_2(t)-{\theta }_1(t)) -\Delta \omega$$

(3)

$$\frac{dN\left(t\right)}{dt}=J-\frac{N\left(t\right)}{{\tau }_r}-G_1\left. [(N\left(t\right)-N_{tr}\right]\times S_1\left(t\right)-G_2\left. [N\left(t\right)-N_{tr}\right]\times S_2\left(t\right)$$

(4)

$S_1\left(t\right)$ and $S_2\left(t\right)$ are the photon numbers of the main lasing mode and sub-lasing mode. $N\left(t\right)$, $N_{th}$, $N_{tr}$, and $\alpha$ are the carrier number, threshold carrier number, transparency carrier number, and linewidth enhancement factor, respectively. $G_1 \mathrm{a}\mathrm{n}\mathrm{d}{ G}_2$ are the gain coefficients of the main lasing mode and sub-lasing mode. ${\tau }_{p1}$ and ${\tau }_{p2}$ are the photon lifetimes of the two lasing modes. In the lasing condition, $G\left(N_{th}-N_{tr}\right)=1/{\tau }_p$. ${\tau }_r$ is the carrier lifetime. ${\theta }_1(t)$ and ${\theta }_2(t)$ are the phase terms in the electrical field of the main lasing mode and sub-lasing mode. $\Delta \omega$ is the frequency difference of the main lasing mode and sub-lasing mode. $J$ is the current injection. $\kappa$ is the coupling efficiency. All parameters used in this paper are listed in

Table 1. Parameters of rate equation with PPR [40,41].

Symbol	Value	Unit
$G_1$	7888	1/s
$G_2$	1972	1/s
$N_{th}$	2.5 × 109	#
α	5	-
$J$	2 × $J_{th}$	1/s
$J_{th}$	7.5 × 1017	1/s
${\tau }_r$	8.3 × 10−9	s
${\tau }_{p1}$	2.8 × 10−12	s
${\tau }_{p2}$	7.34 × 10−12	s
L	360	µm
$\kappa$	125.7	1/ns

. $\kappa$ has been historically defined as follows [33,34,35,36,37]:

$$\kappa =\frac{c}{{2n}_{eff}\times L}$$

(5)

$c$ is the group velocity of light. $n_{eff}$ and $L$ are the effective refractive index and total length of the laser diode (All the value are listed in Table 1).

The differences from the conventional rate equation are listed as follows.

(1)

Equation (2): This equation is the derivative of the photon density for the sub-lasing mode, in addition to the one for the main lasing mode (Equation (1)).

(2)

As indicated, it involves a co-related term between the main and sub-lasing modes as a second term related to the coupling efficiency, $\kappa$. Note that, in Equation (1), this co-related term is neglected as the main lasing mode is strongly associated, rather than a sub-mode laser, in a laser cavity. Equation (3): This equation considers that this phase term relates to the co-relation in longitudinal mode.

(3)

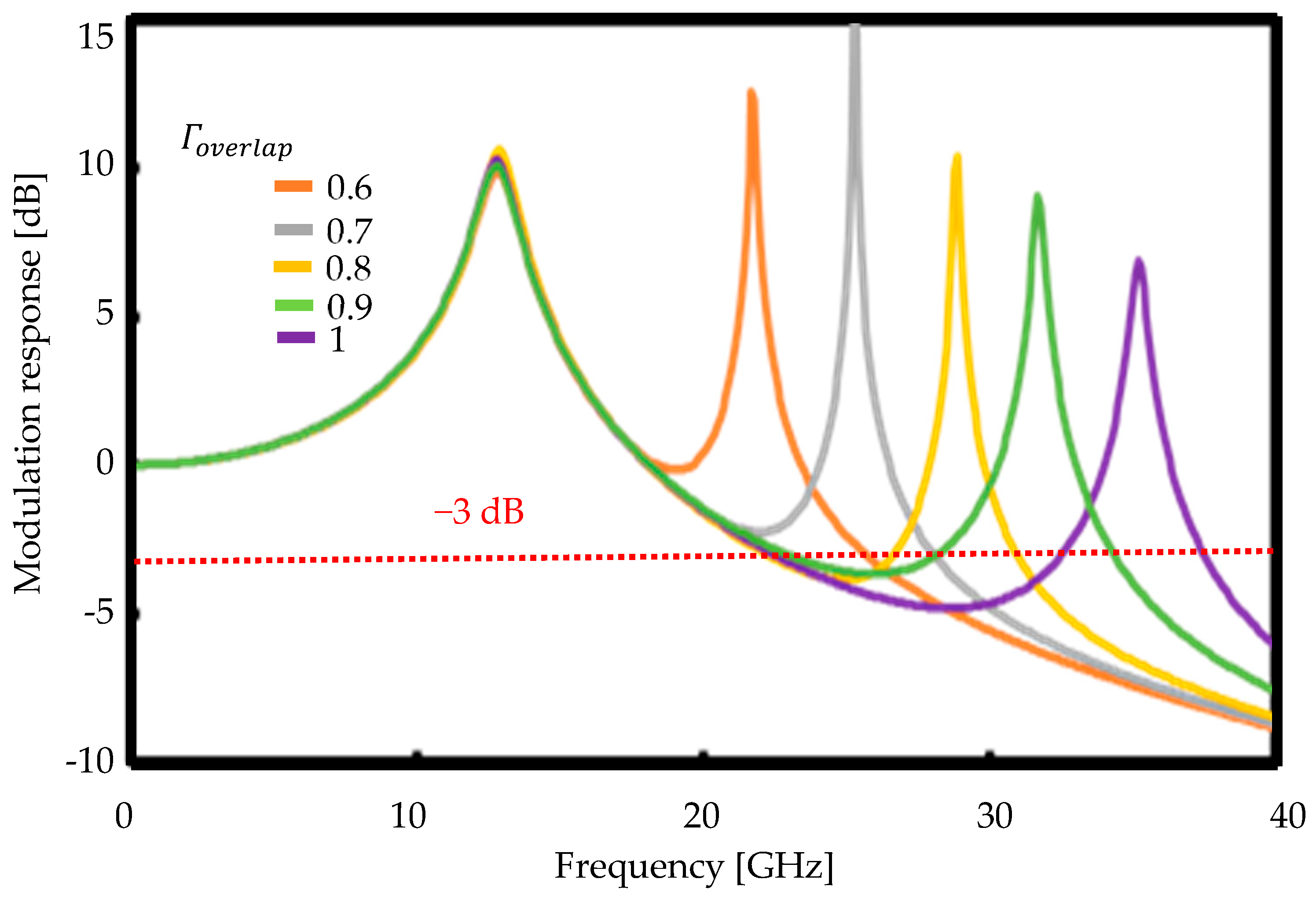
To ensure that $\kappa$ corresponds to the coupling efficiency between the main and sub-lasing modes, we introduce a coupling ratio ${\Gamma }_{overlap}$ in $\kappa$ [27], which satisfies $0⩽{\Gamma }_{overlap}⩽1$. ${\Gamma }_{overlap}$ is defined as the optical profile overlap of the lateral mode between the two lasing modes. In this situation, the coupling efficiency in the active MMI LD is:

$$\kappa ={\Gamma }_{overlap}\times \frac{c}{{2n}_{eff}\times L}$$

(6)

(4)

$\kappa$ affects PPR phenomena directly. The coupling efficiency is defined as the propagation of two lasers along the entire cavity to couple. The two lasing modes in the active MMI LD are not always present together, as visible in Section 1. As the 1 × N active MMI configuration may offer the possibility to control ${\Gamma }_{overlap}$ itself, we verify the effect of ${\Gamma }_{overlap}$ in the following.

Using the rate equations (Equations (1)–(4)), it is possible to analyze the PPR phenomenon in the proposed active MMI configuration. The modulation response of the laser diode with PPR is calculated by applying the small-signal response approach [26]. Similarly to the conventional small-signal analysis, the small-modulated current, carrier number, and photon number (main lasing mode and sub-lasing mode) are assumed in the analysis, and the frequency response in the laser output is obtained (Parameters are listed in

Table 2. Parameters of transfer function in active MMI LD.

Symbol	Value
$m_{s1n}$	$-G_1{S}_1$
$m_{s2s2}$	$zcos{\theta }_0$
$m_{s2\mathrm{\Phi }}$	$2z{S}_{2}sin{\theta }_0$
$m_{s2n}$	${-G}_2{S}_2$
$m_{\mathrm{\Phi }s2}$	$-\frac{z}{2S_2 }sin{\theta }_0$
$m_{\mathrm{\Phi }\mathrm{\Phi }}$	$zcos{\theta }_0$
$m_{\mathrm{\Phi }n}$	$-\frac{\alpha }{2}{G}_2$
$m_{ns1}$	$1/{\tau }_{p1}$
$m_{ns2}$	$1/{\tau }_{p2}$
$m_{nn}$	$\frac{1}{{\tau }_r}+G_1{S}_1+G_2{S}_2$
$m_{s2s2}$	$zcos{\theta }_0$
$z$	$k\sqrt{S_1/S_2}$

).

$$H\left(jw\right)=\frac{{\Delta S}_1+{\Delta S}_2}{\Delta J}=\frac{P({jw)}^2+Q\left(jw\right)+T}{({jw)}^4+A({jw)}^3+B({jw)}^2+C\left(jw\right)+D}$$

(7)

$$P=m_{s1n}+m_{s2n}$$

(8)

$$Q=m_{s1n}\left(m_{s2s2}+m_{\mathrm{\Phi }\mathrm{\Phi }}\right)+m_{s2\mathrm{\Phi }}{m}_{\mathrm{\Phi }n}-m_{s2n}{m}_{\mathrm{\Phi }\mathrm{\Phi }}$$

(9)

$$\mathrm{T}=m_{s1n}(m_{s2s2}{m}_{\mathrm{\Phi }\mathrm{\Phi }}-m_{s2\mathrm{\Phi }}{m}_{\mathrm{\Phi }s2})$$

(10)

$$A=m_{s2s2}+m_{\mathrm{\Phi }\mathrm{\Phi }}+m_{nn}$$

(11)

$$B=m_{nn}+m_{s2s2}{m}_{\mathrm{\Phi }\mathrm{\Phi }}-m_{s1n}{m}_{ns1}+m_{\mathrm{\Phi }\mathrm{\Phi }}{m}_{\mathrm{\Phi }\mathrm{\Phi }}{-m}_{s2\mathrm{\Phi }}{m}_{\mathrm{\Phi }s2}{-m}_{s2n}{m}_{ns2}$$

(12)

$$C=m_{ns2}{m}_{s2\mathrm{\Phi }}{m}_{\mathrm{\Phi }n}{-m}_{s2n}{m}_{\mathrm{\Phi }\mathrm{\Phi }}{m}_{ns2} {+m}_{s2s2}{m}_{\mathrm{\Phi }\mathrm{\Phi }}{m}_{nn}{-m}_{nn}{m}_{s2\mathrm{\Phi }}{m}_{\mathrm{\Phi }s2}$$

(13)

$$D=m_{s1n}{m}_{ns1}(m_{s2\mathrm{\Phi }}{m}_{\mathrm{\Phi }s2}-m_{s2s2}{m}_{\mathrm{\Phi }\mathrm{\Phi }})$$

(14)

The modulation response derived from the four rate equations suggests that the PPR phenomenon is affected by the coupling efficiency. Figure 2 shows the modulation response as a parameter of the coupling ratio. Here, we use the condition of relaxation oscillation frequency (or CPR: carrier photon resonance frequency) f_CPR = 12 GHz, and an intrinsic PPR frequency of 34 GHz with ${\Gamma }_{overlap}$ = 1. Due to the damping problem between CPR and PPR, the modulation bandwidth only reaches a value of 22 GHz. As ${\Gamma }_{overlap}$ decreases from 1 to 0.6, the PPR tends to be shifted to a lower frequency from 34 GHz to 22 GHz. The width of the PPR tends to become narrower, which has a negative impact on the modulation bandwidth improvement. Despite this width narrowing effect, the peak height of PPR increases to ${\Gamma }_{overlap}=$ 0.7. This peak height increase is effective in improving the extension of the modulation bandwidth $f_{3dB}$. It is necessary to ensure that the peak height does not increase continuously as ${\Gamma }_{overlap}$ increases.

From the discussion above, the PPR phenomenon appears to be significantly affected by the coupling ratio. In other words, targeted PPR can be engineered by optimizing the coupling ratio. The optimal ${\Gamma }_{overlap}$ exists between 0.7 and 0.8. The active MMI LD has the potential to improve the control coupling efficiency by changing the configuration.

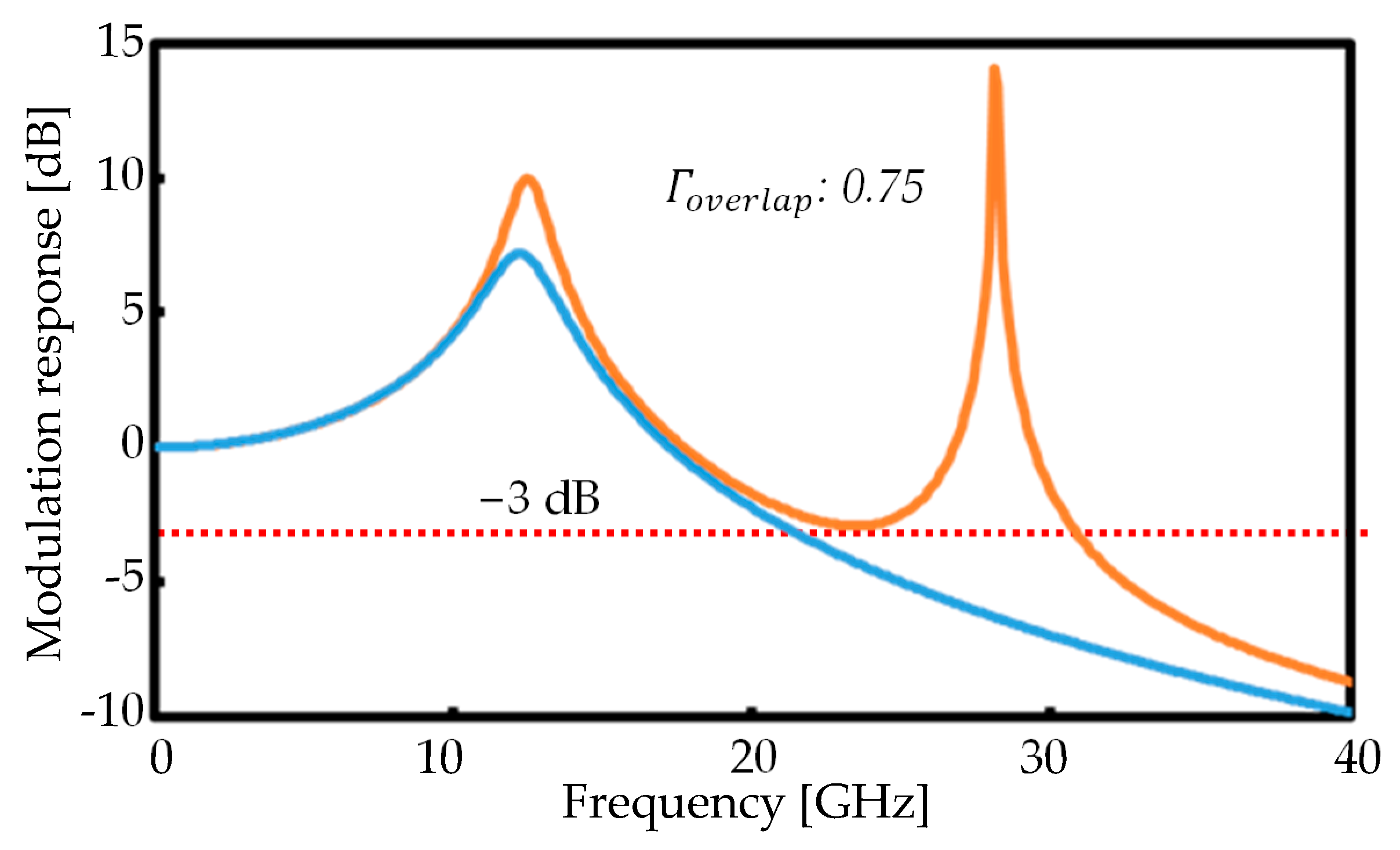
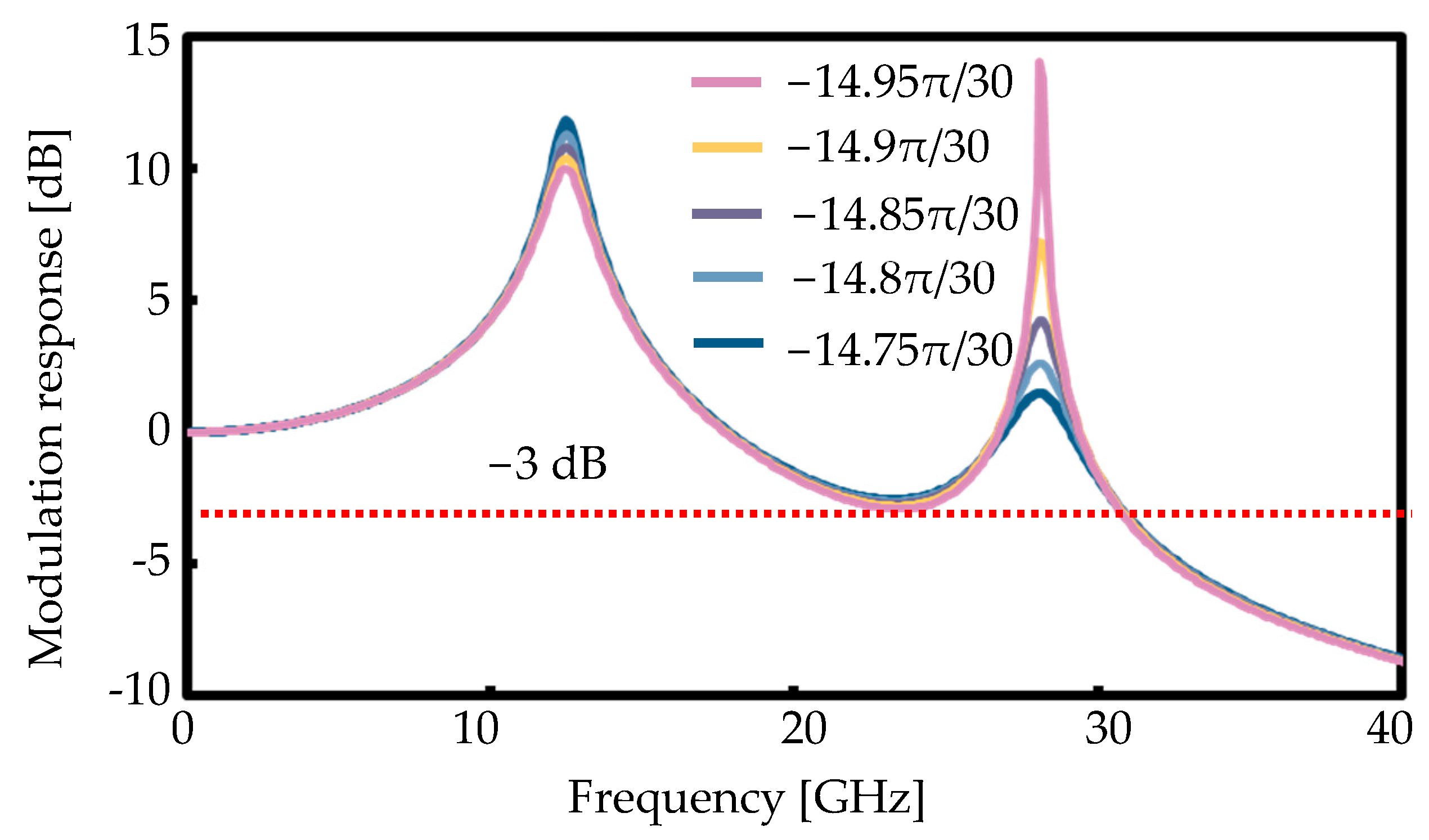
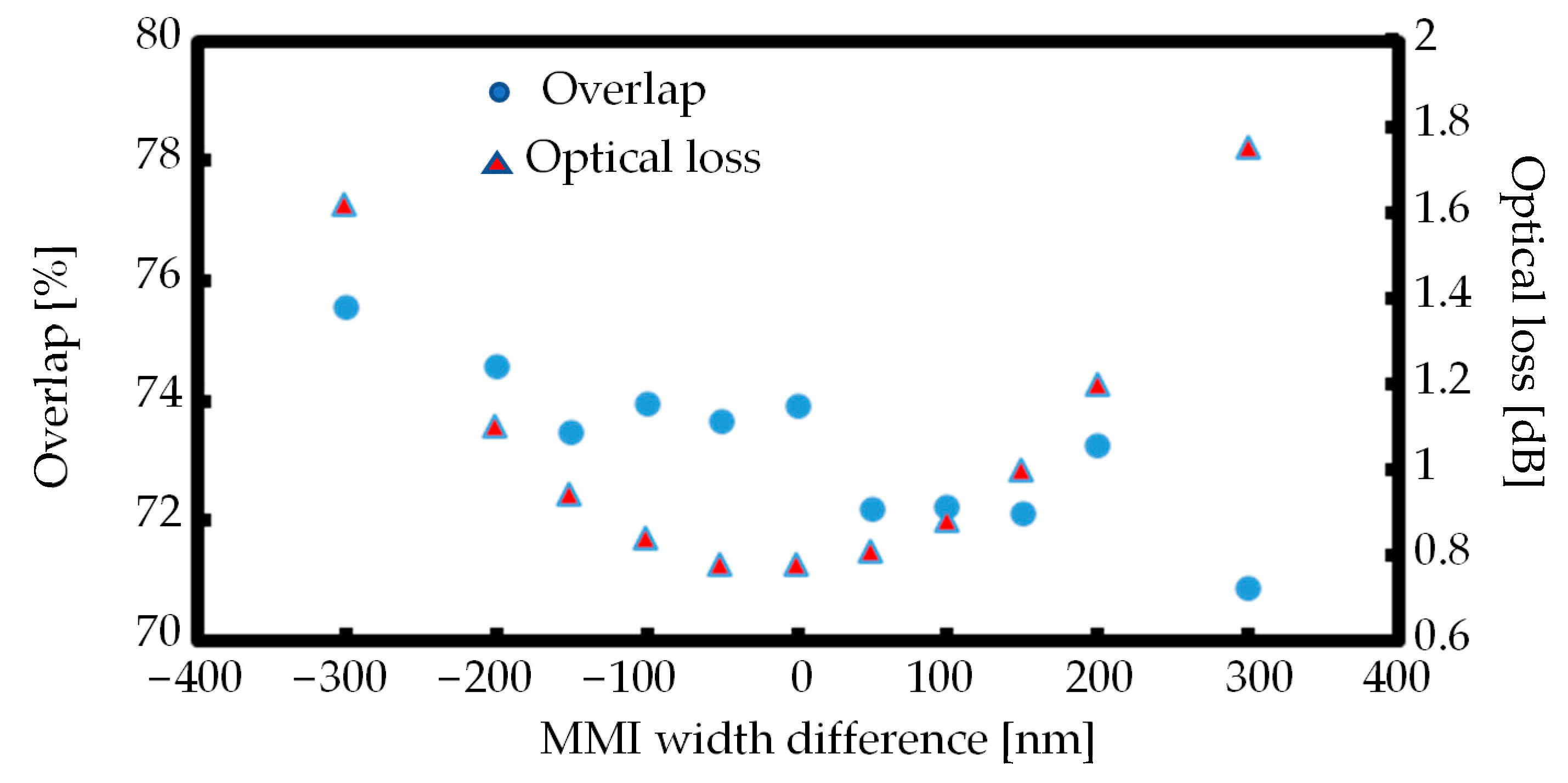
3. Active MMI Configuration for the Control of PPR and Discussion

As explained in Section 2, ${\Gamma }_{overlap}$ related to the coupling efficiency is a key parameter in the PPR phenomenon. In this section, we show how to adjust ${\Gamma }_{overlap}$ by modifying the active MMI LD’s geometry.

Figure 3a shows the waveguide configuration of the 1 × 2 active MMI LD involving different lasing paths in a cavity for each spatial mode. In Section 1, when the upper arm and lower arm are both designed as fundamental mode waveguides, the two different lasing paths do not share the same output port. As the BPM simulation results show in Figure 3b,c, each path for the fundamental mode is designed as cross-propagation in Section 2. The coupling area for the two fundamental modes is only the MMI area. The coupling efficiency and its variation are small. With the aim of solving this problem, different lateral modes are utilized. As shown in Figure 3d, the first-order mode propagates in the bar direction in Section 3. Thus, two different lasing paths exist, whereas the output port is shared. As shown in Figure 3b–d, despite the presence of optical leakages in the absent upper output section, the majority of the incident light will be confined within the single waveguide in Section 3. The optical loss is less than 1 dB. Consequently, the impact on modulation is anticipated to remain minimal.

Considering that the lasing paths of the two lasing modes are different in the 1 × 2 active MMI LD, the coupling efficiency of the two lasing modes in each section has to be discussed. When two space modes are lasing in Section 1, the two lasing modes are separated from each other. In Section 2 and Section 3, the two lasing modes overlap. This overlap is close to 1 in Section 3, whereas the lateral optical field overlap of the two lasing modes is less than 1 in Section 2. The expression of ${\Gamma }_{overlap}$ in the active MMI LD is

$${\Gamma }_{overlap}=\frac{{\Gamma }_{Se2}{L}_{Se2}}{L_{total}}+\frac{L_{Se3}}{L_{total}}$$

(15)

In this expression, ${\Gamma }_{Se2}$, $L_{Se2}$, $L_{Se3}$, and $L_{total}$ are, respectively, the optical field overlap of the two modes in the MMI section (Section 2), the length of Section 2, the length of Section 3, and the total length of the whole active MMI LD. This definition suggests that ${\Gamma }_{overlap}$ is affected by the proportion of the three regions. Thus, the parameter ${\Gamma }_{Se2}$ has to be determined.

The optical field overlap of the two lasing modes in Section 2 is calculated to confirm ${\Gamma }_{Se2}$. In the calculation process, we set the length of Section 1 at 90 μm and the length of Section 3 at 50 μm. The wavelength value in the simulation process is set to 1550 nm. The simulation results of the fundamental mode and first-order mode in Figure 3c,d suggest that the optical field overlap of the two lasing modes varies along the propagation direction. Moreover, Figure 4a illustrates the optical profile of two lateral modes in the middle of Section 2. As indicated in the figure, the optical profiles of the two lasing modes do not entirely overlap. To calculate ${\Gamma }_{Se2}$, from Figure 4b, we divide the MMI area into slices along the propagation direction. Hence, the overlap of the two lasing modes in each slice is calculated. ${\Gamma }_{Se2}$ is obtained. The result shows that ${\Gamma }_{Se2}$ is around 0.74 when the MMI area length changes from 150 μm to 300 μm.

Based on the discussion above, the ${\Gamma }_{overlap}$ is determined by the proportion in the active MMI LD configuration. The length of the MMI area is set at 240 μm. The relationship between the length of the arm part and ${\Gamma }_{overlap}$ is depicted in Figure 5. ${\Gamma }_{overlap}$ can be set from 0.5 to 0.8.

It is necessary to point out that, when utilizing different lateral modes in the active MMI LD, the coupling efficiency is tunable. Multimode light, however, is not commonly used as a light source for optical interconnection due to the dispersion loss in long-distance transmission. An active MMI LD is considered as the optical light source for interconnection in supercomputers or mobile devices. This implies that this device can be applied in short-distance cases for which dispersion loss would not be a serious problem.

Based on the theoretical analysis discussed in Section 2, a wider frequency response may occur for ${\Gamma }_{overlap}=$ 0.7. We verified the response in detail. We found that ${\Gamma }_{overlap}=$ 0.75 was the optimal value in this case. Figure 6 shows the calculated frequency response of the ${\Gamma }_{overlap}=$ 0.75 case. An 8 GHz improvement was successfully achieved in the modulation bandwidth. The control of ${\Gamma }_{overlap}$ is enabled through the proportions of the three parts of the active MMI LD. ${\Gamma }_{overlap}$ at 0.75 is obtained by setting Section 1, Section 2, and Section 3 at 30 μm, 220 μm, and 110 μm, respectively. It is possible that modifying the lengths of Section 1 and Section 3 will impact the free spectral range (FSR) of the Fabry–Perot resonance, which, in turn, influences the CPR and the PPR. In our specific design, the overall cavity length and the length of the primary MMI section remain unchanged. Consequently, the proportion of Section 1 and Section 2 is the focal point of our design efforts. Given that the primary MMI section and the total cavity length remain unaltered, the FSR of the Fabry–Perot resonance will also remain unaffected.

Although the modulation bandwidth extension is confirmed by the existence of PPR, it is worth noting that the PPR intensity stands at approximately 14 dB, which is regarded as a detrimental factor affecting large signal modulation. In order to address this issue, it is important to devise a control scheme to manage the intensity. In the PPR model proposed in Section 2, the PPR phenomenon is treated as a synchronization phenomenon. Based on synchronization theory [27], it is strongly related to the initial phase of two oscillations. The simulation results, which depict the characterization of the PPR at ${\Gamma }_{overlap}=$ 0.75 under varying initial phase differences, are presented in Figure 7. When the initial phase difference is −14.95π/30, the peak in PPR is observed at 14 dB. The intensity of PPR is reduced with the change in the initial phase difference ranging from −14.95π/30 to −14.75π/30. When the initial phase difference is −14.75π/30, the intensity of the PPR is 0 dB. The fluctuation in the PPR intensity suggests that the PPR phenomenon may not manifest under certain circumstances, primarily due to the absence of optimal tuning in the initial phase difference between the two lasing modes. As ${\Gamma }_{overlap}=$ 0.75, an 8 GHz improvement with a lower PPR intensity is confirmed, as shown in Figure 7. To tune the initial phase, it is imperative to implement separate current injections for each waveguide within Section 1 of this active MMI LD. The initial phase is then adjusted when operating the current value.

4. Discussion

A notable enhancement of 8 GHz in the modulation bandwidth is confirmed when the f_CPR is set at 12 GHz. It is necessary to point out that the optimum design for different f_CPR is different. The location of f_CPR is the main determinant of the optimized value of the coupling efficiency. The reason that f_CPR is set at 12 GHz is that, in the former experiments, for an active MMI laser around 400 µm, the highest f_CPR is located at around 10 GHz. If f_CPR is located at 15 GHz, the optimized value changes. In Figure 8, the simulation results show that, with a higher f_CPR, a higher f_PPR is preferred to extend the modulation bandwidth. The optimal value in this case is around ${\Gamma }_{overlap}$= 0.85. Based on the simulation results, it is suggested that, in the design process, different ${\Gamma }_{overlap}$ values need to be considered.

While we successfully enhanced the modulation response, it is important to note that our simulations were conducted under the conditions of the effective refractive index with no lasing operation. The effective refractive index, however, varies with the current injection because of the so-called carrier plasma effect [42]. In the semiconductor field, when a laser is lasing, the carrier density inside the active MMI laser diode accumulates from 0 to its threshold carrier density [40]. Changes in the effective refractive index can significantly influence the propagation paths of lasing modes, leading to alterations in the overlap between these modes. Therefore, it is crucial to consider the impact of variations in the effective refractive index resulting from the accumulation of the carrier density.

When a laser composed of InGaAsP is lasing at 1.55 µm, the increase in the carrier density results in a reduction in the refractive index. The difference in effective refractive index $\Delta n$ would be lower than 0.1 [43]. As shown in Table 1, the refractive index of our device is 3.24. We conducted calculations to determine the overlap between two light sources across a range of effective refractive indices, spanning from 3.14 to 3.34. The calculation results are shown in Figure 9.

Based on the simulation results shown in Figure 9, the stability of the overlap is confirmed. Although the overlap between the two lights changed with different effective refractive indices, it remained within the range of 0.74–0.75. This result suggests that the active MMI laser diode is able to operate stably, even in the presence of variations in the effective refractive index induced by the accumulation of the carrier density.

In addition to the carrier plasma effect, temperature is a factor that needs to be taken into consideration, as rising temperatures lead to a narrower bandgap, consequently resulting in an increase in the refractive index. During experimental procedures, it is essential to employ a temperature controller in order to maintain and stabilize the operating conditions at room temperature. This ensures that temperature-related effects are controlled and minimized. The real temperature inside the active MMI LD, however, is still different from the setting temperature [44]. The Varshni relation [45] for the temperature dependence of semiconductor bandgaps is:

$$E_g\left(T\right)=E_0-\alpha T^2/(T+\beta )$$

(16)

where $\alpha$ and $\beta$ are fitting parameters characteristic of a given material [46]. Gupta and Ravindra [47] express the refractive index and energy bandgap as:

$$n=4.16-1.12E_g+0.31E_g^2-0.08E_g^3$$

(17)

Using Equations (16) and (17), at room temperature, the refractive index variation $\Delta n$ in the active MMI LD is far less than 0.1 when the temperature rises from 290 K to 310 K. As indicated by the simulation result shown in Figure 9, the desired coupling ratio is maintained.

Finally, the fabrication tolerance is discussed. The single output port in Section 3 of the active MMI LD configuration relies on the self-image phenomenon in the MMI section, which is significantly affected by the width of the MMI section [26]. With a fabrication error, the overlap between the two modes and the optical loss will be affected, which can impact the laser performance. Therefore, the optical loss and the overlap variation with the width difference are calculated, and the results are shown in Figure 10. The optical loss of the optimum active MMI LD is 0.78 dB. It rises with the increase in the width difference. When the width difference is within ±150 nm, the optical loss is below 1 dB. Regarding the overlap, with the width difference of ±150 nm, the overlap is stabilized within the range from 72% to 74%. Given that the overlap and the optical loss at a width difference of ±150 nm remain minimal, the fabrication tolerance of the active MMI LD is ±150 nm.

In this work, the control scheme of PPR in a 1 × 2 active MMI LD is discussed. This is a promising method as it offers the potential for broader applicability to active MMI LD structures supporting more sub-modes. When minimizing the length of the LD, a larger modulation bandwidth is expected.

5. Conclusions

In this work, we proposed an active MMI LD configuration to achieve the ideal overlap between the main mode and sub-mode of a laser. The optimized modulation response was obtained when ${\Gamma }_{overlap}$ was 0.75, and the design of an active MMI laser was also proposed. We plan to verify this with experiments and exploit a similar scheme for the control of multiple PPR peaks, thereby aiming for even greater modulation response capabilities.