Interface Engineering and Electron-Hole Wave Function Overlap of InAs/AlSb Superlattice Infrared Detectors
Abstract
:1. Introduction
2. Theoretical Model
3. Results and Discussion
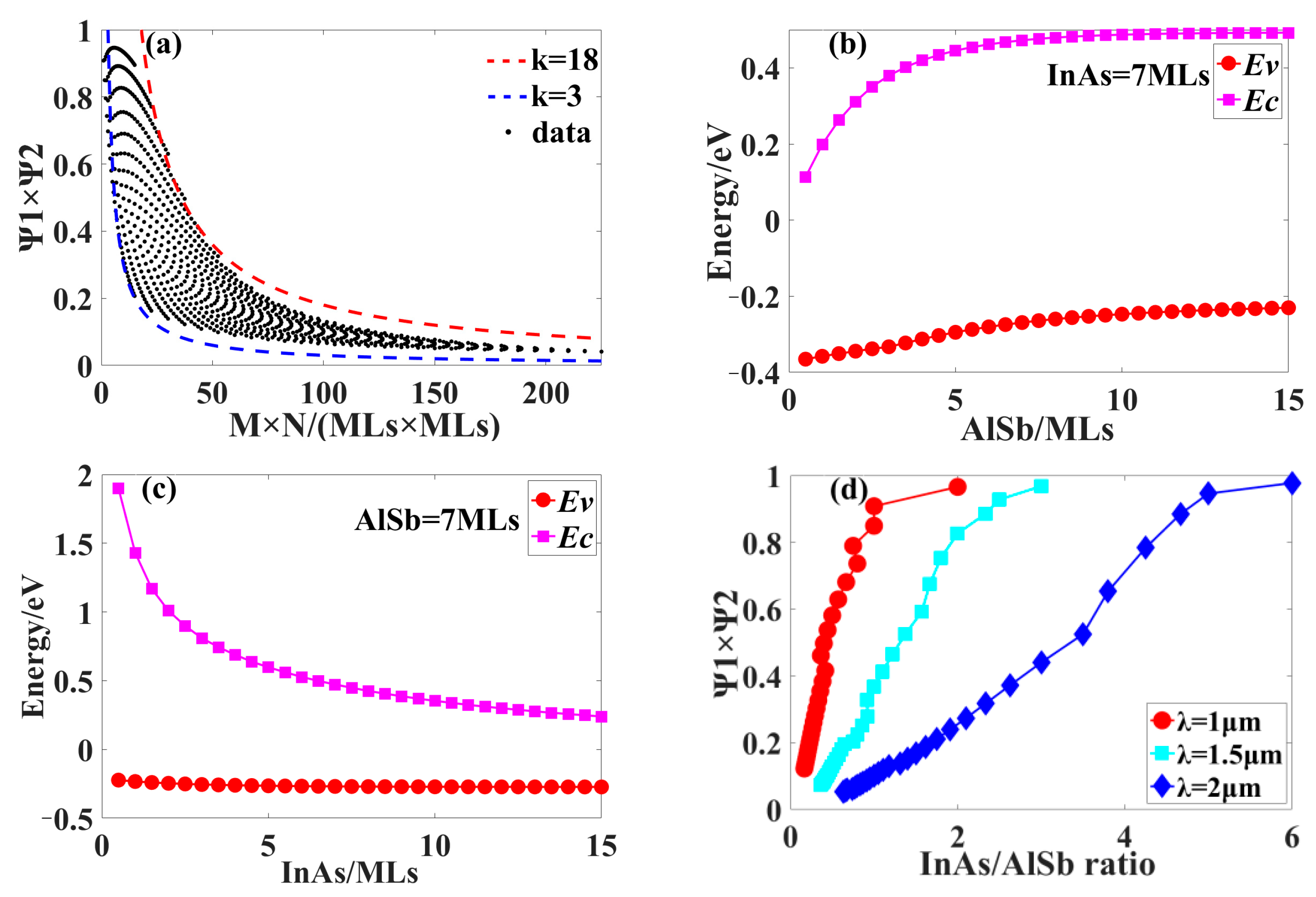
3.1. InAs/AlSb Superlattice
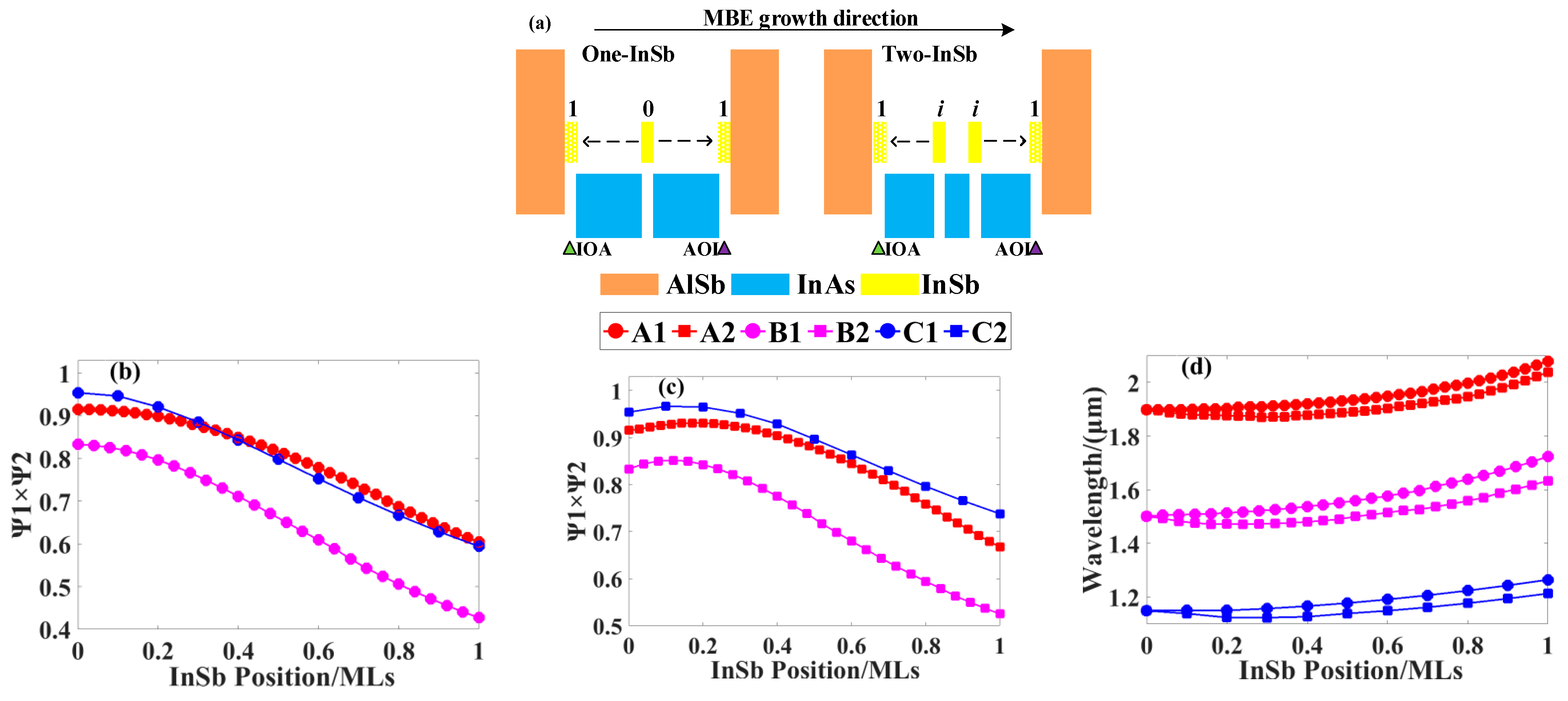
3.2. The Relationship between InSb Interface Layer and WFO
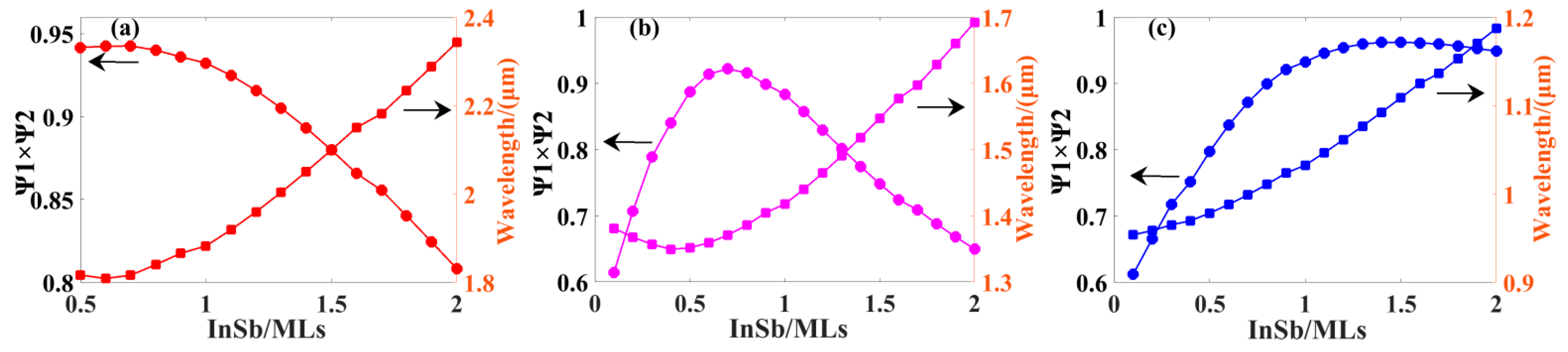
3.2.1. One Interface Layer of InSb
3.2.2. Two Interface Layers of InSb
3.3. The Relationship between AlAs Interface Layer and WFO
3.3.1. One Interface Layer of AlAs
3.3.2. Two Interface Layer of AlAs
3.4. The Relationship between Stress-Balance-Based Interface Engineering and the Size of Electron-Hole WFO
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- John, E.B.; Anand, R.; Dai, D.X.; Wissem, S.Z.; Yimin, K.; Tao, Y.; Mike, M. Recent advances in Ge/Si PIN and APD photodetectors. Phys. Status Solidi C 2010, 7, 2526–2531. [Google Scholar] [CrossRef]
- Sieck, A.; Benecke, M.; Eich, D.; Oelmaier, R.; Wendler, J.; Figuregemeier, H. Short-Wave Infrared HgCdTe Electron Avalanche Photodiodes for Gated Viewing. J. Electron. Mater. 2018, 47, 5705–5714. [Google Scholar] [CrossRef]
- MohammadNejad, S.; Aghaei, F. Noise characteristics improvement of submicron InP/InGaAs avalanche photodiode for laser detection system. Opt. Commun. 2020, 455, 124561. [Google Scholar] [CrossRef]
- Ronningen, T.J.; Kodati, S.H.; Jin, X.; Lee, S.; Jung, H.; Tao, X.; Lewis, H.I.J.; Schwartz, M.; Gajowski, N.; Martyniuk, P.; et al. Ionization coefficients and excess noise characteristics of AlInAsSb on an InP substrate. Appl. Phys. Lett. 2023, 123, 131110. [Google Scholar] [CrossRef]
- Zederbauer, T.; Andrews, A.M.; MacFarland, D.; Detz, H.; Schrenk, W.; Strasser, G. Enhanced Crystal Quality of AlxIn1−xAsySb1−y for Terahertz Quantum Cascade Lasers. Photonics 2016, 3, 20. [Google Scholar] [CrossRef]
- Campbell, J.C.; David, J.P.R.; Bank, S.R. Sb-Based Low-Noise Avalanche Photodiodes. Photonics 2023, 10, 715. [Google Scholar] [CrossRef]
- Kodati, S.H.; Lee, S.; Guo, B.; Jones, A.H.; Schwartz, M.; Winslow, M.; Pfiester, N.A.; Grein, C.H.; Ronningen, T.J.; Campbell, J.C.; et al. AlInAsSb avalanche photodiodes on InP substrates. Appl. Phys. Lett. 2021, 118, 091101. [Google Scholar] [CrossRef]
- Jones, A.H.; March, S.D.; Bank, S.R.; Campbell, J.C. Low-noise high-temperature AlInAsSb/GaSb avalanche photodiodes for 2-μm applications. Nat. Photonics 2020, 14, 559–563. [Google Scholar] [CrossRef]
- Jones, A.H.; March, S.D.; Dadey, A.A.; Muhowski, A.J.; Bank, S.R.; Campbell, J.C. AlInAsSb Separate Absorption, Charge, and Multiplication Avalanche Photodiodes for Mid-Infrared Detection. IEEE J. Quantum Electron. 2022, 58, 1–6. [Google Scholar] [CrossRef]
- Adam, A.; Dadey, J.; Andrew, M.A.; Abhilasha, K.; Seth, R.; Bank, D.W.; Joe, C.C. High-gain low-excess-noise MWIR detection with a 3.5-µm cutoff AlInAsSb-based separate absorption, charge, and multiplication avalanche photodiode. APL Photonics 2023, 8, 036101. [Google Scholar] [CrossRef]
- Maddox, S.J.; March, S.D.; Bank, S.R. Broadly Tunable AlInAsSb Digital Alloys Grown on GaSb. Cryst. Growth Des. 2016, 16, 3582–3586. [Google Scholar] [CrossRef]
- Chang, F.R.; Hao, R.T.; Qi, T.T.; Zhao, Q.C.; Liu, X.X.; Li, Y.; Gu, K.; Guo, J.; Wang, G.W.; Xu, Y.Q. High material quality growth of AlInAsSb thin films on GaSb substrate by molecular beam epitaxy. Chin. Phys. B 2019, 28, 445–449. [Google Scholar] [CrossRef]
- Ren, M.; Maddox, S.J.; Woodson, M.E.; Chen, Y.J.; Bank, S.R.; Campbell, J.C. Characteristics of AlxIn1−xAsySb1−y (x: 0.3–0.7) Avalanche Photodiodes. J. Light. Technol. 2017, 35, 2380–2384. [Google Scholar] [CrossRef]
- Min, R.; Scott, J.M.; Madison, E.W.; Chen, Y.j.; Seth, R.B.; Campbell, J.C. AlInAsSb separate absorption, charge, and multiplication avalanche photodiodes. Appl. Phys. Lett. 2016, 108, 191108. [Google Scholar] [CrossRef]
- Chang, Y.C.; Schulman, J.N. Interband optical transitions in GaAs-Ga1−xAlxAs and InAs-GaSb superlattices. Phys. Rev. B 1985, 31, 2069. [Google Scholar] [CrossRef]
- Nguyen, B.M.; Hoffman, D.; Delaunay, P.Y.; Razeghi, M. Dark current suppression in type II InAs/GaSb superlattice long wavelength infrared photodiodes with M-structure barrier. Appl. Phys. Lett. 2007, 91, 163511. [Google Scholar] [CrossRef]
- Wu, Y.Y.; Zhang, Y.H.; Zhang, Y.; Zhao, Y.H.; Zhang, Y.; Xu, Y.Q.; Liang, C.Y.; Niu, Z.C.; Shi, Y.; Che, R.C. Dual strategy of modulating growth temperature and inserting ultrathin barrier to enhance the wave function overlap in type-II superlattices. Nano Res. 2022, 15, 5626–5632. [Google Scholar] [CrossRef]
- Zhao, Y.H.; Liu, L.; Bi, H.; Han, X.; Zhao, X.B.; Ni, H.Q.; Xu, Y.Q.; Niu, Z.C.; Che, R.C. Quantum efficiency optimization by maximizing wave function overlap in type-II superlattice photodetectors. Nanoscale 2017, 9, 11833–11840. [Google Scholar] [CrossRef]
- Cai, C.Y.; Zhao, Y.H.; Chang, Y.R.; Zhao, X.B.; Yang, L.B.; Liang, C.Y.; Wang, G.W.; Niu, Z.C.; Shi, Y.; Liu, X.H.; et al. Understanding the role of interface in advanced semiconductor nanostructure and its interplay with wave function overlap. Nano Res. 2020, 13, 1536–1543. [Google Scholar] [CrossRef]
- Vurgaftman, I.; Meyer, J.R.; Ram-Mohan, L.R. Band parameters for IIIV compound semiconductors and their alloys. J. Appl. Phys. 2001, 89, 5815–5875. [Google Scholar] [CrossRef]
- Qiao, P.F.; Shin, M.; Shun, L.C. Electronic band structures and optical properties of type-II superlattice photodetectors with interfacial effect. Opt. Express 2012, 20, 2319–2334. [Google Scholar] [CrossRef]
- Xia, J.B.; Zhu, B.F. Semiconductor Superlattice Physics; Science and Technology Press: Shanghai, China, 1995; p. 388. [Google Scholar]
- Kroemer, H. The 6.1Å family (InAs, GaSb, AlSb) and its heterostructures: A selective review. Physica E 2004, 20, 196–203. [Google Scholar] [CrossRef]
- Zheng, J.; Andrew, H.J.; Tan, Y.H.; Rockwell, A.K.; Stephen, M.; Sheikh, Z.A.; Catherine, A.D.; Avik, W.G.; Seth, R.B.; Campbell, J.C. Characterization of band offsets in AlxIn1−xAsySb1−y alloys with varying Al composition. Appl. Phys. Lett. 2019, 115, 122105. [Google Scholar] [CrossRef]
- Scholle, K.; Lamrini, S.; Koopmann, P.; Peter, F. 2 µm laser sources and their possible applications. In Frontiers in Guided Wave Optics and Optoelectronics; IntechOpen: London, UK, 2010. [Google Scholar] [CrossRef]
- Webster, P.T.; Riordan, N.A.; Liu, S.; Steenbergen, E.H.; Synowicki, R.A.; Zhang, Y.H.; Johnson, S.R. Absorption properties of type-II InAs/InAsSb superlattices measured by spectroscopic ellipsometry. Appl. Phys. Lett. 2015, 106, 061907. [Google Scholar] [CrossRef]
- Bi, H.; Han, X.; Liu, L.; Zhao, Y.; Zhao, X.; Wang, G.; Xu, Y.; Niu, Z.; Shi, Y.; Che, R. Atomic Mechanism of Interfacial-Controlled Quantum Efficiency and Charge Migration in InAs/GaSb Superlattice. ACS Appl. Mater. Interfaces 2017, 9, 26642–26647. [Google Scholar] [CrossRef]


| Parameter | Symbol | InAs | AlSb | InSb | AlAs |
|---|---|---|---|---|---|
| Luttinger parameters | 19.4 | 4.15 | 32.4 | 3.76 | |
| 8.545 | 1.28 | 13.3 | 1.23 | ||
| 9.17 | 1.75 | 15.15 | 1.42 | ||
| Corrected Luttinger parameters | 6.3 | 1.76 | 16.78 | 0.737 | |
| 1.99 | 0.085 | 5.61 | 0.125 | ||
| 2.62 | 0.55 | 7.46 | 0.315 |
| Model | Position | InSb Thick | Position-i | InSb Thick |
|---|---|---|---|---|
| A | 0 | 0.6 MLs | 0.17 | 0.3 MLs |
| B | 0 | 1.1 MLs | 0.12 | 0.55 MLs |
| C | 0 | 1.7 MLs | 0.10 | 0.85 MLs |
| Model | Position | AlAs Thick | Position-i | AlAs Thick |
|---|---|---|---|---|
| A | 0 | 1 MLs | 0.3 | 0.5 MLs |
| B | 0 | 2.6 MLs | 0.28 | 1.3 MLs |
| C | 0 | 2.5 MLs | 0.26 | 1.25 MLs |
| Model | K | b | InSb/MLs | AlAs/MLs |
|---|---|---|---|---|
| A | 0.8967 | −0.3341 | 0.7 | 0.3 |
| B | 0.1285 | 0.7 | 0.8 | |
| C | 0.5654 | 1.5 | 1.9 |
| Materials/MLs | Data | Interface Layer | Thick/Å | WFO | QE | Improve |
|---|---|---|---|---|---|---|
| 8InAs/7.5AlSb [17] | Experiment | AlAs | 3.44 | Enhance | - | PL improved 50% |
| 35InAs/10AlSb [19] | Experiment | InAsSb | 1.51 | Enhance | - | Optical performance |
| 17InAs/15InAsSb [26] | Simulation | InAsSb | 6.03 | Enhance 652% | - | absorption coefficient improved 683% |
| InAs/GaSb [27] | Experiment | InSb | ~3 | Enhance | 23% to 50% | QE improved 117% |
| This paper 5InAs/5AlSb | Simulation | AlAs, InSb | 2.26, 2.27 | Enhance 114% | - | Optical performance |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, J.; Zhu, L.; Lu, L.; Chen, W.; Zheng, X.; Zhang, D.; Fu, Y.; Ou, J. Interface Engineering and Electron-Hole Wave Function Overlap of InAs/AlSb Superlattice Infrared Detectors. Photonics 2023, 10, 1268. https://doi.org/10.3390/photonics10111268
Yu J, Zhu L, Lu L, Chen W, Zheng X, Zhang D, Fu Y, Ou J. Interface Engineering and Electron-Hole Wave Function Overlap of InAs/AlSb Superlattice Infrared Detectors. Photonics. 2023; 10(11):1268. https://doi.org/10.3390/photonics10111268
Chicago/Turabian StyleYu, Jing, Lianqing Zhu, Lidan Lu, Weiqiang Chen, Xiantong Zheng, Dongliang Zhang, Yuegang Fu, and Jianzhen Ou. 2023. "Interface Engineering and Electron-Hole Wave Function Overlap of InAs/AlSb Superlattice Infrared Detectors" Photonics 10, no. 11: 1268. https://doi.org/10.3390/photonics10111268
APA StyleYu, J., Zhu, L., Lu, L., Chen, W., Zheng, X., Zhang, D., Fu, Y., & Ou, J. (2023). Interface Engineering and Electron-Hole Wave Function Overlap of InAs/AlSb Superlattice Infrared Detectors. Photonics, 10(11), 1268. https://doi.org/10.3390/photonics10111268






