Influence of High-Intensity Pumping on Gain Medium Temperature Increase and Laser Mode Tunability in a Hemispherical Short Cavity
Abstract
:Featured Application
Abstract
1. Introduction
2. Materials and Methods
2.1. Experiment
2.2. Theory
2.2.1. Quasi-Three-Level (Quasi-Four-Level) Laser Theory
2.2.2. Temperature Increase in the Gain Medium
2.2.3. Thermal Lens
3. Experimental Results and Discussions
4. Theoretical Results and Discussions
4.1. Temperature Increase in the Gain Medium
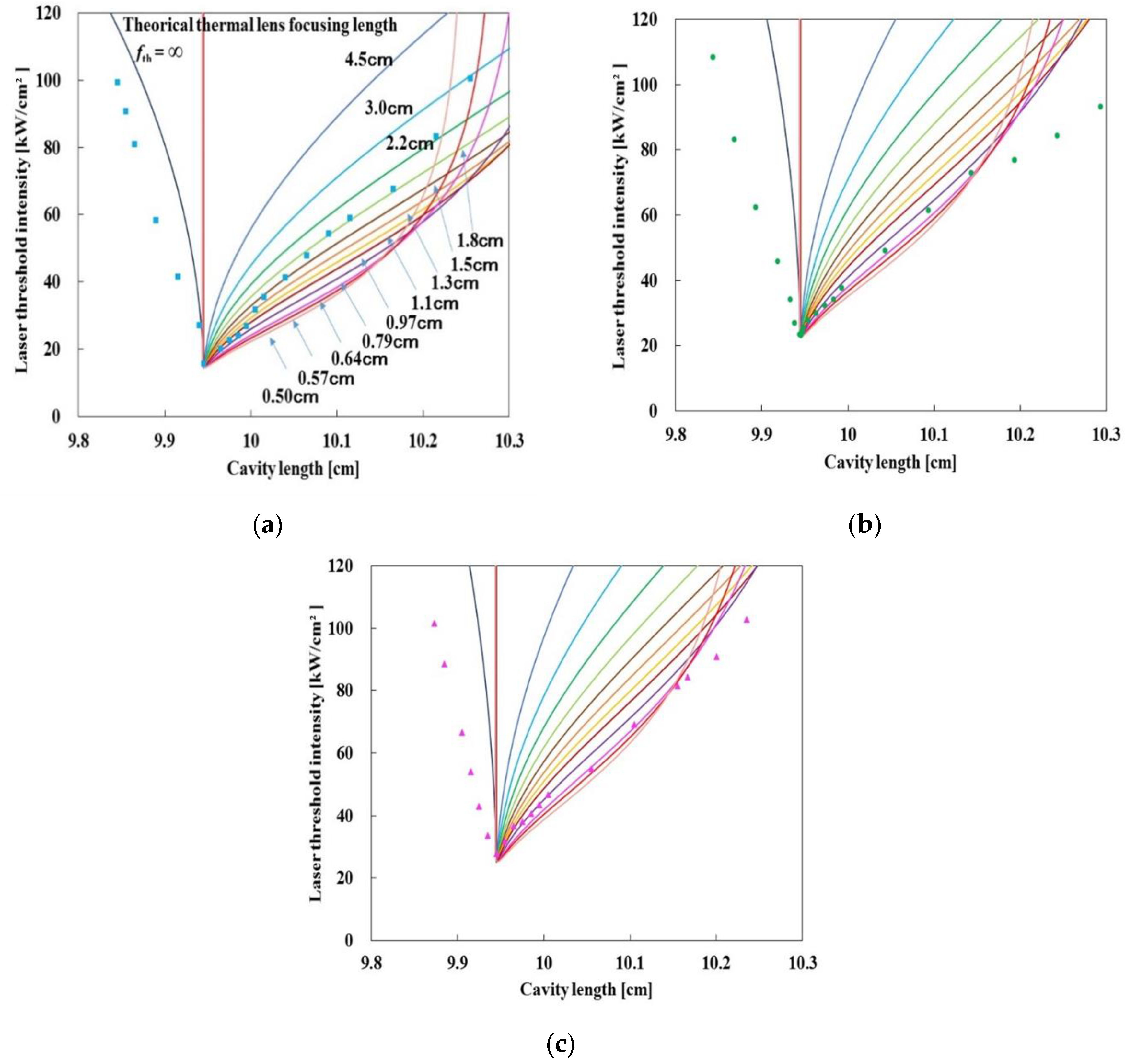
4.2. Dependence of Laser Threshold Intensity on Cavity Length
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Koechner, W. Section 3.4.2. Laser Output. In Solid State Laser Engineering, 6th ed.; Springer: Berlin, Germany, 2006; pp. 118–126. [Google Scholar]
- Yariv, A.; Yeh, P. Section 6.5. Optimum Output Coupling in Laser Oscillators. In Photonics, Optical Electronics in Mordern Communications, 6th ed.; Oxford University Press: New York, NY, USA, 2007; pp. 248–251. [Google Scholar]
- Lacovara, P.; Choi, H.K.; Wang, C.A.; Aggarwal, R.L.; Fan, T.Y. Room-temperature diode-pumped Yb:YAG laser. Opt. Lett. 1991, 16, 1089–1091. [Google Scholar] [CrossRef] [PubMed]
- Taira, T. Yb solid state lasers. Jpn. J. Opt. 1999, 28, 435–442. [Google Scholar]
- Pavel, N.; Saikawa, J.; Taira, T. Radial-Pumped Microchip High-Power Composite Yb:YAG laser: Design and Power Charac-teristics. Jpn. J. Appl. Phys. 2001, 40, 146–152. [Google Scholar] [CrossRef]
- Fan, T.Y. Diode-pumped solid-state lasers. In Laser Sources and Applications; Miller, A., Finlayson, D.M., Eds.; CRC Press: Boca Raton, FL, USA, 2020; pp. 153–193. [Google Scholar]
- White, J.O. Parameters for Quantitative Comparison of Two-, Three-, and Four-Level Laser Media, Operating Wavelengths, and Temperatures. IEEE J. Quantum Electron. 2009, 45, 1213–1220. [Google Scholar] [CrossRef]
- Giesen, A.; Hügel, H.; Voss, A.; Wittig, K.; Brauch, U.; Opower, H. Scalable concept for Diode-Pumped High-Power Solid-state Lasers. Appl. Phys. B 1994, 58, 365–372. [Google Scholar] [CrossRef]
- Peters, R.; Kränkel, C.; Petermann, K.; Huber, G. Broadly tunable high-power Yb:Lu2O3 thin disk laser with 80% slope efficiency. Opt. Express 2007, 15, 7075–7082. [Google Scholar] [CrossRef] [PubMed]
- Jeong, Y.; Sahu, J.K.; Payne, D.N.; Nilsson, J. Ytterbium-doped large-core fiber laser with 1.36 kW continuous-wave output power. Opt. Express 2004, 12, 6088–6092. [Google Scholar] [CrossRef]
- Shoji, T.; Tokita, S.; Kawanaka, J.; Fujita, M.; Izawa, Y. Quantum-defect-limited operation of diode-pumped Yb:YAG laser at low temperature. Jpn. J. Appl. Phys. 2004, 43, L496–L498. [Google Scholar] [CrossRef]
- Ripin, D.J.; Ochoa, J.R.; Aggarwal, R.L.; Fan, T.Y. 165-W cryogenically cooled Yb:YAG laser. Opt. Lett. 2004, 29, 2154–2156. [Google Scholar] [CrossRef]
- Matsubara, S.; Ueda, T.; Kawato, S.; Kobayashi, T. Highly Efficient Continuous-Wave Laser Oscillation in Microchip Yb:YAG Laser at Room Temperature. Jpn. J. Appl. Phys. 2007, 46, L132–L134. [Google Scholar] [CrossRef]
- Takama, M.; Matsubara, S.; Ueda, T.; Inoue, M.; Tanaka, M.; Otani, K.; Kawato, S.; Kobayashi, T. Highly efficient nanosecond-pulse Yb:YAG laser. Smart Process. Technol. 2007, 2, 281–283. [Google Scholar]
- Kawato, S.; Kadoya, H.; Sugiki, F.; Kobayashi, R.; Kanetake, T.; Nakajima, N.; Kataoka, S. Laser efficiency improvement on hemispherical short cavity lasers by high intensity pumping. In Proceedings of the EMN Angkor Meeting & CC Physical Chemistry, Ankor, Cambodia, 12–13 March 2018; p. A14. [Google Scholar]
- Takano, T.; Ogawa, H.; Ohae, C.; Katsuragawa, M. 10 W injection-locked single-frequency continuous-wave titanium:sapphire laser. Opt. Express 2021, 29, 6927–6934. [Google Scholar] [CrossRef] [PubMed]
- Kawato, S.; Kawashima, T. High-Efficiency Continuous-Wave Ti:Sapphire Laser with High-Intensity Pumping Using a Commercially Available Crystal. Appl. Sci. 2022, 12, 4815. [Google Scholar] [CrossRef]
- Liu, J.; Griebner, U.; Petrov, V.; Zhang, H.; Zhang, J.; Wang, J. Efficient continuous-wave and Q-switched operation of a diode-pumped Yb:KLu(WO4)2Yb:KLu(WO4)2 laser with self-Raman conversion. Opt. Lett. 2005, 30, 2427–2429. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Wan, Y.; Zhou, Z.; Tian, X.; Han, W.; Zhang, H. Comparative study on the laser perfor-mance of two Yb-doped disordered garnet crystals YbCNGG and YbCLNGG. Appl. Phys. B 2012, 109, 183–188. [Google Scholar] [CrossRef]
- Han, W.; Wu, K.; Tian, X.; Xia, L.; Zhang, H.; Liu, J. Laser performance of ytterbium-doped gallium garnets: Yb:Re_3Ga_5O_12 (Re = Y, Gd, Lu). Opt. Mater. Express 2013, 3, 920–927. [Google Scholar] [CrossRef]
- Dong, J.; Shirakawa, A.; Ueda, K.; Kaminskii, A. Effect of ytterbium concentration on cw Yb:YAG microchip laser performance at ambient temperature—Part I: Experiments. Appl. Phys. B 2007, 89, 359–365. [Google Scholar] [CrossRef]
- Serres, J.M.; Jambunathan, V.; Loiko, P.; Mateos, X.; Yu, H.; Zhang, H.; Liu, J.; Lucianetti, A.; Mocek, T.; Yumashev, K.; et al. Microchip laser operation of Yb-doped gallium garnets. Opt. Mater. Express 2016, 6, 46–57. [Google Scholar] [CrossRef]
- Loiko, P.; Serres, J.M.; Mateos, X.; Xu, X.; Xu, J.; Jambunathan, V.; Navratil, P.; Lucianetti, A.; Mocek, T.; Zhang, X.; et al. Microchip Yb:CaLnAlO_4 lasers with up to 91% slope efficiency. Opt. Lett. 2017, 42, 2431–2434. [Google Scholar] [CrossRef]
- Stolzenburg, C.; Voss, A.; Graf, T.; Larionov, M.; Giesen, A. Advanced pulsed thin disk laser sources. Proc. SPIE 2008, 6871, 68710H. [Google Scholar] [CrossRef]
- Koechner, W. Thermal Lensing in a Nd:YAG Laser Rod. Appl. Opt. 1970, 9, 2548–2553. [Google Scholar] [CrossRef] [PubMed]
- Foster, J.D.; Osterink, L.M. Thermal effects in a Nd:YAG Laser. J. Appl. Phys. 1970, 41, 3656–3663. [Google Scholar] [CrossRef]
- Innocenzi, M.E.; Yura, H.T.; Fincher, C.L.; Fields, R.A. Thermal modeling of continuous-wave end-pumped solid-state lasers. Appl. Phys. Lett. 1990, 56, 1831–1833. [Google Scholar] [CrossRef]
- Song, F.; Zhang, C.; Ding, X.; Xu, J.; Zhang, G.; Leigh, M.; Peyghambarian, N. Determination of thermal focal length and pumping radius in gain medium in laser-diode-pumped Nd:YVO4 lasers. Appl. Phys. Lett. 2002, 81, 2145–2147. [Google Scholar] [CrossRef]
- Chenais, S.; Balembois, F.; Druon, F.; Lucas-Leclin, G.; Georges, P. Thermal lensing in diode-pumped ytterbium Lasers—Part I: Theoretical analysis and wavefront measurements. IEEE J. Quantum Electron. 2004, 40, 1217–1234. [Google Scholar] [CrossRef]
- Chenais, S.; Balembois, F.; Druon, F.; Lucas-Leclin, G.; Georges, P. Thermal lensing in diode-pumped ytterbium Lasers—Part II: Evaluation of quantum efficiencies and thermo-optic coefficients. IEEE J. Quantum Electron. 2004, 40, 1235–1243. [Google Scholar] [CrossRef]
- Fan, S.; Zhang, X.; Wang, Q.; Li, S.; Ding, S.; Su, F. More precise determination of thermal lens focal length for end-pumped solid-state lasers. Opt. Commun. 2006, 266, 620–626. [Google Scholar] [CrossRef]
- Chénais, S.; Druon, F.; Forget, S.; Balembois, F.; Georges, P. On thermal effects in solid-state lasers: The case of ytterbium-doped materials. Prog. Quantum Electron. 2006, 30, 89–126. [Google Scholar] [CrossRef]
- Wang, S.; Eichler, H.J.; Wang, X.; Kallmeyer, F.; Ge, J.; Riesbeck, T.; Chen, J. Diode end pumped Nd:YAG laser at 946 nm with high pulse energy limited by thermal lensing. Appl. Phys. 2009, B95, 721. [Google Scholar] [CrossRef]
- Shang, J.; Zhu, X.; Zhu, G. Analytical approach to thermal lensing in end-pumped Yb:YAG thin-disk laser. Appl. Opt. 2011, 50, 6103–6120. [Google Scholar] [CrossRef]
- Sato, Y.; Taira, T. Highly accurate interferometric evaluation of thermal expansion and dn/dT of optical materials. Opt. Mater. Express 2014, 4, 876–888. [Google Scholar] [CrossRef]
- Shoji, I.; Taira, T. Intrinsic reduction of the depolarization loss in solid-state lasers by use of a (110)-cut crystal. Appl. Phys. Lett. 2002, 80, 3048–3050. [Google Scholar] [CrossRef]
- Shoji, I.; Sato, Y.; Kurimura, S.; Lupei, V.; Taira, T.; Ikesue, A.; Yoshida, K. Thermal-birefringence-induced depolarization in Nd:YAG ceramics. Opt. Lett. 2002, 27, 234–236. [Google Scholar] [CrossRef] [PubMed]
- Kubodera, K.; Otsuka, K. Single-transverse-mode LiNdP4O12 slab waveguide laser. J. Appl. Phys. 1979, 50, 653–659. [Google Scholar] [CrossRef]
- Fan, T.Y.; Byer, R.L. Medeling and CW Operation of a Quasi-Three-Level 946 nm Nd:YAG Laser. IEEE J. Quantum. Electron. 1987, 23, 605–612. [Google Scholar] [CrossRef]
- Risk, W.P. Modeling of longitudinally pumped solid-state lasers exhibiting reabsorption losses. J. Opt. Soc. Am. 1988, B5, 1412–1423. [Google Scholar] [CrossRef]
- Taira, T.; Tuloch, M.W.; Byer, L.R. Modeling of quasi-three-level lases and operation of cw Yb:YAG lasers. Appl. Opt. 1997, 20, 1867–1874. [Google Scholar] [CrossRef]
- Kawato, S.; Sueda, K.; Kobayashi, T. Development of High-Power Micro-Thickness-Slab Yb: YAG Lasers. Rev. Laser Eng. 2005, 33, 236–242. [Google Scholar] [CrossRef]
- Kawato, S.; Kobayashi, T. Design of End-Pumped Thin Rod Yb:YAG Laser Amplifiers. Jpn. J. Appl. Phys. 2003, 42, 2705–2710. [Google Scholar] [CrossRef]
- Sumida, S.D.; Fan, Y.T. Effect of radiation trapping on fluorescence lifetime and emission cross section measurements in solid-state laser media. Opt. Lett. 1994, 19, 1343–1345. [Google Scholar] [CrossRef]
- Krupke, F.W. Ytteribium Solid-State Lasers—The First Decate. IEEE J. Quantum. Electron. 2000, 6, 1287–1296. [Google Scholar] [CrossRef]
- Koechner, W. Section 2.6. Yb:YAG. In Solid State Laser Engineering, 6th ed.; Springer: Berlin, Germany, 2006; pp. 97–101. [Google Scholar]
- Findlay, D.; Clay, R.A. The Measument of internal losses in 4-level lasers. Phys. Lett. 1966, 20, 277–278. [Google Scholar] [CrossRef]
- Caird, J.A.; Payne, S.A.; Staver, P.R.; Ramponi, A.J.; Chase, L.L. Quantum electronic properties of the Na3Ga2Li3F12:Cr3+ laser. IEEE J. Quantum. Electron. 1988, 24, 1077–1099. [Google Scholar] [CrossRef]
- Oezisik, M.N. Heat Conduction, 2nd ed.; John Wiley & Sons Inc.: New York, NY, USA, 1993; pp. 18–24. [Google Scholar]
- Sueda, K.; Takahashi, H.; Kawato, S.; Kobayashi, T. High-efficiency laser-diodes-pumped microthickness Yb:Y3Al5O12 slab laser. Appl. Phys. Lett. 2005, 87, 151110. [Google Scholar] [CrossRef]
- Russbueldt, P.; Mans, T.; Rotarius, G.; Weitenberg, J.; Hoffmann, H.D.; Poprawe, R. 400W Yb:YAG Innoslab fs-Amplifier. Opt. Express 2009, 17, 12230–12245. [Google Scholar] [CrossRef]
- Koechner, W. Sections 5.1.3. Resonator Configurations. 5.1.4. Stability of Laser Resonators. In Solid State Laser Engineering, 6th ed.; Springer: Berlin, Germany, 2006; pp. 217–222. [Google Scholar]
- Yariv, A.; Yeh, P. Section 4.4. Mode Stability Criteria. In Photonics, Optical Electronics in Mordern Communications, 6th ed.; Oxford University Press: New York, NY, USA, 2007; pp. 176–177. [Google Scholar]
- Brauch, U.; Röcker, C.; Graf, T.; Ahmed, M.A. High-power, high-brightness solid-state laser architectures and their characteristics. Appl. Phys. B 2022, 128, 58. [Google Scholar] [CrossRef]
- Shang, P.; Bai, L.; Wang, S.; Cai, D.; Li, B. Research progress on thermal effect of LD pumped solid state laser. Opt. Laser Technol. 2023, 157, 108640. [Google Scholar] [CrossRef]
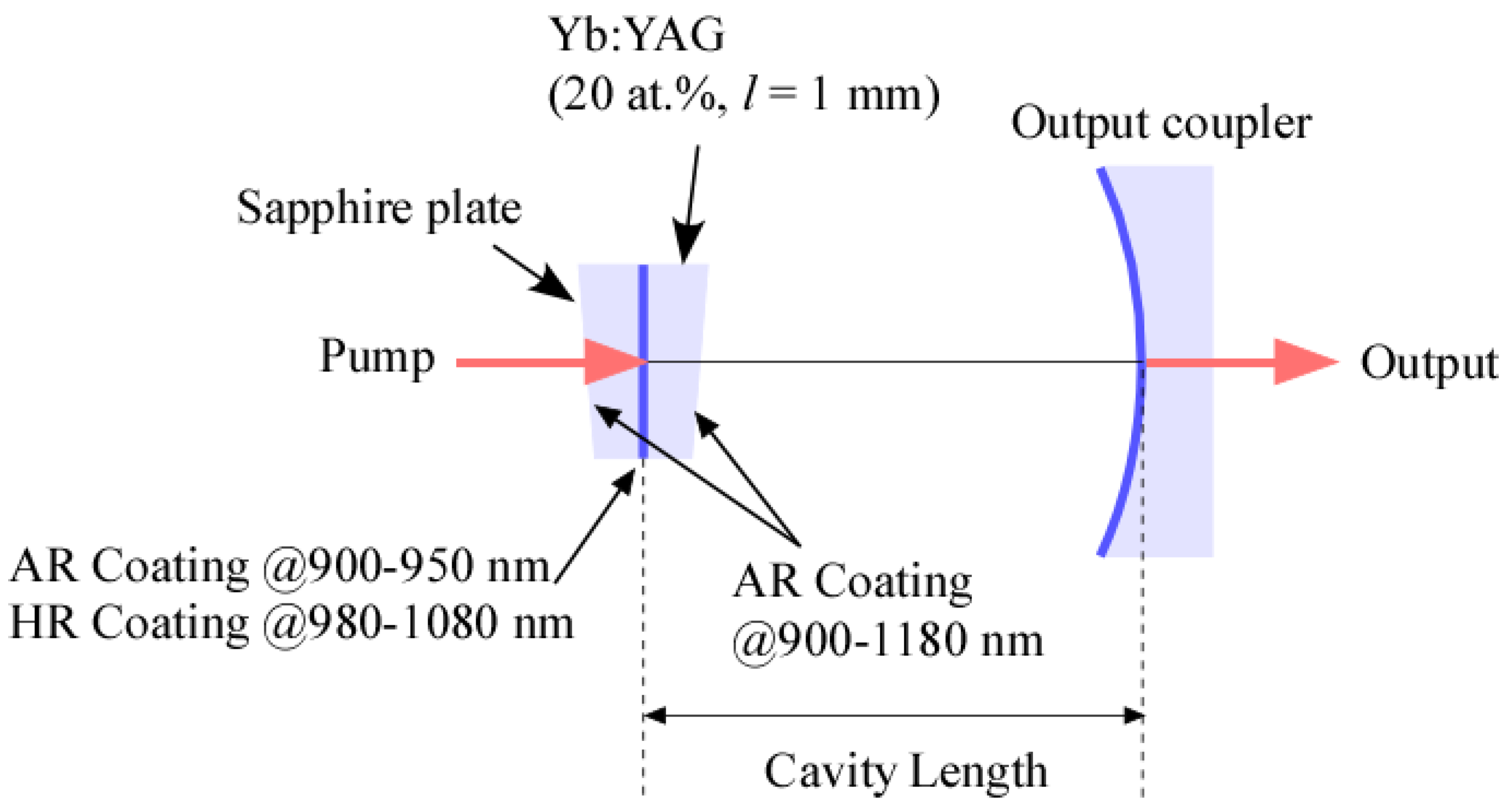



| Gain Medium | Type | Operation | Optical-to-Optical Conversion Efficiency for the Incident Pump Power (%) | Author (Year) |
|---|---|---|---|---|
| Yb:Lu2O3 | Thin disk | CW | Peters et al. (2007) [9] | |
| Yb-glass | Fiber | CW | Jong et al. (2004) [10] | |
| Yb:YAG | Cryogenically cooled | CW | Shoji et al. (2004) [11] | |
| Yb:YAG | Cryogenically cooled | CW | Ripin et at. (2004) [11] | |
| Yb:YAG | High-intensity pumping | CW | Matsubara et al. (2007) [13] | |
| Yb:KLu(W4)2 | Short cavity | CW | Liu et al. (2005) [18] | |
| Yb:CNGG | Short cavity | CW | Liu et al. (2012) [19] | |
| Yb:CLNGG | Short cavity | CW | Liu et al. (2012) [19] | |
| Yb:YGG | Short cavity | CW | Han et al. (2013) [20] | |
| Yb:LuGG | Short cavity | CW | Han et al. (2013) [20] | |
| Yb:GGG | Short cavity | CW | Han et al. (2013) [20] | |
| Yb:YAG | Microchip | CW | Dong et al. (2007) [21] | |
| Yb:LuGG | Microchip | CW | Serres et al. (2016) [22] | |
| Yb:YGG | Microchip | CW | Serres et al. (2016) [22] | |
| Yb:CALGO | Microchip | CW | Lokio et al. (2017) [23] | |
| Yb:CALYO | Microchip | CW | Lokio et al. (2017) [23] | |
| Yb:YAG | Short-cavity, High-intensity pumping | CW | Kawato et al. (2018) [15] | |
| Yb:YAG | Thin disk | Cavity dumped | Stolzenburg et al. (2008) [24] | |
| Yb:YAG | High-intensity pumping | Cavity dumped | Takama et al. (2007) [14] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kawato, S. Influence of High-Intensity Pumping on Gain Medium Temperature Increase and Laser Mode Tunability in a Hemispherical Short Cavity. Photonics 2023, 10, 1239. https://doi.org/10.3390/photonics10111239
Kawato S. Influence of High-Intensity Pumping on Gain Medium Temperature Increase and Laser Mode Tunability in a Hemispherical Short Cavity. Photonics. 2023; 10(11):1239. https://doi.org/10.3390/photonics10111239
Chicago/Turabian StyleKawato, Sakae. 2023. "Influence of High-Intensity Pumping on Gain Medium Temperature Increase and Laser Mode Tunability in a Hemispherical Short Cavity" Photonics 10, no. 11: 1239. https://doi.org/10.3390/photonics10111239
APA StyleKawato, S. (2023). Influence of High-Intensity Pumping on Gain Medium Temperature Increase and Laser Mode Tunability in a Hemispherical Short Cavity. Photonics, 10(11), 1239. https://doi.org/10.3390/photonics10111239





