Determining the Optical Geometry of a Gold Semi-Shell under the Kretschmann Configuration
Abstract
1. Introduction
2. Materials and Methods
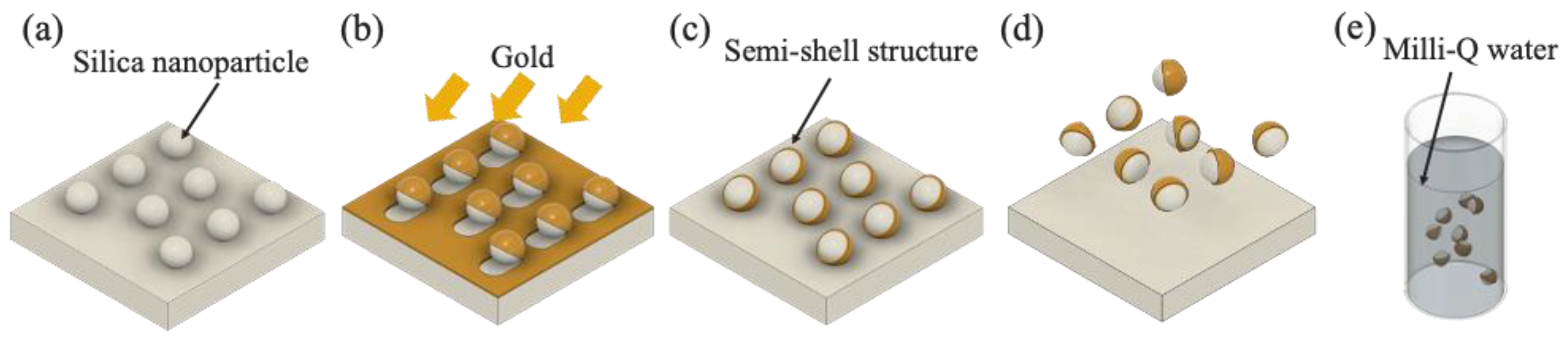
2.1. Gold Semi-Shell Dispersion Liquid Fabrication
2.2. Experimental Setup
2.3. Numerical Calculation
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Correction Statement
References
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Genet, C.; Ebbesen, T.W. Light in Tiny Holes. Nature 2007, 445, 39–46. [Google Scholar] [CrossRef] [PubMed]
- Shalaev, V.M. Optical Cloaking with Metamaterials. Nat. Photonics 2007, 1, 41–48. [Google Scholar] [CrossRef]
- Dolling, G.; Enkrich, C.; Wegener, M.; Zhou, J.F.; Soukoulis, C.M.; Linden, S. Cut-Wire Pairs and Plate Pairs as Magnetic Atoms for Optical Metamaterials. Opt. Lett. 2005, 30, 3198–3200. [Google Scholar] [CrossRef]
- Dolling, G.; Wegener, M.; Soukoulis, C.M.; Linden, S. Negative-Index Metamaterial at 780 Nm Wavelength. Opt. Lett. 2007, 32, 53–55. [Google Scholar] [CrossRef] [PubMed]
- García-Meca, C.; Ortuño, R.; Rodríguez-Fortuño, F.J.; Martí, J.; Martínez, A. Double-Negative Polarization-Independent Fishnet Metamaterial in the Visible Spectrum. Opt. Lett. 2009, 34, 1603–1605. [Google Scholar] [CrossRef]
- Garoli, D.; Natali, M.; Parisi, G.; Ongarello, T.; Sovernigo, E.; Massari, M.; Giorgis, V.; Ruffato, G.; De Zuani, S.; Romanato, F. Fabrication of Metamaterials in the Optical Spectral Range. Microelectron. Eng. 2011, 88, 1951–1954. [Google Scholar] [CrossRef]
- Vala, M.; Ertsgaard, C.T.; Wittenberg, N.J.; Oh, S.-H. Plasmonic Sensing on Symmetric Nanohole Arrays Supporting High-Q Hybrid Modes and Reflection Geometry. ACS Sens. 2019, 4, 3265–3274. [Google Scholar] [CrossRef]
- Zhang, Y.; Grady, N.K.; Ayala-Orozco, C.; Halas, N.J. Three-Dimensional Nanostructures as Highly Efficient Generators of Second Harmonic Light. Nano Lett. 2011, 11, 5519–5523. [Google Scholar] [CrossRef]
- Belardini, A.; Larciprete, M.C.; Centini, M.; Fazio, E.; Sibilia, C.; Bertolotti, M.; Toma, A.; Chiappe, D.; Buatier de Mongeot, F. Tailored Second Harmonic Generation from Self-Organized Metal Nano-Wires Arrays. Opt. Express 2009, 17, 3603–3609. [Google Scholar] [CrossRef]
- Chen, X.; Jia, B.; Saha, J.K.; Cai, B.; Stokes, N.; Qiao, Q.; Wang, Y.; Shi, Z.; Gu, M. Broadband Enhancement in Thin-Film Amorphous Silicon Solar Cells Enabled by Nucleated Silver Nanoparticles. Nano Lett. 2012, 12, 2187–2192. [Google Scholar] [CrossRef]
- Liu, W.; Wang, X.; Li, Y.; Geng, Z.; Yang, F.; Li, J. Surface Plasmon Enhanced GaAs Thin Film Solar Cells. Sol. Energy Mater. Sol. Cells 2011, 95, 693–698. [Google Scholar] [CrossRef]
- Aharon, H.; Shavit, O.; Galanty, M.; Salomon, A. Second Harmonic Generation for Moisture Monitoring in Dimethoxyethane at a Gold-Solvent Interface Using Plasmonic Structures. Nanomaterials 2019, 9, 1788. [Google Scholar] [CrossRef] [PubMed]
- Balaur, E.; O’ Toole, S.; Spurling, A.J.; Mann, G.B.; Yeo, B.; Harvey, K.; Sadatnajafi, C.; Hanssen, E.; Orian, J.; Nugent, K.A.; et al. Colorimetric Histology Using Plasmonically Active Microscope Slides. Nature 2021, 598, 65–71. [Google Scholar] [CrossRef] [PubMed]
- Segal, E.; Haleva, E.; Salomon, A. Ultrasensitive Plasmonic Sensor for Detecting Sub-PPB Levels of Alachlor. ACS Appl. Nano Mater. 2019, 2, 1285–1293. [Google Scholar] [CrossRef]
- Efremushkin, L.; Sukharev, M.; Salomon, A. Molecular Plasmonics: Strong Coupling at the Low Molecular Density Limit. J. Phys. Chem. C 2017, 121, 14819–14825. [Google Scholar] [CrossRef]
- Cheng, P.; Bao, J.; Zhao, H.; Wu, L. Light Emission via Surface Plasmon Modes in Metal Semi-Shell Cavity Shaped Antennas and Film Coupled Structure. Optik 2018, 152, 100–105. [Google Scholar] [CrossRef]
- Mirin, N.A.; Ali, T.A.; Nordlander, P.; Halas, N.J. Perforated Semishells: Far-Field Directional Control and Optical Frequency Magnetic Response. ACS Nano 2010, 4, 2701–2712. [Google Scholar] [CrossRef]
- Charnay, C.; Lee, A.; Man, S.-Q.; Moran, C.E.; Radloff, C.; Bradley, R.K.; Halas, N.J. Reduced Symmetry Metallodielectric Nanoparticles: Chemical Synthesis and Plasmonic Properties. J. Phys. Chem. B 2003, 107, 7327–7333. [Google Scholar] [CrossRef]
- Okamoto, T.; Fukuta, T.; Sato, S.; Haraguchi, M.; Fukui, M. Visible Near-Infrared Light Scattering of Single Silver Split-Ring Structure Made by Nanosphere Lithography. Opt. Express 2011, 19, 7068–7076. [Google Scholar] [CrossRef]
- Watanabe, T.; Okamoto, T.; Yamaguchi, K.; Haraguchi, M. Optical Geometry Dependent Scattering Analysis of Gold Semi-Shell Structures Using Propagating Surface Plasmon Polariton. In Plasmonics: Design, Materials, Fabrication, Characterization, and Applications XX; Lu, Y.-J., Tanaka, T., Tsai, D.P., Eds.; SPIE: Bellingham, WA, USA, 3 October 2022. [Google Scholar]
- Pandey, P.S.; Raghuwanshi, S.K.; Shadab, A.; Ansari, M.T.I.; Tiwari, U.K.; Kumar, S. SPR Based Biosensing Chip for COVID-19 Diagnosis—A Review. IEEE Sens. J. 2022, 22, 13800–13810. [Google Scholar] [CrossRef]
- Zhang, S.; Han, B.; Zhang, Y.-N.; Liu, Y.; Zheng, W.; Zhao, Y. Multichannel Fiber Optic SPR Sensors: Realization Methods, Application Status, and Future Prospects. Laser Photon. Rev. 2022, 16, 2200009. [Google Scholar] [CrossRef]
- Singh, P. SPR Biosensors: Historical Perspectives and Current Challenges. Sens. Actuators B Chem. 2016, 229, 110–130. [Google Scholar] [CrossRef]
- Singh, S.; Singh, P.K.; Umar, A.; Lohia, P.; Albargi, H.; Castañeda, L.; Dwivedi, D.K. 2D Nanomaterial-Based Surface Plasmon Resonance Sensors for Biosensing Applications. Micromachines 2020, 11, 779. [Google Scholar] [CrossRef] [PubMed]
- Kretschmann, E.; Raether, H. Notizen: Radiative Decay of Non Radiative Surface Plasmons Excited by Light. Z. Naturforsch. A Phys. Sci. 1968, 23, 2135–2136. [Google Scholar] [CrossRef]
- Xiong, Y.; Shepherd, S.; Tibbs, J.; Bacon, A.; Liu, W.; Akin, L.D.; Ayupova, T.; Bhaskar, S.; Cunningham, B.T. Photonic Crystal Enhanced Fluorescence: A Review on Design Strategies and Applications. Micromachines 2023, 14, 668. [Google Scholar] [CrossRef]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface Plasmon Subwavelength Optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef]
- Krishnan, A.; Thio, T.; Kim, T.J.; Lezec, H.J.; Ebbesen, T.W.; Wolff, P.A.; Pendry, J.; Martin-Moreno, L.; Garcia-Vidal, F.J. Evanescently Coupled Resonance in Surface Plasmon Enhanced Transmission. Opt. Commun. 2001, 200, 1–7. [Google Scholar] [CrossRef]
- Ariga, K.; Lvov, Y.; Kunitake, T. Assembling Alternate Dye Polyion Molecular Films by Electrostatic Layer-by-Layer Adsorption. J. Am. Chem. Soc. 1997, 119, 2224–2231. [Google Scholar] [CrossRef]
- Kubo, W.; Fujikawa, S. Au Double Nanopillars with Nanogap for Plasmonic Sensor. Nano Lett. 2011, 11, 8–15. [Google Scholar] [CrossRef]
- Johnson, P.B.; Christy, R.W. Optical Constants of the Noble Metals. Phys. Rev. B Condens. Matter 1972, 6, 4370–4379. [Google Scholar] [CrossRef]
- Al-Kuhaili, M.F. Enhancement of Plasmonic Transmittance of Porous Gold Thin Films via Gold/Metal Oxide Bi-Layers for Solar Energy-Saving Applications. Solar Energy 2019, 181, 456–463. [Google Scholar] [CrossRef]
- Shen, P.-T.; Sivan, Y.; Lin, C.-W.; Liu, H.-L.; Chang, C.-W.; Chu, S.-W. Temperature- and Roughness- Dependent Permittivity of Annealed/Unannealed Gold Films. Opt. Express 2016, 24, 19254–19263. [Google Scholar] [CrossRef] [PubMed]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Watanabe, T.; Okamoto, T.; Yamaguchi, K.; Haraguchi, M. Determining the Optical Geometry of a Gold Semi-Shell under the Kretschmann Configuration. Photonics 2023, 10, 1228. https://doi.org/10.3390/photonics10111228
Watanabe T, Okamoto T, Yamaguchi K, Haraguchi M. Determining the Optical Geometry of a Gold Semi-Shell under the Kretschmann Configuration. Photonics. 2023; 10(11):1228. https://doi.org/10.3390/photonics10111228
Chicago/Turabian StyleWatanabe, Tomoki, Toshihiro Okamoto, Kenzo Yamaguchi, and Masanobu Haraguchi. 2023. "Determining the Optical Geometry of a Gold Semi-Shell under the Kretschmann Configuration" Photonics 10, no. 11: 1228. https://doi.org/10.3390/photonics10111228
APA StyleWatanabe, T., Okamoto, T., Yamaguchi, K., & Haraguchi, M. (2023). Determining the Optical Geometry of a Gold Semi-Shell under the Kretschmann Configuration. Photonics, 10(11), 1228. https://doi.org/10.3390/photonics10111228




