A Review of Progress about Birefringent Filter Design and Application in Ti:sapphire Laser
Abstract
:1. Introduction
2. Research Progress of BRF Design
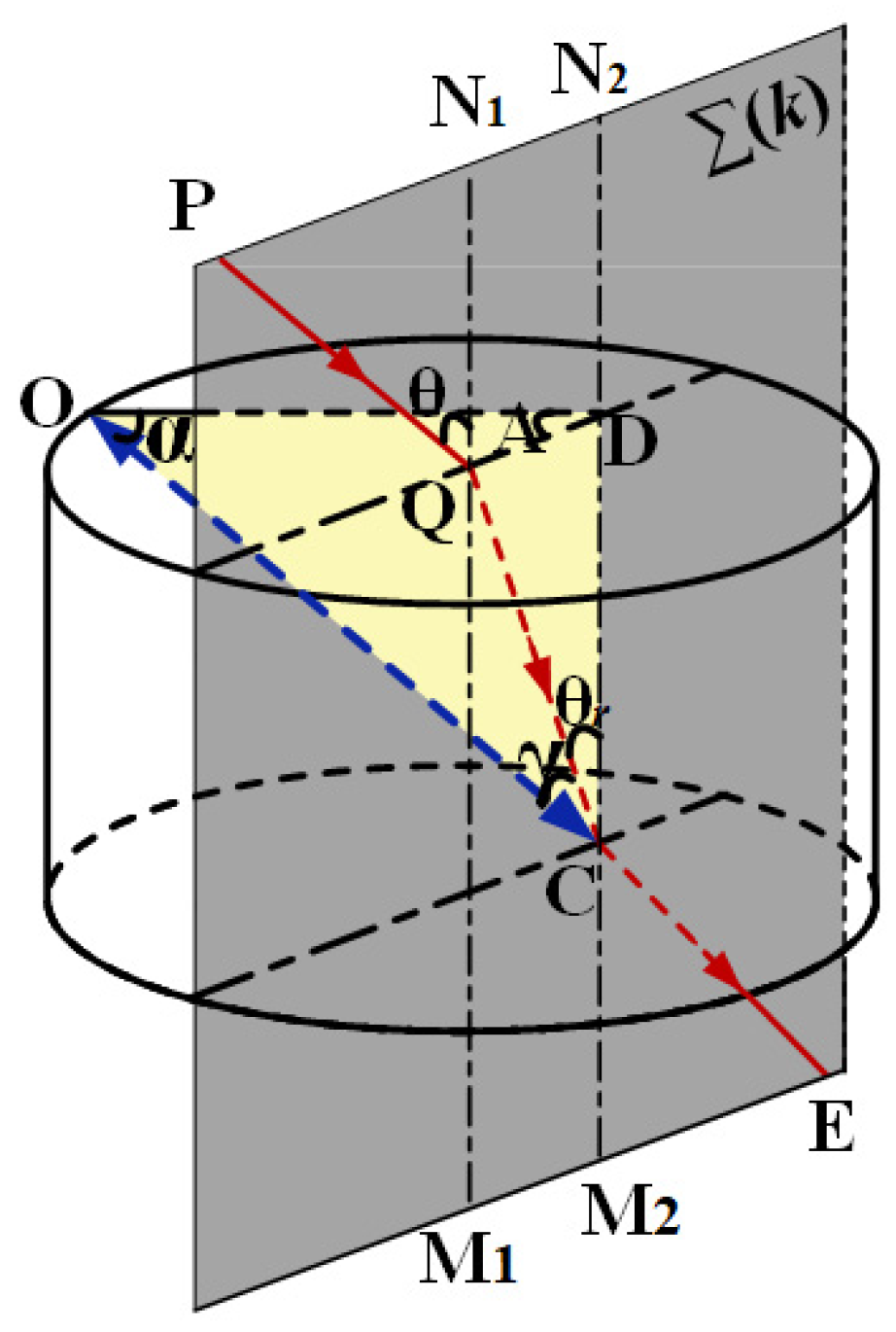
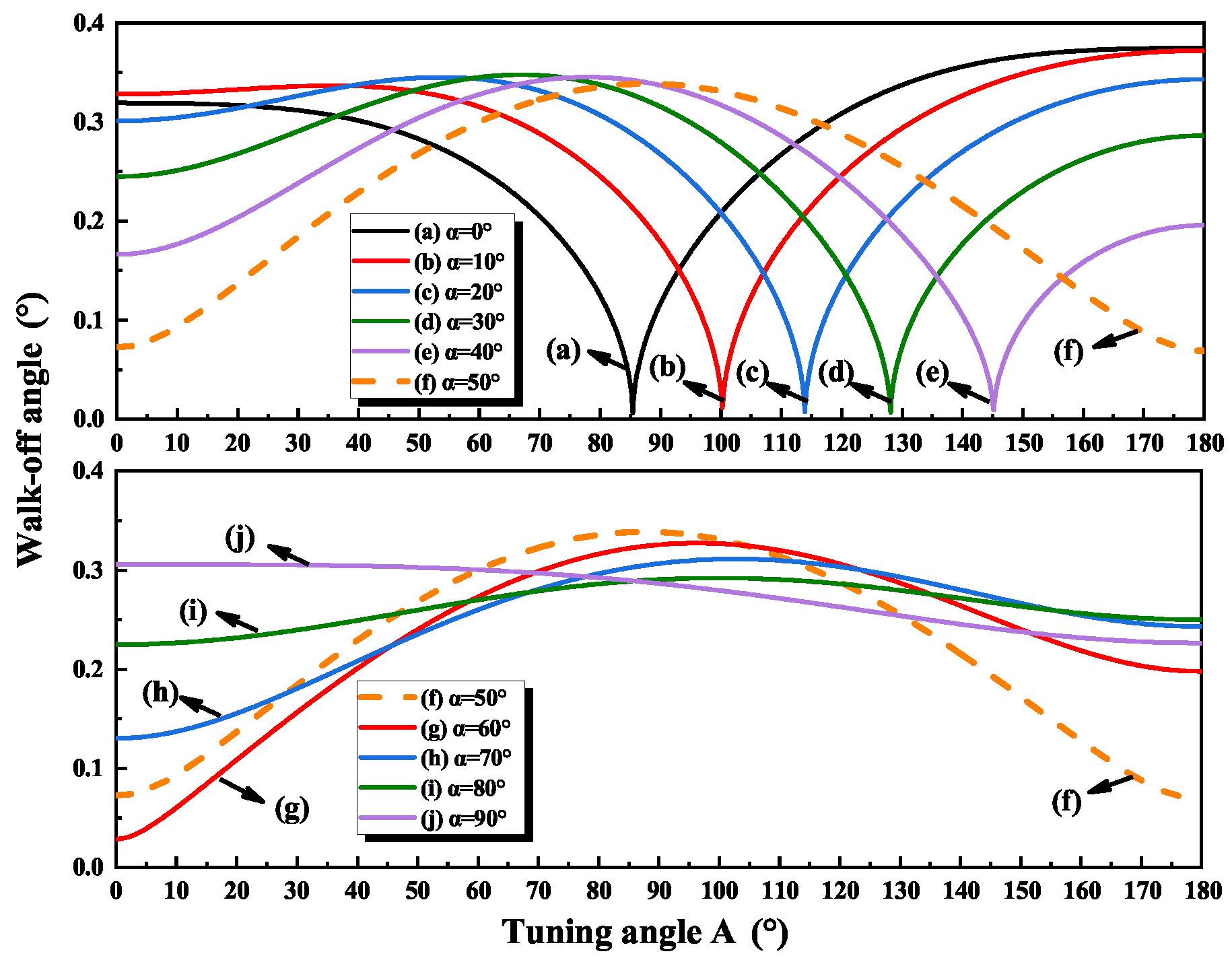
2.1. Tuning Characteristics of Single-Plate BRF
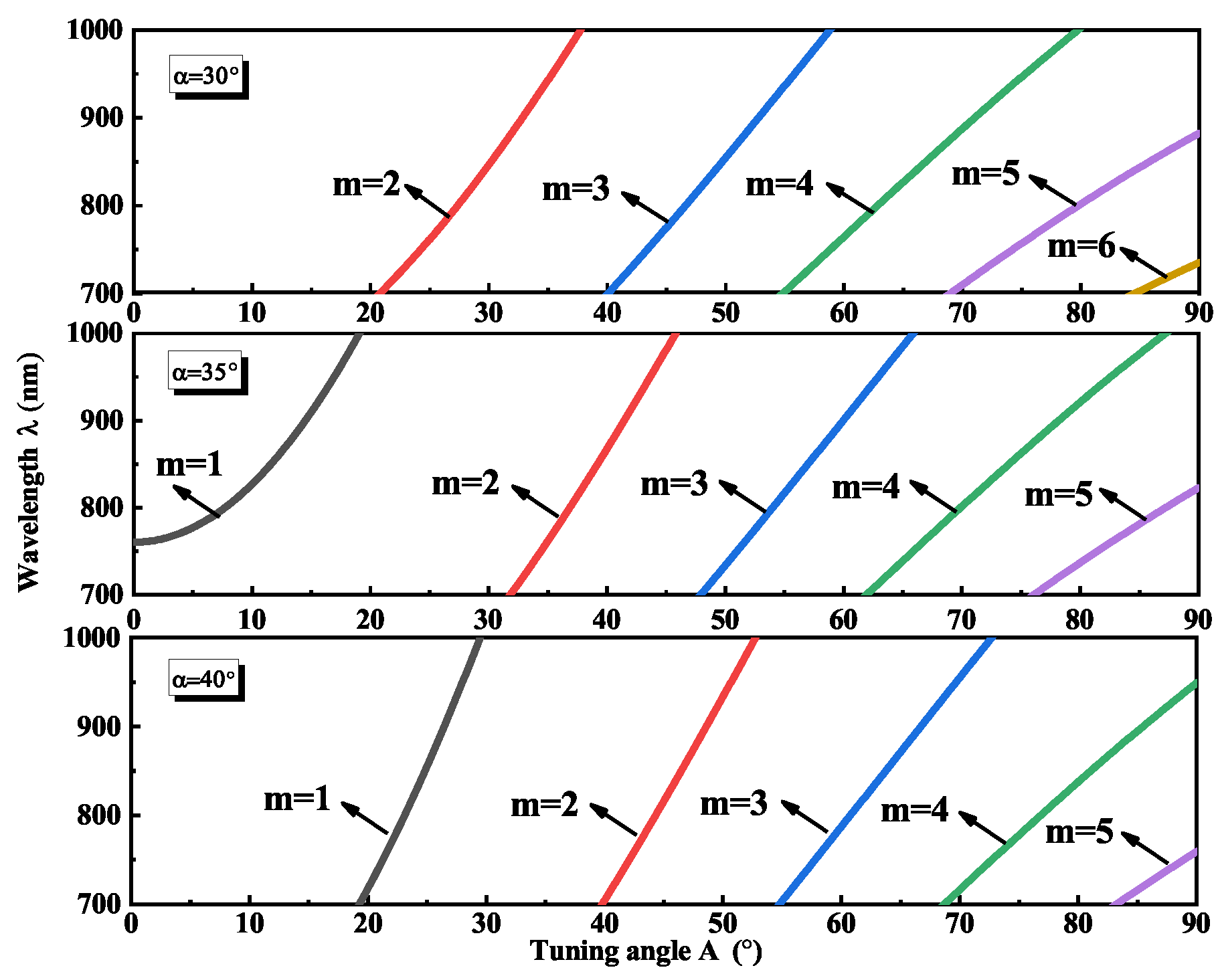
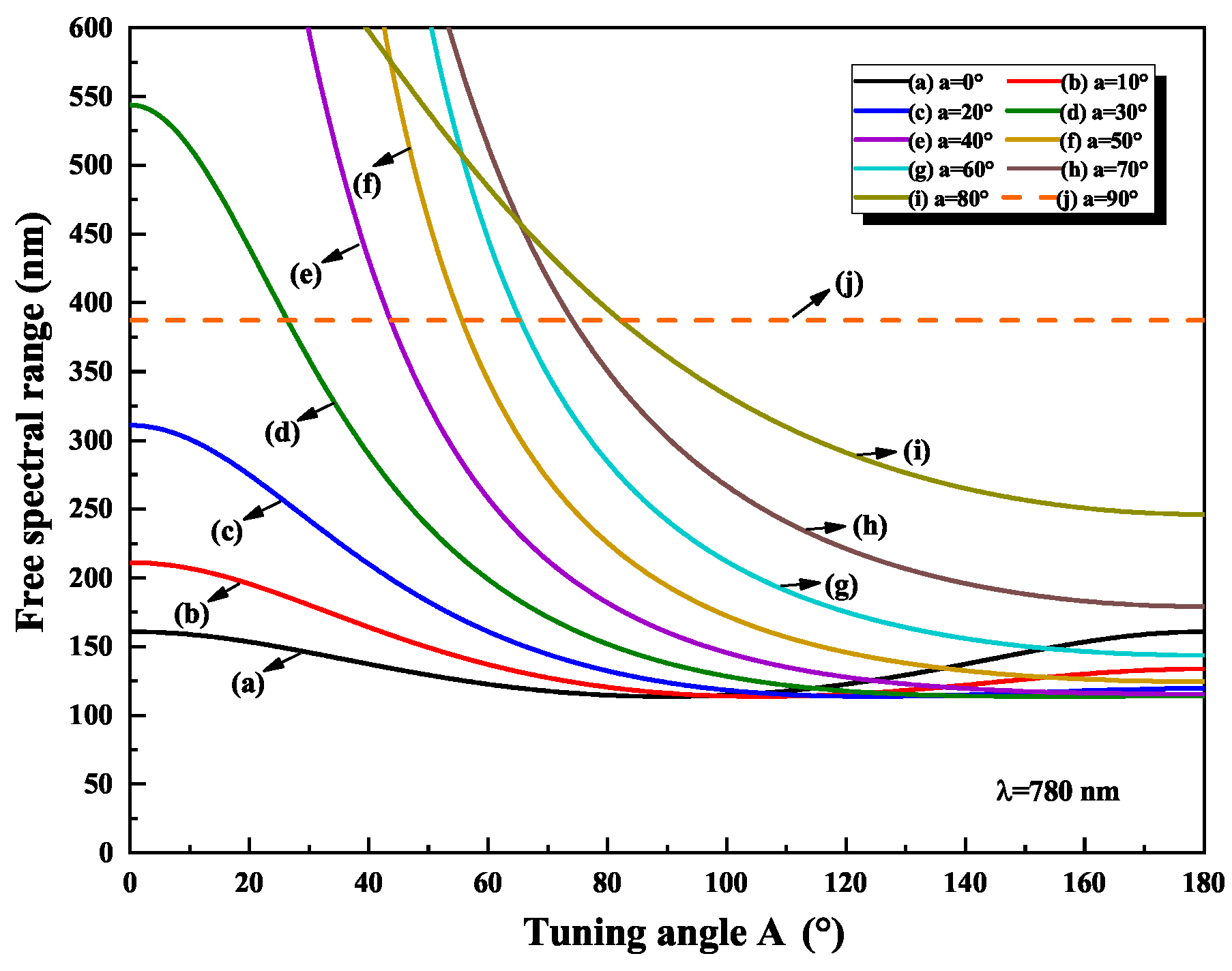
2.2. Tuning Characteristics of Multi-Plate BRF
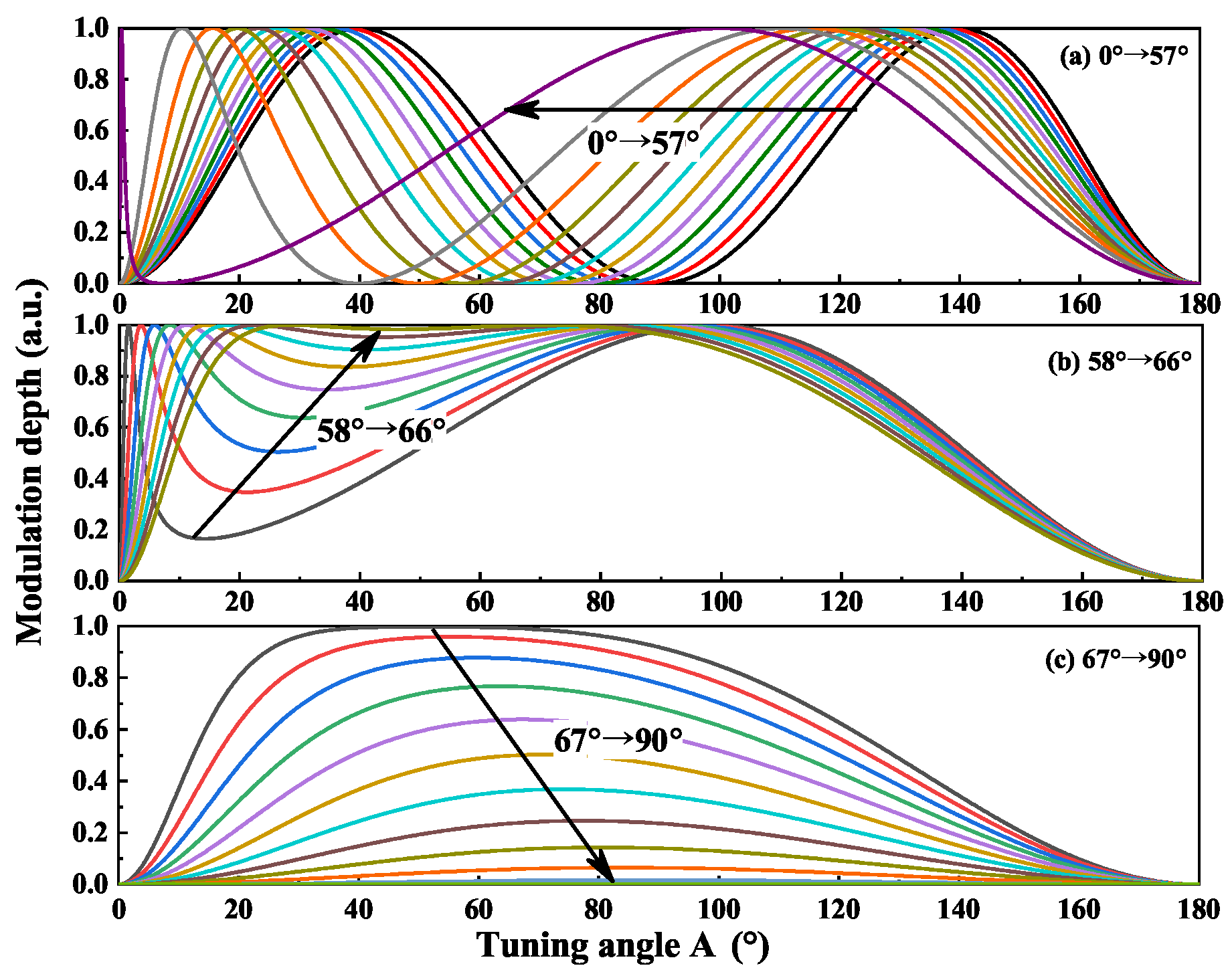
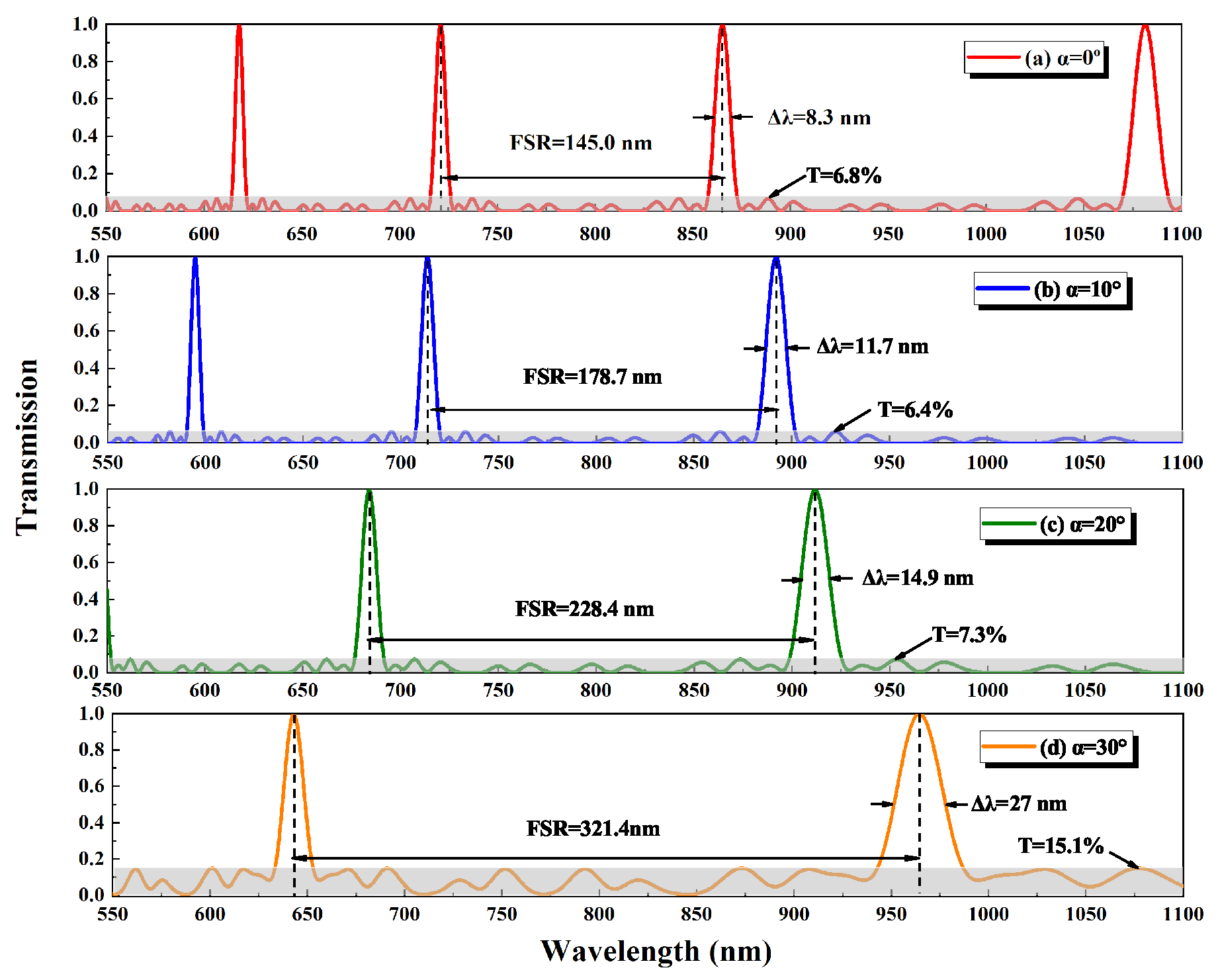
3. Design of BRF for Single-Frequency Ti:sapphire Tunable Laser
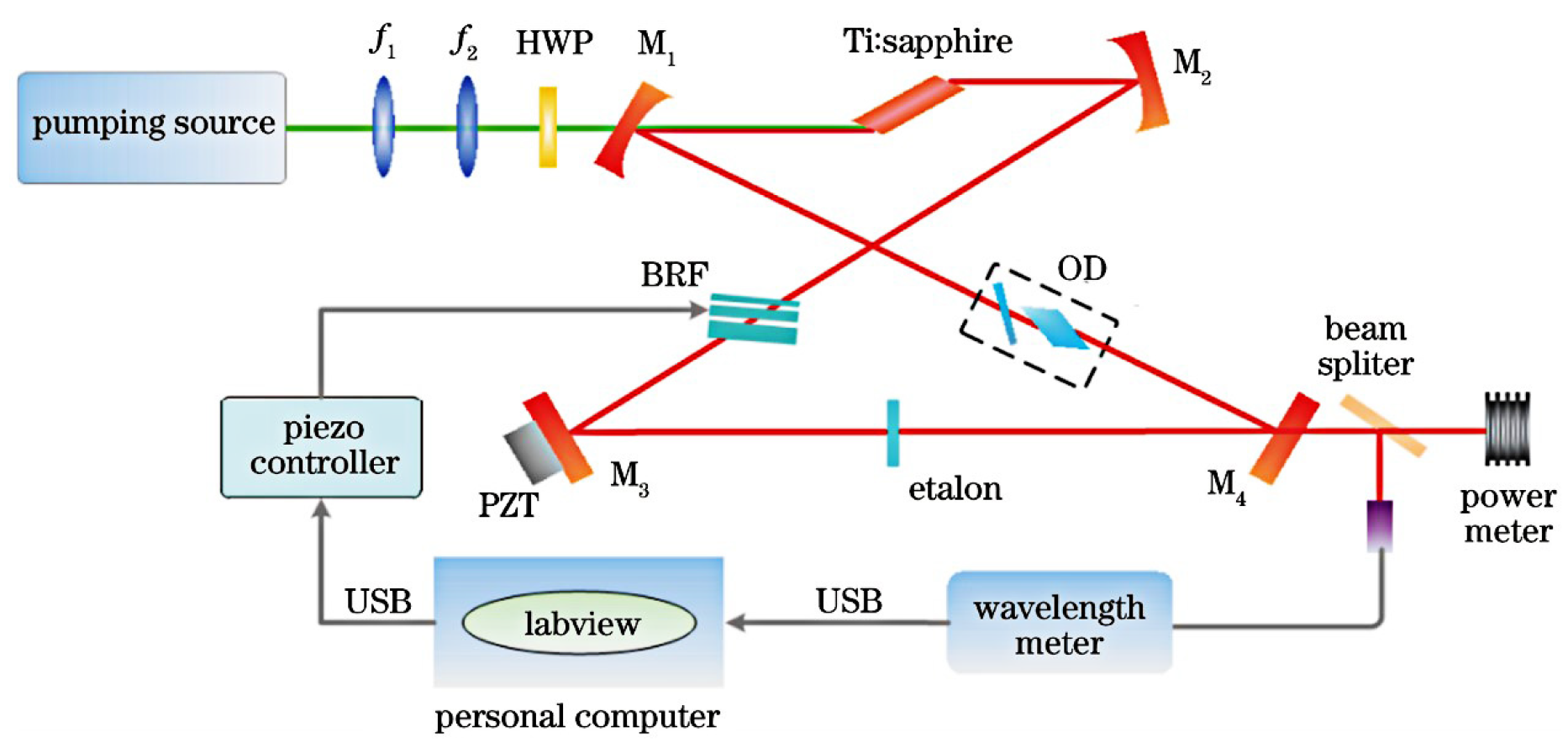
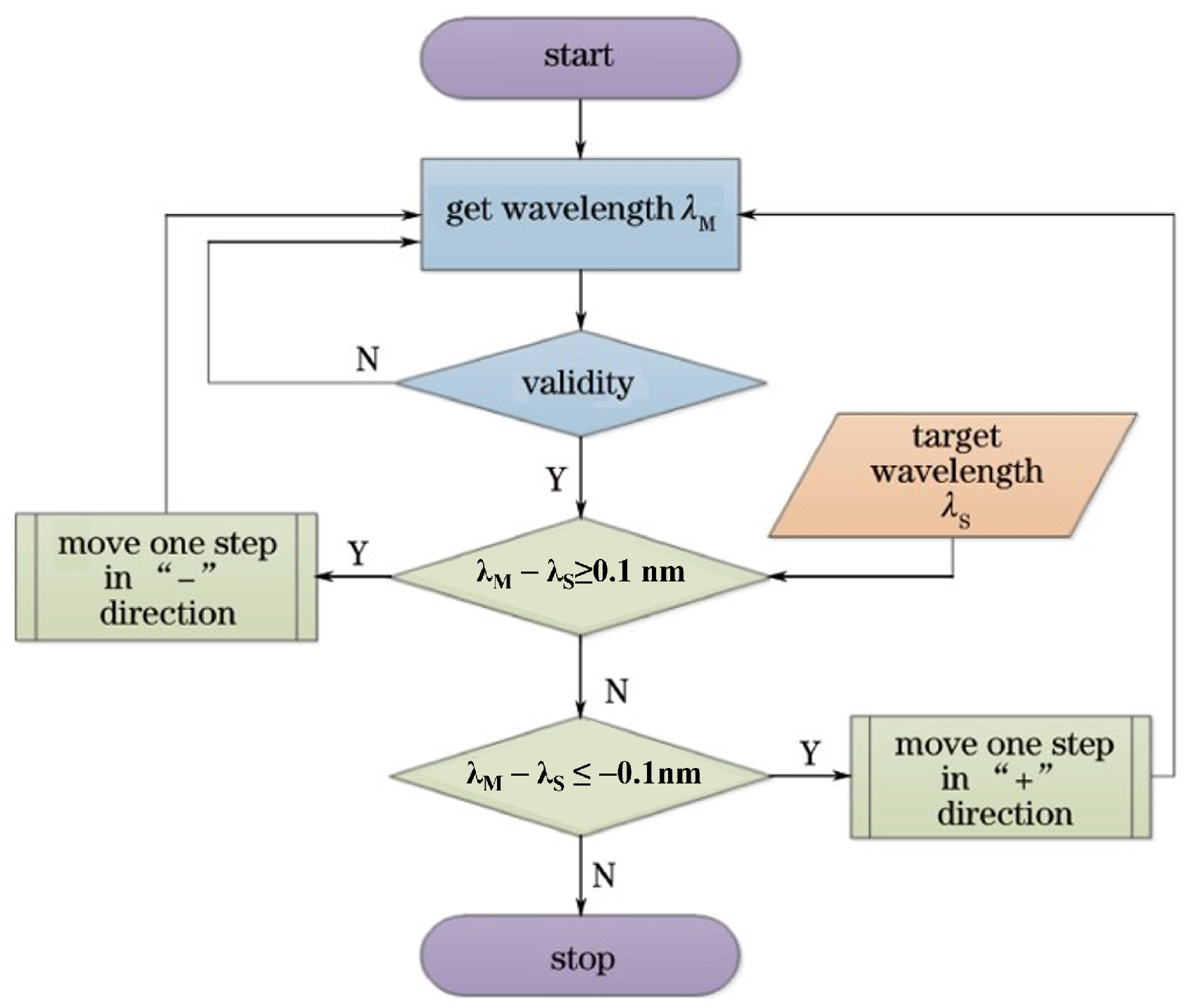
4. Automatic Control of BRF Used in Single-Frequency Ti:sapphire Tunable Laser
5. New Application of Birefringent Tuning Characteristic
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sun, Y.; Lu, H.D.; Su, J. Continuous-wave, single-frequency, all-solid-state Ti:Al2O3 laser. Acta Sin. Quantum Opt. 2008, 414, 344. [Google Scholar]
- Xu, Z.X.; Wu, W.Y.; Tian, L.; Chen, L.R.; Zhang, Z.Y.; Yan, Z.H.; Li, S.J.; Wang, H. Long lifetime and high-fidelity quantum memory of photonics polarization qubit by lifting zeeman degeneracy. Phys. Rev. Lett. 2013, 111, 240503. [Google Scholar] [CrossRef]
- Barber, Z.W.; Hoyt, C.W.; Oates, C.W.; Hollberg, L. Direct Excitation of the Forbidden Clock Transition in Neutral 174Yb Atoms Confined to an Optical Lattice. Phys. Rev. Lett. 2006, 96, 083002. [Google Scholar] [CrossRef]
- Wolfgramm, F.; Cere, A.; Beduini, F.A.; Predojevic, A.; Koschorreck, M.; Mitchell, M.W. Squeezed-light optical magnetometry. Phys. Rev. Lett. 2010, 105, 053601. [Google Scholar] [CrossRef]
- Kuwanmoto, T.; Honda, K.; Takahashi, Y.; Yabuzaki, T. Magneto-optical trapping of Yb atoms using an intercombination transition. Phys. Rev. A 1999, 60, R745. [Google Scholar] [CrossRef]
- Wei, K.; Zhao, T.; Fang, X.J.; Zhai, Y.Y.; Li, H.R.; Quan, W. In-situ measurement of the density ratio of K-Rb hybrid vapor cell using spin-exchange collision mixing of the K and Rb light shifts. Opt. Express 2017, 27, 16169. [Google Scholar] [CrossRef]
- Jia, X.J.; Yan, Z.H.; Duan, Z.Y.; Su, X.L.; Wang, H.; Xie, C.D.; Peng, K.C. Experimental Realization of Three-Color Entanglement at Optical Fiber Communication and Atomic Storage Wavelengths. Phys. Rev. Lett. 2012, 109, 253604. [Google Scholar] [CrossRef]
- Gou, Y.; Zhang, Y.D.; Su, H.Y.; Zhu, F.X.; Yi, G.; Wang, J.F. Magnetic-filed tuning whispering gallery mode based on hollow microbubble resonator with Terfenol-D-fixed. Appl. Opt. 2019, 58, 8889. [Google Scholar]
- Wang, Y.B.; Holguin-Lerma, J.A.; Vezzoli, M.; Guo, Y.; Tang, H.X. Photonic-circuit-integrated titanium:sapphire laser. Nat. Photonics 2023, 17, 338. [Google Scholar] [CrossRef]
- Hwang, H.; Byun, A.; Park, J.; de Léséleuc, S.; Ahn, J. Optical tweezers throw and catch single atoms. Optica 2023, 10, 401. [Google Scholar] [CrossRef]
- Kerridge-Johns, W.R.; Damzen, M.J. Analytical model of tunable Alexandrite lasing under diode end-pumping with experimental comparison. J. Opt. Soc. Am. B 2016, 33, 2525. [Google Scholar] [CrossRef]
- Sahoo, S.P.; Pradhan, S.; Mukherjee, J.; Rawat, V.S. Studies of temporal characteristics of all-solid-state, gain-switched Cr:forsterite laser. Optik 2021, 227, 166024. [Google Scholar] [CrossRef]
- Kopylov, D.A.; Esaulkov, M.N.; Kuritsyn, I.I.; Mavritskiy, A.O.; Perminov, B.E.; Konyashchenko, A.V.; Murzina, T.V.; Maydykovskiy, A.I. Kerr-lens mode-locked Ti:Sapphire laser pumped by a single laser diodeU. Laser Phy. Lett. 2018, 15, 045001. [Google Scholar] [CrossRef]
- Pati, B.; Borysow, J. Single-mode tunable Ti:sapphire laser over a wide frequency range. Appl. Opt. 1997, 36, 9337. [Google Scholar] [CrossRef]
- Jin, P.X.; Lu, H.D.; Wei, Y.X.; Su, J.; Peng, K.C. Single-frequency CW Ti:sapphire laser with intensity noise manipulation and continuous frequency-tuning. Opt. Lett. 2017, 42, 143. [Google Scholar] [CrossRef]
- Lyot, B. Quelques observation de la couronne solaire at des protuberances. L’Astronomie 1937, 51, 203. [Google Scholar]
- Lyot, B. The study of the solar corona and prominences without eclipses. Mon. Not. R. Astron. Soc. 1939, 99, 580. [Google Scholar]
- Camichel, H.; Lyot, B. Observations planetaires au pic du midi. L’Astronomie 1941, 57, 49. [Google Scholar]
- Loyt, B. Le filter monochromatique polarisant et ses applications en physique solaire. Ann. D’ Astrophys. 1944, 7, 31. [Google Scholar]
- Lyot, B. Un monochromateur a grand champ utilisant les interferences en Lumiere polarisee. Compt. Rend 1933, 197, 1593. [Google Scholar]
- Billings, B.H.; Hyde, W.L.; Barr, E.E. An investigation of the properties of evaporated metal bolometers. J. Opt. Soc. Am. 1947, 37, 123. [Google Scholar] [CrossRef]
- Billings, B.H.; Land, E.H. A comparative survey of some possible systems of polarized headlights. J. Opt. Soc. Am. 1948, 38, 819. [Google Scholar] [CrossRef]
- Evans, J.W. The birefringent filter. J. Opt. Soc. Am. 1949, 39, 229. [Google Scholar] [CrossRef]
- Šolc, I. Birefringent Chain Filters. J. Opt. Soc. Am. 1965, 55, 621. [Google Scholar] [CrossRef]
- Maiman, T.H. Stimulated emission of radiation in ruby. Nature 1960, 187, 493. [Google Scholar] [CrossRef]
- Sorokin, P.P.; Stevenson, M.J. Stimulated Infrared Emission from Trivalent Uranium. Phys. Rev. Lett. 1960, 5, 557. [Google Scholar] [CrossRef]
- Snitzer, E. Optical maser action of Nd+3 in a Barium Crown Glass. Phys. Rev. Lett. 1961, 7, 444. [Google Scholar] [CrossRef]
- Geusic, J.E.; Marcos, H.M.; Van Uitert, L.G. Laser oscilations in Nd-doped Yttrium Alumnum, Yttrium Gallium and Gadolinium Garnets. Appl. Phys. Lett. 1964, 4, 182. [Google Scholar] [CrossRef]
- Siegman, A.E.; Phillion, D.W.; Kuizenga, D.J. Rotational relaxation and triplet-state effects in the cw dye laser. Appl. Phys. Lett. 1972, 21, 345. [Google Scholar] [CrossRef]
- Bloom, A.L. Modes of a laser resonator containing tilted birefringent plates. J. Opt. Soc. Am. 1974, 64, 447. [Google Scholar] [CrossRef]
- Hodgkinson, I.J.; Vukusic, J.I. Birefringent filters for tuning flashlamp-pumped dye lasers: Simplified theory and design. Appl. Opt. 1978, 17, 1944. [Google Scholar] [CrossRef] [PubMed]
- Title, A.M. Improvement of Birefringent Filters.2: Achromatic Waveplates. Appl. Opt. 1975, 14, 229. [Google Scholar] [CrossRef]
- Hodgkinson, I.J.; Vukusic, J.I. Birefringent tuning filters without secondary peaks. Opt. Commun. 1978, 24, 133. [Google Scholar] [CrossRef]
- Chakraborty, A.K.; Adhikari, B.M. Birefringent Filters. J. Opt. 1977, 6, 73. [Google Scholar] [CrossRef]
- Yano, T.; Watanabe, A. New noncollinear acousto-optic tunable filter using birefringence in paratellurite. Appl. Phys. Lett. 1974, 24, 256. [Google Scholar] [CrossRef]
- Tang, C.L.; Kreismanis, V.G.; Ballantyne, J.M. Wide-band electro-optical tuning of semiconductor lasers. Appl. Phys. Lett. 1977, 30, 113. [Google Scholar] [CrossRef]
- Preuss, D.R.; Gole, J.L. Three-stage birefringent filter tuning smoothly over the visible region: Theoretical treatment and experimental design. Appl. Opt. 1980, 19, 702. [Google Scholar] [CrossRef]
- Liu, Y.S. Line narrowing and tuning of high-power Nd:glass laser using an an intracavity Brewster-angle birefringent filter. J. Appl. Phys. 1977, 48, 647. [Google Scholar] [CrossRef]
- Holtom, G.; Teschke, O. Design of a Birefringent Filter for High-power dye lasers. IEEE J. Quantum Electron. 1974, 10, 577. [Google Scholar] [CrossRef]
- Kachanow, A.A. Birefringent selector of the emission wavelength for a CW dye laser. Sov. J. Quantum Electron. 1982, 12, 927. [Google Scholar] [CrossRef]
- Mental, J.; Schmidt, E.; Mavrudis, T. Birefringent filter with arbitrary orientation of the optic axis: An analysis of improved accuracy. Appl. Opt. 1992, 31, 5022. [Google Scholar] [CrossRef] [PubMed]
- Title, A.M.; Schoolman, S.A. Recent advances in birefringent filters. Polariz. Light 1976, 88, 23. [Google Scholar]
- Kuhl, J.; Lambrich, R.; Linde, D.V. Generation of near-infrared picosecond pulses by mode-locked synchronous pumping of a jet-stream dye laser. Appl. Phys. Lett. 1977, 31, 657. [Google Scholar] [CrossRef]
- Kuhl, J.; Telle, H.; Schieder, R.; Brinkmann, U. New efficient and stable laser dyes for cw operation in the blue and violet spectral region. Opt. Commun. 1978, 24, 251. [Google Scholar] [CrossRef]
- Moulton, P.F.; Mooradian, A. Broadly tunable CW operation of Ni:MgF2 and Co:MgF2 lasers. Appl. Phys. Lett. 1979, 35, 838. [Google Scholar] [CrossRef]
- Moulton, P.F. Pulsed-pumped operation of divalent transition-metal lasers. IEEE J. Quantum Electron. 1982, 18, 1185. [Google Scholar] [CrossRef]
- Lovold, S.; Moulton, P.F.; Killinger, D.K.; Menyuk, N. Frequency tuning characteristics of a Q-Switched Co:MgF2 laser. IEEE J. Quantum Electron. 1985, 21, 202. [Google Scholar] [CrossRef]
- Bair, C.H.; Brockman, P.; Hess, R.V.; Modlin, E.A. Demonstration of frequency control and CW diode laser injection control of a Titanium-doped sapphire ring laser with no internal optical elements. IEEE J. Quantum Electron. 1988, 24, 1045. [Google Scholar] [CrossRef]
- Zhu, S.D. Birefringent filter with tilted optic axis for tuning dye lasers: Theory and design. Appl. Opt. 1990, 29, 410. [Google Scholar] [CrossRef]
- Demirbas, U.; Thesinga, J.; Beyatli, E.; Kellert, M.; Pergament, M.; Kartner, F.X. Continuous-wave Tm:YLF laser with ultrabroad tuning (1772–2145 nm). Opt. Express 2022, 30, 41219. [Google Scholar] [CrossRef]
- Lai, W.Y.; Zhang, H.; Zhu, Z.X.; Yan, P.G.; Ruan, S.C.; Sun, Z.P.; Wang, J.Z. Sub-200 fs, 344MHz mode-locked Tm-doped fiber laser. Opt. Lett. 2020, 45, 5492. [Google Scholar] [CrossRef] [PubMed]
- Nadimi, M.; Major, A. Continuous-wave dual-wavelength operation of a diode-pumped Nd:GdVO4 laser at the 1063&1071 nm, 1063&1083 nm and 1083&1086 nm wavelength pairs. Laser Phys. 2018, 28, 095001. [Google Scholar]
- Kobtsev, S.M.; Sventsitskaya, N.A. Application of birefringent filters in continuous-wave tunable lasers: A review. Opt. Spectrosc. 1992, 73, 114. [Google Scholar]
- Demirbas, U. Cr:Colquiriite Lasers: Current status and challenges for further progress. Prog. Quantum Electron. 2019, 68, 100227. [Google Scholar] [CrossRef]
- Erturk, Z.; Okuyucu, S.; Kilinc, M.; Ozturk, Y.; Pergament, M.; Kärtner, F.X.; Demirbas, U. Broadly tunable two-color lasing of Cr:LiCAF with on-surface and off-surface optical axis birefringent filters: Performance comparison. Appl. Opt. 2022, 61, 10735. [Google Scholar] [CrossRef]
- Xue, Y.C.; Dai, Z.D.; Cao, W.H.; Wang, Z.Y.; Xiao, B.; Xu, H.; Cai, Z. Watt-Level Pr3+:LiYF4 Novel Green Lasers at 519 nm, 538 nm, and 550 nm. IEEE J. Quantum Electron. 2023, 59, 1700305. [Google Scholar] [CrossRef]
- Johnston, T.F.; Brady, R.H.; Proffitt, W. Supercontinua for high-resolution absorption multiplex infrared spectroscopy. Appl. Opt. 1982, 21, 2307. [Google Scholar] [CrossRef]
- Wei, Y.X.; Lu, H.D.; Jin, P.X.; Peng, K.C. Self-injection locked CW single-frequency tunable Ti:sapphire laser. Opt. Express 2017, 25, 21379. [Google Scholar] [CrossRef]
- Ding, X.; Ma, H.M.; Zou, L.; Zou, Y.; Wen, W.Q.; Wang, P.; Yao, J.Q. An all-solid-state high power quasi-continuous-wave tunable dual-wavelength Ti:sapphire laser system using birefringence filter. Chin. Phys. 2007, 16, 1991. [Google Scholar]
- Demirbas, U.; Petrich, G.S.; Li, D.; Sennaroglu, A.; Kolodziejski, L.A.; Kartner, F.X.; Fujimoto, J.G. Femtosecond tuning of Cr:colquiriite lasers with AlGaAs-based saturable Bragg reflectors. J. Opt. Soc. Am. B 2011, 28, 986. [Google Scholar] [CrossRef]
- Walling, J.; Peterson, O.; Morris, R. Tunable CW Alexandrite laser. IEEE J. Quantum. Electron. 1980, 16, 120. [Google Scholar] [CrossRef]
- Tawy, G.; Minassian, A.; Damzen, M.J. High-power 7.4W TEM00 and wavelength-tunable alexandrite laser with a novel cavity design and efficient fibre-coupled diode-pumping. OSA Contin. 2020, 3, 1638. [Google Scholar] [CrossRef]
- Dodge, M.J. Refractive properties of magnesium fluoride. Appl. Opt. 1984, 23, 1980. [Google Scholar] [CrossRef] [PubMed]
- Demirbas, U. Off-surface optic axis birefringent filters for smooth tuning of broadband lasers. Appl. Opt. 2017, 56, 7815. [Google Scholar] [CrossRef] [PubMed]
- Beyatl, E.; Demırbas, U. Widely tunable dual-wavelength operation of Tm:YLF, Tm:LuAG, and Tm:YAG lasers using off-surface optic axis birefringent filters. Appl. Opt. 2018, 57, 6679. [Google Scholar] [CrossRef]
- Demirbas, U.; Cankaya, H.; Thesinga, J.; Kärtner, F.X.; Pergament, M. Efficient, diode-pumped, high-power (>300W) cryogenic Yb:YLF laser with broad-tunability (995–1020.5 nm): Investigation of E//a-axis for lasing. Opt. Express 2019, 27, 36562. [Google Scholar] [CrossRef]
- Demirbas, U.; Kärtner, F.X.; Pergament, M. Cavity-dumped nanosecond Cr:LiSAF laser in the 985–1030 nm region for versatile seeding of Yb-based amplifiers. Appl. Phys. B 2022, 128, 20. [Google Scholar] [CrossRef]
- Mekteplioglu, M.F.; Ozturk, Y.; Pergament, M. Broadly tunable (402–535 nm) intracavity frequency-doubled Cr:LiSAF laser. Appl. Phys. B 2023, 129, 22. [Google Scholar] [CrossRef]
- Naganuma, K.; Lenz, G. Variable bandwidth birefringent filter for tunable femtosecond lasers. IEEE J. Quantum Electron. 1992, 28, 2142. [Google Scholar] [CrossRef]
- Wang, X.L.; Yao, J.Q. Transmitted and tuning characteristics of birefringent filters. Appl. Opt. 1992, 31, 4505. [Google Scholar] [CrossRef]
- Wei, J.; Cao, X.C.; Jin, P.X.; Su, J.; Lu, H.D.; Peng, K.C. Diving angle optimization of BRF in a single-frequency continuous-wave wideband tunable titanium:sapphire laser. Opt. Express 2021, 29, 6714. [Google Scholar] [CrossRef]
- Su, J.; Jin, P.X.; Wei, Y.X.; Lu, H.D.; Peng, K.C. Automatically and broadly tunable all-solid-state continuous single-frequency Ti:sapphire laser. Chin. J. Lasers 2017, 44, 0701006. [Google Scholar]
- Fernandez, J.; Iparraguirre, I.; Aramburu, I.; Illarramendi, A.; Azkargorta, J.; Voda, M. K5Nd(MoO4)4: A self-tunable laser crystal. Opt. Lett. 2003, 28, 1341. [Google Scholar] [CrossRef]
- Fernandez, J.; Illarramendi, M.A.; Iparraguirre, I.; Aramburu, I.; Azkargorta, J.; Voda, M.; Al-Saleh, M.; Balda, R. Rb5Nd(MoO4)4 a self-tunable birefringent laser crystal. Opt. Mater. 2004, 26, 483. [Google Scholar] [CrossRef]
- Aramburu, I.; Iparraguirre, I.; Illarramendi, M.A.; Azkargorta, J.; Fernandez, J.; Balda, R. Self-tuning in birefringent La3Ga5SiO14:Nd3+ laser crystal. Opt. Mater. 2005, 27, 1692. [Google Scholar] [CrossRef]
- Iparraguirre, I.; Aramburu, I.; Azkargorta, J.; Illarramendi, M.A.; Fernandez, J.; Balda, R. Wavelength tuning of Titanium Sapphire Laser by its own crystal birefringence. Opt. Express 2005, 13, 1254. [Google Scholar] [CrossRef] [PubMed]
- Iparraguirre, I.; Azkargorta, J.; Fernandez, J.; Balda, R.; Gatelurrutia, T.; Illarramendi, M.A.; Aramburu, I. A self-tunable Titanium Sapphire Laser by rotating a set of parallel plates of active material. Opt. Express 2009, 17, 3771. [Google Scholar] [CrossRef]
- Wei, J.; Cao, X.C.; Jin, P.X.; Shi, Z.; Su, J.; Lu, H.D. Realization of compact Watt-level single-frequency continuous-wave self-tuning titanium: Sapphire laser. Opt. Express 2021, 29, 2679. [Google Scholar] [CrossRef]






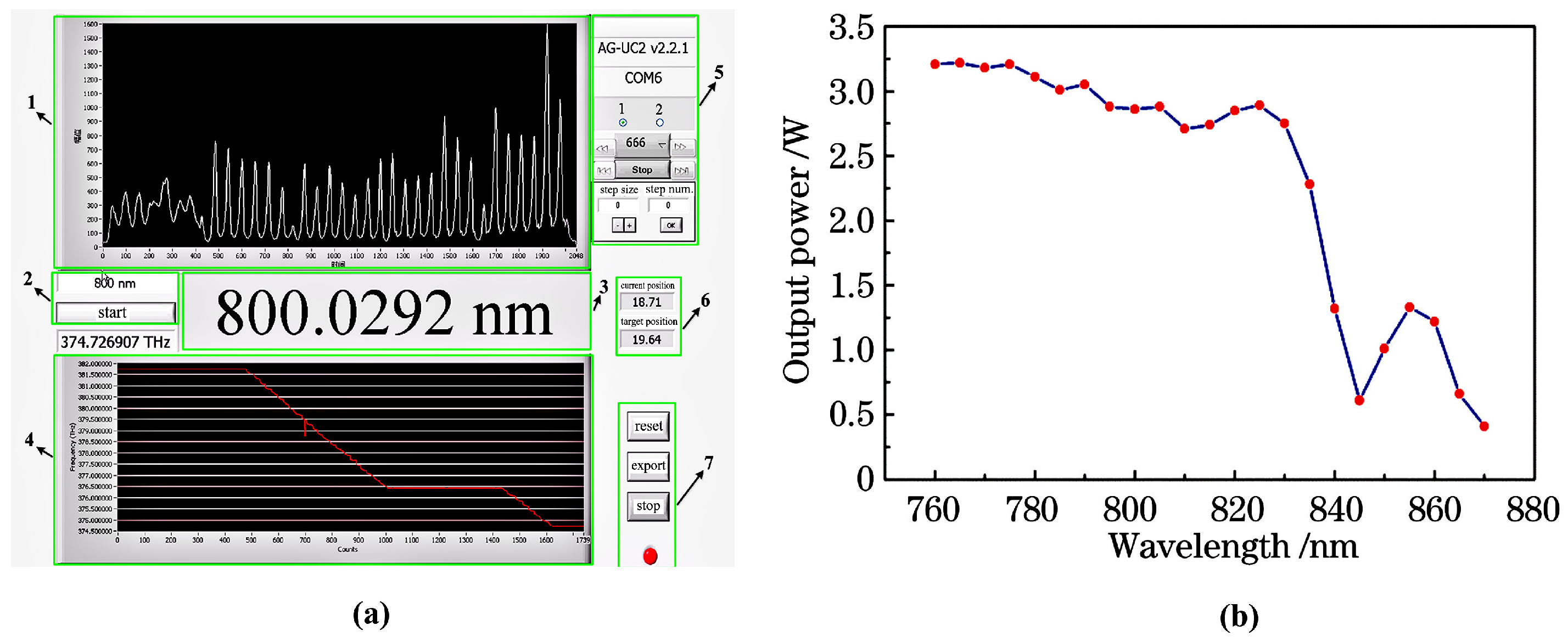
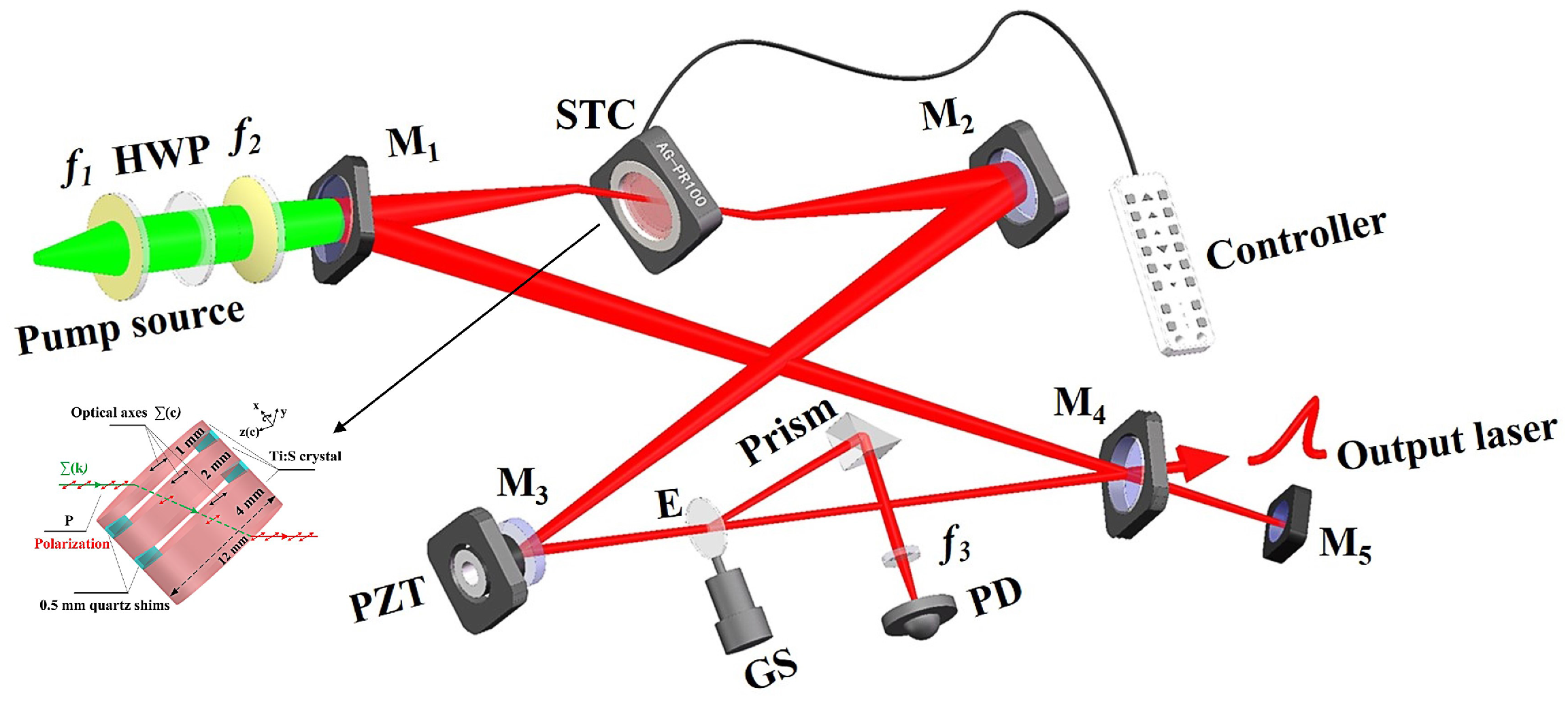
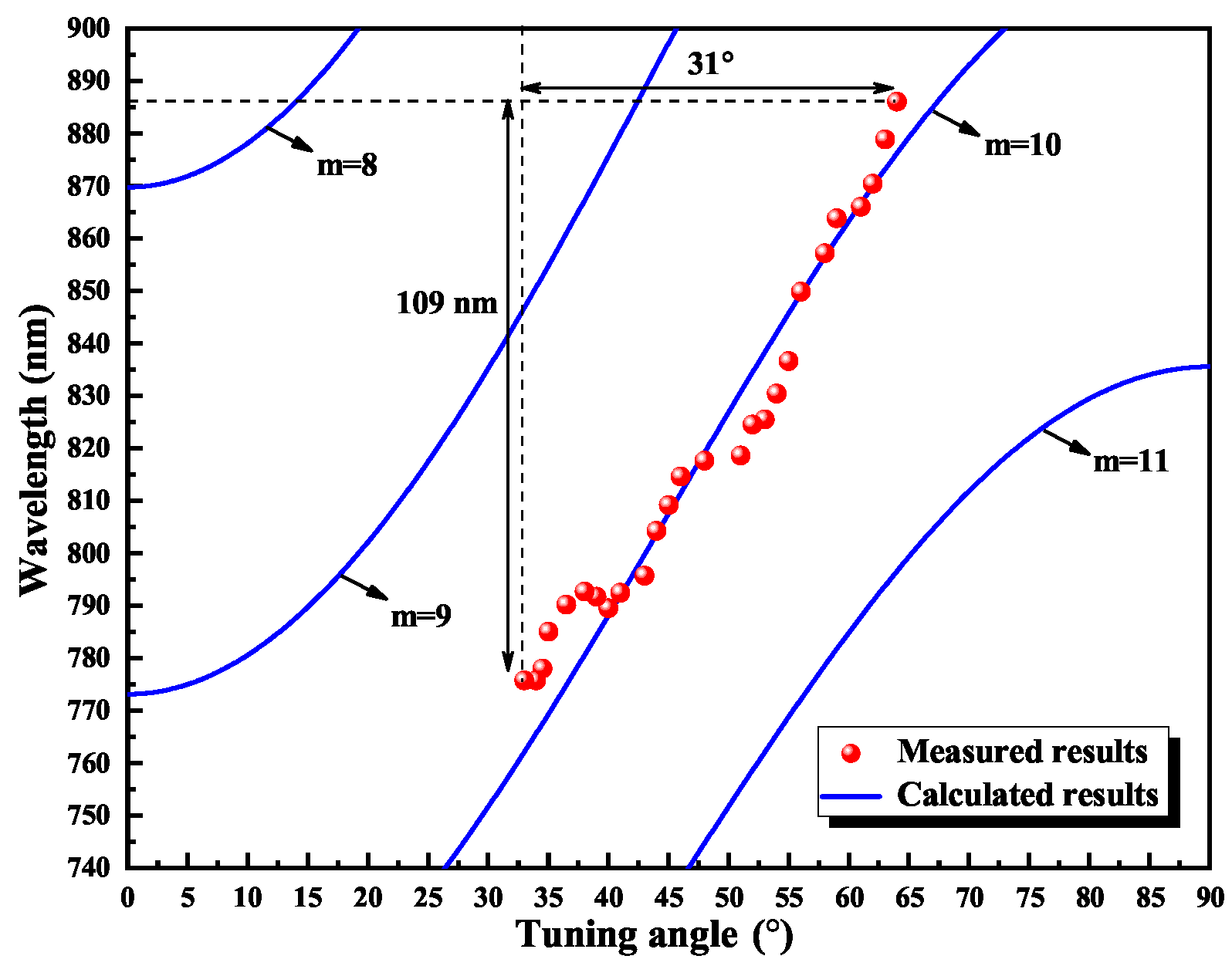
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, J.; Su, J.; Lu, H.; Peng, K. A Review of Progress about Birefringent Filter Design and Application in Ti:sapphire Laser. Photonics 2023, 10, 1217. https://doi.org/10.3390/photonics10111217
Wei J, Su J, Lu H, Peng K. A Review of Progress about Birefringent Filter Design and Application in Ti:sapphire Laser. Photonics. 2023; 10(11):1217. https://doi.org/10.3390/photonics10111217
Chicago/Turabian StyleWei, Jiao, Jing Su, Huadong Lu, and Kunchi Peng. 2023. "A Review of Progress about Birefringent Filter Design and Application in Ti:sapphire Laser" Photonics 10, no. 11: 1217. https://doi.org/10.3390/photonics10111217
APA StyleWei, J., Su, J., Lu, H., & Peng, K. (2023). A Review of Progress about Birefringent Filter Design and Application in Ti:sapphire Laser. Photonics, 10(11), 1217. https://doi.org/10.3390/photonics10111217




