Microresonator Effective Thermal Parameters Definition via Thermal Modes Decomposition
Abstract
:1. Introduction
2. Modeling of Thermal Effects in Optical Microresonators
3. Effective Parameters Determination Methods
3.1. Analytical Formulas
3.2. Thermal Dynamics Approximation by the Sum of Exponents
3.3. Thermal Mode Decomposition Method
3.3.1. Thermal Modes Existence Range
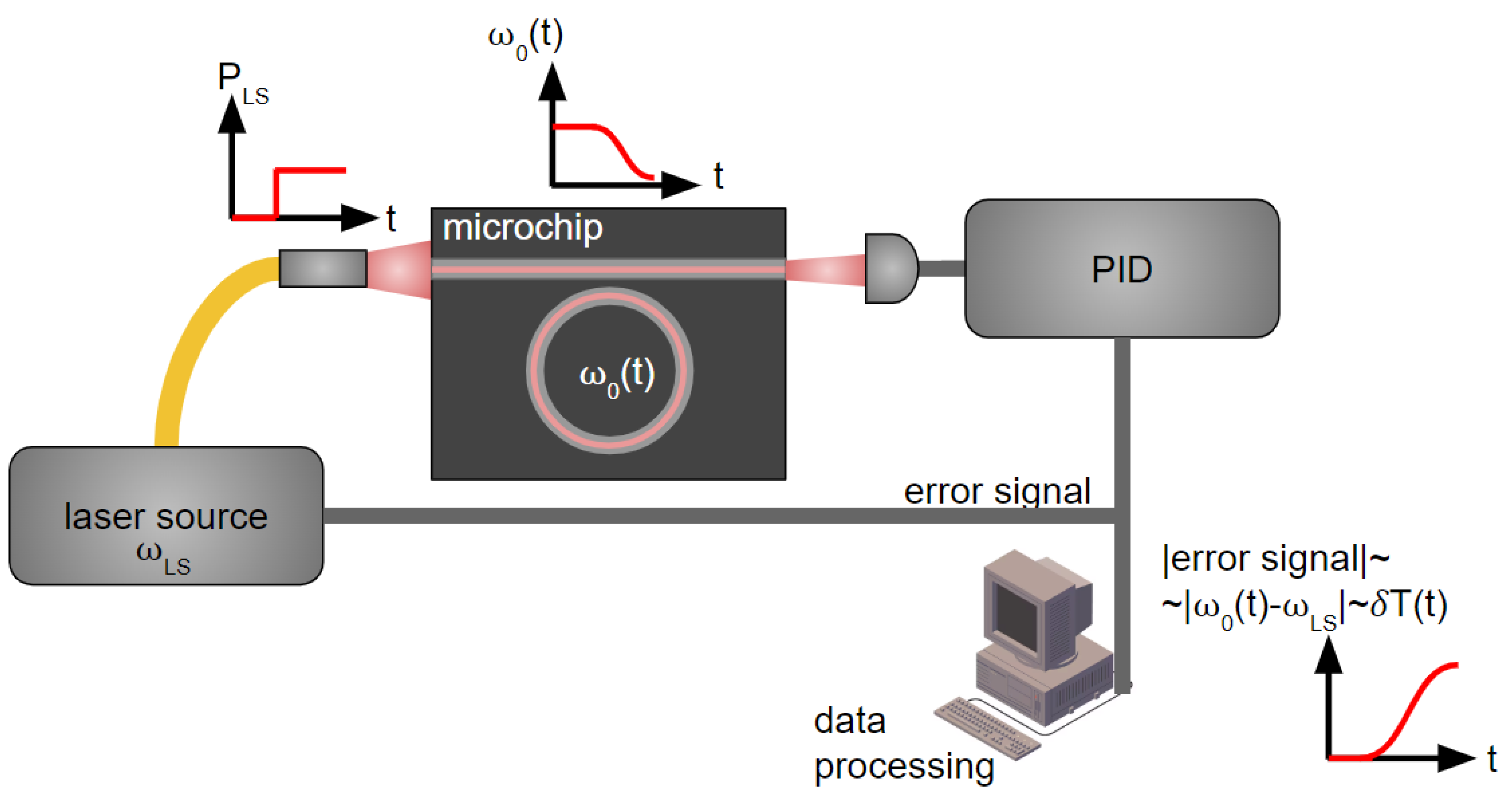
3.3.2. Comparison with Experiment
4. Discussion
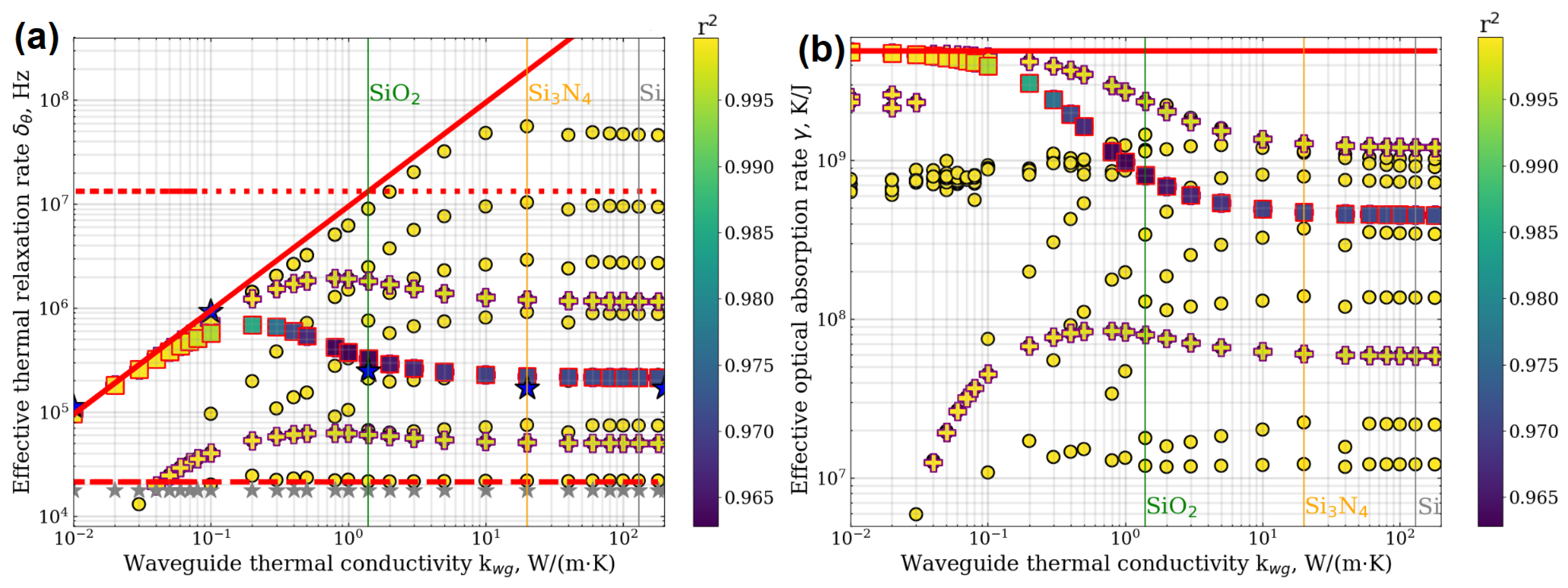
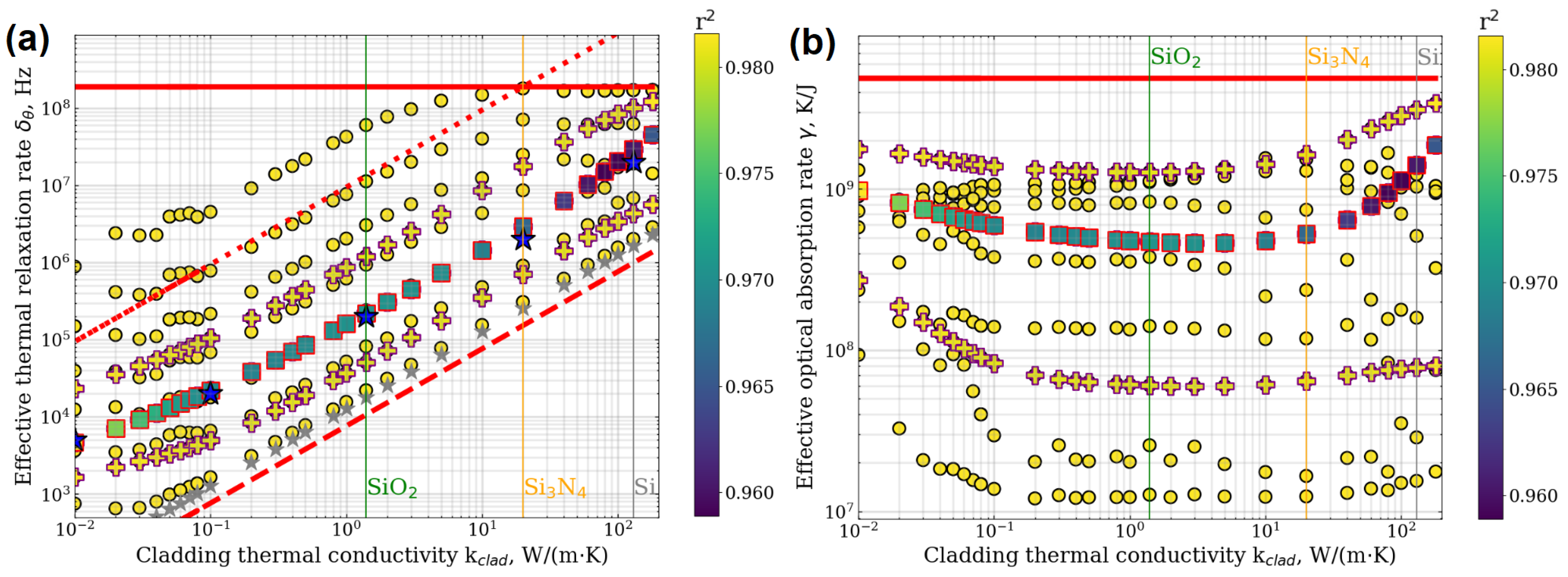
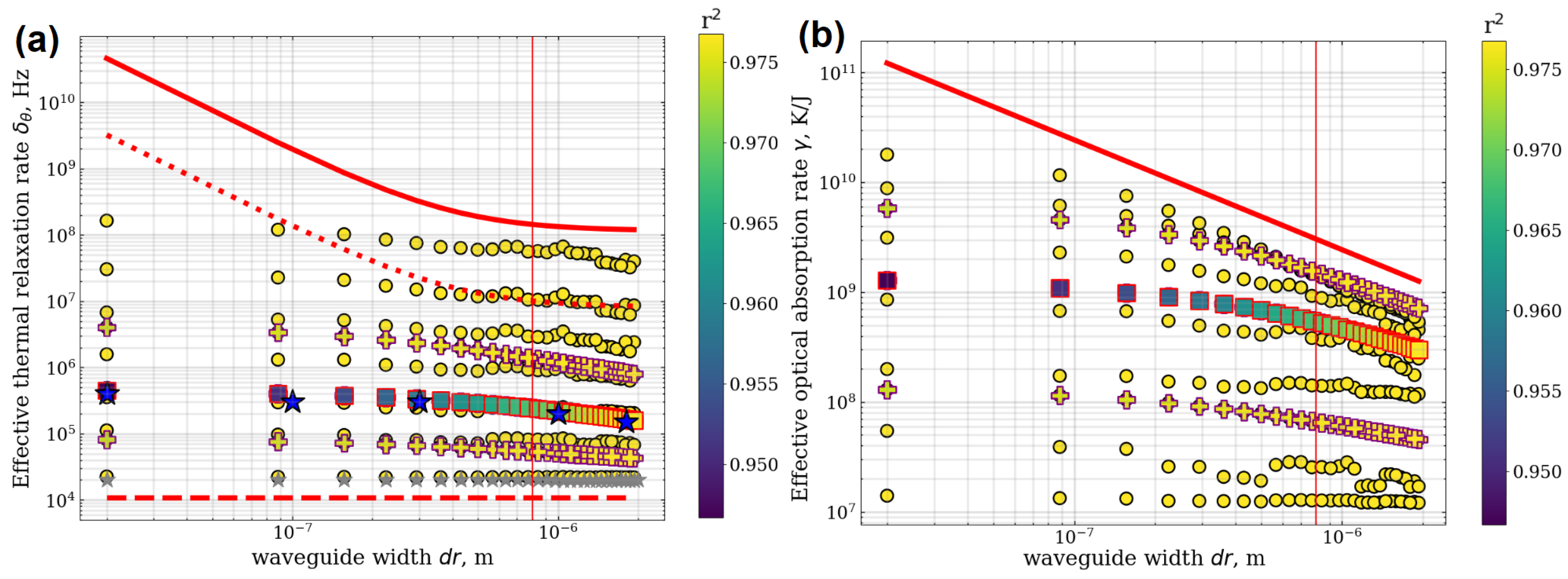
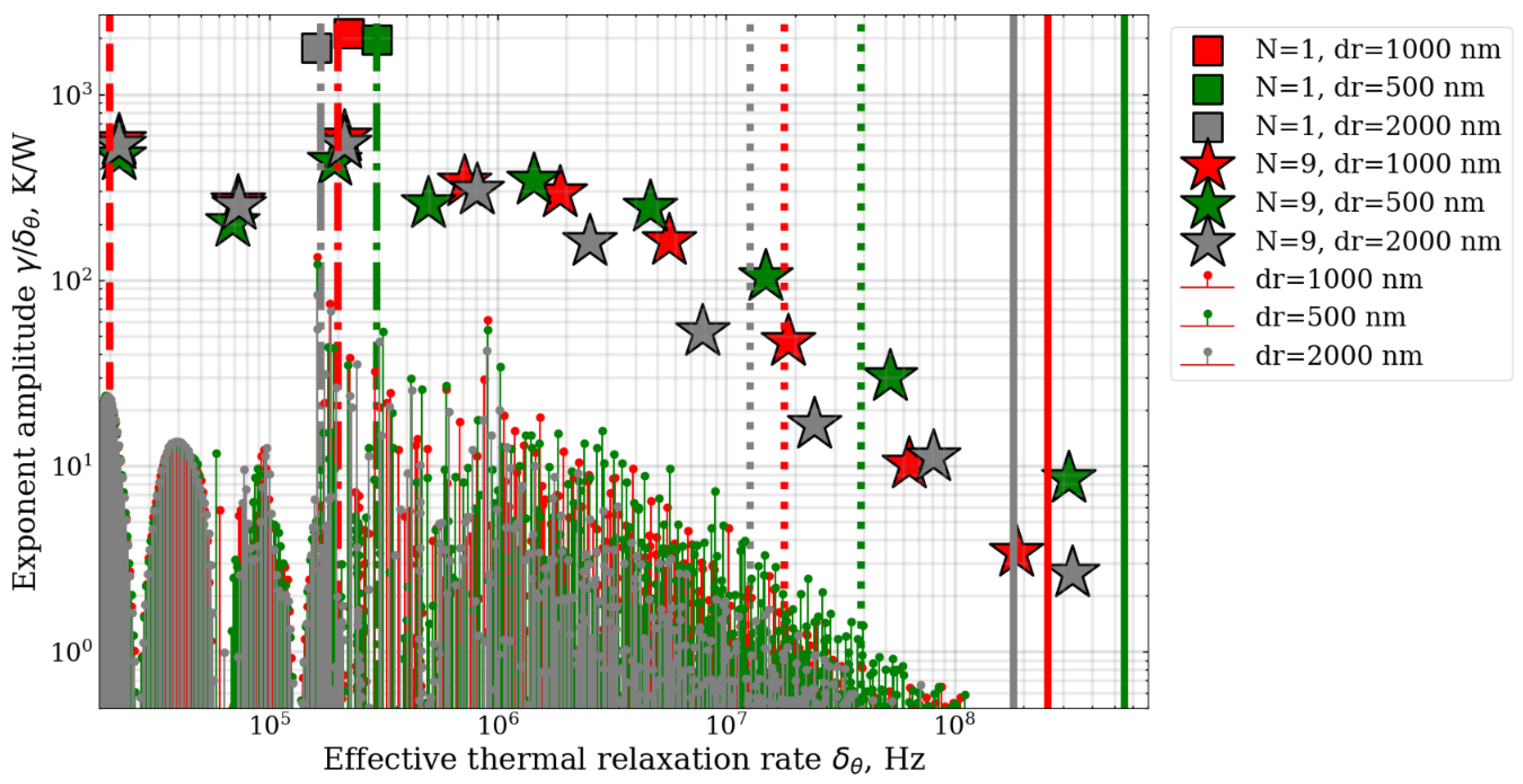
4.1. Applicability Range and Accuracy Analysis of Microresonator Thermal Parameters Estimation Methods
4.2. Experimental Limitations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Braginsky, V.B.; Gorodetsky, M.L.; Ilchenko, V.S. Quality-factor and nonlinear properties of optical whispering-gallery modes. Phys. Lett. A 1989, 137, 393–397. [Google Scholar] [CrossRef]
- Vahala, K.J. Optical microcavities. Nature 2003, 424, 839. [Google Scholar] [CrossRef]
- Vassiliev, V.V.; Velichansky, V.L.; Ilchenko, V.S.; Gorodetsky, M.L.; Hollberg, L.; Yarovitsky, A.V. Narrowline- width diode laser with a high-Q microsphere resonator. Opt. Commun. 1998, 158, 305. [Google Scholar]
- Matsko, A.B.; Ilchenko, V.S. Optical resonators with whispering-gallery modes-part II: Applications. IEEE J. Sel. Top. Quantum Electron. 2006, 12, 15–32. [Google Scholar] [CrossRef]
- Ward, J.; Benson, O. WGM microresonators: Sensing, lasing and fundamental optics with microspheres. Laser Photon. Rev. 2011, 5, 553. [Google Scholar] [CrossRef]
- Strekalov, D.V.; Marquardt, C.; Matsko, A.B.; Schwefel, H.G.L.; Leuchs, G. Nonlinear and quantum optics with whispering gallery resonators. J. Opt. 2016, 18, 123002. [Google Scholar] [CrossRef]
- Lin, G.; Coillet, A.; Chembo, Y.K. Nonlinear photonics with high-Q whispering-gallery-mode resonators. Adv. Opt. Photonics 2017, 9, 828–890. [Google Scholar] [CrossRef]
- Kues, M.; Reimer, C.; Lukens, J.M.; Munro, W.J.; Weiner, A.M.; Moss, D.J.; Morandotti, R. Quantum optical microcombs. Nat. Photonics 2019, 13, 170. [Google Scholar] [CrossRef]
- Kondratiev, N.M.; Lobanov, V.E.; Shitikov, A.E.; Galiev, R.R.; Chermoshentsev, D.A.; Dmitriev, N.Y.; Danilin, A.N.; Lonshakov, E.A.; Min’kov, K.N.; Sokol, D.M.; et al. Recent advances in laser self-injection locking to high-Q microresonators. Front. Phys. 2023, 18, 21305. [Google Scholar] [CrossRef]
- Sun, Y.; Wu, J.; Tan, M.; Xu, X.; Li, Y.; Morandotti, R.; Mitchell, A.; Moss, D.J. Applications of optical microcombs. Adv. Opt. Photonics 2023, 15, 86. [Google Scholar] [CrossRef]
- Ilchenko, V.S.; Gorodetskii, M.L. Thermal nonlinear effects in optical whispering gallery microresonators. Laser Phys. 1992, 2, 1004–1009. [Google Scholar]
- Carmon, T.; Yang, L.; Vahala, K.J. Dynamical thermal behavior and thermal self-stability of microcavities. Opt. Express 2004, 12, 4742–4750. [Google Scholar] [CrossRef] [PubMed]
- Fomin, A.E.; Gorodetsky, M.L.; Grudinin, I.S.; Ilchenko, V.S. Nonstationary nonlinear effects in optical microspheres. J. Opt. Soc. Am. 2005, B 22, 459–465. [Google Scholar] [CrossRef]
- Gorodetsky, M.L.; Grudinin, I.S. Fundamental thermal fluctuations in microspheres. J. Opt. Soc. Am. 2004, B 21, 697–705. [Google Scholar] [CrossRef]
- Grudinin, I.S.; Vahala, K.J. Thermal instability of a compound resonator. Opt. Express 2009, 17, 14088. [Google Scholar] [CrossRef] [PubMed]
- Diallo, S.; Lin, G.; Chembo, Y.K. Giant thermo-optical relaxation oscillations in millimeter-size whispering gallery mode disk resonators. Opt. Lett. 2015, 40, 3834–3837. [Google Scholar] [CrossRef]
- Kondratiev, N.; Gorodetsky, M. Thermorefractive noise in whispering gallery mode microresonators: Analytical results and numerical simulation. Phys. Lett. A 2017, 382, 2265–2268. [Google Scholar] [CrossRef]
- Huang, G.; Lucas, E.; Liu, J.; Raja, A.S.; Lihachev, G.; Gorodetsky, M.L.; Engelsen, N.J.; Kippenberg, T.J. Thermorefractive noise in silicon–nitride microresonators. Phys. Rev. 2019, 99, 061801. [Google Scholar] [CrossRef]
- Panuski, C.; Englund, D.; Hamerly, R. Fundamental thermal noise limits for optical microcavities. Phys. Rev. 2020, 10, 041046. [Google Scholar] [CrossRef]
- Leshem, A.; Qi, Z.; Carruthers, T.F.; Menyuk, C.R.; Gat, O. Thermal instabilities, frequency-comb formation, and temporal oscillations in Kerr microresonators. Phys. Rev. 2021, A 103, 013512. [Google Scholar] [CrossRef]
- Lobanov, V.E.; Kondratiev, N.M.; Shitikov, A.E.; Borovkova, O.V.; Cordette, S.J.; Bilenko, I.A. Platicon stability in hot cavities. Opt. Lett. 2023, 48, 2353–2356. [Google Scholar] [CrossRef] [PubMed]
- Herr, T.; Brasch, V.; Jost, J.D.; Wang, C.Y.; Kondratiev, N.M.; Gorodetsky, M.L.; Kippenberg, T.J. Temporal solitons in optical microresonators. Nat. Photonics 2014, 8, 145–152. [Google Scholar] [CrossRef]
- Bao, C.; Xuan, Y.; Jaramillo-Villegas, J.A.; Leaird, D.E.; Qi, M.; Weiner, A.M. Direct soliton generation in microresonators. Opt. Lett. 2017, 42, 2519–2522. [Google Scholar] [CrossRef] [PubMed]
- Kobatake, T.; Kato, T.; Itobe, H.; Nakagawa, Y.; Tanabe, T. Thermal Effects on Kerr Comb Generation in a CaF2 Whispering-Gallery Mode Microcavity. IEEE Photonics J. 2016, 8, 1–9. [Google Scholar] [CrossRef]
- Drake, T.E.; Stone, J.R.; Briles, T.C.; Papp, S.B. Thermal decoherence and laser cooling of Kerr microresonator solitons. Nat. Photonics 2020, 14, 480. [Google Scholar] [CrossRef]
- Lei, F.; Ye, Z.; Torres-Company, V. Thermal noise reduction in soliton microcombs via laser self-cooling. Opt. Lett. 2022, 47, 513. [Google Scholar] [CrossRef]
- Lobanov, V.E.; Kondratiev, N.M.; Bilenko, I.A. Thermally induced generation of platicons in optical microresonators. Opt. Lett. 2021, 46, 2380–2383. [Google Scholar] [CrossRef]
- Lim, J.; Savchenkov, A.A.; Dale, E.; Liang, W.; Eliyahu, D.; Ilchenko, V.; Matsko, A.B.; Maleki, L.; Wong, C.W. Chasing the thermodynamical noise limit in whispering-gallery-mode resonators for ultrastable laser frequency stabilization. Nat. Commun. 2017, 8, 8. [Google Scholar] [CrossRef]
- Shi, W.; Zou, C.; Cao, Y.; Liu, J. Influence of thermal effects in microresonators on the locking range in self-injection locking of laser diodes. J. Opt. Soc. Am. B 2023, 40, 874–880. [Google Scholar] [CrossRef]
- Chermoshentsev, D.A.; Shitikov, A.E.; Lonshakov, E.A.; Grechko, G.V.; Sazhina, E.A.; Kondratiev, N.M.; Masalov, A.V.; Bilenko, I.A.; Lvovsky, A.I.; Ulanov, A.E. Dual-laser self-injection locking to an integrated microresonator. Opt. Express 2022, 30, 17094–17105. [Google Scholar] [CrossRef]
- Rahman, A. Temperature sensor based on dielectric optical microresonator. Opt. Fiber Technol. 2011, 17, 536–540. [Google Scholar] [CrossRef]
- Ioppolo, T.; Rubino, E. Room-temperature micro-photonic bolometer based on dielectric optical resonators. In Proceedings of the SPIE 8704, Infrared Technology and Applications XXXIX, Baltimore, MA, USA, 29 April–3 May 2013. [Google Scholar]
- Zhu, J.; Ozdemir, S.K.; Yang, L. Infrared light detection using a whispering-gallery-mode optical microcavity. Appl. Phys. Lett. 2014, 104, 171114. [Google Scholar] [CrossRef]
- Foreman, M.R.; Swaim, J.D.; Vollmer, F. Whispering gallery mode sensors. Adv. Opt. Photon. 2015, 7, 168–240. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Li, J.; Guo, X.; Wan, L.; Liu, J.; Chen, Z.; Pan, J.; Zhang, B.; Li, Z.; Qin, Y. On-chip high-sensitivity photonic temperature sensor based on a GaAs microresonator. Opt. Lett. 2020, 45, 5105–5108. [Google Scholar] [CrossRef]
- Andrianov, A.V.; Marisova, M.P.; Anashkina, E.A. Thermo-Optical Sensitivity of Whispering Gallery Modes in As2S3 Chalcogenide Glass Microresonators. Sensors 2022, 22, 4636. [Google Scholar] [CrossRef] [PubMed]
- Komrakova, S.; An, P.; Kovalyuk, V.; Golikov, A.; Gladush, Y.; Mkrtchyan, A.; Chermoshentsev, D.; Krasnikov, D.; Nasibulin, A.; Goltsman, G. Hybrid Silicon Nitride Photonic Integrated Circuits Covered by Single-Walled Carbon Nanotube Films. Nanomaterials 2023, 13, 2307. [Google Scholar] [CrossRef]
- Little, B.E.; Chu, S.; Absil, P.P.; Hryniewicz, J.V.; Johnson, F.; Seiferth, F.; Gill, D.; Van, V.; King, O.; Trakalo, M. Very high-order microring resonator filters for WDM applications. IEEE Photon. Technol. Lett. 2004, 16, 2263–2265. [Google Scholar] [CrossRef]
- Hu, T.; Wang, W.; Qiu, C.; Yu, P.; Qiu, H.; Zhao, Y.; Jiang, X.; Yang, J. Thermally Tunable Filters Based on Third-Order Microring Resonators for WDM Applications. IEEE Photonics Technol. Lett. 2012, 24, 524–526. [Google Scholar] [CrossRef]
- Ren, Y.; Perron, D.; Aurangozeb, F.; Jiang, Z.; Hossain, M.; Van, V. A Continuously Tunable Silicon Double-Microring Filter With Precise Temperature Tracking. IEEE Photonics J. 2018, 10, 6602310. [Google Scholar] [CrossRef]
- Li, Y.; Abolmaali, F.; Allen, K.W.; Limberopoulos, N.I.; Urbas, A.; Rakovich, Y.; Maslov, A.V.; Astratov, V.N. Whispering gallery mode hybridization in photonic molecules. Laser Photonics Rev. 2017, 11, 1600278. [Google Scholar] [CrossRef]
- Liao, K.; Hu, X.; Gan, T.; Liu, Q.; Wu, Z.; Fan, C.; Feng, X.; Lu, C.; Liu, Y.; Gong, Q. Photonic molecule quantum optics. Adv. Opt. Photon. 2020, 12, 60–134. [Google Scholar] [CrossRef]
- Helgason, Ó.B.; Arteaga-Sierra, F.R.; Ye, Z.; Twayana, K.; Andrekson, P.A.; Karlsson, M.; Schröder, J.; Torres-Company, V. Dissipative solitons in photonic molecules. Nat. Photonics 2021, 15, 305–310. [Google Scholar] [CrossRef]
- Xue, X.; Xuan, Y.; Wang, C.; Wang, P.-H.; Liu, Y.; Niu, B.; Leaird, D.E.; Qi, M.; Weiner, A.M. Thermal tuning of Kerr frequency combs in silicon nitride microring resonators. Opt. Express 2016, 24, 687. [Google Scholar] [CrossRef] [PubMed]
- Joshi, C.; Jang, J.K.; Luke, K.; Ji, X.; Miller, S.A.; Klenner, A.; Okawachi, Y.; Lipson, M.; Gaeta, A.L. Thermally controlled comb generation and soliton modelocking in microresonators. Opt. Lett. 2016, 41, 2565. [Google Scholar] [CrossRef] [PubMed]
- Guo, H.; Karpov, M.; Lucas, E.; Kordts, A.; Pfeiffer, M.H.P.; Brasch, V.; Lihachev, G.; Lobanov, V.E.; Gorodetsky, M.L.; Kippenberg, T.J. Universal dynamics and deterministic switching of dissipative Kerr solitons in optical microresonators. Nat. Phys. 2017, 13, 94. [Google Scholar] [CrossRef]
- Anashkina, E.A.; Marisova, M.P.; Andrianov, A.V. Thermo-Optical Control of Raman Solitons in a Functionalized Silica Microsphere. Micromachines 2022, 13, 1616. [Google Scholar] [CrossRef]
- Li, J.; Wan, S.; Peng, J.-L.; Wang, Z.-Y.; Niu, R.; Zou, C.-L.; Guo, G.-C.; Dong, C.-H. Thermal tuning of mode crossing and the perfect soliton crystal in a Si3N4 microresonator. Opt. Express 2022, 30, 13690–13698. [Google Scholar] [CrossRef]
- Anashkina, E.A.; Marisova, M.P.; Dorofeev, V.V.; Andrianov, A.V. Raman Lasing in a Tellurite Microsphere with Thermo-Optical on/off Switching by an Auxiliary Laser Diode. Micromachines 2023, 14, 1796. [Google Scholar] [CrossRef]
- Wang, J.; Zhu, B.; Hao, Z.; Bo, F.; Wang, X.; Li, Y.; Zhang, G.; Xu, J. Thermo-optic effects in on-chip lithium niobate microdisk resonators. Opt. Express. 2016, 24, 21869–21879. [Google Scholar] [CrossRef]
- Boes, A.; Corcoran, B.; Chang, L.; Bowers, J.; Mitchell, A. Status and Potential of Lithium Niobate on Insulator (LNOI) for Photonic Integrated Circuits. Laser Photonics Rev. 2018, 12, 1700256. [Google Scholar] [CrossRef]
- Lu, C.; Li, H.; Qiu, J.; Zhang, Y.; Liu, S.; Zheng, Y.; Chen, X. Second and cascaded harmonic generation of pulsed laser in a lithium niobate on insulator ridge waveguide. Opt. Express 2022, 30, 1381. [Google Scholar] [CrossRef] [PubMed]
- Ye, F.; Yu, Y.; Xi, X.; Sun, X. Second-Harmonic Generation in Etchless Lithium Niobate Nanophotonic Waveguides with Bound States in the Continuum. Laser Photonics Rev. 2022, 16, 3. [Google Scholar] [CrossRef]
- Griffith, A.; Lau, R.; Cardenas, J.; Okawachi, Y.; Mohanty, A.; Fain, R.; Lee, Y.; Yu, M.; Phare, C.; Poitras, C.; et al. Silicon-chip mid-infrared frequency comb generation. Nat. Commun. 2014, 6, 6299. [Google Scholar] [CrossRef]
- Lin, Q.; Painter, O.J.; Agrawal, G.P. Nonlinear optical phenomena in silicon waveguides: Modeling and applications. Opt. Express. 2007, 15, 16604–16644. [Google Scholar] [CrossRef] [PubMed]
- Desiatov, B.; Goykhman, I.; Levy, U. Demonstration of submicron square-like silicon waveguide using optimized LOCOS process. Opt. Express. 2010, 18, 18592–18597. [Google Scholar] [CrossRef] [PubMed]
- Chang, L.; Xie, W.; Shu, H.; Yang, Q.-F.; Shen, B.; Boes, A.; Peters, J.; Jin, W.; Xiang, C.; Liu, S.; et al. Ultra-efficient frequency comb generation in AlGaAs-on-insulator microresonators. Nat. Commun. 2020, 11, 1331. [Google Scholar] [CrossRef]
- Chang, L.; Boes, A.; Pintus, P.; Xie, W.; Peters, J.D.; Kennedy, M.J.; Jin, W.; Guo, X.-W.; Yu, S.-P.; Papp, S.B.; et al. Low loss (Al)GaAs on an insulator waveguide platform. Opt. Lett. 2019, 44, 4075–4078. [Google Scholar] [CrossRef]
- Scaccabarozzi, L.; Fejer, M.M.; Huo, Y.; Fan, S.; Yu, X.; Harris, J.S. Enhanced second-harmonic generation in AlGaAs/AlxOy tightly confining waveguides and resonant cavities. Opt. Lett. 2006, 31, 3626–3628. [Google Scholar] [CrossRef]
- Pu, M.; Ottaviano, L.; Semenova, E.; Yvind, K. Efficient frequency comb generation in AlGaAs-on-insulator. Optica 2016, 3, 823–826. [Google Scholar] [CrossRef]
- Schneider, K.; Welter, P.; Baumgartner, Y.; Hahn, H.; Czornomaz, L.; Seidler, P. Gallium Phosphide-on-Silicon Dioxide Photonic Devices. J. Light. Technol. 2018, 36, 2994–3002. [Google Scholar] [CrossRef]
- Hegeman, I.; Dijkstra, M.; Segerink, F.B.; Lee, W.; Garcia-Blanco, S.M. Development of low-loss TiO2 waveguides. Opt. Express. 2020, 28, 5982–5990. [Google Scholar] [CrossRef] [PubMed]
- Pfeiffer, M.H.P.; Kordts, A.; Brasch, V.; Zervas, M.; Geiselmann, M.; Jost, J.D.; Kippenberg, T.J. Photonic Damascene process for integrated high-Q microresonator based nonlinear photonics. Optica 2016, 3, 20–25. [Google Scholar] [CrossRef]
- Pfeiffer, M.H.P.; Herkommer, C.; Liu, J.; Morais, T.; Zervas, M.; Geiselmann, M.; Kippenberg, T.J. Photonic Damascene Process for Low-Loss, High-Confinement Silicon Nitride Waveguides. IEEE J. Sel. Top. Quantum Electron. 2018, 24, 1–11. [Google Scholar] [CrossRef]
- Liu, J.; Huang, G.; Wang, R.N.; He, J.; Raja, A.S.; Liu, T.; Engelsen, N.J.; Kippenberg, T.J. High-yield, wafer-scale fabrication of ultralow-loss, dispersion-engineered silicon nitride photonic circuits. Nat. Commun. 2021, 12, 2236–2244. [Google Scholar] [CrossRef] [PubMed]
- Brasch, V.; Chen, Q.-F.; Schiller, S.; Kippenberg, T. Radiation hardness of high-Q silicon nitride microresonators for space compatible integrated optics. Opt. Express 2014, 22, 30786–30794. [Google Scholar] [CrossRef]
- Jin, W.; Yang, Q.-F.; Chang, L.; Shen, B.; Wang, H.; Leal, M.; Wu, L.; Gao, M.; Feshali, A.; Paniccia, M.; et al. Hertz-linewidth semiconductor lasers using CMOS-ready ultra-high-Q microresonators. Nat. Photonics 2021, 15, 346–353. [Google Scholar] [CrossRef]
- Huang, D.; Tran, M.A.; Guo, J.; Peters, J.; Komljenovic, T.; Malik, A.; Morton, P.A.; Bowers, J.E. High-power sub-khz linewidth lasers fully integrated on silicon. Optica 2019, 6, 745–752. [Google Scholar] [CrossRef]
- Pavlov, V.I.; Balakireva, I.V.; Brazhnikov, M.K.; Khatyrev, N.P. Frequency stabilization of diode lasers using lithium tantalate microresonators for laser cooling systems of a rubidium fountain. Alm. Mod. Metrol. 2022, 4, 18–26. [Google Scholar]
- Pfeiffer, M.H.P.; Herkommer, C.; Liu, J.; Guo, H.; Karpov, M.; Lucas, E.; Zervas, M.; Kippenberg, T.J. Octave-spanning dissipative Kerr soliton frequency combs in Si3N4 microresonators. Optica 2017, 4, 684–691. [Google Scholar] [CrossRef]
- Xuan, Y.; Liu, Y.; Varghese, L.T.; Metcalf, A.J.; Xue, X.; Wang, P.-H.; Han, K.; Jaramillo-Villegas, J.A.; Noman, A.A.; Wang, C.; et al. High-Q silicon nitride microresonators exhibiting low-power frequency comb initiation. Optica 2016, 3, 1171–1180. [Google Scholar] [CrossRef]
- Bao, C.; Xuan, Y.; Wang, C.; Jaramillo-Villegas, J.A.; Leaird, D.E.; Qi, M.; Weiner, A.M. Soliton repetition rate in a silicon-nitride microresonator. Opt. Lett. 2017, 42, 759–762. [Google Scholar] [CrossRef]
- Gaeta, A.; Lipson, M.; Kippenberg, T. Photonic-chip-based frequency combs. Nat. Photonics 2019, 13, 158–169. [Google Scholar] [CrossRef]
- Gao, M.; Yang, Q.-F.; Ji, Q.-X.; Wang, H.; Wu, L.; Shen, B.; Liu, J.; Huang, G.; Chang, L.; Xie, W.; et al. Probing material absorption and optical nonlinearity of integrated photonic materials. Nat. Commun. 2022, 13, 3323. [Google Scholar] [CrossRef] [PubMed]
- Pavlov, V.; Kondratiev, N.; Cordette, S.; Lobanov, V. Numerical simulation of pump power-induced heating processes in silicon nitride photonic chips. Proc. SPIE 2023, 12322, 73. [Google Scholar]
- Matsko, A.B.; Savchenkov, A.A.; Yu, N.; Maleki, L. Whispering-gallery-mode resonators as frequency references. I. Fundamental limitations. J. Opt. Soc. Am. 2007, B 24, 1324–1335. [Google Scholar] [CrossRef]
- Ariaga, N.; Longstaff, A.; Fletcher, S.; Pan, W.; Mian, N. Thermal modelling using dynamic mode decomposition for thermal error compensation in the temperature domain. In Proceedings of the Special Interest Group Meeting: Thermal Issues, Aachen, Germany, 26–27 February 2020. [Google Scholar]
- Brasch, V.; Geiselmann, M.; Pfeiffer, M.H.P.; Kippenberg, T.J. Bringing short-lived dissipative Kerr soliton states in microresonators into a steady state. Opt. Express. 2016, 24, 29312–29320. [Google Scholar] [CrossRef]
- Lydiate, J. Modelling and simulation of a thermally induced optical transparency in a dual micro-ring resonator. R. Soc. Open Sci. 2017, 4, 170381. [Google Scholar] [CrossRef]
- Pavlov, V.I.; Khatyrev, N.P.; Kondratiev, N.M.; Bilenko, I.A. Comparison of Methods for Calculating Thermal Frequency Shifts in Integrated Si3N4 Microresonators. In Proceedings of the 2022 International Conference Laser Optics (ICLO), Saint Petersburg, Russia, 20–24 June 2022. [Google Scholar]
- Black, E.D. An introduction to Pound–Drever–Hall laser frequency stabilization. Am. J. Phys. 2001, 69, 79–87. [Google Scholar] [CrossRef]
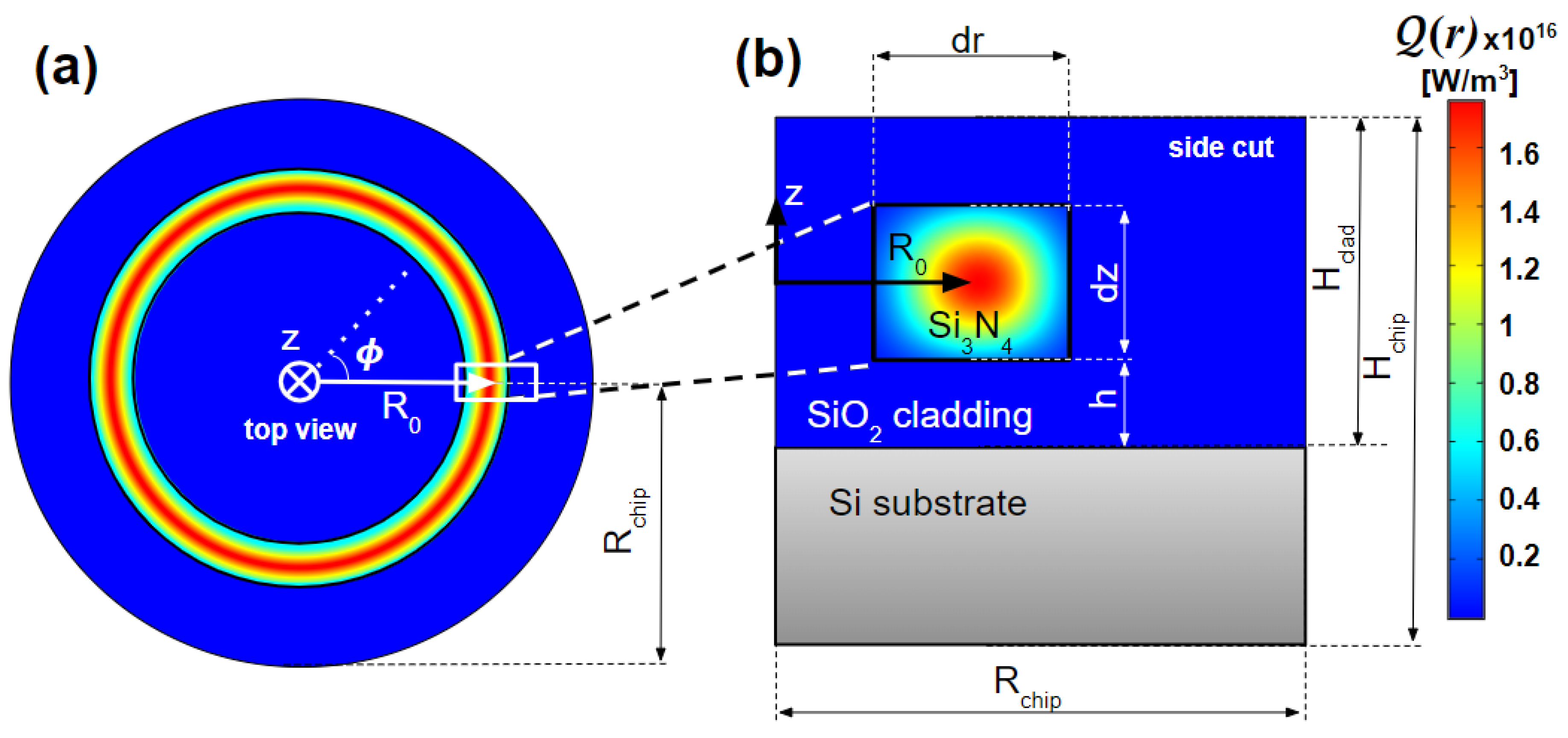
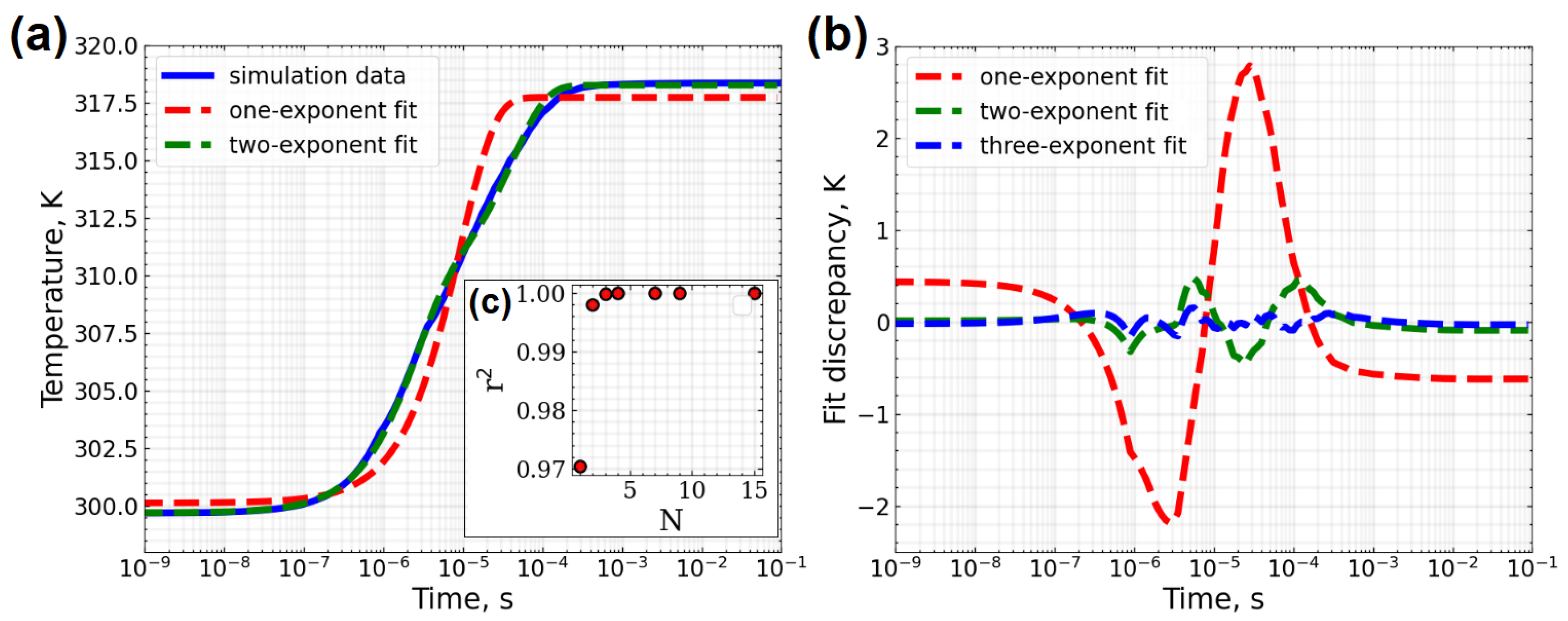
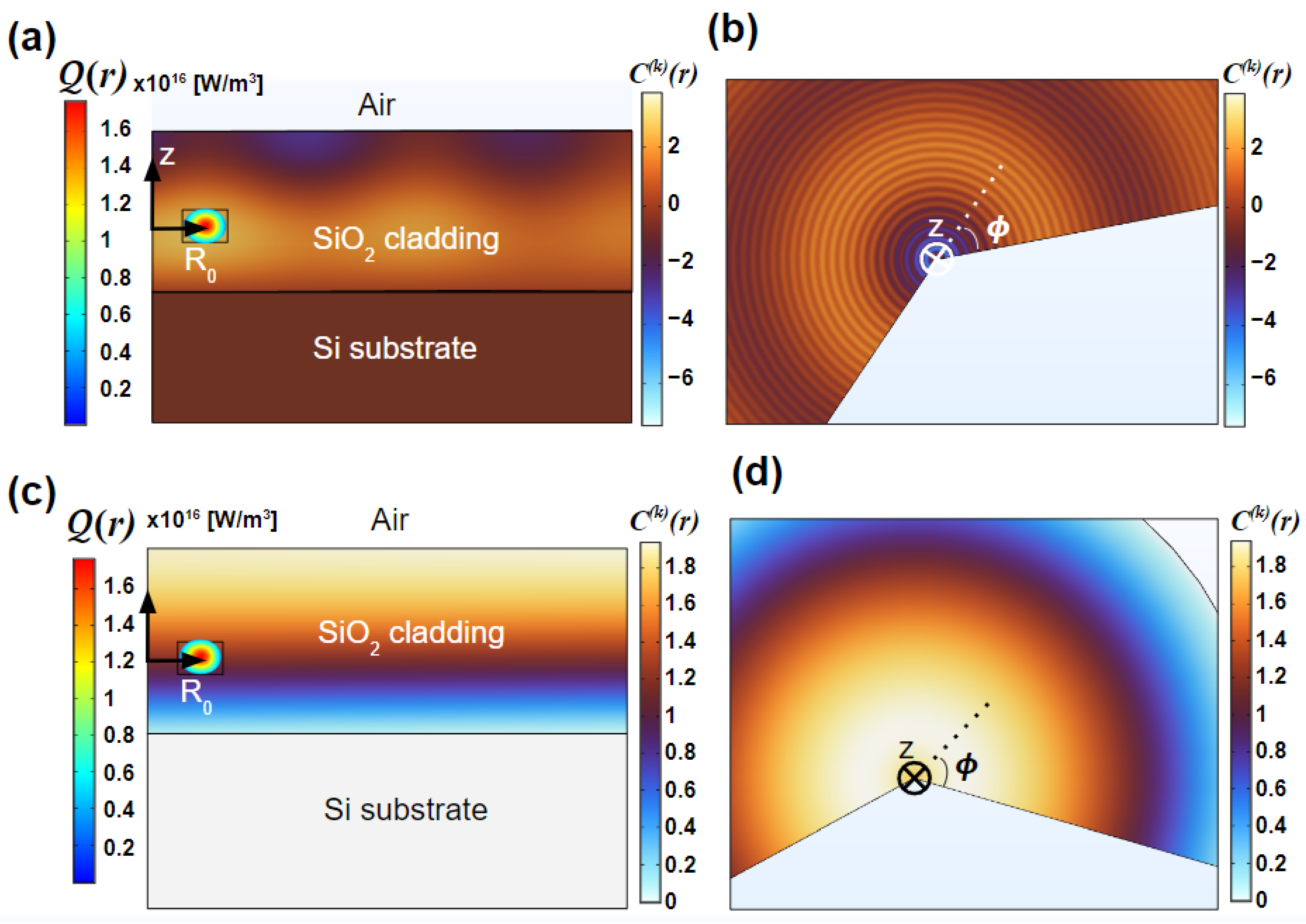

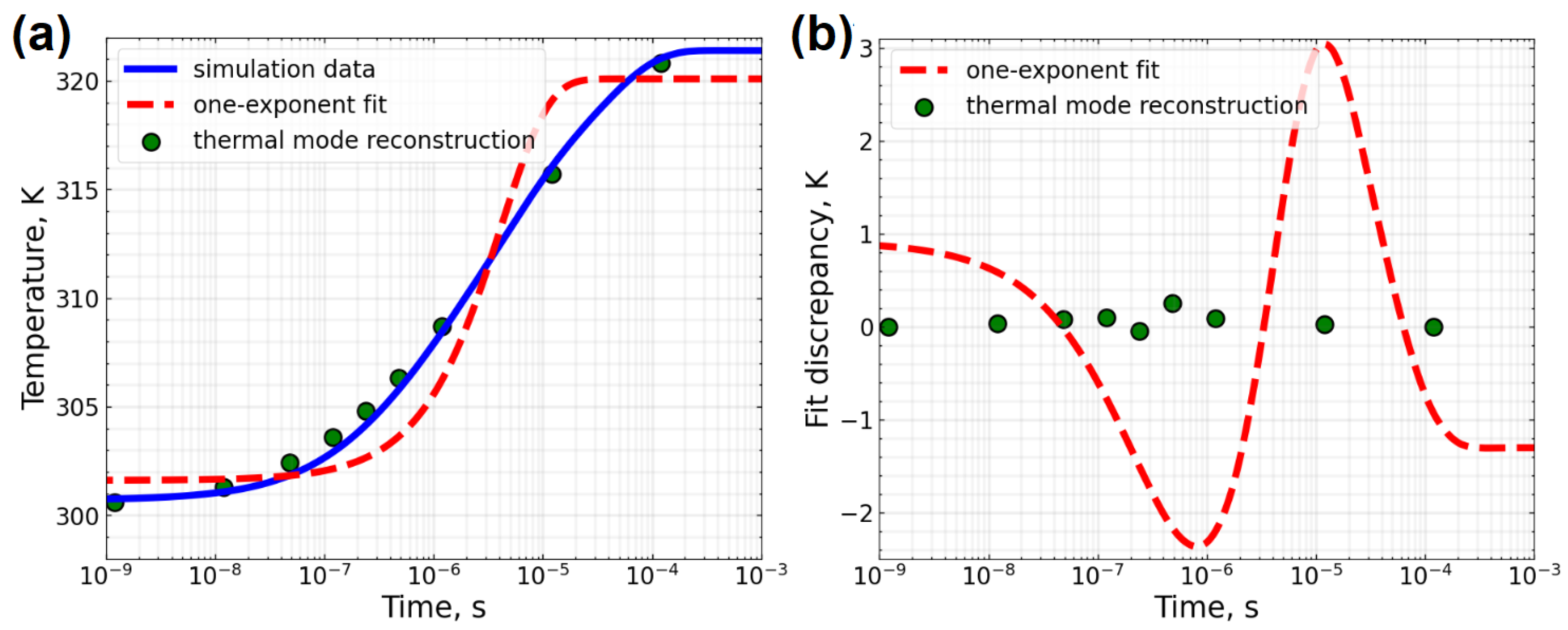


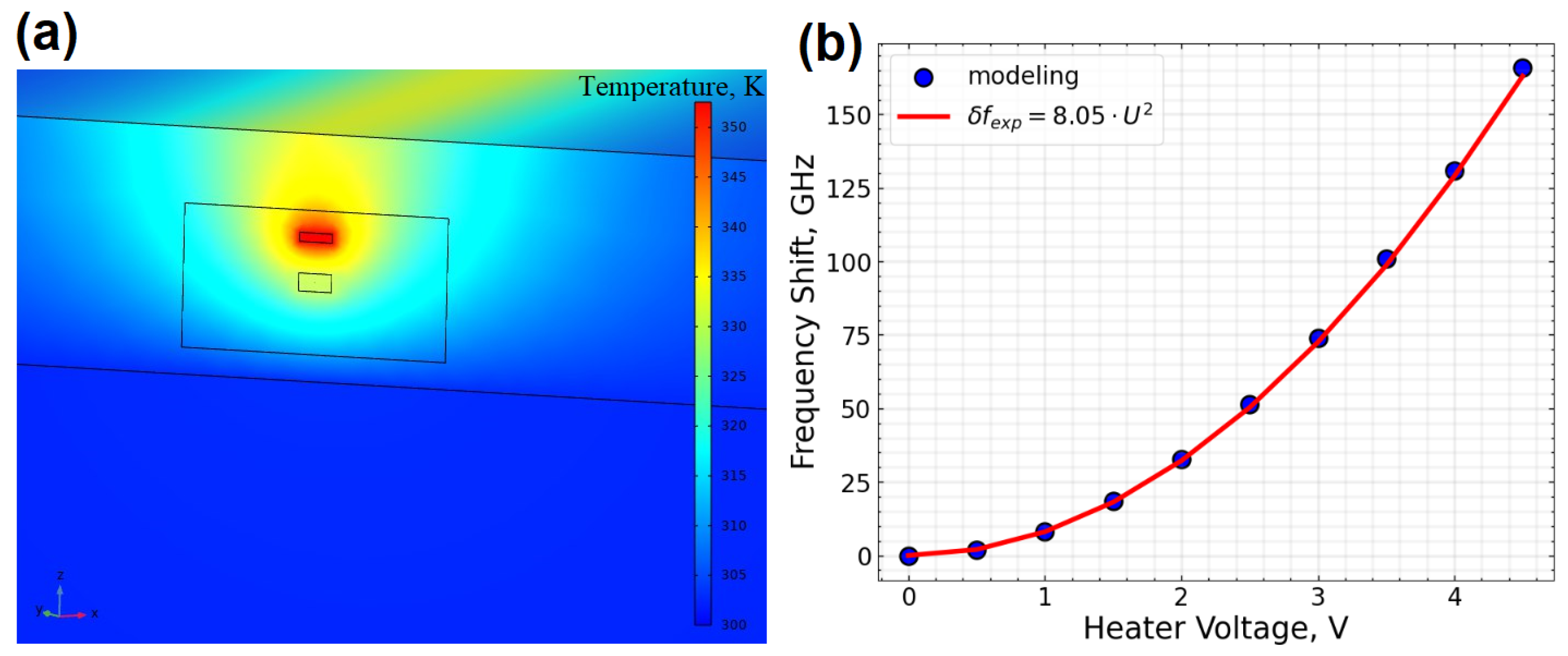
| Parameter | Value |
|---|---|
| Intracavity heating power P | 10 mW |
| Ambient temperature | 300 K |
| Heat transfer coefficient | 10 W/(mK) |
| Microresonator radius R | 24 µm |
| Waveguide width | 1 µm |
| Waveguide height | 0.8 µm |
| Chip height H | 240 µm |
| SiO cladding height H | 11 µm |
| Waveguide-substrate distance h in SiO cladding | 4 µm |
| Chip radius R | 1 mm |
| Physical Properties | SiN | SiO | Si |
|---|---|---|---|
| Density (kg/m) | 3290 | 2200 | 2329 |
| Specific heat capacity (J/(kg · K) | 800 | 730 | 700 |
| Thermal conductivity k (W/(m · K) | 20 | 1.4 | 130 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pavlov, V.I.; Kondratiev, N.M.; Shitikov, A.E.; Lobanov, V.E. Microresonator Effective Thermal Parameters Definition via Thermal Modes Decomposition. Photonics 2023, 10, 1131. https://doi.org/10.3390/photonics10101131
Pavlov VI, Kondratiev NM, Shitikov AE, Lobanov VE. Microresonator Effective Thermal Parameters Definition via Thermal Modes Decomposition. Photonics. 2023; 10(10):1131. https://doi.org/10.3390/photonics10101131
Chicago/Turabian StylePavlov, Vladislav I., Nikita M. Kondratiev, Artem E. Shitikov, and Valery E. Lobanov. 2023. "Microresonator Effective Thermal Parameters Definition via Thermal Modes Decomposition" Photonics 10, no. 10: 1131. https://doi.org/10.3390/photonics10101131
APA StylePavlov, V. I., Kondratiev, N. M., Shitikov, A. E., & Lobanov, V. E. (2023). Microresonator Effective Thermal Parameters Definition via Thermal Modes Decomposition. Photonics, 10(10), 1131. https://doi.org/10.3390/photonics10101131





