Analysis of Error Sources in the Lissajous Scanning Trajectory Based on Two-Dimensional MEMS Mirrors
Abstract
:1. Introduction
2. Methods
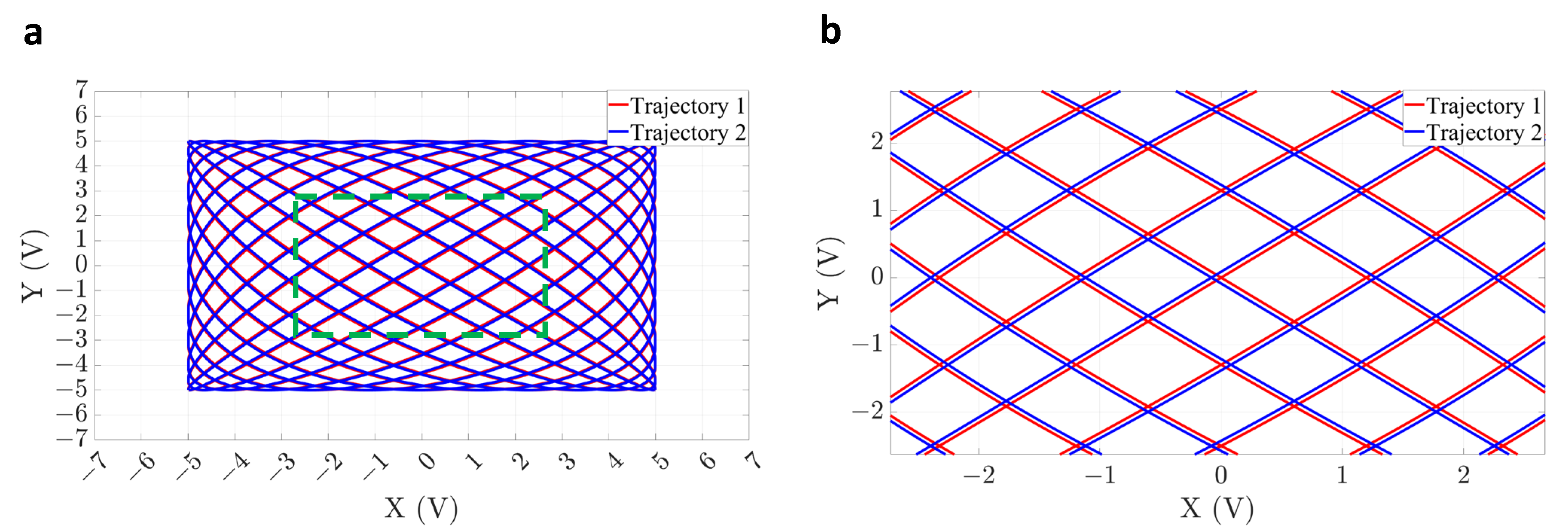
2.1. Lissajous Theory
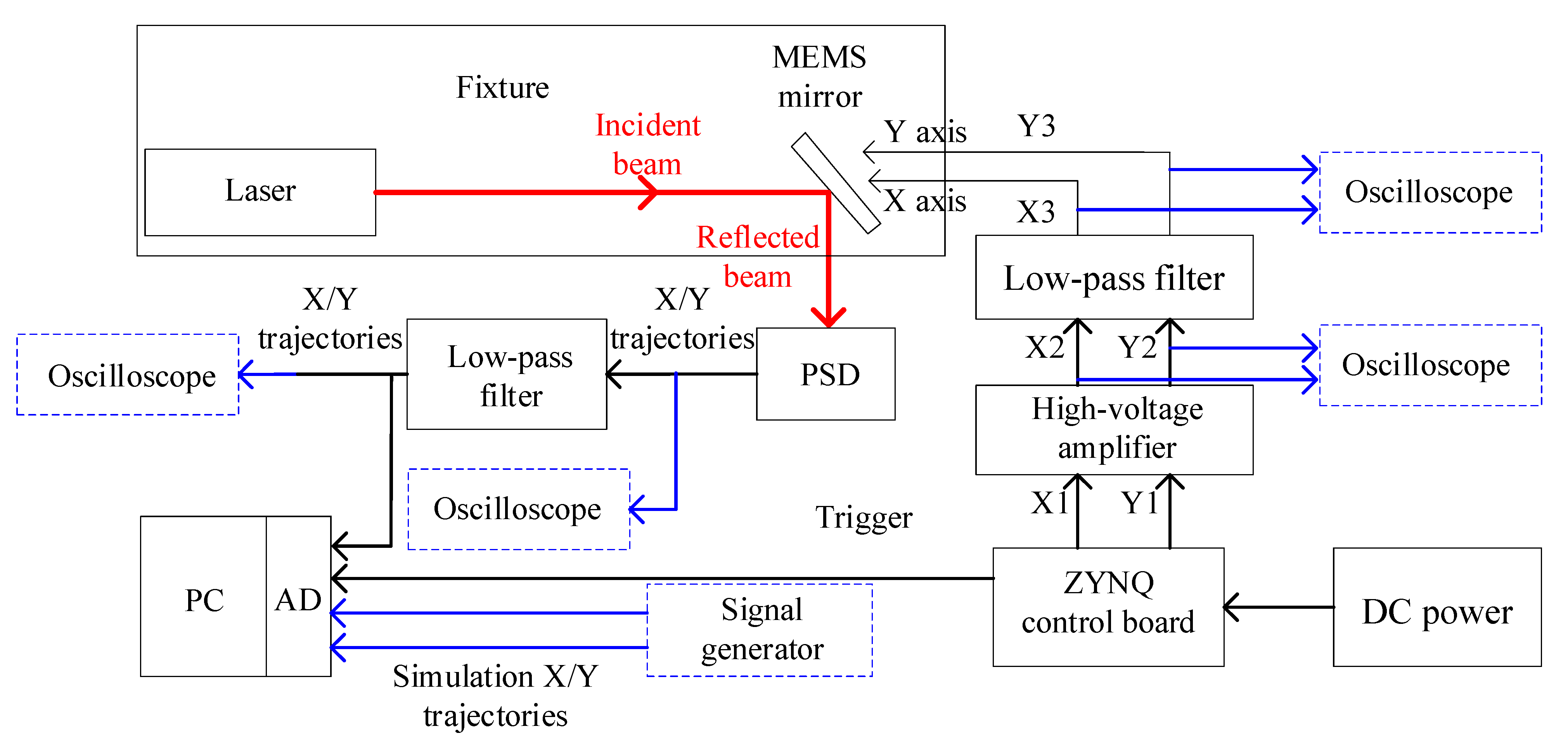
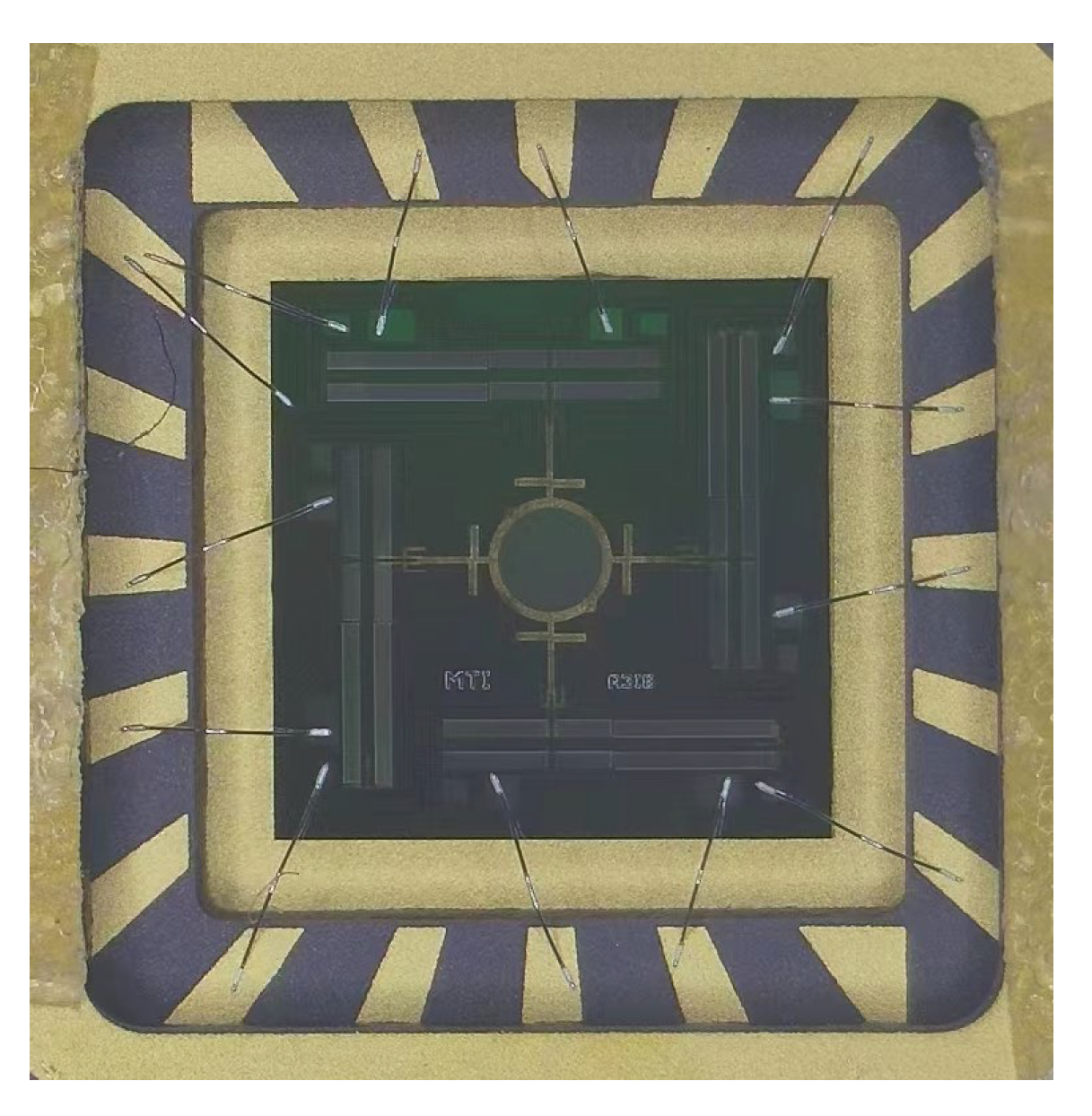
2.2. MEMS Scanning Lissajous Trajectory Test Platform Overview
3. Experimental Validation
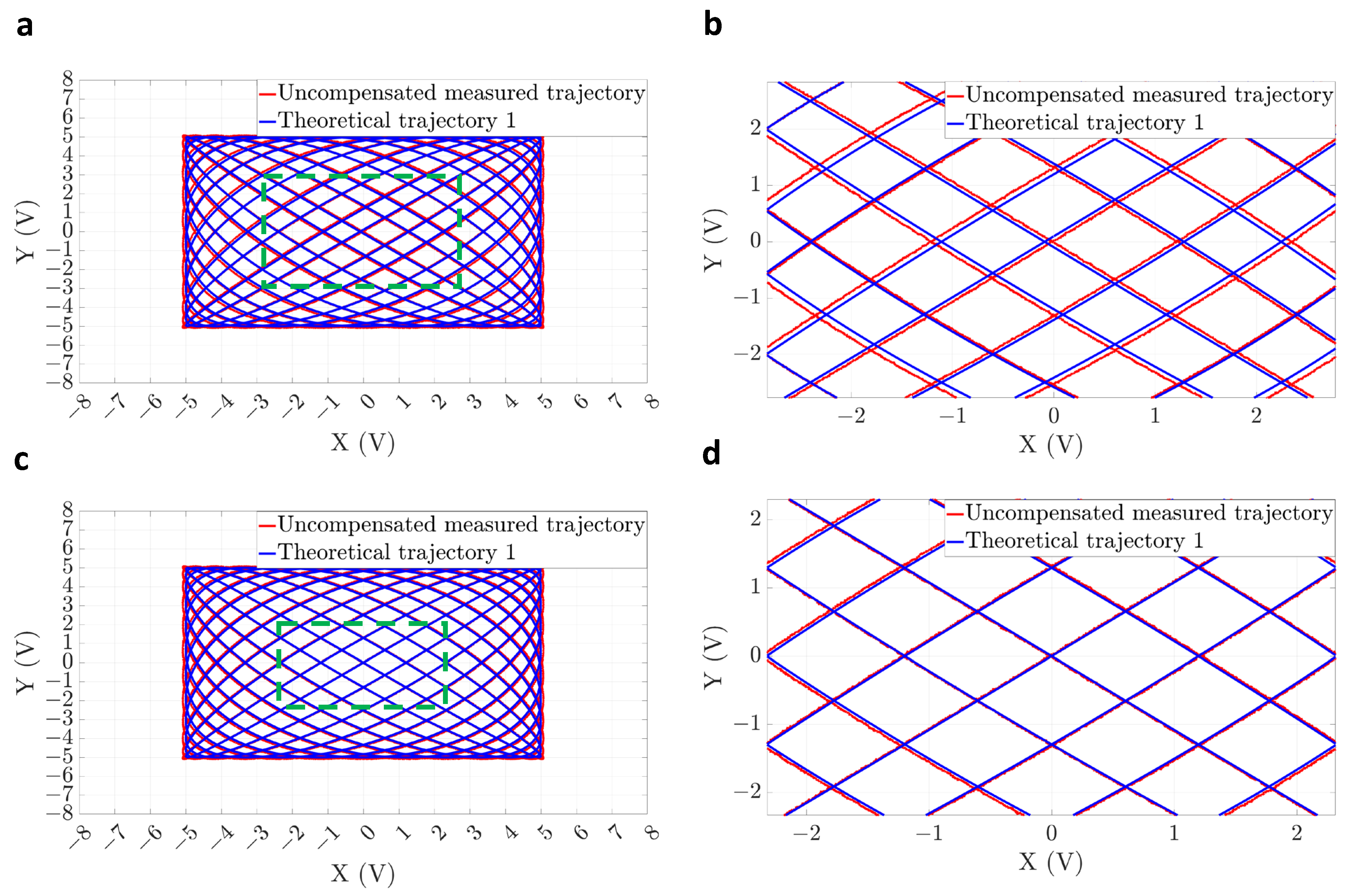
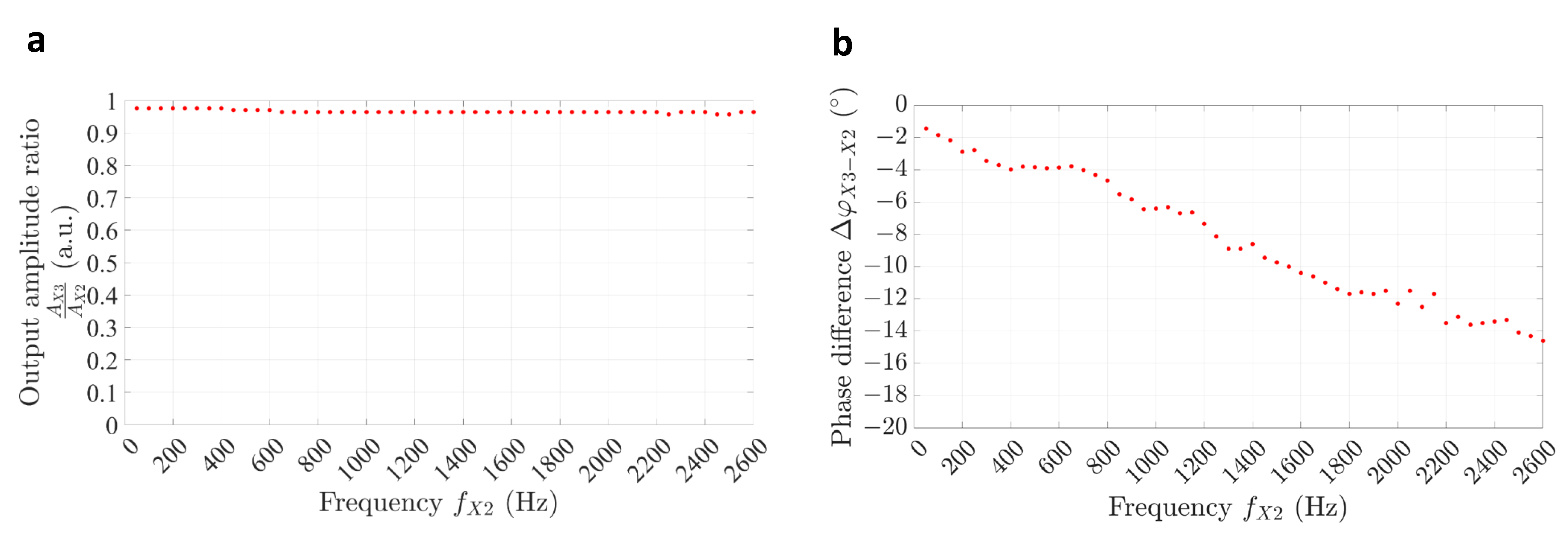
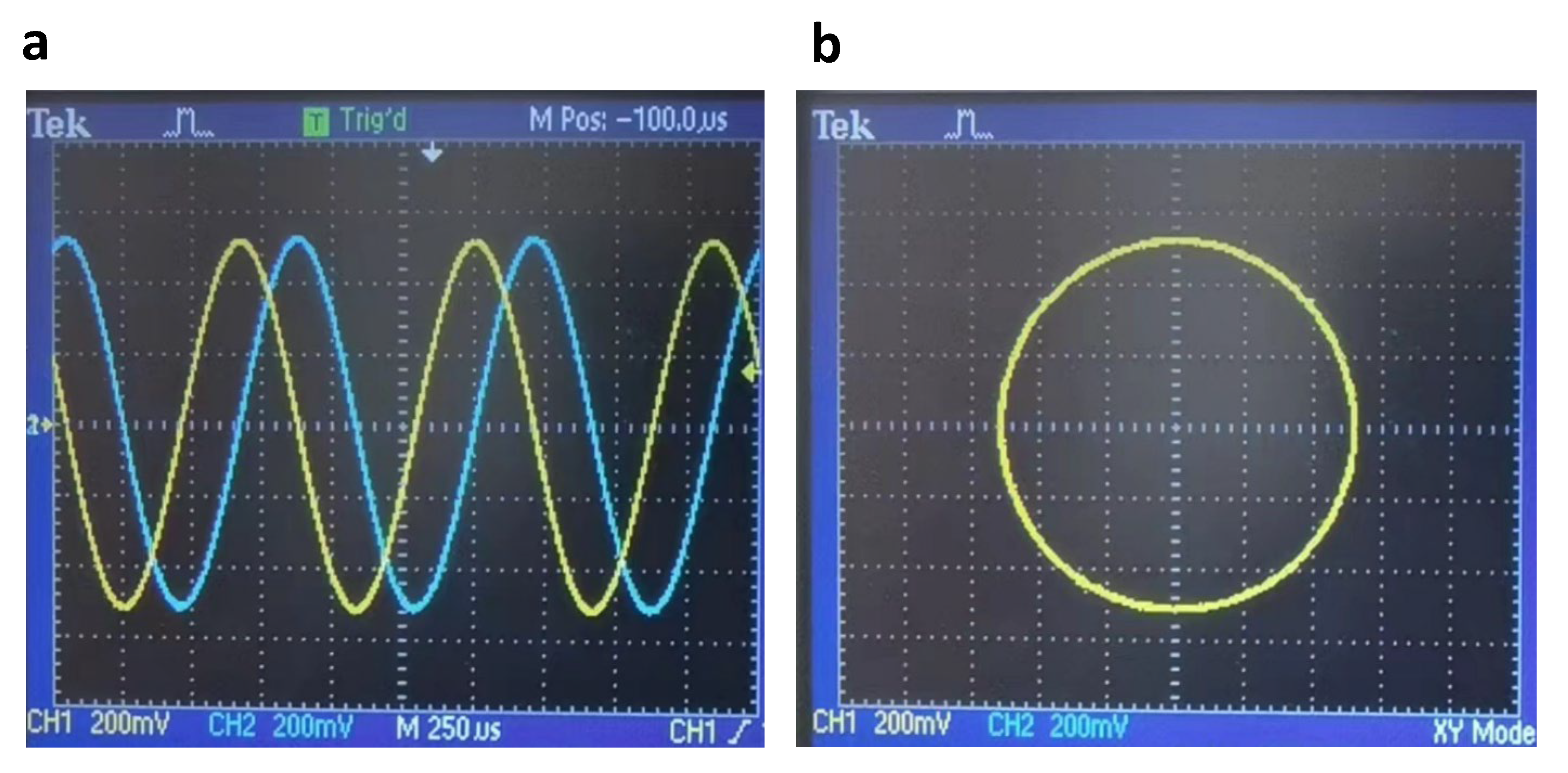
3.1. Frequency Response Error of a Two-Dimensional MEMS Mirror
3.2. AD Acquisition Synchronization Error
3.3. Drive Source Error
3.4. Cross-Coupling Error between the MEMS Mirror Axes
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Holmström, S.T.S.; Baran, U.; Urey, H. MEMS laser scanners: A review. J. Microelectromech. Syst. 2014, 23, 259–275. [Google Scholar] [CrossRef]
- Fujita, T.; Maenaka, K.; Takayama, Y. Dual-axis MEMS mirror for large deflection-angle using SU-8 soft torsion beam. Sens. Actuators. A. 2005, 121, 16–21. [Google Scholar] [CrossRef]
- Piyawattanametha, W.; Wang, T.D. MEMS-based dual-axes confocal microendoscopy. IEEE J. Sel. Top. Quantum Electron. 2009, 16, 804–814. [Google Scholar] [CrossRef] [PubMed]
- Jeon, J.; Kim, H.; Jang, H.; Hwang, K.; Kim, K.; Park, Y.G.; Jeong, K.H. Handheld laser scanning microscope catheter for real-time and in vivo confocal microscopy using a high definition high frame rate Lissajous MEMS mirror. Biomed. Opt. Express 2022, 13, 1497–1505. [Google Scholar] [CrossRef]
- Pan, T.; Gao, X.; Yang, H.; Cao, Y.; Zhao, H.; Chen, Q.; Xie, H. A MEMS mirror-based confocal laser endomicroscope with image distortion correction. IEEE Photonics J. 2023, 15, 3900408. [Google Scholar] [CrossRef]
- Tang, S.; Jung, W.; McCormick, D.; Xie, T.; Su, J.; Ahn, Y.C.; Tromberg, B.J.; Chen, Z. Design and implementation of fiber-based multiphoton endoscopy with microelectromechanical systems scanning. J. Biomed. Opt. 2009, 14, 034005. [Google Scholar] [CrossRef]
- Murugkar, S.; Smith, B.; Srivastava, P.; Moica, A.; Naji, M.; Brideau, C.; Stys, P.K.; Anis, H. Miniaturized multimodal CARS microscope based on MEMS scanning and a single laser source. Opt. Express 2010, 18, 23796–23804. [Google Scholar] [CrossRef]
- Duan, X.; Li, H.; Qiu, Z.; Joshi, B.P.; Pant, A.; Smith, A.; Kurabayashi, K.; Oldham, K.R.; Wang, T.D. MEMS-based multiphoton endomicroscope for repetitive imaging of mouse colon. Biomed. Opt. Express 2015, 6, 3074–3083. [Google Scholar] [CrossRef] [PubMed]
- Klioutchnikov, A.; Wallace, D.J.; Frosz, M.H.; Zeltner, R.; Sawinski, J.; Pawlak, V.; Voit, K.M.; Russell, P.S.J.; Kerr, J.N.D. Three-photon head-mounted microscope for imaging deep cortical layers in freely moving rats. Nat. Methods 2020, 17, 509–513. [Google Scholar] [CrossRef]
- Zong, W.; Wu, R.; Chen, S.; Wu, J.; Wang, H.; Zhao, Z.; Chen, G.; Tu, R.; Wu, D.; Hu, Y.; et al. Miniature two-photon microscopy for enlarged field-of-view, multi-plane and long-term brain imaging. Nat. Methods 2021, 18, 46–49. [Google Scholar] [CrossRef]
- Guo, H.; Song, C.; Xie, H.; Xi, L. Photoacoustic endomicroscopy based on a MEMS scanning mirror. Opt. Lett. 2017, 42, 4615–4618. [Google Scholar] [CrossRef]
- Lee, C.; Kim, J.Y.; Kim, C. Recent progress on photoacoustic imaging enhanced with microelectromechanical systems (MEMS) technologies. Micromachines 2018, 9, 584. [Google Scholar] [CrossRef]
- Li, L.; Liang, X.; Qin, W.; Guo, H.; Qi, W.; Jin, T.; Tang, J.; Xi, L. Double spiral resonant MEMS scanning for ultra-high-speed miniaturized optical microscopy. Optica 2023, 10, 1195–1202. [Google Scholar] [CrossRef]
- Hwang, K.; Seo, Y.H.; Jeong, K.H. Microscanners for optical endomicroscopic applications. Micro Nano Syst. Lett. 2017, 5, 1. [Google Scholar] [CrossRef]
- Liang, W.; Murari, K.; Zhang, Y.; Chen, Y.; Li, M.J.; Li, X. Increased illumination uniformity and reduced photodamage offered by the Lissajous scanning in fiber-optic two-photon endomicroscopy. J. Biomed. Opt. 2012, 17, 021108. [Google Scholar] [CrossRef]
- Kim, D.Y.; Hwang, K.; Ahn, J.; Seo, Y.H.; Kim, J.B.; Lee, S.; Yoon, J.H.; Kong, E.; Jeong, Y.; Jon, S.; et al. Lissajous scanning two-photon endomicroscope for in vivo tissue imaging. Sci. Rep. 2019, 9, 3560. [Google Scholar] [CrossRef]
- Du, W.; Zhang, G.; Ye, L. Image quality analysis and optical performance requirement for micromirror-based Lissajous scanning displays. Sensors 2016, 16, 675. [Google Scholar] [CrossRef]
- Seo, Y.H.; Hwang, K.; Kim, H.; Jeong, K.H. Scanning MEMS mirror for high definition and high frame rate Lissajous patterns. Micromachines 2019, 10, 67. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, G.; You, Z. Design rules for dense and rapid Lissajous scanning. Microsyst. Nanoeng. 2020, 6, 101. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, Y.; Wang, L.; Su, Y.; Zhang, Y.; Yu, Z.; Zhu, W.; Wang, Y.; Wu, Z. Resolution adjustable Lissajous scanning with piezoelectric MEMS mirrors. Opt. Express 2023, 31, 2846–2859. [Google Scholar] [CrossRef]
- Xu, D.; Zhang, F. Parameters of Lissajous graphs. J. Qufu Norm. Univ. 2001, 27, 54–56. [Google Scholar]
- Zhang, X. Analysis of influence of initial phase on Lissajous graph. J. Hubei Normal. Univ. 2000, 20, 56–60. [Google Scholar]
- Wong, L.; Yie, J.; Park, S. Selective Infiltration Damping of MEMS Scanning Mirrors. In Proceedings of the 2018 International Conference on Optical MEMS and Nanophotonics (OMN), Lausanne, Switzerland, 29 July–2 August 2018; pp. 1–2. [Google Scholar]
- Wong, L.; Yie, J.; Park, S. Convex optimization on differential pair of actuation voltages for quasi-Static MEMS Mirrors in scanning applications. In Proceedings of the 22nd International Conference on Control, Automation and Systems, Jeju, Republic of Korea, 27 November–1 December 2022; pp. 592–596. [Google Scholar]
- Tanguy, Q.A.A.; Gaiffe, O.; Passilly, N.; Cote, J.M.; Cabodevila, G.; Bargiel, S.; Lutz, P.; Xie, H.; Gorecki, C. Real-time Lissajous imaging with a low-voltage 2-axis MEMS scanner based on electrothermal actuation. Opt. Express. 2020, 28, 8512–8527. [Google Scholar] [CrossRef]
- Birla, M.; Duan, X.; Li, H.; Lee, M.; Li, G.; Wang, T.; Oldham, K.R. Image processing metrics for phase identification of a multiaxis MEMS scanner used in single pixel imaging. IEEE-ASME Trans. Mechatron. 2021, 26, 1445–1454. [Google Scholar] [CrossRef]
- Chen, H.; Sun, Z.; Sun, W.; Yeow, J.T.W. Twisting sliding mode control of an electro-static MEMS micromirror for a laser scanning system. IEEE/CAA J. Autom. Sinica. 2019, 6, 1060–1067. [Google Scholar] [CrossRef]
- Han, X. Study on the Features of Lissajous’ Figure. J. XinZhou Teach. Univ. 2009, 25, 18–22. [Google Scholar]
- Pologruto, T.A.; Sabatini, B.L.; Svoboda, K. ScanImage: Flexible software for operating laser scanning microscopes. BioMed. Eng. OnLine 2003, 2, 13. [Google Scholar] [CrossRef]
- Frigerio, P.; Molinari, L.; Barbieri, A.; Zamprogno, M.; Mendicino, G.; Boni, N.; Langfelder, G. Nested closed-Loop control of Quasi-Static MEMS scanners with large dynamic range. IEEE Trans. Ind. Electron. 2022, 70, 4217–4225. [Google Scholar] [CrossRef]
- Wang, J.; Li, H.; Tian, G.; Deng, Y.; Liu, Q.; Fu, L. Near-infrared probe-based confocal microendoscope for deep-tissue imaging. Biomed. Opt. Express 2018, 9, 5011–5025. [Google Scholar] [CrossRef]
- Schelinski, U.; Knobbe, J.; Dallmann, H.G.; Grüger, H.; Förster, M.; Scholles, M.; Schwarzenberg, M.; Rieske, R. MEMS Based Laser Scanning Microscope for Endoscopic Use; MOEMS and Miniaturized Systems X; SPIE: San Francisco, CA, USA, 2011; Volume 7930, pp. 23–32. [Google Scholar]
- Li, G.; Duan, X.; Lee, M.; Birla, M.; Chen, J.; Oldham, K.R.; Wang, T.D.; Li, H. Ultra-Compact microsystems-based confocal endomicroscope. IEEE Trans. Med. Imaging. 2017, 36, 1482–1490. [Google Scholar] [CrossRef]
- Tatar, E.; Alper, S.E.; Akin, T. Effect of quadrature error on the performance of a fully-decoupled MEMS gyroscope. In Proceedings of the 24th International Conference on Micro Electro Mechanical Systems (MEMS), Cancun, Mexico, 23–27 January 2011; pp. 569–572. [Google Scholar]
- Weinberg, M.S.; Kourepenis, A. Error sources in in-plane silicon tuning-fork MEMS gyroscopes. J. Microelectromech. Syst. 2006, 15, 479–491. [Google Scholar] [CrossRef]
- Yang, B.; Wang, S.; Li, H.; Huang, L.; Li, K.; Yin, Y. The coupling error analysis of the decoupled silicon micro-gyroscope. In Proceedings of the 5th IEEE International Conference on Nano/Micro Engineered and Molecular Systems, Xiamen, China, 20–23 January 2010; pp. 356–361. [Google Scholar]
- Shi, Q.; Qiu, A.; Su, Y.; Zhu, X. Mechanical coupling error of silicon microgyroscope. Opt. Precis. Eng. 2008, 16, 894–898. [Google Scholar]
- Weinstein, D.; Bhave, S.A.; Tada, M.; Mitarai, S.; Ikeda, K. Mechanical coupling of 2D resonator arrays for MEMS filter applications. In Proceedings of the IEEE International Frequency Control Symposium Joint with the 21st European Frequency and Time Forum, Geneva, Switzerland, 29 May–1 June 2007; pp. 1362–1365. [Google Scholar]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Wang, C.; Han, Y.; Wang, J.; Hu, Y.; Wang, J.; Fu, Q.; Wang, A.; Feng, L.; Hu, X. Analysis of Error Sources in the Lissajous Scanning Trajectory Based on Two-Dimensional MEMS Mirrors. Photonics 2023, 10, 1123. https://doi.org/10.3390/photonics10101123
Zhang X, Wang C, Han Y, Wang J, Hu Y, Wang J, Fu Q, Wang A, Feng L, Hu X. Analysis of Error Sources in the Lissajous Scanning Trajectory Based on Two-Dimensional MEMS Mirrors. Photonics. 2023; 10(10):1123. https://doi.org/10.3390/photonics10101123
Chicago/Turabian StyleZhang, Xiulei, Conghao Wang, Yongxuan Han, Junjie Wang, Yanhui Hu, Jie Wang, Qiang Fu, Aimin Wang, Lishuang Feng, and Xiaoguang Hu. 2023. "Analysis of Error Sources in the Lissajous Scanning Trajectory Based on Two-Dimensional MEMS Mirrors" Photonics 10, no. 10: 1123. https://doi.org/10.3390/photonics10101123
APA StyleZhang, X., Wang, C., Han, Y., Wang, J., Hu, Y., Wang, J., Fu, Q., Wang, A., Feng, L., & Hu, X. (2023). Analysis of Error Sources in the Lissajous Scanning Trajectory Based on Two-Dimensional MEMS Mirrors. Photonics, 10(10), 1123. https://doi.org/10.3390/photonics10101123



