Point-by-Point Induced High Birefringence Polymer Optical Fiber Bragg Grating for Strain Measurement
Abstract
1. Introduction
2. Axial Strain-Sensing Principles
3. FBG Inscription
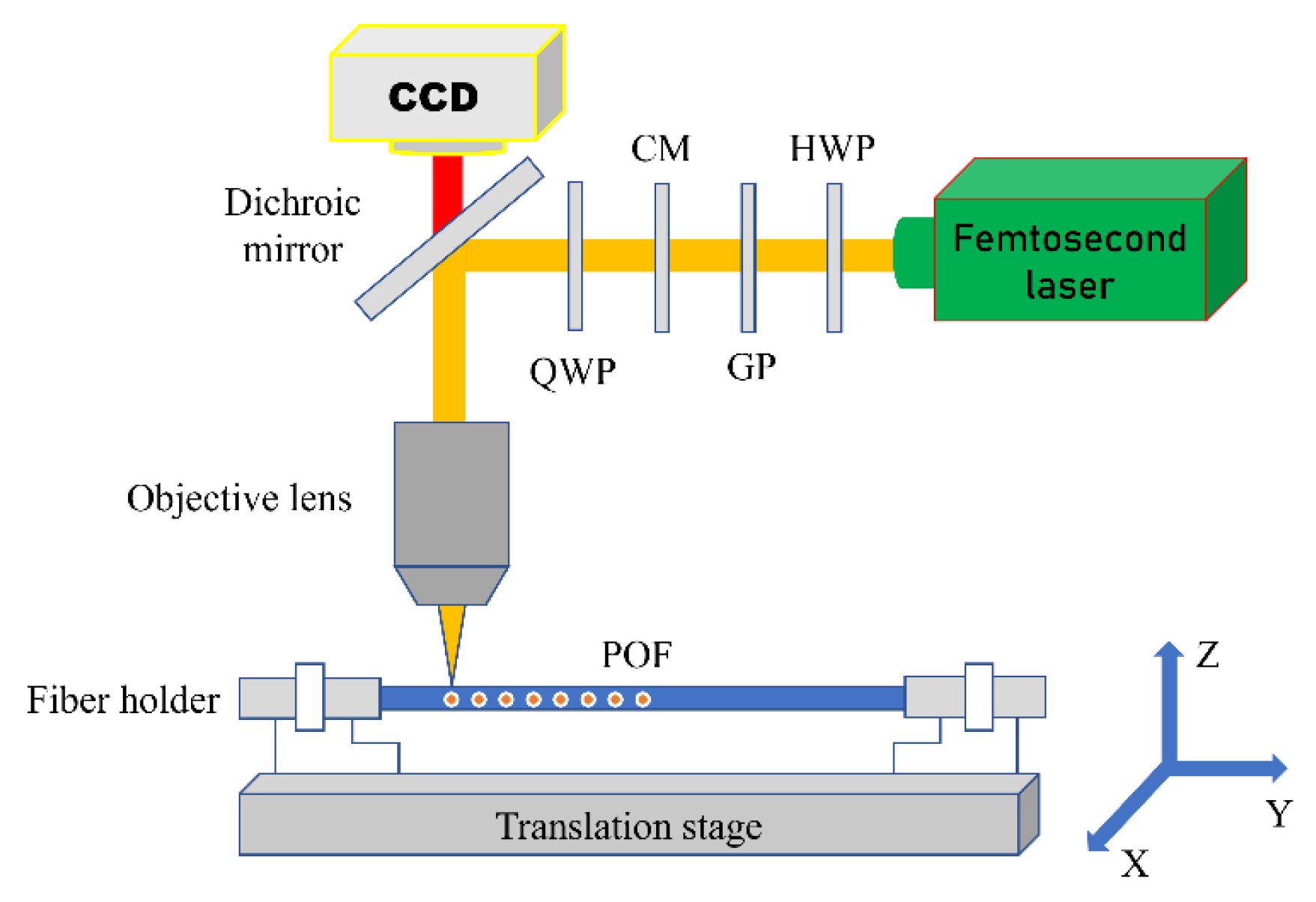
3.1. Experimental Setup
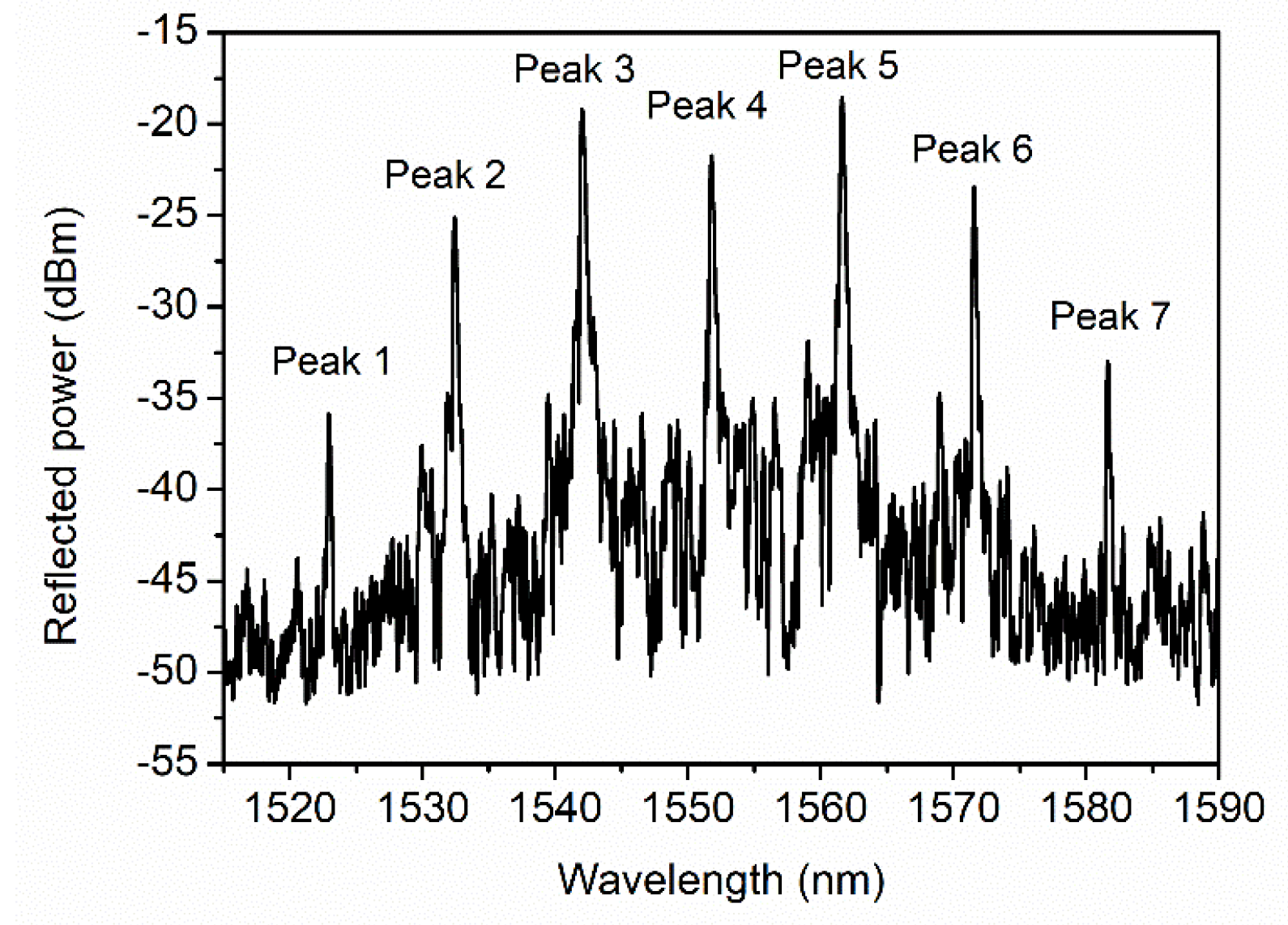
3.2. Experimental Results
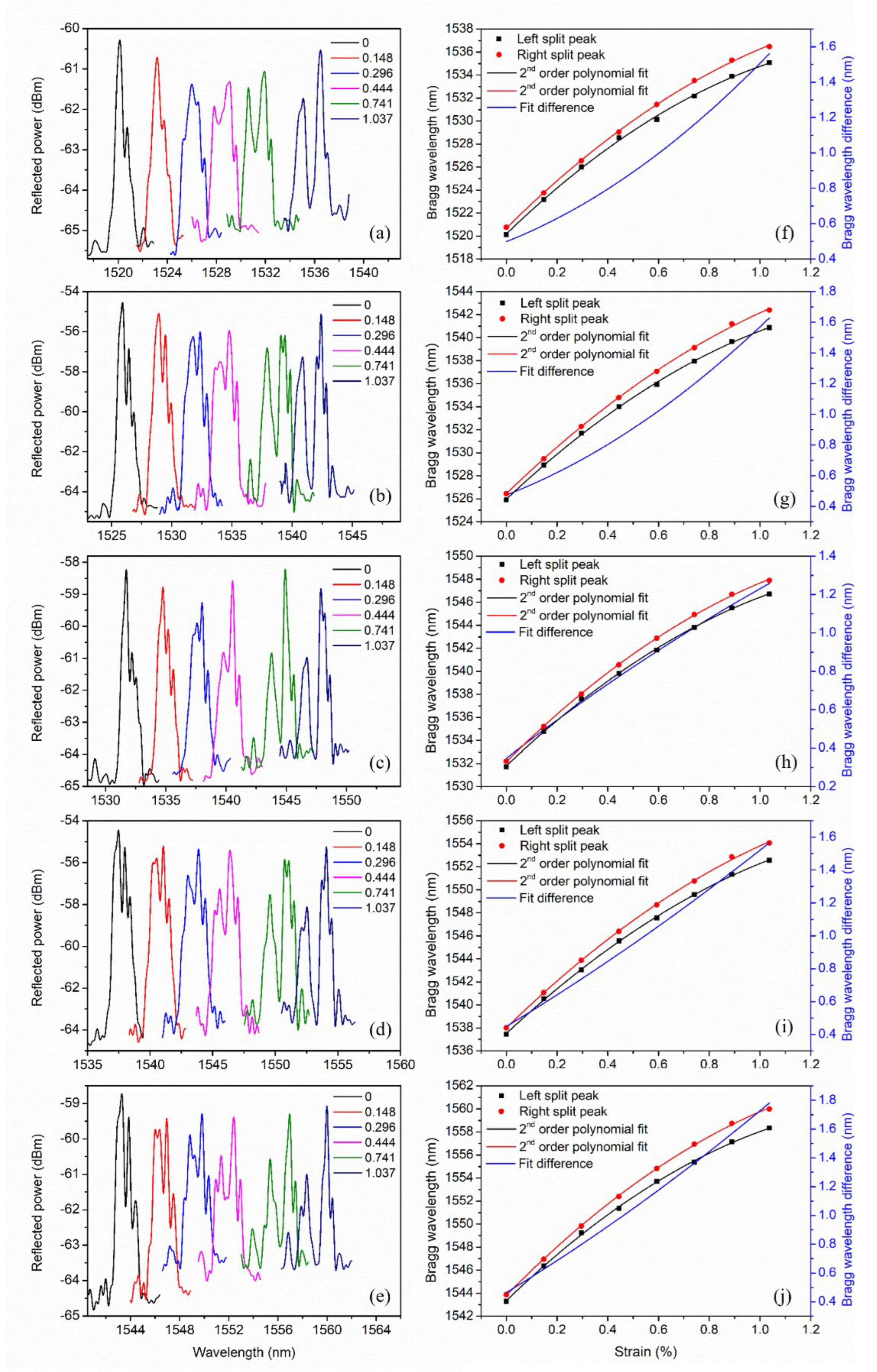
4. Axial Strain Measurements
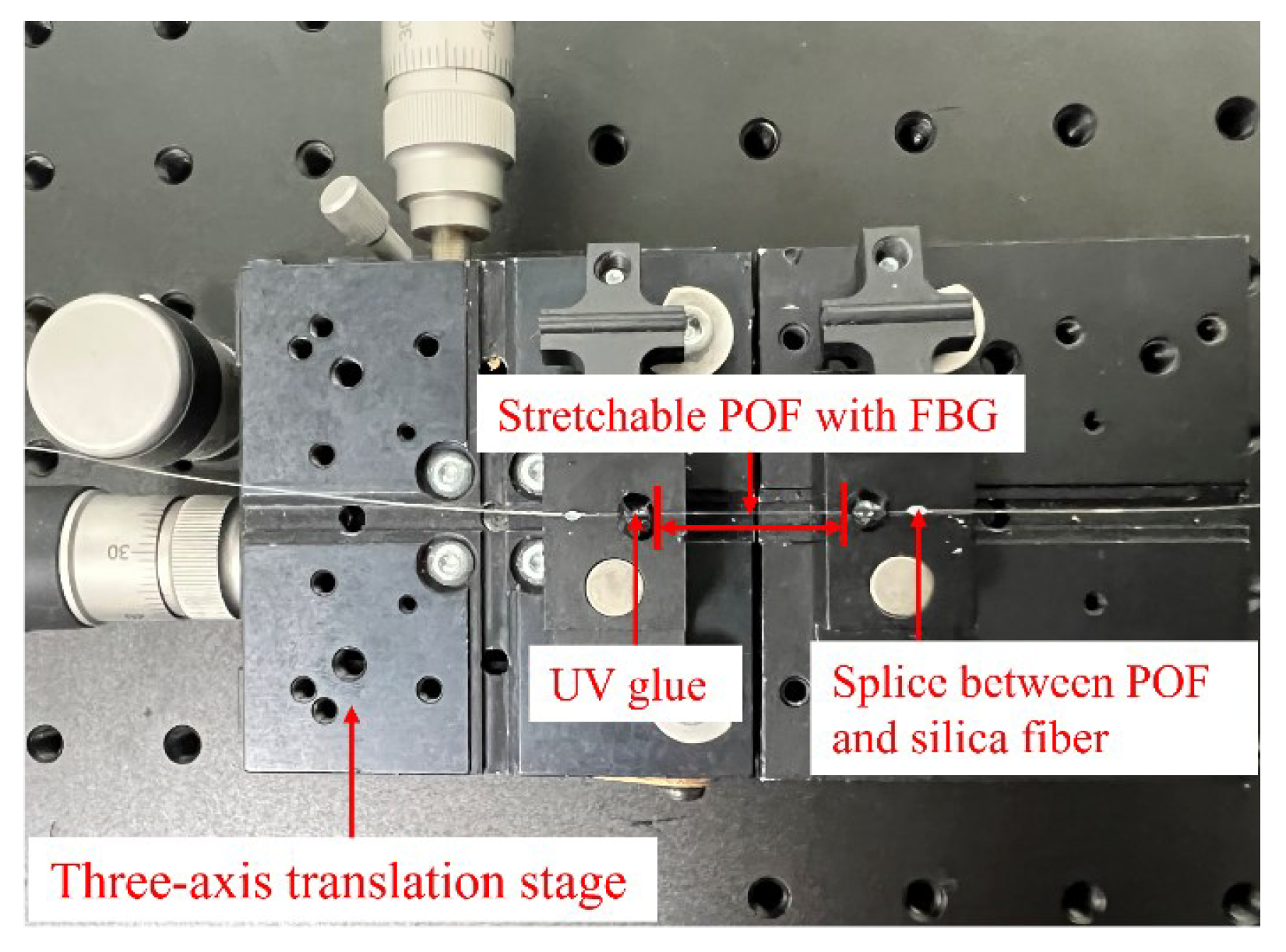
4.1. Experimental Setup
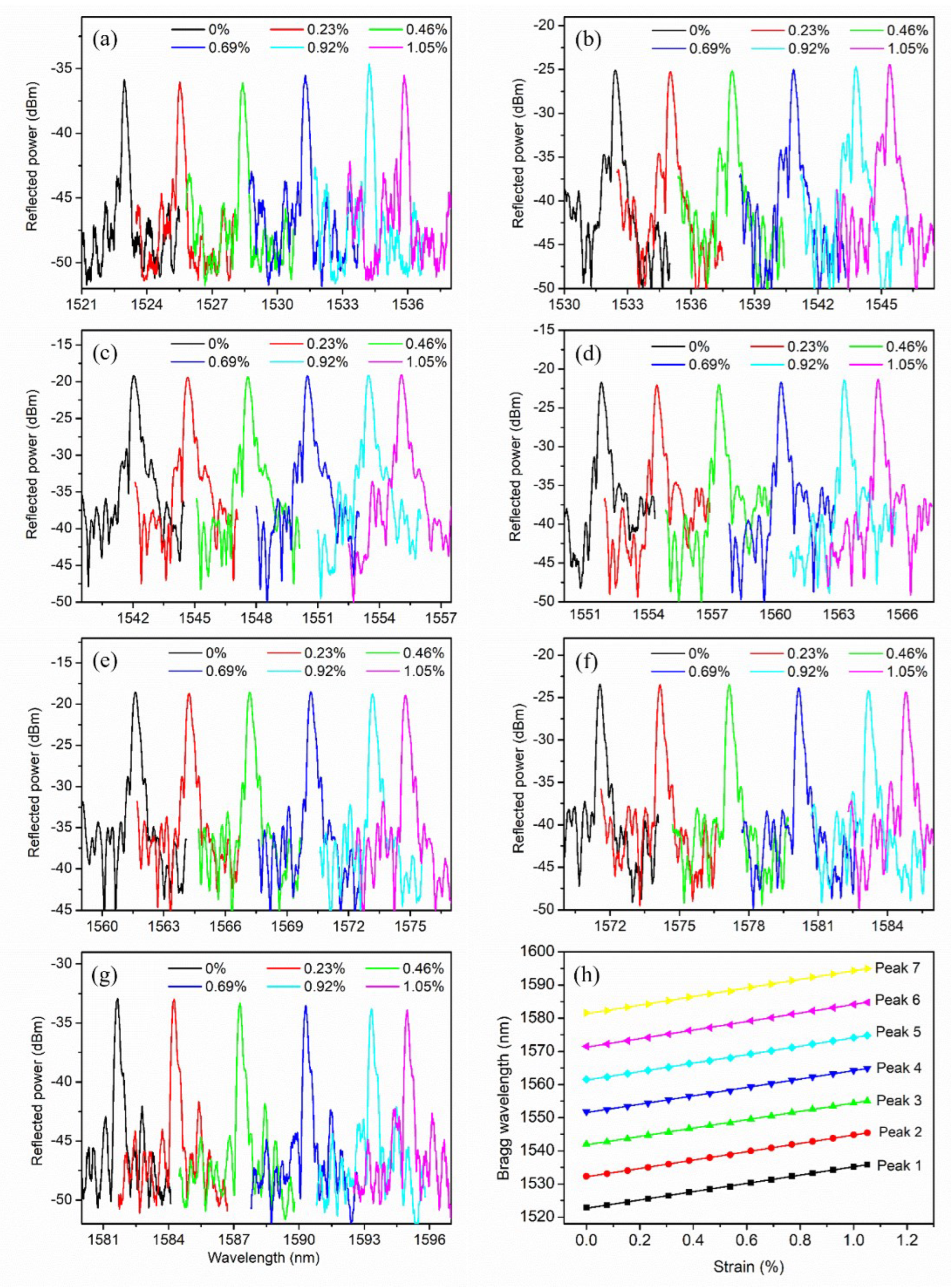
4.2. Experimental Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xiong, Z.; Peng, G.D.; Wu, B.; Chu, P.L. Highly tunable Bragg gratings in single-mode polymer optical fibers. IEEE Photon. Technol. Lett. 1999, 11, 352–354. [Google Scholar] [CrossRef]
- Johnson, I.P.; Yuan, W.; Stefani, A.; Nielsen, K.; Rasmussen, H.K.; Khan, L.; Webb, D.J.; Kalli, K.; Bang, O. Optical fibre Bragg grating recorded in TOPAS cyclic olefin copolymer. Electron. Lett. 2011, 47, 271. [Google Scholar] [CrossRef]
- Nan, Y.-G.; Kinet, D.; Chan, K.; Chapalo, I.; Caucheteur, C.; Megret, P. Ultra-fast fiber Bragg grating inscription in CYTOP polymer optical fibers using phase mask and 400 nm femtosecond laser. Opt. Express. 2021, 29, 25824. [Google Scholar] [CrossRef]
- Theodosiou, A.; Kalli, K. Recent trends and advances of fibre Bragg grating sensors in CYTOP polymer optical fibres. Opt. Fiber Technol. 2020, 54, 102079. [Google Scholar] [CrossRef]
- Woyessa, G.; Fasano, A.; Markos, C.; Stefani, A.; Rasmussen, H.K.; Bang, O. Zeonex microstructured polymer optical fiber: Fabrication friendly fibers for high temperature and humidity insensitive Bragg grating sensing. Opt. Mater. Express. 2017, 7, 286. [Google Scholar] [CrossRef]
- Fasano, A.; Woyessa, G.; Stajanca, P.; Markos, C.; Stefani, A.; Nielsen, K.; Rasmussen, H.K.; Krebber, K.; Bang, O. Fabrication and characterization of polycarbonate microstructured polymer optical fibers for high-temperature-resistant fiber Bragg grating strain sensors. Opt. Mater. Express. 2016, 6, 649–659. [Google Scholar] [CrossRef]
- Webb, D.J. Fibre Bragg grating sensors in polymer optical fibres. Meas. Sci. Technol. 2015, 26, 092004. [Google Scholar] [CrossRef]
- Theodosiou, A.; Lacraz, A.; Stassis, A.; Koutsides, C.; Komodromos, M.; Kalli, K. Plane-by-Plane femtosecond laser inscription method for single-peak Bragg gratings in multimode CYTOP polymer optical fiber. J. Light. Technol. 2017, 35, 5404–5410. [Google Scholar] [CrossRef]
- Beresna, M.; GeceviIus, M.; Kazansky, P.G. Ultrafast laser direct writing and nanostructuring in transparent materials. Adv. Opt. Photon. 2014, 6, 293. [Google Scholar]
- Stefani, A.; Stecher, M.; Town, G.E.; Bang, O. Direct Writing of Fiber Bragg Grating in Microstructured Polymer Optical Fiber. IEEE Photon. Technol. Lett. 2012, 24, 1148–1150. [Google Scholar] [CrossRef]
- Lacraz, A.; Polis, M.; Theodosiou, A.; Koutsides, C.; Kalli, K. Femtosecond Laser Inscribed Bragg Gratings in Low Loss CYTOP Polymer Optical Fiber. IEEE Photonics Technol. Lett. 2015, 27, 693. [Google Scholar] [CrossRef]
- Dash, J.N.; Cheng, X.; Gunawardena, D.S.; Tam, H.-Y. Rectangular single-mode polymer optical fiber for femtosecond laser inscription of FBGs. Photonics Res. 2021, 9, 1931–1938. [Google Scholar]
- Hu, X.; Chen, Z.; Cheng, X.; Min, R.; Qu, H.; Caucheteur, C.; Tam, H.-Y. Femtosecond laser point-by-point Bragg grating inscription in BDK-doped step-index PMMA optical fibers. Opt. Lett. 2022, 47, 249. [Google Scholar] [CrossRef] [PubMed]
- Hu, X.; Chen, Y.; Gao, S.; Min, R.; Woyessa, G.; Bang, O.; Qu, H.; Wang, H.; Caucheteur, C. Direct Bragg grating inscription in single mode step-index TOPAS/ZEONEX polymer optical fiber using 520 nm femtosecond pulses. Polymers. 2022, 14, 1350. [Google Scholar] [CrossRef] [PubMed]
- Abang, A.; Webb, D.J. Influence of mounting on the hysteresis of polymer fiber Bragg grating strain sensors. Opt. Lett. 2013, 38, 1376–1378. [Google Scholar] [CrossRef]
- Rajan, G.; Noor, M.Y.M.; Lovell, N.H.; Ambikaizrajah, E.; Farrell, G.; Peng, G.-D. Polymer micro-fiber Bragg grating. Opt. Lett. 2013, 38, 3359–3362. [Google Scholar] [CrossRef]
- Yuan, W.; Khan, L.; Webb, D.J.; Kalli, K.; Rasmussen, H.K.; Stefani, A.; Bang, O. Humidity insensitive TOPAS polymer fiber Bragg grating sensor. Opt. Express. 2011, 19, 19731–19739. [Google Scholar] [CrossRef]
- Woyessa, G.; Fasano, A.; Stefani, A.; Markos, C.; Nielsen, K.; Rasmussen, H.K.; Bang, O. Single mode step-index polymer optical fiber for humidity insensitive high temperature fiber Bragg grating sensors. Opt. Express. 2016, 24, 1253–1260. [Google Scholar] [CrossRef]
- Stefani, A.; Andresen, S.; Yuan, W.; Herholdt-Rasmussen, N.; Bang, O. High Sensitivity Polymer Optical Fiber-Bragg-Grating-Based Accelerometer. IEEE Photon. Technol. Lett. 2012, 24, 763. [Google Scholar] [CrossRef]
- Oliveira, R.; Marques, T.H.R.; Bilro, L.; Nogueira, R.; Cordeiro, C.M.B. Multiparameter POF sensing based on multimode interference and fiber Bragg grating. J. Light. Technol. 2016, 35, 3–9. [Google Scholar] [CrossRef]
- Pereira, L.; Min, R.; Paixão, T.; Marques, C.; Woyessa, G.; Bang, O.; Pinto, J.L.; Antunes, P.F.d.C. Compact dual-strain sensitivity polymer optical fiber grating for multi-parameter sensing. J. Light. Technol. 2021, 39, 2230. [Google Scholar] [CrossRef]
- Bertholds, A.; Dandliker, R. Determination of the individual strain optic coefficients in single-mode optical fibres. J. Light. Technol. 1988, 6, 17. [Google Scholar] [CrossRef]
- Wang, Y.; Yun, B.; Chen, N.; Cui, Y. Characterization of a high birefringence fibre Bragg grating sensor subjected to nonhomogeneous transverse strain fields. Meas. Sci. Technol. 2006, 17, 939. [Google Scholar] [CrossRef]
- Cao, Y.; Wood, S.; Richheimer, F.; Blakesley, J.; Young, R.J.; Castro, F.A. Enhancing and quantifying spatial homogeneity in monolayer WS2. Sci. Rep. 2021, 11, 14831. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Chen, G.; Ma, P.; Sun, L.; Wu, C.; Guan, B. Sampled Bragg gratings formed in helically twisted fibers and their potential application for the simultaneous measurement of mechanical torsion and temperature. Opt. Express. 2018, 26, 12903–12911. [Google Scholar] [CrossRef]
- Chen, C.; Zhang, X.-Y.; Wei, W.-H.; Chen, Y.-Y.; Qin, L.; Ning, Y.-Q.; Yu, Y.-S. Multi-resonance peaks fiber Bragg gratings based on largely-chirped structure. Opt. Commun. 2018, 412, 150–154. [Google Scholar] [CrossRef]
- Statkiewicz-Barabach, G.; Tarnowski, K.; Kowal, D.; Mergo, P.; Urbanczyk, W. Fabrication of multiple Bragg gratings in microstructured polymer fibers using a phase mask with several diffraction orders. Opt. Express. 2013, 21, 8521–8534. [Google Scholar] [CrossRef]
- Hu, X.; Saez-Rodriguez, D.; Marques, C.; Bang, O.; Webb, D.J.; Mégret, P.; Caucheteur, C. Polarization effects in polymer FBGs: Study and use for transverse force sensing. Opt. Express. 2015, 23, 4581. [Google Scholar] [CrossRef]
- Chah, K.; Kinet, D.; Wuilpart, M.; Mégret, P.; Caucheteur, C. Femtosecond-laser-induced highly birefringent Bragg gratings in standard optical fiber. Opt. Letters. 2013, 38, 594–596. [Google Scholar] [CrossRef]
- Guo, K.; He, J.; Shao, L.; Xu, G.; Wang, Y. Simultaneous measurement of strain and temperature by a sawtooth stressor-assisted highly birefringent fiber Bragg grating. J. Light. Technol. 2019, 38, 2060–2066. [Google Scholar] [CrossRef]
- Oh, S.T.; Han, W.T.; Paek, U.C.; Chung, Y. Discrimination of temperature and strain with a single FBG based on the birefringence effect. Opt. Express 2004, 12, 724–729. [Google Scholar] [CrossRef] [PubMed]
- Huang, T.; Fu, S.; Ke, C.; Shum, P.P.; Liu, D. Characterization of fiber Bragg grating inscribed in few-mode silica-germanate fiber. IEEE Photon. Technol. Lett. 2014, 26, 1908–1911. [Google Scholar] [CrossRef]



| Regime | 1 | 2 | |
|---|---|---|---|
| NA of objective lens | 1.42 | 0.75 | |
| FBG | 1 | 2 | 3 |
| Pulse energy (nJ) | 11.22 | 10.15 | 13.54 |
| Focused-beam diameter (μm) | 0.45 | 0.85 | 0.85 |
| Pulse fluence (J/cm2) | 7.16 | 1.81 | 2.41 |
| Translation velocity (μm/s) | 25.95 | 101 | 100 |
| Grating length (mm) | 2 | 4 | 4 |
| Grating period (μm) Bragg wavelength (nm) | 0.519 1584 | 2.02 1522~1582 | 2 1520~1540 |
| Peak | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| Slope (pm/με) | 1.249 | 1.260 | 1.262 | 1.267 | 1.276 | 1.287 | 1.296 |
| Standard error (pm/με) | 0.008 | 0.007 | 0.008 | 0.008 | 0.008 | 0.008 | 0.008 |
| Peak | 1 | 2 | 3 | 4 | 5 | |
|---|---|---|---|---|---|---|
| Left split peak | Slope (pm/με) | 1.43 | 1.44 | 1.44 | 1.46 | 1.45 |
| Standard error (pm/με) | 0.08 | 0.07 | 0.08 | 0.07 | 0.08 | |
| Right split peak | Slope (pm/με) | 1.54 | 1.55 | 1.53 | 1.56 | 1.57 |
| Standard error (pm/με) | 0.08 | 0.07 | 0.08 | 0.07 | 0.08 | |
| Slope (pm/με) | 0.10 | 0.11 | 0.09 | 0.11 | 0.13 | |
| Standard error (pm/με) | 0.02 | 0.01 | 0.01 | 0.01 | 0.02 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, S.; Wang, H.; Chen, Y.; Wei, H.; Woyessa, G.; Bang, O.; Min, R.; Qu, H.; Caucheteur, C.; Hu, X. Point-by-Point Induced High Birefringence Polymer Optical Fiber Bragg Grating for Strain Measurement. Photonics 2023, 10, 91. https://doi.org/10.3390/photonics10010091
Gao S, Wang H, Chen Y, Wei H, Woyessa G, Bang O, Min R, Qu H, Caucheteur C, Hu X. Point-by-Point Induced High Birefringence Polymer Optical Fiber Bragg Grating for Strain Measurement. Photonics. 2023; 10(1):91. https://doi.org/10.3390/photonics10010091
Chicago/Turabian StyleGao, Shixin, Heng Wang, Yuhang Chen, Heming Wei, Getinet Woyessa, Ole Bang, Rui Min, Hang Qu, Christophe Caucheteur, and Xuehao Hu. 2023. "Point-by-Point Induced High Birefringence Polymer Optical Fiber Bragg Grating for Strain Measurement" Photonics 10, no. 1: 91. https://doi.org/10.3390/photonics10010091
APA StyleGao, S., Wang, H., Chen, Y., Wei, H., Woyessa, G., Bang, O., Min, R., Qu, H., Caucheteur, C., & Hu, X. (2023). Point-by-Point Induced High Birefringence Polymer Optical Fiber Bragg Grating for Strain Measurement. Photonics, 10(1), 91. https://doi.org/10.3390/photonics10010091









