Optimal Control and Computational Method for the Resolution of Isoperimetric Problem in a Discrete-Time SIRS System
Abstract
1. Introduction
2. Materials
- S: the number of susceptible people to infection or who are not yet infected,
- : the number of susceptible people who are temporarily controlled, so they cannot move to the removed class due to the limited effect of control. It can represent the compartment of vaccinated people in case a vaccination is not effective due to the difficulty of producing a perfect vaccine, the heterogeneity of the population or a vaccine not conferring a lifelong immunity [17,27],
- I: the number of infected people who are capable of spreading the epidemic to those in the susceptible and temporarily controlled categories,
- R: the number of removed people from the epidemic, but can return to the susceptible class because of the short-term removal individuals’ immunity.
3. Methods
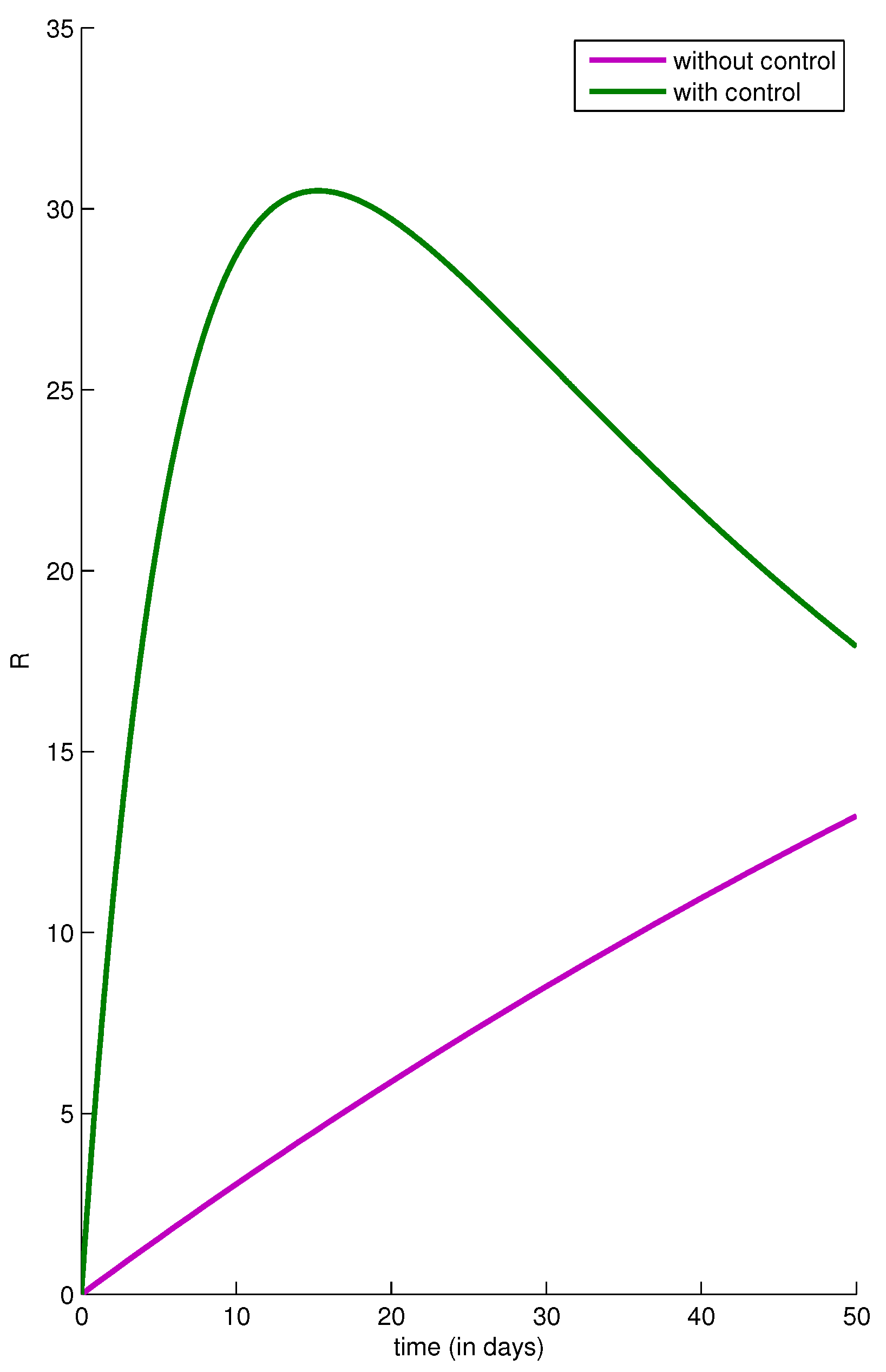
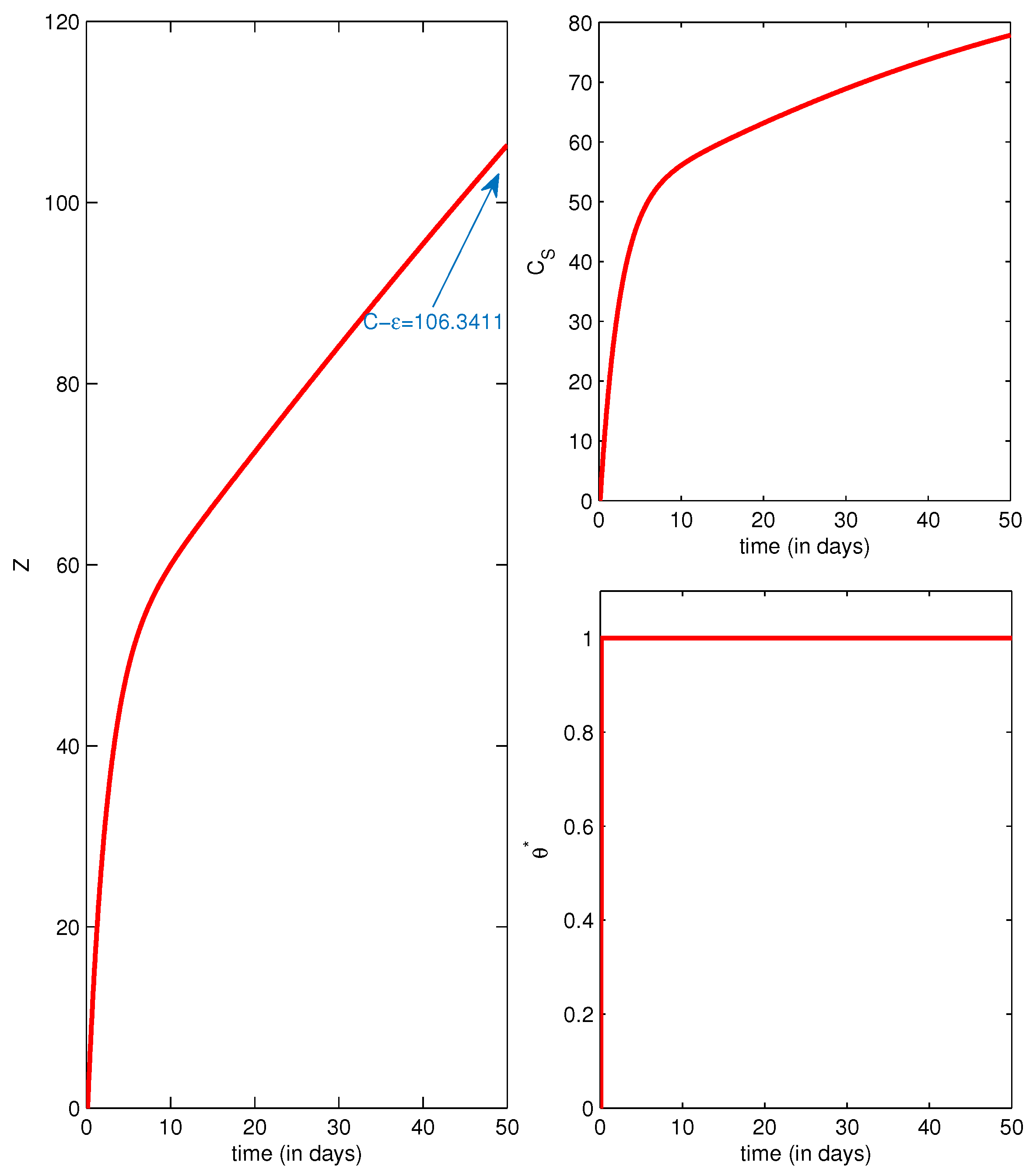
4. Numerical Results and Discussion
| Algorithm 1: Resolution steps of the discrete two-point boundary value optimal control problem (6)–(11). |
| Step 0: Guess an initial estimation of θ. Step 1: Use the initial condition , , , and and the stocked values by θ. Find the optimal states , , , and , which iterate forward in the discrete two-point boundary value problem (6). Step 2: Use the stocked values by θ and the transversality conditions for while searching the constant using the secant-method. More precisely, the secant method is used to obtain the zero of the function where is the value of Z at final iteration N for various values of , and is the value fixed by C. In addition, due its structure in (4), we choose the constant C in a way that it cannot exceed an upper bound where is the initial population size and N is the number of iterations. Find the adjoint variables for , which iterate backward in the discrete two-point boundary value problem (6). Step 3: Update the control utilizing new S, , I, R, Z and for in the characterization of as presented in (12). Step 4: Test the convergence. If the values of the sought variables in this iteration and the final iteration are sufficiently small, check out the recent values as solutions. If the values are not small, go back to Step 1. |
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A
- I: the set
- : the real n-component column vector;
- : the real m-component column control vector;
- : the set of admissible controls
- , , , continuously differentiable functions.
References
- Kermack, W.O.; McKendrick, A.G. A Contribution to the Mathematical Theory of Epidemics. Proc. R. Soc. A 1927, 115, 700–721. [Google Scholar] [CrossRef]
- Acedo, L.; González-Parra, G.; Arenas, A.J. An exact global solution for the classical SIRS epidemic model. Nonlinear Anal. Real World Appl. 2010, 11, 1819–1825. [Google Scholar] [CrossRef]
- Alexander, M.E.; Moghadas, S.M. Bifurcation analysis of an SIRS epidemic model with generalized incidence. SIAM J. Appl. Math. 2005, 65, 1794–1816. [Google Scholar] [CrossRef]
- Hu, Z.; Bi, P.; Ma, W.; Ruan, S. Bifurcations of an SIRS epidemic model with nonlinear incidence rate. Discret. Contin. Dyn. Syst. Ser. B 2011, 15, 93–112. [Google Scholar]
- Teng, Z.; Liu, Y.; Zhang, L. Persistence and extinction of disease in non-autonomous SIRS epidemic models with disease-induced mortality. Nonlinear Anal. Theory Methods Appl. 2008, 69, 2599–2614. [Google Scholar] [CrossRef]
- Xamxinur, A.; Teng, Z. On the persistence and extinction for a non–autonomous SIRS epidemic model. J. Biomath. 2006, 21, 167–176. [Google Scholar]
- Jin, Y.; Wang, W.; Xiao, S. An SIRS model with a nonlinear incidence rate. Chaos Solitons Fractals 2007, 34, 1482–1497. [Google Scholar]
- Liu, J.; Zhou, Y. Global stability of an SIRS epidemic model with transport-related infection. Chaos Solitons Fractals 2009, 40, 145–158. [Google Scholar]
- Chen, J. An SIRS epidemic model. Appl. Math. J. Chin. Univ. 2004, 19, 101–108. [Google Scholar]
- Hu, Z.; Teng, Z.; Jiang, H. Stability analysis in a class of discrete SIRS epidemic models. Nonlinear Anal. Real World Appl. 2012, 13, 2017–2033. [Google Scholar] [CrossRef]
- Mukhopadhyay, B.B.; Tapaswi, P.K. An SIRS epidemic model of Japanese encephalitis. Int. J. Math. Math. Sci. 1994, 17, 347–355. [Google Scholar] [CrossRef]
- Abouelkheir, I.; El Kihal, F.; Rachik, M.; Zakary, O.; Elmouki, I. A multi-regions SIRS discrete epidemic model with a travel-blocking vicinity optimal control approach on cells. Br. J. Math. Comput. Sci. 2017, 20, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Zakary, O.; Rachik, M.; Elmouki, I. On the impact of awareness programs in HIV/AIDS prevention: An SIR model with optimal control. Int. J. Comput. Appl. 2016, 133. [Google Scholar] [CrossRef]
- Zakary, O.; Larrache, A.; Rachik, M.; Elmouki, I. Effect of awareness programs and travel-blocking operations in the control of HIV/AIDS outbreaks: A multi-domains SIR model. Adv. Differ. Equ. 2016, 2016, 169. [Google Scholar] [CrossRef]
- Shim, E. A note on epidemic models with infective immigrants and vaccination. Math. Biosci. Eng. 2006, 3, 557. [Google Scholar] [CrossRef] [PubMed]
- Roy, P.K.; Saha, S.; Al Basir, F. Effect of awareness programs in controlling the disease HIV/AIDS: An optimal control theoretic approach. Adv. Differ. Equ. 2015, 2015, 217. [Google Scholar] [CrossRef]
- Rodrigues, H.S.; Monteiro, M.T.T.; Torres, D.F. Vaccination models and optimal control strategies to dengue. Math. Biosci. 2014, 247, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Kumar, A.; Srivastava, P.K. Vaccination and treatment as control interventions in an infectious disease model with their cost optimization. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 334–343. [Google Scholar] [CrossRef]
- Liu, X.; Takeuchi, Y.; Iwami, S. SVIR epidemic models with vaccination strategies. J. Theor. Biol. 2008, 253, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Nainggolan, J.; Supian, S.; Supriatna, A.K.; Anggriani, N. Mathematical model of tuberculosis transmission with reccurent infection and vaccination. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2013; Volume 423, No. 1; p. 012059. [Google Scholar]
- Zhou, L.; Fan, M. Dynamics of an SIR epidemic model with limited medical resources revisited. Nonlinear Anal. Real World Appl. 2012, 13, 312–324. [Google Scholar] [CrossRef]
- Abdelrazec, A.; Bélair, J.; Shan, C.; Zhu, H. Modeling the spread and control of dengue with limited public health resources. Math. Biosci. 2016, 271, 136–145. [Google Scholar] [CrossRef] [PubMed]
- Yu, T.; Cao, D.; Liu, S. Epidemic model with group mixing: Stability and optimal control based on limited vaccination resources. Commun. Nonlinear Sci. Numer. Simul. 2018, 61, 54–70. [Google Scholar] [CrossRef]
- Neilan, R.M.; Lenhart, S. An Introduction to Optimal Control with an Application in Disease Modeling. In Modeling Paradigms and Analysis of Disease Trasmission Models; American Mathematical Society: Providence, RI, USA, 2010; pp. 67–82. [Google Scholar]
- Elmouki, I.; Saadi, S. BCG immunotherapy optimization on an isoperimetric optimal control problem for the treatment of superficial bladder cancer. Int. J. Dyn. Control 2016, 4, 339–345. [Google Scholar] [CrossRef]
- Alkama, M.; Rachik, M.; Elmouki, I. A discrete isoperimetric optimal control approach for BCG immunotherapy in superficial bladder cancer: Discussions on results of different optimal doses. Int. J. Appl. Comput. Math. 2017, 3, 1–18. [Google Scholar] [CrossRef]
- Sharomi, O.; Malik, T. Optimal control in epidemiology. Ann. Oper. Res. 2017, 251, 55–71. [Google Scholar] [CrossRef]
- Kornienko, I.; Paiva, L.T.; De Pinho, M.D.R. Introducing state constraints in optimal control for health problems. Procedia Technol. 2014, 17, 415–422. [Google Scholar] [CrossRef]
- De Pinho, M.D.R.; Kornienko, I.; Maurer, H. Optimal control of a SEIR model with mixed constraints and L 1 cost. In Proceedings of the 11th Portuguese Conference on Automatic Control, CONTROLO’2014, Porto, Portugal, 21–23 July 2014; Springer: Cham, Switzerland, 2015; pp. 135–145. [Google Scholar]
- Zakary, O.; Rachik, M.; Elmouki, I. On the analysis of a multi-regions discrete SIR epidemic model: An optimal control approach. Int. J. Dyn. Control 2017, 5, 917–930. [Google Scholar] [CrossRef]
- Zakary, O.; Rachik, M.; Elmouki, I.; Lazaiz, S. A multi-regions discrete-time epidemic model with a travel-blocking vicinity optimal control approach on patches. Adv. Differ. Equ. 2017, 2017, 120. [Google Scholar] [CrossRef]
- Zakary, O.; Rachik, M.; Elmouki, I. A new epidemic modeling approach: Multi-regions discrete-time model with travel-blocking vicinity optimal control strategy. Infect. Dis. Model. 2017, 2, 304–322. [Google Scholar] [CrossRef] [PubMed]
- Lenhart, S.; Workman, J.T. Optimal Control Applied to Biological Models; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Sethi, S.P.; Thompson, G.L. What Is Optimal Control Theory? Springer: New York, NY, USA, 2000; pp. 1–22. [Google Scholar]

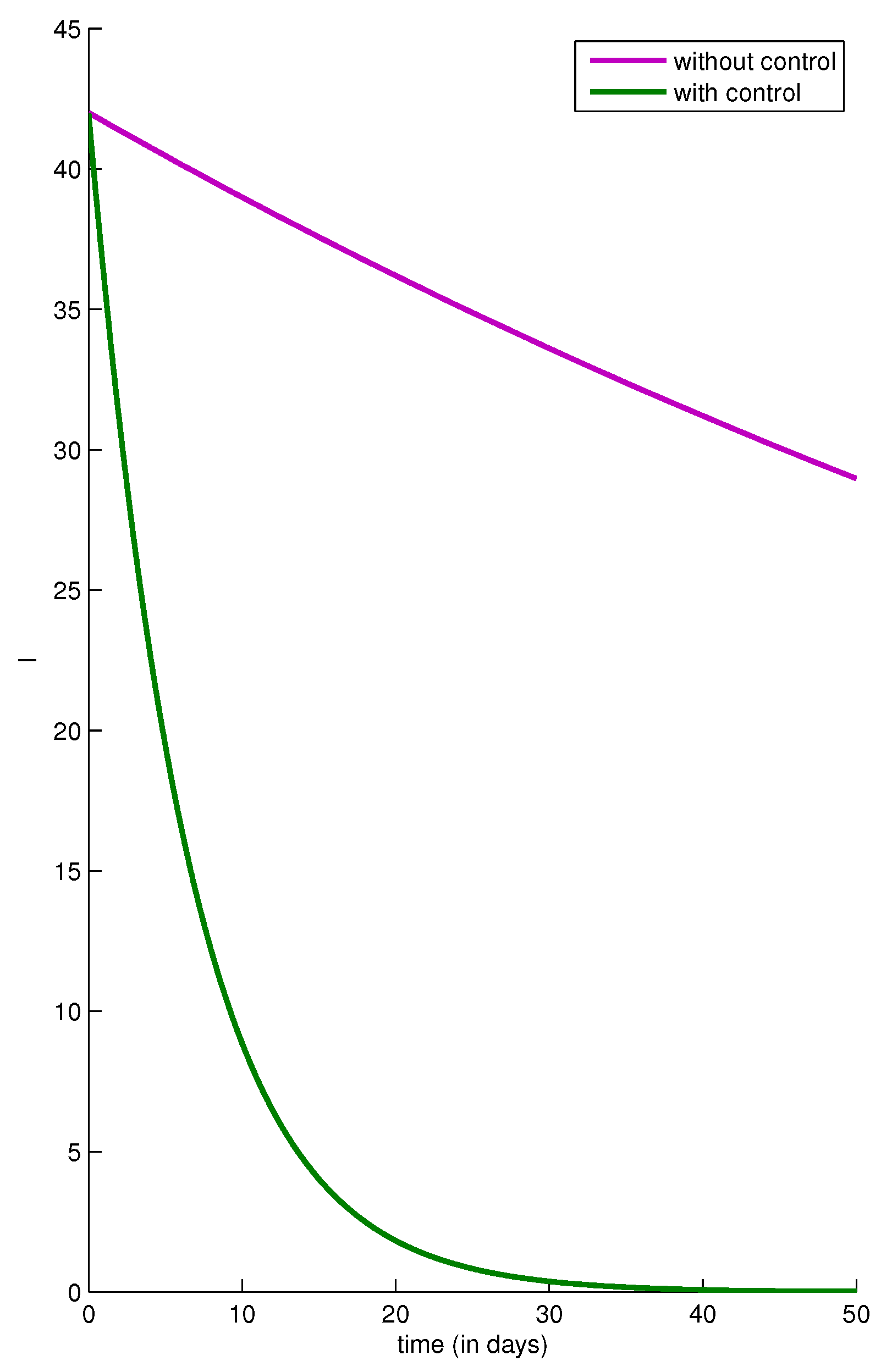
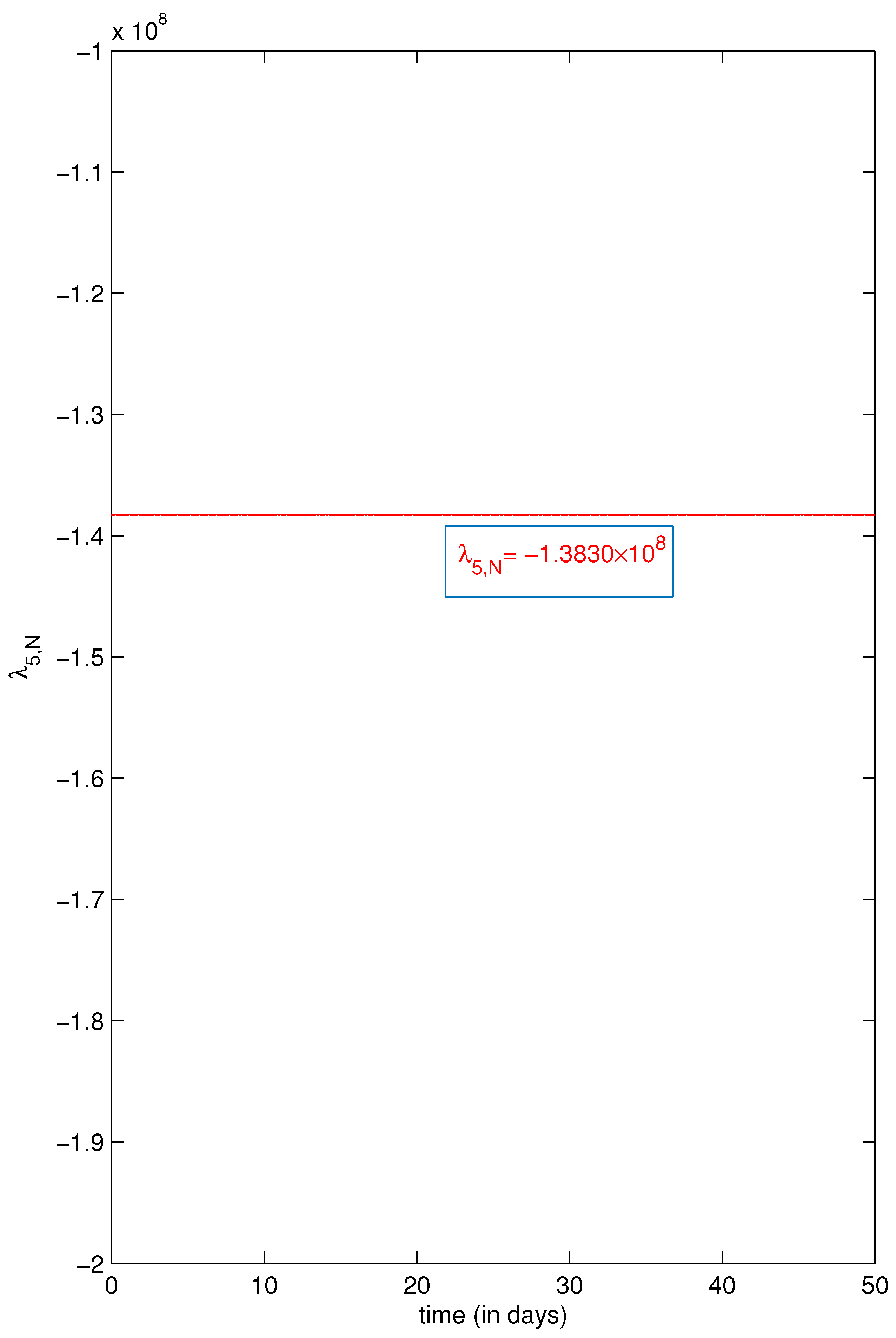

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
El Kihal, F.; Abouelkheir, I.; Rachik, M.; Elmouki, I. Optimal Control and Computational Method for the Resolution of Isoperimetric Problem in a Discrete-Time SIRS System. Math. Comput. Appl. 2018, 23, 52. https://doi.org/10.3390/mca23040052
El Kihal F, Abouelkheir I, Rachik M, Elmouki I. Optimal Control and Computational Method for the Resolution of Isoperimetric Problem in a Discrete-Time SIRS System. Mathematical and Computational Applications. 2018; 23(4):52. https://doi.org/10.3390/mca23040052
Chicago/Turabian StyleEl Kihal, Fadwa, Imane Abouelkheir, Mostafa Rachik, and Ilias Elmouki. 2018. "Optimal Control and Computational Method for the Resolution of Isoperimetric Problem in a Discrete-Time SIRS System" Mathematical and Computational Applications 23, no. 4: 52. https://doi.org/10.3390/mca23040052
APA StyleEl Kihal, F., Abouelkheir, I., Rachik, M., & Elmouki, I. (2018). Optimal Control and Computational Method for the Resolution of Isoperimetric Problem in a Discrete-Time SIRS System. Mathematical and Computational Applications, 23(4), 52. https://doi.org/10.3390/mca23040052




