Process Intensification of Gas–Liquid Separations Using Packed Beds: A Review
Abstract
1. Introduction
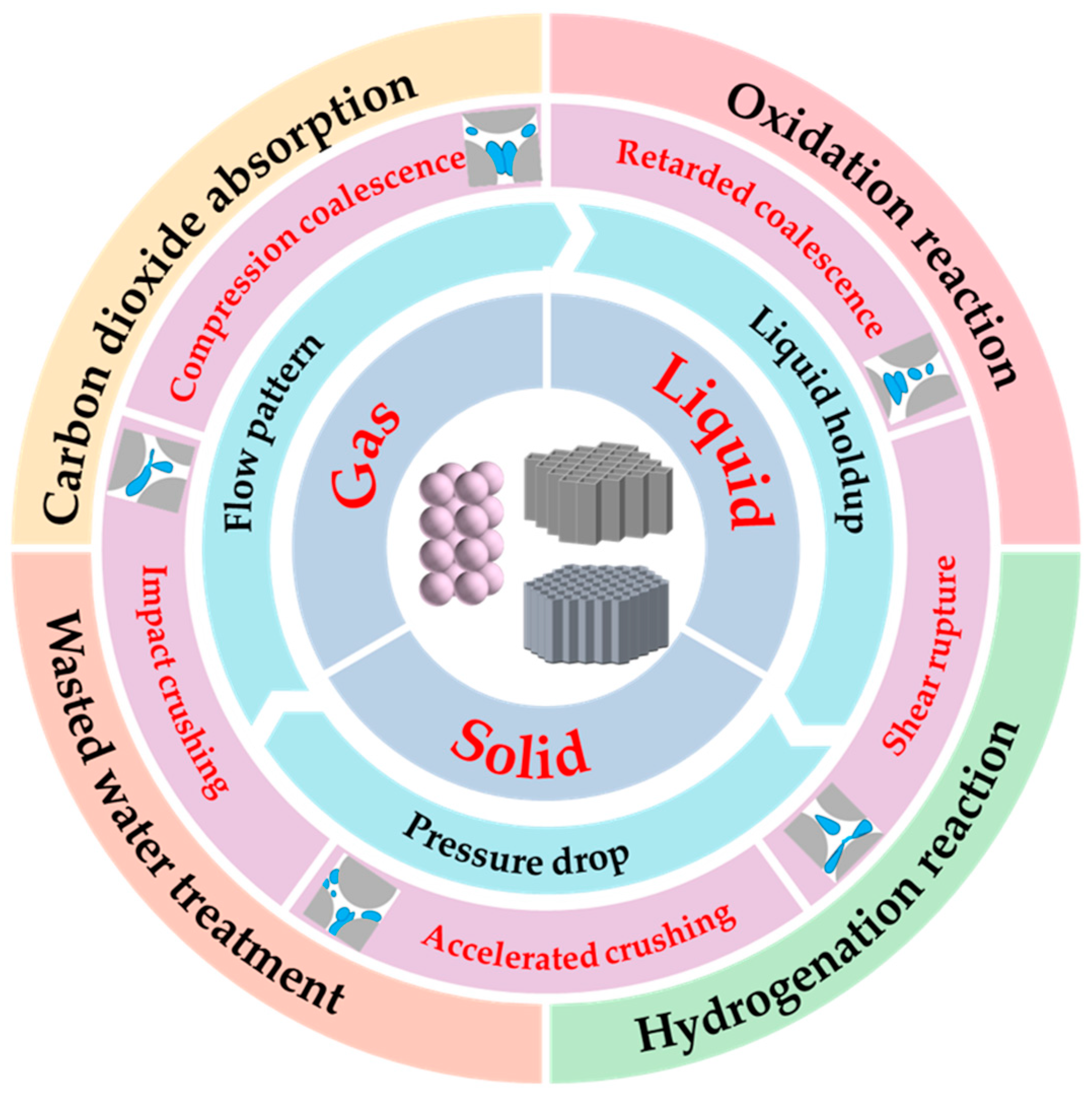
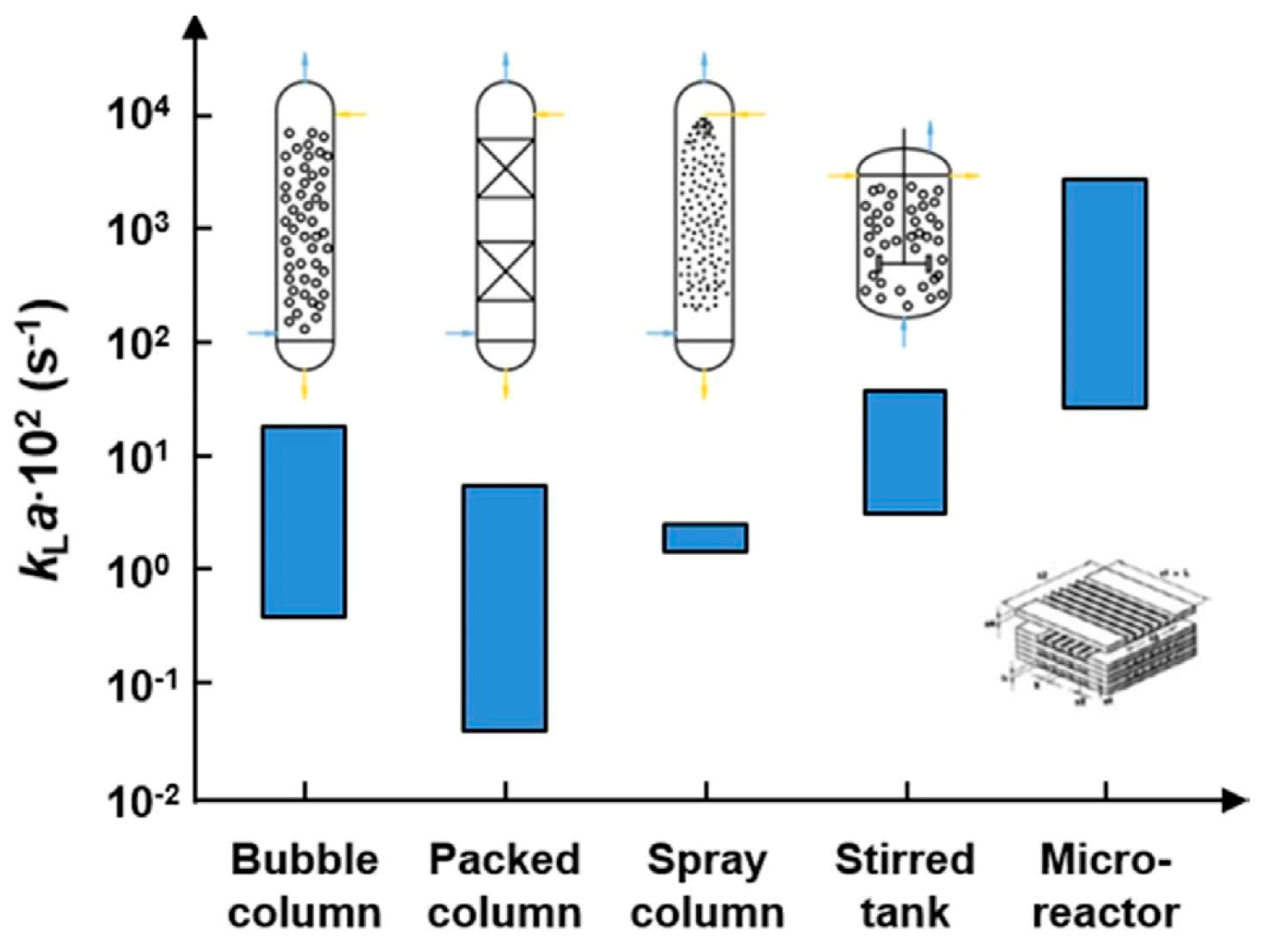
2. Gas–Liquid Flow Dynamics
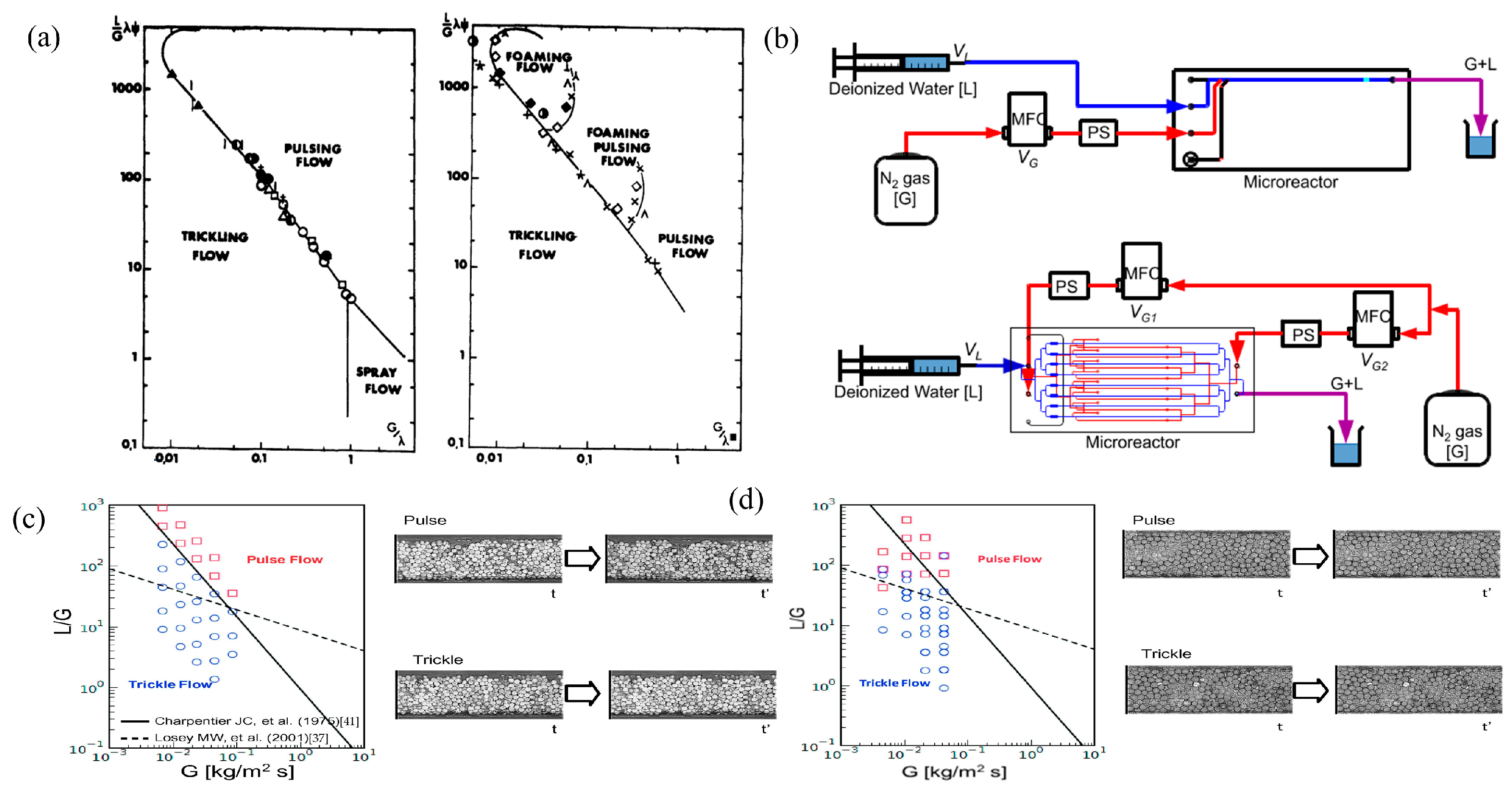
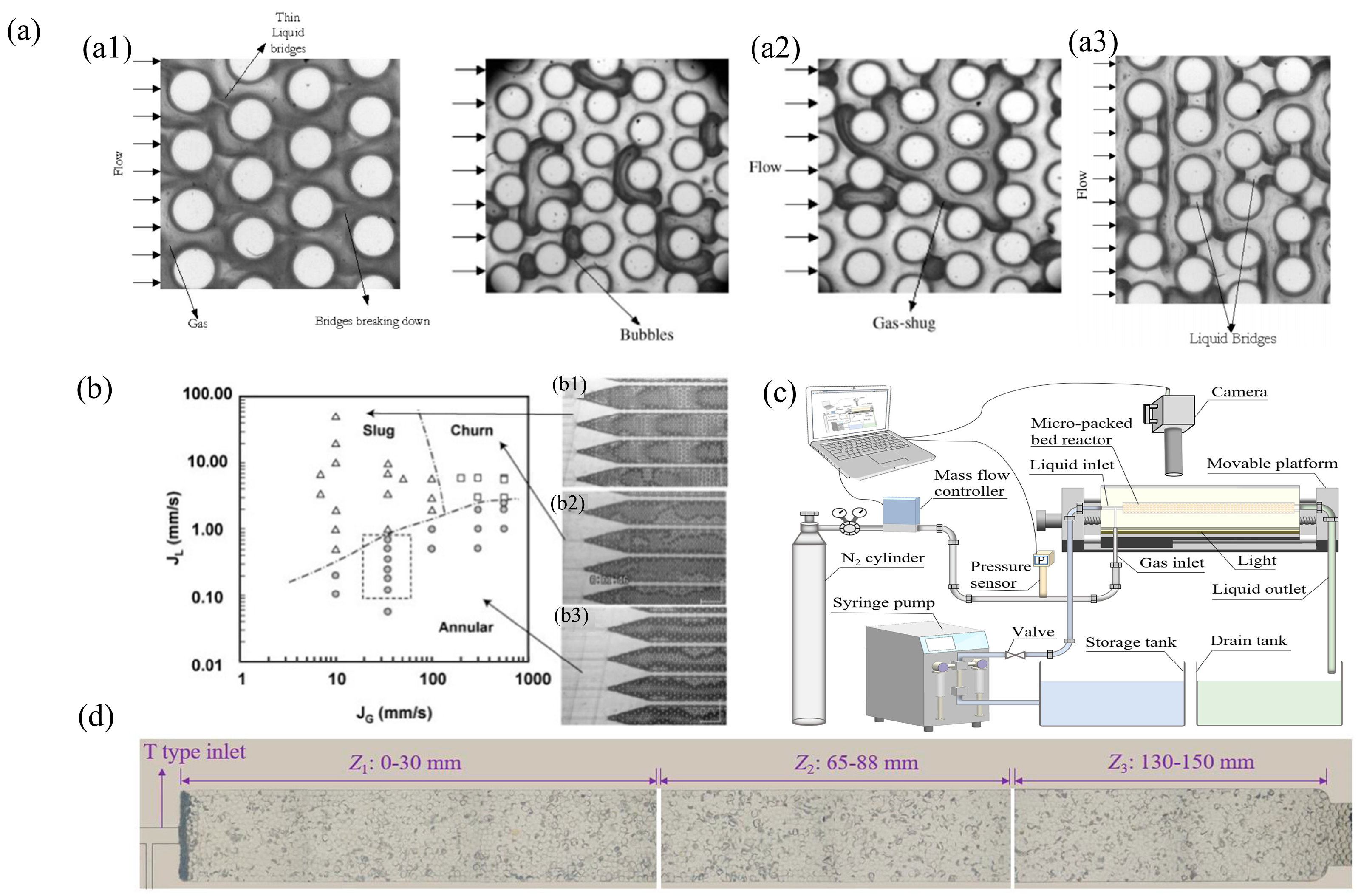
2.1. Gas–Liquid Flow Pattern
2.2. Liquid Holdup
2.3. Pressure Drop
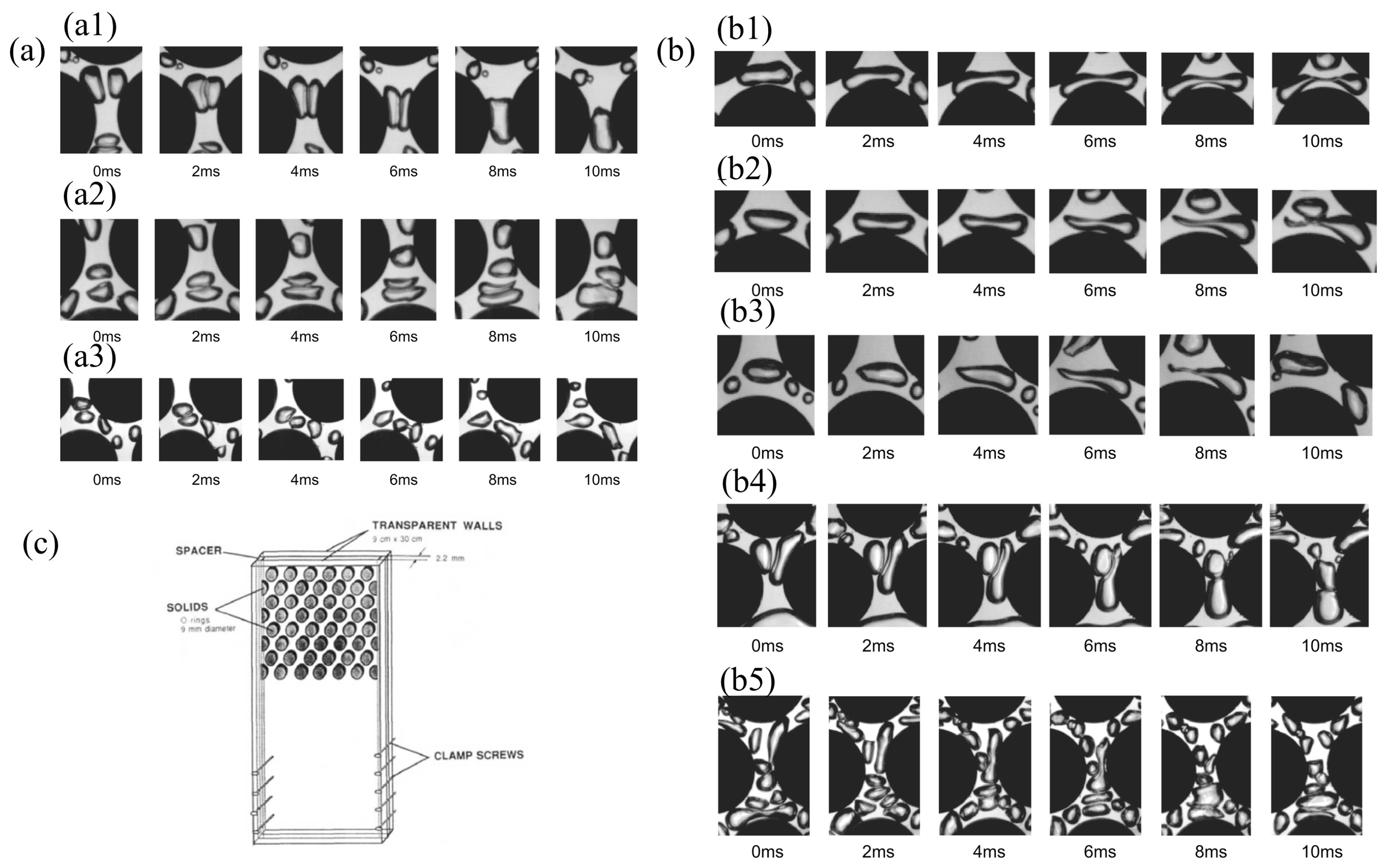
3. Basic Gas–Liquid Dispersion Mechanism in Continuous flow Packed Beds
3.1. Coalescence Mechanisms
3.2. Breakup Mechanisms
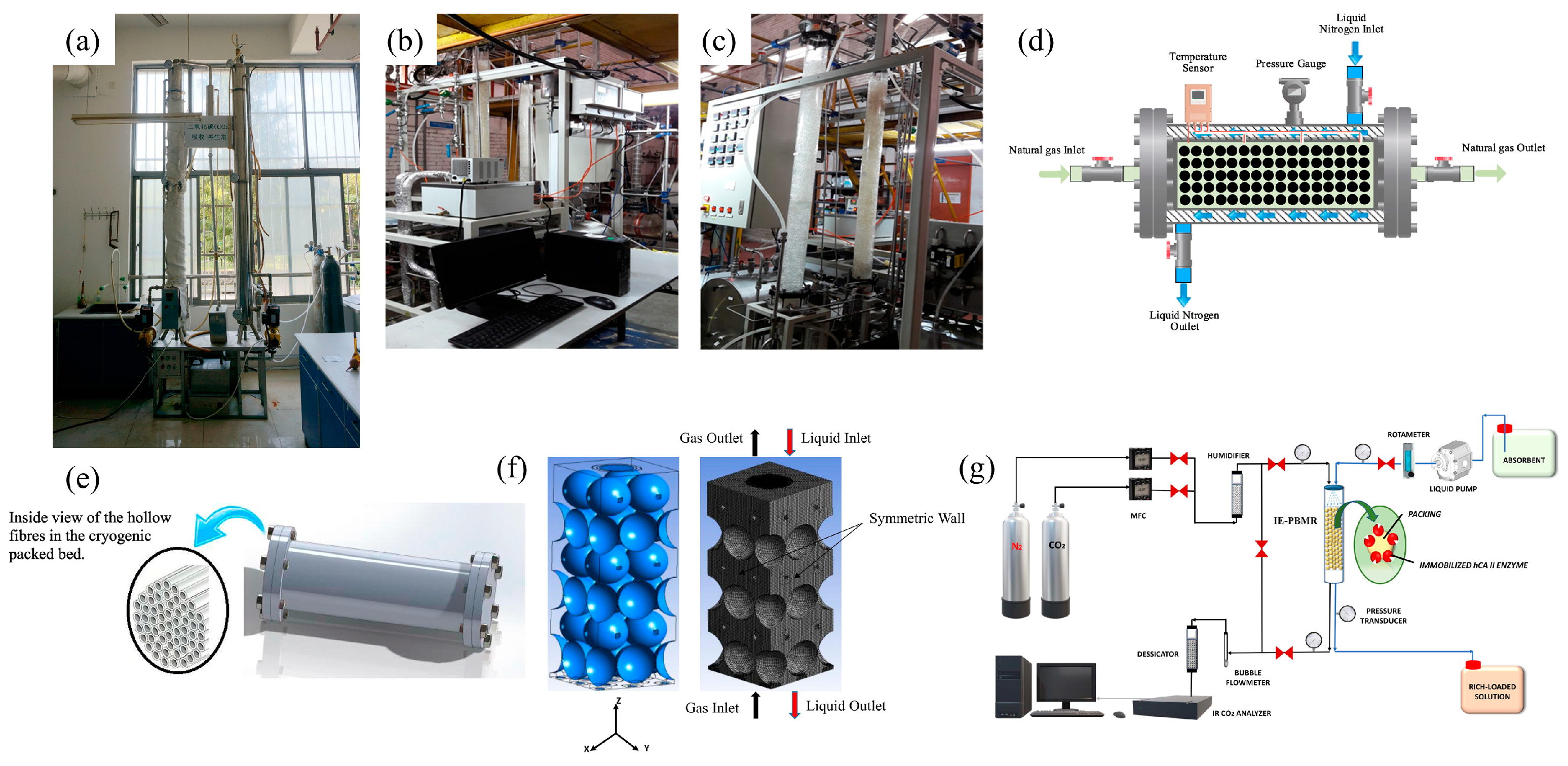
4. Applications of Gas–Liquid Separation
4.1. CO2 Absorption
4.2. Oxidation/Hydrogenation Reactions
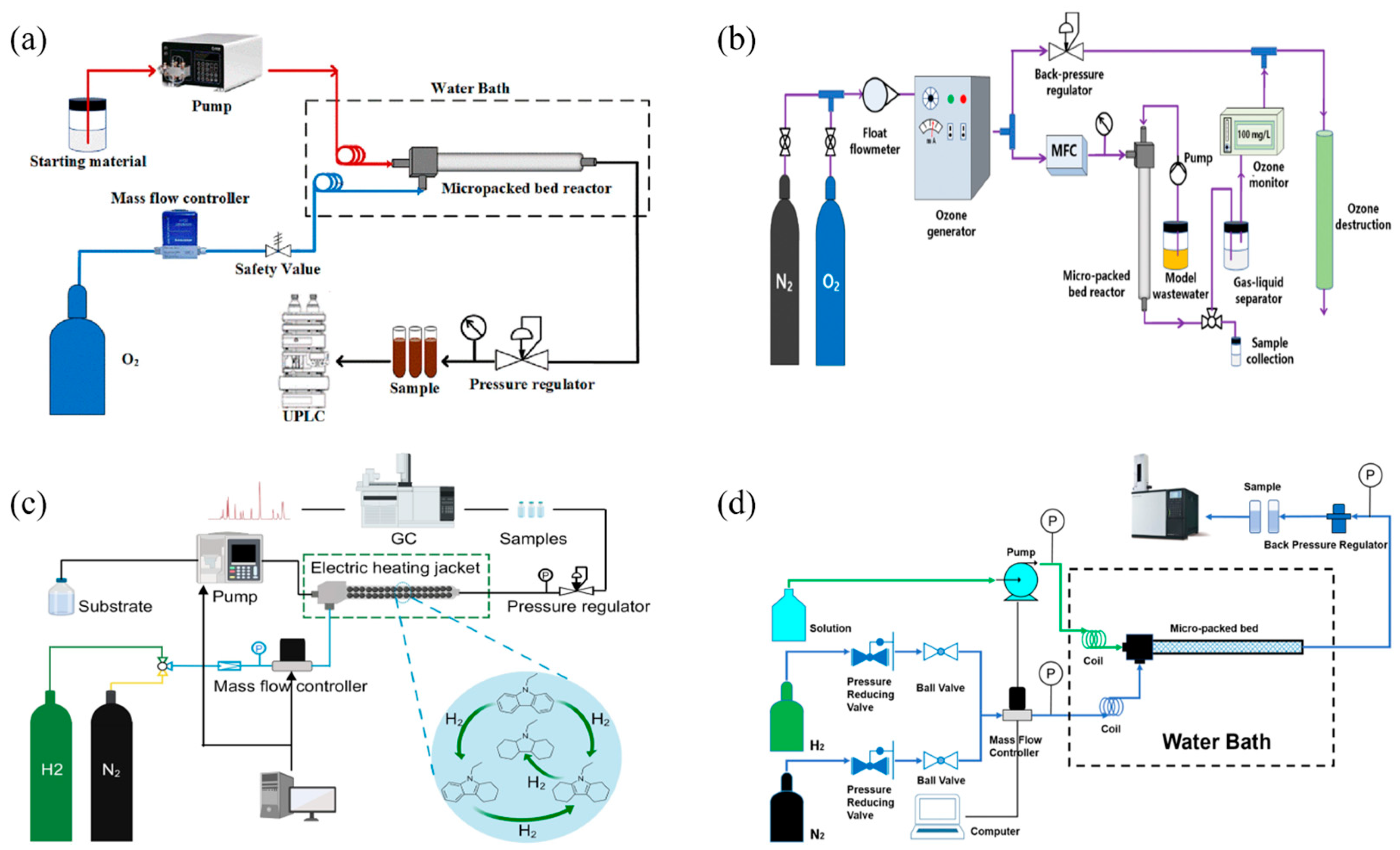
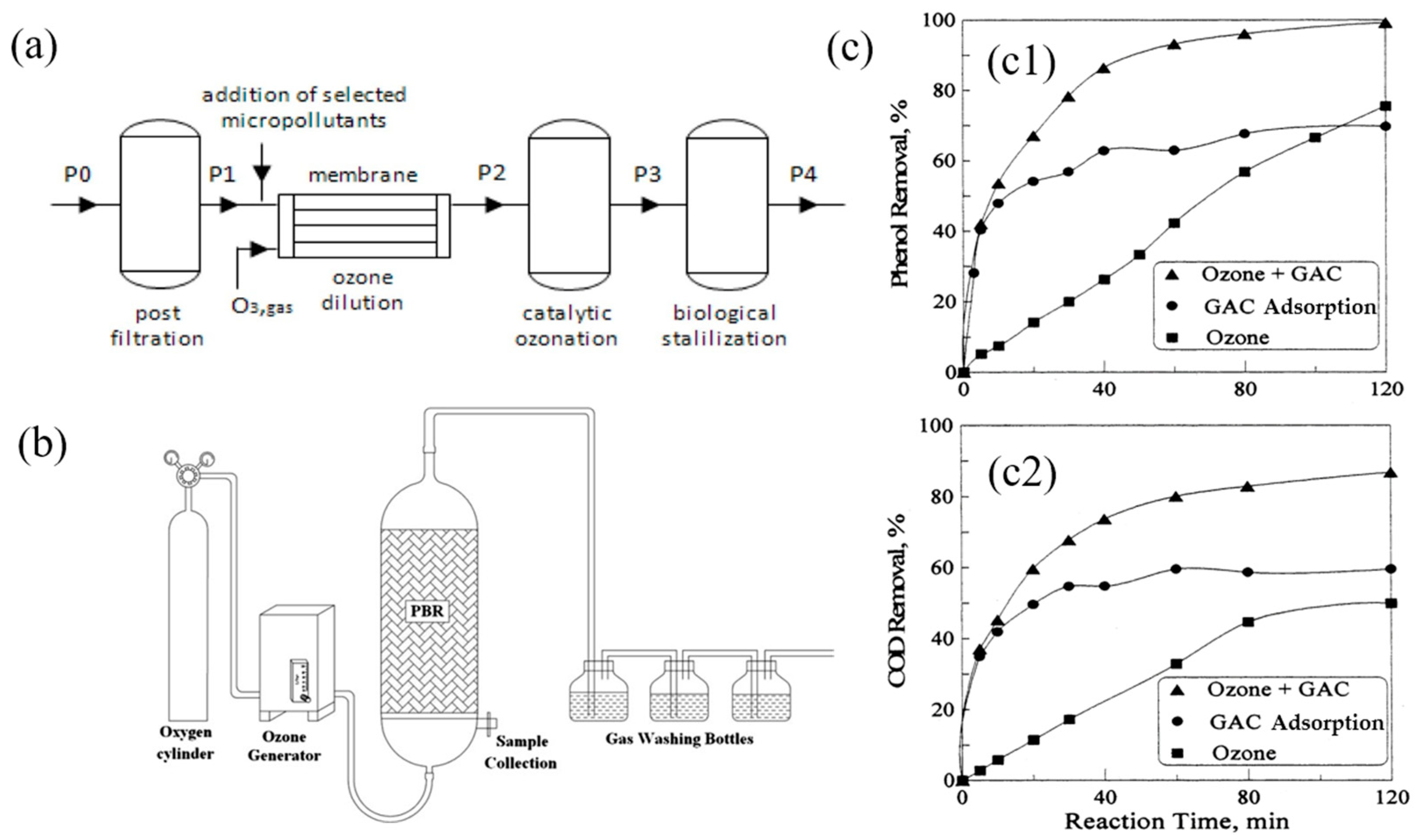
4.3. Wastewater Treatment
5. Development Prospects
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
References
- Albo, J.; Qadir, M.I.; Samperi, M.; Fernandes, J.A.; de Pedro, I.; Dupont, J. Use of an optofluidic microreactor and Cu nanoparticles synthesized in ionic liquid and embedded in TiO2 for an efficient photoreduction of CO2 to methanol. Chem. Eng. J. 2021, 404, 126643. [Google Scholar] [CrossRef]
- Park, C.P.; Kim, D.-P. Dual-channel microreactor for gas−liquid syntheses. J. Am. Chem. Soc. 2010, 132, 10102–10106. [Google Scholar] [CrossRef] [PubMed]
- Polyzos, A.; O’Brien, M.; Petersen, T.P.; Baxendale, I.R.; Ley, S.V. The continuous-flow synthesis of carboxylic acids using CO2 in a tube-in-tube gas permeable membrane reactor. Angew. Chem.-Int. Ed. 2011, 50, 1190. [Google Scholar] [CrossRef] [PubMed]
- Susanti; Winkelman, J.G.; Schuur, B.; Heeres, H.J.; Yue, J. Lactic acid extraction and mass transfer characteristics in slug flow capillary microreactors. Ind. Eng. Chem. Res. 2016, 55, 4691–4702. [Google Scholar] [CrossRef]
- Wang, K.; Luo, G. Microflow extraction: A review of recent development. Chem. Eng. Sci. 2017, 169, 18–33. [Google Scholar] [CrossRef]
- Nguyen, D.T.; Leho, Y.T.; Esser-Kahn, A.P. A three-dimensional microvascular gas exchange unit for carbon dioxide capture. Lab A Chip 2012, 12, 1246–1250. [Google Scholar] [CrossRef]
- Zhang, Q.; Dong, Z.; Liu, Z.; Chen, G. Effect of ultrasonic waveforms on gas–liquid mass transfer in microreactors. AIChE J. 2022, 68, e17689. [Google Scholar] [CrossRef]
- Han, C.; Hu, Y.; Wang, K.; Luo, G. Preparation and in-situ surface modification of CaCO3 nanoparticles with calcium stearate in a microreaction system. Powder Technol. 2019, 356, 414–422. [Google Scholar] [CrossRef]
- Lu, Y.; Liu, Y.; Zhou, C.; Luo, G. Preparation of Li2CO3 nanoparticles by carbonation reaction using a microfiltration membrane dispersion microreactor. Ind. Eng. Chem. Res. 2014, 53, 11015–11020. [Google Scholar] [CrossRef]
- Yuan, P.; Lei, X.-Q.; Sun, H.-M.; Zhang, H.-W.; Cui, C.-S.; Yue, Y.-Y.; Liu, H.-Y.; Bao, X.-J.; Wang, T. Effects of pore size, mesostructure and aluminum modification on FDU-12 supported NiMo catalysts for hydrodesulfurization. Pet. Sci. 2020, 17, 1737–1751. [Google Scholar] [CrossRef]
- Behnejad, B.; Abdouss, M.; Tavasoli, A. Comparison of performance of Ni–Mo/γ-alumina catalyst in HDS and HDN reactions of main distillate fractions. Pet. Sci. 2019, 16, 645–656. [Google Scholar] [CrossRef]
- Kaiser, M.J. A review of refinery complexity applications. Pet. Sci. 2017, 14, 167–194. [Google Scholar] [CrossRef]
- Ghassabzadeh, H.; Rashidzadeh, M.; Niaei, A. A novel fast evaluation method for mesoporous NiMo/Al2O3 hydrodemetallization (HDM) catalysts: Activity and metal uptake capacity measurements. Mech. Catal. 2020, 130, 381–402. [Google Scholar] [CrossRef]
- Chi, K.; Zhao, Z.; Tian, Z.; Hu, S.; Yan, L.; Li, T.; Wang, B.; Meng, X.; Gao, S.; Tan, M. Hydroisomerization performance of platinum supported on ZSM-22/ZSM-23 intergrowth zeolite catalyst. Pet. Sci. 2013, 10, 242–250. [Google Scholar] [CrossRef]
- Dashliborun, A.M.; Larachi, F.; Hamidipour, M. Cyclic operation strategies in inclined and moving packed beds—Potential marine applications for floating systems. AIChE J. 2016, 62, 4157–4172. [Google Scholar] [CrossRef]
- Gorshkova, E.; Manninen, M.; Alopaeus, V.; Laavi, H.; Koskinen, J.; Simulation, N. Three-phase CFD-model for trickle bed reactors. Int. J. Nonlinear Sci. Numer. Simul. 2012, 13, 397–404. [Google Scholar] [CrossRef]
- Devarapalli, M.; Atiyeh, H.K.; Phillips, J.R.; Lewis, R.S.; Huhnke, R.L. Ethanol production during semi-continuous syngas fermentation in a trickle bed reactor using Clostridium ragsdalei. Bioresour. Technol. 2016, 209, 56–65. [Google Scholar] [CrossRef]
- Karimi, A.; Vahabzadeh, F.; Mohseni, M.; Mehranian, M. Decolorization of Maxilon-Red by Kissiris Immobilized Phanerochaete Chrysosporium in a Trickle-Bed Bioreactor-Involvement of Ligninolytic Enzymes. Iran. J. Chem. Chem. Eng. 2009, 28, 1–13. [Google Scholar]
- Rachbauer, L.; Voitl, G.; Bochmann, G.; Fuchs, W. Biological biogas upgrading capacity of a hydrogenotrophic community in a trickle-bed reactor. Appl. Energy 2016, 180, 483–490. [Google Scholar] [CrossRef]
- Üresin, E.; Saraç, H.İ.; Sarıoğlan, A.; Ay, Ş.; Akgün, F.J.P.S.; Protection, E. An experimental study for H2S and CO2 removal via caustic scrubbing system. Process Saf. Environ. Prot. 2015, 94, 196–202. [Google Scholar] [CrossRef]
- Abdullah, G.H.; Xing, Y. Hydrogen peroxide generation in divided-cell trickle bed electrochemical reactor. Ind. Eng. Chem. Res. 2017, 56, 11058–11064. [Google Scholar] [CrossRef]
- Bonrath, W. New trends in (heterogeneous) catalysis for the fine chemicals industry. Chimia 2014, 68, 485. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.; Yin, G.; Li, B.; Wang, X.; Jiang, S.; Yuan, Z. Improved process for 2, 3, 5-trimethylhydroquinone manufacture: Highly efficient catalytic hydrogenation of 2, 3, 5-trimethylbenzoquinone. Res. Chem. Intermed. 2015, 41, 663–677. [Google Scholar] [CrossRef]
- Ng, K. A model for flow regime transitions in cocurrent down-flow trickle-bed reactors. AIChE J. 1986, 32, 115–122. [Google Scholar] [CrossRef]
- Revankar, S.T. Pore scale model for flow regime transition in a trickle bed reactor. Chem. Eng. Commun. 2001, 184, 125–138. [Google Scholar] [CrossRef]
- Attou, A.; Ferschneider, G. A two-fluid hydrodynamic model for the transition between trickle and pulse flow in a cocurrent gas–liquid packed-bed reactor. Chem. Eng. Sci. 2000, 55, 491–511. [Google Scholar] [CrossRef]
- Boelhouwer, J.; Piepers, H.; Drinkenburg, A. Liquid-induced pulsing flow in trickle-bed reactors. Chem. Eng. Sci. 2002, 57, 3387–3399. [Google Scholar] [CrossRef]
- Cheaters, A. The Modeling of Coalescence Processes in Fluid-liquid Dispersions. Trans. Inst. Chem. Eng. 1991, 69, 259–270. [Google Scholar]
- Luo, H.; Svendsen, H.F. Theoretical model for drop and bubble breakup in turbulent dispersions. AIChE J. 1996, 42, 1225–1233. [Google Scholar] [CrossRef]
- Martínez-Bazán, C.; Montanes, J.; Lasheras, J.C. On the breakup of an air bubble injected into a fully developed turbulent flow. Part 1. Breakup frequency. J. Fluid Mech. 1999, 401, 157–182. [Google Scholar] [CrossRef]
- Jo, D.; Revankar, S.T. Bubble mechanisms and characteristics at pore scale in a packed-bed reactor. Chem. Eng. Sci. 2009, 64, 3179–3187. [Google Scholar] [CrossRef]
- Al-Dahhan, M.H.; Larachi, F.; Dudukovic, M.P.; Laurent, A. High-pressure trickle-bed reactors: A review. Ind. Eng. Chem. Res. 1997, 36, 3292–3314. [Google Scholar] [CrossRef]
- Duduković, M.P.; Larachi, F.; Mills, P.L. Multiphase catalytic reactors: A perspective on current knowledge and future trends. Catal. Rev. Sci. Eng. 2002, 44, 123–246. [Google Scholar] [CrossRef]
- Kister, H.Z. Distillation Design; McGraw-Hill: New York, NY, USA, 1992; Volume 1. [Google Scholar]
- Lau, V.; Rezkallah, K. New data on two-phase water-air hydrodynamics in vertical upward and downward tubes. In Proceedings of the annual conference of the Canadian Nuclear Association and 16th annual conference of the Canadian Nuclear Society, Saskatoon, SK, Canada, 28 February–2 March 1995. annual conference; Volume I and II. [Google Scholar]
- Liu, L.; Huang, N.; Liu, D. Divergency and consistency between frictional pressure drops from momentum balance and energy balance for two-phase flow. Chem. Eng. Sci. 2022, 247, 117070. [Google Scholar] [CrossRef]
- Losey, M.W.; Schmidt, M.A.; Jensen, K.F. Microfabricated Multiphase Packed-Bed Reactors: Characterization of Mass Transfer and Reactions. Ind. Eng. Chem. Res. 2001, 40, 2555–2562. [Google Scholar] [CrossRef]
- Inoue, T.; Adachi, J.; Ohtaki, K.; Lu, M.; Murakami, S.; Sun, X.; Wang, D.F. Direct hydrogen peroxide synthesis using glass microfabricated reactor—Paralleled packed bed operation. Chem. Eng. J. 2015, 278, 517–526. [Google Scholar] [CrossRef]
- Jo, D.; Revankar, S.T. Investigation of bubble breakup and coalescence in a packed-bed reactor–Part 1: A comparative study of bubble breakup and coalescence models. Int. J. Multiph. Flow 2011, 37, 995–1002. [Google Scholar] [CrossRef]
- Charpentier, J.C.; Favier, M. Some liquid holdup experimental data in trickle-bed reactors for foaming and nonfoaming hydrocarbons. AIChE J. 1975, 21, 1213–1218. [Google Scholar] [CrossRef]
- Satterfield, C.N. Trickle-bed reactors. AIChE J. 1975, 21, 209–228. [Google Scholar] [CrossRef]
- Charpentier, J.C.; Bakos, M.; Le Goff, P. Hydrodynamics of two-phase concurrent downflow in packed bed reactors: Gas-liquid flow regimes, liquid axial dispersion, and dead zones. In Proceedings of the Second Congress Applied Physical Chemistry, Veszprem, Hungary, 2–5 August 1971; Volume 1. [Google Scholar]
- Yasugi, N.; Fujitsu, A.; Odaira, N.; Ito, D.; Ito, K.; Saito, Y. Characteristics of Two-Phase Flow in Packed Bed Systems. In Proceedings of the International Conference on Nuclear Engineering ICONE, virtual conferenc, 4–6 August 2021; p. V004T014A066. [Google Scholar]
- Krishnamurthy, S.; Peles, Y. Gas-liquid two-phase flow across a bank of micropillars. Phys. Fluids 2007, 19, 043302. [Google Scholar] [CrossRef]
- Wada, Y.; Schmidt, M.A.; Jensen, K.F. Flow distribution and ozonolysis in gas—Liquid multichannel microreactors. Ind. Eng. Chem. Res. 2006, 45, 8036–8042. [Google Scholar] [CrossRef]
- Faridkhou, A.; Larachi, F. Hydrodynamics of Gas–Liquid Cocurrent Flows in Micropacked Beds Wall Visualization Study. Ind. Eng. Chem. Res. 2012, 51, 16495–16504. [Google Scholar] [CrossRef]
- Al-Rifai, N.; Galvanin, F.; Morad, M.; Cao, E.; Cattaneo, S.; Sankar, M.; Dua, V.; Hutchings, G.; Gavriilidis, A. Hydrodynamic effects on three phase micro-packed bed reactor performance–Gold–palladium catalysed benzyl alcohol oxidation. Chem. Eng. Sci. 2016, 149, 129–142. [Google Scholar] [CrossRef]
- Yang, L.; Shi, Y.; Abolhasani, M.; Jensen, K.F. Characterization and modeling of multiphase flow in structured microreactors: A post microreactor case study. Lab Chip 2015, 15, 3232–3241. [Google Scholar] [CrossRef] [PubMed]
- Márquez, N.; Moulijn, J.A.; Makkee, M.; Kreutzer, M.T.; Castaño, P. Tailoring the multiphase flow pattern of gas and liquid through micro-packed bed of pillars. React. Chem. Eng. 2019, 4, 838–851. [Google Scholar] [CrossRef]
- Liu, W.; Xie, B.Q.; Zhang, C.H.; Duan, X.N.; Zhang, J.S. Nature and characteristics of gas-liquid flow regimes in a micro-packed bed reactor. AIChE J. 2023, 69, 13. [Google Scholar] [CrossRef]
- Shulman, H.; Ullrich, C.; Wells, N. Performance of packed columns. I. Total, static, and operating holdups. AIChE J. 1955, 1, 247–253. [Google Scholar] [CrossRef]
- Hoogendoorn, C.; Lips, J. Axial mixing of liquid in gas-liquid flow through packed beds. Can. J. Chem. Eng. 1965, 43, 125–131. [Google Scholar] [CrossRef]
- Nemec, D.; Berčič, G.; Levec, J. Gravimetric method for the determination of liquid holdup in pressurized trickle-bed reactors. Ind. Eng. Chem. Res. 2001, 40, 3418–3422. [Google Scholar] [CrossRef]
- Boyer, C.; Fanget, B. Measurement of liquid flow distribution in trickle bed reactor of large diameter with a new gamma-ray tomographic system. Chem. Eng. Sci. 2002, 57, 1079–1089. [Google Scholar] [CrossRef]
- Chen, J.; Rados, N.; Al-Dahhan, M.H.; Duduković, M.P.; Nguyen, D.; Parimi, K. Particle motion in packed/ebullated beds by CT and CARPT. AIChE J. 2001, 47, 994–1004. [Google Scholar] [CrossRef]
- Yin, F.; Afacan, A.; Nandakumar, K.; Chuang, K.T. Liquid holdup distribution in packed columns: Gamma ray tomography and CFD simulation. Chem. Eng. Process. Process Intensif. 2002, 41, 473–483. [Google Scholar] [CrossRef]
- Olujic, Ž.; Behrens, M. Holdup and pressure drop of packed beds containing a modular catalytic structured packing. Chem. Eng. Technol. 2006, 29, 979–985. [Google Scholar] [CrossRef]
- Zakeri, A.; Einbu, A.; Svendsen, H.F. Experimental investigation of liquid holdup in structured packings. Chem. Eng. Res. Des. 2012, 90, 585–590. [Google Scholar] [CrossRef]
- Mohammed, I.; Bauer, T.; Schubert, M.; Lange, R. Hydrodynamic multiplicity in a tubular reactor with solid foam packings. Chem. Eng. J. 2013, 231, 334–344. [Google Scholar] [CrossRef]
- Sang, L.; Tu, J.; Cheng, H.; Luo, G.; Zhang, J. Hydrodynamics and mass transfer of gas–liquid flow in micropacked bed reactors with metal foam packing. Chem. Eng. J. 2020, 66, e16803. [Google Scholar] [CrossRef]
- Moreira, M.F.; Ferreira, M.C.; Freire, J.T. Total Liquid Saturation in Gas−Liquid Cocurrent Downflow and Upflow through Packed Beds and Analysis of Correlations for Predicting the Total Liquid Saturation. Ind. Eng. Chem. Res. 2004, 43, 1096–1102. [Google Scholar] [CrossRef]
- Kumar, R.K.; Rao, A.; Sankarshana, T.; Khan, A. Liquid holdup in concurrent gas liquid upflow through packed column with random and corrugated structured packing. In Proceedings of the World Congress on Engineering and Computer Science 2012, San Francisco, CA, USA, 23–25 October 2013. [Google Scholar]
- Zhang, J.; Teixeira, A.R.; Kögl, L.T.; Yang, L.; Jensen, K.F. Hydrodynamics of gas–liquid flow in micropacked beds: Pressure drop, liquid holdup, and two-phase model. AIChE J. 2017, 63, 4694–4704. [Google Scholar] [CrossRef]
- Hutter, C.; Zenklusen, A.; Lang, R.; von Rohr, P.R. Axial dispersion in metal foams and streamwise-periodic porous media. Chem. Eng. Sci. 2011, 66, 1132–1141. [Google Scholar] [CrossRef]
- Forchheimer, P. Water movement through ground. Z. Des Vereines Dtsch. Ingenieure 1901, 45, 1736–1741. [Google Scholar]
- Melli, T.R.; De Santos, J.M.; Kolb, W.B.; Scriven, L. Cocurrent downflow in networks of passages. Microscale roots of macroscale flow regimes. Ind.Eng. Chem.Res. 1990, 29, 2367–2379. [Google Scholar] [CrossRef]
- Tsochatzidis, N.A.; Karabelas, A.J. Experiments in trickle beds at the micro-and macroscale. Flow characterization and onset of pulsing. Ind.Eng. Chem.Res. 1994, 33, 1299–1309. [Google Scholar] [CrossRef]
- Benkrid, K.; Rode, S.; Pons, M.N.; Pitiot, P.; Midoux, N. Bubble flow mechanisms in trickle beds—An experimental study using image processing. Chem. Eng. Sci. 2002, 57, 3347–3358. [Google Scholar] [CrossRef]
- Rode, S.; Benkrid, K.; Gillier, T.; Midoux, N. Bubble flow in trickle beds: Investigations using resistive sensors. Chem. Eng. Sci. 2003, 58, 2995–3004. [Google Scholar] [CrossRef]
- Bordas, M.-L.; Cartellier, A.; Séchet, P.; Boyer, C. Bubbly flow through fixed beds: Microscale experiments in the dilute regime and modeling. AIChE J. 2006, 52, 3722–3743. [Google Scholar] [CrossRef]
- KIM, J.W.; LEE, W.K.J. Coalescence behavior of two bubbles in stagnant liquids. J. Chem. Eng. Jpn. 1987, 20, 448–453. [Google Scholar] [CrossRef]
- Kirkpatrick, R.; Lockett, M. The influence of approach velocity on bubble coalescence. Chem. Eng. Sci. 1974, 29, 2363–2373. [Google Scholar] [CrossRef]
- Heldebrant, D.J.; Kothandaraman, J.; Mac Dowell, N.; Brickett, L. Next steps for solvent-based CO2 capture; integration of capture, conversion, and mineralisation. Chem. Sci. 2022, 13, 6445–6456. [Google Scholar] [CrossRef]
- Sheng, L.; Wang, K.; Deng, J.; Chen, G.W.; Luo, G.S. Gas-liquid microdispersion and microflow for carbon dioxide absorption and utilization: A review. Curr. Opin. Chem. Eng. 2023, 40, 100917. [Google Scholar] [CrossRef]
- Khan, A.A.; Halder, G.N.; Saha, A.K. Carbon dioxide capture characteristics from flue gas using aqueous 2-amino-2-methyl-1-propanol (AMP) and monoethanolamine (MEA) solutions in packed bed absorption and regeneration columns. Int. J. Greenh. Gas Control 2015, 32, 15–23. [Google Scholar] [CrossRef]
- Gao, J.; Yin, J.; Zhu, F.F.; Chen, X.; Tong, M.; Kang, W.Z.; Zhou, Y.B.; Lu, J. Integration study of a hybrid solvent MEA-Methanol for post combustion carbon dioxide capture in packed bed absorption and regeneration columns. Sep. Purif. Technol. 2016, 167, 17–23. [Google Scholar] [CrossRef]
- Mirzaei, S.; Shamiri, A.; Aroua, M.K. CO2 Absorption/Desorption in Aqueous Single and Novel Hybrid Solvents of Glycerol and Monoethanolamine in a Pilot-Scale Packed Bed Column. Energy Fuels 2020, 34, 8503–8515. [Google Scholar] [CrossRef]
- Babar, M.; Bustam, M.A.; Ali, A.; Maulud, A.S.; Shafiq, U.; Shariff, A.M.; Man, Z. Eficient CO2 capture using NH2-MIL-101/CA composite cryogenic packed bed column. Cryogenics 2019, 101, 79–88. [Google Scholar] [CrossRef]
- Yang, L.; Liu, F.; Song, Z.C.; Liu, K.L.; Saito, K. 3D Numerical Study of Multiphase Counter-Current Flow within a Packed Bed for Post Combustion Carbon Dioxide Capture. Energies 2018, 11, 1441. [Google Scholar] [CrossRef]
- Iliuta, I.; Rasouli, H.; Iliuta, M.C. Evaluation of intensified CO2 capture in packed-bed microreactors with immobilized carbonic anhydrase by combined theory and experiment. Chem. Eng. J. 2023, 455, 140625. [Google Scholar] [CrossRef]
- Jaberi, H.; Mosleh, S.; Dashtian, K.; Salehi, Z. Fluid based cigarette carbonaceous hydrochar supported ZIF-8 MOF for CO2 capture process: The engineering parameters determination for the packed bed column design. Chem. Eng. Process.-Process Intensif. 2020, 153, 108001. [Google Scholar] [CrossRef]
- Miramontes, E.; Love, L.J.; Lai, C.H.; Sun, X.; Tsouris, C. Additively manufactured packed bed device for process intensification of CO2 absorption and other chemical processes. Chem. Eng. J. 2020, 388, 124092. [Google Scholar] [CrossRef]
- Zhu, K.; Yao, C.Q.; Liu, Y.Y.; Chen, G.W. Using expansion units to improve CO2 absorption for natural gas purification—A study on the hydrodynamics and mass transfer. Chin. J. Chem. Eng. 2021, 29, 35–46. [Google Scholar] [CrossRef]
- Zhang, C.H.; Luo, J.; Xie, B.Q.; Liu, W.; Zhang, J.S. Green and continuous aerobic oxidation of ethylbenzene over homogeneous and heterogeneous NHPI in a micro-packed bed reactor. Chem. Eng. J. 2023, 468, 143674. [Google Scholar] [CrossRef]
- Zhang, C.H.; Luo, J.; Xie, B.Q.; Liu, W.; Zhang, J.S. Continuous Cu/keto-ABNO catalyzed aerobic oxidation of ethyl lactate to ethyl pyruvate in a micro-packed bed reactor: Process optimization and reaction kinetics. Chem. Eng. Sci. 2024, 292, 119985. [Google Scholar] [CrossRef]
- Cao, Q.; Sang, L.; Tu, J.C.; Xiao, Y.S.; Liu, N.; Wu, L.D.; Zhang, J.S. Rapid degradation of refractory organic pollutants by continuous ozonation in a micro-packed bed reactor. Chemosphere 2021, 270, 128621. [Google Scholar] [CrossRef] [PubMed]
- Yang, W.Y.; Tang, X.J.; Li, W.J.; Luo, X.; Zhang, C.Y.; Shen, C. Fast and continuous synthesis of 2,5-furandicarboxylic acid in a micropacked-bed reactor. Chem. Eng. J. 2022, 442, 136110. [Google Scholar] [CrossRef]
- Duan, X.N.; Wang, X.P.; Chen, X.K.; Zhang, J.S. Continuous and Selective Hydrogenation of Heterocyclic Nitroaromatics in a Micropacked Bed Reactor. Org. Process Res. Dev. 2021, 25, 2100–2109. [Google Scholar] [CrossRef]
- Fan, Y.W.; Wang, P.X.; Zhang, J.H.; Huang, M.M.; Liu, W.; Xu, Y.L.; Duan, X.A.; Li, Y.Y.; Zhang, J.S. Continuous hydrogenation of N-ethylcarbazole in a micro-packed bed reactor for hydrogen storage. Chem. Eng. J. 2024, 484, 149404. [Google Scholar] [CrossRef]
- Wang, P.X.; Peng, Z.P.; Wang, X.P.; Lin, Y.; Hong, H.B.; Chen, F.; Chen, X.K.; Zhang, J.S. Continuous hydrogenation of nitriles to primary amines with high selectivity in flow. Chem. Eng. Sci. 2023, 269, 118460. [Google Scholar] [CrossRef]
- Duan, X.N.; Yin, J.B.; Huang, M.M.; Feng, A.X.; Fu, W.S.; Chen, H.X.; Huang, Z.F.; Ding, Y.G.; Zhang, J.S. Hydrogenation kinetics of m-dinitrobenzene in a continuous micro-packed bed reactor. Chem. Eng. Sci. 2022, 248, 117113. [Google Scholar] [CrossRef]
- Duan, X.N.; Yin, J.B.; Huang, M.M.; Wang, P.X.; Zhang, J.S. Hydrogenation kinetics of halogenated nitroaromatics over Pt/C in a continuous Micro-packed bed reactor. Chem. Eng. Sci. 2022, 251, 117483. [Google Scholar] [CrossRef]
- Akach, J.; Kabuba, J.; Ochieng, A.J.I.; Research, E.C. Simulation of the light distribution in a solar photocatalytic bubble column reactor using the Monte Carlo method. Ind. Eng. Chem. Res. 2020, 59, 17708–17719. [Google Scholar] [CrossRef]
- Chavez, A.M.; Ribeiro, A.R.; Moreira, N.F.E.; Silva, A.M.T.; Rey, A.; Alvarez, P.M.; Beltran, F.J. Removal of Organic Micropollutants from a Municipal Wastewater Secondary Effluent by UVA-LED Photocatalytic Ozonation. Catalysts 2019, 9, 472. [Google Scholar] [CrossRef]
- Narayan Thorat, B.; Kumar Sonwani, R. Current technologies and future perspectives for the treatment of complex petroleum refinery wastewater: A review. Bioresour. Technol. 2022, 355, 127263. [Google Scholar] [CrossRef]
- Tabar, I.B. Ozone as Oxidant for Biomass Pretreatment and Nanocellulose Production. Ph.D. Thesis, Purdue University, West Lafayette, IN, USA, 2017. [Google Scholar]
- Chen, S.; Ren, T.; Zhou, Z.; Lu, K.; Huang, X.; Zhang, X. Insights into Mn loaded carbon-silica-membrane based catalytic ozonation process for efficient wastewater treatment: Performance and mechanism. Chem. Eng. J. 2023, 475, 145874. [Google Scholar] [CrossRef]
- Suthanthararajan, R.; Ravindranath, E.; Chits, K.; Umamaheswari, B.; Ramesh, T.; Rajamam, S. Membrane application for recovery and reuse of water from treated tannery wastewater. Desalination 2004, 164, 151–156. [Google Scholar] [CrossRef]
- Vedaraman, N.; Begum, S.S.; Srinivasan, S.V. Response surface methodology for decolourisation of leather dye using ozonation in a packed bed reactor. Clean Technol. Environ. Policy 2013, 15, 607–616. [Google Scholar] [CrossRef]
- Lin, S.H.; Wang, C.H. Ozonation of phenolic wastewater in a gas-induced reactor with a fixed granular activated carbon bed. Ind. Eng. Chem. Res. 2003, 42, 1648–1653. [Google Scholar] [CrossRef]
- Faridkhou, A.; Hamidipour, M.; Larachi, F. Hydrodynamics of gas–liquid micro-fixed beds–measurement approaches and technical challenges. Chem. Eng. J. 2013, 223, 425–435. [Google Scholar] [CrossRef]
- Tadepalli, S.; Qian, D.; Lawal, A. Comparison of performance of microreactor and semi-batch reactor for catalytic hydrogenation of o-nitroanisole. Catal. Today 2007, 125, 64–73. [Google Scholar] [CrossRef]
- Bellos, G.; Papayannakos, N. The use of a three phase microreactor to investigate HDS kinetics. Catal. Today 2003, 79, 349–355. [Google Scholar] [CrossRef]
- Guettel, R.; Turek, T. Assessment of micro-structured fixed-bed reactors for highly exothermic gas-phase reactions. Chem. Eng. Sci. 2010, 65, 1644–1654. [Google Scholar] [CrossRef]
- Knobloch, C.; Güttel, R.; Turek, T. Holdup and pressure drop in micro packed-bed reactors for Fischer-Tropsch synthesis. Chem. Ing. Tech. 2013, 85, 455–460. [Google Scholar] [CrossRef]
- Knochen, J.; Güttel, R.; Knobloch, C.; Turek, T. Fischer–Tropsch synthesis in milli-structured fixed-bed reactors: Experimental study and scale-up considerations. Chem. Eng. Process.-Process Intensif. 2010, 49, 958–964. [Google Scholar] [CrossRef]
| Correlations | Reference |
|---|---|
| (valid for downflow) | Marcos F. P. Moreira et al. (2004) [61] |
| (valid for upflow) | |
| Žarko Olujic and M. Behrens (2006) [59] | |
| R. Kishore Kumar et al. (2012) [62] | |
| Ali Zakeri et al. (2012) [60] | |
| Iman Mohammed et al. (2013) [61] | |
| Zhang et al. (2017) [63] | |
| Sang et al. (2019) [60] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Du, C.; Zhang, Z.; Du, J.; Tu, Y.; Ren, Z. Process Intensification of Gas–Liquid Separations Using Packed Beds: A Review. Separations 2024, 11, 284. https://doi.org/10.3390/separations11100284
Zhang Y, Du C, Zhang Z, Du J, Tu Y, Ren Z. Process Intensification of Gas–Liquid Separations Using Packed Beds: A Review. Separations. 2024; 11(10):284. https://doi.org/10.3390/separations11100284
Chicago/Turabian StyleZhang, Yafang, Chencan Du, Zhibo Zhang, Jiawei Du, Yuming Tu, and Zhongqi Ren. 2024. "Process Intensification of Gas–Liquid Separations Using Packed Beds: A Review" Separations 11, no. 10: 284. https://doi.org/10.3390/separations11100284
APA StyleZhang, Y., Du, C., Zhang, Z., Du, J., Tu, Y., & Ren, Z. (2024). Process Intensification of Gas–Liquid Separations Using Packed Beds: A Review. Separations, 11(10), 284. https://doi.org/10.3390/separations11100284







