Prediction of Plate Height Curves of Porous-Shell Pillar Array Columns Micro-Pillar Array Columns
Abstract
1. Introduction
2. Materials and Methods
3. Results
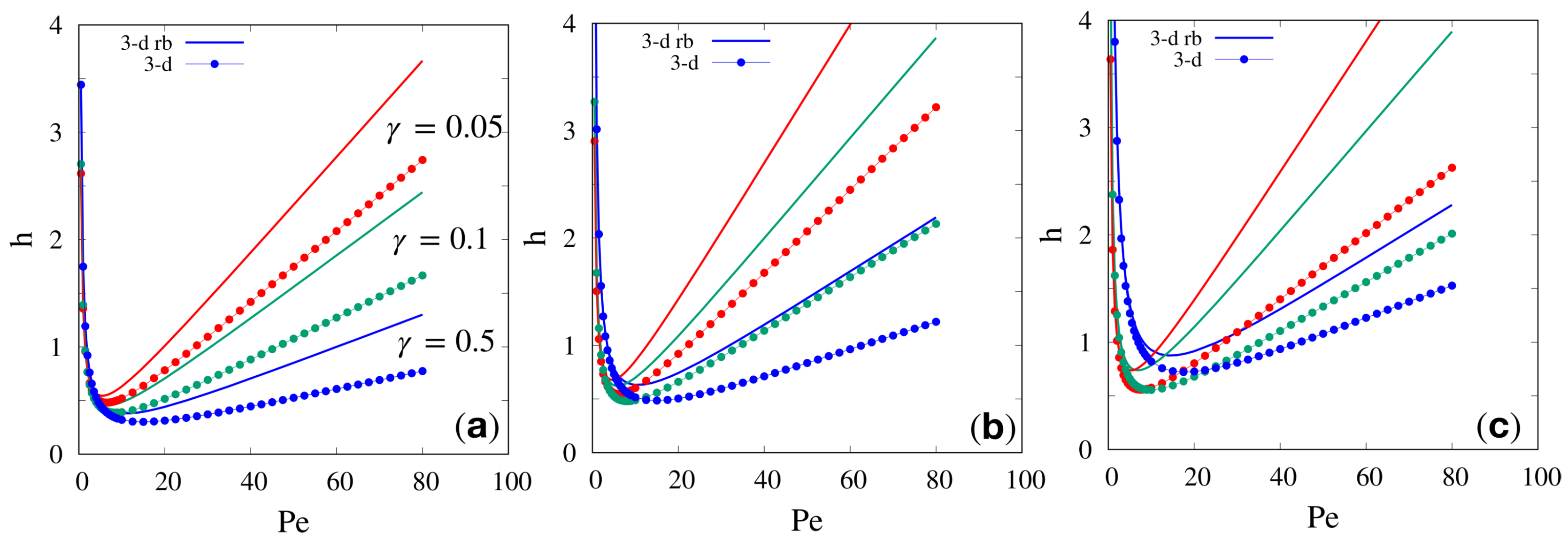
3.1. Comparison between 2-D, 3-D, and 3-D rb Cases
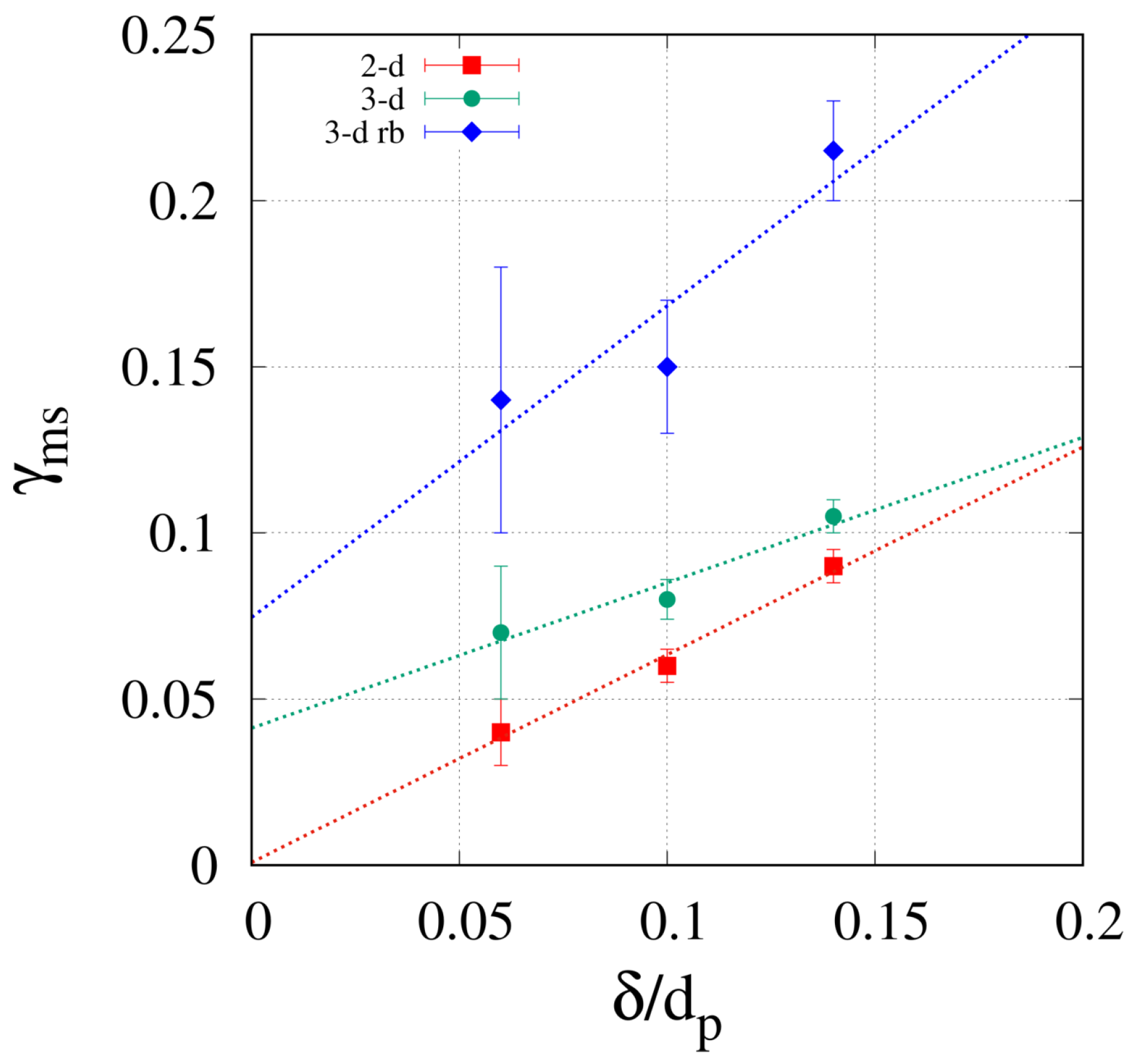
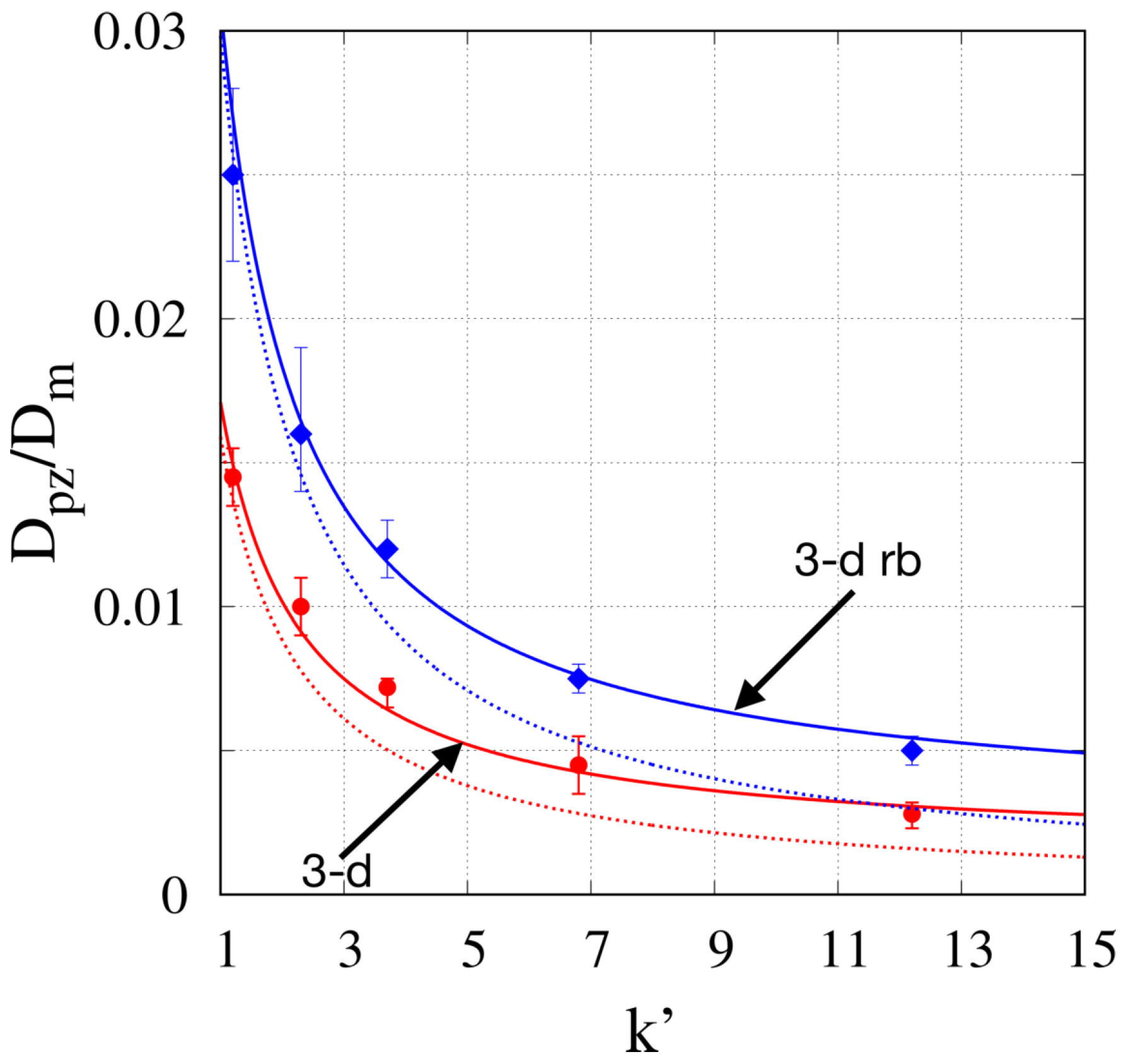
3.2. Analysis of Experimental Plate Height Curves
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Cavazzini, A.; Gritti, F.; Kaczmarski, K.; Marchetti, N.; Guiochon, G. Mass-transfer kinetics in a shell packing material for chromatography. Anal. Chem. 2007, 79, 5972–5979. [Google Scholar] [CrossRef] [PubMed]
- Horváth, K.; Gritti, F.; Fairchild, J.N.; Guiochon, G. On the optimization of the shell thickness of superficially porous particles. J. Chromatogr. A 2010, 1217, 6373–6381. [Google Scholar] [CrossRef] [PubMed]
- Felletti, S.; Catani, M.; Mazzoccanti, G.; De Luca, C.; Lievore, G.; Buratti, A.; Pasti, L.; Gasparrini, F.; Cavazzini, A. Mass transfer kinetics on modern Whelk-O1 chiral stationary phases made on fully-and superficially-porous particles. J. Chromatogr. A 2021, 1637, 461854. [Google Scholar] [CrossRef] [PubMed]
- Blumberg, L.M. Methodology of quantitative comparison of practically achievable kinetic performance of differently structured liquid chromatography columns. J. Chromatogr. A 2022, 1672, 463039. [Google Scholar] [CrossRef] [PubMed]
- Blumberg, L.M. Transport diameters of liquid chromatography columns. J. Chromatogr. A 2023, 1687, 463688. [Google Scholar] [CrossRef] [PubMed]
- Knox, J.H. Band dispersion in chromatography—A universal expression for the contribution from the mobile zone. J. Chromatogr. A 2002, 960, 7–18. [Google Scholar] [CrossRef] [PubMed]
- Schure, M.R.; Maier, R.S.; Kroll, D.M.; Davis, H.T. Simulation of packed-bed chromatography utilizing high-resolution flow fields: Comparison with models. Anal. Chem. 2002, 74, 6006–6016. [Google Scholar] [CrossRef]
- Schure, M.R.; Maier, R.S.; Kroll, D.M.; Davis, H.T. Simulation of ordered packed beds in chromatography. J. Chromatogr. A 2004, 1031, 79–86. [Google Scholar] [CrossRef]
- De Malsche, W.; Eghbali, H.; Clicq, D.; Vangelooven, J.; Gardeniers, H.; Desmet, G. Pressure-driven reverse-phase liquid chromatography separations in ordered nonporous pillar array columns. Anal. Chem. 2007, 79, 5915–5926. [Google Scholar] [CrossRef]
- Nawada, S.; Dimartino, S.; Fee, C. Dispersion behavior of 3D-printed columns with homogeneous microstructures comprising differing element shapes. Chem. Eng. Sci. 2017, 164, 90–98. [Google Scholar] [CrossRef]
- Umatheva, U.; Chen, G.; Ghosh, R. Computational fluid dynamic (CFD) simulation of a cuboid packed-bed chromatography device. Chem. Eng. Res. Des. 2019, 152, 393–401. [Google Scholar] [CrossRef]
- Parmentier, F. Effect of diffusional bridging in multicapillary packing. Comptes Rendus Chim. 2020, 23, 415–431. [Google Scholar] [CrossRef]
- Broeckhoven, K.; Desmet, G. Advances and innovations in liquid chromatography stationary phase supports. Anal. Chem. 2020, 93, 257–272. [Google Scholar] [CrossRef] [PubMed]
- Regnier, F.E. Microfabricated monolith columns for liquid chromatography. sculpting supports for liquid chromatography. J. High Resolut. Chromatogr. 2000, 23, 19–26. [Google Scholar] [CrossRef]
- Slentz, B.E.; Penner, N.A.; Regnier, F. Geometric effects of collocated monolithic support structures on separation performance in microfabricated systems. J. Sep. Sci. 2002, 25, 1011–1018. [Google Scholar] [CrossRef]
- De Pra, M.; Kok, W.T.; Gardeniers, J.G.; Desmet, G.; Eeltink, S.; van Nieuwkasteele, J.W.; Schoenmakers, P.J. Experimental study on band dispersion in channels structured with micropillars. Anal. Chem. 2006, 78, 6519–6525. [Google Scholar] [CrossRef] [PubMed]
- De Pra, M.; De Malsche, W.; Desmet, G.; Schoenmakers, P.J.; Kok, W.T. Pillar-structured microchannels for on-chip liquid chromatography: Evaluation of the permeability and separation performance. J. Sep. Sci. 2007, 30, 1453–1460. [Google Scholar] [CrossRef]
- De Pra, M.; Kok, W.T.; Schoenmakers, P.J. Topographic structures and chromatographic supports in microfluidic separation devices. J. Chromatogr. A 2008, 1184, 560–572. [Google Scholar] [CrossRef]
- Callewaert, M.; De Beeck, J.O.; Maeno, K.; Sukas, S.; Thienpont, H.; Ottevaere, H.; Gardeniers, H.; Desmet, G.; De Malsche, W. Integration of uniform porous shell layers in very long pillar array columns using electrochemical anodization for liquid chromatography. Analyst 2014, 139, 618–625. [Google Scholar] [CrossRef]
- De Malsche, W.; Clicq, D.; Verdoold, V.; Gzil, P.; Desmet, G.; Gardeniers, H. Integration of porous layers in ordered pillar arrays for liquid chromatography. Lab Chip 2007, 7, 1705–1711. [Google Scholar] [CrossRef]
- Malsche, W.D.; Gardeniers, H.; Desmet, G. Experimental study of porous silicon shell pillars under retentive conditions. Anal. Chem. 2008, 80, 5391–5400. [Google Scholar] [CrossRef]
- Brenner, H.; Gaydos, L.J. The constrained brownian movement of spherical particles in cylindrical pores of comparable radius: Models of the diffusive and convective transport of solute molecules in membranes and porous media. J. Colloid Interface Sci. 1977, 58, 312–356. [Google Scholar] [CrossRef]
- Brenner, H.; Edwards, D.A. Macrotransport Processes; Butterworth-Heinemann: Oxford, UK, 1993. [Google Scholar]
- Adrover, A.; Cerbelli, S.; Giona, M. Taming axial dispersion in hydrodynamic chromatography columns through wall patterning. Phys. Fluids 2018, 30, 042002. [Google Scholar] [CrossRef]
- Adrover, A.; Cerbelli, S. Laminar dispersion at low and high Peclet numbers in finite-length patterned microtubes. Phys. Fluids 2017, 29, 062005. [Google Scholar] [CrossRef]
- Adrover, A.; Passaretti, C.; Venditti, C.; Giona, M. Exact moment analysis of transient dispersion properties in periodic media. Phys. Fluids 2019, 31, 112002. [Google Scholar] [CrossRef]
- Adrover, A.; Venditti, C.; Giona, M. Laminar dispersion at low and high Peclet numbers in a sinusoidal microtube: Point-size versus finite-size particles. Phys. Fluids 2019, 31, 062003. [Google Scholar] [CrossRef]
- Giona, M.; Venditti, C.; Adrover, A. On the long-term simulation of stochastic differential equations for predicting effective dispersion coefficients. Phys. A Stat. Mech. Its Appl. 2020, 543, 123392. [Google Scholar] [CrossRef]
- Biagioni, V.; Venditti, C.; Adrover, A.; Giona, M.; Cerbelli, S. Taming Taylor-Aris dispersion through chaotic advection. J. Chromatogr. A 2022, 1673, 463110. [Google Scholar] [CrossRef]
- Venditti, C.; Cerbelli, S.; Procopio, G.; Adrover, A. Comparison between one-and two-way coupling approaches for estimating effective transport properties of suspended particles undergoing Brownian sieving hydrodynamic chromatography. Phys. Fluids 2022, 34, 042010. [Google Scholar] [CrossRef]
- Venditti, C.; Biagioni, V.; Adrover, A.; Cerbelli, S. Impact of transversal vortices on the performance of Open-Tubular Liquid Chromatography. J. Chromatogr. A 2022, 1685, 463623. [Google Scholar] [CrossRef]
- Venditti, C.; Giona, M.; Adrover, A. Exact moment analysis of transient/asymptotic dispersion properties in periodic media with adsorbing/desorbing walls. Phys. Fluids 2022, 34, 122013. [Google Scholar] [CrossRef]
- Venditti, C.; Huygens, B.; Desmet, G.; Adrover, A. Moment analysis for predicting effective transport properties in hierarchical retentive porous media. J. Chromatogr. A 2023, 1703, 464099. [Google Scholar] [CrossRef] [PubMed]
- Huygens, B.; Venditti, C.; Adrover, A.; Desmet, G. Nonadditivity and Nonlinearity of Mobile and Stationary Zone Mass Transfer Resistances in Chromatography. Anal. Chem. 2023, 95, 15199–15207. [Google Scholar] [CrossRef] [PubMed]
- Moussa, A.; Huygens, B.; Venditti, C.; Adrover, A.; Desmet, G. Theoretical computation of the band broadening in micro-pillar array columns. J. Chromatogr. A 2024, 1715, 464607. [Google Scholar] [CrossRef] [PubMed]
- Adrover, A.; Venditti, C.; Desmet, G. An alternative general model for the effective longitudinal diffusion in chromatographic beds filled with ordered porous particles. J. Chromatogr. A 2023, 1715, 464598. [Google Scholar] [CrossRef] [PubMed]
- Plumb, O.; Whitaker, S. Diffusion, adsorption and dispersion in porous media: Small-scale averaging and local volume averaging. In Dynamics of Fluids in Hierarchical Porous Media; Academic Press, Inc.: San Diego, CA, USA, 1990; pp. 97–148. [Google Scholar]
- Paine, M.; Carbonell, R.; Whitaker, S. Dispersion in pulsed systems—I: Heterogenous reaction and reversible adsorption in capillary tubes. Chem. Eng. Sci. 1983, 38, 1781–1793. [Google Scholar] [CrossRef]
- Plumb, O.A.; Whitaker, S. Dispersion in heterogeneous porous media: 1. Local volume averaging and large-scale averaging. Water Resour. Res. 1988, 24, 913–926. [Google Scholar] [CrossRef]
- Yan, X.; Wang, Q.; Bau, H.H. Dispersion in retentive pillar array columns. J. Chromatogr. A 2010, 1217, 1332–1342. [Google Scholar] [CrossRef]
- Yan, X.; Wang, Q.; Li, N. Predictive model of solute transport with reversible adsorption in spatially periodic hierarchical porous media. J. Chromatogr. A 2015, 1407, 69–75. [Google Scholar] [CrossRef]
- Giddings, J.C. The role of lateral diffusion as a rate-controlling mechanism in chromatography. J. Chromatogr. A 1961, 5, 46–60. [Google Scholar] [CrossRef]
- Desmet, G.; Broeckhoven, K.; De Smet, J.; Deridder, S.; Baron, G.; Gzil, P. Errors involved in the existing B-term expressions for the longitudinal diffusion in fully porous chromatographic media: Part I: Computational data in ordered pillar arrays and effective medium theory. J. Chromatogr. A 2008, 1188, 171–188. [Google Scholar] [CrossRef] [PubMed]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Venditti, C.; Desmet, G.; Adrover, A. Prediction of Plate Height Curves of Porous-Shell Pillar Array Columns Micro-Pillar Array Columns. Separations 2024, 11, 22. https://doi.org/10.3390/separations11010022
Venditti C, Desmet G, Adrover A. Prediction of Plate Height Curves of Porous-Shell Pillar Array Columns Micro-Pillar Array Columns. Separations. 2024; 11(1):22. https://doi.org/10.3390/separations11010022
Chicago/Turabian StyleVenditti, Claudia, Gert Desmet, and Alessandra Adrover. 2024. "Prediction of Plate Height Curves of Porous-Shell Pillar Array Columns Micro-Pillar Array Columns" Separations 11, no. 1: 22. https://doi.org/10.3390/separations11010022
APA StyleVenditti, C., Desmet, G., & Adrover, A. (2024). Prediction of Plate Height Curves of Porous-Shell Pillar Array Columns Micro-Pillar Array Columns. Separations, 11(1), 22. https://doi.org/10.3390/separations11010022






