Effect of the Position of Overflow Pipe with Mixed Spiral Structures on the Separation Performance of Hydrocyclones
Abstract
1. Introduction
2. Mathematical Model and Mesh Generation
3. Model Description
- (1)
- The RSM model
- (2)
- The VOF model
- (3)
- The TFM model
3.1. Simulation Parameters
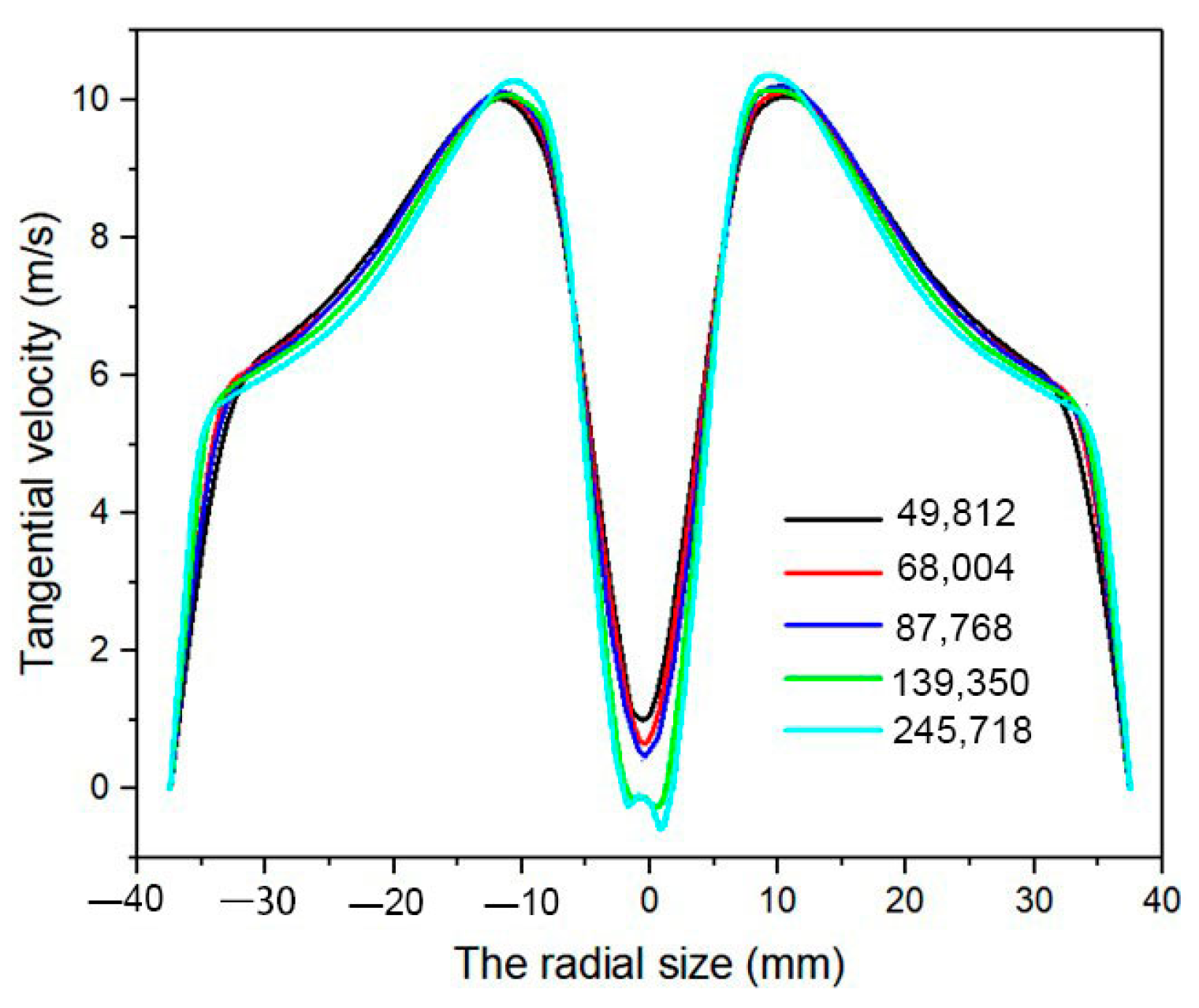
3.2. Mesh Independence Verification
3.3. Model Validation
4. Results Analysis
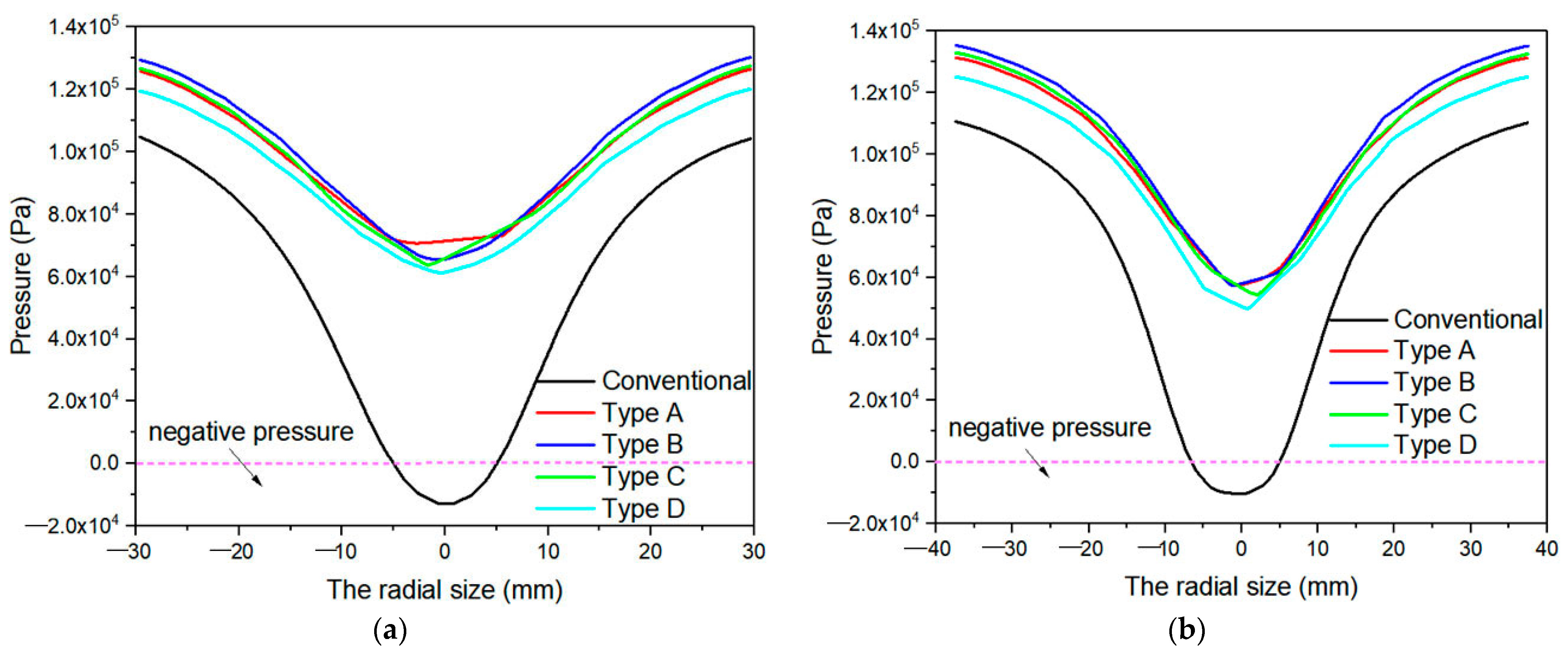
4.1. Pressure Analysis
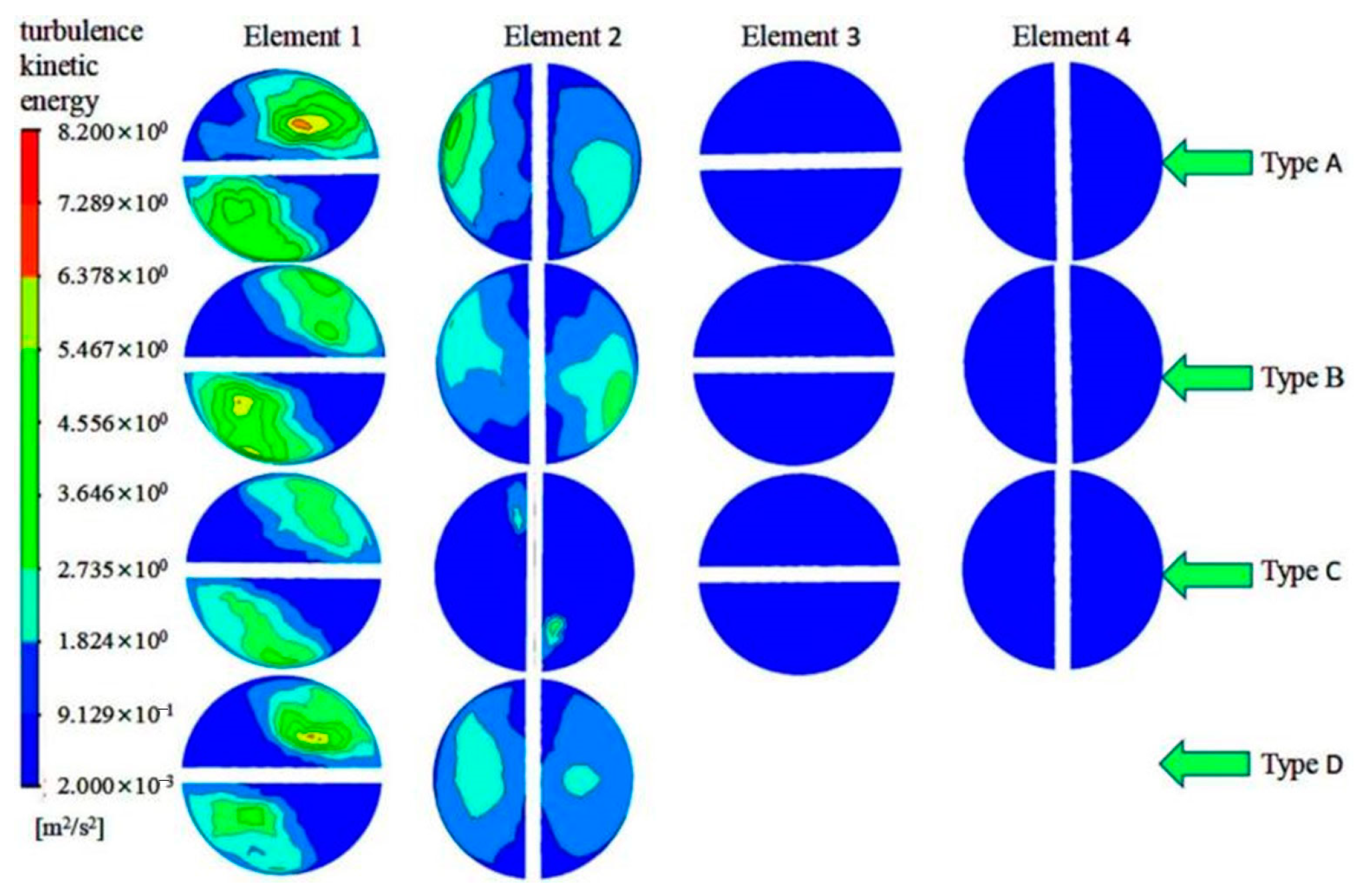
4.2. Turbulence Intensity Analysis
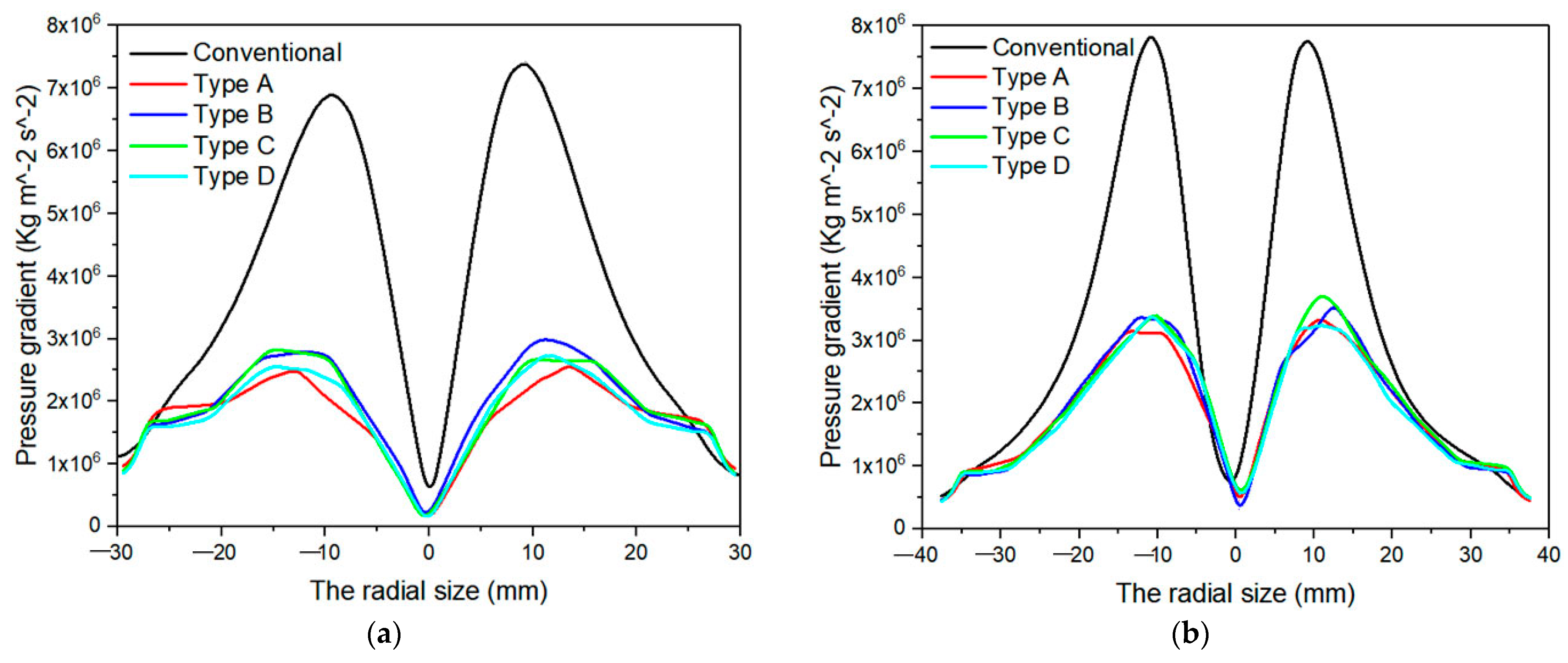
4.3. Analysis of Pressure Gradient
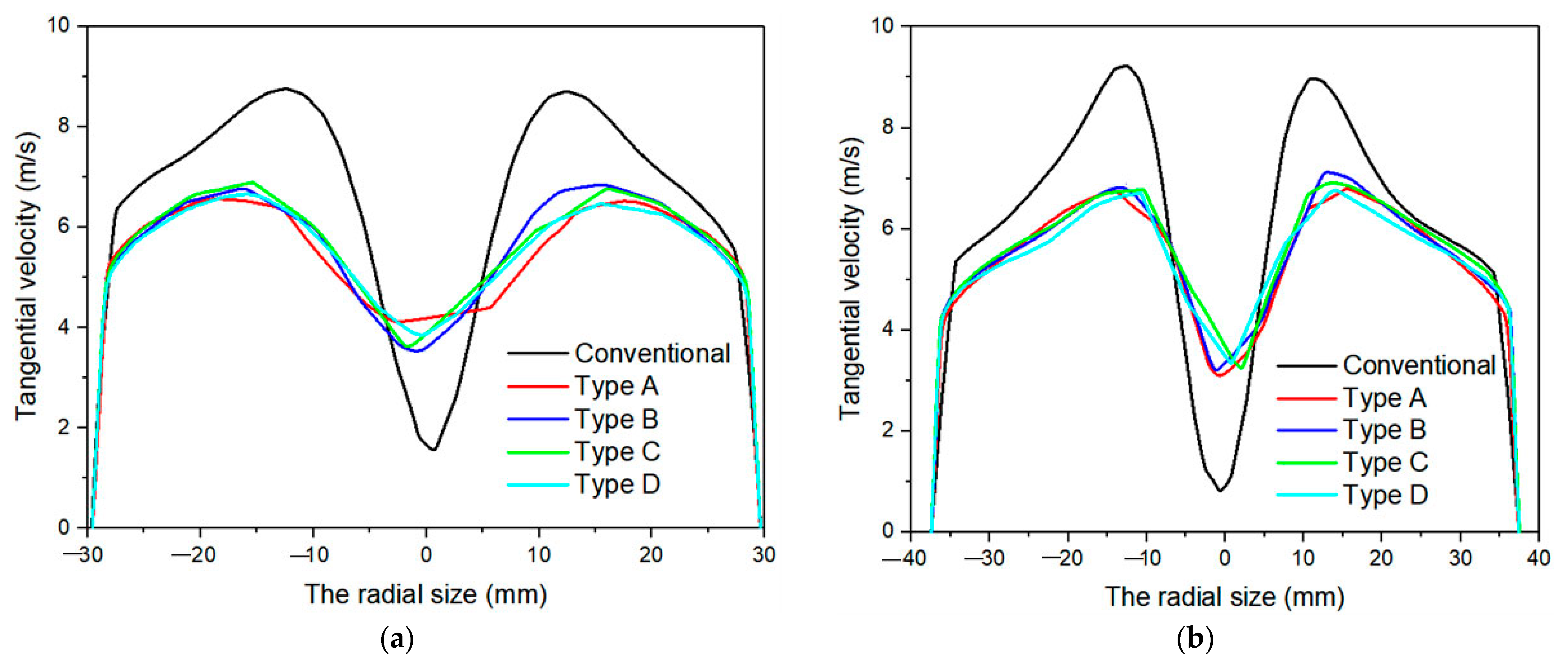
4.4. Analysis of Tangential Velocity
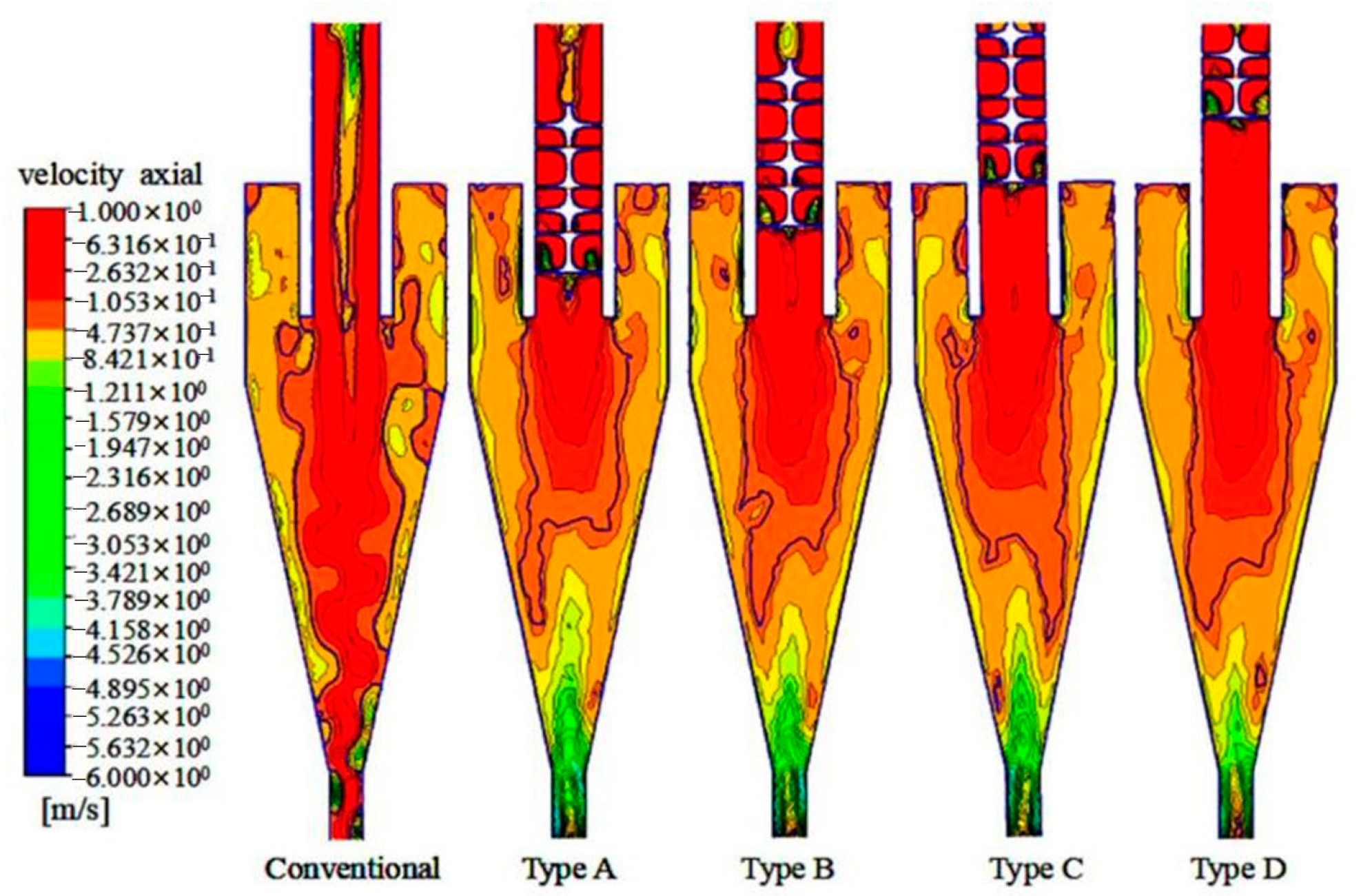
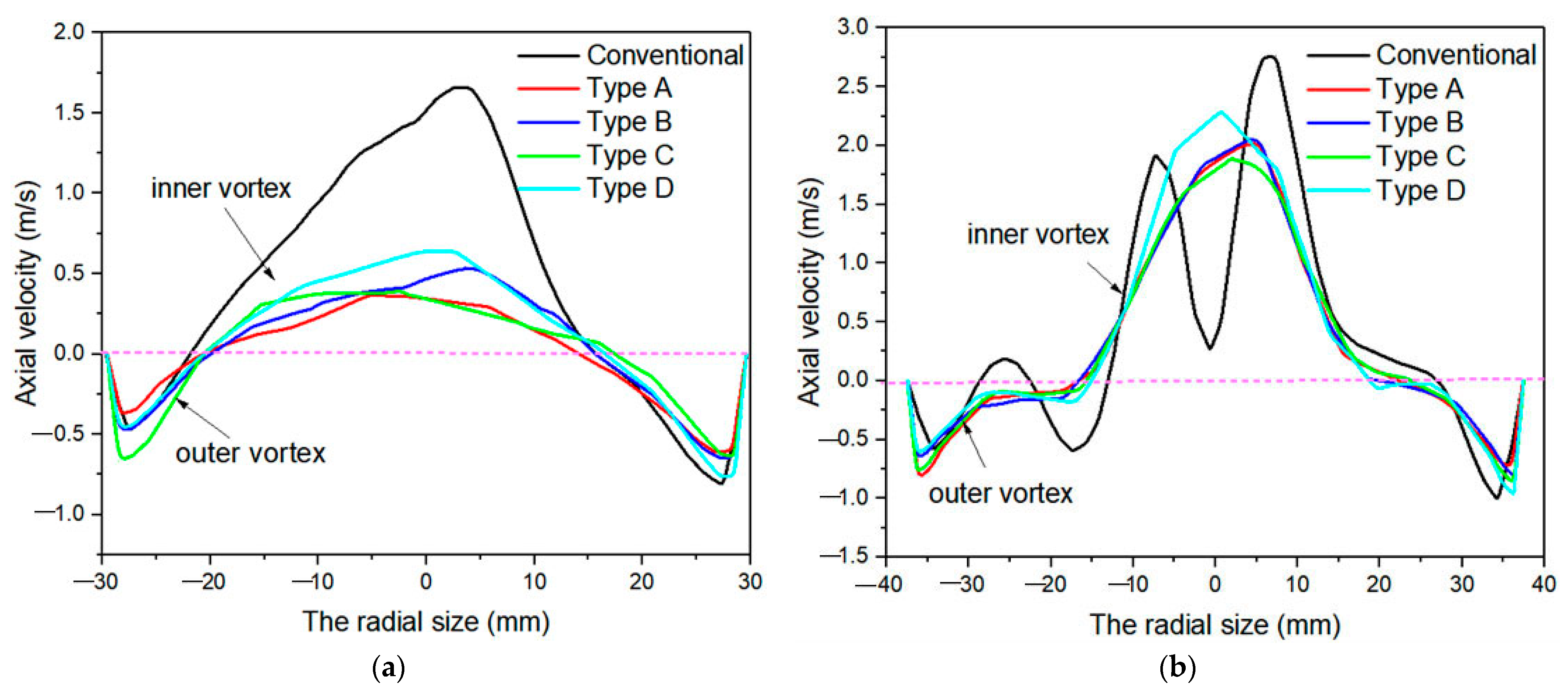
4.5. Analysis of Axial Velocity
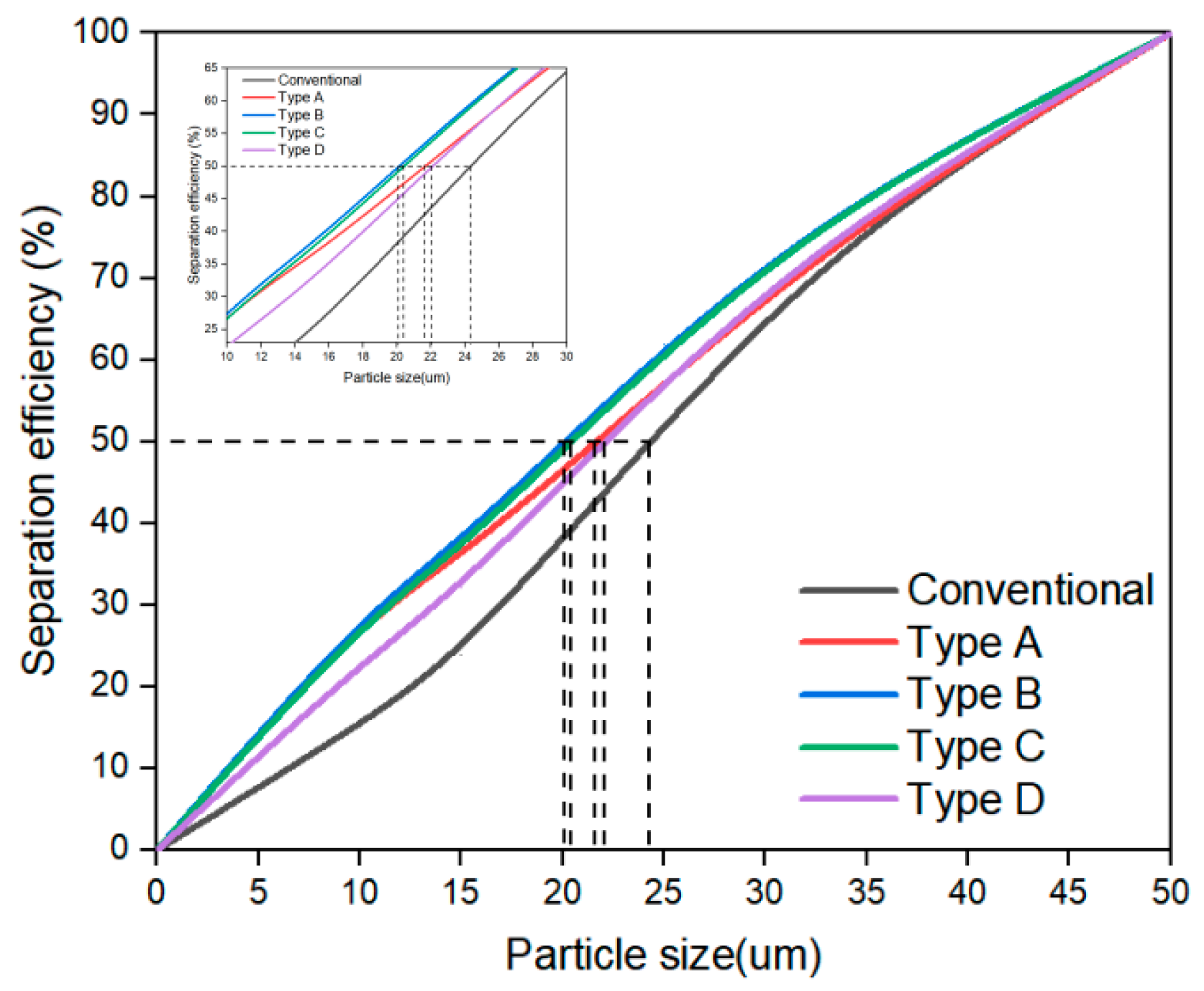
4.6. Analysis of Separation Efficiency, Pressure Drop, and Split Ratio
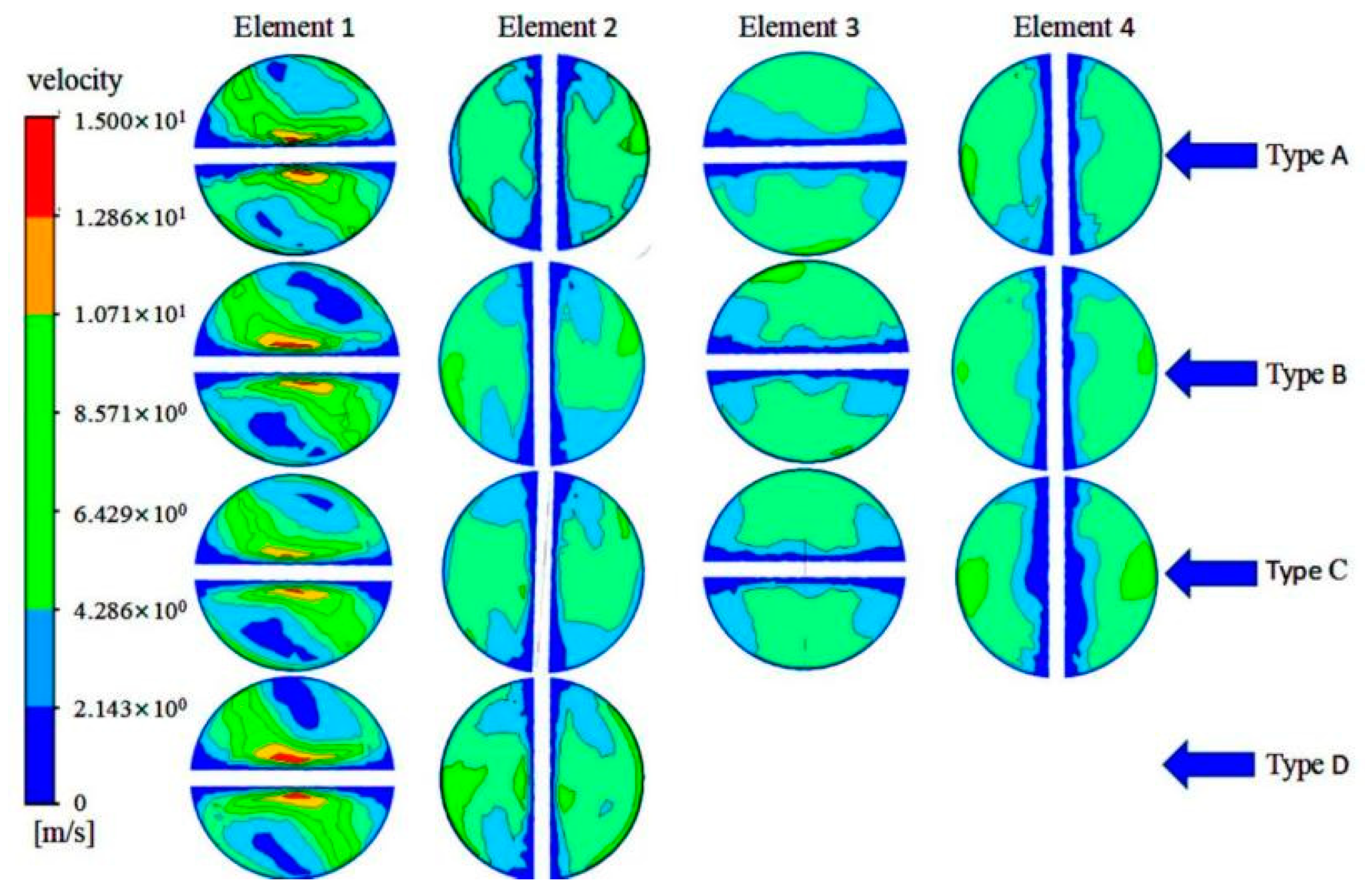
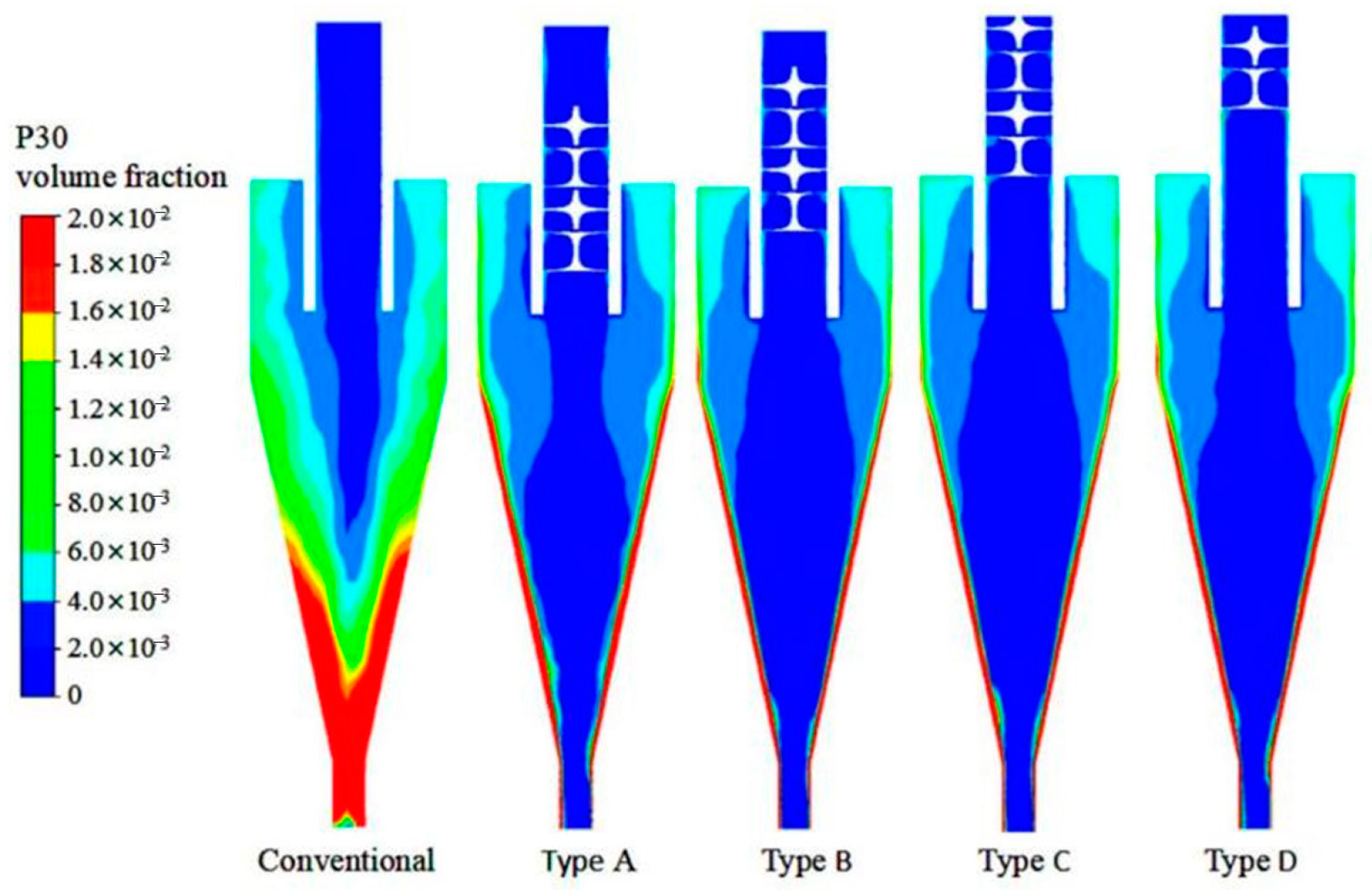
4.7. Analysis of Particle Cloud Chart
5. Conclusions
- A suitable spiral structure can reduce the pressure decrease of a hydrocyclone, and the Type B hydrocyclone has the smallest pressure drop with a 67.11% reduction compared to the conventional one.
- As the install position of the spiral structure rose, tangential velocity first increased and then reduced, and the tangential velocity was reduced by nearly 20% compared with the conventional hydrocyclone.
- As the install position of the spiral structure rose, cutting size first decreased and then increased, and minimum cutting size of 20.1 μm can be observed in the Type B hydrocyclone.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Atkinson, R.W.; Mills, I.C.; Walton, H.A.; Anderson, H.R. Fine particle components and health-a systematic review and meta-analysis of epidemiological time series studies of daily mortality and hospital admissions. J. Expo. Sci. Environ. Epidemiol. 2015, 25, 208–214. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.D.; Wang, S.X.; Jiang, J.K.; Ding, A.J.; Zheng, M.; Zhao, B.; Wong, D.C.; Zhou, W.; Zheng, G.J.; Wang, L.; et al. Impact of aerosol-meteorology interactions on fine particle pollution during China’s severe haze episode in January 2013. Environ. Res. Lett. 2014, 9, 094002. [Google Scholar] [CrossRef]
- Thurston, G.D.; Burnett, R.T.; Turner, M.C.; Shi, Y.L.; Krewski, D.; Lall, R.; Ito, K.; Jerrett, M.; Gapstur, S.M.; Diver, W.R.; et al. Ischemic Heart Disease Mortality and Long-Term Exposure to Source-Related Components of U.S. Fine Particle Air Pollution. Environ. Health Perspect. 2016, 124, 785. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.P.; Bevis WL, M.; Leong, E.; Kan, H.S.; Ip, S.-K.; Liu, T.K. Optimized centre-feed clarifier design for the Tai Po Sewage Treatment Works, Hong Kong. Water Environ. J. 2010, 24, 140–146. [Google Scholar] [CrossRef]
- Alanya, S.; Yilmazel, Y.D.; Park, C.; Willis, J.L.; Keaney, J.; Kohl, P.M.; Hunt, J.A.; Duran, M. Anaerobic co-digestion of sewage sludge and primary clarifier skimmings for increased biogas production. Water Sci. Technol. J. Int. Assoc. Water Pollut. Res. 2013, 67, 174–179. [Google Scholar] [CrossRef]
- Lossing, H.; Champagne, P.; McLellan, P.J. Examination of sludge accumulation rates and sludge characteristics for a decentralized community wastewater treatment systems with individual primary clarifier tanks located in Wardsville (Ontario, Canada). Water Sci. Technol. 2010, 62, 2944–2952. [Google Scholar] [CrossRef] [PubMed]
- Kyriakidis, Y.N.; Silva, D.O.; Barrozo, M.A.S.; Vieira, L.G.M. Effect of variables related to the separation performance of a hydrocyclone with unprecedented geometric relationships. Powder Technol. 2018, 338, 645–653. [Google Scholar] [CrossRef]
- Mognon, J.L.; Silva, J.M.D.; Bicalho, I.C.; Ataide, C.H.; Duarte, C.R. Modular mini-hydrocyclone desilter type of 30 mm: An experimental and optimization study. Petrol. Sci. Eng. 2015, 129, 145–152. [Google Scholar] [CrossRef]
- Zhang, L.J.; Xia, X.H.; Zhang, J.F. Improving energy efficiency of cyclone circuits in coal beneficiation plants by pump-storage systems. Appl. Energy 2014, 119, 306–313. [Google Scholar] [CrossRef]
- Ghodrat, M.; Qi, Z.; Kuang, S.B.; Ji, L.; Yu, A.B. Computational investigation of the effect of particle density on the multiphase flows and performance of hydrocyclone. Miner. Eng. 2016, 90, 55–69. [Google Scholar] [CrossRef]
- Razmi, H.; Goharrizi, A.S.; Mohebbi, A. CFD simulation of an industrial hydrocyclone based on multiphase particle in cell (MPPIC) method. Sep. Purif. Technol. 2018, 209, 851–862. [Google Scholar] [CrossRef]
- Liu, L.; Zhao, L.X.; Sun, Y.; Gao, S.; Jiang, M.Z.; Jiang, M.H.; Rosso, D. Separation performance of hydrocyclones with medium rearrangement internals. J. Environ. Chem. Eng. 2021, 9, 105642. [Google Scholar] [CrossRef]
- Kumar, M.; Reddy, R.; Banerjee, R.; Mangadoddy, N. Effect of particle concentration on turbulent modulation inside hydrocyclone using coupled MPPIC-VOF method. Sep. Purif. Technol. 2021, 266, 118206. [Google Scholar] [CrossRef]
- Tian, J.Y.; Ni, L.; Song, T.; Zhao, J.N. CFD simulation of hydrocyclone-separation performance influenced by reflux device and different vortex-finder lengths. Sep. Purif. Technol. 2020, 233, 116013. [Google Scholar] [CrossRef]
- Lv, W.J.; Chen, J.Q.; Chang, Y.L.; Liu, H.L.; Wang, H.L. UU-type parallel mini-hydrocyclone group separation of fine particles from methanol-to-olefin industrial wastewater. Chem. Eng. Process.-Process Intensif. 2018, 131, 34–42. [Google Scholar] [CrossRef]
- Gayatree, P.; Priyam, D.; Chakraborty, S.; Meikap, B.C. Removal of fluoride from wastewater using HCl-treated activated alumina in a ribbed hydrocyclone separator. J. Environ. Sci. Health. Part A Toxic/Hazard. Subst. Environ. Eng. 2018, 53, 601–608. [Google Scholar] [CrossRef]
- Li, S.H.; Liu, Z.M.; Chang, Y.L.; Li, J.P.; Hu, J.K.; Shen, Q.S.; Wang, H.L. Removal of coke powders in coking wastewater using a hydrocyclone optimized by n-value. Sci. Total Environ. 2021, 752, 141887. [Google Scholar] [CrossRef] [PubMed]
- Yu, J.F.; Fu, J. Separation performance of an 8 mm mini-hydrocyclone and its application to the treatment of rice starch wastewater. Sep. Sci. Technol. 2020, 55, 313–320. [Google Scholar] [CrossRef]
- Youngseok, H.; Minsoo, M.; Seok, D. Development of a hybrid system for advanced wastewater treatment using high-rate settling and a flotation system with ballasted media. Int. Biodeterior. Biodegrad. 2016, 113, 256–261. [Google Scholar] [CrossRef]
- Gao, S.L.; Wei, D.Z.; Liu, W.G.; Ma, L.Q.; Lu, T.; Zhang, R.Y. CFD numerical simulation of flow velocity characteristics of hydrocyclone. Trans. Nonferrous Met. Soc. China 2011, 21, 2783–2789. [Google Scholar] [CrossRef]
- Hwang, K.J.; Hwang, Y.W.; Yoshida, H. Design of novel hydrocyclone for improving fine particle separation using computational fluid dynamics. Chem. Eng. Sci. 2011, 85, 62–68. [Google Scholar] [CrossRef]
- Padhi, M.; Mangadoddy, N.; Sreenivas, T.; Vakamalla, T.R.; Mainza, A.N. Study on multi-component particle behaviour in a hydrocyclone classifier using experimental and computational fluid dynamics techniques. Sep. Purif. Technol. 2019, 229, 115698. [Google Scholar] [CrossRef]
- Guo, D.; Jiang, X.Y.; Guo, M.Z.; Zeng, M.; Wu, N.; Hao, L.L.; Wang, C. Role of hydrocyclone separator on the formation and separation of aerobic granular sludge: Evaluating granulation efficiency and simulating hydrodynamic behavior. Sep. Purif. Technol. 2022, 283, 120231. [Google Scholar] [CrossRef]
- Zhao, Q.; Cui, B.Y.; Wei, D.Z.; Song, T.; Feng, Y.Q. Numerical analysis of the flow field and separation performance in hydrocyclones with different vortex finder wall thickness. Powder Technol. 2019, 345, 478–491. [Google Scholar] [CrossRef]
- Tripathy, S.K.; Bhoja, S.K.; Kumar, C.R.N. Suresh A short review on hydraulic classification and its development in mineral industry. Powder Technol. 2015, 270, 205–220. [Google Scholar] [CrossRef]
- Li, F.; Liu, P.K.; Yang, X.H.; Zhang, Y.K. Numerical simulation on the effects of different inlet pipe structures on the flow field and seperation performance in a hydrocyclone. Powder Technol. 2020, 373, 254–266. [Google Scholar] [CrossRef]
- Yang, X.H.; Simmons, M.J.H.; Liu, P.K.; Zhang, Y.K.; Jiang, L.Y. Effect of feed body geometry on separation performance of hydrocyclone. Sep. Sci. Technol. 2019, 54, 2959–2970. [Google Scholar] [CrossRef]
- Raviraju, V.; Kumar, P.A.; Sivakumar, S. Computational investigation of a novel hydrocyclone for fines bypass reduction. Powder Technol. 2022, 395, 501–515. [Google Scholar] [CrossRef]
- Hou, D.X.; Cui, B.Y.; Zhao, Q. Research on the structure of the cylindrical hydrocyclone spigot to mitigate the misplacement of particles. Powder Technol. 2021, 387, 61–71. [Google Scholar] [CrossRef]
- Vega-Garciaa, D.; Cilliersb, J.J.; Brito-Parada, P.R. CFD modelling of particle classification in mini-hydrocyclones. Sep. Purif. Technol. 2020, 251, 117253. [Google Scholar] [CrossRef]
- Hou, D.X.; Cui, B.Y.; Zhang, H.; Zhao, Q.; Ji, A.K.; Wei, D.Z.; Feng, Y.Q. Designing the hydrocyclone with flat bottom structure for weakening bypass effect. Powder Technol. 2021, 394, 724–734. [Google Scholar] [CrossRef]
- Hsieh, K.T. Phenomenological Model of the Hydrocyclone; The University of Utah: Salt Lake City, UT, USA, 1988. [Google Scholar]
- Delgadillo, J. Modeling of 75 and 250 mm Hydrocyclones and Exploration of Novel Design Using Computation Fluid Dhnamics. Ph.D. Thesis, The University of Utah, Salt Lake City, UT, USA, 2006. [Google Scholar]
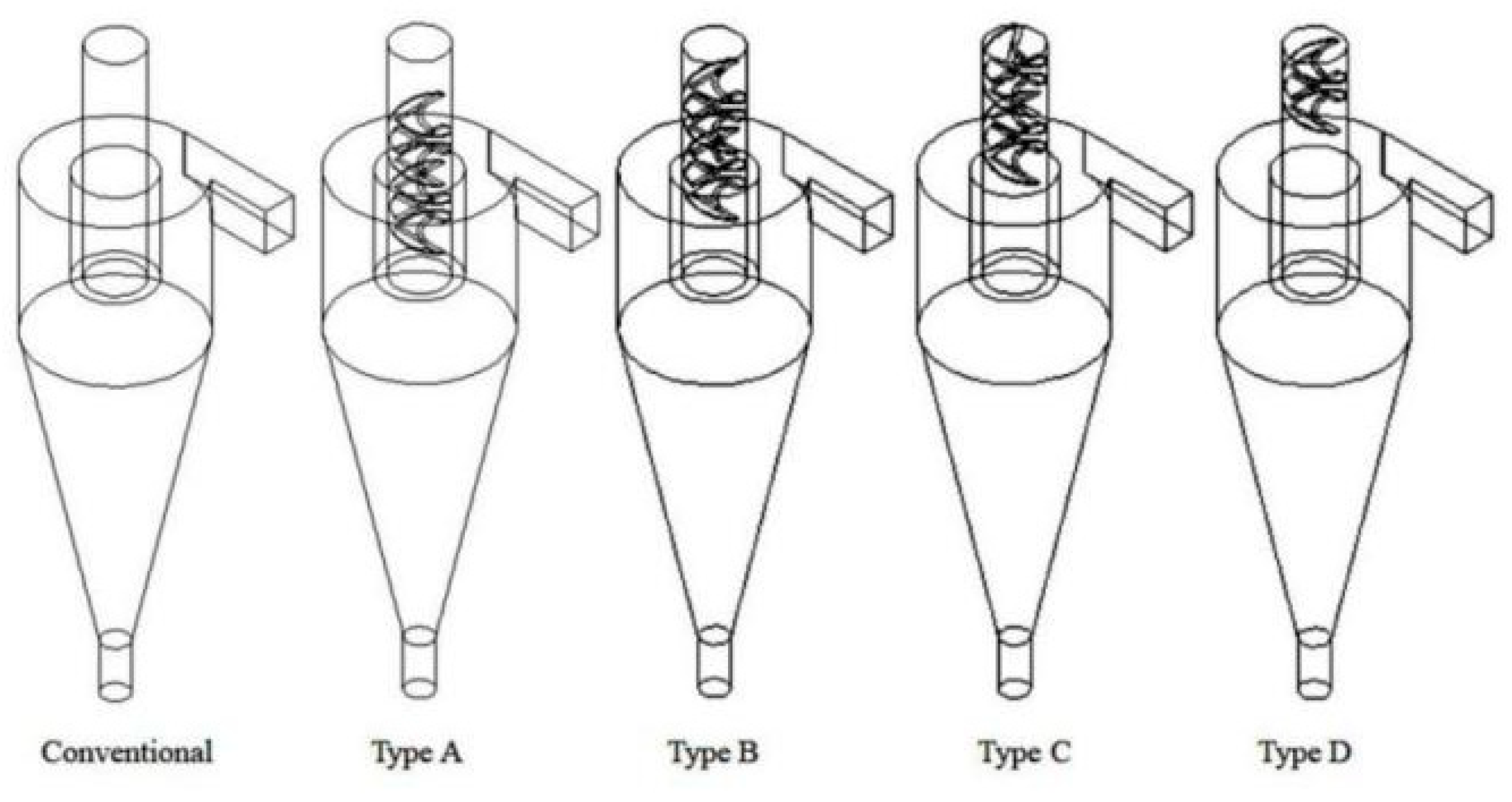

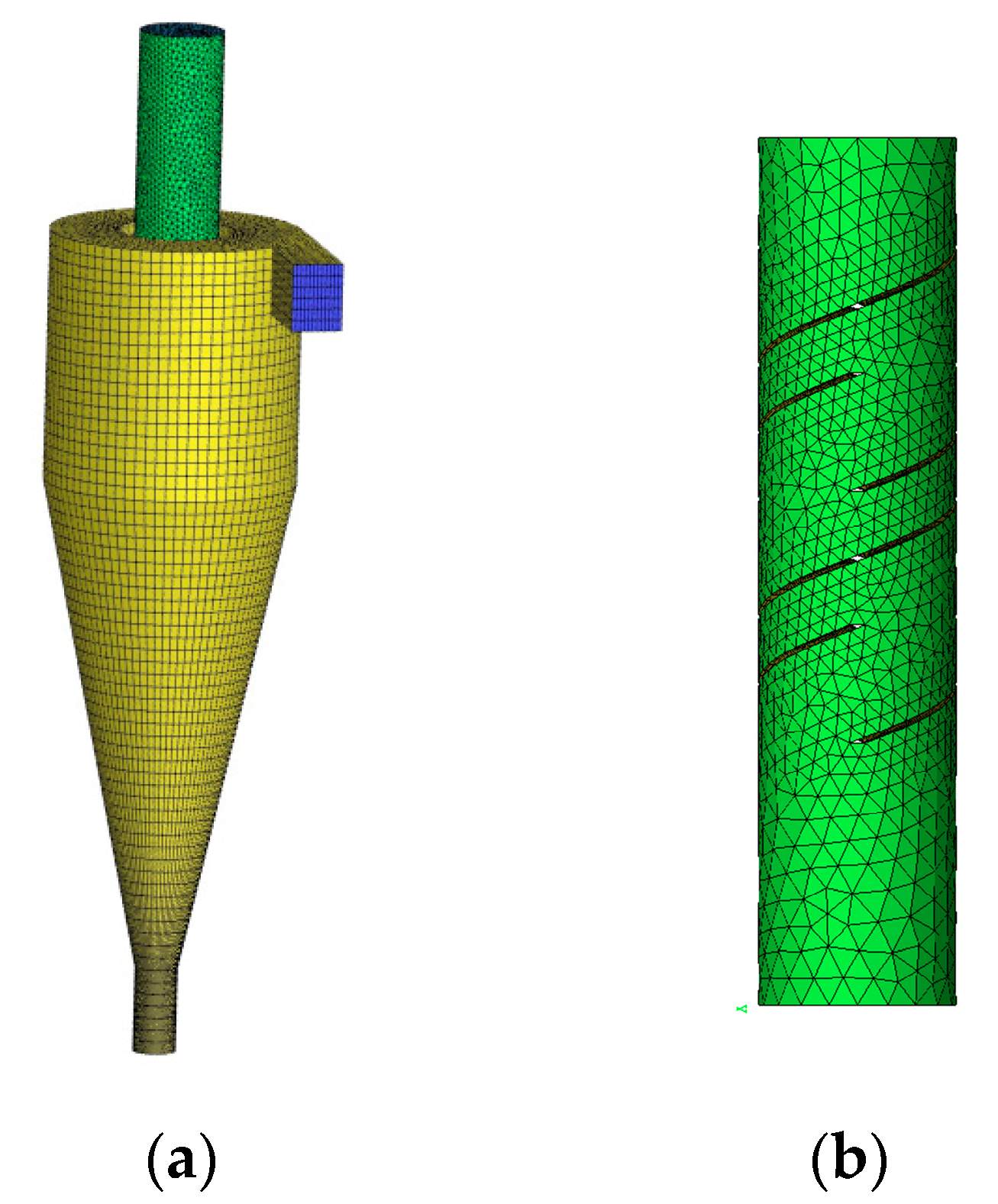








| Symbol/Units | Structure Parameter | Spiral Structure Size | Conventional |
|---|---|---|---|
| D/mm | Column diameter | 75 | 75 |
| Di/mm | Inlet equivalent diameter | 17 | 17 |
| Do/mm | Overflow diameter | 25 | 25 |
| Du/mm | Underflow diameter | 12.5 | 12.5 |
| H1/mm | Insertion depth | 50 | 50 |
| H2/mm | Column length | 75 | 75 |
| H3/mm | Cone length | 146 | 146 |
| H4/mm | Underflow length | 25 | 25 |
| L/mm | Spiral thickness | 2 | 2 |
| P/mm | Pitch | 30 |
| Size Interval/μm | Mean Size/μm | Volumetric Feed Concentration/% |
|---|---|---|
| −50 + 45 | 50 | 0.3 |
| −45 + 40 | 40 | 0.3 |
| −40 + 35 | 35 | 0.5 |
| −35 + 20 | 20 | 1.0 |
| −20 + 15 | 15 | 0.5 |
| −15 + 10 | 10 | 0.3 |
| −10 + 0 | 5 | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, P.; Fu, W.; Jiang, L.; Yang, X.; Zhang, Y.; Li, X.; Zhang, Y. Effect of the Position of Overflow Pipe with Mixed Spiral Structures on the Separation Performance of Hydrocyclones. Separations 2023, 10, 84. https://doi.org/10.3390/separations10020084
Liu P, Fu W, Jiang L, Yang X, Zhang Y, Li X, Zhang Y. Effect of the Position of Overflow Pipe with Mixed Spiral Structures on the Separation Performance of Hydrocyclones. Separations. 2023; 10(2):84. https://doi.org/10.3390/separations10020084
Chicago/Turabian StyleLiu, Peikun, Wenxiu Fu, Lanyue Jiang, Xinghua Yang, Yuekan Zhang, Xiaoyu Li, and Yulong Zhang. 2023. "Effect of the Position of Overflow Pipe with Mixed Spiral Structures on the Separation Performance of Hydrocyclones" Separations 10, no. 2: 84. https://doi.org/10.3390/separations10020084
APA StyleLiu, P., Fu, W., Jiang, L., Yang, X., Zhang, Y., Li, X., & Zhang, Y. (2023). Effect of the Position of Overflow Pipe with Mixed Spiral Structures on the Separation Performance of Hydrocyclones. Separations, 10(2), 84. https://doi.org/10.3390/separations10020084







