A Comparative Study on the Modelling of Soybean Particles Based on the Discrete Element Method
Abstract
1. Introduction
2. Modelling Approach
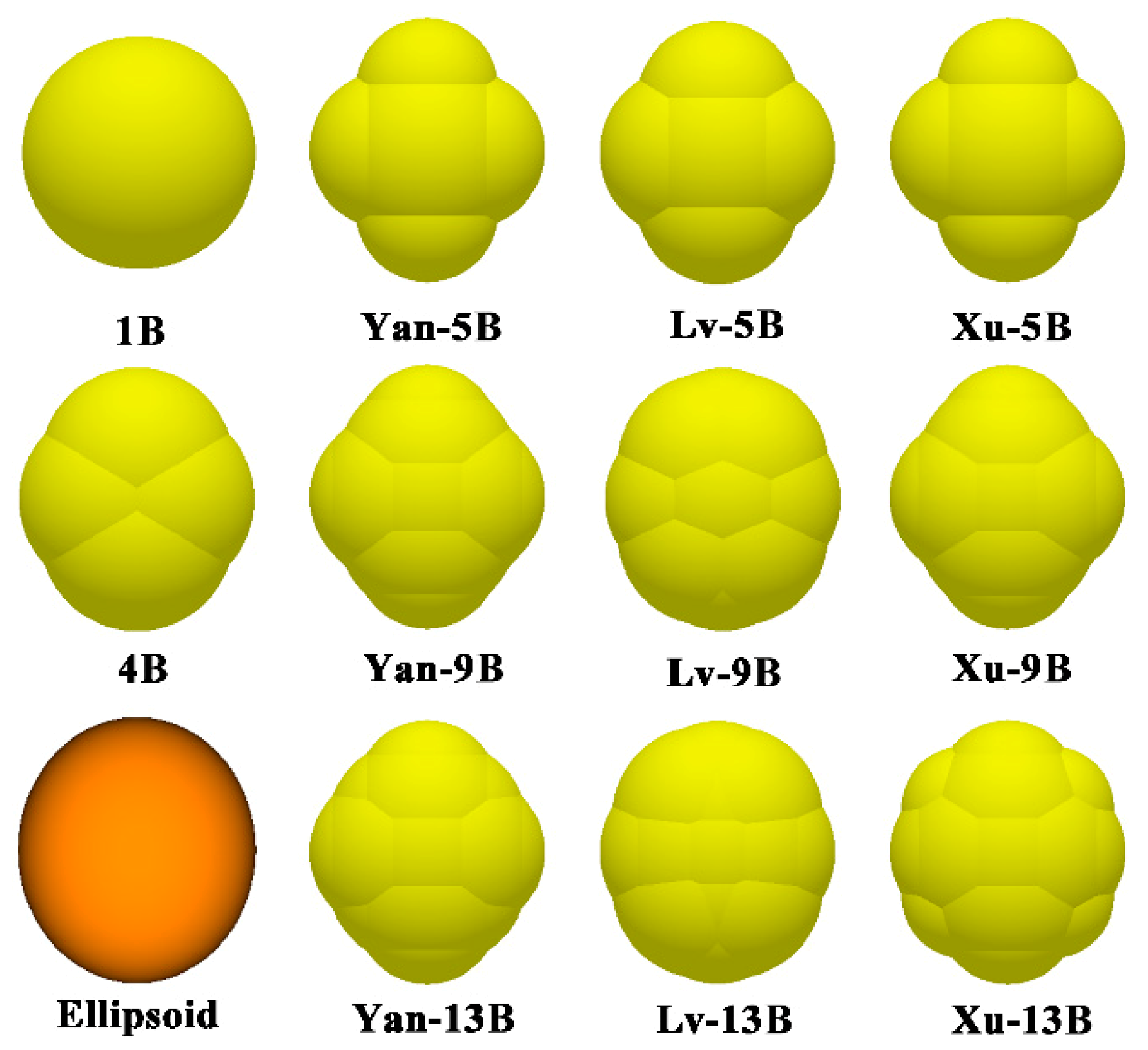
2.1. The Modelling Approach of the Five-Ball Model
2.2. The Modelling Approach of the Nine-Ball Model
2.3. The Modelling Approach of the 13-Ball Model
3. Comparison and Analysis of Different Approaches
3.1. Test Analysis
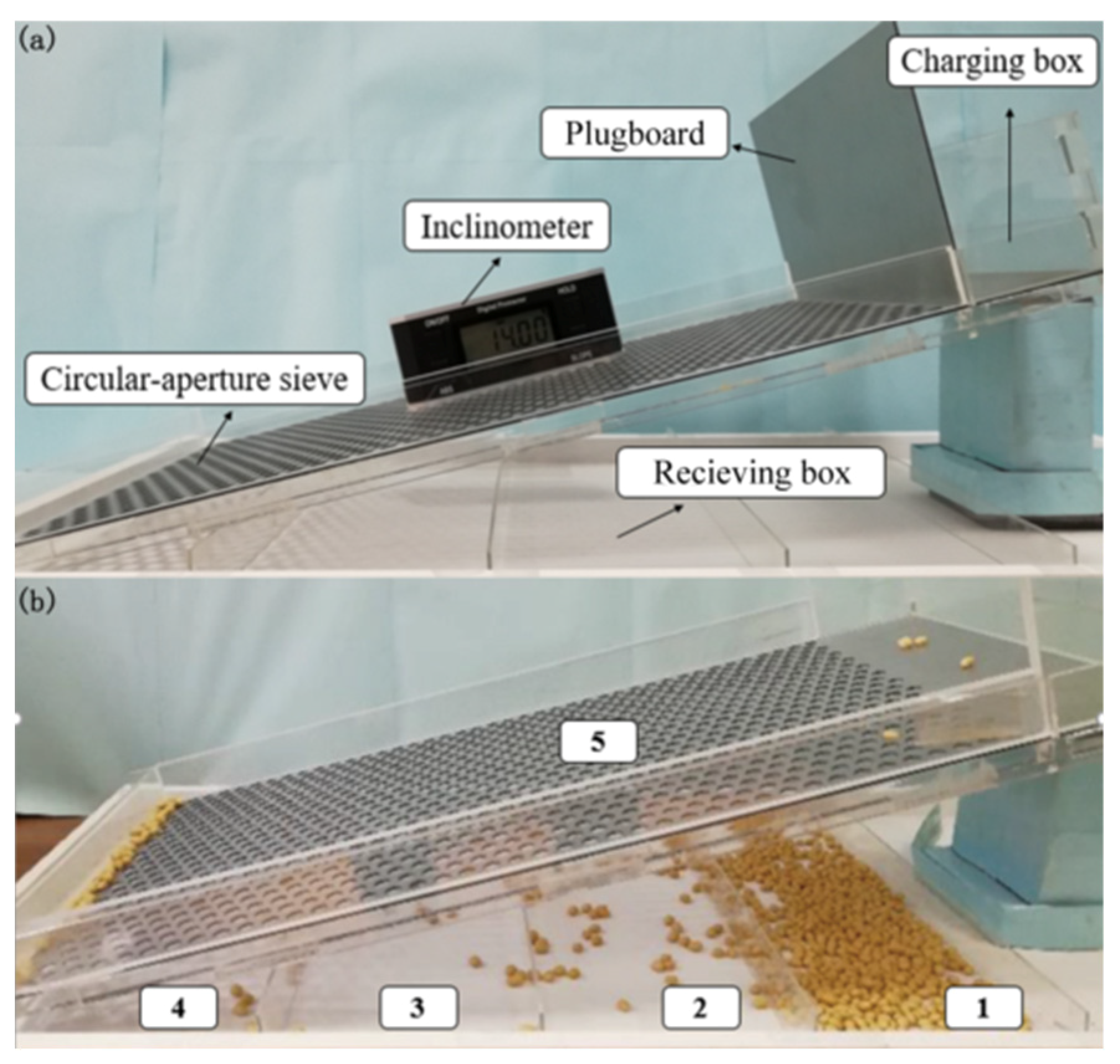
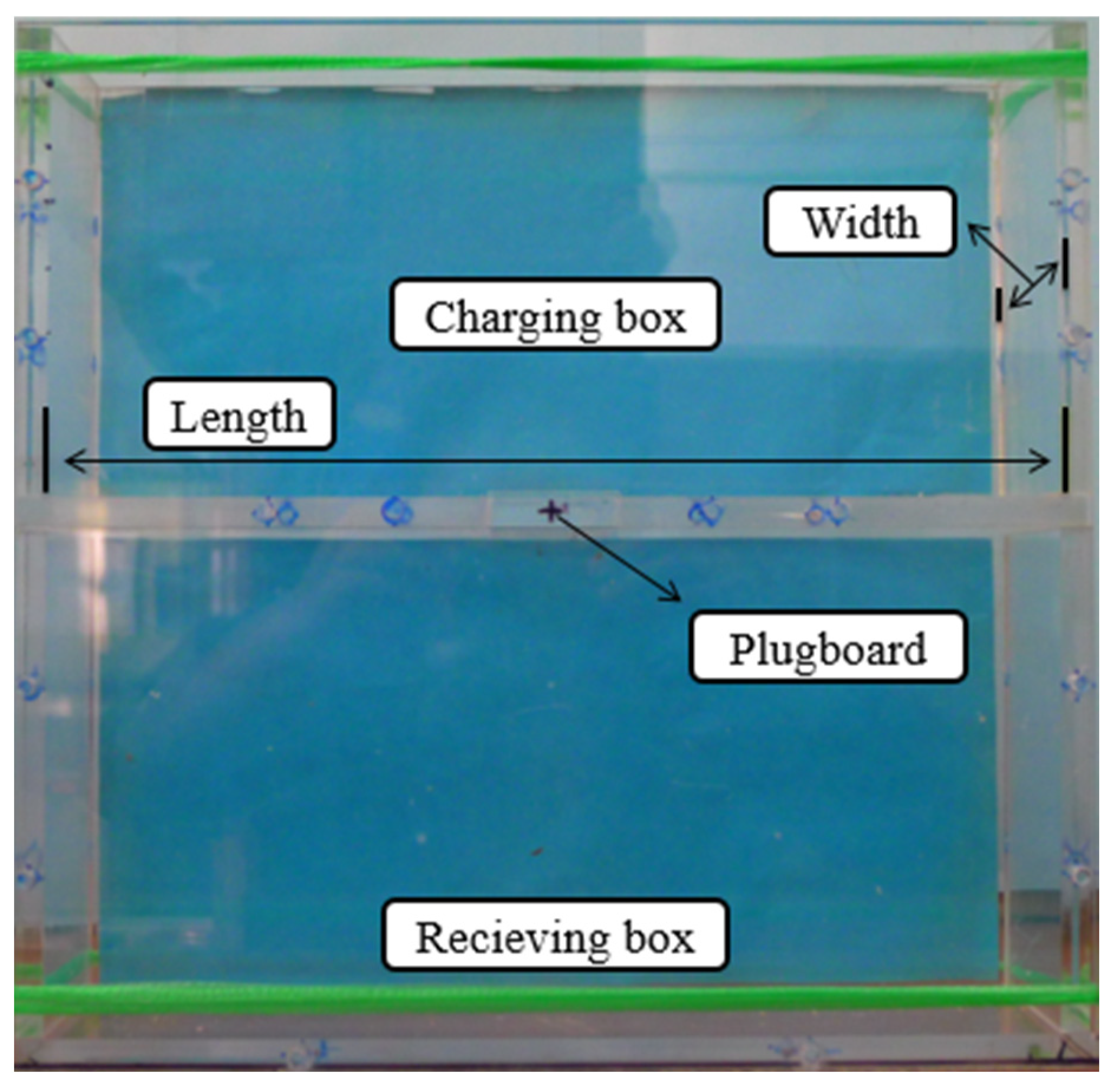
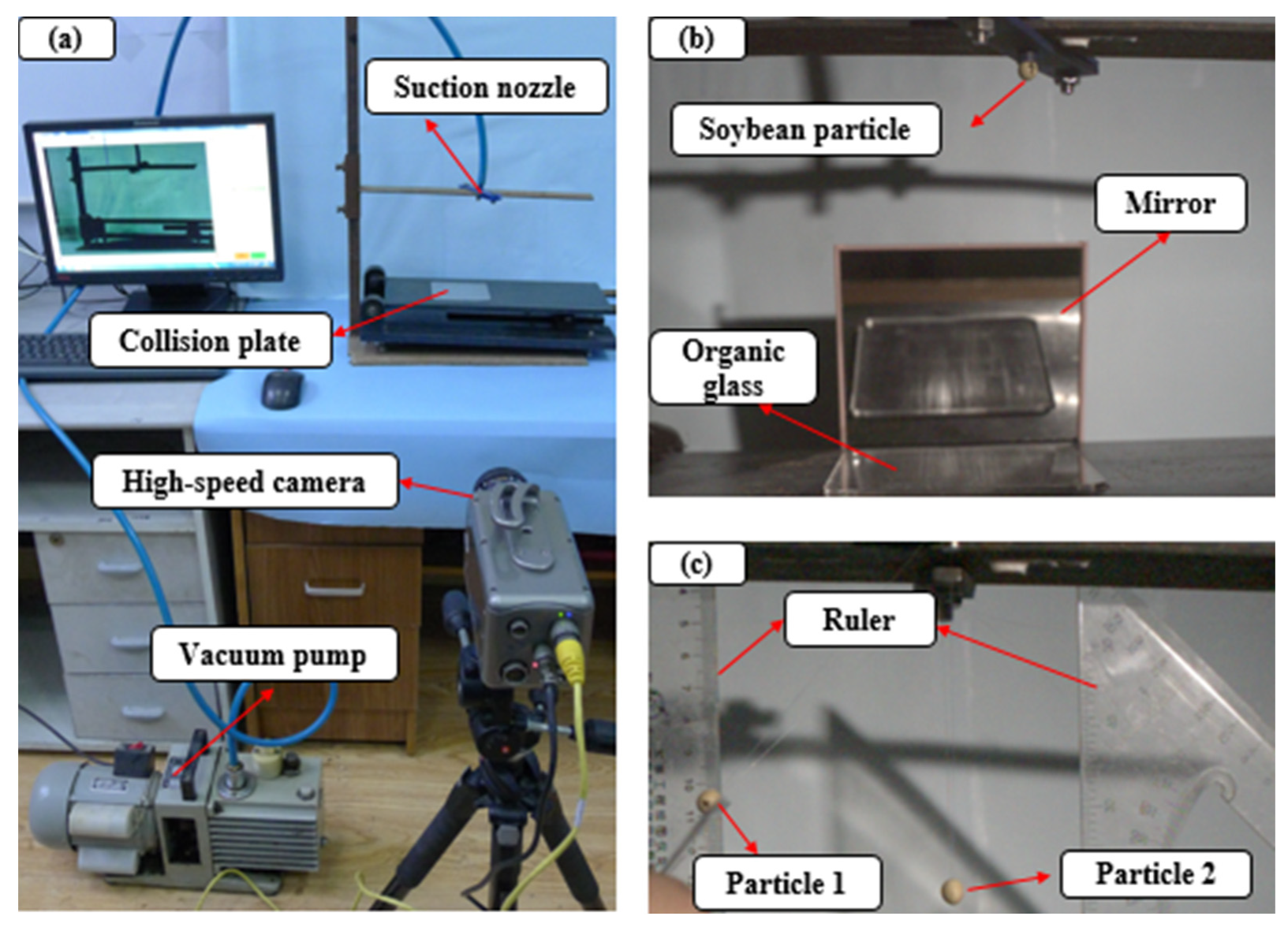
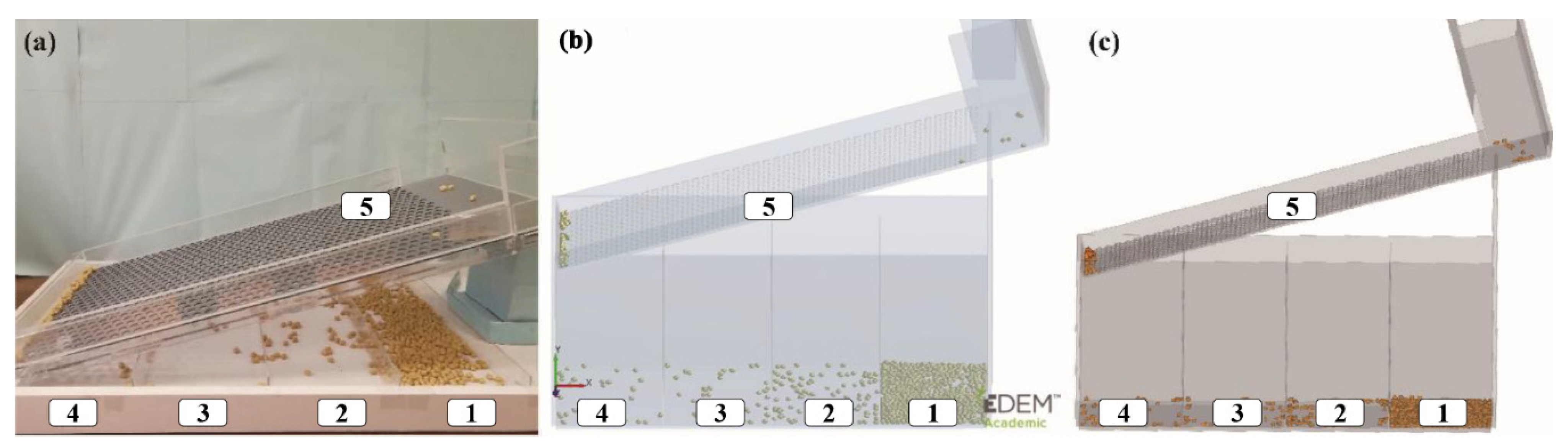
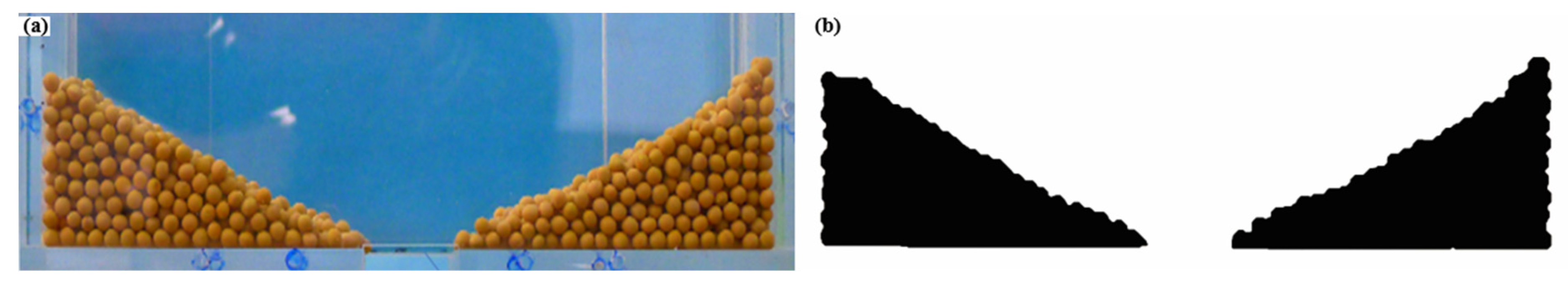
3.1.1. “Self-Flow Screening” Test
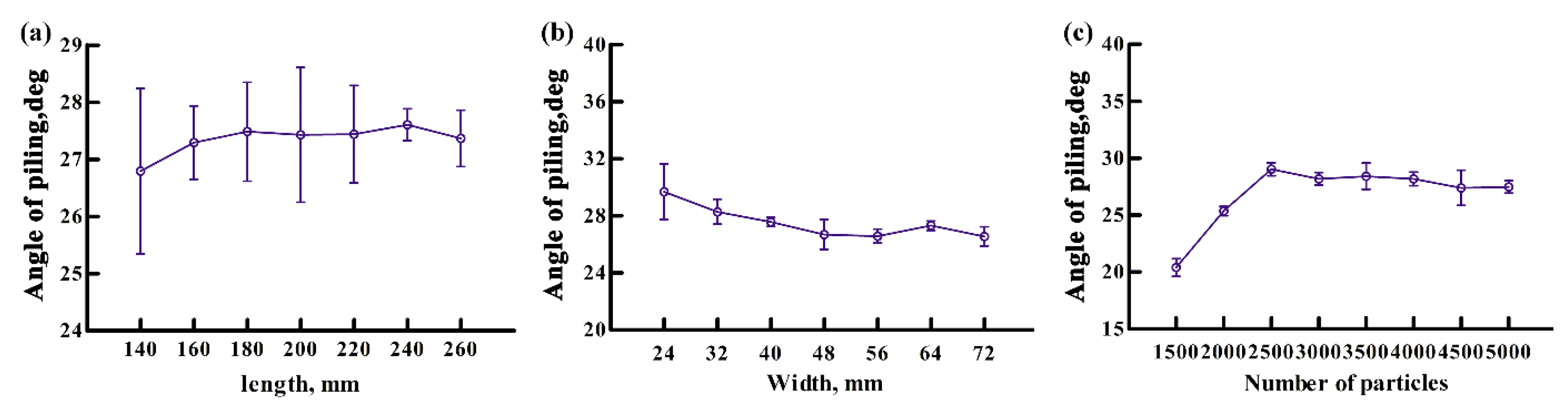
3.1.2. “Piling Angle” Test
3.2. Simulation Analysis
3.2.1. Selection of the Simulation Parameters
3.2.2. Generation of Assembly of Particles
3.2.3. Simulation Setup
- (1)
- “Self-flow screening” simulation
- (2)
- “Piling angle” simulation
3.3. Result Analysis
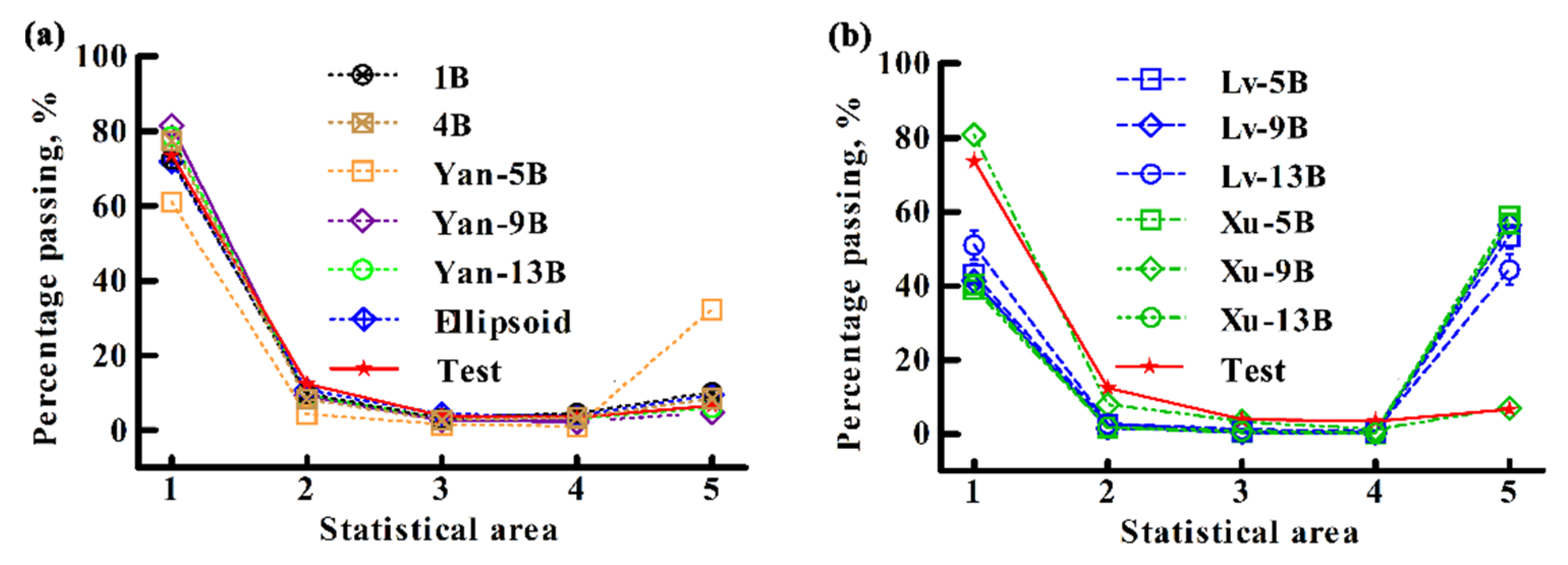
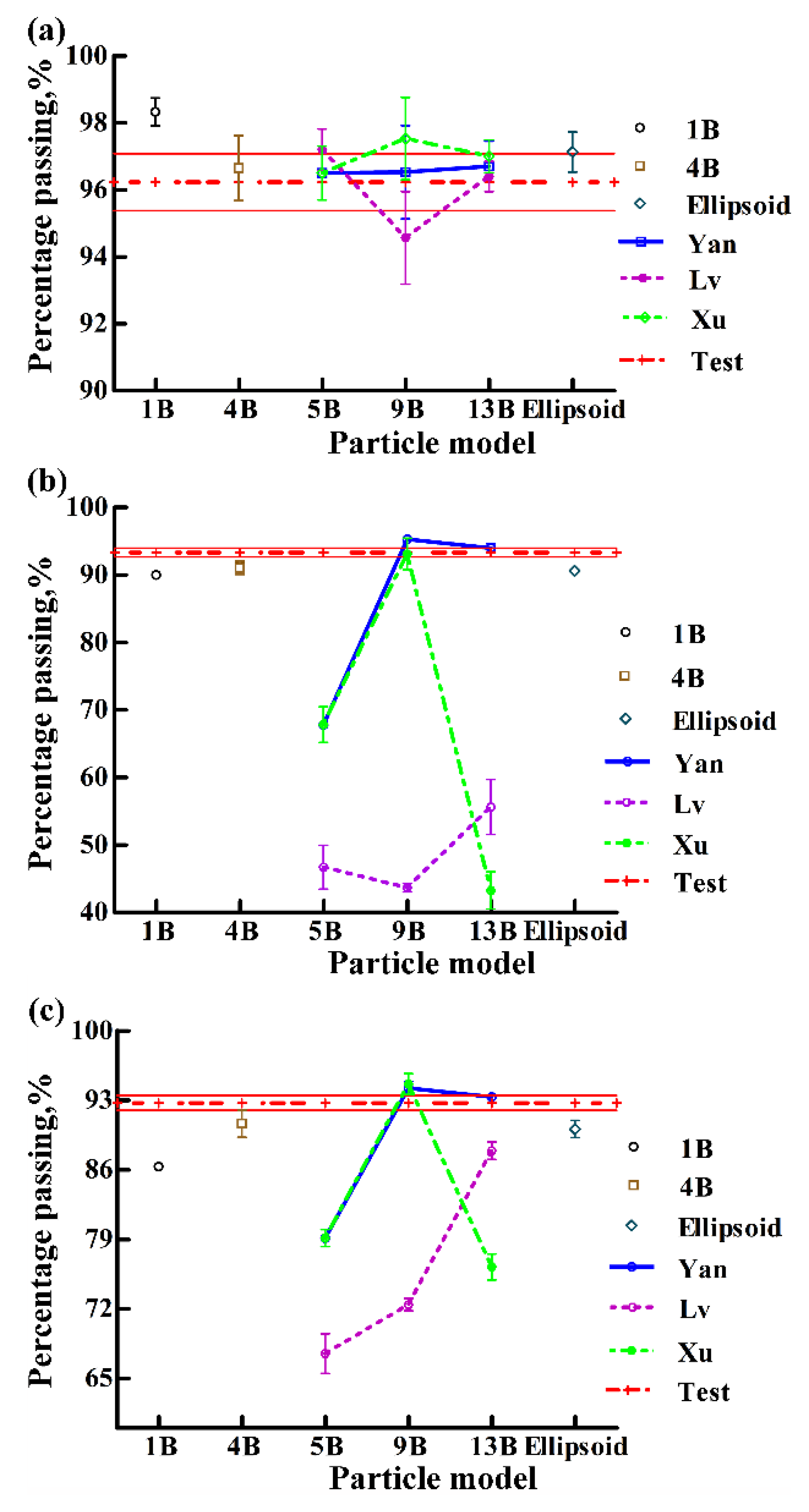
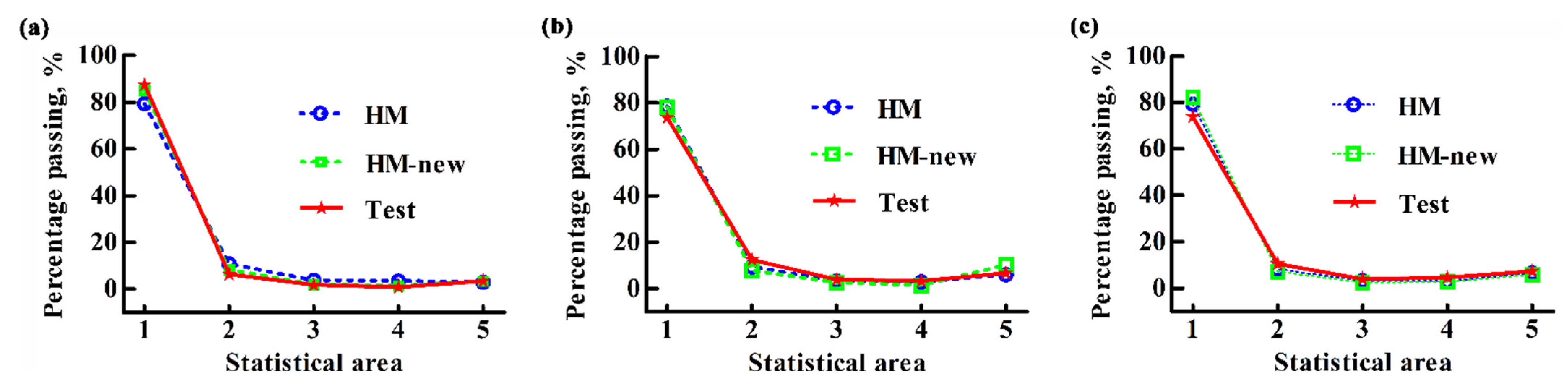
3.3.1. Result Analysis of the “Self-Flow Screening” Test
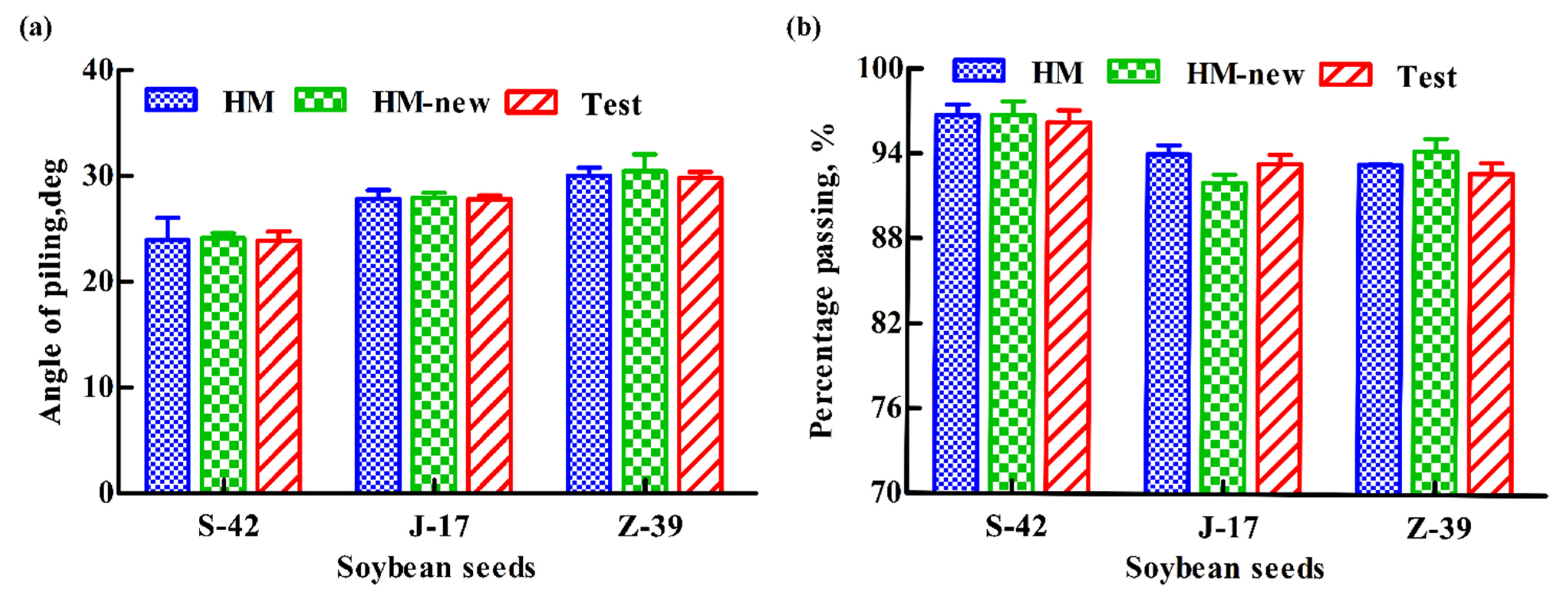
3.3.2. Result Analysis of the “Piling Angle” Test
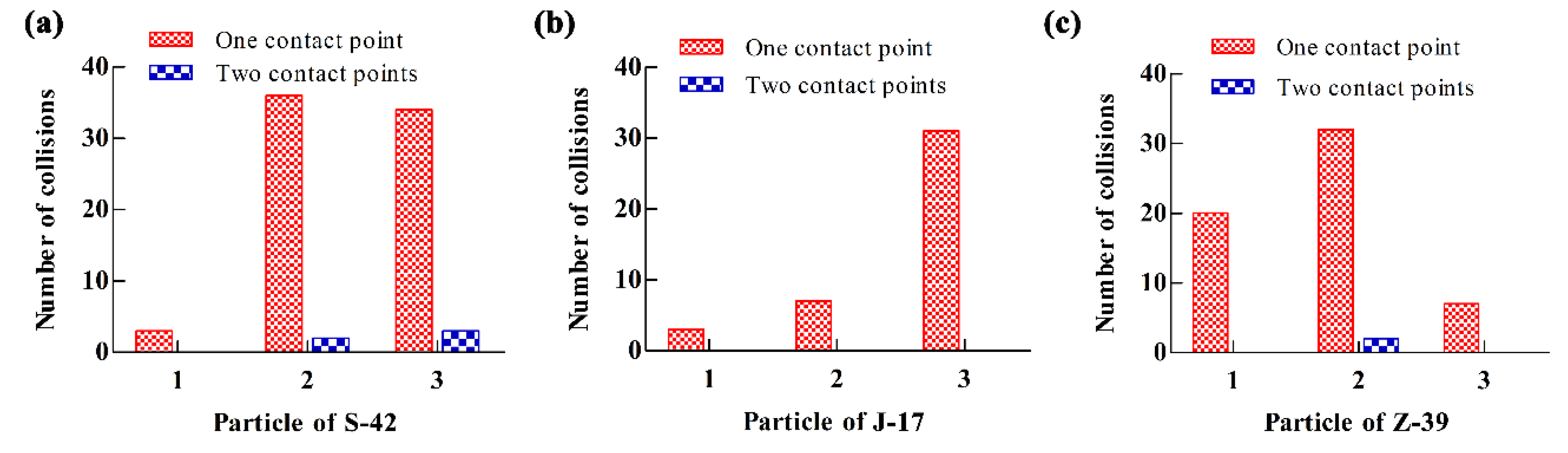
4. Influence of the Multiple Contacts
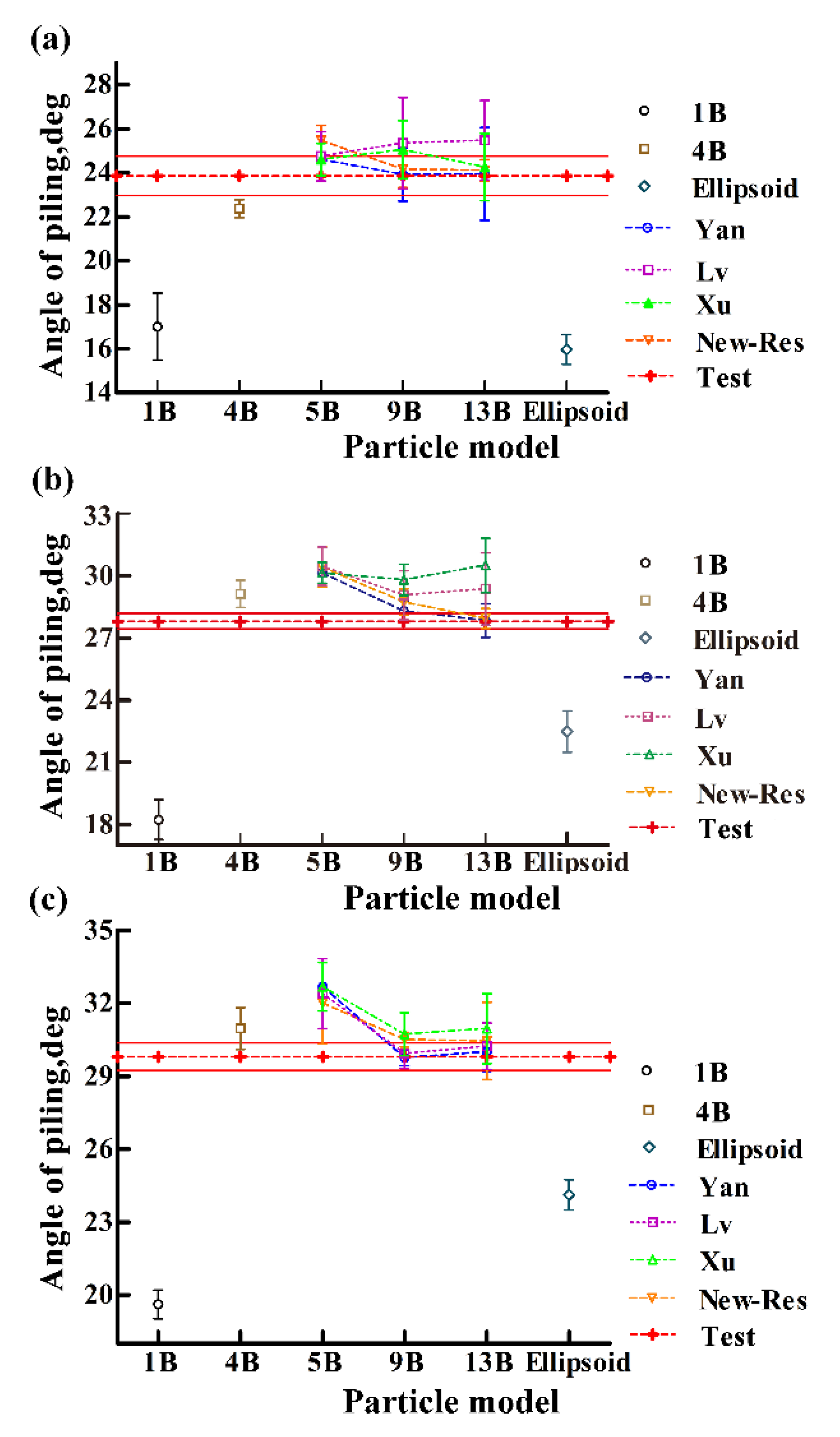
4.1. Simulation Analysis of the Multiple Contacts
4.2. Reason Analysis
5. Conclusions
- A novel modelling approach for soybean particles presented in this paper is introduced, namely one single soybean particle modelled by five, nine, and 13 balls “gluing” together, respectively.
- The multi-ball models presented in this paper are compared with the ellipsoidal model, as well as the models other researchers (home and abroad) established by comparison between the simulation results and the test results, in terms of the “self-flow screening” and “piling angle” tests. It is shown that the modelling approach presented in this paper has stronger applicability than other approaches. For the soybean particle with high sphericity, the five-ball model is appropriate; for those with low sphericity, the nine- and 13-ball models are more applicable. Additionally, if the time cost is not considered, and the consistency between the simulation and the experiment is only concerned, the 13-ball model is the most recommended.
- The simulation results of the “piling angle” and “self-flow screening” using the HM-new model are basically the same as those employing the HM model and are close to the test results. This proves that the multiple contacts has little impact on the movement of particles modelled by the multi-ball approach.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cundall, P.A.; Strack, O.L. A discrete numerical model for granular assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Lu, Z.; Negi, S.C.; Jofriet, J.C. A numerical model for flow of granular materials in silos. Part 1: Model development. J. Agric. Eng. Res. 1997, 68, 223–229. [Google Scholar] [CrossRef]
- Vu-Quoc, L.; Zhang, X.; Walton, O.R. A 3-D discrete-element method for dry granular flows of ellipsoidal particles. Comput. Methods Appl. Mech. Eng. 2000, 187, 483–528. [Google Scholar] [CrossRef]
- Boac, J.M.; Casada, M.E.; Maghirang, R.G.; Harner, J.P. Material and interaction properties of selected grains and oilseeds for modeling discrete particles. Trans. ASABE 2010, 53, 1201–1216. [Google Scholar] [CrossRef]
- Lv, F. Investigation of Physical and Mechanical Properties and Modelling Method for Soybean Grains. Available online: https://kns.cnki.net/KCMS/detail/detail.aspx?filename=1017160847.nh&dbname=CMFDTEMP (accessed on 10 January 2021).
- Xu, T.; Yu, J.; Wang, Y. A modelling and verification approach for soybean seed particles using the discrete element method. Adv. Powder Technol. 2018, 29, 3274–3290. [Google Scholar] [CrossRef]
- Lin, X.S.; Ng, T.T. Contact detection algorithms for 3-dimensional ellipsoids in discrete element modeling. Int. J. Numer. Anal. Methods Geomech. 1995, 19, 653–659. [Google Scholar] [CrossRef]
- Ouadfel, H.; Rothenburg, L. An algorithm for detecting inter-ellipsoid contacts. Comput. Geotech. 1999, 24, 245–263. [Google Scholar] [CrossRef]
- Saini, N.; Kleinstreuer, C. A new collision model for ellipsoidal particles in shear flow. J. Comput. Phys. 2019, 376, 1028–1050. [Google Scholar] [CrossRef]
- Markauskas, D.; Kačianauskas, R.; Džiugys, A.; Navakas, R. Investigation of adequacy of multi-ball approximation of elliptical particles for DEM simulations. Granul. Matter 2010, 12, 107–123. [Google Scholar] [CrossRef]
- Kodama, M.; Bharadwajb, R.; Curtisc, J.; Hancockb, B.; Wassgren, C. Force model considerations for glued-ball discrete element method simulations. Chem. Eng. Sci. 2009, 64, 3466–3475. [Google Scholar] [CrossRef]
- Wang, X. A Multi-ball Based Modelling Method for Maize Grain Assemblies. Adv. Powder Technol. 2017, 28, 584–595. [Google Scholar] [CrossRef]
- Kruggel-Emden, H.; Rickelt, S.; Wirtz, S.; Scherer, V. A study on the validity of the multi-ball discrete element method. Powder Technol. 2008, 188, 153–165. [Google Scholar] [CrossRef]
- Yan, D.; Yu, J.; Wang, Y.; Zhou, L.; Yu, Y. A general modelling method for soybean seeds based on the discrete element method. Powder Technol. 2020, 372, 212–226. [Google Scholar] [CrossRef]
- Boac, J.M.; Ambrose, R.K.; Casada, M.E.; Maghirang, R.G.; Maier, D.E. Applications of Discrete Element Method in Modeling of Grain Postharvest Operations. Food Eng. Rev. 2014, 6, 128–149. [Google Scholar] [CrossRef]
- You, Y.; Zhao, Y. Discrete elementmodelling of ellipsoidal particles using super-ellipsoids and multi-balls: A comparative study. Powder Technol. 2018, 331, 179–191. [Google Scholar] [CrossRef]
- Horabik, J.; Molenda, M. Parameters and contact models for DEM simulations of agricultural granular materials: A review. Biosyst. Eng. 2016, 147, 206–225. [Google Scholar] [CrossRef]
- Coskun, M.B.; Yalçin, Ì.; Özarslan, C. Physical properties of sweet corn seed (Zea mays saccharata Sturt). J. Food Eng. 2006, 74, 523–528. [Google Scholar] [CrossRef]
- USA, American Society of Agricultural Engineers. Compression Test of Food Materials of Convex Shape, ASAE Standards 2002: Standards Engineering Practices; American Society of Agricultural Engineers: St. Joseph, MI, USA, 2002; Volume 49, pp. 592–599. Available online: http://www.doc88.com/p-3347853614932.html (accessed on 10 January 2021).
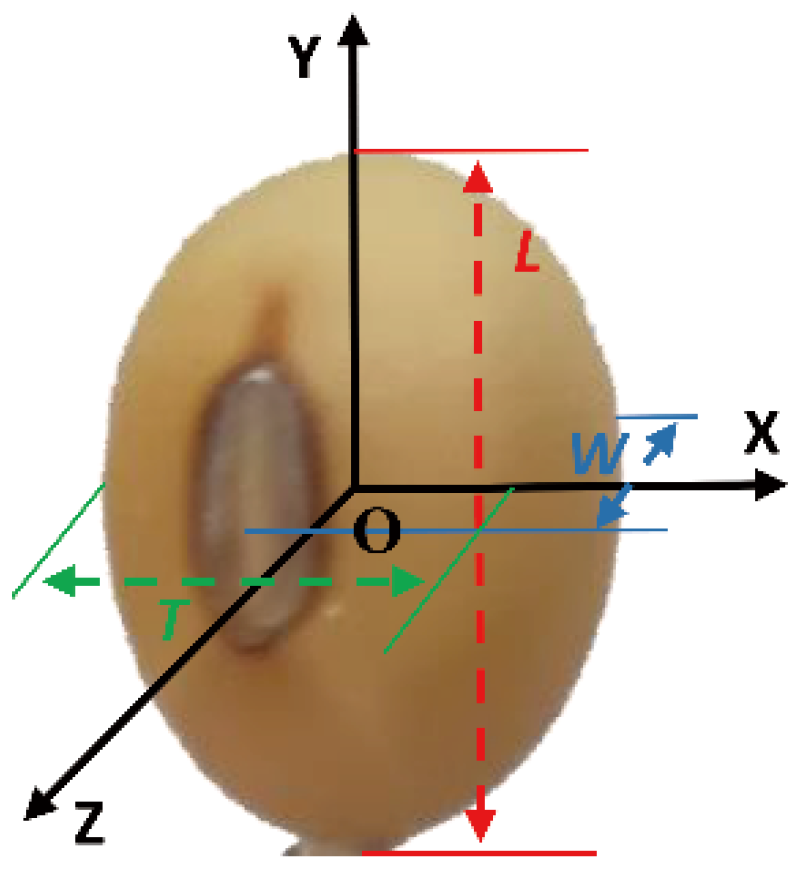
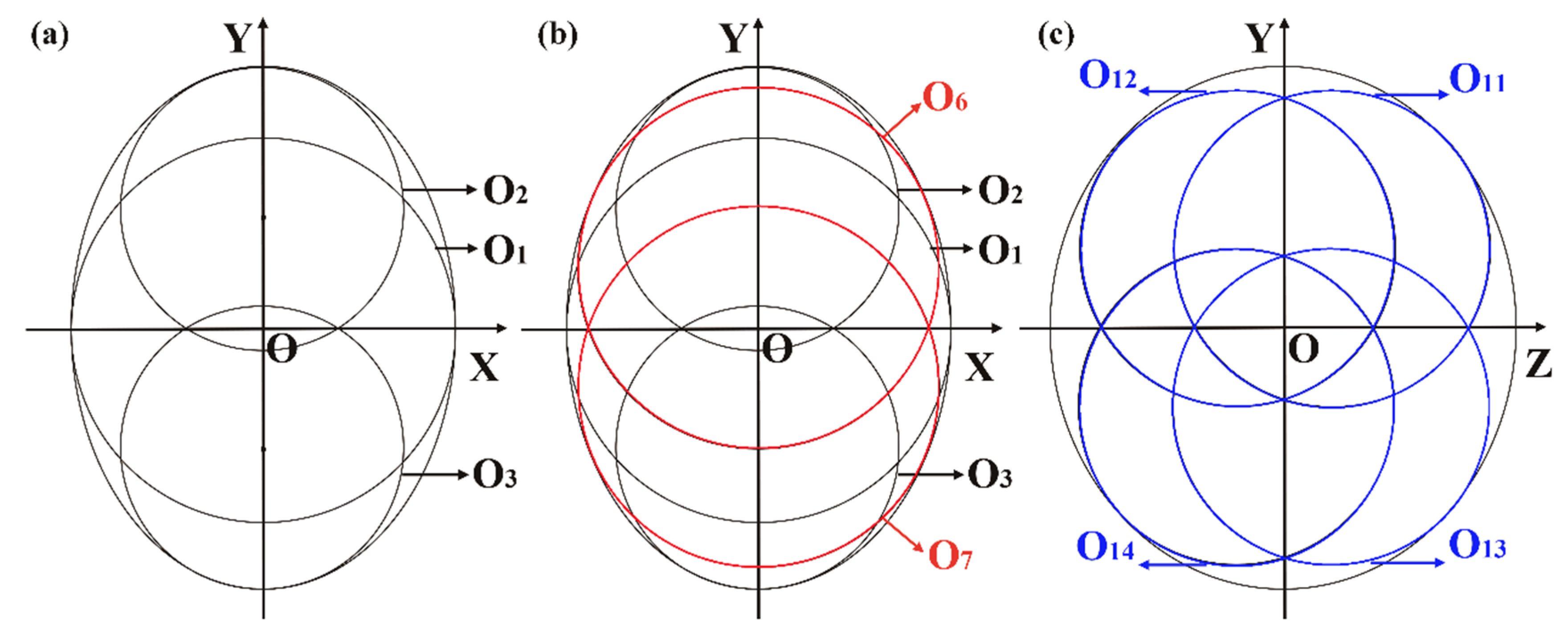










| No. | Centre Coordinate | Radii of the Balls | ||
|---|---|---|---|---|
| X | Y | Z | ||
| 1 | 0 | 0 | 0 | c |
| 2 | 0 | 0 | ||
| 3 | 0 | 0 | ||
| 4 | 0 | 0 | ||
| 5 | 0 | 0 | ||
| 6 | 0 | 0 | ||
| 7 | 0 | 0 | ||
| 8 | 0 | 0 | ||
| 9 | 0 | 0 | ||
| 10 | 0 | |||
| 11 | 0 | |||
| 12 | 0 | |||
| 13 | 0 | |||
| Parameters | Symbol | S-42 | J-17 | Z-39 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Soybean Seed | Organic Glass | Galvanized Steel | Soybean Seed | Organic Glass | Galvanized Steel | Soybean Seed | Organic Glass | Galvanized Steel | ||
| Density, kg/m3 | ρ | 1257 | 1800 | 7850 | 1213 | 1800 | 7850 | 1192 | 1800 | 7850 |
| Poisson ratio | ν | 0.4 | 0.25 | 0.3 | 0.4 | 0.25 | 0.3 | 0.4 | 0.25 | 0.3 |
| Modulus of elasticity, Pa | E | 7.6 × 108 | 1.3 × 108 | 7.9 × 1011 | 6.1 × 108 | 1.3 × 108 | 7.9 × 1011 | 2.6 × 108 | 1.3 × 108 | 7.9 × 1011 |
| Coefficient of static friction | μ | 0.2 | 0.228 | 0.259 | 0.2 | 0.228 | 0.247 | 0.2 | 0.235 | 0.277 |
| Coefficient of rolling friction | μr | 0.02 | 0.05 | 0.02 | 0.03 | 0.04 | 0.05 | 0.03 | 0.04 | 0.04 |
| Coefficient of restitution | 0.627 | 0.542 | 0.647 | 0.562 | 0.642 | 0.715 | 0.607 | 0.705 | 0.726 | |
| Normal stiffness, N/m | 2219 | 759 | 1.1 × 106 | 1655 | 709 | 1.0 × 106 | 751 | 751 | 1.1 × 106 | |
| Tangential stiffness, N/m | 792 | 303 | 4.2 × 105 | 591 | 283 | 4.0 × 105 | 268 | 300 | 4.2 × 105 | |
| Normal damping coefficient, kg/s | 0.185 | 0.123 | 4.02 | 0.185 | 0.08 | 2.66 | 0.105 | 0.071 | 2.84 | |
| Tangential damping coefficient, kg/s | 0.061 | 0.041 | 1.331 | 0.06 | 0.026 | 0.880 | 0.034 | 0.024 | 0.940 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, D.; Yu, J.; Liang, L.; Wang, Y.; Yu, Y.; Zhou, L.; Sun, K.; Liang, P. A Comparative Study on the Modelling of Soybean Particles Based on the Discrete Element Method. Processes 2021, 9, 286. https://doi.org/10.3390/pr9020286
Yan D, Yu J, Liang L, Wang Y, Yu Y, Zhou L, Sun K, Liang P. A Comparative Study on the Modelling of Soybean Particles Based on the Discrete Element Method. Processes. 2021; 9(2):286. https://doi.org/10.3390/pr9020286
Chicago/Turabian StyleYan, Dongxu, Jianqun Yu, Liusuo Liang, Yang Wang, Yajun Yu, Long Zhou, Kai Sun, and Ping Liang. 2021. "A Comparative Study on the Modelling of Soybean Particles Based on the Discrete Element Method" Processes 9, no. 2: 286. https://doi.org/10.3390/pr9020286
APA StyleYan, D., Yu, J., Liang, L., Wang, Y., Yu, Y., Zhou, L., Sun, K., & Liang, P. (2021). A Comparative Study on the Modelling of Soybean Particles Based on the Discrete Element Method. Processes, 9(2), 286. https://doi.org/10.3390/pr9020286





