Impact of Thermal Radiation and Heat Source/Sink on MHD Time-Dependent Thin-Film Flow of Oldroyed-B, Maxwell, and Jeffry Fluids over a Stretching Surface
Abstract
:1. Introduction
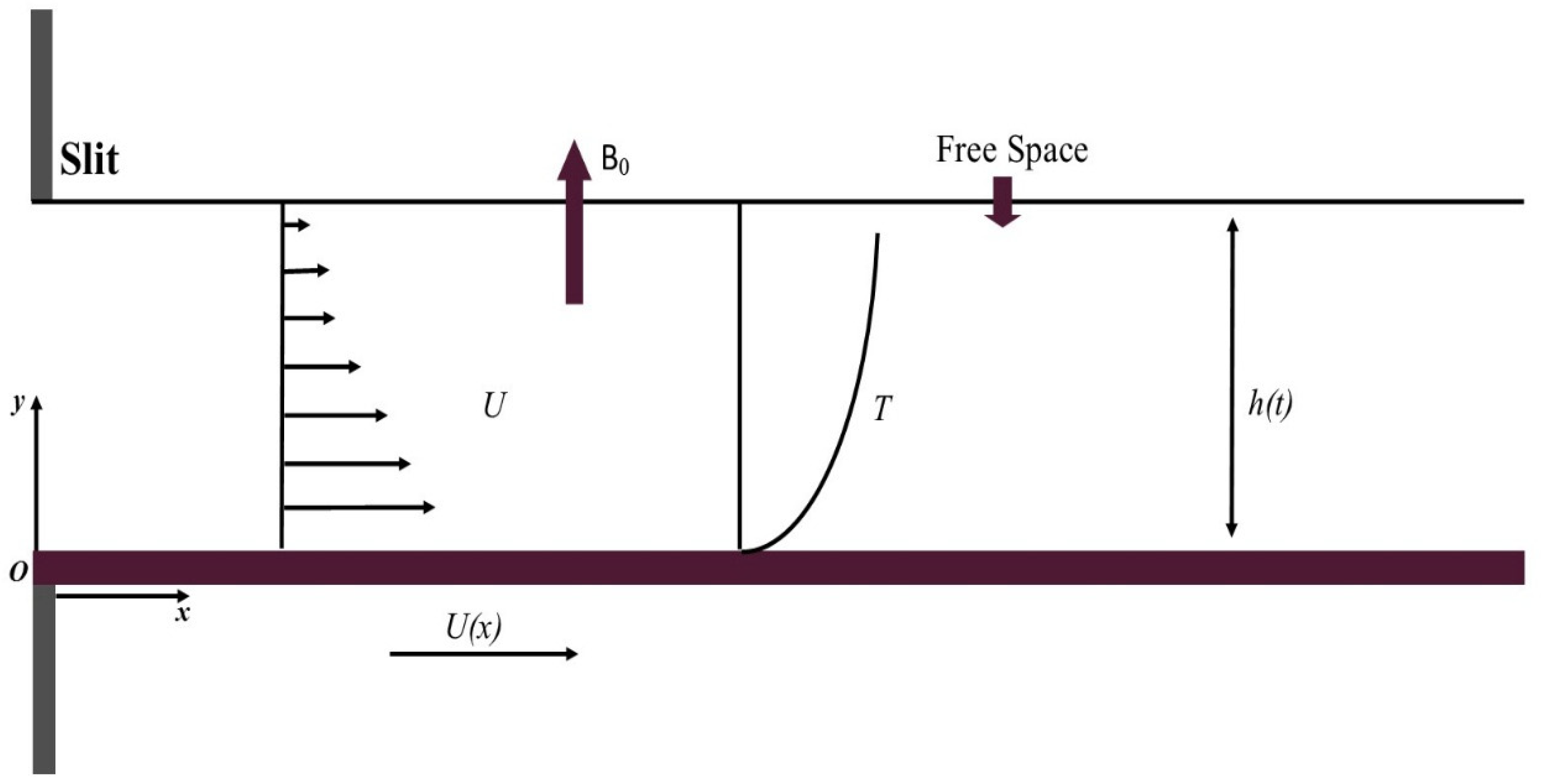
2. Problem Formulation
- The fluid should be Jeffrey fluid when ;
- The fluid should be Maxwell fluid when
- The fluid should be Oldroyed-B fluid when .
3. Solution by HAM
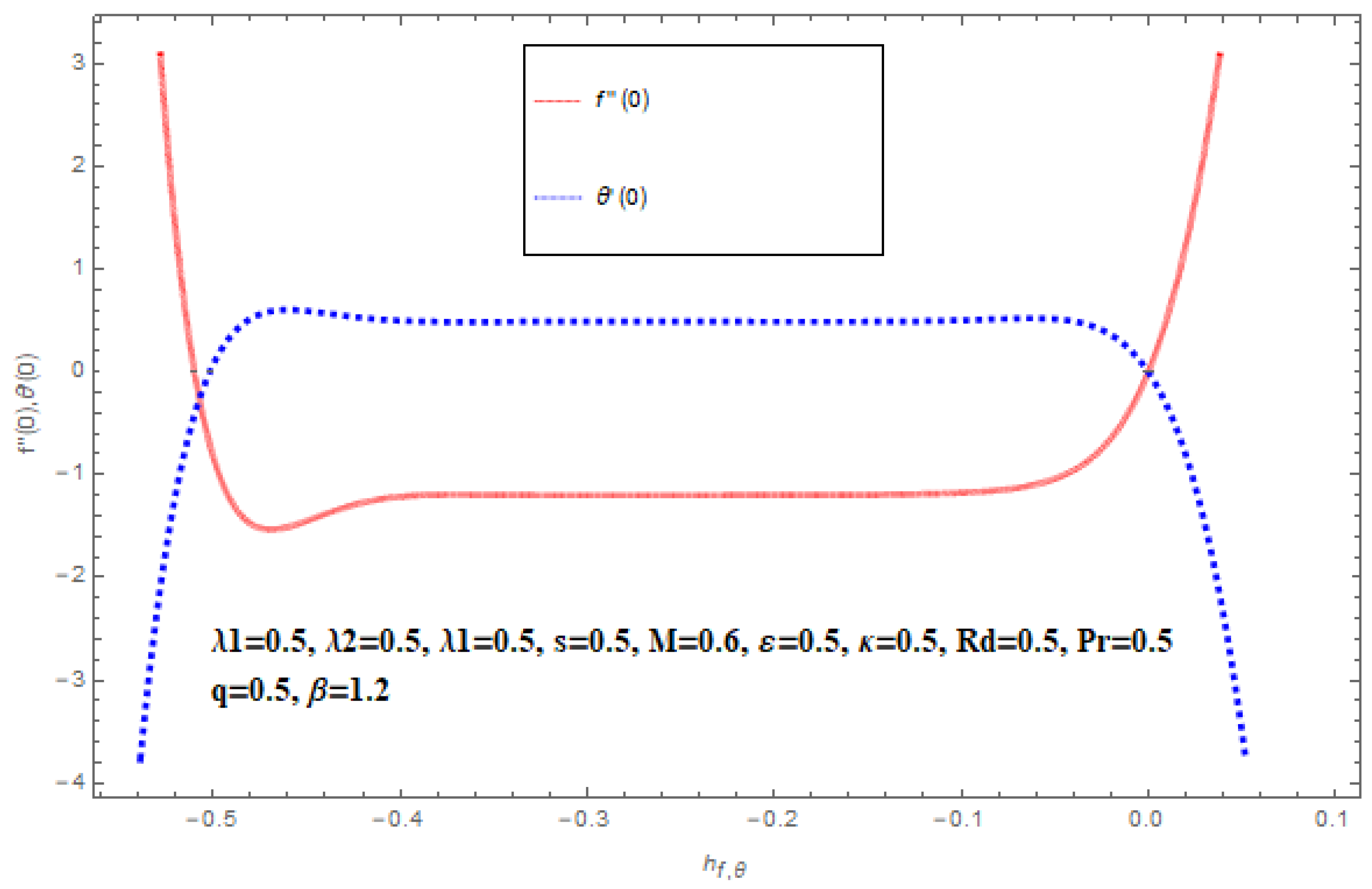
HAM Solution Convergence
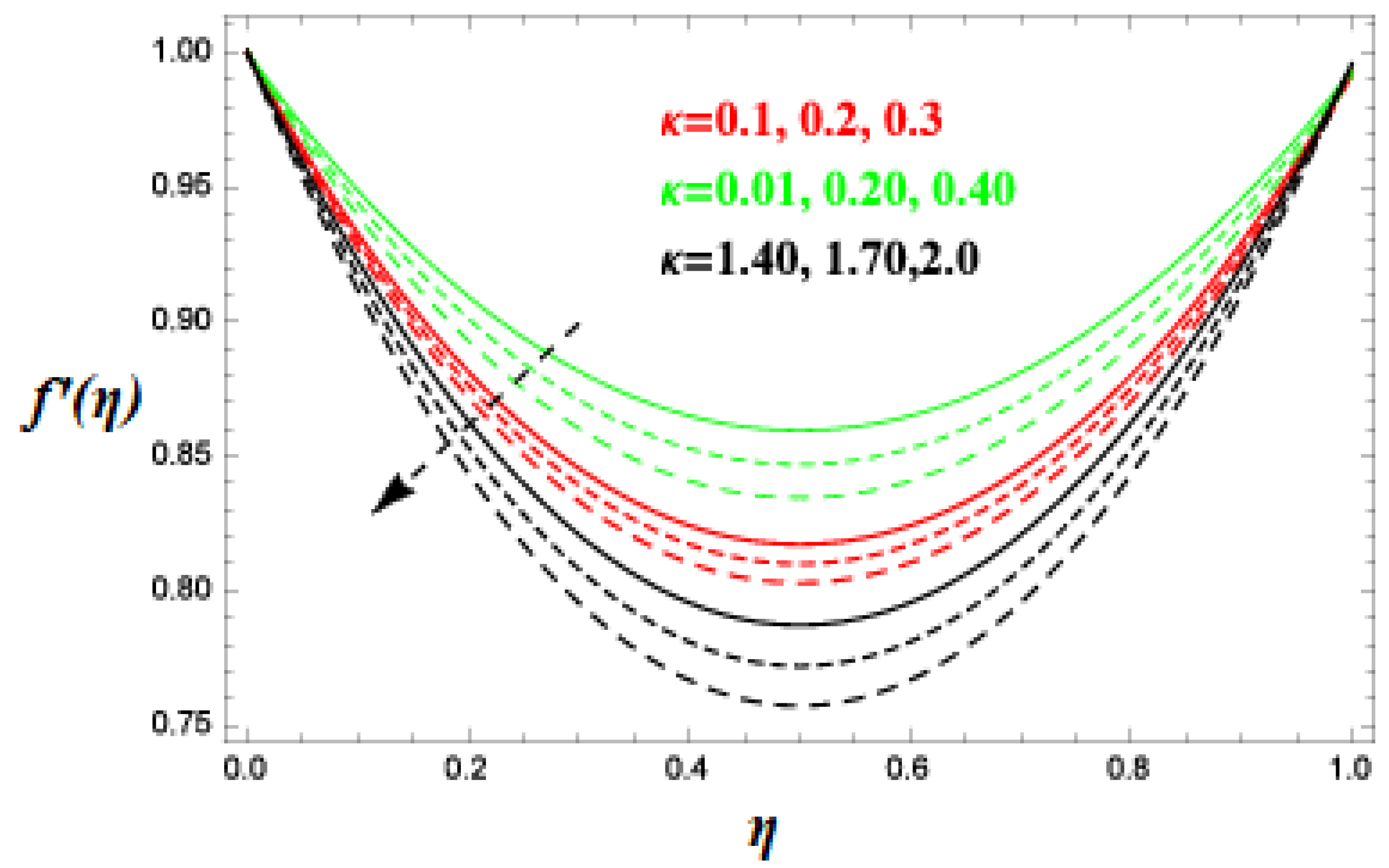
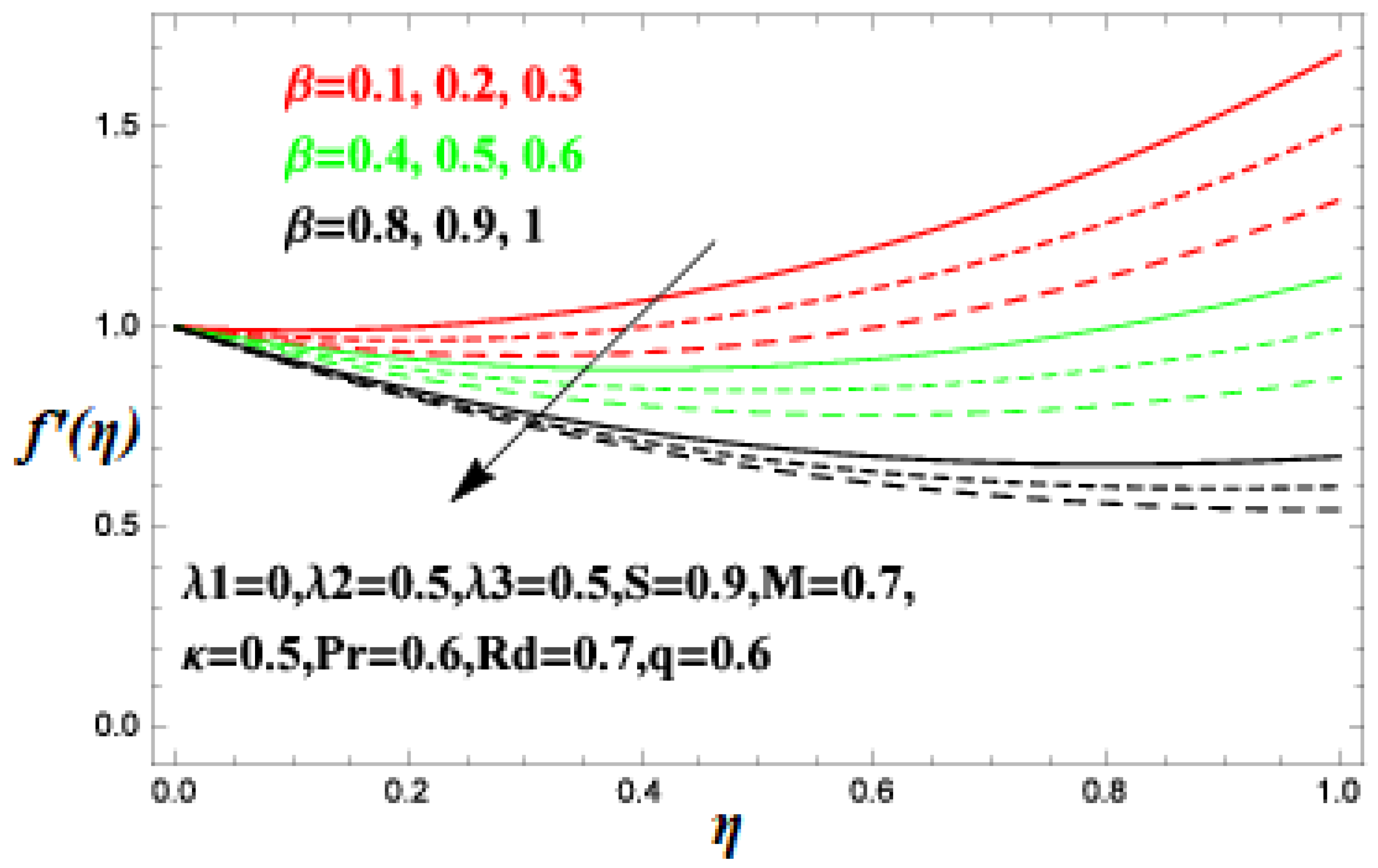
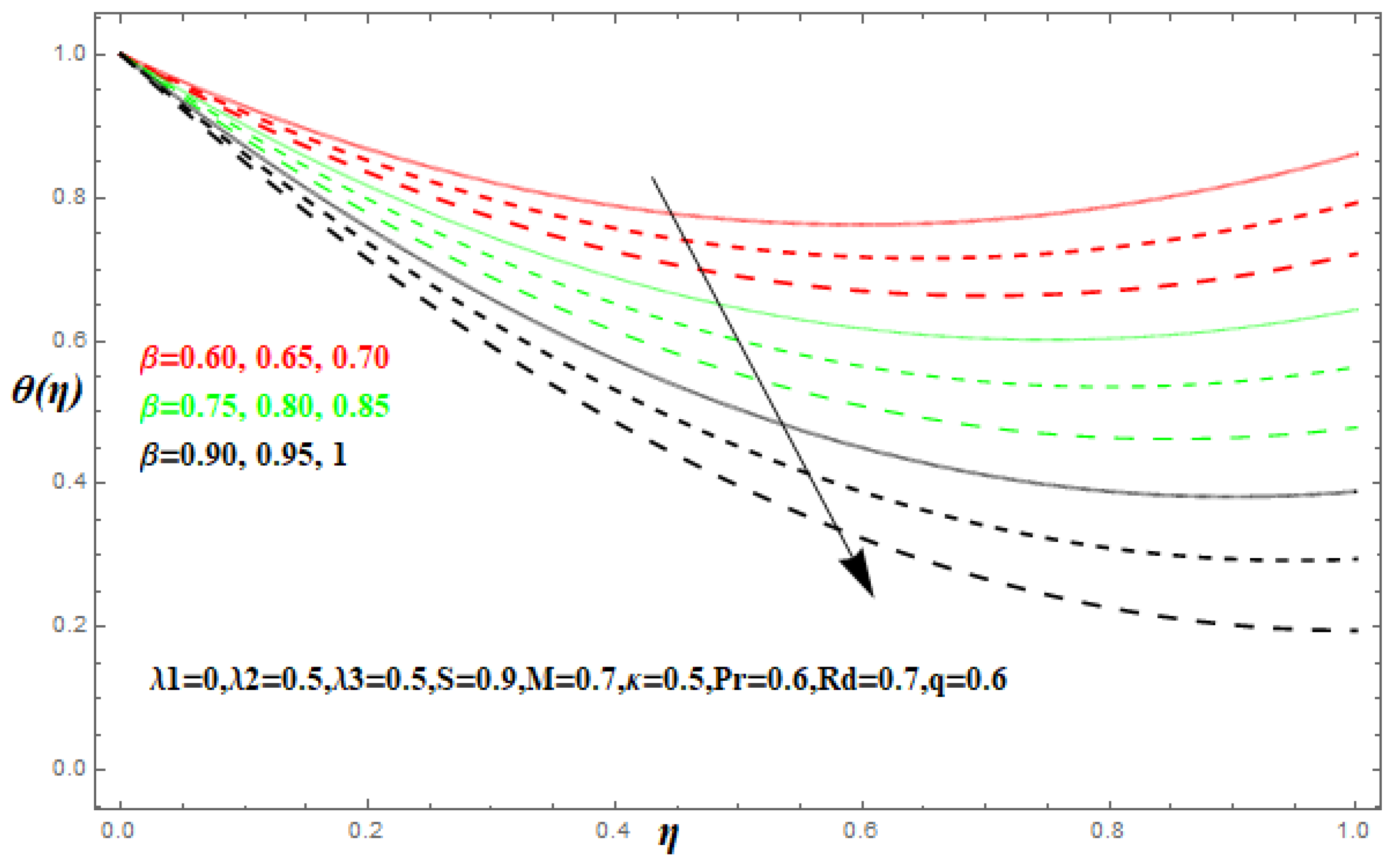
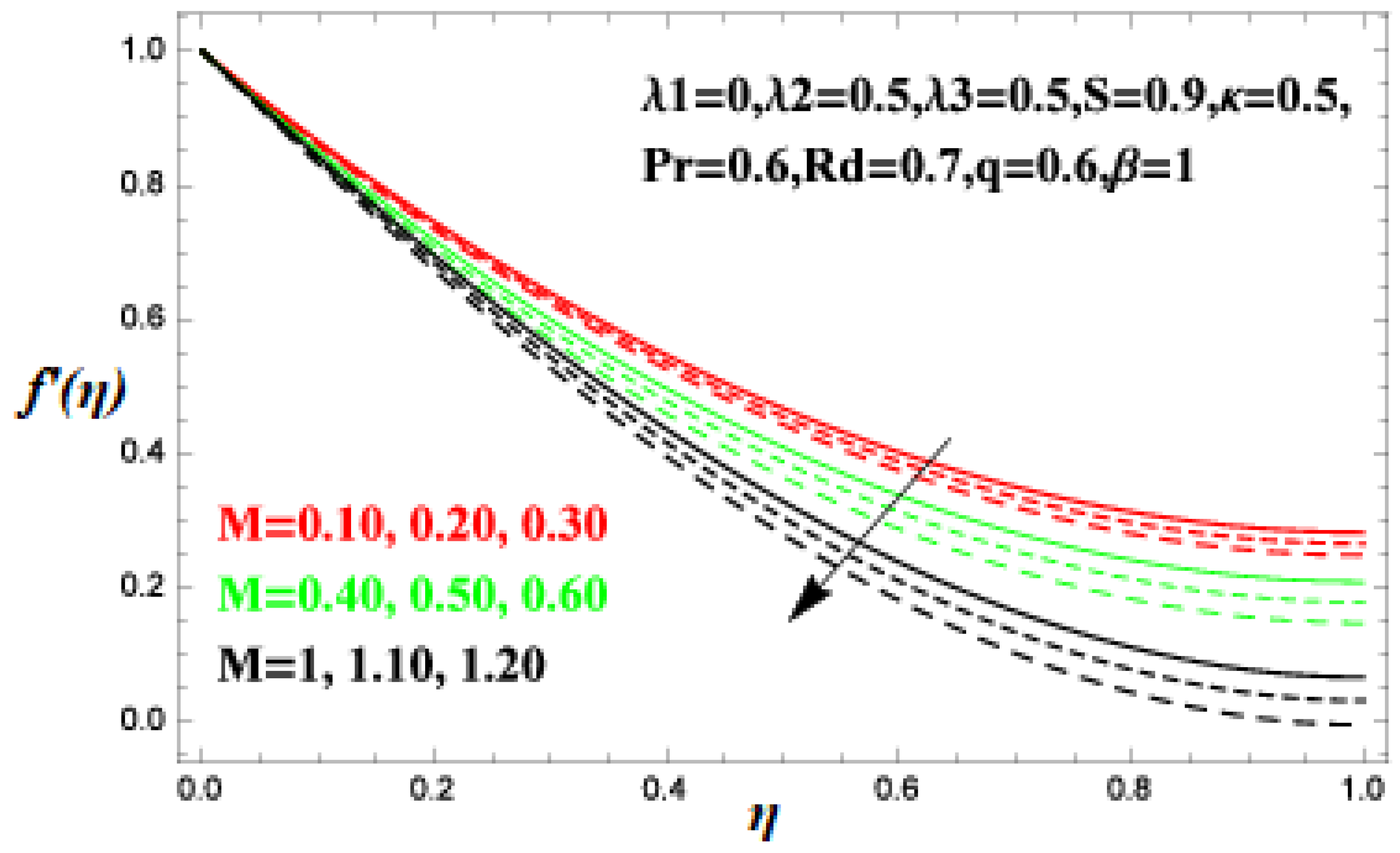
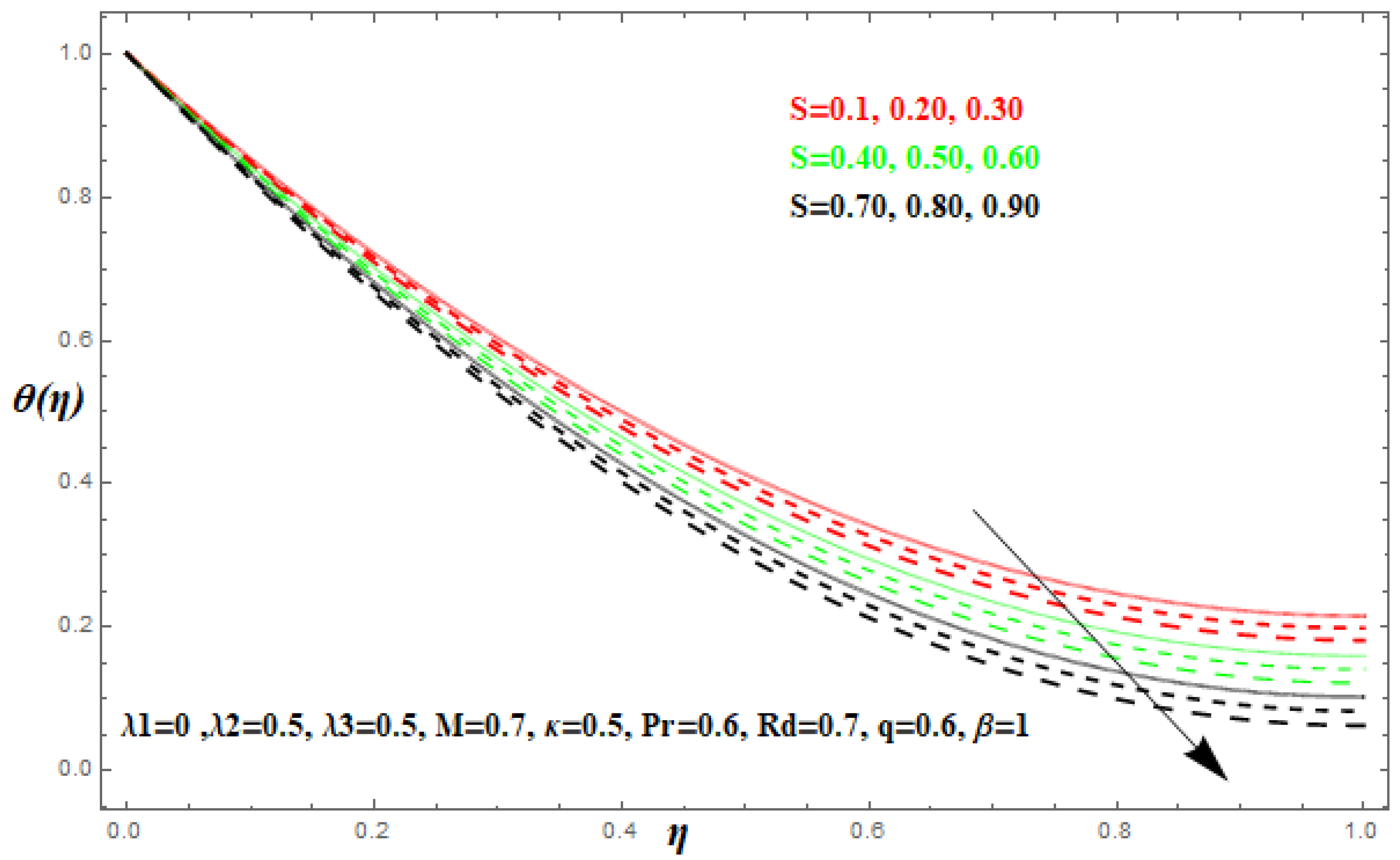
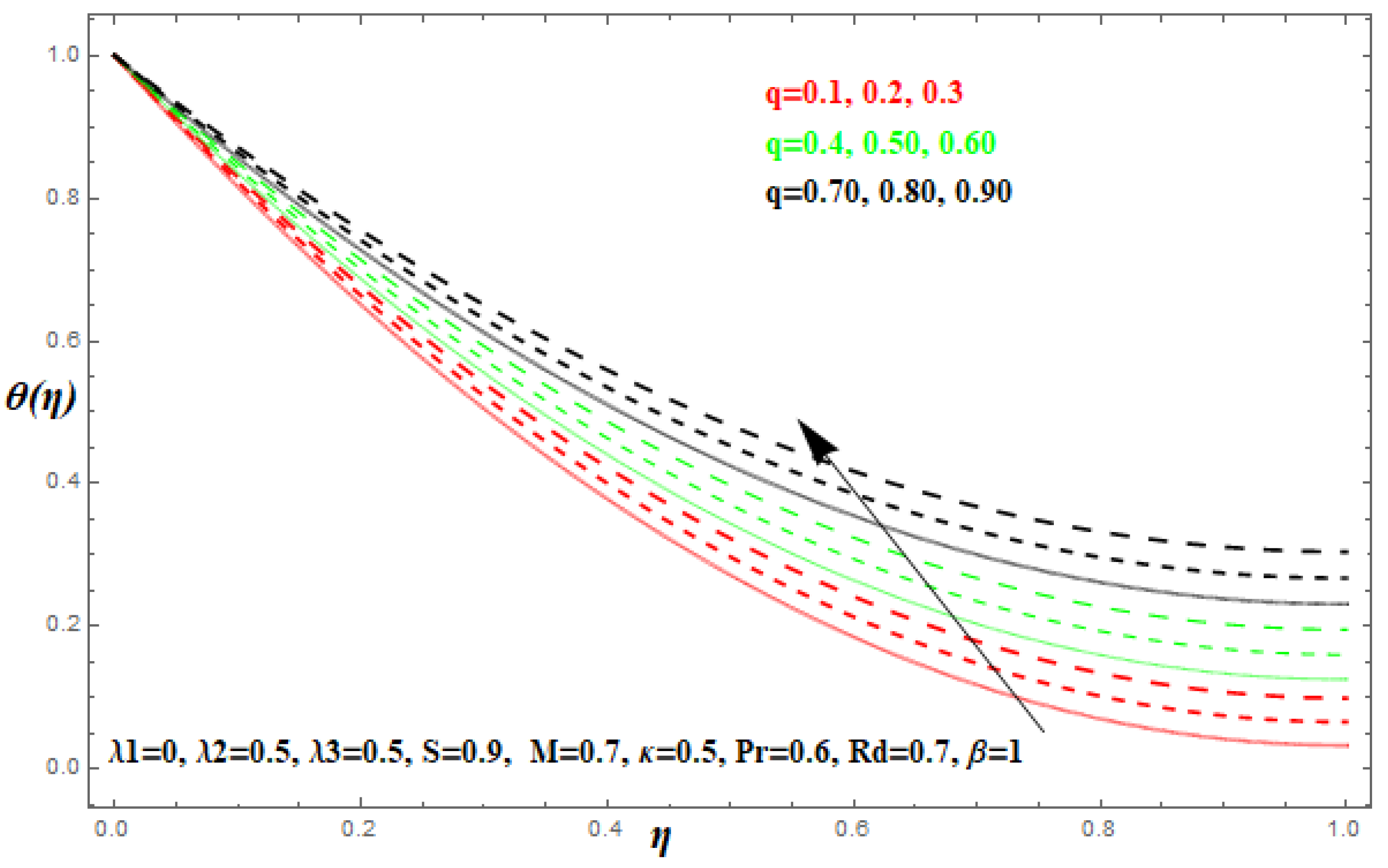
4. Results and Discussion
5. Conclusions
- The velocity profile shows an increase with increasing values of the unsteadiness parameter , while increasing values of the magnetic parameter cause the decline of the velocity profile of the nanofluid film.
- Rd augmented heat flux
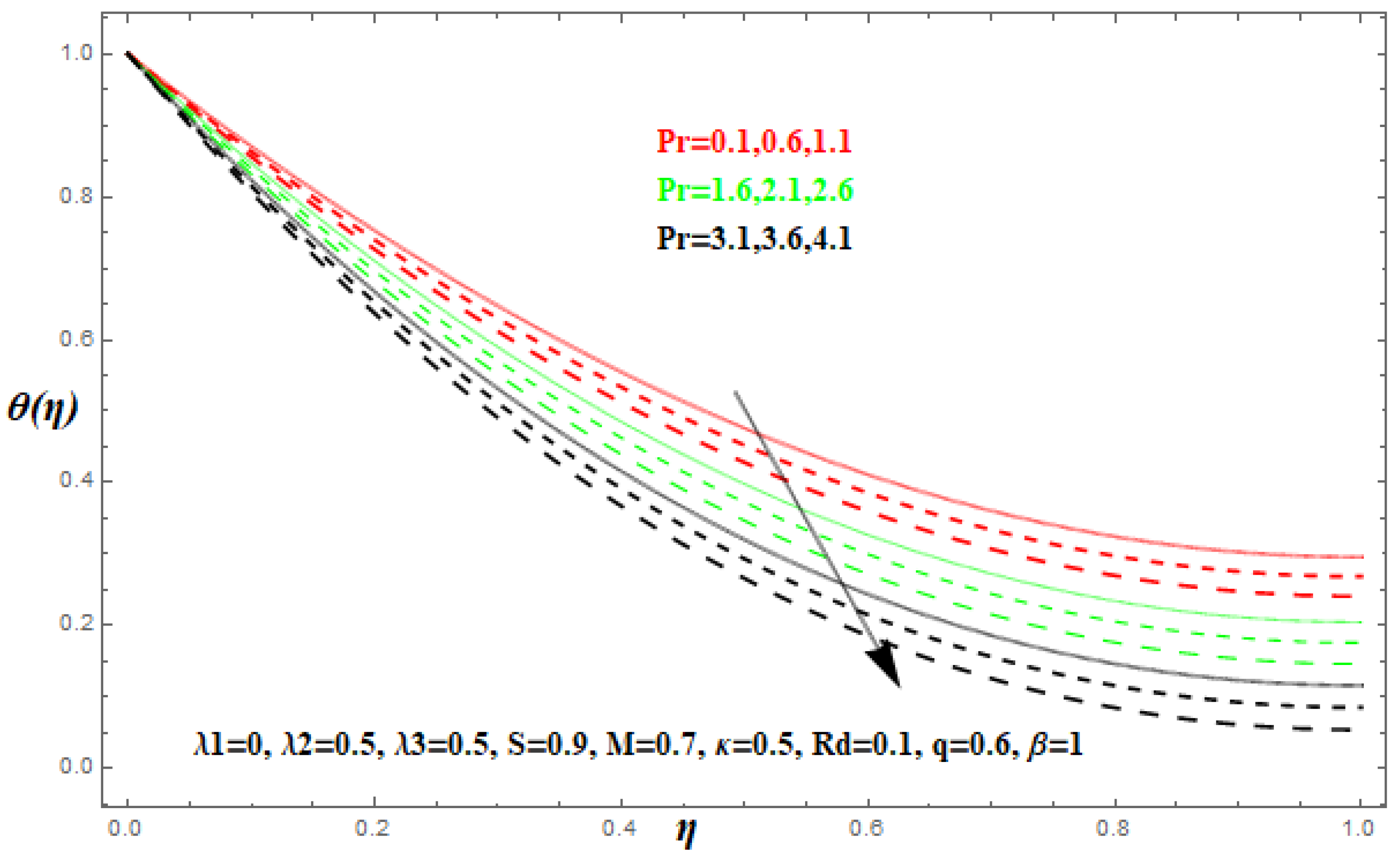
- Higher values of Pr increase the surface temperature.
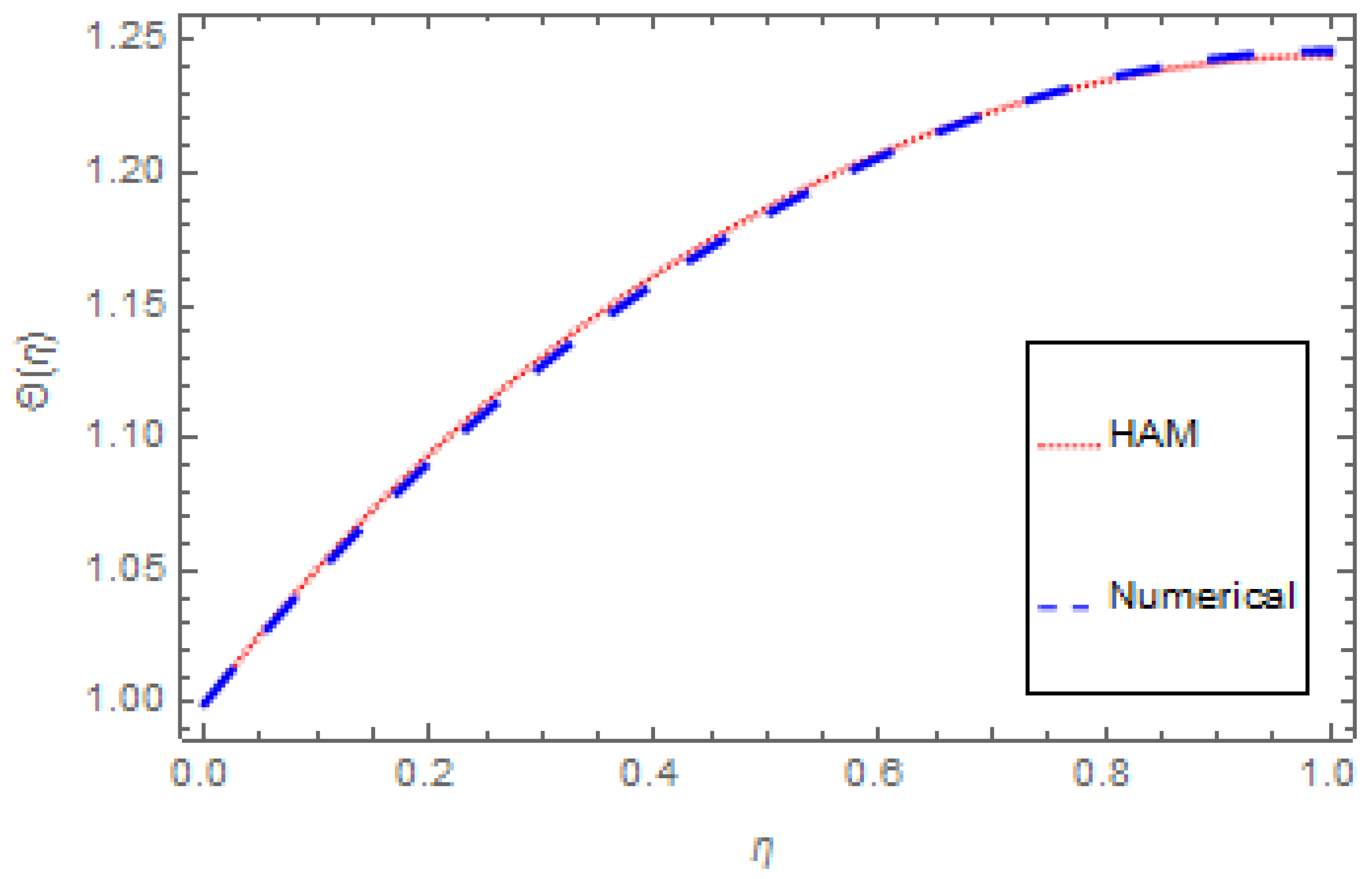
- Fast convergence is shown numerically and graphically.
- The liquid film flow effects on the flow of three fluids (Jeffrey, Maxwell, and Oldroyd-B fluids) are presented using graphs and tables.
- It was found that the coefficient of skin friction rises with the larger rates of the magnetic parameter and the unsteadiness parameter , whereas the coefficient of skin friction decreases with the higher values of the stretching and thickness parameters.
- It was found that liquid film flow is affected by the Lorentz’s force.
- The obtained analytical and numerical consequences are presented graphically and tabulated. An excellent agreement was obtained between HAM and the numerical method.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Current density Coefficient of viscosity Cauchy stress tensor Electric conductivity Electric field Frequency parameter pressure Identity tensor chord Unsteadiness parameter | Material constants Magnetic permeability Magnetic parameter non-Newtonian parameter Pressure gradient parameter and Rivlin–Ericksen tensor Time variable Radiation paramete |
| Prandtl number Skin friction | Nusselt number |
References
- Emslie, A.G.; Bonner, F.T.; Peck, L.G. Flow of a viscous liquid on a rotating disk. J. Appl. Phys. 1958, 5, 858–862. [Google Scholar] [CrossRef]
- Higgins, B.G. Film flow on a rotating disk. Phy. Fluids. 1986, 29. [Google Scholar] [CrossRef]
- Dorfman, I.A. Flow and heat transfer in a film of viscous liquid on a rotating disk. J. Eng. Phys. 1967, 12, 162–166. [Google Scholar] [CrossRef]
- Wang, C.Y.; Watson, L.T.; Alexander, K.A. Spinning of a liquid.lm from an accelerating disc. IMA J. Appl. Math. 1991, 46, 201–210. [Google Scholar] [CrossRef]
- Andersson, H.I.; Holmedal, B.; Dandapat, B.S.; Gupta, A.S. Magnetohydrodynamic melting flow from a horizontal rotating disk. Math. Models Methods Appl. Sci. 1993, 3. [Google Scholar] [CrossRef]
- Dandapat, B.S.; Singh, S.K. Unsteady two-layer film flow on a non-uniform rotating disk in presence of uniform transverse magnetic field. Appl. Math. Comput. 2015, 258, 545–555. [Google Scholar] [CrossRef]
- Sandeep, N.; Malvandi, A. Enhanced heat transfer in liquid thin film flow of non-Newtonian nanofluids embedded with graphene nanoparticles. Adv. Powder Technolog. 2016, 27, 2448–2456. [Google Scholar] [CrossRef]
- Khan, N.; Zuhra, S.; Shah, Z.; Bonyah, E.; Khan, W.; Islam, S. Slip flow of Eyring-Powell nanoliquid film containing graphene nanoparticles. AIP Adv. 2018, 8. [Google Scholar] [CrossRef]
- Ullah, A.; Alzahrani, E.O.; Shah, Z.; Ayaz, M.; Islam, S. Nanofluids Thin Film Flow of Reiner-Philippoff Fluid over an Unstable Stretching Surface with Brownian Motion and Thermophoresis Effects. Coatings 2019, 9, 21. [Google Scholar] [CrossRef]
- Shah, Z.; Bonyah, E.; Islam, S.; Khan, W.; Ishaq, M. Radiative MHD thin film flow of Williamson fluid over an unsteady permeable stretching. Heliyon 2018, 4. [Google Scholar] [CrossRef]
- Ishaq, M.; Ali, G.; Shah, S.I.A.; Shah, Z.; Muhammad, S.; Hussain, S.A. Nanofluid Film Flow of Eyring Powell Fluid with Magneto Hydrodynamic Effect on Unsteady Porous Stretching Sheet. J. Math. 2019, 51, 131–153. [Google Scholar]
- Jawad, M.; Shah, Z.; Islam, S.; Islam, S.; Bonyah, E.; Khan, Z.A. Darcy-Forchheimer flow of MHD nanofluid thin film flow with Joule dissipation and Navier’s partial slip. J. Phys. Commun. 2018, 2. [Google Scholar] [CrossRef]
- Jawad, M.; Shah, Z.; Islam, S.; Majdoubi, J.; Tlili, I.; Khan, W.; Khan, I. Impact of Nonlinear Thermal Radiation and the Viscous Dissipation Effect on the Unsteady Three-Dimensional Rotating Flow of Single-Wall Carbon Nanotubes with Aqueous Suspensions. Symmetry 2019, 11, 207. [Google Scholar] [CrossRef]
- Khan, N.S.; Gul, T.; Islam, S.; Khan, A.; Shah, Z. Brownian Motion and Thermophoresis Effects on MHD Mixed Convective Thin Film Second-Grade Nanofluid Flow with Hall Effect and Heat Transfer Past a Stretching Sheet. J. Nanofluids 2017, 6, 1–18. [Google Scholar] [CrossRef]
- Tahir, F.; Gul, T.; Islam, S.; Shah, Z.; Khan, A.; Khan, W.; Ali, L.; Muradullah. Flow of a Nano-Liquid Film of Maxwell Fluid with Thermal Radiation and Magneto Hydrodynamic Properties on an Unstable Stretching Sheet. J. Nanofluids 2017, 6, 1–10. [Google Scholar] [CrossRef]
- Kartini, A.; Zahir, H.; Anuar, I. Mixed convection Jeffrey fluid flow over an exponentially stretching sheet with magnetohydrodynamic effect. AIP Adv. 2016, 6. [Google Scholar] [CrossRef]
- Hayat, T.; Hussain, T.; Shehzad, S.A.; Alsaedi, A. Flow of Oldroyd-B fluid with nano particles and thermal radiation. Appl. Math. Mech. Engl. Ed. 2015, 36, 69–80. [Google Scholar] [CrossRef]
- Raju, C.S.K.; Sandeep, N.; Gnaneswar, R.M. Effect of nonlinear thermal radiation on 3D Jeffrey fluid flow in the presence of homogeneous–heterogeneous reactions. Int. J. Eng. Res. Afr. 2016, 21, 52–68. [Google Scholar] [CrossRef]
- Hayat, T.; Abbas, Z.; Sajid, M. Series solution for the upper convected Maxwell fluid over a porous stretching plate. Phys. Lett. A 2006, 358, 396–403. [Google Scholar] [CrossRef]
- Sandeep, N.; Sulochana, C. Dual solutions for unsteady mixed convection flow of MHD micropolar fluid over a stretching/ shrinking sheet with non-uniform heat source/sink. Eng. Sci. Technol. Int. J. 2015, 18. [Google Scholar] [CrossRef]
- Nadeem, S.; Akbar, N.S. Influence of haet and mass transfer on a peristaltic motion of a Jeffrey-six constant fluid in an annulus. Heat Mass. Transfer. 2010, 46, 485–493. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Shah, Z.; Shafi, A.; Khan, I.; Itili, I. Uniform magnetic force impact on water based nanofluid thermal behavior in a porous enclosure with ellipse shaped obstacle. Sci. Rep. 2019, 9, 1196. [Google Scholar] [CrossRef] [PubMed]
- Sheikholeslami, M.; Shah, Z.; Tassaddiq, A.; Shafee, A.; Khan, I. Application of Electric Field for Augmentation of Ferrofluid Heat Transfer in an Enclosure Including Double Moving Walls. IEEE Access 2019, 7, 21048–21056. [Google Scholar] [CrossRef]
- Shah, Z.; Dawar, A.; Islam, S.; Khan, I.; Ching, D.L.C. Darcy-Forchheimer Flow of Radiative Carbon Nanotubes with Microstructure and Inertial Characteristics in the Rotating Frame. Case Stud. Thermal Eng. 2018, 12, 823–832. [Google Scholar] [CrossRef]
- Shah, Z.; Islam, S.; Ayaz, H.; Khan, S. Radiative Heat and Mass Transfer Analysis of Micropolar Nanofluid Flow of Casson Fluid between Two Rotating Parallel Plates with Effects of Hall Current. ASME J. Heat Transf. 2019, 141. [Google Scholar] [CrossRef]
- Shah, Z.; Islam, S.; Gul, T.; Bonyah, E.; Altaf Khan, M. The Elcerical MHD And Hall Current Impact On Micropolar Nanofluid Flow Between Rotating Parallel Plates. Res. Phys. 2018, 9. [Google Scholar] [CrossRef]
- Shah, Z.; Bonyah, E.; Islam, S.; Gul, T. Impact of thermal radiation on electrical mhd rotating flow of carbon nanotubes over a stretching sheet. AIP Adv. 2019, 9. [Google Scholar] [CrossRef]
- Shah, Z.; Dawar, A.; Islam, S.; Khan, I.; Ching, D.L.C.; Khan, A.Z. Cattaneo-Christov model for Electrical MagnetiteMicropoler Casson Ferrofluid over a stretching/shrinking sheet using effective thermal conductivity model. Case Stud. Therm. Eng. 2018, 13, 100352. [Google Scholar] [CrossRef]
- Shah, Z.; Tassaddiq, A.; Islam, S.; Alklaibi, A.; Khan, I. Cattaneo–Christov Heat Flux Model for Three-Dimensional Rotating Flow of SWCNT and MWCNT Nanofluid with Darcy–Forchheimer Porous Medium Induced by a Linearly Stretchable Surface. Symmetry 2019, 11, 331. [Google Scholar] [CrossRef]
- Dawar, A.; Shah, Z.; Islam, S.; Idress, M.; Khan, W. Magnetohydrodynamic CNTs Casson Nanofl uid and Radiative heat transfer in a Rotating Channels. J. Phys. Res. Appl. 2018, 1, 17–32. [Google Scholar]
- Khan, A.S.; Nie, Y.; Shah, Z.; Dawar, A.; Khan, W.; Islam, S. Three-Dimensional Nanofluid Flow with Heat and Mass Transfer Analysis over a Linear Stretching Surface with Convective Boundary Conditions. Appl. Sci. 2018, 8, 2244. [Google Scholar] [CrossRef]
- Lee, L.L. Boundary layer over a thin needle. Phys. Fluids. 1967, 10, 822–828. [Google Scholar] [CrossRef]
- Usha, S.; Ramachandra, R.R. Peristaltic transport of two-layered power-law fluids. J. Biomech. Eng. 1997, 119, 483–488. [Google Scholar] [CrossRef]
- Eegunjobi, A.S.; Makinde, O.D. Combined effect of buoyancy force and Navier slip on entropy generation in a vertical porous channel. Entropy 2012, 14, 1028–1044. [Google Scholar] [CrossRef]
- Makinde, O.D.; Chinyoka, T. Numerical investigation of buoyancy effects on hydromagnetic unsteady flow through a porous channel with suction/injection. Mech. Sci. Technol. 2013, 27, 1557–1568. [Google Scholar] [CrossRef]
- Liao, S.J. An explicit totally analytic approximate solution for Blasius viscous flow problems. Int. J. Non-Linear Mech. 1999, 34, 759–778. [Google Scholar] [CrossRef]
- Liao, S.J. On the analytic solution of Magneto hydrodynamic flows of non-Newtonian fluids over a stretching sheet. J. Fluid Mech. 2003, 488, 189–212. [Google Scholar] [CrossRef]
- Nasir, S.; Shah, Z.; Islam, S.; Khan, W.; Khan, S.N. Radiative flow of magneto hydrodynamics single-walled carbon nanotube over a convectively heated stretchable rotating disk with velocity slip effect. Adv. Mech. Eng. 2019, 11, 1–11. [Google Scholar] [CrossRef]
- Nasir, S.S.; Shah, Z.; Islam, S.; Khan, W.; Bonyah, E.; Ayaz, M.; Khan, A. Three dimensional Darcy-Forchheimer radiated flow of single and multiwall carbon nanotubes over a rotating stretchable disk with convective heat generation and absorption. AIP Adv. 2019, 9. [Google Scholar] [CrossRef]
- Khan, A.; Shah, Z.; Islam, S.; Dawar, A.; Bonyah, E.; Ullah, H.; Khan, Z.A. Darcy-Forchheimer flow of MHD CNTs nanofluid radiative thermal behaviour andconvective non uniform heat source/sink in the rotating frame with microstructureand inertial characteristics. AIP Adv. 2018, 8. [Google Scholar] [CrossRef]
- Khan, A.; Shah, Z.; Islam, S.; Khan, S.; Khan, W.; Khan, Z.A. Darcy–Forchheimer flow of micropolar nanofluid between two plates in the rotating frame with non-uniform heat generation/absorption. Adv. Mech. Eng. 2018, 10, 1–16. [Google Scholar] [CrossRef]
- Feroz, N.; Shah, Z.; Islam, S.; Alzahrani, E.O.; Khan, W. Entropy Generation of Carbon Nanotubes Flow in a Rotating Channel with Hall and Ion-Slip Effect Using Effective Thermal Conductivity Model. Entropy 2019, 21, 52. [Google Scholar] [CrossRef]
- Alharbi, S.O.; Dawar, A.; Shah, Z.; Khan, W.; Idrees, M.; Islam, S.; Khan, I. Entropy Generation in MHD Eyring–Powell Fluid Flow over an Unsteady Oscillatory Porous Stretching Surface under the Impact of Thermal Radiation and Heat Source/Sink. Appl. Sci. 2018, 8, 2588. [Google Scholar] [CrossRef]
- Muhammad, S.; Ali, G.; Shah, Z.; Islam, S.; Hussain, S.A. The Rotating Flow of Magneto Hydrodynamic Carbon Nanotubes over a Stretching Sheet with the Impact of Non-Linear Thermal Radiation and Heat Generation/Absorption. Appl. Sci. 2018, 8, 482. [Google Scholar] [CrossRef]
- Saeed, A.; Islam, S.; Dawar, A.; Shah, Z.; Kumam, P.; Khan, W. Influence of Cattaneo–Christov Heat Flux on MHD Jeffrey, Maxwell, and Oldroyd-B Nanofluids with Homogeneous-Heterogeneous Reaction. Symmetry 2019, 11, 439. [Google Scholar] [CrossRef]
- Shang, Y. Analytical Solution For An In-Host Viral Infection Model With Time-Inhomogeneous Rates. Acta Phys. Polon. B 2015, 46, 1567–1577. [Google Scholar] [CrossRef]
- Shang, Y. A lie algebra approach to susceptible-infected-susceptible epidemics. Electr. J. Different. Equat. 2012, 233, 1–7. [Google Scholar]
- Shang, Y. Lie algebraic discussion for aflnity based information diffusion in social networks. Open Phys. 2017, 15, 705–711. [Google Scholar] [CrossRef]




| Solution Approximations | ||
|---|---|---|
| 1 | 0.448400 | 0.122519 |
| 4 | 0.858574 | −0.282846 |
| 7 | 0.912994 | −0.356251 |
| 10 | 0.920043 | −0.369590 |
| 13 | 0.920946 | −0.372026 |
| 16 | 0.921062 | −0.372472 |
| 19 | 0.921077 | −0.372554 |
| 22 | 0.921080 | −0.372569 |
| 25 | 0.921080 | −0.372572 |
| 28 | 0.921080 | −0.372572 |
| HAM Solution | Numerical Solution | Absolute Error | |
|---|---|---|---|
| 0.0 | 1.00000 | 1.00000 | 0.00000 |
| 0.1 | |||
| 0.2 | |||
| 0.3 | |||
| 0.4 | |||
| 0.5 | |||
| 0.6 | |||
| 0.7 | |||
| 0.8 | |||
| 0.9 | |||
| 1.0 |
| HAM Solution | Numerical Solution | Absolute Error | |
|---|---|---|---|
| 0.0 | 1.0000 | 1.00000 | 0.00000 |
| 0.1 | |||
| 0.2 | |||
| 0.3 | |||
| 0.4 | |||
| 0.5 | |||
| 0.6 | |||
| 0.7 | |||
| 0.8 | |||
| 0.9 | |||
| 1.0 |
Jeffrey Fluid | Maxwell Fluid | Oldroyed-B Fluid | ||||
|---|---|---|---|---|---|---|
| 0.5 | 0.5 | 0.6 | 1.0 | −0.705657 | −1.40417 | −1.29373 |
| 0.6 | −0.690279 | −1.34383 | −1.25575 | |||
| 0.7 | −0.676699 | −2.19921 | −1.23210 | |||
| 0.5 | 0.5 | −0.705657 | −1.40417 | −1.29373 | ||
| 0.6 | −0.719191 | −1.43646 | −1.32213 | |||
| 0.7 | −0.732514 | −1.46831 | −1.35012 | |||
| 0.5 | 0.6 | −0.705657 | −1.40417 | −1.29373 | ||
| 0.7 | −0.725666 | −1.44510 | −1.33013 | |||
| 0.8 | −0.745259 | −1.48521 | −1.36548 | |||
| 0.6 | 1.0 | −0.705657 | −1.40417 | −1.29373 | ||
| 1.1 | −0.725279 | −1.469866 | −1.33341 | |||
| 1.2 | −0.740967 | −1.54394 | −0.268147 | |||
| 1.0 | −0.705657 | −1.40417 | −1.29373 |
Jeffrey Fluid | Maxwell Fluid | Oldroyed-B Fluid | ||||||
|---|---|---|---|---|---|---|---|---|
| 0.5 | 0.5 | 0.6 | 1.0 | 0.5 | 0.5 | −0.550388 | −0.590996 | −0.616292 |
| 0.6 | −0.545322 | −0.590885 | −0.611008 | |||||
| 0.7 | −0.539440 | −0.683523 | −0.605811 | |||||
| 0.5 | 0.5 | −0.550388 | −0.590996 | −0.616292 | ||||
| 0.6 | −0.543228 | −0.582409 | −0.608402 | |||||
| 0.7 | −0.536265 | −0.574031 | −0.600680 | |||||
| 0.5 | 0.6 | −0.550388 | −0.590996 | −0.616292 | ||||
| 0.7 | −0.538941 | −0.580121 | −0.605984 | |||||
| 0.8 | −0.527911 | −0.569593 | −0.595950 | |||||
| 0.6 | 1.0 | −0.550388 | −0.590996 | −0.616292 | ||||
| 1.1 | −0.551698 | −0.589585 | −0.619001 | |||||
| 1.2 | −0.547039 | −0.580929 | −0.376664 | |||||
| 1.0 | 0.5 | −0.550388 | −0.590996 | −0.616292 | ||||
| 0.6 | −0.517715 | −0.555700 | −0.579345 | |||||
| 0.7 | −0.488677 | −0.524350 | −0.546542 | |||||
| 0.5 | 0.5 | −0.550388 | −0.590996 | −0.616292 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, A.S.; Nie, Y.; Shah, Z. Impact of Thermal Radiation and Heat Source/Sink on MHD Time-Dependent Thin-Film Flow of Oldroyed-B, Maxwell, and Jeffry Fluids over a Stretching Surface. Processes 2019, 7, 191. https://doi.org/10.3390/pr7040191
Khan AS, Nie Y, Shah Z. Impact of Thermal Radiation and Heat Source/Sink on MHD Time-Dependent Thin-Film Flow of Oldroyed-B, Maxwell, and Jeffry Fluids over a Stretching Surface. Processes. 2019; 7(4):191. https://doi.org/10.3390/pr7040191
Chicago/Turabian StyleKhan, Abdul Samad, Yufeng Nie, and Zahir Shah. 2019. "Impact of Thermal Radiation and Heat Source/Sink on MHD Time-Dependent Thin-Film Flow of Oldroyed-B, Maxwell, and Jeffry Fluids over a Stretching Surface" Processes 7, no. 4: 191. https://doi.org/10.3390/pr7040191
APA StyleKhan, A. S., Nie, Y., & Shah, Z. (2019). Impact of Thermal Radiation and Heat Source/Sink on MHD Time-Dependent Thin-Film Flow of Oldroyed-B, Maxwell, and Jeffry Fluids over a Stretching Surface. Processes, 7(4), 191. https://doi.org/10.3390/pr7040191






