Investment Decisions of Fired Power Plants on Carbon Utilization under the Imperfect Carbon Emission Trading Schemes in China

Abstract

Carbon capture, utilization, and storage (CCUS) is one of the most effective technologies to reduce CO₂ emissions and has attracted wide attention all over the world. This paper proposes a real option model to analyze the investment decisions of a coal-fired power plant on CCUS technologies under imperfect carbon emission trading schemes in China. Considering multiple uncertainties, which include carbon trading price volatility, carbon utilization revenue fluctuation, and changes in carbon transport and storage cost, the least squares Monte Carlo simulation method is used to solve the problems of path dependence. The research results show that the independent effects of carbon trading mechanisms on investment stimulation and emission reduction are limited. The utilization ratio of captured CO₂ has significant impacts on the net present value and investment value of the CCUS project. Moreover, the investment threshold is highly sensitive to the utilization proportion of food grade CO₂ with high purity. It is suggested that the Chinese government should take diverse measures simultaneously, including increasing grants for research and development of carbon utilization technologies, introducing policies to motivate investments in CCUS projects, and also improving the carbon emission trading scheme, to ensure the achievement of the carbon emission reduction target in China.

1. Introduction

Climate change is one of the most serious challenges faced by mankind, and has drawn high attention from most countries in the world. Administrators from different countries are all searching for efficient technologies to reduce carbon dioxide emissions, which are major contributors to climate change and global warming [1]. The Paris Agreement, signed by nearly 200 parties around the world in December 2015, aims to control the global temperature rise within 2 °C in this century [2]. To achieve this challenging goal, carbon capture, utilization, and storage (CCUS) has gained wide attention in recent years as a potential technological option for large-scale CO₂ emission reduction in the future. Some researchers find that CCUS technology contributes to the achievement of near-zero emissions of CO₂ in a fossil fuel-fired electricity generation department [3,4,5]. According to the latest report on the state of global energy and CO₂ by the International Energy Agency (IEA) in March 2019, the global energy demand continued to grow in 2018 and global CO₂ emissions hit a new record, having risen by 1.7%. The pace of development of efficient renewable energy has failed to keep up with energy demand, which has led to the increase of coal usage again from the rebound in 2017 [6]. In China, carbon dioxide emissions increased by 2.3% in 2018, which mainly come from fossil fuel combustion and account for 28.6% of total global CO₂ emissions [7]. Coal contributes about 60% of China’s total energy consumption, and thermal power accounts for about 75% of total power generation [8,9]. It is clear that the coal-based energy consumption structure is difficult to change in the short term in China [10,11]. As the 23rd country to ratify the Paris agreement, China pledged that carbon emissions will peak around 2030, and the proportion of non-fossil energy consumption will be increased to 20% in the meantime. To fulfill these challenging tasks, CCUS is a vital means to reduce carbon dioxide emissions in China, and it is also a new cleaner production technology for coal-fired power plants. The realization of large-scale low-carbon utilization of fossil energy will lead us to sustainable development in the future [12,13,14]. However, for coal-fired power plants to develop CCUS technologies, there are some serious problems that need to be solved, such as huge initial investment, high operational cost, technological breakthrough, and construction of an external market environment [15,16]. Therefore, ways to accelerate the wide application and large-scale deployment of CCUS project in China need to be identified urgently.

According to practice in other countries, the carbon emission trading scheme seems to have played an important role in promoting the deployment of Carbon Capture and Storage (CCS) or CCUS projects. In 2013, the carbon exchange in Shenzhen was the first trial for trading carbon emission rights in China, and then another six exchanges were launched successively. The construction of a national carbon market started at the end of 2017, and simulation runs were planned for 2019. Considered as a breakthrough, the national carbon trading market started entirely from the power generation industry. All over China, at least 1700 thermal power enterprises, whose total carbon emissions exceed 3 billion tons every year and account for one third of the gross emissions in the whole country, will be involved in the trading system. However, the improvement and construction of the trading market moved slowly in 2018, and emission reduction was less significant than expected. Meanwhile, some problems were revealed gradually, such as the poor fluidity and weak efficiency in the carbon trading market. It will take a long time before the market mechanism becomes adequately effective. Due to the lack of a perfect carbon trading system, other solutions are urgently required to stimulate investments from fired power plants on CCUS projects.

Compared with CCS technologies, more attention has been given to carbon utilization in CCUS projects. Carbon utilization means the carbon dioxide captured by coal-fired power plant are purified and further processed, and the highly purified carbon dioxide is put into other manufacturing processes as an essential productive factor. In the CCUS system, the treatment method for captured CO₂ is cyclic utilization, rather than simply being buried under the ground or somewhere else. Nowadays, approaches to CO₂ utilization can be divided into two categories: Enhanced oil recovery (EOR) and non-EOR methods. EOR has been the most widely applied approach in utilizing carbon dioxide captured by the CCUS system. However, although CO₂-EOR has been developed in the USA for nearly 40 years, it still contains inherent drawbacks, which have not been solved well until now. For instance, due to the high costs of carbon dioxide capture and transportation in fired power plants, oil field companies consequently bear the burden of high CO₂-EOR costs. In recent years, increasingly more oil production enterprises have converted to chemical flooding techniques, the costs of which have been lower than that of CO₂-EOR at present and will fall continuously with rapid speed in the future. Furthermore, the risk of causing geological disasters due to underground CO₂ storage always exists. Hence, it is more important for coal-fired plants to figure out efficient methods of non-EOR utilization of captured CO₂.

At the moment, non-EOR utilization of CO₂ contains two kinds of forms: Industrial utilization and food utilization [17,18,19]. Industrial utilization includes the synthesis of urea from CO₂, production of light nano-scale ultrafine active carbonate, and the obtainment of methanol by catalytic hydrogenation of CO₂, inorganic and organic chemicals, polymer materials, biological fertilizer, and so on. Food utilization involves the usage of liquid or solid CO₂ in refrigeration, food storage, and transportation in CO₂; and food additives used in beer or drinks [20,21,22]. Although industrial utilization has a wider application range than food utilization, the process requirements and quality standards of food-grade CO₂ are obviously higher than those of industrial CO₂, and then the market price of food-grade CO₂ is consequently usually several times greater than industrial CO₂ prices. Regardless of whether food usages or industrial applications are used, non-EOR utilization has more advantages than EOR utilization due to non-EOR utilization earning earn more revenue from the market and having lower transport and storage costs of captured CO₂ for fired power plants. Unlike the nascent market of carbon emissions, the market of physical carbon dioxide trading has been well established in China. Therefore, the key link of carbon utilization should be technical breakthroughs in CO₂ capture, purification, and concentration rather than market development.

However, to the best of our knowledge, there is a lack of research on investment in coal power plants on carbon utilization technology. Much attention is paid to the construction of the national carbon emission trading market, but very few former studies are concerned with to what extent the market mechanism would influence a reduction of carbon emissions in China. Furthermore, none of them investigate the critical factors that could indeed activate enthusiasm for investment in fired power plants of CCUS projects when the market mechanism appears to malfunction. Therefore, in this paper, an investment decision model based on real option theories was built to analyze the effects of the carbon trading market and low-carbon technologies on CCUS project value. This study aimed to reveal the determinants that motivate investment in coal power plants, and how these factors interact with each other and influence the firepower generation enterprise’s investment decisions eventually. The results provide some suggestions for government departments and investors around the world to promote the industrialization of CCUS technologies.

The rest of the paper is organized as follows: Section 2 provides a literature review of investment decisions on CCUS technologies; Section 3 describes the model, and variable assumptions and model solutions are provided; Section 4 estimates the related parameters and Section 5 presents the numerical simulation and sensitivity analysis; and conclusions and policy implications are given in the final section.

2. Literature Review

CCUS is the upgrading technology scheme of CCS. Compared with CCS, CCUS recycles captured carbon dioxide to produce higher economic benefit, which is more operational in reality. Most of the former literature focuses on the investment decision of CCS technologies, and this literature can be divided into two main streams.

First, the investment values of CCS projects under various uncertainties, including the uncertain factors in the carbon trading market, have been discussed systematically. With the uncertainty in the price of carbon emission and electricity, Abadie and Chamorro [23] built a quadtree model of supercritical investment made by coal-fired power plants in CCS and calculated the option value of the project, and then gave the critical value of the carbon emission right to decide whether power plants should invest or not. Wu [24] constructed an investment decision-making model of two investment phases using the Black-Scholes model under multiple uncertainties, and the results showed that the updated options increased the enterprise value and reduced the investment threshold of power generation. Zhang et al. [25] adopted the trinary-tree real option method to study two typical investment decision-making problems of the renovation of a coal-fired power plant under several uncertain conditions, and found that it was unwise to start CCS renovation and investment right away under the current market. By incorporating uncertainties in the carbon trading price, fuel price, government subsidy, and investment cost into the model, Wang and Du [26] analyzed CCS investment decisions of coal-fired plants by the real option method.

Second, the incentive effects of various policies to stimulate CCS technology advancement have been analyzed from different point of views. Considering many uncertain factors, Zhu and Fan [27] adopted sequential game theories to assess the value of CCS investment projects, and showed that a government subsidy is helpful for the research and development of CCS technology. Chen, Wang, and Ye [28] studied the significance of a power generation subsidy in the investment decisions of CCS under the dual influence of the carbon trading market and subsidy policies, and found that the effects of a government subsidy on CCS investment and carbon reduction depends on market conditions. Wang and Zhang [29] pointed out that carbon tax is an important policy instrument to control greenhouse gases, and explored the decision behavior of a potential investor to design a suitable carbon tax rate from a microeconomic perspective. The results showed that the optimal carbon tax rate is sensitive to uncertainties, which includes carbon price volatility and initial carbon price.

From the above review, it could be concluded that most research has considered multiple uncertainties in CCS technology projects, including fluctuations in market prices, risks in technology development, and different subsidy levels, and generally adopted real option methods to solve the investment decision problems. All of the theories and methods applied in studies about CCS can provide useful references for relative research in the context of CCUS projects.

With the advancements of several large-scale demonstrative projects in China, the low-carbon emission effect of CCUS technologies is attracting increasingly more attention from researchers. Some academics claim that carbon utilization in CCUS systems would increase project profits, encourage relative technology investment, reduce the CO₂ emissions of power plants, and promote energy structure transition. Based on a technology roadmap for the development of CCUS technology, Zhang et al. [30] identified the critical technologies in each part of the CCUS chain and presented technical priorities in different stages from each technical aspect. The economic and social benefits of carbon utilization are explored in several papers. Pershad et al. [31] revealed in their report that for several fields, CO₂-EOR projects yield a positive net present value at current oil prices, and financial modelling suggested that the highest rates of EOR deployment in the UK Continental Shelf would bring 2.7 billion pounds in gross value added to the Scottish economy. Hasan et al. [32] indicated that CO₂ can be captured and utilized by improved CCUS supply chain networks, and a profit of 555 million dollars can be acquired annually.

Although many previous studies have verified the importance of government subsidies for the development of CCS technologies, things seem to be different due to the involvement of carbon utilization. Several studies in recent years pointed out that not all financial support has a significant positive effect on triggering investment in CCUS technologies. Fan et al. [33] constructed three subsidy ways and two different settings in a model to study the investment decisions of coal-fired power plants. Their results revealed that the three subsidy modes play a significant role in stimulating emission reduction investment and the 45Q subsidy mode effectively promotes the power plant as a reduction option for the long term. On the contrary, the operation and maintenance subsidy mode is only appropriate for a reduction incentive for shorter periods. In the same vein, Yang et al. [34] also pointed out that even the full investment subsidy cannot boost investment immediately and the subsidy of carbon dioxide utilization exerts an increasingly crucial role in promoting CCUS investment compared with the electricity tariff subsidy in the model analysis. In comparison with subsidies for initial investment and operation and maintenance cost of the CCUS system, the measures to promote the research and development of utilization techniques are probably more important for advancing the industrialization of CCUS technologies. In addition, quite a few studies keep a more watchful eye on EOR utilization of CO₂. As known to people, carbon utilization in EOR has distinct disadvantages, such as large investment, long construction period, and potential geology risks. In the context of the immature market transaction mechanism, it is essential to investigate the effect of other non-EOR carbon utilization approaches on CCUS project investment, and then reveal the influence of utilization technologies on carbon emission reduction in China.

Generally, the investment decision is typically supported by cost–benefit analysis. The most commonly used method is discounted cash flow (DFC), which depreciates the cash flows of the project in the future to the present by a given discount rate. However, the DCF method cannot evaluate the investment project accurately enough because of its defects under an uncertain environment [24]. The rising real option approach is able to capture the potential value of managerial flexibility in response to the emergence of new information. According to technical solutions used under different settings in the presence of uncertainty, relative studies focus on two main categories: The binomial model and the Monte Carlo simulation method. When only a few uncertain factors, which follow geometric Brownian motion (GBM), are involved in the investigated issue, the binomial model is the most suitable approach to use to analyze the influence of risky factors on htee CCUS project value, and the analysis results can give the optimal investment time simultaneously [35]. In some studies, a trinomial tree model is adopted to improve the analysis accuracy. As, in most cases, the process of the CCUS project involve various uncertainties, including several kinds of market risk and technical risk in different stages, the Monte Carlo simulation method becomes the preferable choice to carry out numerical analysis with multiple uncertainties [36]. In this paper, four variables with uncertainty were considered in the model, and the least square Monto Carlo method was selected to solve the early exercise problem in American options.

Consequently, the existing literature relevant to CCUS deployment is still under-researched. First, the carbon trading market is one of the most critical mechanisms in other countries while the carbon trading scheme in China is still under construction. The current carbon price in the foreseeable future is quite possibly too low to trigger investments in coal power plants. Moreover, the roles of carbon capture and carbon storage have been widely regarded as the key measures to reduce carbon emissions, but the participation of carbon utilization will undoubtedly affect the operational mechanism and industrialization of CCUS technologies. Taking the coal-fired power plant in China as an example, this paper builds a real option model to investigate the influence of carbon utilization on CCUS project value under imperfect carbon market mechanisms, and the results will be of great help to fill the research gaps.

3. Methodology

Under multiple uncertainties in the market, economic environment, and policies, investment in CCUS technology projects by coal-fired power plants is irreversible and highly risky, and the investment decisions under uncertainty have general characteristics displayed in real option models. Based on real option theories, the investment model for a coal plant’s CCUS project was constructed, and the investor with the option to delay in a coal power plant can choose to invest immediately or delay by the option value.

Assuming the remaining lifetime of the coal power plant is T years from time 0, the power plant can invest in the CCUS project as it wishes in any year, $t_0$($0\le t_0\le T$). Supposing the time to construct the CCUS project is not considered, the project can be run immediately when the investment occurs, and it will operate normally until the end of the lifecycle. The timeline of the CCUS project is shown in Figure 1.

There are four technological processes during the procedure of CCUS technology, i.e., carbon capture, utilization, transport, and storage [4]. The investment value of the CCUS project is affected by several uncertain factors, which are considered in the model with four uncertain elements, namely carbon price, CO₂ utilization price, CO₂ transport cost, and storage cost, and vary with the market and policy environment.

3.1. Assumptions

3.1.1. Subsidy for Electricity

According to the policies of the National Development and Reform Commission and the Ministry Environmental Protection, coal power plants, which improve air quality by reducing emissions of CO₂, oxynitride, and smoke powder in the process of electricity generation, can receive electricity sale subsidies from the government. Assuming that the subsidy per unit electricity, $p_E$, as a constant remains unchanged during the operating period of the CCUS project, and the electric energy production of the coal plant every year is fixed, then the income from the electricity subsidy is expressed as $p_E{q}_E$.

3.1.2. Profits from Carbon Emission Trading

Under the scheme of carbon emission trading, the spare carbon emission allowance due to CO₂ capture is considered a commodity and can be traded in the market. Therefore, the coal power plant with CCUS facilities can obtain gains from selling its carbon emission allowance. Many studies assume that the carbon prices in the Chinese market follow the geometric Brownian motion (GBM). The carbon price in this paper was also characterized as follows:

$$d{p}_C={\mu }_C{p}_C{d}_t+{\sigma }_C{p}_{C}d{z}_C,$$

(1)

where $p_C$ is the price of the carbon emission trade at time t; ${\mu }_c$ represents the drift rate of the carbon price; ${\sigma }_c$ denotes the volatility rate of the carbon price; and $d{z}_c$ is the increment to a standard Weiner process. To simplify the analysis, the electricity production of the coal plant, $q_E$, is assumed to be fixed, and then the reduction of annual CO₂ emissions, $q_C$, for this plant is also fixed, thus the carbon trade profit for each year is $p_C{q}_C$.

3.1.3. CO₂ Utilization Profits

Coal power accounts for 70% of China’s whole electrical energy structure. At present, CCUS projects for coal-fired power plants have become a critical measure for carbon dioxide reduction in China. Captured CO₂ will be treated subsequently in two ways: Carbon utilization and carbon storage. The proportions of utilization and storage are expressed by ${\eta }_U$ and ${\eta }_S$, respectively, and the sum of the proportion accounts for 1; that is, ${\eta }_U+{\eta }_S=1$. The utilization of CO₂ can produce economic benefits directly and reduce carbon dioxide transport and storage costs. Then, lots of large-scale investments in CCUS technologies can be encouraged, especially in the coal-fired power industry. There are mainly two ways for CCUS utilization: Industrial utilization and food-grade utilization. Assuming ${\eta }_I$ and ${\eta }_F$, respectively, denote the proportion of industrial utilization and food-grade utilization, the formula ${\eta }_I+{\eta }_F={\eta }_U\le 1$ can be obtained. Meanwhile, the prices of industrial CO₂ and food-grade CO₂ are expressed by $p_I$ and $p_F$, and the total utilization profits can be described as $\left({\eta }_I{p}_I+{\eta }_F{p}_F\right)q_C$, where the values of ${\eta }_I$ and ${\eta }_F$ are constants. Affected by the relations of supplies and demands in the market, the prices $p_I$ and $p_F$ are random fluctuations, and both follow the geometric Brownian motion. As such, we can obtain:

$$d{p}_I={\mu }_I{p}_I{d}_t+{\sigma }_I{p}_{I}d{z}_I,$$

(2)

$$d{p}_F={\mu }_F{p}_F{d}_t+{\sigma }_F{p}_{F}d{z}_F,$$

(3)

where ${\mu }_I$ and ${\mu }_F$ are the drift rates of industrial CO₂ and food-grade CO₂, respectively; ${\sigma }_I$ and ${\sigma }_F$ are the volatility rates of prices; and $d{z}_I$ and $d{z}_F$ are the increments to the standard Weiner process.

3.1.4. CCUS Project Investment Cost

Due to the learning effect in the development of CCUS technologies [37], which results in an increase of the installed capacity, the investment cost will be continuously reduced. The learning rate can be calculated through the accumulated installed capacity. Assuming the initial CCUS investment cost needed at time 0 is $C{I}_0$, the investment cost at time t, $C{I}_t$, is obtained with the following formula:

$$C{I}_t=C{I}_0{(\frac{x_t}{x_0})}^{-\alpha },$$

(4)

where $x_t$ and $x_0$ represent the accumulated installed capacity of the coal-fired power industry, which has invested in CCUS technologies in year t and in the benchmark year. $\alpha$ reflects the parameter for the learning ability in CCUS technologies. Assuming the annual growth rate of the industrial installed capacity is $\beta$, then $x_t=x_0{(1+\beta )}^t$; that is:

$$C{I}_t=C{I}_0{(1+\beta )}^{-\alpha t}.$$

(5)

3.1.5. CCUS Additional Operating Costs

Operating costs, including fuel consumption increments of the CCUS system, labor costs, and maintenance costs, are essential for installed CCUS facilities to run normally [38]. In general, coal price, labor, and maintenance costs are relatively stable. Assuming the additional operating cost per unit CO₂, $c_O$, remains unchanged, the annual operating cost can be denoted as $c_O{q}_C$.

3.1.6. CO₂ Transportation and Storage Costs

The remaining part of captured CO₂, which cannot be reused, must be transported to some prespecified places and treated with some special storage methods. CO₂ transportation is mainly carried out by vehicles or pipelines [4]. The transport cost by vehicles can be considered as a variable cost while the cost of transportation by pipelines is primarily a one-time fixed cost at the start-up time. The initial investment cost for pipeline transport is rather huge, and vehicle transport seems to be more realistic for early CCUS projects. As ${\eta }_S$ denotes the ratio of stored CO₂ to total captured CO₂, we can obtain ${\eta }_I+{\eta }_F+{\eta }_S=1$. Because the unit cost of CO₂ transportation is $c_T$ and the unit cost of CO₂ storage is $c_S$, the annual cost of transport and storage can be represented by ${\eta }_S\left(c_T+c_S\right)q_C$. Assuming $c_T$ and $c_S$ are stochastic variables, they can also be modeled by the geometric Brownian motion as shown in the two formulas below:

$$d{c}_T={\mu }_T{c}_T{d}_t+{\sigma }_T{c}_{T}d{z}_T,$$

(6)

$$d{c}_S={\mu }_S{c}_S{d}_t+{\sigma }_S{c}_{S}d{z}_S,$$

(7)

where ${\mu }_T$ and ${\mu }_S$ are the drift rate of the transport and storage cost, respectively; ${\sigma }_T$ and ${\sigma }_S$ represent the fluctuation rate; and $d{z}_T$ and $d{z}_S$ are increments to the standard Weiner process, $d{z}_T={\epsilon }_t\sqrt{dt},{\epsilon }_t~N\left(0,1\right)$ and $d{z}_S={\epsilon }_t\sqrt{dt},{\epsilon }_t~N\left(0,1\right)$.

3.2. CCUS Project Value Based on the Real Option Model

Setting $r_0$ to be the base discount rate, the residual value of CCUS equipment at the end of project lifespan equals zero. During the period of the CCUS project’s lifespan, the cash flows involved in the CCUS project include the electricity sale subsidy, carbon trading revenue, carbon utilization revenue, initial investment cost, equipment operating cost, transportation and storage cost, etc. Therefore, the net present value (NPV) of the CCUS project at time $t_0$ can be expressed as:

$$\begin{gathered} Npv={{\displaystyle \sum }}_{t=t_0+1}^T\left[P_E{q}_E+ P_C{q}_C+\left({\eta }_I{P}_I+{\eta }_I{P}_I\right) q_C\right]{\left(1+r_0\right)}^{-\left(t-t_0\right)} \\ -{{\displaystyle \sum }}_{t=t_0+1}^T\left[C_O{q}_C+ \left(C_T+C_S\right) {\eta }_S{q}_C\right]{\left(1+r_0\right)}^{-\left(t-t_0\right)}-C{I}_0{\left(1+\beta \right)}^{-\alpha t_0}. \end{gathered}$$

(8)

According to real option theory, the investment value of the CCUS project with delay options included at time t can be described as:

$$IO{V}_t=max\left\{I{V}_t,E\left[e^{-r_{0}dt}IO{V}_{t+1}\right]\right\},$$

(9)

$$I{V}_t=max\left\{NP{V}_t,0\right\}.$$

(10)

3.3. Model Solution

Under the carbon trade schemes, the investment decision in the CCUS project can be regarded as an optimal stopping problem with multiple uncertainties, which interact and have complex relationships with each other. As several underlying assets are involved, neither the finite difference method nor the binominal tree can obtain the analytic solutions for the problem. Without solving differential equations, the Monte Carlo simulation method can be used to solve multidimensional real option problems and obtain the numerical results of the model, which are easier to be understood. Thus, it is most suitable to use the Monte Carlo simulation method in this study. Further analysis shows that the investment value of the CCUS project is related to the historical information of the underlying assets; that is, it has the characteristics of path dependence. Traditional Monte Carlo simulation is merely used to solve problems with European options, and the problem of the optimal stopping time is used to deal with American options that can be executed in advance before the expiration date. Thus, an improved method, least square Monte Carlo simulation, is finally adopted to analyze the solution in the model. The solution processes are divided into four steps and the details are shown as follows:

Step 1. First, the uncertain factors in the model are discretized; the expression after discretization is shown as Equations (11)–(15). According to the discrete approximations, random numbers are generated that follow the standard normal distribution. To reduce the computational complexity and accelerate convergence, the antithetic variable variance reduction technique is applied in the process of simulation. There are 10,000 different paths presented eventually:

$$P_C\left(t+\Delta t\right)=P_C\left(t\right)exp\left(\left({\mu }_C-{\sigma }_C/2\right)\Delta t+{\sigma }_C{\left(\Delta t\right)}^{\frac{1}{2}}{\epsilon }_{ct}\right),$$

(11)

$$P_i\left(t+\Delta t\right)=P_i\left(t\right)exp\left(\left({\mu }_i-{\sigma }_i/2\right)\Delta t+{\sigma }_i{\left(\Delta t\right)}^{\frac{1}{2}}{\epsilon }_{it}\right),$$

(12)

$$P_f\left(t+\Delta t\right)=P_f\left(t\right)exp\left(\left({\mu }_f-{\sigma }_f/2\right)\Delta t+{\sigma }_f{\left(\Delta t\right)}^{\frac{1}{2}}{\epsilon }_{ft}\right),$$

(13)

$$C_t\left(t+\Delta t\right)=P_t\left(t\right)exp\left(\left({\mu }_t-{\sigma }_t/2\right)\Delta t+{\sigma }_t{\left(\Delta t\right)}^{\frac{1}{2}}{\epsilon }_{tt}\right),$$

(14)

$$C_s\left(t+\Delta t\right)=P_s\left(t\right)exp\left(\left({\mu }_s-{\sigma }_s/2\right)\Delta t+{\sigma }_s{\left(\Delta t\right)}^{\frac{1}{2}}{\epsilon }_{st}\right).$$

(15)

Step 2. Based on the discrete paths of uncertain factors, the expected value of the cumulative investment income, cumulative cost, and net revenue at each timepoint in each path is calculated backwards, which means the calculation should start from the last period (t = T). The NPV of the project is obtained by averaging the value of net revenues at time 0 over all the paths.

Step 3. At each timepoint of each path, the project NPV is compared to the zero value and whether it is worthwhile to invest is decided. Then, the value of investment opportunities can be calculated. At time t_ij, that is timepoint i in path j, if the NPV is greater than 0, the investment should be carried out and the investment opportunity value is equal to the NPV; otherwise, the opportunity value is 0 and the investor should abandon the project because the project has no investment value. We can get:

$$I{V}_{ij}=max\left\{NP{V}_{ij},0\right\}.$$

(16)

On the basis of investment opportunity values, the waiting option value should be taken into account to calculate the investment value of the project. The investment values in every period depend on whether to exercise the waiting options. At timepoint $t_{ij}$, the investment value embedded waiting option is expressed as $IO{V}_{ij}$, and it equals the maximum between the immediate value and continuation value, that is:

$$IO{V}_{ij}=max\left\{I{V}_{ij},e^{-r\Delta t}E\left(IO{V}_{i+1,j}\right)\right\},$$

(17)

where $I{V}_{ij}$ denotes the immediate investment value, which the opportunity value above. The continuation value is estimated by least square regression. The dependent variable is the discount value of the investment value at timepoint $i+1$; that is, $e^{-r\Delta t}I{V}_{i+1,j}$. The independent variables are the initial investment cost, cumulative cash flow, and cumulative cost in the remaining period. The estimated sample only includes the path where the investment value, $I{V}_{ij}$, is greater than 0. The dynamic programming backward recursion is performed repeatedly and rolled back to the beginning time.

Step 4. The optimal investment timepoints, $t_j^{\ast }$, for all paths can be determined, and the optimal investment time for the project is the timepoint with the highest frequency in all paths. The investment value at the optimal timepoint of each path to time 0 is discounted, and the average of all paths is taken. Then, the investment value of the project is obtained, V*, which can be expressed as follows:

$$V^{\ast }=\frac{1}{n}{\displaystyle \sum }_{j=1}^n{e}^{-r{t}_j^{\ast }}IO{V}_{t_j^{\ast }},j=1,2,3\dots n.$$

(18)

4. Parameter Estimation

4.1. Carbon Price

In December 2017, the national carbon emission trading market was launched officially, and with construction completed an full operation started in 2020. Lacking national historical data, the data used to estimate the carbon price parameters are from pilot cities in the past years. In practical terms, the sample data are composed of the daily carbon price of the Beijing market from June 2018 to May 2019. The maximum likelihood estimation method was used to estimate the drift rate and volatility of the carbon price, and the results were basically consistent with Zhang et al. [39]. For the convenience of the calculation, the estimated results were rounded, and the drift rate and volatility were set as 0.02 and 0.03, respectively.

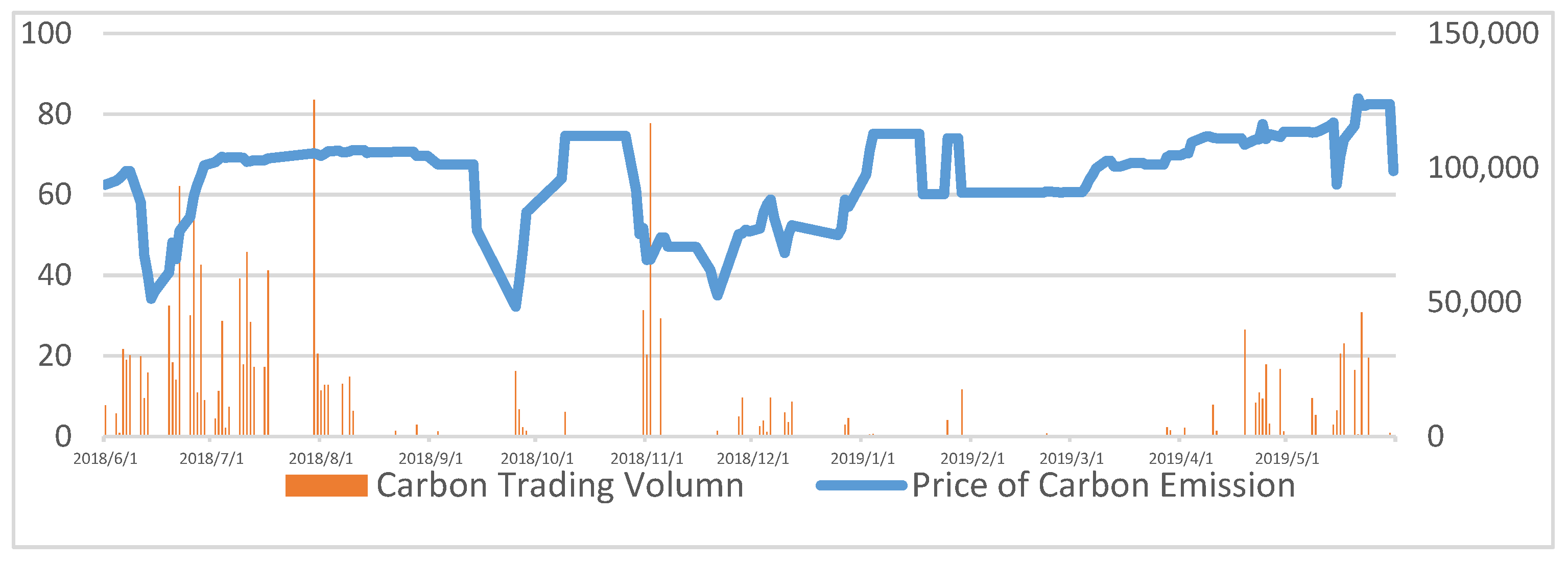
According to the China Carbon Pricing Survey in 2018, the predication of the average carbon price in China is 7.71 USD/ton in 2020, which is supported by the fact that the carbon price in Beijing market is almost maintained above 7.56 USD/ton from June 2018 to May 2019, as shown in Figure 2. Therefore, in this study, the initial price of carbon trading, $P_{C0}$, was set as 7.56 USD/ton. The currency conversion relation used in this study was 6.6174 CNY to 1 USD, which is the average exchange rate between the two currencies in 2018.

4.2. CO₂ Utilization Parameters

CO₂ utilization mainly includes two ways: Industrial utilization and food-grade utilization. From 2016 to 2018, the price of industrial carbon dioxide in China varied in a range from 30.22 to 75.56 USD per ton, and the price of food-grade carbon dioxide fluctuated in the range from 60.44 to 98.23 USD per ton. As a reasonable inference, the development of carbon utilization industries will increase the supply of CO₂ substantially and affect the price of CO₂ utilization. Based on the average carbon dioxide price, the initial value of industrial CO₂ and food-grade CO₂ were assumed to be 45.34 and 75.56 USD per ton, respectively. Since the price of CO₂ utilization is closely related to that of CO₂ emission trading, we assigned the drift rate and volatility of CO₂ utilization price the same value as those of the carbon price, ${\mu }_{pu}=0.02$ and ${\sigma }_{pu}=0.03$.

In addition, three ratios are required: ${\eta }_I$, ${\eta }_F$, and ${\eta }_S$. Data from the Intergovernmental Panel on Climate Change (IPCC) report show that about 80% of captured CO₂ is stored underground without recycling. Disregarding the loss in the CCUS process, the utilization rate was assumed to be 0.2. In China, the consumption structure of CO₂ varies in different provinces and cities, and the ratio between the CO₂ volume of industrial utilization and that of food-grade utilization changes as well. According to the statistical data on the national market in 2018, the proportion of industrial utilization was assumed to be 0.8 in the basic computation example.

4.3. CCUS Initial Investment Cost

The investment cost of a CCUS project is very high. According to statistics in China, many demonstrative CCUS projects in recent years, whose investments are several hundred million of yuan, are designed to possess a treatment capacity of 100,000 to 1,000,000 tons CO₂ every year. The study object in this paper was a fired power plant with a 600-MW supercritical boiler. The available factor was assumed to be 0.75 and the capacity factor was 0.8. In general, the power plant will produce electricity without interruption. Then, the annual work time is 8760 h due to running 365 days per year and 24 h every day. Therefore, the annual energy output of the coal power plant is about $3\times {10}^9$ kwh. According to IPCC research results, 762 g of carbon dioxide are emitted per kilowatt hour. If the capture rate of CO₂ is 80%, the annual carbon emission scale of the 600-MW supercritical thermal power unit will reach two million tons.

Many prior studies have shown that the initial investment cost of a CCUS project has the features of a learning curve, and the learning efficiency depends on the annual cumulative installed capacity and learning ability. Consistent with Rubin’s result [40], the learning coefficient was set as $\alpha =0.168$. To achieve the emission reduction goal, the focus should be put on the reconstruction and transformation of the newer fired power plants that have met the ultra-low emission (ULE) standards. The development of CCUS technologies should follow the growth routine of the ULE power set. Until March 2018, coal power plants with a 580 million kwh capacity in China finished the ULE transformation ahead of schedule. Adding the newly built ULE power plants, the ULE coal power plants in China have surpassed 750 million kWh. It is estimated that the installed capacity of coal-fired plants in China will reach 1.1 billion kwh by 2020. So, the growth rate of CCUS projects in coal power plants is estimated to be 16% per year; that is, $\beta =0.16$.

According to Abadie and Chamorro [23], the initial investment cost is about 664.31 USD/kW, which means a plant with a 600-MW supercritical boiler needs 2.6 billion to construct a CCUS system. Following the rules of the learning curve, after 10 years of development of CCUS technologies, the initial investment of the CCUS project with 2 million tons of CO₂ treatment capacity would be $0.3\times {10}^9$ USD in 2019.

4.4. Operating Cost of the CCUS System

The operating costs of CCUS equipment are mainly composed of additional energy consumption to capture carbon and the additional resources needed to run CCUS equipment normally. For most CCUS systems, capture costs account for the largest share of operating costs, usually about 75%. Referring to the setting in Abadie and Chamorro [23], the unit operation and maintenance cost of the CCUS system is 2.08 USD/MWH, so the annual operation and maintenance cost of CCUS equipment of the coal power plant is $6.25\times {10}^6$ USD.

According to IPCC, to run a CCUS system well, the power plants will increase their fuel consumption by about 24% to 40%. We used the average value, 32%, in this paper. Based on the statistical data of the electric power industry in 2018 by the National Energy Administration (NEA), the standard coal consumption of a fired power plant is 308 g/kWh, and the average price of electricity coal was 80.24 USD/ton in 2018. In consequence, the increased cost of fuel consumption to capture CO₂ for a power plant with a 600-MW installed capacity is $0.24\times {10}^8$ USD per year. The sum of the two parts of the cost above is $0.3\times {10}^8$ USD, and the annual carbon dioxide emission of the power plant is assumed to be 200,000,000 tons. Then, the unit operating cost of CCUS equipment is 14.99 USD per ton CO₂, which was rounded up to 15.11 USD/ton to simplify computing. The result is consistent with the unit of CO₂ capture cost of the Huaneng Group power station, which is the first demonstrative CCUS project in China.

4.5. Transportation Cost and Storage Cost

The transport model includes tank trucking, pipelining, and shipping. If the size of the CCUS project is small, and the relationship between the source and sink is unstable, trucking is the most economical mode. When the CCUS project moves into the mass production stage, the transport scale will grow rapidly and destinations are determined in a long-term cooperation agreement, pipelining should be planned. Shipping always corresponds to ocean disposal of CO₂. There are many places with favorable geological conditions for the storage of CO₂ in China, and the CO₂ storage capacity in the deep underground layer is rather huge.

This paper adopts geological storage in the model, which seems safer and more stable at present. Based on the assumption of 250-km pipelines being constructed, the unit transport cost is below 5 dollars, and the unit storage cost is in the range from 6 dollars to 8.3 dollars. By converting to RMB and taking the average, the transport cost and storage cost were set as 3.02 USD/ton (20 CNY/ton) and 6.04 USD/ton (40 CNY/ton), respectively.

Based on the analysis above, the parameters of CCUS investment projects are shown in

Table 1. Setting of related parameters of the CCUS investment project in a coal-fired power plant.

Parameter	Symbol	Value	Explanation
Annual energy output	$q_E$	3 × 109 kwh	Total annual power generation in coal-fired power plants.
Environmental electricity price subsidy	$p_E$	0.0015 USD/kwh (0.01 CNY/kwh)	Relevant provisions of the measures for the Supervision of Environmental Protection electricity Price and Environmental Protection facilities of Coal-fired generating sets.
Approved emission reduction	$q_c$	2 × 106 ton	Calculation on the basis of annual electricity generation, CO2 emissions per kwh generation and capture rate.
Initial value of carbon trading price	$p_{C0}$	7.56 USD/ton (50 CNY/ton)	According to the average carbon price predicted in 2018 China carbon Price Survey, the average carbon price in 2020 is predicted.
Carbon valence drift rate	${\mu }_{pc}$	0.02	Maximum likelihood estimation with reference Zhang et al. [39] setting.
Carbon valence volatility	${\sigma }_{pc}$	0.03	Maximum likelihood estimation with reference Zhang et al. [39] setting.
Initial value of industrial utilization price	$p_{I0}$	45.34 USD/ton (300 CNY/ton)	According to the price range of industrial CO2, the average value is taken.
Initial value of food utilization price	$p_{F0}$	75.56 USD/ton (500 CNY/ton)	According to the price range of food grade CO2, the average value is taken.
Carbon utilization price drift rate	${\mu }_{pu}$	0.02	Reference carbon trading price drift rate setting.
Carbon utilization price volatility	${\sigma }_{pu}$	0.03	Reference carbon trading price volatility setting.
Initial cost of CCUS investment	$C_{I0}$	0.3 × 109 USD (2 × 109 CNY)	Using the learning curve model, the unit construction cost of CCUS unit refers to Abadie and Chamorro [23] and Wang and Du [26].
Learning ability parameters	$\alpha$	0.168	Refer to Rubin et al. [40] and Wang and Du [26].
CCUS installed capacity growth rate	$\beta$	0.16	Reference to the Development path of Ultra-low Emission Transformation of Coal-fired Units in China.
Operating cost of CCUS equipment	$C_0$	15.11 USD/ton (100 CNY/ton)	Including CO2 capture system operation and maintenance cost and extra fuel consumption, operating cost reference Abadie and Chamorro [23], system energy consumption ratio referring to IPCC research results and taking the mean value.
Initial value of CO2 transportation cost	$C_T$	3.02 USD/ton (20 CNY/ton)	Referring to IPCC report, it is assumed that under the condition of large-scale pipeline transportation, the CO2 transportation cost range of coal-fired power plant is taken as the mean value.
Transport cost drift rate	${\mu }_{CT}$	−0.03	Refer to Zhang et al. [39] settings.
Transport cost volatility	${\sigma }_{CT}$	0.04	Refer to Zhang et al. [39] settings.
Initial value of CO2 storage cost	$C_S$	6.04 USD/ton (40 CNY/ton)	Referring to the IPCC report, the CO2 storage cost range takes the average.
Storage cost drift rate	${\mu }_{CS}$	−0.03	Refer to Zhang et al. [39] settings.
Storage cost volatility	${\sigma }_{CS}$	0.04	Refer to Zhang et al. [39] settings.
Remaining period of coal power plant	$T$	40 year	Refer to Wang and Du [26] settings.
CCUS project discount rate	$r$	8%	The discount rate of the CCUS project.
Number of simulated paths	$n$	10,000	The dual technology to enhance the accuracy.

, including the item names, symbols, values, and description.

5. Sensitivity Analysis of Influencing Factors

5.1. The Simulated Example

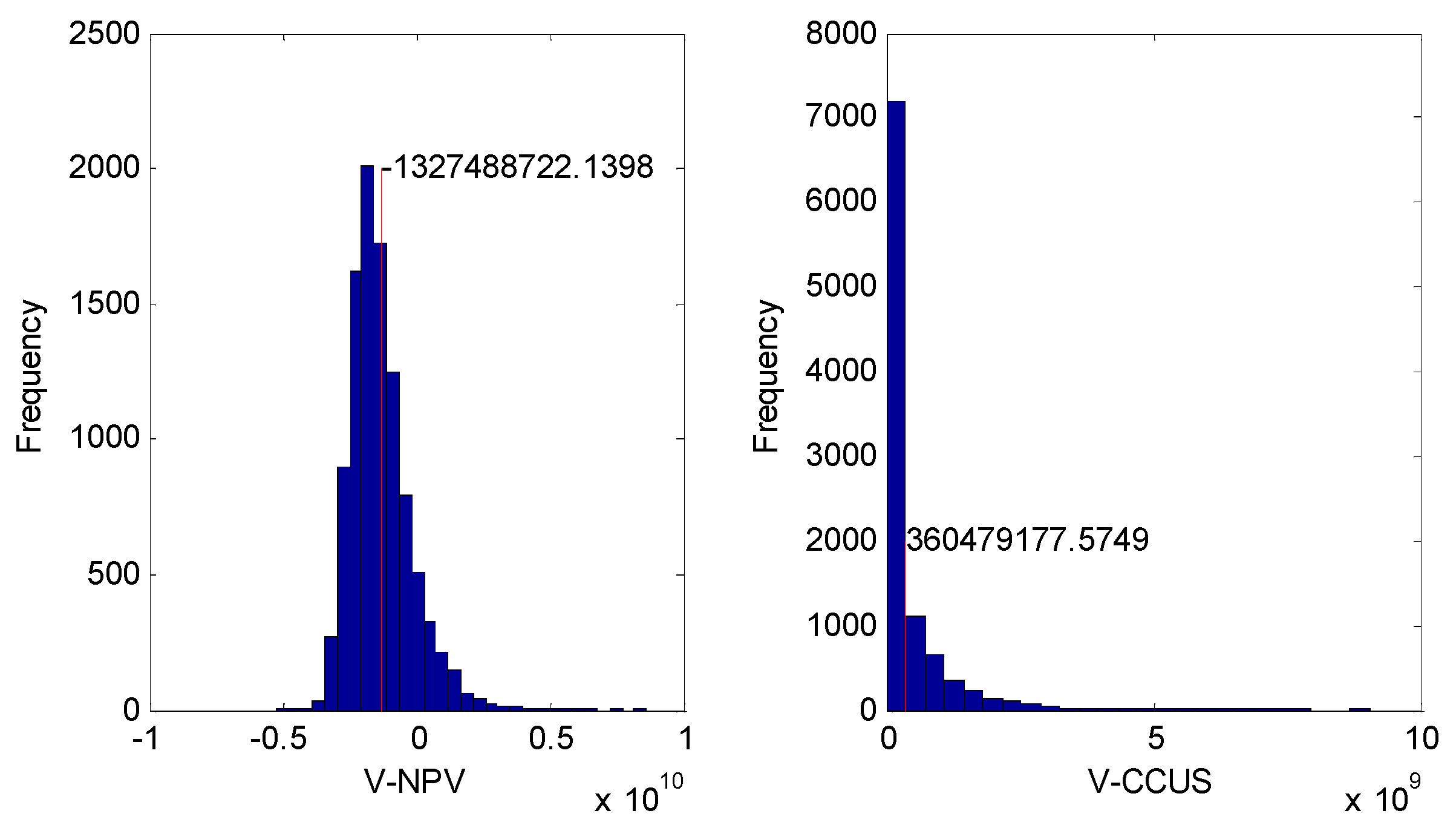
We applied MATLAB software (R2018a, MathWorks Inc., Natick, MA, USA, 2018) to simulate the model and make numerical analysis. In order to improve the convergence and reduce the variance, the dual variable technique was used to ensure the accuracy of the simulation. Basing on 10,000 random paths, the NPV and the investment value of CCUS project were obtained, which are shown in Figure 3.

With the assumptions and the given parameters above, the NPV of CCUS project is −1.33 billion CNY (−200.98 million USD). Figure 3 shows that nearly 90% of the simulating paths have a negative net present value and the investment cost cannot be recovered by the profit obtained from the sales of electricity, which indicates that it is unwise to invest in the project at present without any government subsidies. If the coal-fired power plant is allowed to postpone the launch of the CCUS project to cope with the high risk at the early stage, and chooses the optimal time to invest, then the investment value of the project will be 0.36 billion CNY (54.4 million USD). To sum up, the power plant should wait until the optimal time rather than investing immediately at present. Taking the flexibility of investment into account, the value of the CCUS project changes from negative to positive, which means the investing flexibility is valuable. The value of the delay option equals the difference between the project investment value and the net present value of the same project, i.e., $\mathrm{OV}=\mathrm{IOV}-\mathrm{NPV}=16.9$ billion CNY = 2.55 billion USD.

5.2. Carbon Trading Price

5.2.1. Initial Value of Carbon Price

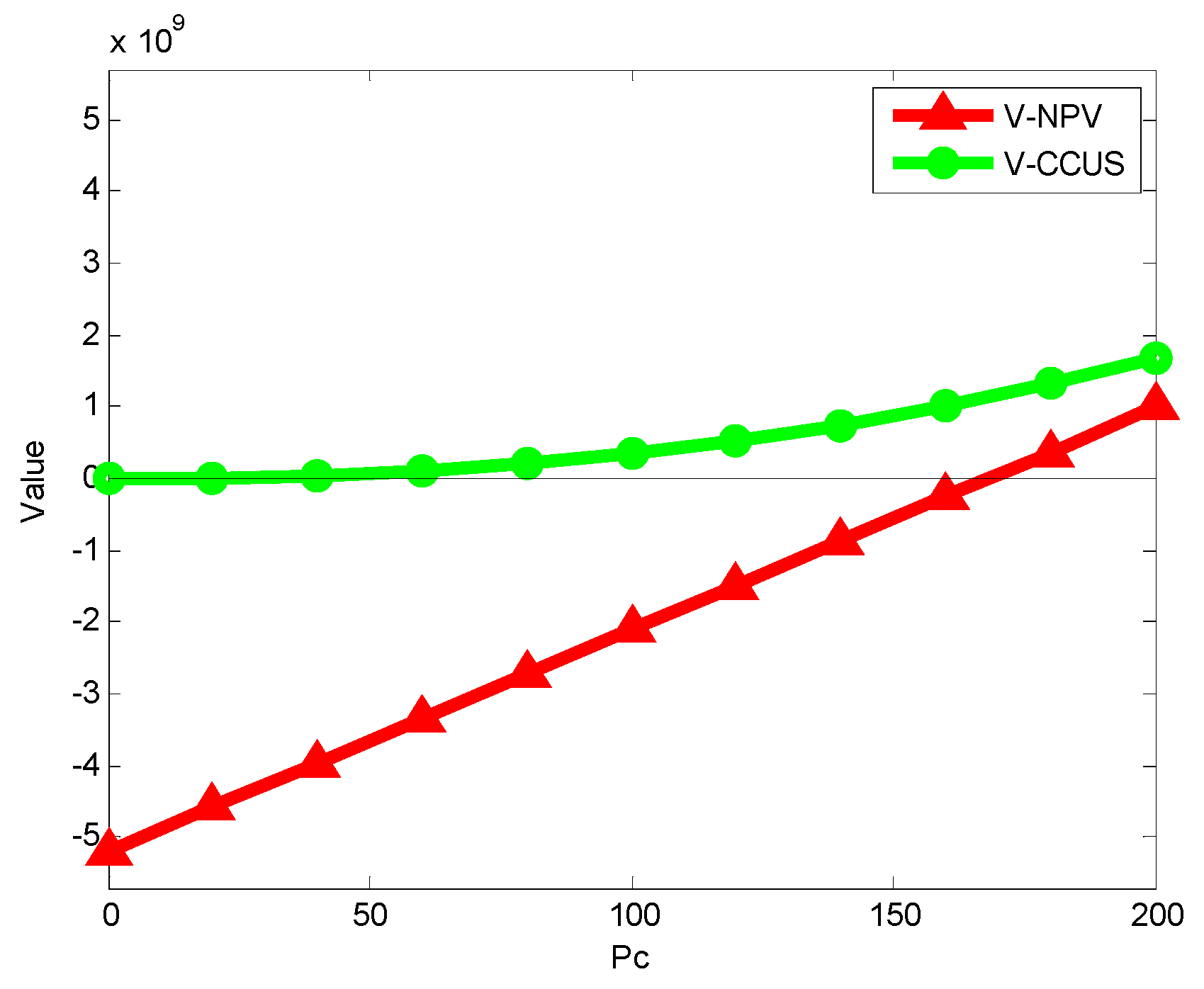
In order to investigate the effectiveness of carbon trading markets, it is assumed that all captured carbon dioxide is sequestered directly, without carbon utilization. In the current conditions of marketing and technologies in China, the impact of the initial carbon price on CCUS project investment is shown in Figure 4. The solid line with triangles describes the NPV value of the CCUS project while the solid line with circles gives the trend of the project investment value. It is assumed that the initial value of the carbon price, which is the current carbon price, changes in the range of below 30.22 USD/ton. With the increase of the carbon price, the net present value of the CCUS project increases continuously. Even though the carbon price reaches 15.11 USD/ton, the net present value of the project is still negative and far below 0. Until the carbon price reaches 25.36 USD/ton, the net present value of the CCUS project can break through zero and become positive. The higher carbon price is unpractical, as the highest price globally in 2018 was only 138.98 CNY/ton, which is 21 USD/ton as the average exchange rate in 2018 was 6.6174 CNY to 1 US dollar. For investors, it seems better to postpone investments under unfavorable conditions and wait for a more suitable opportunity; that is, to exercise the waiting option. The investment value of the project including the waiting option increases with the carbon price, but the rising degree is limited. The waiting options will be more valuable as the market condition becomes more disadvantageous. It is shown by the spacing between the two lines in Figure 4.

Currently, the carbon trading market in China is still in the stage of deeply improving, so it is necessary to fully consider the risk of market failure. Therefore, in the context of an imperfect carbon trading mechanism, promoting the development of CCUS and achieving the carbon reduction goal in China only through the carbon trading scheme will have little success.

5.2.2. The Uncertainty of the Carbon Price

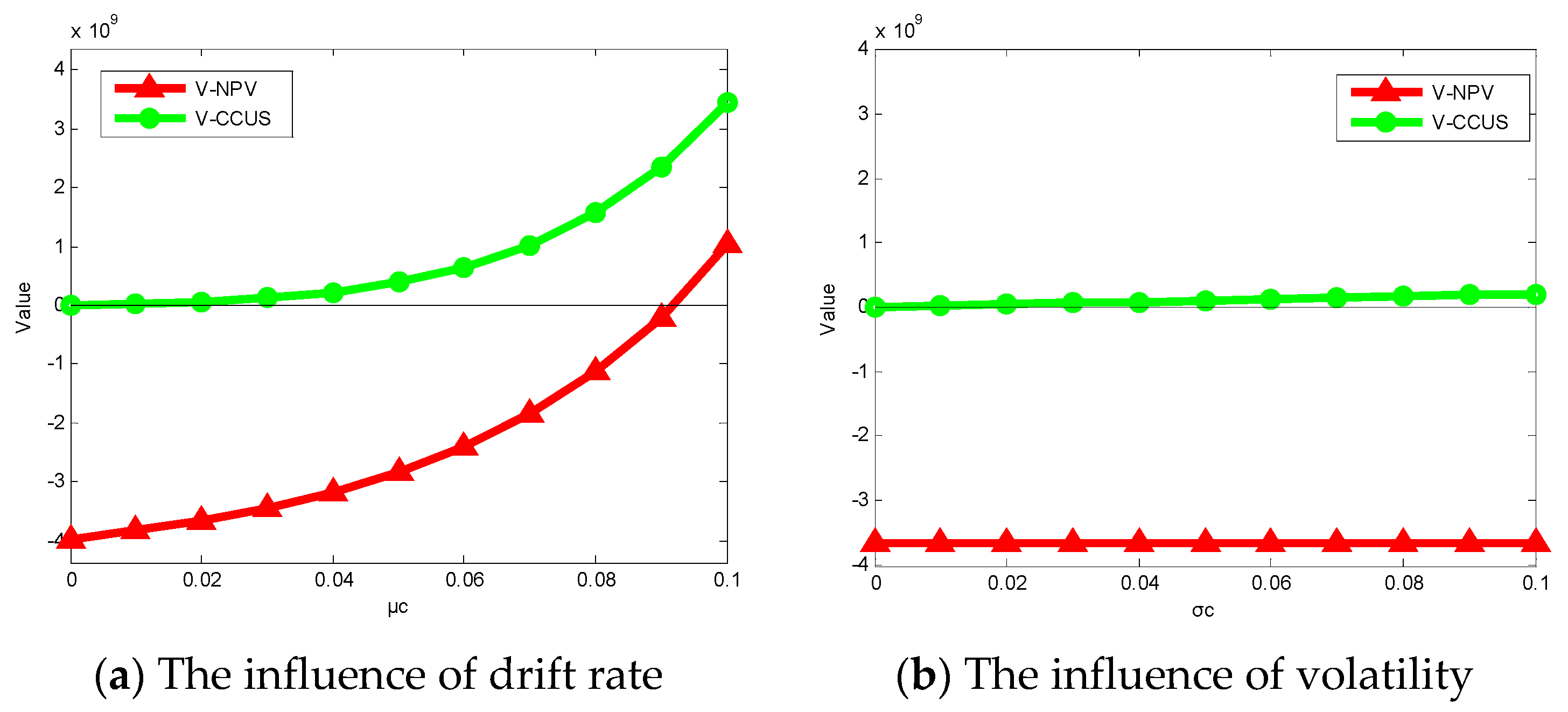
The uncertainty of the carbon price can be reflected by two factors: The expected growth rate and the volatility of the carbon price. Without considering carbon utilization and keeping other parameters unchanged, the impact of carbon price uncertainty on the investment value of the CCUS project is shown in Figure 5.

With the increase of the drift rate, both the net present value and investment value of the project increase correspondingly. As the initial value of the carbon price is 7.56 USD/ton, when the drift rate is slightly higher than 0.09, the net present value of the CCUS project breaks through the zero value, which makes immediate investment feasible. At the same time, the investment value of the project with the delay option embedded rises obviously with the drift rate. It is shown that the drift rate of the carbon price can greatly stimulate the investment willingness of power plants and promote them to invest in CCUS technologies immediately at present.

Volatility reflects the level of risk in the carbon trading market. The greater the volatility, the higher the risk. Due to the investment in large-scale and high running cost CCUS projects, the impact of carbon price fluctuation on the project value is relatively small, and the hedging effect of thte waiting options remains almost unchanged. For the coal power plant, the carbon price and its rising space in the future are important factors to make investment decisions on the CCUS project.

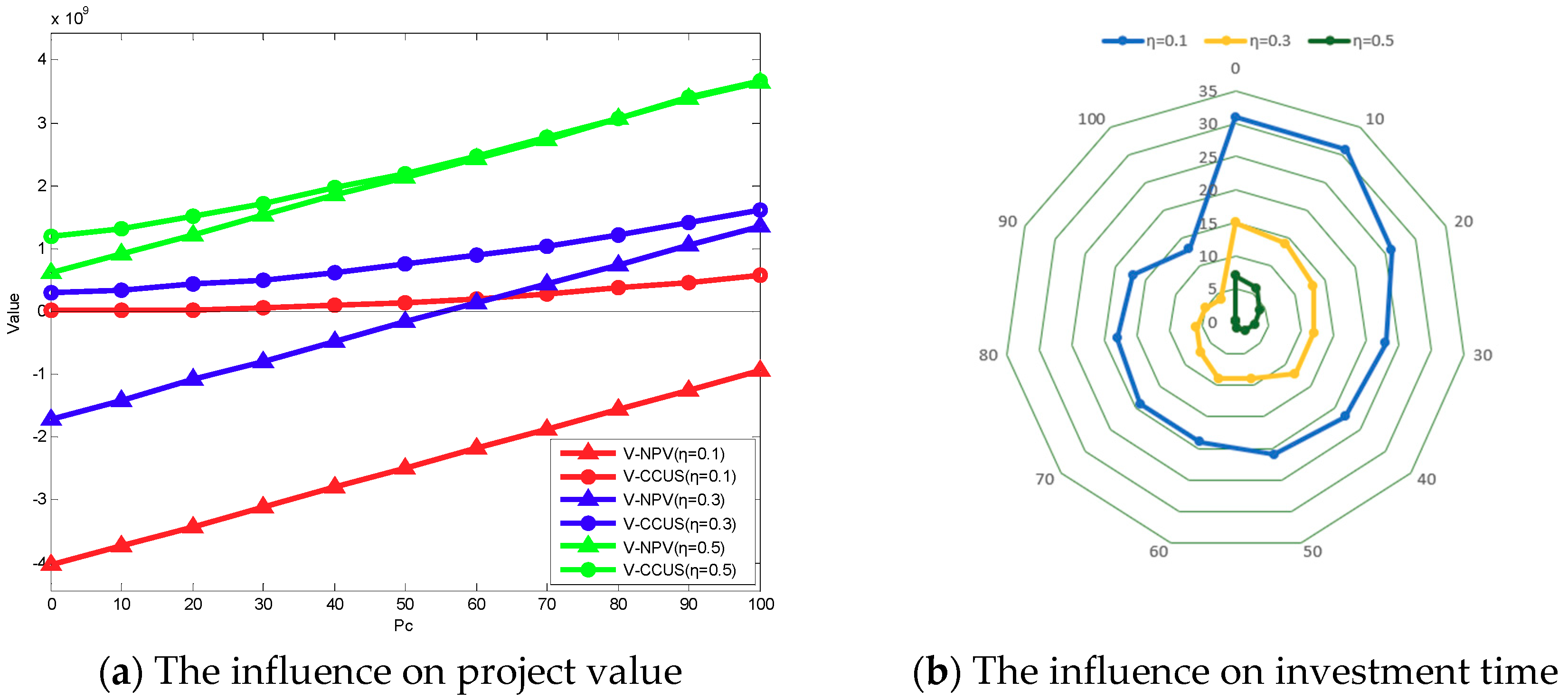
5.3. Carbon Utilization Rate, $\eta$

It is only when the carbon price is high enough that it can have an effect on the conducting behaviors of carbon emission plants in various regions. In the context of the carbon-dominant energy structure in China, it is essential that the various emission reduction measures are integrated to achieve the expected goals. The utilization of carbon dioxide is one of the requisite approaches. Keeping other conditions unchanged, the influence of the carbon price on the NPV and investment value of the CCUS project is significant, as shown in Figure 6. From the bottom up, the red lines, blue lines, and green lines represent the cases of how the NPV and investment value of the CCUS project change with the carbon price when the utilization rate is 10%, 30%, and 50%, respectively.

It is assumed that the unit carbon price varies from 0 to 15.11 USD. When the utilization rate ${\eta }_U=0.1$, it is shown that investment is immediately infeasible all the way and investment value of the project grows with the carbon price very slowly. When the carbon utilization rate, ${\eta }_U$, rises from 0.1 to 0.3, the immediate investment threshold for CCUS project will fall sharply to 8.37 USD/ton, which seems very easy to realize. Carbon utilization will partially solve the problem of high operational costs in the CCUS project, because it increases revenues and reduces costs at the same time. As a result, the payback period of the initial investment cost of the CCUS project will be shortened significantly. When the carbon utilization rate is ${\eta }_U=0.5$, the NPV and investment value of CCUS project increase to a high level, and the project value is more sensitive to the carbon price. Even when the carbon price is rather low, the power plant still has enough motivation to invest immediately.

Meanwhile, the carbon utilization rate affects the optimal investment time of the project significantly. When the carbon utilization rate is relatively low, such as ${\eta }_U=0.1$, the optimal investment time of the project shifts earlier from the 31st year to the 13th year, as the carbon price rises gradually. That means the plant will wait for a long time until the middle of its lifecycle, and invest in the CCUS project only when the carbon price is high enough. The investment will go more smoothly when the utilization rate is higher. As the utilization rate is 50%, the optimal time to invest will be earlier than the seventh year, especially when the carbon price rises to 9.07 USD/ton (60 CNY/ton, immediate investment is the optimal decision for the fired power plant. Therefore, the active research and development of carbon utilization technologies is effective in stimulating investments in CCUS.

5.4. Carbon Utilization Method

CO₂ utilization involves two ways: Industrial utilization and food-grade utilization. At present, industrial utilization is dominant in China, for example, enhancing oil recovery (EOR) is about 80%, while food-grade utilization is only 20%, and ${\eta }_I/{\eta }_F=4$. Different utilization approaches require different quality levels of CO₂, and the production costs and market prices of CO₂ are correspondingly different. Food-grade CO₂ requires a higher purity, the production process is more complex, and the cost is much higher. Thus, its market price is nearly twice that of industrial CO₂. Assuming the total utilization rate of CO₂ is 40%, the influence of the ratio between industrial utilization and food-grade utilization on the CCUS project value is shown in Figure 7.

When the proportion of food-grade utilization of CO₂ is relatively low, the contribution of carbon utilization to the profitability of the CCUS system is limited. As the food-grade utilization rate increases, the NPV and investment value of the CCUS project rise obviously. The influence of the utilization style of CO₂ on the CCUS project values is more significant on the optimal investment time. With the same carbon price, the higher the food-grade utilization rate is, the earlier the optimal invest time is. Using the case of $p_{C0}=7.56\mathrm{USD}/\mathrm{ton}$ ($50$ CNY/ton) as an example, when the ratio between two utilization styles is 3/7, 5/5, and 7/3, respectively, the optimal investment time is obviously different: The fourth year, the second year, and the first year. Further analysis of the investment threshold indicates that as the food-grade utilization rate increases from 0.3 to 0.7, the carbon price for immediate investment drops down from 13.6 USD/ton (90 CNY/ton) to 9.07 USD/ton (60 CNY/ton). In conclusion, the development of purification technologies of food-grade CO₂ is a critical factor for CCUS project investment. Therefore, more resources should be invested in the research and development of CCUS technologies. Particularly, an emphasis should be placed on the efficiency improvement of food-grade CO₂-capturing technologies, and then production costs should be lowered. All of these measures will provide an important guarantee for CCUS technologies to play the core role in CO₂ emission reduction for China.

6. Conclusions

Due to the advantages in terms of earning more profits, as well as the ability to lower carbon transport and storage costs, carbon utilization continues to attract increasing attention around the world. Carbon utilization is one of the critical links of CCUS systems with the greatest potential and has received great support. China has made great efforts to develop CCUS technologies. To attract more investment, the Chinese government has made great efforts in constructing and perfecting the carbon emission trading scheme. However, the stimulation effect of the trading mechanism seems to be limited as neither emission reduction nor investment enlargement have achieved the results that administrators have anticipated. The purpose of this paper was to figure out the critical factors involved in activating investment in CCUS technologies in the context of an imperfect carbon trading mechanism. Taking the coal-fired power plant in China as an example, this paper incorporated many uncertain factors into the model, including uncertainties in carbon trading prices, physical CO₂ prices, initial investment cost, and transport and storage cost. Based on real option theories, we proposed an investment decision model. The model was solved by the least squares Monte Carlo simulation and backward dynamic programming algorithm. The results of this study provide optimal investment threshold values in different technical and market environments. Based on these results, some important policy implications were proposed for the development of CCUS in China.

At first, it is wise for coal-fired power plants to wait before implementing investment in CCUS projects under the current carbon market conditions. In the absence of the participation of carbon utilization, the immediate investment threshold price of carbon emission rights for power plants reaches 25.36 USD/ton, which is obviously unachievable at present. In countries where carbon trading mechanisms have been operating, the highest carbon price all over the world in 2018 was only 21 USD/ton, which is equal to 138.98 CNY/ton with the average exchange rate in 2018. Similarly, Wu et al. [24] also found that the carbon price required to justify the investment on CCS for a coal power plant is 61 USD/ton, which is significantly higher than the international carbon price. For example, the prices of certified emission reduction at the European Climate Exchange are estimated as 10 to 20 EUR/ton in the same period. This high CO₂ price threshold indicates a great financial barrier to low-carbon investment in China. However, Wu et al. [24] left the uncertainties out of consideration and adopted NPV methodology. It will be concluded that the values of CCUS projects are underestimated, and investment threshold prices are over-measured in Wu et al.’ research. The results in this paper are rather more reasonable and can reflect the true situation in China. Consequently, the active construction of a national carbon emission trading market is necessary, but the independent effects of carbon trading schemes on stimulating investment and reducing carbon emission are limited. In view of the fact that it will be a long time before the carbon trading market mechanism has been developed and is perfect, the government must take some other measures simultaneously to motivate investment in the market.

Secondly, adding the carbon utilization into the low-carbon system is significantly helpful. If 30% of the CO₂ captured can be utilized, the threshold value of the carbon price will come down to 8.37 USD/ton, which is nearly at the same level as the current carbon price in China. Meanwhile, the waiting options become fairly valuable for decision makers to deal with various uncertainties and select the optimal investment time. According to the growth trend of carbon prices, coal power plants should invest in CCUS technologies in around 2030 at the latest. The investment incentives of electricity power plants are significantly sensitive to the carbon utilization rate. The effects of carbon utilization on investment stimulation are bidirectional regulation, which means increasing incomes and reducing costs concurrently. Similar results were obtained by Fan et al. [33] and Yang et al. [34]. Regarding CO₂ as a commodity and improving its economic value can effectively promote CCUS development, which also helps to reduce the financial burden of the government. The government should formulate targeted support policies for carbon utilization, such as increasing the expenditure on R&D of carbon utilization technologies, and providing subsidies for carbon utilization costs. Although two recent studies in 2019 focused on carbon utilization in the EOR field, our study extended the investigation using a more extensive utilization method.

Thirdly, the utilization rate of high-purity CO₂, such as food-grade carbon dioxide, has a significant effect on the CCUS project value and optimal investment time. When the food utilization ratio reaches 0.3, the immediate investment threshold falls to 5.65 USD/ton, and most of the optimal invest time are advanced to sometime in 4 years. When the market conditions are better, investors prefer to execute the investment option immediately. Food-grade carbon dioxide is highly purifies, and the manufacturing process need more effective purification technologies. CO₂ captured at power plants also has another prospective class of utilization, and can be converted to many kinds of value-added chemicals, such as urea synthesis and methanol production [19,41,42]. These articles highlight the importance of technical advancements in decreasing the emissions of CO₂. Based on the developed carbon dioxide market, the feasibility and efficiency of utilization technologies are key factors for sustainability. High value-added utilization methods of CO₂ deserve to be supported emphatically by government financing. The government should take steps to promote electricity power enterprises to strengthen their efforts on more economical carbon utilization technologies.

In conclusion, the government should formulate various CCUS incentive measures that exhibit a certain degree of flexibility to meet the particular needs of coal-fired power plants and match the specific characteristics of imperfect carbon trading schemes. This is a reasonable direction to devote the early realization of carbon emission reduction targets in China to.